Towards Ginzburg–Landau Bogomolny Approach and a Perturbative Description of Superconducting Structures
Abstract
1. Motivation Behind Bogomolny Approach
2. Elementary Introduction to Bogomolny Theory
3. A Short Description of the Bogomolny Equations for a Usual Gauged G-L Model
4. The Gauged G-L Model in a Curved Space and the Concept of Strong Necessary Conditions in the Equations of Motion
- Make a certain part of the dual equations linearly dependent—the remaining equations are just the Bogomolny equations;
- Obtain a condition for the potential of the given field-theoretical model. The Bogomolny decomposition (the Bogomolny equations) exists only for this model, whose potential satisfies such a condition.
5. Derivation of the Bogomolny Equations for the Gauged G-L Model in a Flat Space
6. Deriving the Bogomolny Equations of the G-L Model in a Curved Space
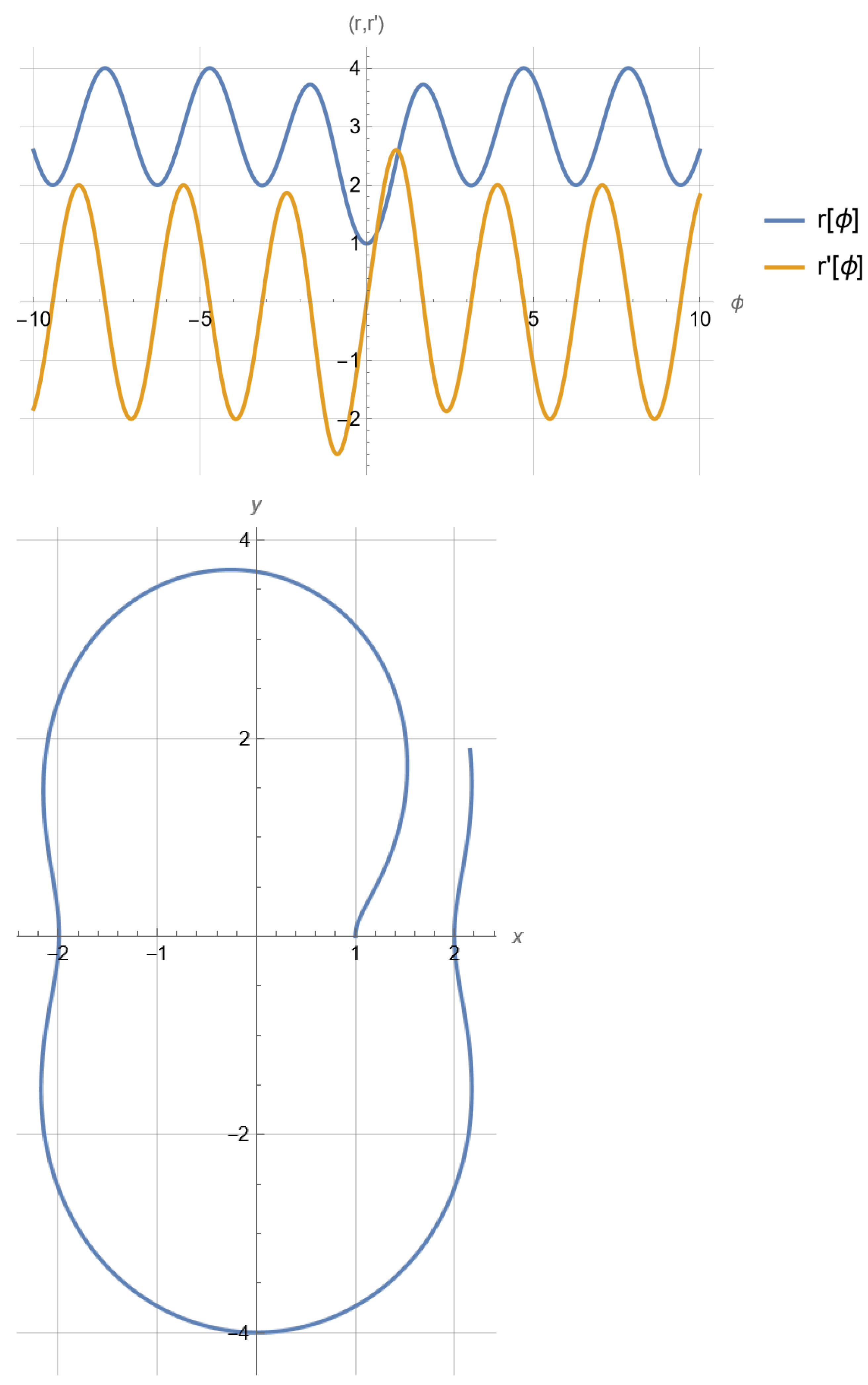
7. Perturbative Approach in the Description of the Josephson Junction

Perturbative G-L Approach
8. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bogomolny, E.E.B. Stability of Classical Solutions. Sov. J. Nucl. Phys. 1976, 24, 449. [Google Scholar]
- Belavin, A.A.; Polyakov, A.M.; Schwartz, A.S.; Tyupkin, Y.S. Pseudoparticle solutions of the Yang-Mills equations. Phys. Lett. 1975, B59, 85. [Google Scholar] [CrossRef]
- Prasad, K.M.; Sommerfield, C.M. Exact Classical Solution for the ’t Hooft Monopole and the Julia-Zee Dyon. Phys. Rev. Lett. 1975, 35, 760–762. [Google Scholar] [CrossRef]
- Hosoya, A. On Vanishing of Energy-Momentum Tensor for a Class of Instanton-Like Solutions. Prog. Theor. Phys. 1978, 59, 1781. [Google Scholar] [CrossRef]
- Białynicki-Birula, I. On the stability of solitons. In Nonlinear Problems in Theoretical Physcis; Lecture Notes in Physics; Ran˜ada, A.F., Ed.; Birkhäuser-Springer: Basel, Switzerland, 1979; Volume 98, p. 15. [Google Scholar]
- Manton, N.; Sutcliffe, P. Topological Solitons; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Meissner, K.A. The Classical Field Theory; PWN, Polish Scientific Publishers: Warsaw, Poland, 2013. (In Polish) [Google Scholar]
- Contatto, F. Integrable Abelian vortex-like solitons. Phys. Lett. B 2017, 768, 23. [Google Scholar] [CrossRef]
- Acalapati, E.; Ramadhan, H. First-order formalism for Alice string. Ann. Phys. 2024, 465, 169665. [Google Scholar] [CrossRef]
- Atmaja, A.N.; Gunara, B.E.; Prasetyo, I. BPS submodels of the generalized Skyrme model and how to find them. Nucl. Phys. B 2020, 955, 115062. [Google Scholar] [CrossRef]
- Canfora, F.; Lagos, M.; Vera, A. Superconducting multi-vortices and a novel BPS bound in chiral perturbation theory. arXiv 2024, arXiv:2405.08082. [Google Scholar] [CrossRef]
- Adam, C.; Romańczukiewicz, T.; Wachla, M.; Wereszczyxnxski, A. Exactly solvable gravitating perfect fluid solitons in (2+1) dimensions. J. High Energy Phys. 2018, 2018, 97. [Google Scholar] [CrossRef]
- Adam, C.; Queiruga, J.; Sanchez-Guillen, J.; Wereszczynski, A. Nuclear binding energies from a BPS Skyrme model. J. High Energy Phys. 2013, 88, 1–33. [Google Scholar]
- Comtet, A.; Forgacs, P.; Horvathy, P. Bogomolny-type equations in curved spacetime. Phys. Rev. D 1984, 30, 468. [Google Scholar] [CrossRef]
- Dobrowolski, T. Kink profile in a curved space. Acta Phys. Pol. B 2015, 46, 1457–1472. [Google Scholar] [CrossRef]
- Fadhilla, E.S.; Gunara, B.E.; Atmaja, A.N. Local existence of regular solutions in dynamical massless Einstein-Skyrme system. AIP Conf. Proc. 2023, 2975, 030001. [Google Scholar]
- Klimas, P. Composite BPS skyrmions from an exact isospin symmetry breaking. Acta Phys. Pol. B 2016, 47, 2245–2271. [Google Scholar] [CrossRef]
- Saxena, A.; Kevrekidis, P.G.; Cuevas-Maraver, J. Nonlinearity and Topology. In Emerging Frontiers in Nonlinear Science; Nonlinear Systems and Complexity; Springer: Cham, Switzerland, 2020. [Google Scholar]
- Tama, L.Y.P.; Gunara, B.E.; Atmaja, A.N. Electric-dual BPS vortices in the generalized self-dual Maxwell-Chern-Simons-Higgs model. Phys. Scr. 2023, 98, 025208. [Google Scholar] [CrossRef]
- Wachla, M. Gravitating gauged BPS baby Skyrmions. Phys. Rev. D 2019, 99, 065006. [Google Scholar] [CrossRef]
- Sokalski, K. Instantons in anisotropic ferromagnets. Acta Phys. Pol. 1979, A56, 571. [Google Scholar]
- Sokalski, K. Dynamical Stability of instantons. Phys. Lett. 1981, A81, 102. [Google Scholar] [CrossRef]
- Jochym, P.T.; Sokalski, K. Variational approach to the Bogomolny separation. J. Phys. A Math. Gen. 1993, 26, 3837. [Google Scholar] [CrossRef]
- Sokalski, K. Instantons in three-dimensional Heisenberg ferromagnets. Acta Phys. Pol. 1984, A65, 457. [Google Scholar]
- Sokalski, K.; Wietecha, T.; Lisowski, Z. A Concept of Strong Necessary Condition in Nonlinear Field Theory. Acta Phys. Pol. 2001, B32, 17. [Google Scholar]
- Sokalski, K.; Stȩpień, Ł.; Sokalska, D. The existence of Bogomolny decomposition by means of strong necessary conditions. J. Phys. A Math. Gen. 2002, A35, 6157. [Google Scholar] [CrossRef]
- Sokalski, K.; Wietecha, T.; Sokalska, D. Existence of Dual Equations by Means of Strong Necessary Conditions - Analysis of Integrability of Partial Differential Nonlinear Equations. J. Nonl. Math. Phys. 2005, 12, 31. [Google Scholar] [CrossRef]
- Sokalski, K.; Wietecha, T.; Lisowski, Z. Unified Variational Approach to the Bäcklund Transformations and the Bogomolny Decomposition. Int. J. Theor. Phys. Group Theory Nonl. Opt. NOVA 2002, 9, 331. [Google Scholar]
- Adam, C.; Santamaria, F. The First-Order Euler-Lagrange equations and some of their uses. J. High Energy Phys. 2016, 2016, 47. [Google Scholar] [CrossRef]
- Adam, C.; Naya, C.; Sanchez-Guillen, J.; Wereszczynski, A. Gauged BPS baby Skyrme model. Phys. Rev. D 2012, 86, 045010. [Google Scholar] [CrossRef]
- Schroers, B. Gauged sigma models and magnetic Skyrmions. Scipost Phys. 2019, 7, 030. [Google Scholar] [CrossRef]
- Stȩpień, Ł.T. The Existence of Bogomolny Decompositions for Gauged Nonlinear Sigma Model and for Gauged Baby Skyrme Models. Acta Phys. Pol. B 2015, 46, 999. [Google Scholar] [CrossRef]
- Stȩpień, Ł.T. On bogomolny equations in the Skyrme model. Acta Phys. Pol. B 2019, 50, 65. [Google Scholar] [CrossRef]
- Atmaja, A.N.; Ramadhan, H.S. Bogomol’nyi equations of classical solutions. Phys. Rev. D 2014, 90, 105009. [Google Scholar] [CrossRef]
- Akkermans, E.; Mallick, K. Aspects Topologiques de la Physique en Basse Dimension. Topological Aspects of Low Dimensional Systems; Les Houches Summer School; Comtet, A., Jolicoeur, T., Ouvry, S., David, F., Eds.; Springer: Berlin/Heidelberg, Germany, 1999; Volume 69, p. 843. [Google Scholar]
- Albert, J. The Abrikosov Vortex in Curved Space. J. High Energy Phys. 2021, 2021, 12. [Google Scholar] [CrossRef]
- Bystrov, A.S.; Mel’nikov, A.S.; Ryzhov, D.A.; Nefedov, I.M.; Shereshevskii, I.A.; Vysheslavtsev, P.P. Singular and Nonsingular Vortex Structures in High-Temperature Superconductors. Mod. Phys. Lett. B 2003, 17, 621. [Google Scholar] [CrossRef]
- Conti, S.; Otto, F.; Serfaty, S. Branched Microstructures in the G-L Model of Type-I Superconductors. SIAM J. Math. Anal. 2015, 48, 2994. [Google Scholar] [CrossRef]
- Jaykka, J.; Palmu, J. Knot solitons in modified G-L model. Phys. Rev. D 2011, 83, 105015. [Google Scholar] [CrossRef]
- Penin, A.; Weller, Q. A Theory of Giant Vortices. J. High Energy Phys. 2021, 2021, 056. [Google Scholar] [CrossRef]
- Serfaty, S. Emergence of the Abrikosov lattice in several models with two dimensional Coulomb interaction. In Proceedings of the European Congress of Mathematics, Kraków, Poland, 2–7 July 2012. [Google Scholar]
- Sandier, E.; Serfaty, S. Improved lower bounds for G-L energies via mass displacement. Anal. PDE 2011, 4, 757. [Google Scholar] [CrossRef]
- Weinan, E. Dynamics of vortices in G-L theories with applications to superconductivity. Phys. D Nonl. Phen. 1994, 77, 383. [Google Scholar] [CrossRef]
- Yang, Y. On the existence of multivortices in a generalized Bogomol’nyi system. Z. Angew. Math. Phys. 1992, 43, 677. [Google Scholar] [CrossRef]
- Burzlaff, J. A finite-energy SU(3) solution which does not satisfy the Bogomolny equations. Czech. J. Phys. B 1982, 32, 624. [Google Scholar] [CrossRef]
- Pomorski, K. Electrostatically Interacting Wannier Qubits in Curved Space. Materials 2024, 17, 4846. [Google Scholar] [CrossRef]
- Pomorski, K. Analytical view on topological defects of superconducting order parameter in various topologies of nanowires with focus on quantum detectors and Josephson junctions. Mol. Cryst. Liq. Cryst. 2022, 750, 105–134. [Google Scholar] [CrossRef]
- Stȩpień, Ł.T. Strong Necessary Conditions and the Cauchy Problem. Symmetry 2023, 15, 1622. [Google Scholar] [CrossRef]
- Felsager, B. Geometry, Particles and Fields; Springer: Berlin/Heidelberg, Germany, 1998. [Google Scholar]
- Morandi, G. The Role of Topology in Classical and Quantum Physics; Lecture Notes in Physics; Springer: Berlin/Heidelberg, Germany, 1992. [Google Scholar]
- Pomorski, K.D.; Pęczkowski, P.; Staszewski, R.B. Analytical solutions for N interacting electron system confined in graph of coupled electrostatic semiconductor and superconducting quantum dots in tight-binding model. Cryogenics 2020, 109, 103117. [Google Scholar] [CrossRef]
- Rezlescu, N.; Agop, M.; Buzea, C.G. Perturbative solutions of the G-L equation and the superconducting parameters. Phys. Rev. B 1996, 53, 2229. [Google Scholar] [CrossRef]
- Kita, T. Gor’kov, Eilenberger, and Ginzburg–Landau Equations, Statistical Mechanics of Superconductivity; Springer: Tokyo, Japan, 2015. [Google Scholar] [CrossRef]
- Gorkov, L.P. Microscopic Derivation of the G-L Equations in the Theory of Superconductivity. Sov. Phys.-JETP 1959, 36, 1364–1367. [Google Scholar]
- Abrikosov, A.; Gorkov, L.; Dzyaloshinski, I. Methods of Quantum Field Theory in Statistical Physics; Dover Publications: Mineola, NY, USA, 1975; ISBN 9780486632285. [Google Scholar]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Stȩpień, Ł.T.; Pomorski, K. Towards Ginzburg–Landau Bogomolny Approach and a Perturbative Description of Superconducting Structures. Quantum Rep. 2025, 7, 4. https://doi.org/10.3390/quantum7010004
Stȩpień ŁT, Pomorski K. Towards Ginzburg–Landau Bogomolny Approach and a Perturbative Description of Superconducting Structures. Quantum Reports. 2025; 7(1):4. https://doi.org/10.3390/quantum7010004
Chicago/Turabian StyleStȩpień, Łukasz T., and Krzysztof Pomorski. 2025. "Towards Ginzburg–Landau Bogomolny Approach and a Perturbative Description of Superconducting Structures" Quantum Reports 7, no. 1: 4. https://doi.org/10.3390/quantum7010004
APA StyleStȩpień, Ł. T., & Pomorski, K. (2025). Towards Ginzburg–Landau Bogomolny Approach and a Perturbative Description of Superconducting Structures. Quantum Reports, 7(1), 4. https://doi.org/10.3390/quantum7010004






