An Ultra-Energy-Efficient Reversible Quantum-Dot Cellular Automata 8:1 Multiplexer Circuit
Abstract
1. Introduction
2. Energy Dissipation Analysis of QCA Cells
3. Fully Reversible Design Concept
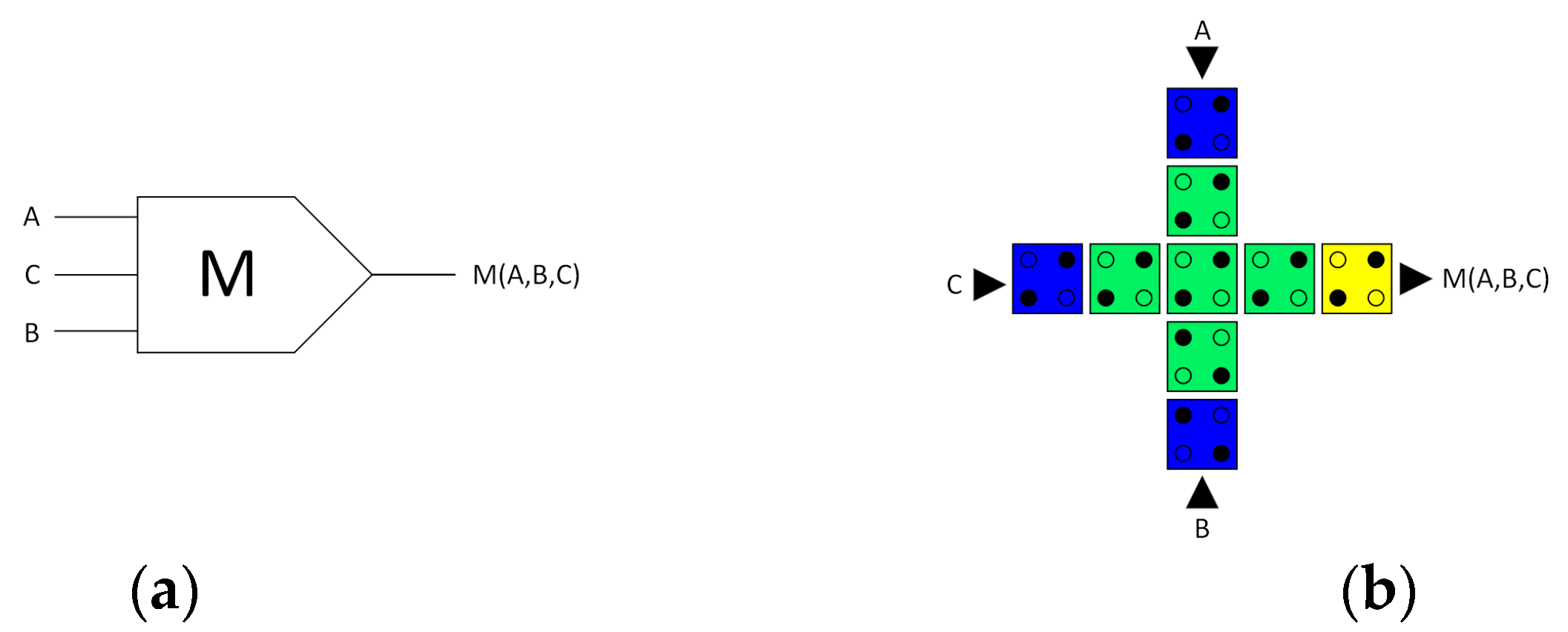
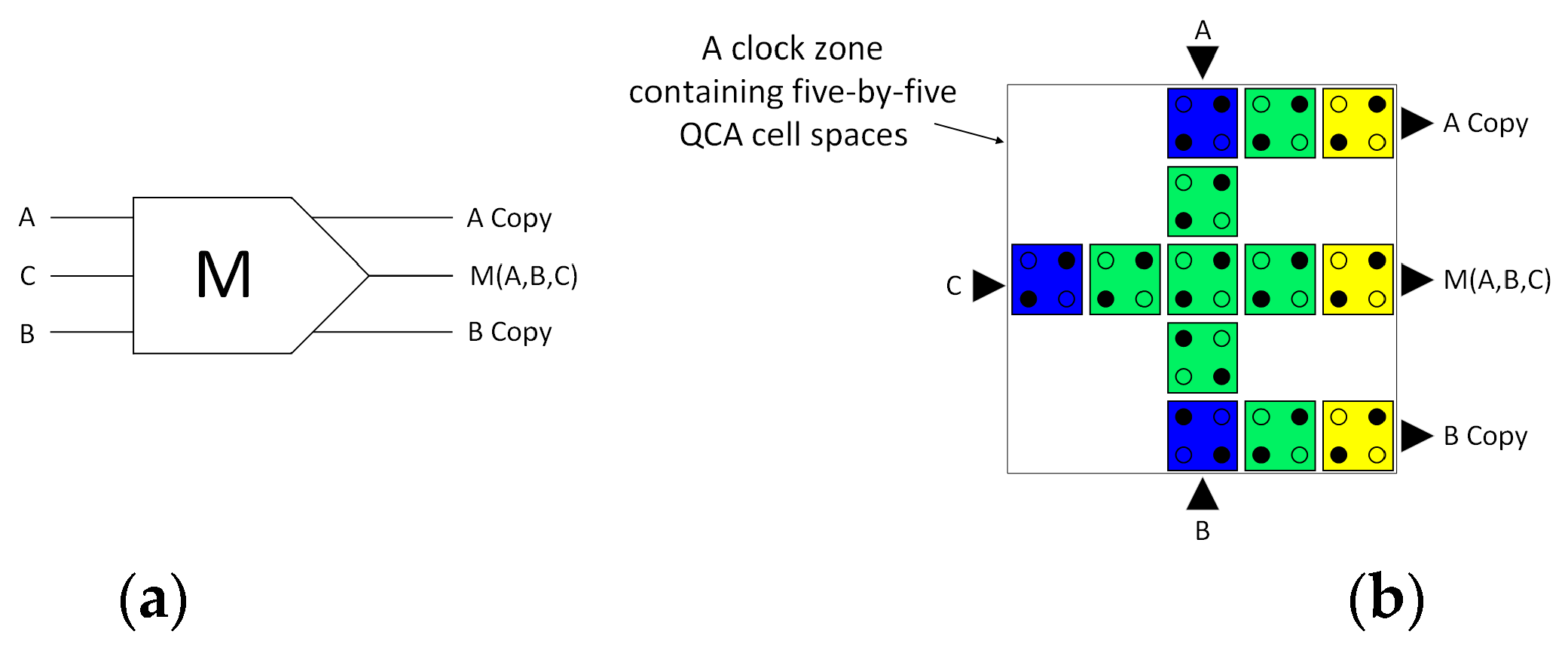
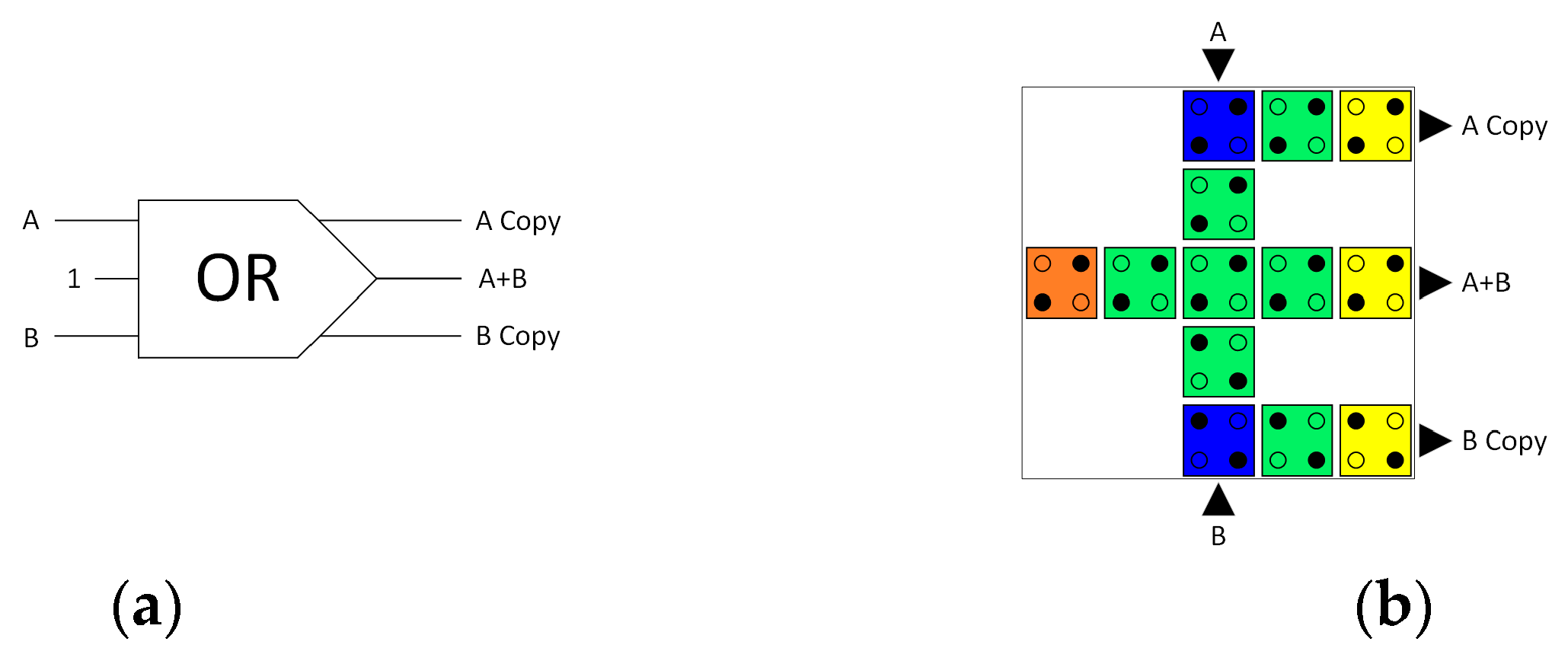
- Designing a QCA three-input-three-output majority gate. Subsequently, utilise it to produce reversible ‘AND’ and reversible ‘OR’ gates.
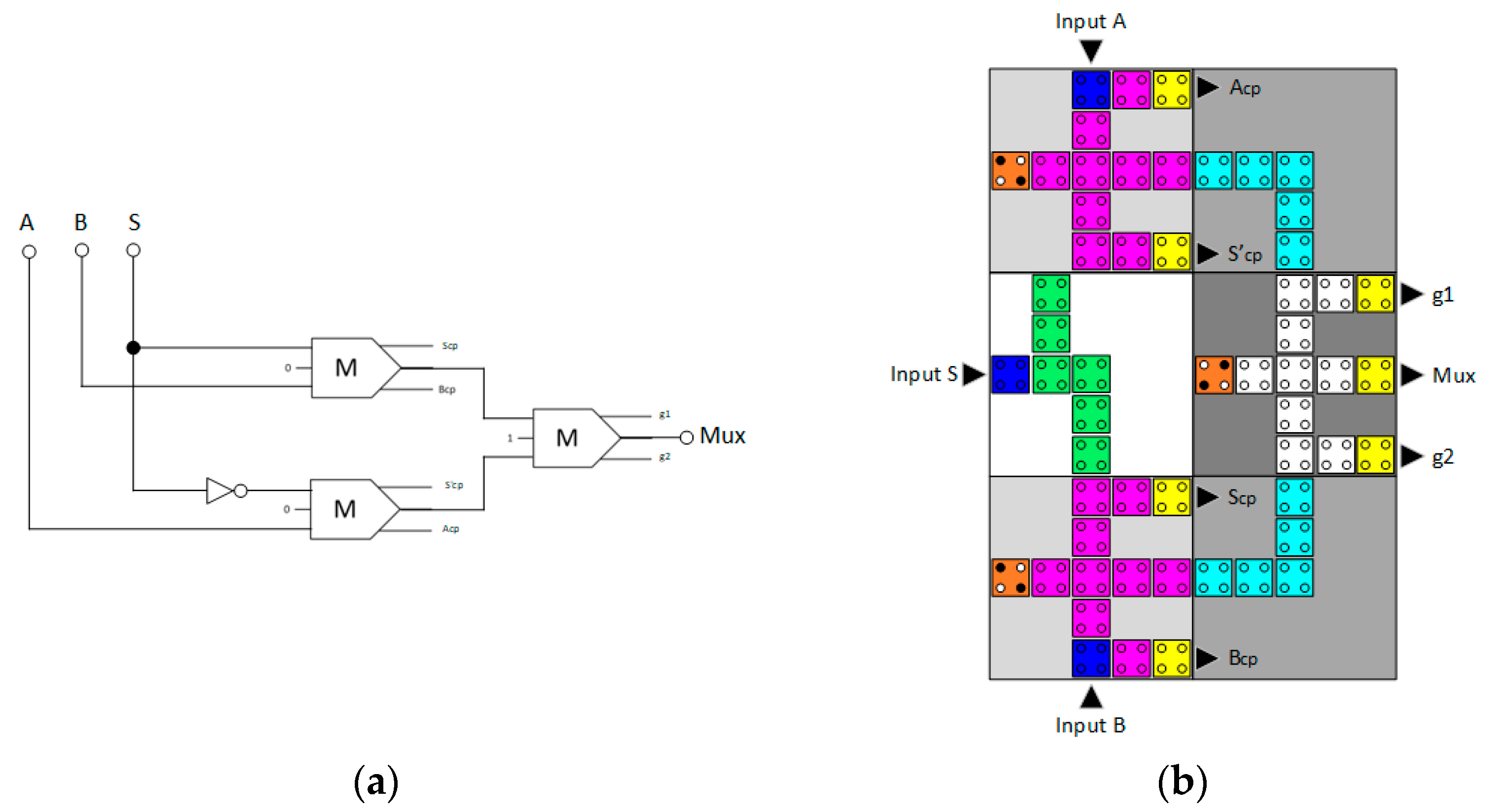
- Reversibly designing a QCA 2:1 multiplexer using two reversible AND gates, one reversible OR gate, and one single-branch inverter.
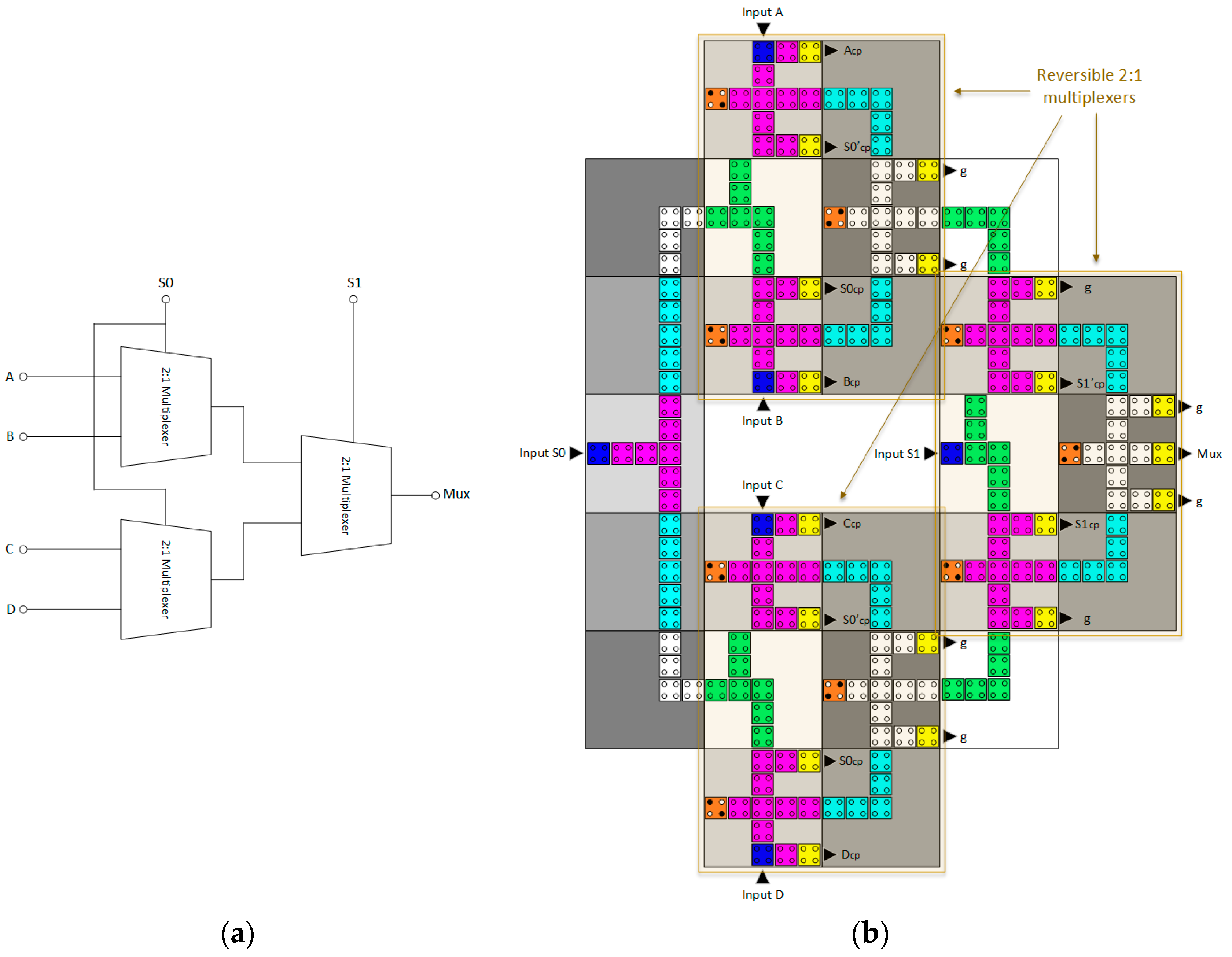
- Reversibly designing a QCA 4:1 multiplexer containing three reversible QCA 2:1 multiplexers.
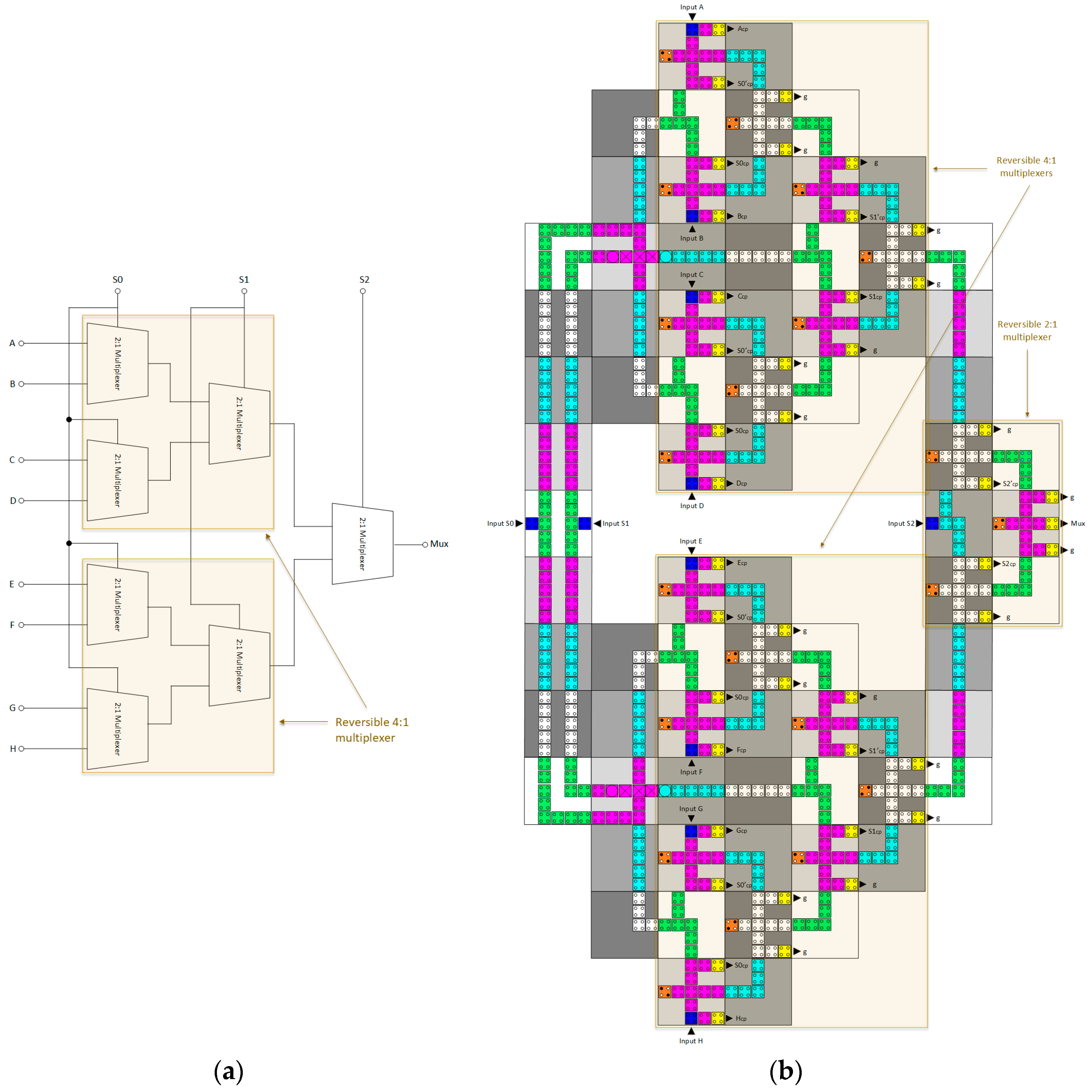
- Reversibly designing a QCA 8:1 multiplexer containing two reversible QCA 4:1 multiplexers and an additional single reversible QCA 2:1 multiplexer.
4. Design Process of the Proposed Fully Reversible QCA 8:1 Multiplexer
4.1. Reversible Fundamental Logic Gates
4.2. Reversible 2:1 Multiplexer
4.3. Reversible 4:1 Multiplexer
4.4. Reversible 8:1 Multiplexer
- At the first level, a set of four 2:1 multiplexers generates four output signals dependent upon the value of S0.
- At the second level, two 2:1 multiplexers produce two outputs, dependent upon the value of S1.
- At the third level, a single 2:1 multiplexer generates the final result, dependent upon the value of S2.
5. Energy Dissipation Simulation Results and Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Sen, B.; Sengupta, A.; Dalui, M.; Sikdar, B.K. Design of Testable Universal Logic Gate Targeting Minimum Wire-Crossings in QCA Logic Circuit. In Proceedings of the 2010 13th Euromicro Conference on Digital System Design: Architectures, Methods and Tools, Lille, France, 1–3 September 2010; pp. 613–620. [Google Scholar]
- Knobloch, T.; Selberherr, S.; Grasser, T. Challenges for nanoscale CMOS logic based on two-dimensional materials. Nanomaterials 2022, 12, 3548. [Google Scholar] [CrossRef]
- Lent, C.S.; Tougaw, P.D.; Porod, W.; Bernstein, G.H. Quantum cellular automata. Nanotechnology 1993, 4, 49. [Google Scholar] [CrossRef]
- DeBenedictis, E.P.; Frank, M.P.; Ganesh, N.; Anderson, N.G. A path toward ultra-low-energy computing. In Proceedings of the 2016 IEEE International Conference on Rebooting Computing (ICRC), San Diego, CA, USA, 17–19 October 2016; pp. 1–8. [Google Scholar]
- Bennett, C.H. Logical reversibility of computation. IBM J. Res. Dev. 1973, 17, 525–532. [Google Scholar] [CrossRef]
- Orlov, A.O.; Amlani, I.; Bernstein, G.H.; Lent, C.S.; Snider, G.L. Realization of a functional cell for quantum-dot cellular automata. Science 1997, 277, 928–930. [Google Scholar] [CrossRef]
- Garlando, U.; Riente, F.; Turvani, G.; Ferrara, A.; Santoro, G.; Vacca, M.; Graziano, M. Architectural exploration of perpendicular Nano Magnetic Logic based circuits. Integration 2018, 63, 275–282. [Google Scholar] [CrossRef]
- Orlov, A.; Imre, A.; Csaba, G.; Ji, L.; Porod, W.; Bernstein, G. Magnetic quantum-dot cellular automata: Recent developments and prospects. J. Nanoelectron. Optoelectron. 2008, 3, 55–68. [Google Scholar] [CrossRef]
- Lent, C.S.; Isaksen, B.; Lieberman, M. Molecular quantum-dot cellular automata. J. Am. Chem. Soc. 2003, 125, 1056–1063. [Google Scholar] [CrossRef]
- Huff, T.; Labidi, H.; Rashidi, M.; Livadaru, L.; Dienel, T.; Achal, R.; Vine, W.; Pitters, J.; Wolkow, R.A. Binary atomic silicon logic. Nat. Electron. 2018, 1, 636–643. [Google Scholar] [CrossRef]
- Wang, Y.; Lieberman, M. Thermodynamic behavior of molecular-scale quantum-dot cellular automata (QCA) wires and logic devices. IEEE Trans. Nanotechnol. 2004, 3, 368–376. [Google Scholar] [CrossRef]
- Lent, C.S. Bypassing the transistor paradigm. Science 2000, 288, 1597–1599. [Google Scholar] [CrossRef]
- Ardesi, Y.; Beretta, G.; Vacca, M.; Piccinini, G.; Graziano, M. Impact of molecular electrostatics on field-coupled nanocomputing and quantum-dot cellular automata circuits. Electronics 2022, 11, 276. [Google Scholar] [CrossRef]
- Sill Torres, F.; Wille, R.; Niemann, P.; Drechsler, R. An Energy-Aware Model for the Logic Synthesis of Quantum-Dot Cellular Automata. IEEE Trans. Comput.-Aided Des. Integr. Circuits Syst. 2018, 37, 3031–3041. [Google Scholar] [CrossRef]
- Timler, J.; Lent, C.S. Power gain and dissipation in quantum-dot cellular automata. J. Appl. Phys. 2002, 91, 823–831. [Google Scholar] [CrossRef]
- Srivastava, S.; Sarkar, S.; Bhanja, S. Estimation of upper bound of power dissipation in QCA circuits. IEEE Trans. Nanotechnol. 2008, 8, 116–127. [Google Scholar] [CrossRef]
- Rahimi, E. Energy dissipation of quantum-dot cellular automata logic gates. Micro Nano Lett. 2016, 11, 369–371. [Google Scholar] [CrossRef]
- Walus, K.; Jullien, G.A. Design tools for an emerging SoC technology: Quantum-dot cellular automata. Proc. IEEE 2006, 94, 1225–1244. [Google Scholar] [CrossRef]
- Taucer, M.; Karim, F.; Walus, K.; Wolkow, R.A. Consequences of many-cell correlations in clocked quantum-dot cellular automata. IEEE Trans. Nanotechnol. 2015, 14, 638–647. [Google Scholar] [CrossRef]
- Alharbi, M.; Edwards, G.; Stocker, R. Design and Simulation of Reversible Time-Synchronized Quantum-Dot Cellular Automata Combinational Logic Circuits with Ultralow Energy Dissipation. Int. Trans. J. Eng. Manag. Appl. Sci. Technol. 2022, 13, 1–22. [Google Scholar] [CrossRef]
- Alharbi, M.; Edwards, G.; Stocker, R. Novel ultra-energy-efficient reversible designs of sequential logic quantum-dot cellular automata flip-flop circuits. J. Supercomput. 2023, 79, 11530–11557. [Google Scholar] [CrossRef]
- Alharbi, M.; Edwards, G.; Stocker, R. Reversible Quantum-Dot Cellular Automata-Based Arithmetic Logic Unit. Nanomaterials 2023, 13, 2445. [Google Scholar] [CrossRef]
- Torres, F.S.; Niemann, P.; Wille, R.; Drechsler, R. Near Zero-Energy Computation Using Quantum-Dot Cellular Automata. ACM J. Emerg. Technol. Comput. Syst. 2020, 16, 1–16. [Google Scholar] [CrossRef]
- Landauer, R. Irreversibility and heat generation in the computing process. IBM J. Res. Dev. 1961, 5, 183–191. [Google Scholar] [CrossRef]
- Safaiezadeh, B.; Mahdipour, E.; Haghparast, M.; Sayedsalehi, S.; Hosseinzadeh, M. Novel design and simulation of reversible ALU in quantum dot cellular automata. J. Supercomput. 2022, 78, 868–882. [Google Scholar] [CrossRef]
- Darji, P.G.; Makwana, J. Reversible QCA based Full-adder and Subtractor in Nanotechnology. In Proceedings of the 2022 IEEE Region 10 Symposium (TENSYMP), Mumbai, India, 1–3 July 2022; pp. 1–5. [Google Scholar]
- Naz, S.F.; Ahmed, S.; Sharma, S.; Ahmad, F.; Ajitha, D. Fredkin gate based energy efficient reversible D flip flop design in quantum dot cellular automata. Mater. Today Proc. 2021, 46, 5248–5255. [Google Scholar] [CrossRef]
- Norouzi, M.; Heikalabad, S.R.; Salimzadeh, F. A reversible ALU using HNG and Ferdkin gates in QCA nanotechnology. Int. J. Circuit Theory Appl. 2020, 48, 1291–1303. [Google Scholar] [CrossRef]
- Pandiammal, K.; Meganathan, D. Design of 8 bit reconfigurable ALU using quantum dot cellular automata. In Proceedings of the 2018 IEEE 13th Nanotechnology Materials and Devices Conference (NMDC), Portland, OR, USA, 14–17 October 2018; pp. 1–4. [Google Scholar]
- Lent, C.S.; Tougaw, P.D. A device architecture for computing with quantum dots. Proc. IEEE 1997, 85, 541–557. [Google Scholar] [CrossRef]
- Vankamamidi, V.; Ottavi, M.; Lombardi, F. Two-dimensional schemes for clocking/timing of QCA circuits. IEEE Trans. Comput.-Aided Des. Integr. Circuits Syst. 2007, 27, 34–44. [Google Scholar] [CrossRef]
- Campos, C.A.T.; Marciano, A.L.; Vilela Neto, O.P.; Torres, F.S. USE: A Universal, Scalable, and Efficient Clocking Scheme for QCA. IEEE Trans. Comput.-Aided Des. Integr. Circuits Syst. 2016, 35, 513–517. [Google Scholar] [CrossRef]
- Bajec, I.L.; Pečar, P. Two-layer synchronized ternary quantum-dot cellular automata wire crossings. Nanoscale Res. Lett. 2012, 7, 221. [Google Scholar] [CrossRef]
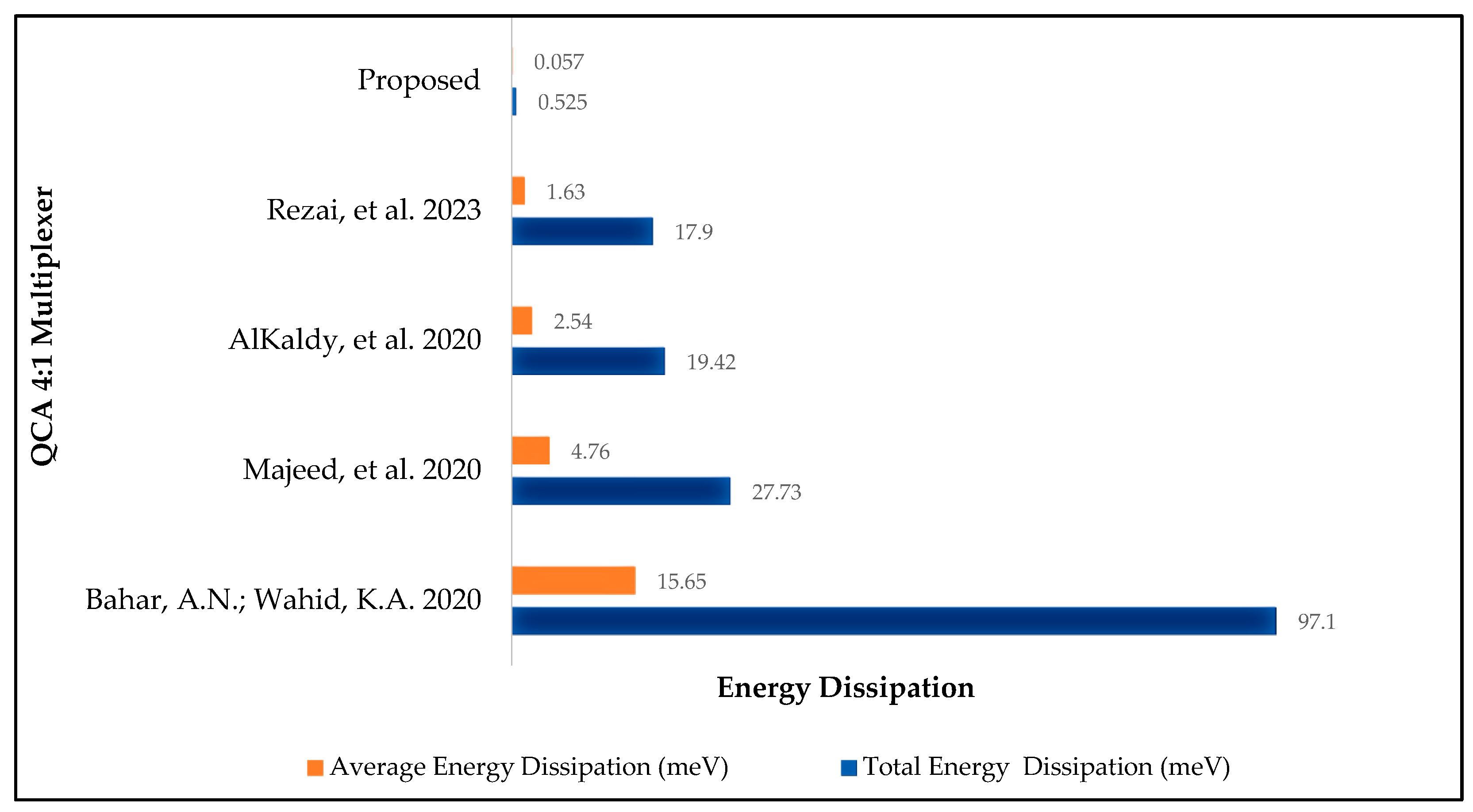
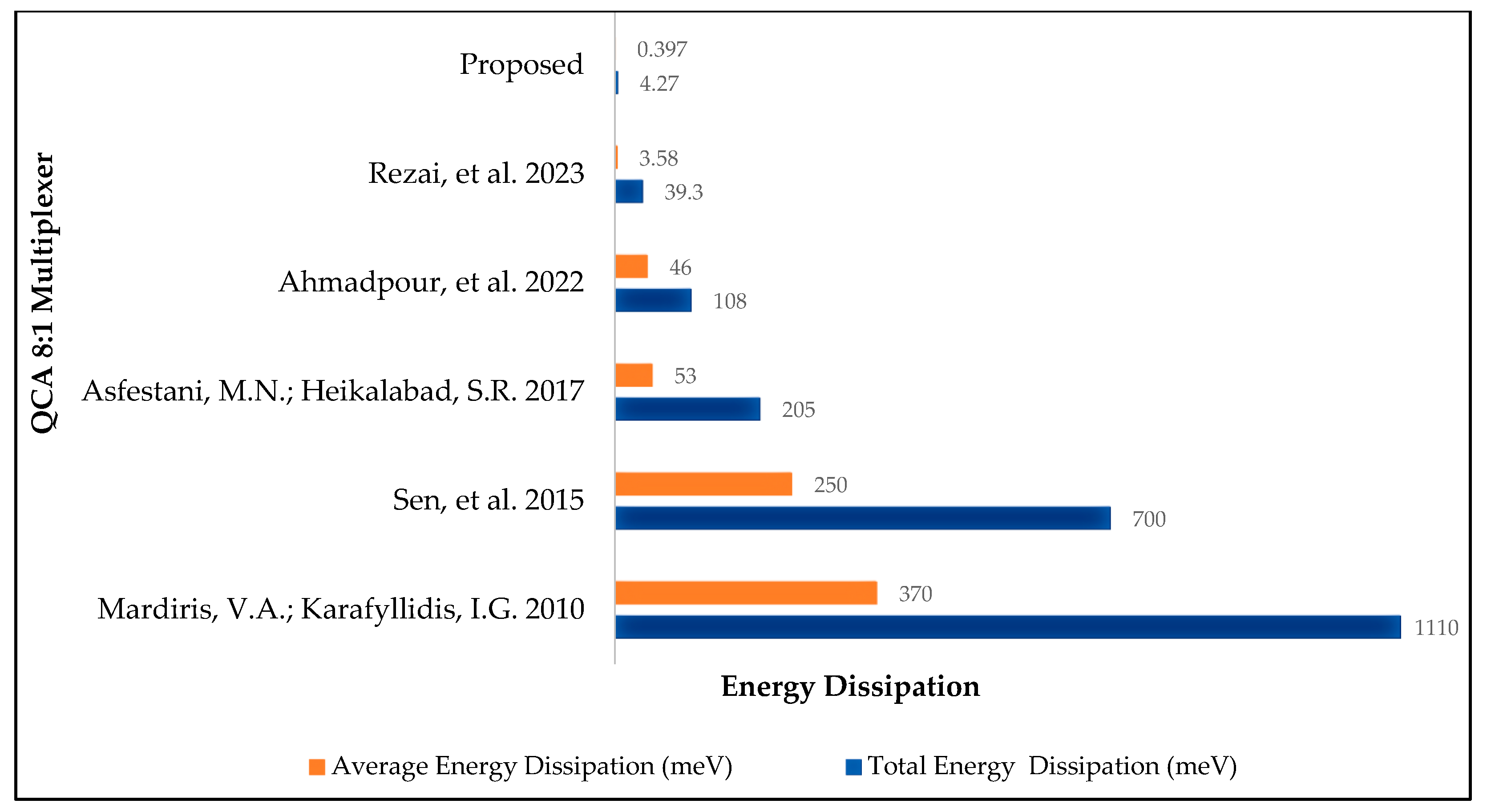
- Rezai, A.; Aliakbari, D.; Karimi, A. Novel multiplexer circuit design in quantum-dot cellular automata technology. Nano Commun. Netw. 2023, 35, 100435. [Google Scholar] [CrossRef]
- Ahmadpour, S.-S.; Mosleh, M. A novel fault-tolerant multiplexer in quantum-dot cellular automata technology. J. Supercomput. 2018, 74, 4696–4716. [Google Scholar] [CrossRef]
- Jeon, J.-C. Designing nanotechnology QCA–multiplexer using majority function-based NAND for quantum computing. J. Supercomput. 2021, 77, 1562–1578. [Google Scholar] [CrossRef]
- Asfestani, M.N.; Heikalabad, S.R. A unique structure for the multiplexer in quantum-dot cellular automata to create a revolution in design of nanostructures. Phys. B Condens. Matter 2017, 512, 91–99. [Google Scholar] [CrossRef]
- Khan, A.; Mandal, S. Robust multiplexer design and analysis using quantum dot cellular automata. Int. J. Theor. Phys. 2019, 58, 719–733. [Google Scholar] [CrossRef]
- Khan, A.; Arya, R. Design and energy dissipation analysis of simple QCA multiplexer for nanocomputing. J. Supercomput. 2022, 78, 8430–8444. [Google Scholar] [CrossRef]
- Bahar, A.N.; Wahid, K.A. Design and implementation of approximate DCT architecture in quantum-dot cellular automata. IEEE Trans. Very Large Scale Integr. (VLSI) Syst. 2020, 28, 2530–2539. [Google Scholar] [CrossRef]
- Majeed, A.H.; Alkaldy, E.; Zainal, M.S.; Navi, K.; Nor, D. Optimal design of RAM cell using novel 2:1 multiplexer in QCA technology. Circuit World 2020, 46, 147–158. [Google Scholar] [CrossRef]
- AlKaldy, E.; Majeed, A.H.; Zainal, M.S.; Nor, D.M. Optimum multiplexer design in quantum-dot cellular automata. arXiv 2020, arXiv:2002.00360. [Google Scholar] [CrossRef]
- Mardiris, V.A.; Karafyllidis, I.G. Design and simulation of modular 2n to 1 quantum-dot cellular automata (QCA) multiplexers. Int. J. Circuit Theory Appl. 2010, 38, 771–785. [Google Scholar] [CrossRef]
- Sen, B.; Goswami, M.; Mazumdar, S.; Sikdar, B.K. Towards modular design of reliable quantum-dot cellular automata logic circuit using multiplexers. Comput. Electr. Eng. 2015, 45, 42–54. [Google Scholar] [CrossRef]
- Ahmadpour, S.-S.; Mosleh, M.; Rasouli Heikalabad, S. Efficient designs of quantum-dot cellular automata multiplexer and RAM with physical proof along with power analysis. J. Supercomput. 2022, 78, 1672–1695. [Google Scholar] [CrossRef]


| Parameter | Description | Value |
|---|---|---|
| QD size | Quantum-dot size | 5 nm |
| Cell area | Dimensions of each cell | 18 × 18 nm |
| Cell distance | Distance between two cells | 2 nm |
| Layer separation | Distance between QCA layers in multilayer crossing | 11.5 nm |
| Clock high | Max. saturation energy of clock signal | 9.8 × 10−22 J |
| Clock low | Min. saturation energy of clock signal | 3.8 × 10−23 J |
| Relative permittivity | Relative permittivity of QCA materials (GaAs and AlGaAs) | 12.9 |
| Radius of effect | Maximum interaction distance between cells | 80 nm |
| Temp | Operating temperature | 1 K |
| Parameter | Description | Value |
|---|---|---|
| τ | Relaxation time | 1 × 10−15 s |
| Tγ | Period of the clock signal | 1 × 10−9 s |
| Tin | Period of the input signals | 1 × 10−9 s |
| Tstep | Time interval of each iteration step | 1 × 10−16 s |
| Tsim | Total simulation time | 8 × 10−9 s |
| γshape | Shape of the clock signal slopes | Gaussian |
| γslope | Rise and fall time of the clock signal slopes | 1 × 10−10 s |
| S | Mux |
|---|---|
| 0 | A |
| 1 | B |
| S1 | S0 | Mux |
|---|---|---|
| 0 | 0 | A |
| 0 | 1 | B |
| 1 | 0 | C |
| 1 | 1 | D |
| S2 | S1 | S0 | Output |
|---|---|---|---|
| 0 | 0 | 0 | A |
| 0 | 0 | 1 | B |
| 0 | 1 | 0 | C |
| 0 | 1 | 1 | D |
| 1 | 0 | 0 | E |
| 1 | 0 | 1 | F |
| 1 | 1 | 0 | G |
| 1 | 1 | 1 | H |
| Proposed QCA Circuit | Total Energy Dissipation (meV) | Average Energy Dissipation (meV) |
|---|---|---|
| Reversible AND gate | 0.009 | 0.003 |
| Reversible OR gate | 0.009 | 0.002 |
| Reversible 2:1 multiplexer | 0.112 | 0.014 |
| Reversible 4:1 multiplexer | 0.525 | 0.057 |
| Reversible 8:1 multiplexer | 4.27 | 0.397 |
| QCA Multiplexer Circuit | Used Inverters | Used AND Gates | Used OR Gates | QCA Cells | Area (µm) | Dealy (Clock Cycle) |
|---|---|---|---|---|---|---|
| Reversible 2:1 multiplexer | 1 | 2 | 1 | 56 | 0.09 | 1 |
| Reversible 4:1 multiplexer | 3 | 6 | 3 | 213 | 0.46 | 3 |
| Reversible 8:1 multiplexer | 7 | 14 | 7 | 646 | 1.36 | 5.5 |
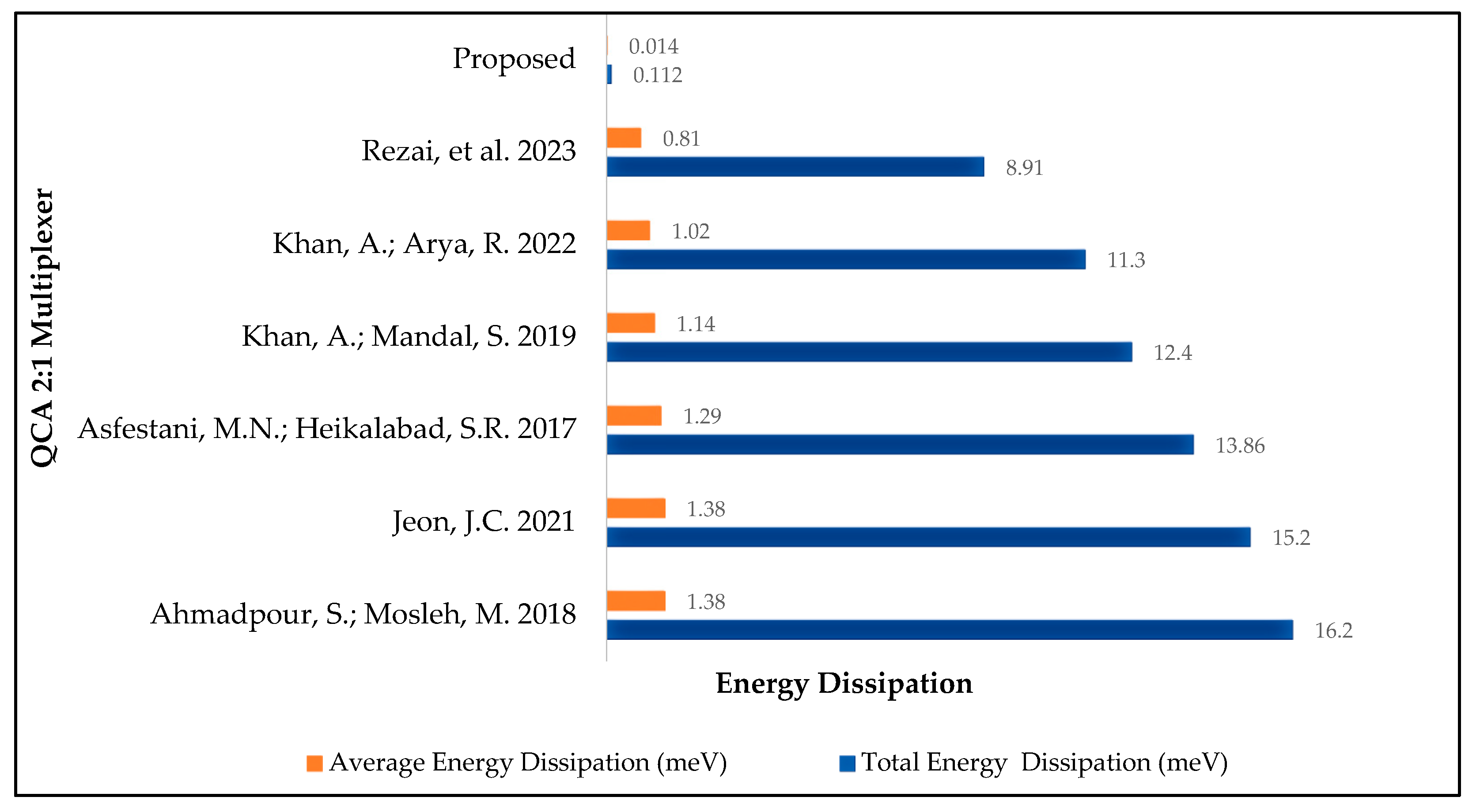
| QCA 2:1 Multiplexer | Total Energy Dissipation (meV) | Average Energy Dissipation (meV) |
|---|---|---|
| [35] | 16.20 | 1.38 |
| [36] | 15.20 | 1.38 |
| [37] | 13.86 | 1.29 |
| [38] | 12.40 | 1.14 |
| [39] | 11.30 | 1.02 |
| [34] | 8.91 | 0.810 |
| Proposed | 0.112 | 0.014 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alharbi, M.; Edwards, G.; Stocker, R. An Ultra-Energy-Efficient Reversible Quantum-Dot Cellular Automata 8:1 Multiplexer Circuit. Quantum Rep. 2024, 6, 41-57. https://doi.org/10.3390/quantum6010004
Alharbi M, Edwards G, Stocker R. An Ultra-Energy-Efficient Reversible Quantum-Dot Cellular Automata 8:1 Multiplexer Circuit. Quantum Reports. 2024; 6(1):41-57. https://doi.org/10.3390/quantum6010004
Chicago/Turabian StyleAlharbi, Mohammed, Gerard Edwards, and Richard Stocker. 2024. "An Ultra-Energy-Efficient Reversible Quantum-Dot Cellular Automata 8:1 Multiplexer Circuit" Quantum Reports 6, no. 1: 41-57. https://doi.org/10.3390/quantum6010004
APA StyleAlharbi, M., Edwards, G., & Stocker, R. (2024). An Ultra-Energy-Efficient Reversible Quantum-Dot Cellular Automata 8:1 Multiplexer Circuit. Quantum Reports, 6(1), 41-57. https://doi.org/10.3390/quantum6010004