Fricke Topological Qubits

Abstract

We recently proposed that topological quantum computing might be based on $SL(2,\mathbb{C})$ representations of the fundamental group ${\pi }_1(S^3\backslash K)$ for the complement of a link K in the three-sphere. The restriction to links whose associated $SL(2,\mathbb{C})$ character variety $\mathcal{V}$ contains a Fricke surface ${\kappa }_d=xyz-x^2-y^2-z^2+d$ is desirable due to the connection of Fricke spaces to elementary topology. Taking K as the Hopf link $L2a1$, one of the three arithmetic two-bridge links (the Whitehead link $5_1^2$, the Berge link $6_2^2$ or the double-eight link $6_3^2$) or the link $7_3^2$, the $\mathcal{V}$ for those links contains the reducible component ${\kappa }_4$, the so-called Cayley cubic. In addition, the $\mathcal{V}$ for the latter two links contains the irreducible component ${\kappa }_3$, or ${\kappa }_2$, respectively. Taking $\rho$ to be a representation with character ${\kappa }_d$ ($d<4$), with $\left|x\right|,\left|y\right|,\left|z\right|\le 2$, then $\rho \left({\pi }_1\right)$ fixes a unique point in the hyperbolic space ${\mathcal{H}}_3$ and is a conjugate to a $SU\left(2\right)$ representation (a qubit). Even though details on the physical implementation remain open, more generally, we show that topological quantum computing may be developed from the point of view of three-bridge links, the topology of the four-punctured sphere and Painlevé VI equation. The 0-surgery on the three circles of the Borromean rings L6a4 is taken as an example.

1. Introduction

Building a quantum computer is still challenging. However, progress has been made using natural and artificial atoms [1], superconducting technology [2] and other physical techniques [3,4]. One of the greatest challenges involved with constructing quantum computers is controlling or removing quantum decoherence. One possible solution is to create a topological quantum computer.

The paper describes progress towards an understanding and possibly an implementation of quantum computation based on algebraic surfaces. In the orthodox acceptation, a topological quantum computer deploys two-dimensional quasiparticles called anyons that are braids in three dimensions. The braids lead to logic gates used for computation. The topological nature of the braids makes the quantum computation less sensitive to the decoherence errors than in a standard quantum computer [5,6]. One theoretical proposal of universal quantum computation is based on Fibonacci anyons that are non-Abelian anyons with fusion rules. In particular, a fractional quantum Hall device would, in principle, realize a topological qubit. Owing to the lack of evidence that such quantum Hall-based anyons have been obtained, other theoretical proposals are worthwhile to develop. A recent paper of our group proposed a correspondence between the fusion Hilbert space of Fibonacci anyons and the tiling two-dimensional space of the one-dimensional Fibonacci chain [7].

In this paper, following our recent proposal [8] (see also [9]), we propose a non-anyonic theory of a topological quantum computer based on surfaces in a three-dimensional topological space. Such surfaces are part of the $SL(2,\mathbb{C})$ character variety underlying the symmetries of a properly chosen manifold. In our earlier work, we were interested in basing topological quantum computing on three- or four-manifolds defined from the complement of a knot or link. In [10,11], our goal was to define informationally complete quantum measurements from three-manifolds and, in [12], from four-manifolds, seeing the embedding four-dimensional ‘exotic’ space $R^4$ of the manifold as a the physical Euclidean space-time. In the later paper, exotism means that one can define homeomorphic but non-diffeomorphic four-dimensional manifolds to interpret a type of ‘many-world’ quantum measurements.

Our concepts in [8] and in the present paper are different in the sense that the $SL(2,\mathbb{C})$ character variety is the three-dimensional locus of the supposed qubit prior to its measurement. The Lorentz group $SL(2,\mathbb{C})$ reads the symmetries of the selected topology like that of the punctured torus, the quadruply punctured sphere or the topology obtained from the complement of a knot or a link. Our work in [8] focused on the complement of the Hopf link—the linking of two unknotted curves—where the character variety consists of the Cayley cubic ${\kappa }_4(x,y,z)$. Here, we took the broader context of Fricke surfaces, whose compact bounded component consists of the $SU\left(2\right)$ representations [13]. Such representations are our proposed model of the topological qubits.

In Section 2, we recall the definition of the $SL(2,\mathbb{C})$-character variety for a manifold M whose fundamental group is ${\pi }_1\left(M\right)$ and the method used to build it in an explicit way.

In Section 3, we focus on the character variety ${\kappa }_2(x,y,z)$ for the fundamental group $F_2$ (the free group of rank 2) of the once-punctured torus $S_{1,1}$ and on the character variety ${\kappa }_4(x,y,z)$ attached to the fundamental group of the Hopf link L2a1. The former case is found to be related to the two-bridge link $L7a4$. The role of the extended mapping class group ${\mathrm{Mod}}^{\pm }\left(S_{1,1}\right)$ on a character variety of type ${\kappa }_d(x,y,z)$, $d\in \mathbb{C}$, is emphasized. We also introduce the concept of a topological qubit associated to the bounded $SU\left(2\right)$ component of the surface ${\kappa }_2(x,y,z)$.

In Section 4, we focus on the character variety $V_{a,b,c,d}(x,y,z)$ for the fundamental group (the free group of index 3) $F_3$ of the quadruply punctured sphere $S_{4,2}$. In particular, we recall the conditions that separate the compact (and bounded) $SU\left(2\right)$ component and the non-compact $SL(2,\mathbb{R})$ component. The investigation of the coverings of the four-manifold ${\tilde{E}}_8$ (the Kodaira singular fiber $I{I}^{\ast }$) allows for an application of the theory. In the same section, we describe the Riemann–Hilbert correspondence for the case of $S_{4,2}$, as well as the so-called Painlevé-Okomoto correspondence. The Painlevé VI equation plays a special role.

In Section 5, we apply some perspectives of the present research toward topological quantum computing related to cosmology.

2. The $\mathit{SL}(\mathbf{2},\mathbb{C})$-Character Variety of a Manifold $\mathbf{M}$

Let ${\pi }_1$ be the fundamental group of a topological surface S. We describe the representations of ${\pi }_1$ in the Lorentz group $SL(2,\mathbb{C})$, the group of $(2\times 2)$ matrices with complex entries and determinant 1. Such a group contains representations as degrees of freedom for all quantum fields and is the gauge group for the Einstein–Cartan theory, which contains the Einstein–Hilbert action and Einstein’s field equations [14]. Topological formulations of gravity have been introduced [15,16,17] and the relationship of entanglement with spacetime has been articulated [18,19], which motivates the exploration of $SL(2,\mathbb{C})$ character varieties for quantum computation.

Representations of ${\pi }_1$ in $SL(2,\mathbb{C})$ are homomorphisms $\rho :{\pi }_1\to SL(2,\mathbb{C})$ with character ${\kappa }_{\rho }\left(g\right)=tr\left(\rho \left(g\right)\right)$, $g\in {\pi }_1$. The set of characters allows us to define an algebraic set by taking the quotient of the set of representations $\rho$ by the group $S{L}_2\left(\mathbb{C}\right)$, which acts by conjugation on representations [13,20].

Below, we need the distinction between the two real forms $SU\left(2\right)$ and $SL(2,\mathbb{R})$ of the group $SL(2,\mathbb{C})$. The $SU\left(2\right)$ representations are those that fix a point in the three-dimensional hyperbolic space ${\mathbb{H}}_3$ and $SL(2,\mathbb{R})$ representations are those that preserve a two-dimensional hyperbolic space ${\mathbb{H}}_2$ in ${\mathbb{H}}_3$, as well as an orientation of ${\mathbb{H}}_2$. Both real forms of $SL(2,\mathbb{C})$ play an important role in our attempt to define and stabilize the potential Fricke topological qubits.

A Sage Program for Computing the $SL(2,\mathbb{C})$-Character Variety of ${\pi }_1\left(S\right)$

The SL$(2,\mathbb{C})$ character variety of a manifold M defined in SnapPy may be calculated in Sage using a program [21] written by the last author of [20] as follows:

from snappy import Manifold

M = Manifold(‘M’)

G = M.fundamental_group()

I = G.character_variety_vars_and_polys(as_ideal=True)

I.groebner_basis()

In some cases, a Groebner basis is not obtained from Sage. One may also use Magma [22] to obtain the Groebner basis or a small basis with the shortest length of the ideal I.

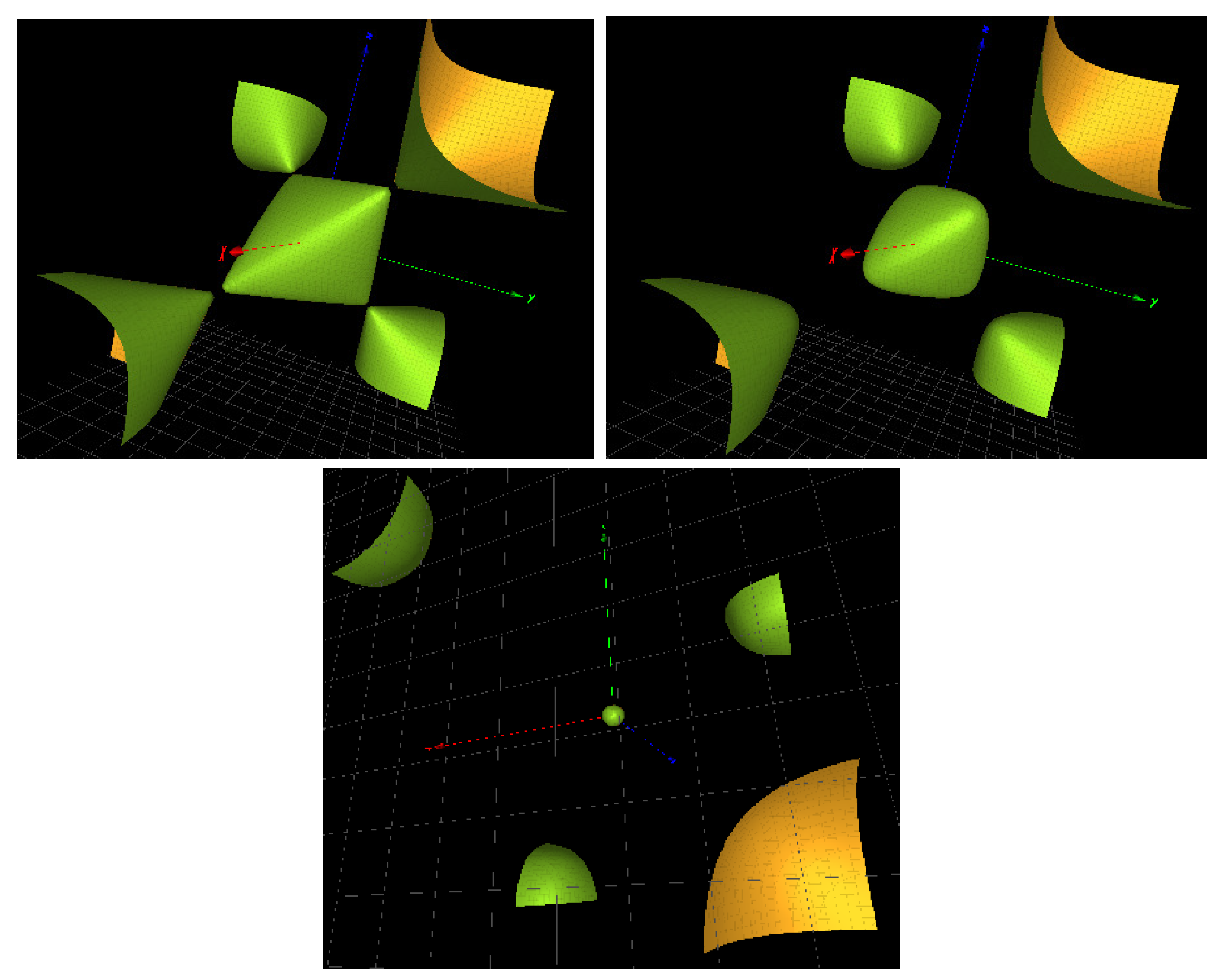
3. The Cubic Surface ${\mathit{\kappa }}_{\mathit{d}}(\mathit{x},\mathit{y},\mathit{z})$ and Two-Bridge Links

Following [23], in this section, we describe the special case of representations for the punctured torus $S_{1,1}$ and the relevance of the extended mapping class group ${\mathrm{Mod}}^{\pm }\left(S_{1,1}\right)$ in its action on surfaces of type ${\kappa }_d(x,y,z)$, $d\in \mathbb{C}$. Then, we find that surfaces ${\kappa }_2(x,y,z)$ and ${\kappa }_3(x,y,z)$ are contained in the character variety for the fundamental group of links L7a4 and L6a1, respectively. The $SU\left(2\right)$ representations and the concept of a Fricke topological qubit is outlined.

3.1. The $SL(2,\mathbb{C})$-Character Variety for a Once Punctured Torus

Let us take the example of the punctured torus $T_{1,1}$ whose fundamental group ${\pi }_1$ is the free group $F_2=〈a,b|Ø〉$ on two generators a and b. The boundary component of $T_{1,1}$ is a single loop around the puncture expressed by the commutator $[a,b]=abAB$ with $A=a^{-1}$ and $B=b^{-1}$. We introduce the traces

$$x=\mathrm{tr}\left(\rho \right(a\left)\right),y=\mathrm{tr}\left(\rho \right(b\left)\right),z=\mathrm{tr}\left(\rho \right(ab\left)\right).$$

The trace of the commutator is the surface [13,23]

$$\mathrm{tr}\left([a,b]\right)={\kappa }_2(x,y,z)=x^2+y^2+z^2-xyz-2.$$

Another noticeable surface is obtained from the character variety attached to the fundamental group of the Hopf link $L2a1$ that links two unknotted curves. For the Hopf link, the fundamental group is

$${\pi }_1(S^3\backslash L2a1)=〈a,b\left|\right[a,b]〉={\mathbb{Z}}^2,$$

and the corresponding character variety is the Cayley cubic [8]

$${\kappa }_4(x,y,z)=x^2+y^2+z^2-xyz-4.$$

Both surfaces ${\kappa }_4$ and ${\kappa }_2$ are shown in Figure 1.

Surfaces ${\kappa }_2$ and ${\kappa }_4$ have been obtained from two different mathematical concepts, from topological and algebraic concepts in dimension two, respectively. To relate them, one makes use of the Dehn–Nielsen–Baer theorem applied to the once-punctured torus [24]. According to this theorem, for a surface of genus $g\ge 1$, we have

$${\mathrm{Mod}}^{\pm }\left(S_g\right)\cong \mathrm{Out}\left({\pi }_1\left(S_g\right)\right),$$

where the mapping class group $\mathrm{Mod}\left(S\right)$ denotes the group of isotopy classes of orientation-preserving diffeomorphisms of S (that restrict to the identity on the boundary $\partial S$ if $\partial S\ne Ø$), the extended mapping class group ${\mathrm{Mod}}^{\pm }\left(S\right)$ denotes the group of isotopy classes of all homeomorphisms of S (including the orientation-reversing ones) and $\mathrm{Out}\left({\pi }_1\right)$ denotes the outer automorphism group of ${\pi }_1\left(S\right)$. This leads to the (topological) action of ${\mathrm{Mod}}^{\pm }$ on the punctured torus as follows:

$${\mathrm{Mod}}^{\pm }\left(S_{1,1}\right)=\mathrm{Out}\left(F_2\right)=GL(2,\mathbb{Z}).$$

(1)

The automorphism group $\mathrm{Aut}\left(F_2\right)$ acts by composition on the representations $\rho$ and induces an action of the extended mapping class group ${\mathrm{Mod}}^{\pm }$ on the character variety by polynomial diffeomorphisms of the surface ${\kappa }_d$ defined by [25]

$${\kappa }_d(x,y,z)=xyz-x^2-y^2-z^2+d.$$

(2)

3.2. The Surface ${\kappa }_2$, the Link $L7a4=7_3^2$ and Fricke Topological Qubits

The surface ${\kappa }_2$ corresponds to representations $\rho :{\pi }_1\left({\kappa }_2\right)\to SL(2,\mathbb{C})$ of the group ([25] Section 4.2)

$${\pi }_1\left({\kappa }_2\right)=〈{a,b|[a,b]}^4〉.$$

(3)

Since the surface ${\kappa }_4(x,y,z)$ is the character variety of the Hopf link, we would also like to obtain a link whose character variety contains the surface ${\kappa }_2(x,y,z)$. Making use of the Thistlethwaite link table [26], we find that the only two-bridge link that has this property is the link $L7a4=7_3^2$; see Figure 2. Taking 0-surgery on both cusps of $L7a4$, Snappy calculates the fundamental group as

$$\begin{array}{cc}& {\pi }_1(S^3\backslash L7a4(0,1)(0,1))=\\ & 〈a,b|aBAbabAbabABaBAB,abAbaabAbabABBBBBBAb〉.\end{array}$$

The corresponding Groebner base for the character variety is

$${\kappa }_{L7a4}(x,y,z)=xyz(z^2-2){\kappa }_4(x,y,z){\kappa }_2(x,y,z),$$

whose factorization contains both surfaces ${\kappa }_4(x,y,z)$ and ${\kappa }_2(x,y,z)$.

Topological Qubits from ${\kappa }_2(x,y,z)$

Qubits are the elements of group $SU\left(2\right)$. There is an interesting connection of the group ${\pi }_1\left({\kappa }_2\right)$ in Equation (3) to $SU\left(2\right)$ representations.

According to [25] [Theorem 1.1], there exists a representation $\rho :{\pi }_1\left({\kappa }_2\right)\to SL(2,\mathbb{C})$ such that the closure of the orbit of its conjugacy class $\kappa \left(\rho \right)$ under the action of the extended mapping class group $\mathrm{Out}\left(F_2\right)$ in Equation (1) contains the whole set of $SU\left(2\right)$ representations of ${\pi }_1\left({\kappa }_2\right)$. The subset of the real surface ${\kappa }_2(x,y,z)$ consisting of $SU\left(2\right)$ representations is the unique bounded connected component of ${\kappa }_2(x,y,z)$ homeomorphic to a sphere; see Figure 1 (Right).

The bounded component is invariant under the mapping class $\Psi =\left(\begin{array}{cc}2& 1\\ 1& 1\end{array}\right)$ and there are two fixed points of the polynomial transformation $f_{\Psi }(x,y,z)=(z,yz-x,z(yz-x)-y)$ made of points $(x,x/(x-1),x)$ with irrational values $x\sim 0.52$ and $x\sim -1.1$ ([25], p. 19).

3.3. The Surface ${\kappa }_3(x;y,z)$ and the Link $6_3^2=L6a1$

We would like to obtain a link whose character variety contains the surface ${\kappa }_3(x,y,z)$. Making use of the Thistlethwaite link table [26], we find that the only two-bridge link that has this property is the link $6_3^2=L6a1$; see Figure 2. Taking 0-surgery on both cusps of $L6a1$, Snappy calculates the fundamental group as

$$\begin{array}{cc}& {\pi }_1(S^3\backslash L6a1(0,1)(0,1))=\\ & 〈a,b|abbaBAbaabAB,aBabABabaBAb〉.\\ \hfill \end{array}$$

The corresponding Groebner base for the character variety is

$${\kappa }_{L6a1}(x,y,z)=x{\kappa }_3(x,y,z)(x^2+y^2-xyz)(-x{y}^{2}z+y^3+x^{2}y+xz-2y)\ast f_9(x,y,z)$$

whose factorization contains surface ${\kappa }_3(x,y,z)$ and a ninth-order trivariate polynomial $f_9(x,y,z)$ not made explicit here.

4. The Fricke Cubic Surface and Three-Bridge Links

Our main object in this section is the four-punctured sphere $S_{4,2}$, for which, the fundamental group is the free group $F_3$ of rank three whose character variety generalizes the Fricke cubic surface (2) to the hypersurface $V_{a,b,c,d}\left(\mathbb{C}\right)$ in ${\mathbb{C}}^7$. It is shown how this hypersurface is realized in the variety of a covering of index 6 of the four-manifold ${\tilde{E}}_8$, the 0-surgery on all circles of the Borromean rings $B{R}_0$. The Okamoto–Painlevé correspondence is re-examined in terms of Dynkin diagrams of the appropriate four-manifolds.

4.1. The $SL(2,\mathbb{C})$-Character Variety for the Quadruply Punctured Sphere $S_{4,2}$

We follow the work of references [13,25,27].

The fundamental group for $S_{4,2}$ can be expressed in terms of the boundary components A, B, C and D as ${\pi }_1\left(S_{4,2}\right)=〈A,B,C,D|ABCD〉\cong F_3$.

A representation ${\pi }_1\to SL(2,\mathbb{C})$ is a quadruple

$$\alpha =\rho \left(A\right),\beta =\rho \left(B\right)\gamma =\rho \left(C\right),\delta =\rho \left(D\right)\in SL(2,\mathbb{C})\mathrm{where}\alpha \beta \gamma \delta =I.$$

Let us associate the seven traces

$$\begin{array}{cc}& a=\mathrm{tr}\left(\rho \right(\alpha \left)\right),b=tr\left(\rho \right(\beta \left)\right),c=\mathrm{tr}\left(\rho \right(\gamma \left)\right),d=\mathrm{tr}\left(\rho \right(\delta \left)\right)\\ & x=\mathrm{tr}\left(\rho \right(\alpha \beta \left)\right),y=\mathrm{tr}\left(\rho \right(\beta \gamma \left)\right),z=\mathrm{tr}\left(\rho \right(\gamma \alpha \left)\right),\end{array}$$

where a, b, c and d are boundary traces and x, y and z are traces of elements $AB$, $BC$ and $CA$ representing simple loops on $S_{4,2}$.

The character variety for $S_{4,2}$ satisfies the equation ([13] Section 5.2, [25] Section 2.1, [27] Section 3B, [28] Equation (1.9) or [29] Equation (39))

$$V_{a,b,c,d}\left(\mathbb{C}\right)=V_{a,b,c,d}(x,y,z)=x^2+y^2+z^2+xyz-{\theta }_{1}x-{\theta }_{2}y-{\theta }_{3}z-{\theta }_4=0$$

(4)

with ${\theta }_1=ab+cd$, ${\theta }_2=ad+bc$, ${\theta }_3=ac+bd$ and ${\theta }_4=4-a^2-b^2-c^2-d^2-abcd.$

4.2. A Compact Component of $SL(2,\mathbb{R})$

As shown in the previous section, for the real surface ${\kappa }_2(x,y,z)$, the compact component is made of $SU\left(2\right)$ representations.

For the real surface $V_{a,b,c,d}\left(\mathbb{R}\right)$, there exists a compact component if and only if [27], Proposition 1.4

$$\begin{array}{cc}& \Delta (a,b,c,d)=\\ & {(2(a^2+b^2+c^2+d^2)-abcd-16)}^2-(4-a^2)(4-b^2)(4-c^2)(4-d^2)>0\\ & \mathrm{and}16-abcd-2(a^2+b^2+c^2+d^2)>0\end{array}$$

(5)

When Equation (5) is satisfied and $(a,b,c,d)\in (-2,2)$, then $V_{a,b,c,d}\left(\mathbb{R}\right)$ contains a compact component made of $SL(2,\mathbb{R})$ representations. Otherwise, each element of the component is the character of an $SU\left(2\right)$ representation; see also ([23], Theorem 9.6).

The former case occurs in the following example, $a,b,c=\frac{3}{2}$ and $d=-\frac{3}{2}$, for which, the surface is $V_{a,b,c,d}=x^2+y^2+z^2+xyz-\frac{1}{16}$ ([27], p. 102). A sketch of this surface is given in Figure 1.

4.3. The $SL(2,\mathbb{C})$ Character Variety for the Manifold $\widetilde{E_8}$ and for Its Covering Manifolds

In a recent paper ([8], Section 3.2), we noticed connections between the coverings of the manifold $\widetilde{E_8}$ and the matter of topological quantum computing. The affine Coxeter–Dynkin diagram ${\tilde{E}}_8$ corresponds to the fiber $I{I}^{\ast }$ in Kodaira’s classification of minimal elliptic surfaces ([30], p. 320). Alternatively, one can see ${\tilde{E}}_8$ as the 0-surgery on the trefoil knot $3_1$. The boundary of the manifold associated to ${\tilde{E}}_8$ is the Seifert fibered toroidal manifold [31,32].

The coverings of the fundamental group ${\pi }_1(S^4\backslash 3_1(0,1))$ are fundamental groups of the manifolds in the following sequence:

$$[{\tilde{E}}_8,{\tilde{E}}_6,\{{\tilde{D}}_4,{\tilde{E}}_8\},\{{\tilde{E}}_6,{\tilde{E}}_8\},{\tilde{E}}_8,\{B{R}_0,{\tilde{D}}_4,{\tilde{E}}_6\},\{{\tilde{E}}_8\},\{{\tilde{E}}_6\},\{{\tilde{D}}_4,{\tilde{E}}_8\},{\tilde{E}}_6,\cdots ]$$

The subgroups/coverings are fundamental groups for ${\tilde{E}}_8$${\tilde{E}}_6$, ${\tilde{D}}_4$ or $B{R}_0$, where $B{R}_0$ is the manifold obtained by 0-surgery on all circles of Borromean rings.

A Groebner base for the $SL(2,\mathbb{C})$ character variety of ${\pi }_1({\tilde{E}}_8)$ is

$$z(x-z)(y-z^2+2)(y+z^2-1),$$

where the latter two factors are quadrics.

A Groebner base for the $SL(2,\mathbb{C})$ character variety of ${\pi }_1({\tilde{E}}_6)$ is

$${\kappa }_4(x,y,z)(x-y)(xy-z+1)(x^2+xy+y^2-3)f_1(x,y,z)f_2(x,y,z),$$

where ${\kappa }_4(x,y,z)$ is the $SL(2,\mathbb{C})$ character variety for the fundamental group of the Hopf link complement, $f_1(x,y,z)=x{y}^3-y^{2}z-x^2-2xy+z+2$ and $f_2(x,y,z)=y^4-x^{2}z+xy-4y^2+z+2$. A plot of the latter surfaces is in ([8], Figure 4). In the three-dimensional projective space, the two surfaces are birationally equivalent to a conic bundle and to the projective plane $P^2$, respectively. Both show a Kodaira dimension zero characteristic of $K_3$ surfaces.

A Groebner base for the $SL(2,\mathbb{C})$ character variety of ${\pi }_1({\tilde{D}}_4)$ contains the five- dimensional hypersurface

$$f(x,y,z,w,k)={\kappa }_4(x,y,z)-wxk-2k^2,$$

which is close to (but different from) the Fricke form $V_{0,0,w,k}\left(\mathbb{C}\right)={\kappa }_4(x,y,z)-wxk+w^2+k^2$.

Finally, for ${\pi }_1\left(B{R}_0\right)$, a Groebner base obtained from Magma contains 28 polynomials. However, a simpler small basis with 10 polynomials, like the size of I, is available. The ideal ring for ${\pi }_1\left(B{R}_0\right)$ is

$$\begin{array}{cc}& I=\{36f_{B{R}_0}(x,y,z,u,v,w,k),\\ & x{\kappa }_4(x,y,z),y{\kappa }_4(x,y,z),x{\kappa }_4(x,u,v),y{\kappa }_4(y,u,v),\\ & -xyk+xv+yw+zk-2u,x{u}^2-uzw+yz-uv+wk-2x,.\}\end{array}$$

(6)

where the seventh variable polynomial reads

$$f_{B{R}_0}(x,y,z,u,v,w,k)=-xyz+x^2+y^2+z^2+xyuk-{\theta }_{1}x-{\theta }_{2}y-{\theta }_3^{0}z+{\theta }_4^0$$

and ${\theta }_1=uv+wk$, ${\theta }_2=uw+vk$, ${\theta }_3^0=uk-vw$, ${\theta }_4^0=u^2+v^2+w^2+k^2-4$.

Taking the new variable $z^{\prime }=-z+k$, the polynomial $f_{B{R}_0}$ transforms into the Fricke form (4).

$$V_{u,v,w,k}(x,y,z)=xyz+x^2+y^2+z^2-{\theta }_{1}x-{\theta }_{2}x-{\theta }_{3}z+{\theta }_4,$$

(7)

with ${\theta }_3=uk+vw$ and ${\theta }_4={\theta }_4^0+uvwk$.

The missing term in (6) is a fifth-order polynomial.

4.4. Painlevé VI and the Riemann-Hilbert Correspondence

Equation (7) corresponds to a four-punctured sphere with four singular points and a monodromy group ${\pi }_1$ isomorphic to the free group on three-generators. The existence of a certain class of linear differential equations with such singular points and a monodromy group is known as Hilbert’s twenty first problem, the original setting of Riemann–Hilbert correspondence. For the present case of the four-punctured sphere, the searched differential (dynamical) equation is the sixth Painlevé equation (or Painlevé VI) [23]

$$\begin{array}{cc}& q_{tt}=\frac{1}{2}(\frac{1}{q}+\frac{1}{q-1}+\frac{1}{q-t})q_t^2-(\frac{1}{t}+\frac{1}{t-1}+\frac{1}{q-t})q_t\\ & +\frac{q(q-1)(q-t)}{2t^2{(t-1)}^2}\{{\alpha }_4^2-{\alpha }_1^2\frac{t}{q_2}+{\alpha }_2^2\frac{t-1}{{(q-1)}^2}+(1-{\alpha }_3^2)\frac{t(t-1)}{{(q-t)}^2}\}\end{array}$$

(8)

with complex parameters ${\alpha }_1,{\alpha }_2,{\alpha }_3,{\alpha }_4$. The Painlevé property is the absence of movable singular points. The essential singularities of all solutions $q\left(t\right)$ of Equation (8) only appear when $t\in \{0,1,\infty \}$.

Analyzing the nonlinear monodromy of Painlevé VI leads to the relation between parameters a, b, c and d of the family of cubic surfaces $V_{a,b,c,d}(x,y,z)$ given in (7) and parameters ${\alpha }_i$, $i=1\dots 4$ of Painlevé VI equation ([29], Section 4.2):

$$(a,b,c,d)=[2cos\left(\pi {\alpha }_1\right),2cos\left(\pi {\alpha }_2\right),2cos\left(\pi {\alpha }_3\right),-2cos\left(\pi {\alpha }_4\right)].$$

(9)

The relation between the two classes of parameters has been found to be controlled by the so-called Okamoto–Painlevé pairs. The Painlevé equation corresponding to ${\tilde{E}}_8$ is Painlevé I, the Painlevé equation corresponding to ${\tilde{E}}_7$ is Painlevé II, the Painlevé equation corresponding to ${\tilde{E}}_6$ is Painlevé IV and the Painlevé equation corresponding to ${\tilde{D}}_4$ is Painlevé VI ([33] Table 1, [23] Section 9.1.2). These mathematical results fit our approach developed in the previous subsection.

Incidentally, the Painlevé equation corresponding to the manifold ${\tilde{D}}_5$ is Painlevé V. We find that the Groebner base for the $SL(2,\mathbb{C})$ character variety of ${\pi }_1({\tilde{D}}_5)$ contains the surface ${\kappa }_d(x,y,z)$ defined in Equation (2) (apart from trivial quadratic factors).

Finally, Painlevé III corresponds to one of the three types ${\tilde{D}}_6$, ${\tilde{D}}_7$ or ${\tilde{D}}_8$. We find that, for ${\tilde{E}}_7$ and ${\tilde{D}}_7$, the character variety is trivial (up to quadratic factors), for ${\tilde{D}}_6$, it is of type ${\kappa }_d(x,y,z)$ and, for ${\tilde{D}}_8$, it is close (but different from the form $V_{0,0,c,d}\left(\mathbb{C}\right)$, as for ${\tilde{D}}_4$ investigated in Section 4.3.

The Okamoto–Painlevé correspondence and the type of main factor in the related Groebner base is summarized in

Table 1. The manifold type according to the Dynkin diagram (row 1 ), the corresponding Painlevé equation (row 2) and the main factor in the Groebner base for the corresponding $SL(2,\mathbb{C})$ variety. The symbol T means that the variety is trivial (up to quadratic factors).

manifold	${\tilde{E}}_8$	${\tilde{E}}_7$	${\tilde{D}}_8$	${\tilde{D}}_7$	${\tilde{D}}_6$	${\tilde{E}}_6$	${\tilde{D}}_5$	${\tilde{D}}_4$
Painlevé type	$P_I$	$P_{II}$	$P_{III}^{{\tilde{D}}_8}$	$P_{III}^{{\tilde{D}}_7}$	$P_{III}^{{\tilde{D}}_6}$	$P_{IV}$	$P_V$	$P_{VI}$
char var	T	T	≈$V_{o,o,c,d}$	T	${\kappa }_d$	${\kappa }_4$	${\kappa }_d$	≈$V_{o,o,c,d}$

.

5. Discussion and Conclusions

In this paper, using the $SL(2,\mathbb{C})$ character variety of the punctured torus $S_{1,1}$ and of the quadruply punctured sphere $S_{4,2}$, we focused on the interest in defining topological qubits from the cubic surface ${\kappa }_d(x,y,z)$ in (2) or $V_{a,b,c,d}(x,y,z)$ in (4) in the compact bounded domain of real variables x, y and z. We explored the connection of such real surfaces to the character variety of some two- and three-bridge links. We pointed out their relationship to Painlevé VI transcendents through Okamoto Equation (9). While possible experimental directions remain open for further investigation, recent advances in the field are noteworthy [34,35,36,37].

Let us now add that there exists a link between Painlevé transcendents and Einstein’s equations of cosmology when the metric is chosen to be self-dual. The six Painlevé equations are ‘essentially’ equivalent to $SL(2,\mathbb{C})$ self-dual Yang–Mills equations with appropriate three-dimensional Abelian groups of conformal symmetries [38]. The symmetry groups are taken to be groups of conformal transformations of the complex Minkowski space–time with the metric

$$d{s}^2=d\tau d\overline{\tau }-d\xi d\overline{\xi }.$$

For Painlevé VI, the Higgs fields $P_i=\Phi \left(X_i\right)$, $i=0$, 1, t are $sl(2,\mathbb{C})$ valued functions of the time variable $t=\frac{\xi \overline{\xi }}{\tau \overline{\tau }}$. The self-dual equations

$$S^{\prime }=0,t{P}_0^{\prime }+[P_0,P_t],(t-1)P_1^{\prime }+[P_1,P_t]=0,$$

with $S=-(P_0+P_1+P_t)$ are equivalent to Painlevé VI with parameters calculated from the constant determinants of the $P_i$ and S ([38], p. 573). As a result, the Fricke surfaces that we investigated in this paper correspond to relevant solutions of self-dual Einstein’s equations.