Violation of Bell-CHSH Inequalities through Optimal Local Filters in the Vacuum
Abstract
1. Introduction
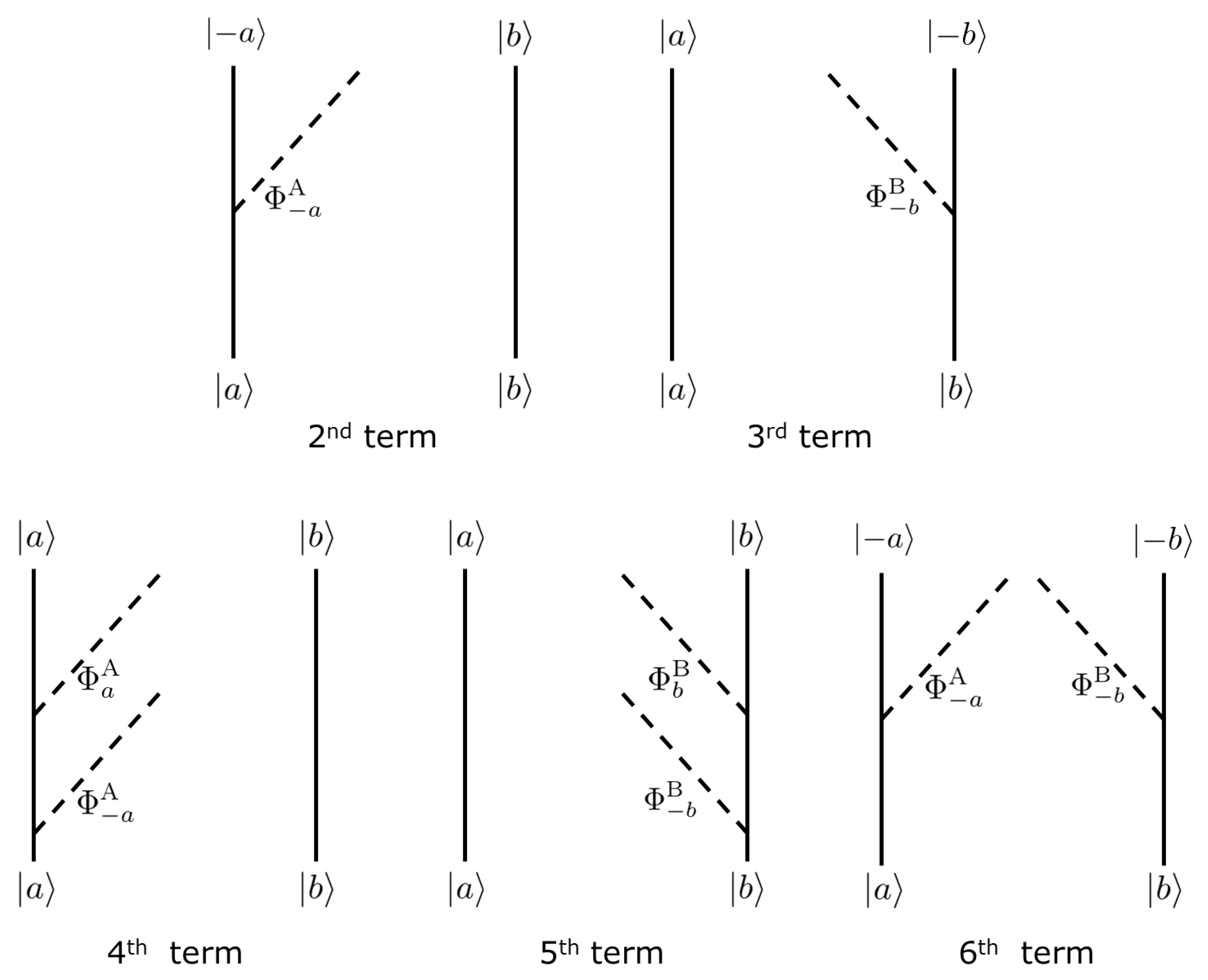
2. Perturbative Dynamics of Two Detectors Coupled to Scalar Field
3. Negativity and Bell-CHSH Inequality for X State
4. Local Filtering Operation for X States
4.1. Key Theorems
4.2. Quantum Correlation of the Bell Diagonal State and Coherence of X State
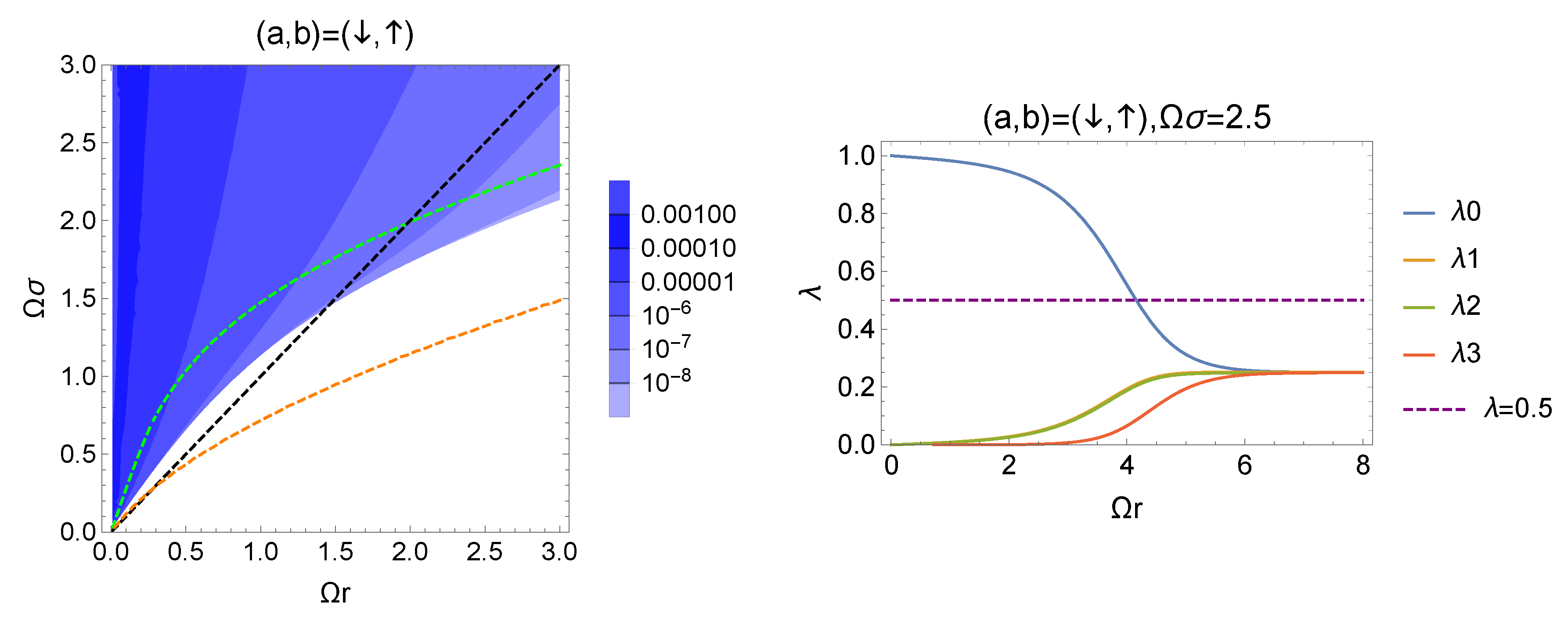
5. Violation of Bell-CHSH Inequality by Using Optimal Local Filtering
5.1. The Initial Condition
5.2. The Initial Condition
5.3. The Initial Condition
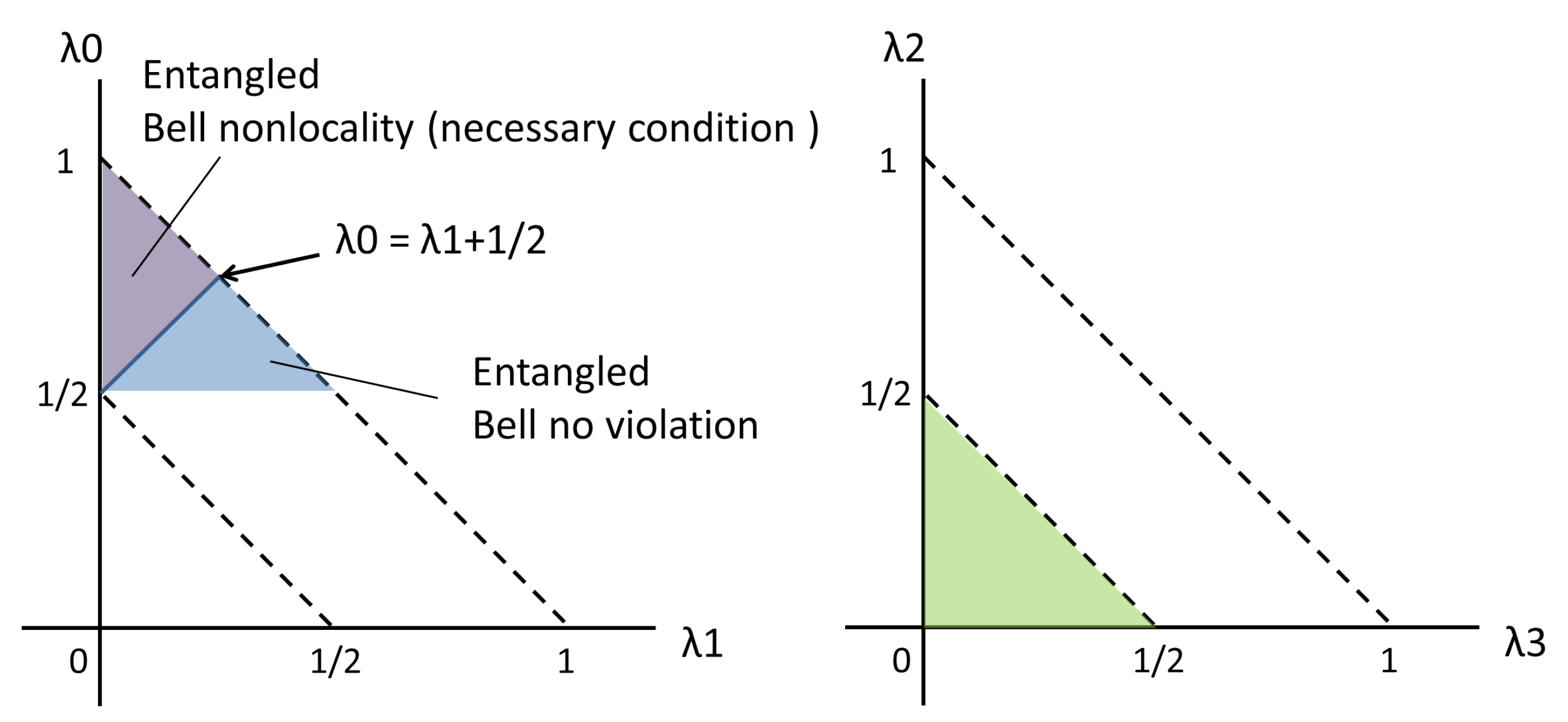
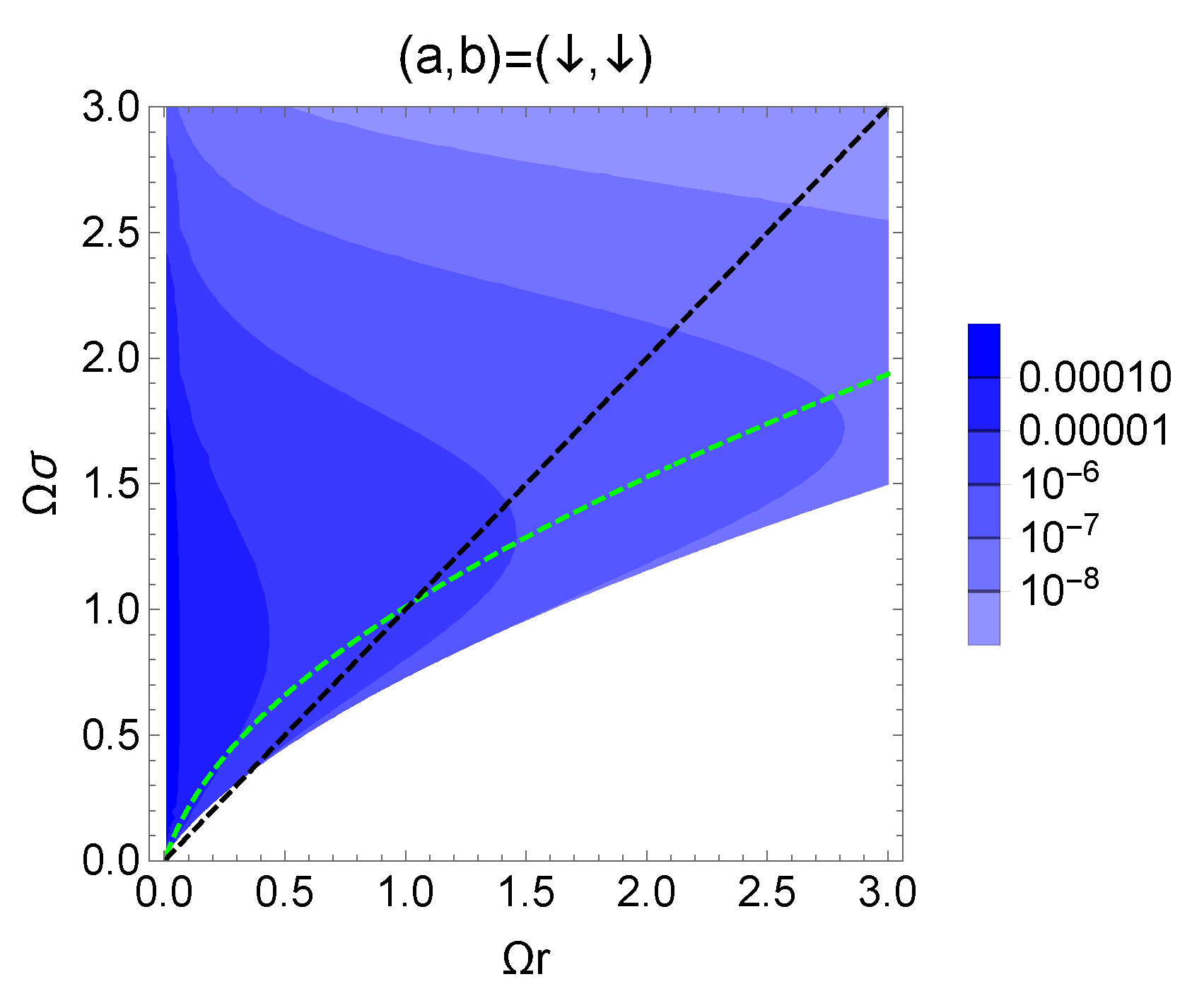
6. Effect of Local Emissions for the Detection Region of Bell Nolocality and Its Success Probability
7. Summary and Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A. Components of Reduced Density Matrix
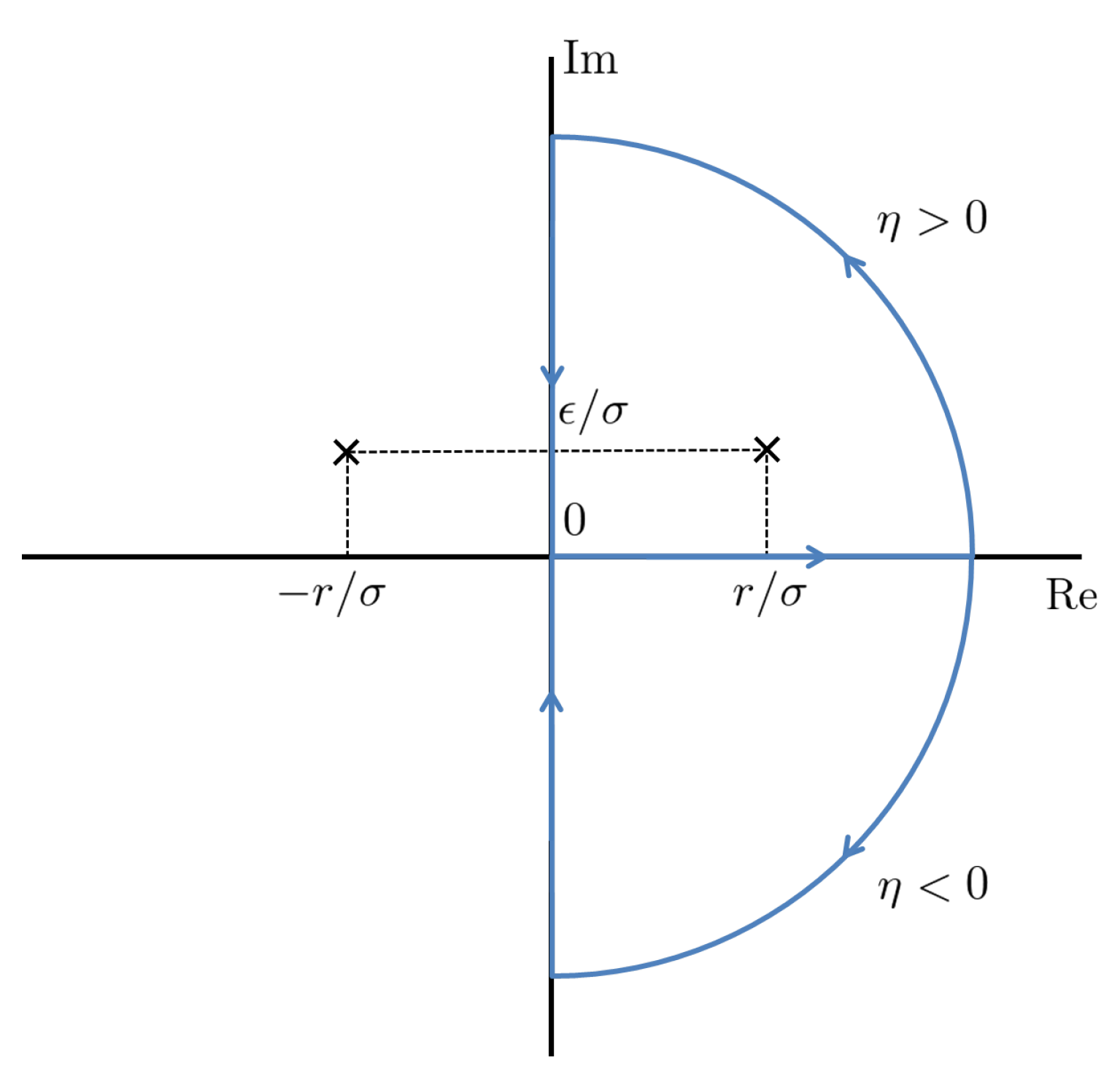
Appendix B. Equality of |X(−1,−1)| and |X(+1,+1)|
References
- Nielsen, M.A.; Chuang, I.L. Quantum Computation and Quantum Information; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Bell, J. On the Einstein Podolsky Rosen paradox. Physics 1964, 1, 195–200. [Google Scholar] [CrossRef]
- Clauser, J.; Horne, M.; Shimony, A.; Holt, R. Proposed Experiment to Test Local Hidden-Variable Theories. Phys. Rev. Lett. 1969, 23, 880–884. [Google Scholar] [CrossRef]
- Werner, R. Quantum states with Einstein-Podolsky-Rosen correlations admitting a hidden-variable model. Phys. Rev. A 1989, 40, 4277. [Google Scholar] [CrossRef] [PubMed]
- Unruh, W.G. Notes on black-hole evaporation. Phys. Rev. D 1976, 14, 870. [Google Scholar] [CrossRef]
- Reeh, H.; Schlieder, S. Bemerkungen zur unitäräquivalenz von lorentzinvarianten feldern. Nuovo Cimento 1961, 22, 1051. [Google Scholar] [CrossRef]
- Summers, S.J.; Werner, R. The vacuum violates Bell’s inequalities. Phys. Lett. A 1985, 110, 257. [Google Scholar] [CrossRef]
- Lin, S.-Y.; Hu, B.L. Accelerated detector-quantum field correlations: From vacuum fluctuations to radiation flux. Phys. Rev. D 2006, 73, 124018. [Google Scholar] [CrossRef]
- Brown, E.G.; Martin-Martinez, E.; Menicucci, N.C.; Mann, R.B. Detectors for probing relativistic quantum physics beyond perturbation theory. Phys. Rev. D 2013, 87, 084062. [Google Scholar] [CrossRef]
- Brown, E.G. Thermal amplification of field-correlation harvesting. Phys. Rev. A 2013, 88, 062336. [Google Scholar] [CrossRef]
- Reznik, B.; Retzker, A.; Silman, J. Violating Bell’s inequalities in vacuum. Phys. Rev. A 2005, 71, 042104. [Google Scholar] [CrossRef]
- Retzker, A.; Cirac, J.I.; Reznik, B. Detecting Vacuum Entanglement in a Linear Ion Trap. Phys. Rev. Lett. 2005, 94, 050504. [Google Scholar] [CrossRef] [PubMed]
- Silman, J.; Reznik, B. Long-range entanglement in the Dirac vacuum. Phys. Rev. A 2007, 75, 052307. [Google Scholar] [CrossRef]
- Leon, J.; Sabin, C. Entanglement swapping between spacelike-separated atoms. Phys. Rev. A 2008, 78, 052314. [Google Scholar] [CrossRef]
- Pozas-Kerstjens, A.; Martin-Martinez, E. Harvesting correlations from the quantum vacuum. Phys. Rev. D 2015, 92, 064042. [Google Scholar] [CrossRef]
- Salton, G.; Mann, R.B.; Menicucci, N.C. Acceleration-assisted entanglement harvesting and rangefinding. New J. Phys. 2015, 17, 035001. [Google Scholar] [CrossRef]
- Sachs, A.; Mann, R.B.; Martin-Martinez, E. Entanglement harvesting and divergences in quadratic Unruh-DeWitt detector pairs. Phys. Rev. D 2017, 96, 085012. [Google Scholar] [CrossRef]
- Pozas-Kerstjens, A.; Louko, J.; Martin-Martinez, E. Degenerate detectors are unable to harvest spacelike entanglement. Phys. Rev. D 2017, 95, 105009. [Google Scholar] [CrossRef]
- Gisin, N. Hidden quantum nonlocality revealed by local filters. Phys. Lett. A 1996, 210, 151. [Google Scholar] [CrossRef]
- Nambu, Y.; Ohsumi, Y. Classical and quantum correlations of scalar field in the inflationary universe. Phys. Rev. D 2011, 84, 044028. [Google Scholar] [CrossRef]
- Verstraete, F.; Dehaene, J.; DeMoor, B. Local filtering operations on two qubits. Phys. Rev. A 2001, 64, 010101. [Google Scholar] [CrossRef]
- Verstraete, F.; Wolf, M.M. Entanglement versus Bell Violations and Their Behavior under Local Filtering Operations. Phys. Rev. Lett. 2002, 89, 170401. [Google Scholar] [CrossRef]
- Vidal, G.; Werner, R. Computable measure of entanglement. Phys. Rev. A 2002, 65, 032314. [Google Scholar] [CrossRef]
- Horodecki, M.; Horodecki, R.; Horodecki, P. Separability of mixed states: Necessary and sufficient conditions. Phys. Lett. A 1996, 223, 1–8. [Google Scholar] [CrossRef]
- Tsirelson, B. Quantum generalizations of Bell’s inequality. Lett. Math. Phys. 1980, 4, 93. [Google Scholar] [CrossRef]
- Horodecki, R.; Horodecki, P.; Horodecki, M. Violating Bell inequality by mixed spin-1/2 states: Necessary and sufficient condition. Phys. Lett. A 1995, 200, 340. [Google Scholar] [CrossRef]

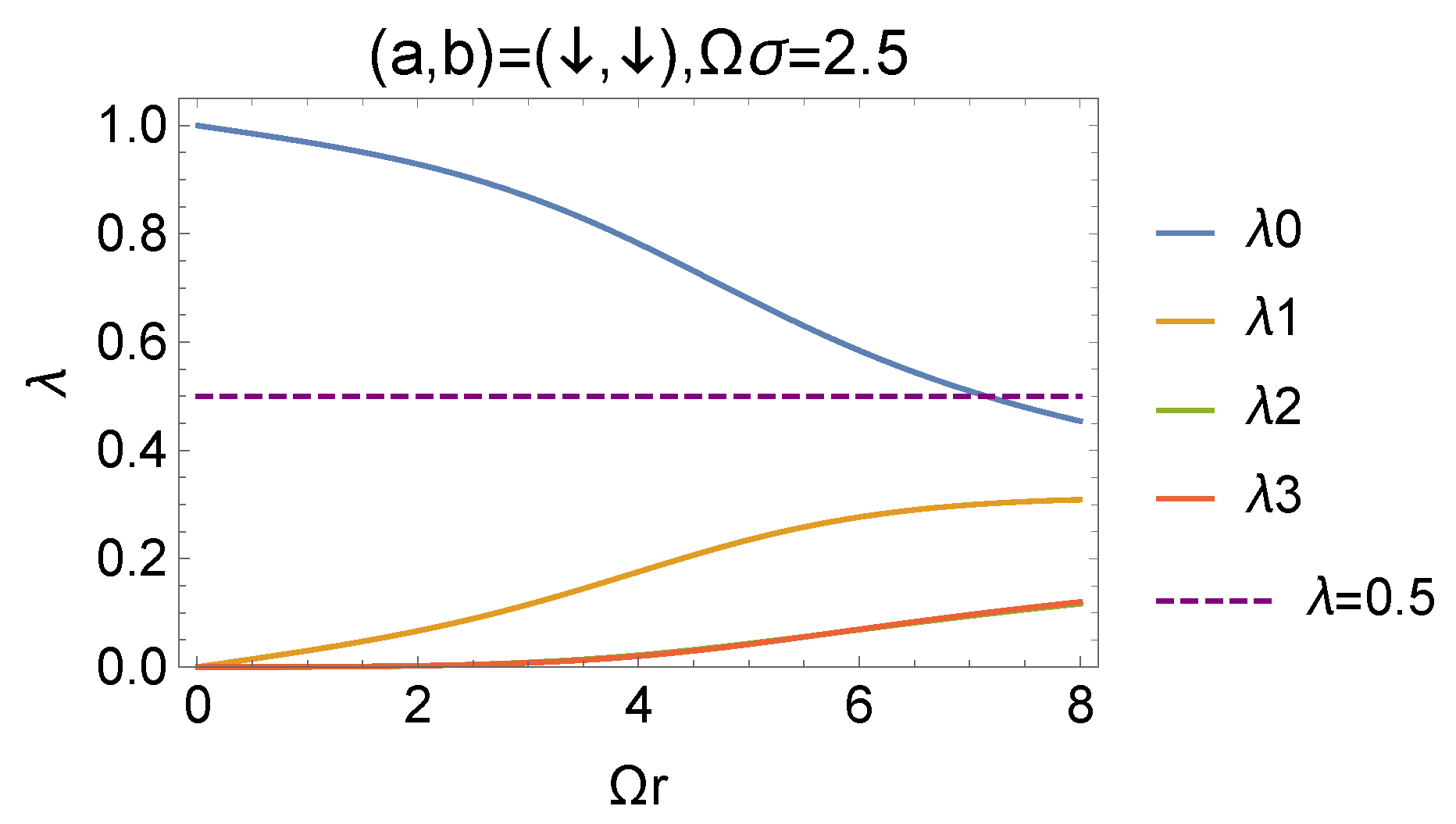
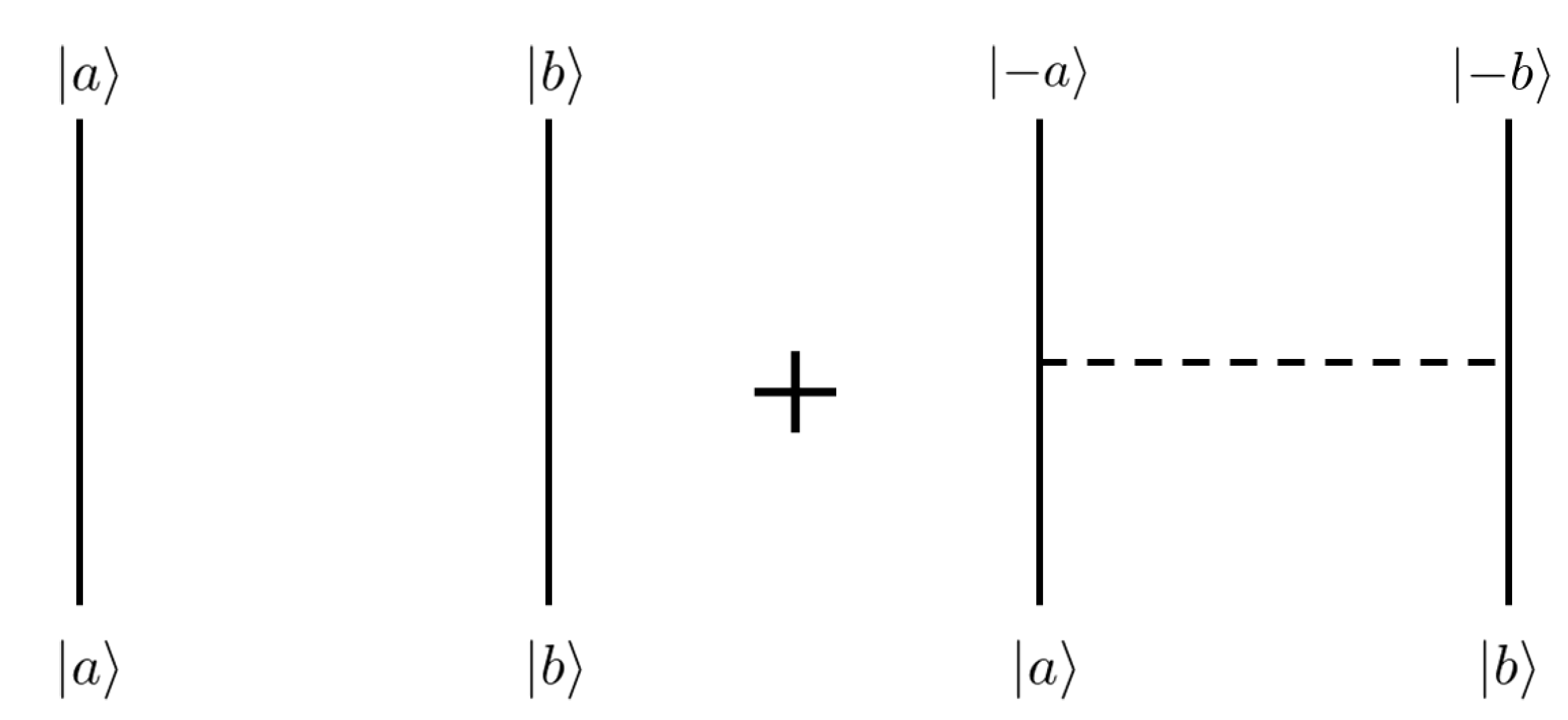


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Matsumura, A.; Nambu, Y. Violation of Bell-CHSH Inequalities through Optimal Local Filters in the Vacuum. Quantum Rep. 2020, 2, 542-559. https://doi.org/10.3390/quantum2040038
Matsumura A, Nambu Y. Violation of Bell-CHSH Inequalities through Optimal Local Filters in the Vacuum. Quantum Reports. 2020; 2(4):542-559. https://doi.org/10.3390/quantum2040038
Chicago/Turabian StyleMatsumura, Akira, and Yasusada Nambu. 2020. "Violation of Bell-CHSH Inequalities through Optimal Local Filters in the Vacuum" Quantum Reports 2, no. 4: 542-559. https://doi.org/10.3390/quantum2040038
APA StyleMatsumura, A., & Nambu, Y. (2020). Violation of Bell-CHSH Inequalities through Optimal Local Filters in the Vacuum. Quantum Reports, 2(4), 542-559. https://doi.org/10.3390/quantum2040038




