Design of Fast Charging Station with Energy Management for eBuses
Abstract
:1. Introduction
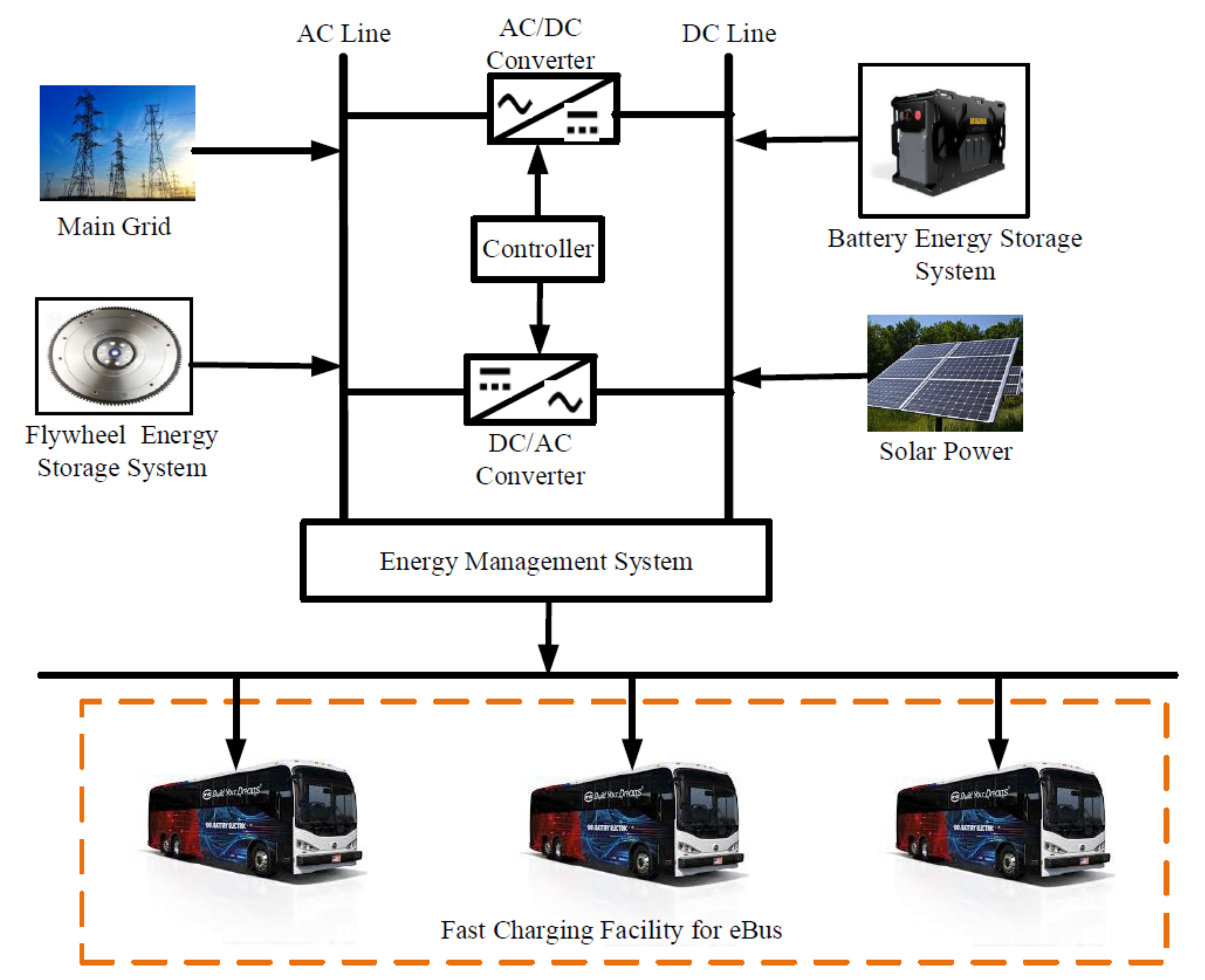
2. System Configuration
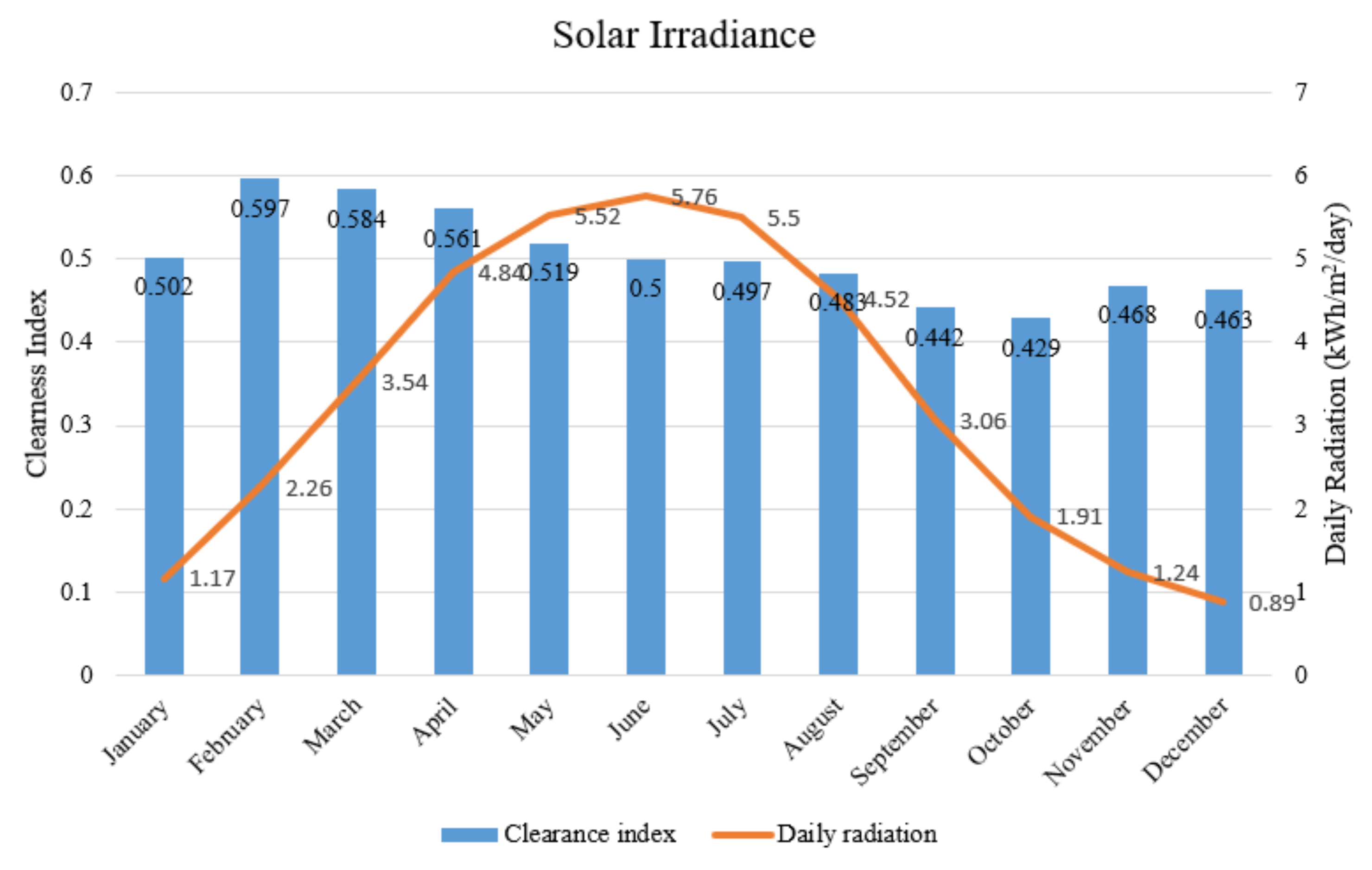
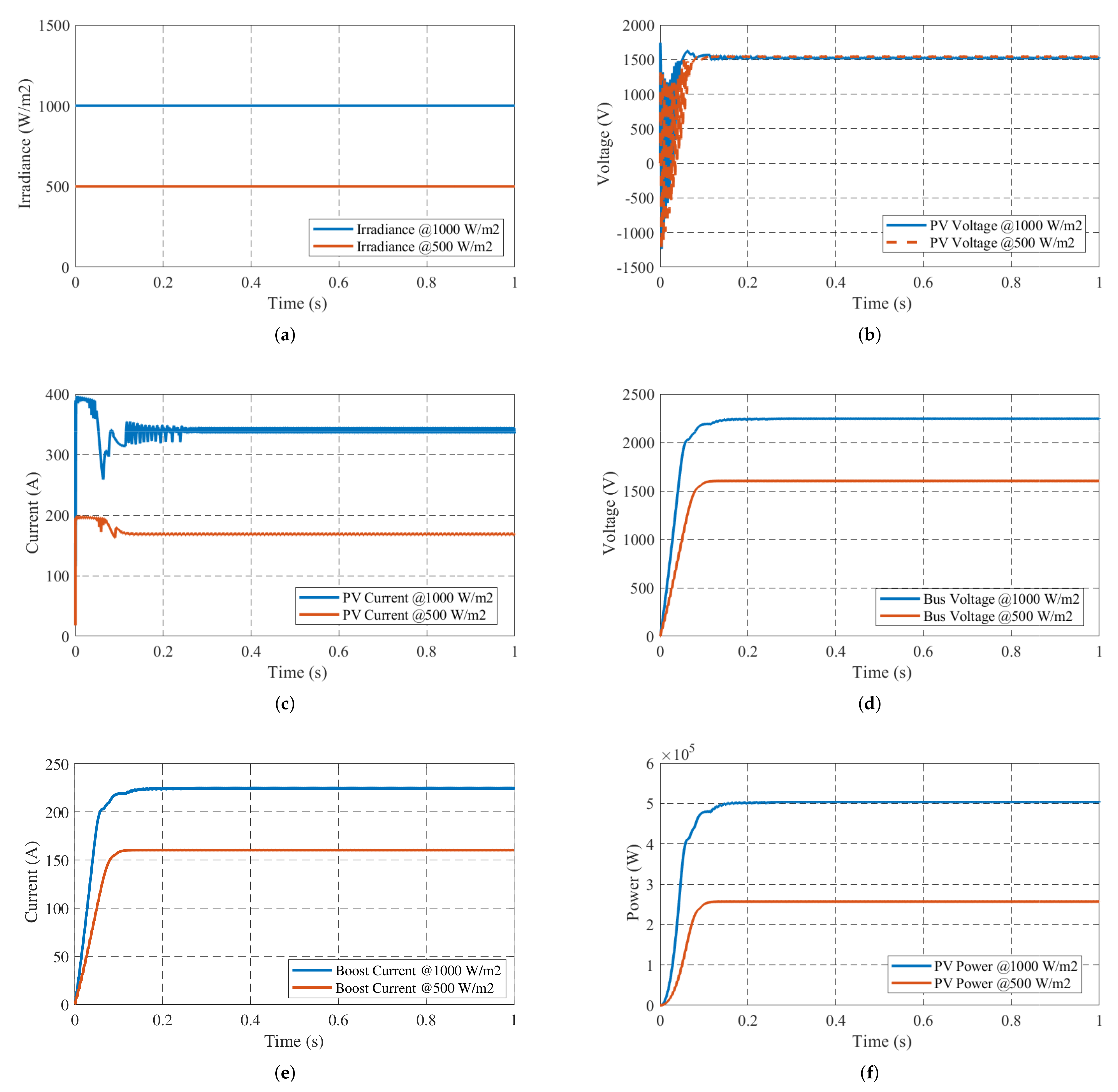
2.1. Solar Power
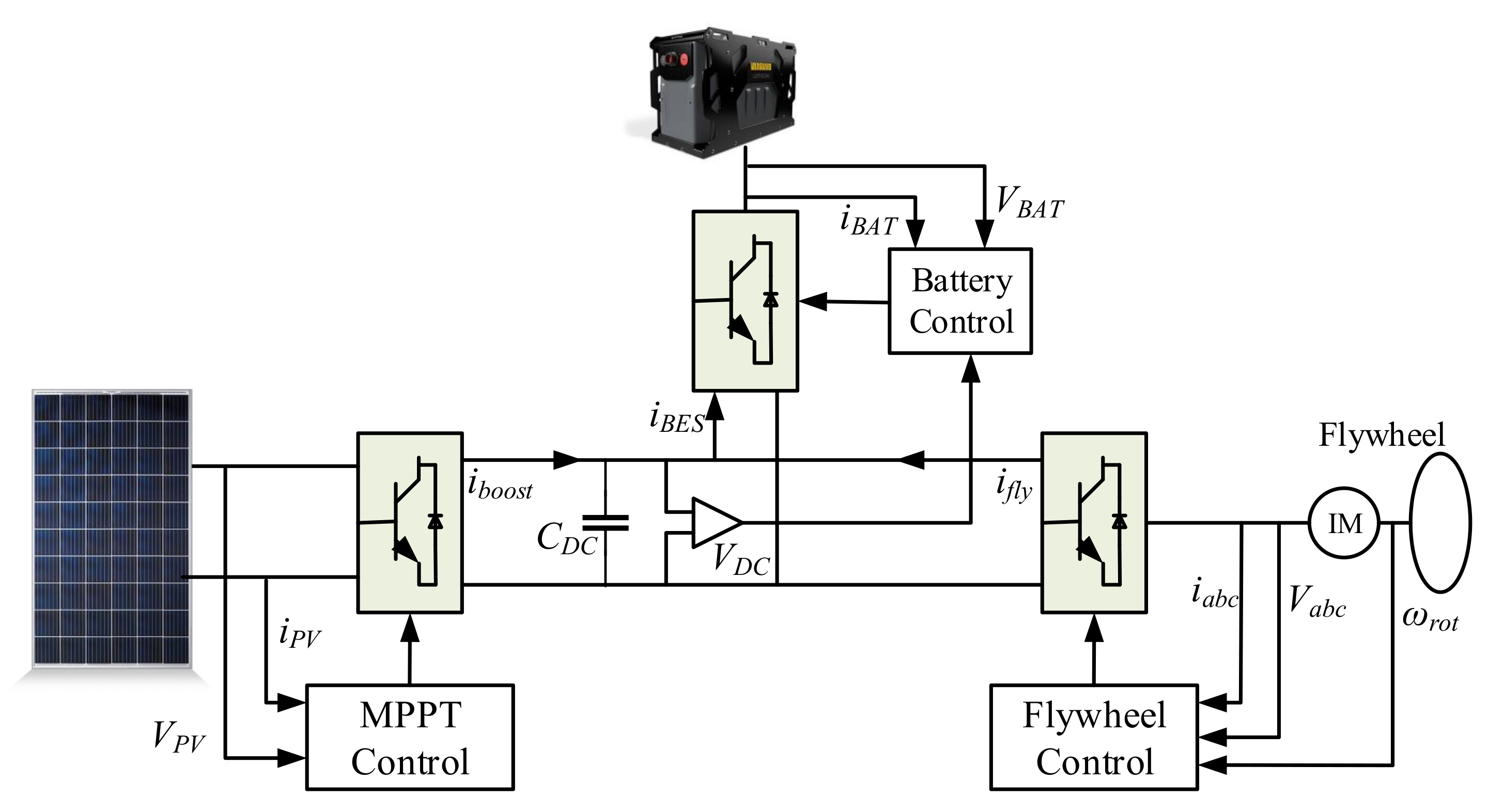
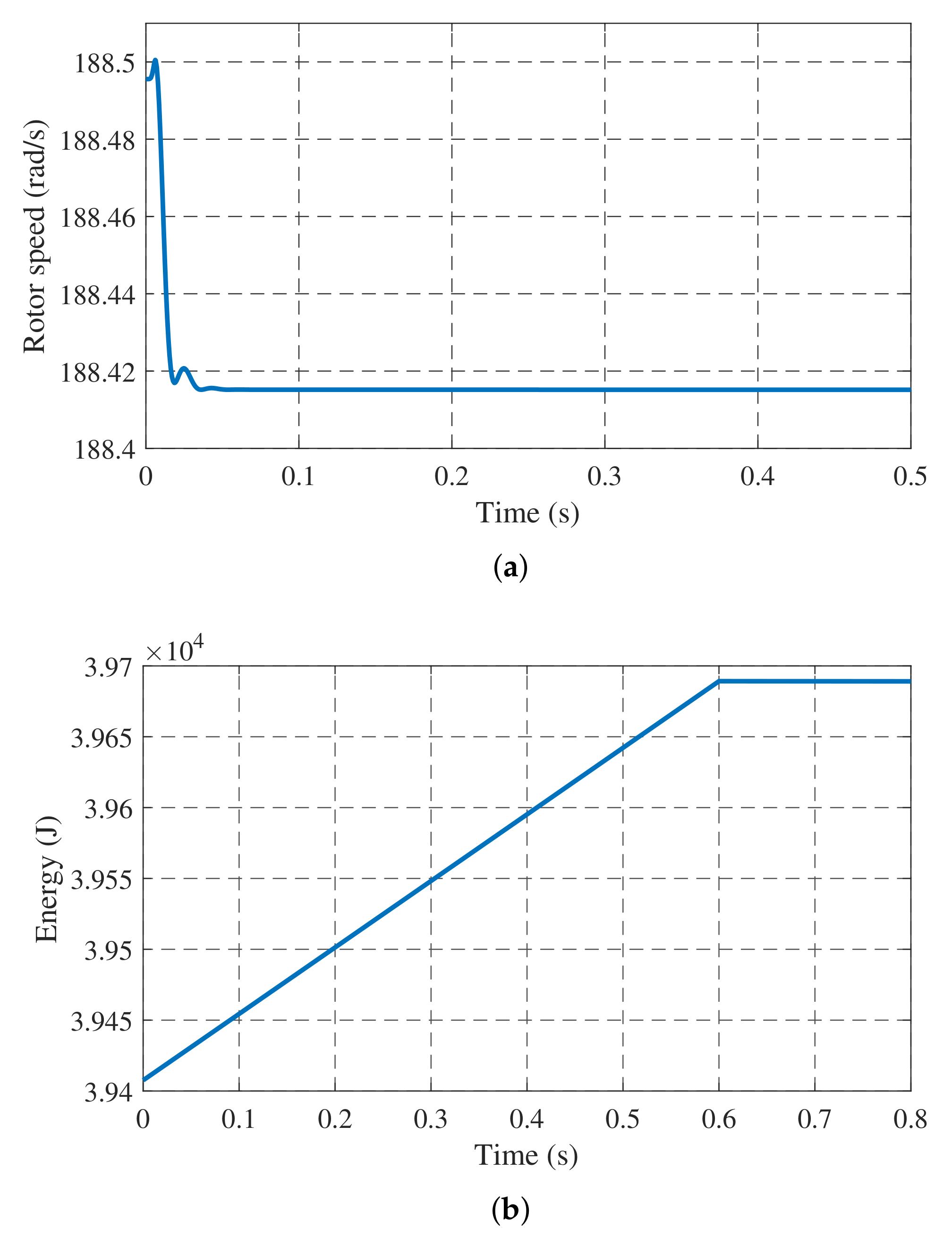
2.2. Flywheel Storage System
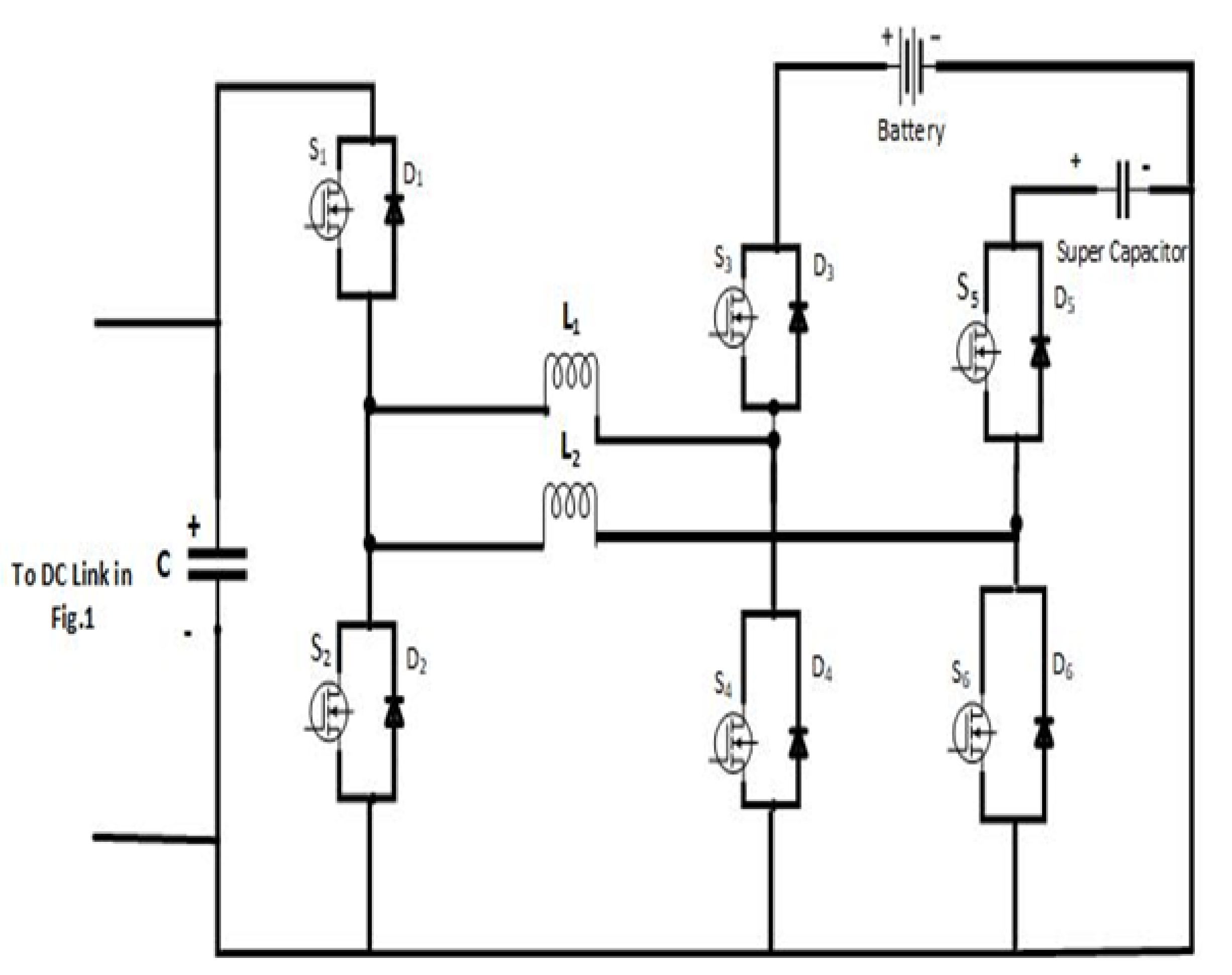
2.3. Multi Input Bidirectional DC/DC Converter
3. Control Strategy for Energy Management System
3.1. Design of MRAC
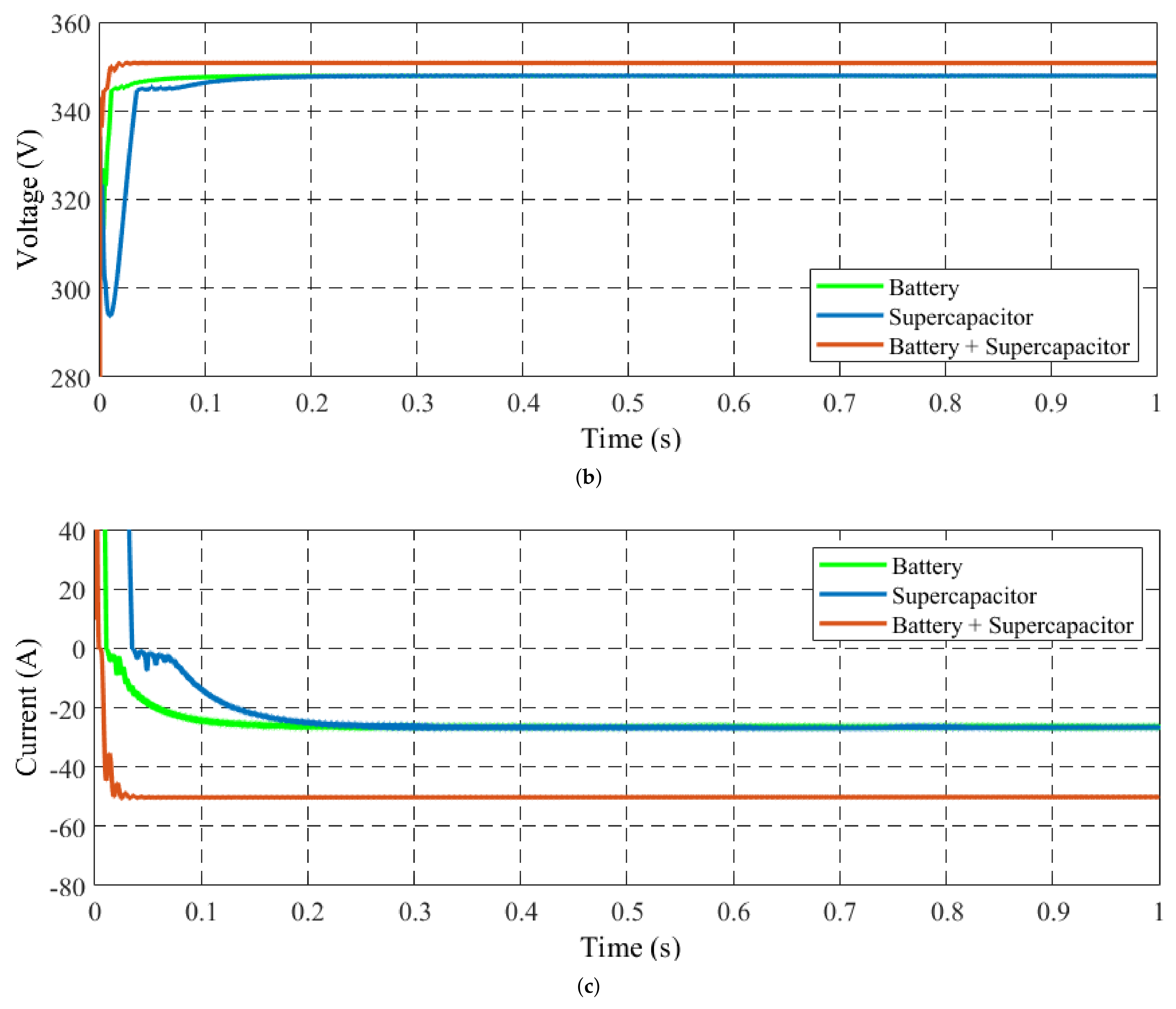
3.2. Converter Control
4. Performance Evaluation
4.1. Solar Power Output
4.2. Flywheel Performance
4.3. Proposed DC/DC Converter Performance
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Tie, S.F.; Tan, C.W. A review of energy sources and energy management system in electric vehicles. Renew. Sustain. Energy Rev. 2013, 20, 82–102. [Google Scholar] [CrossRef]
- Clarke, A.D.; Makram, E.B. An Innovative Approach in Balancing Real Power Using Plug in Hybrid Electric Vehicles. J. Power Energy Eng. 2014, 2, 1. [Google Scholar] [CrossRef] [Green Version]
- Clement-Nyns, K.; Haesen, E.; Driesen, J. The impact of charging plug-in hybrid electric vehicles on a residential distribution grid. IEEE Trans. Power Syst. 2009, 25, 371–380. [Google Scholar] [CrossRef] [Green Version]
- Yilmaz, M.; Krein, P.T. Review of battery charger topologies, charging power levels, and infrastructure for plug-in electric and hybrid vehicles. IEEE Trans. Power Electron. 2012, 28, 2151–2169. [Google Scholar] [CrossRef]
- Abdussami, M.R.; Gabbar, H.A. Nuclear-Powered Hybrid Energy Storage-Based Fast Charging Station for Electrification Transportation. In Proceedings of the 2019 IEEE 7th International Conference on Smart Energy Grid Engineering (SEGE), Oshawa, ON, Canada, 12–14 August 2019; pp. 304–308. [Google Scholar]
- Xu, Z.; Hu, Z.; Song, Y.; Luo, Z.; Zhan, K.; Wu, J. Coordinated charging strategy for PEVs charging stations. In Proceedings of the 2012 IEEE Power and Energy Society General Meeting, San Diego, CA, USA, 22–26 July 2012; pp. 1–8. [Google Scholar]
- Cao, Y.; Tang, S.; Li, C.; Zhang, P.; Tan, Y.; Zhang, Z.; Li, J. An optimized EV charging model considering TOU price and SOC curve. IEEE Trans. Smart Grid 2011, 3, 388–393. [Google Scholar] [CrossRef]
- Fazelpour, F.; Vafaeipour, M.; Rahbari, O.; Rosen, M.A. Intelligent optimization to integrate a plug-in hybrid electric vehicle smart parking lot with renewable energy resources and enhance grid characteristics. Energy Convers. Manag. 2014, 77, 250–261. [Google Scholar] [CrossRef]
- Zhang, P.; Qian, K.; Zhou, C.; Stewart, B.G.; Hepburn, D.M. A methodology for optimization of power systems demand due to electric vehicle charging load. IEEE Trans. Power Syst. 2012, 27, 1628–1636. [Google Scholar] [CrossRef]
- Vermaak, H.J.; Kusakana, K. Design of a photovoltaic–wind charging station for small electric Tuk–tuk in DR Congo. Renew. Energy 2014, 67, 40–45. [Google Scholar] [CrossRef]
- Fathabadi, H. Novel wind powered electric vehicle charging station with vehicle-to-grid (V2G) connection capability. Energy Convers. Manag. 2017, 136, 229–239. [Google Scholar] [CrossRef]
- Hutson, C.; Venayagamoorthy, G.K.; Corzine, K.A. Intelligent scheduling of hybrid and electric vehicle storage capacity in a parking lot for profit maximization in grid power transactions. In Proceedings of the 2008 IEEE Energy 2030 Conference, Atlanta, GA, USA, 17–18 November 2008; pp. 1–8. [Google Scholar]
- Sortomme, E.; El-Sharkawi, M.A. Optimal charging strategies for unidirectional vehicle-to-grid. IEEE Trans. Smart Grid 2010, 2, 131–138. [Google Scholar] [CrossRef]
- Aswantara, I.K.A.; Ko, K.S.; Sung, D.K. A centralized EV charging scheme based on user satisfaction fairness and cost. In Proceedings of the 2013 IEEE Innovative Smart Grid Technologies-Asia (ISGT Asia), Bangalore, India, 10–13 November 2013; pp. 1–4. [Google Scholar]
- Acha, S.; Green, T.C.; Shah, N. Effects of optimised plug-in hybrid vehicle charging strategies on electric distribution network losses. In Proceedings of the IEEE PES T&D 2010, New Orleans, LA, USA, 19–22 April 2010; pp. 1–6. [Google Scholar]
- Huang, J.; Gupta, V.; Huang, Y.F. Scheduling algorithms for PHEV charging in shared parking lots. In Proceedings of the 2012 American Control Conference (ACC), Montreal, QC, Canada, 27–29 June 2012; pp. 276–281. [Google Scholar]
- Vaya, M.G.; Andersson, G. Centralized and decentralized approaches to smart charging of plug-in vehicles. In Proceedings of the 2012 IEEE Power and Energy Society General Meeting, San Diego, CA, USA, 22–26 July 2012; pp. 1–8. [Google Scholar]
- Torreglosa, J.P.; García-Triviño, P.; Fernández-Ramirez, L.M.; Jurado, F. Decentralized energy management strategy based on predictive controllers for a medium voltage direct current photovoltaic electric vehicle charging station. Energy Convers. Manag. 2016, 108, 1–13. [Google Scholar] [CrossRef]
- García-Triviño, P.; Torreglosa, J.P.; Fernández-Ramírez, L.M.; Jurado, F. Control and operation of power sources in a medium-voltage direct-current microgrid for an electric vehicle fast charging station with a photovoltaic and a battery energy storage system. Energy 2016, 115, 38–48. [Google Scholar] [CrossRef]
- González-Rivera, E.; Sarrias-Mena, R.; García-Triviño, P.; Fernández-Ramírez, L.M. Predictive energy management for a wind turbine with hybrid energy storage system. Int. J. Energy Res. 2020, 44, 2316–2331. [Google Scholar] [CrossRef]
- Zheng, Y.; Song, Y.; Hill, D.J.; Meng, K. Online distributed MPC-based optimal scheduling for EV charging stations in distribution systems. IEEE Trans. Ind. Inform. 2018, 15, 638–649. [Google Scholar] [CrossRef]
- Zheng, H.; Wu, J.; Wu, W.; Wang, Y. Integrated motion and powertrain predictive control of intelligent fuel cell/battery hybrid vehicles. IEEE Trans. Ind. Inform. 2019, 16, 3397–3406. [Google Scholar] [CrossRef]
- Gabbar, H.A.; Adham, M.I.; Abdussami, M.R. Analysis of nuclear-renewable hybrid energy system for marine ships. Energy Rep. 2021, 7, 2398–2417. [Google Scholar] [CrossRef]
- Dragičević, T.; Sučić, S.; Vasquez, J.C.; Guerrero, J.M. Flywheel-based distributed bus signalling strategy for the public fast charging station. IEEE Trans. Smart Grid 2014, 5, 2825–2835. [Google Scholar] [CrossRef] [Green Version]
- Jain, P.; Nigam, M. Design of a model reference adaptive controller using modified MIT rule for a second order system. Adv. Electron. Electr. Eng. 2013, 3, 477–484. [Google Scholar]




| Maximum Power | Open Circuit Voltage, | Voltage at Maximum Power Point, | Short Circuit Current, | Current at Maximum Power Point, | Cells per Module |
|---|---|---|---|---|---|
| 213.15 | 36.3 | 29 | 7.84 | 7.35 | 60 |
| j | |||||||
|---|---|---|---|---|---|---|---|
| 2.2 mF | 10.46 mH | 10.76 mH | 10.76 mH | 0.0148 | 0.0093 | 0.055 | 10 |
| 1 | 2 | 3 | 4 | 5 | |
|---|---|---|---|---|---|
| - | |||||
| - |
| Specification | Battery Voltage () | Supercapacitor Voltage () | DC Link Voltage () | Switching Frequency () | Inductors ( and ) | Capacitor | Power |
|---|---|---|---|---|---|---|---|
| Values | 200 V | 160 V | 500 V | 20 kHz | 0.75 mH and 0.75 mH | 500 F | 15 kW |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gabbar, H.A.; Elsayed, Y.; Siddique, A.B.; Elshora, A.; Adeleke, A. Design of Fast Charging Station with Energy Management for eBuses. Vehicles 2021, 3, 807-820. https://doi.org/10.3390/vehicles3040048
Gabbar HA, Elsayed Y, Siddique AB, Elshora A, Adeleke A. Design of Fast Charging Station with Energy Management for eBuses. Vehicles. 2021; 3(4):807-820. https://doi.org/10.3390/vehicles3040048
Chicago/Turabian StyleGabbar, Hossam A., Yasser Elsayed, Abu Bakar Siddique, Abdalrahman Elshora, and Ajibola Adeleke. 2021. "Design of Fast Charging Station with Energy Management for eBuses" Vehicles 3, no. 4: 807-820. https://doi.org/10.3390/vehicles3040048
APA StyleGabbar, H. A., Elsayed, Y., Siddique, A. B., Elshora, A., & Adeleke, A. (2021). Design of Fast Charging Station with Energy Management for eBuses. Vehicles, 3(4), 807-820. https://doi.org/10.3390/vehicles3040048







