Too Persistent to Resist: Aromaticity in 16e Osmapentalene Radicals Survives Regardless of Redox

Abstract

As one of the most important concepts in organic chemistry, aromaticity has attracted considerable attention from both theoretical and experimental chemists. Limited by the traditional rules (Hückel’s rules and Baird’s rules), species can only achieve aromaticity in a single state (S₀ or T₁) in most cases. In 2018, our group first reported 16 electron osmapentalene that showed aromaticity in both the S₀ and T₁ states, which is defined as adaptive aromaticity. In recent years, although adaptive aromatic compounds have been expanded, the adaptive aromatics containing metal-centered radical has not been reported. Here, we carry out density functional theory calculations to explore the aromaticity of the corresponding radicals based on osmapentalyne and osmapentalenes in their S₀ states. It is found that the corresponding radicals of adaptive aromatic osmapentalene exhibit aromaticity regardless of the radicals formed by oxidation or reduction, supported by a series of aromaticity indices including ΔBL, NICS, AICD, EDDB, and ELF. In contrast, for the nonaromatic or antiaromatic compound in the T₁ state, only its cationic radical shows aromaticity. Furthermore, the spin density localization on the metal center is the key factor for the radicals to achieve aromaticity.

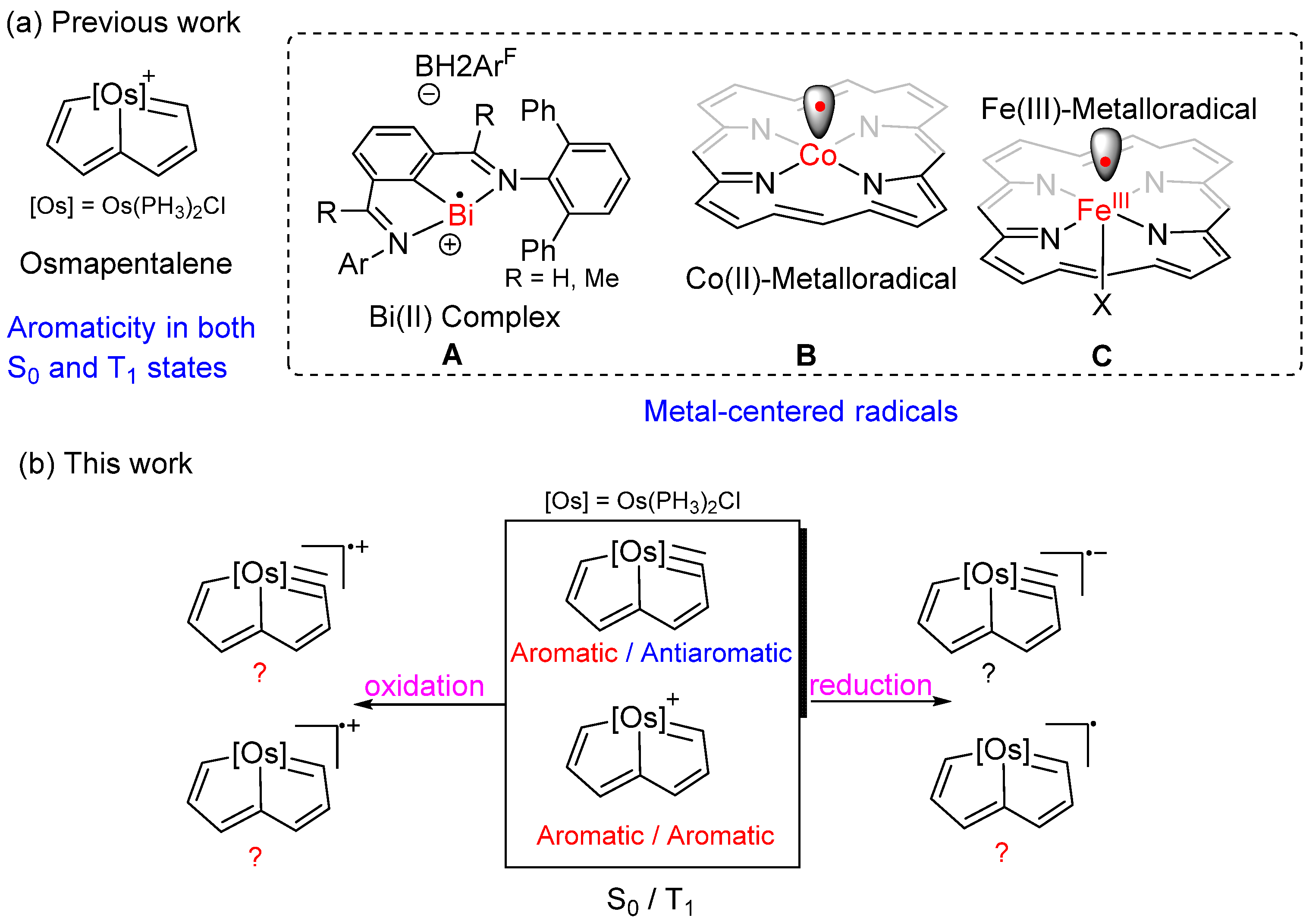
1. Introduction

The concept of aromaticity is of increasing interest to both experimental and theoretical chemists. In 1931, Erich Hückel studied the molecular orbital theory of π-conjugated hydrocarbons and proposed the classical [4n + 2] rule for aromaticity [1]. In the 1960s, Dewar gave an interpretation of Hückel’s theory [2]. In addition, Heilbronner [3] and Zimmerman [4,5] proposed and extended the concept of Möbius aromaticity. Dating back to 1979, Thorn and Hoffmann first predicted the existence of metalla-aromatics [6]. Three years later, Roper and co-workers synthesized the inaugural metallabenzene [7]. Subsequently, a series of metalla-aromatics were synthesized, encompassing metallabenzene [8,9,10,11,12,13,14], metallabenzyne [15,16,17], metallanaphthalyne [18,19,20], metallapentalyne [21,22,23], metallapentalene [24,25], and certain heteroatom-containing metallacycles such as metallafuran and its derivatives [26,27,28]. In 2013, Xia and co-workers reported the synthesis of aromatic metallapentalyne [29], which was a stark contrast to classical organic chemistry’s antiaromatic pentalyne counterpart. According to Hückel’s and Baird’s rules [1,30], cyclic conjugated species with 4n + 2 π-electrons have aromaticity in their singlet electronic ground state (S₀) whereas they are antiaromatic in their lowest triplet state (T₁); conversely, species with 4n π-electrons are deemed antiaromatic in the S₀ state but show aromaticity in the T₁ state. Limited by the two rules, species can only achieve aromaticity in a single state (S₀ or T₁). Up until 2018, our group conducted density functional theory calculations on osmapentalyne and osmapentalenes, unveiling an unprecedented example showcasing adaptive aromaticity (compound shows aromaticity in both the S₀ and T₁ states) [31], subsequently extending this phenomenon to ruthenacycles alongside other species (Scheme 1a) [32,33,34,35,36]. In addition, as one of the novel types among nonclassical aromaticity, metalloaromaticity gradually expanded the exploration from relatively small ring systems to macrocyclic metallaaromatic molecules both theoretically and experimentally [37,38,39,40]. Moreover, the concept of aromaticity has also been applied to catalysis [41,42].

As one of the most important active substances in organic chemistry, free radicals play an important role in the synthesis of complex natural products [43]. More attention has been paid to radicals in organic systems, especially carbon-centered radicals [44,45,46,47,48,49,50,51]. The study of heteroatom-centered radicals has also been steadily advancing [52,53], and it was surprising to find that the aromaticity in boron clusters survives radical structural changes [54]. There is also a lot of research on metal-centered radicals [55,56,57,58,59], which are used in a variety of reactions and helpful to the drug development [60]. In 2023, Cornella and co-workers reported the synthesis and characterization of two organobismuth(II) compounds (Scheme 1a Compound A), which were proven as metal-centered radicals with little delocalization onto the ligands by experimental data and DFT calculations [61]. Recently, Zhang and co-workers [62,63,64] combined experimental and theoretical studies to reveal stable metalloradicals (Compounds B and C in Scheme 1a) in the cyclopropanation reaction of asymmetric olefins and proposed a potential stepwise radical mechanism. Specifically, a cobalt-based metal radical catalyzed realization of the radical chemoselective intermolecular amination of the C-H bond at an allyl position effectively advanced the radical approach in the design of stereoselective organic synthesis and showed the importance of metalloradicals. In addition, metal radicals have also been used in the supramolecular field to form highly luminescent metallosupramolecular radical cages [65]. Inspired by the role of metal-centered radicals and our continuing interest in aromaticity, here we explore the aromaticity of the corresponding radicals based on osmapentalynes and osmapentalenes in the T₁ state, respectively, forming by oxidation and reduction (Scheme 1b). We carry out density functional theory (DFT) calculations on the metal-centered radicals to investigate whether the corresponding radicals based on adaptive aromatic compound still hold aromaticity in order to extend the scope of this novel family.

2. Methods

All the DFT calculations were carried out with Gaussian 16 software package [66]. Geometric optimizations, together with frequency calculations, were performed at the B3LYP level of theory. The 6-311++G (d, p) basis set was employed for C, H, and O atoms. For P, Cl and Os atoms, the pseudopotential basis set LANL2DZ was used, with polarization functions for P (ζ(d) = 0.340), Cl (ζ(d) = 0.514), and Os (ζ(f) = 0.886) [67,68]. Visualizations of structures are achieved by the CYLview program (version 1.0) [69]. NICS calculations [70] were carried out at (U)B3LYP-GIAO/def2-TZVP-level. Frequency calculations were performed to confirm that all optimized structures were energy minima. NICS(1)_zz values were obtained by placing ghost atoms at 1Å above/below the ring centers at the (U)B3LYP/def2-TZVP-level of theory. The electron density of delocalized bond (EDDB) analysis was carried out with RunEDDB [71,72]. EDDB was employed at the B3LYP/def2-TZVP-level. The anisotropy of the induced current density (AICD) plots was obtained using the AICD 2.0 program. The topological analysis implemented by Multiwfn (3.8 dev.) [73] is used to accurately locate the ELF_π bifurcation points by searching the critical points in the sphere and taking each nucleus in the molecule as the center of the sphere in turn.

3. Results and Discussion

3.1. The Geometries of the Corresponding Radicals from Complexes 1 to 3

Due to the different Gaussian versions used (the previous work was carried out with Gaussian 03), we initially recalculated the previous studies on model complexes 1–3 (Figure S1) for better comparative analysis. Based on the various analysis, all these three complexes in the S₀ state (1-S₀, 2-S₀, and 3-S₀) and complex 2 in the T₁ state (2-T₁) are aromatic, which indicates that the complex 2 has adaptive aromaticity, in line with our previous findings [31]. Similarly, we examined their corresponding radicals, which are obtained by oxidation (complexes 1-rad.1, 2-rad.1 and 3-rad.1) and reduction (complexes 1-rad.2, 2-rad.2 and 3-rad.2) by removing and adding one electron, respectively. As shown in Figure 1, the C–C bond lengths of these metal radicals are in the range of 1.358–1.448 Å, which are between the carbon–carbon double bond (1.333 Å in CH₂=CH₂) and the single bond (1.527 Å in CH₃–CH₃) calculated at the same level of theory. The bond length alternations (ΔBL) of the three radical cations (1-rad.1, 2-rad.1 and 3-rad.1) are 0.035, 0.042, 0.028Å, which indicate their aromaticity. On the contrary, the bond length alternations (ΔBL) of the radicals obtained by reduction are quite different. Only the complex 2-rad.2 has a relatively small bond length alternation (0.012Å), showing the potential of aromaticity. By the way, the relative energies of T₁ and D₀ with respect to the S₀ state are shown in

Table 1. The relative energy (kcal/mol) of T₁ and D₀ states with respect to the S₀ state. The values of ΔE_S-T are equal to E_S−E_T, standing for the relative energy between the S₀ state and the T₁ state. Similarly, the values of ΔE_S-D show the relative energy of radicals with respect to the S₀ state (D1: rad.1; D2: rad.2).

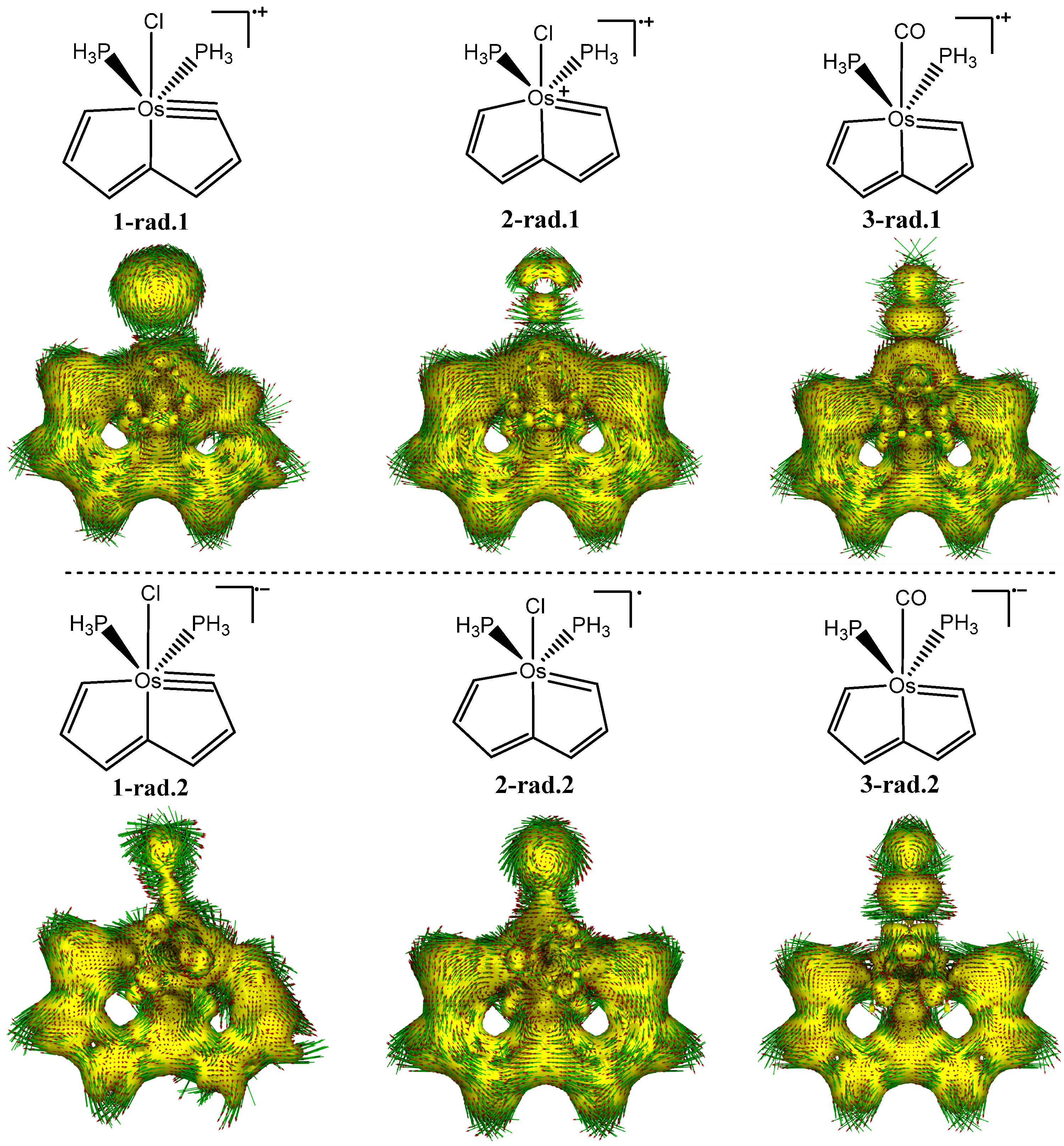
Compounds	ΔES-T	ΔES-D1	ΔES-D2
1	−44.2	−168.8	23.6
2	−21.6	−269.3	140.2
3	−36.0	−155.1	24.0

.

3.2. Aromaticity Analyses Based on Magnetic Properties

To further assess the (anti-)aromaticity of these complexes, we conducted the nucleus-independent chemical shift (NICS) calculations in their different states. NICS, proposed by Schleyer and co-workers has emerged as one of the most widely accepted criteria for determining aromaticity [74,75,76]. It is derived from computing the magnetic shielding effect of virtual atoms at any point on the ring (typically at the ring center for σ-aromaticity or 1 Å above the ring center for π-aromaticity). Commonly, significantly negative NICS values indicate aromaticity whereas positive NICS ones suggest antiaromaticity. The two-dimensional NICS grids are capable to show the magnetic shielding in aromatic rings while de-shielding in antiaromatic rings [77,78]. A value close to zero can be considered non-aromatic. In comparison with NICS(0)_zz, the NICS(1)_zz value is used here as it was reported to be more suitable in evaluating π aromaticity [79]. Surprisingly, the corresponding radical cations (1-rad.1, 2-rad.1, 3-rad.1) all show aromaticity (Figure 2) within significantly negative (shielded) NICS(1)_zz values (ranging from −21.0 ppm to −28.2 ppm), regardless of the aromaticity of the T₁ state itself. However, when the corresponding radicals are obtained by reduction, their aromaticity has different characteristics. Only the radical from compound 2 (2-rad.2) remains aromatic within significant negative NICS(1)_zz values (−24.5 ppm), whereas the corresponding radical anions, 1-rad.2 (0.6/4.3 ppm) and 3-rad.2 (0.6 ppm), exhibit non-aromaticity.

To further support the (anti-)aromaticity of these complexes, anisotropy of the induced current density (AICD) analyses was performed to examine the magnetic anisotropy, which has been proven to be a general method for visualizing delocalized electrons [80,81]. Generally, the clockwise ring current indicates aromaticity in the fused rings, and the anti-clockwise ring suggests anti-aromaticity. Clockwise currents along the perimeter of the fused rings in 1-rad.1, 2-rad.1, 3-rad.1, and 2-rad.2 indicate aromaticity, consistent with the NICS grids. In sharp contrast, the ring currents in 1-rad.2 and 3-rad.2 are tiny and disordered, characteristic of non-aromaticity (Figure 3). High-resolution plots are provided in the Supporting Information (Figures S4–S15).

3.3. Electron Density of Delocalized Bonds (EDDB) Analysis

The essence of aromaticity is the delocalization of electrons, and EDDB, proposed by Szczepanik and co-workers in 2014 [71], exhibits an advantage in evaluating the delocalized electrons quantitatively. It can be used to efficiently achieve the separation of σ and π contributions, and provides contribution of delocalized electrons from the specific fragments. The π electrons of the 8MR play an important role in aromaticity and the EDDB method is powerful in quantifying the delocalized electrons. Therefore, the π-EDDB_F method for the complex 8MR fragment was used in the aromaticity analysis (Figure 4). For a given system, the relatively larger EDDB values indicate more delocalized electrons and stronger aromaticity. The π-EDDB_F values of the radical cations (1-rad.1, 2-rad.1 and 3-rad.1) are relatively large (ranging from 4.83 e to 5.31 e), indicating their aromaticity, whereas among the radicals formed by reduction, only the corresponding radical of compound 2, within adaptive aromaticity, has a large π-EDDB_F value (5.87 e) and shows its aromaticity. According to the π-EDDB values of the 8MR, the results are in line with that from NICS and AICD analyses. In addition, we selected the key natural orbitals for bond delocalization (NOBDs) with π-contributions and provided them in Figures S16–S18.

3.4. Aromaticity Analyses Based on Electron Localization Function

For a better determination of aromaticity, we also manipulated topological analysis of electron localization function (ELF) which was used to assess the (anti-)aromaticity of systems by linking the properties of molecular electronic structure. It is worth noting that ELF_π (π-contribution to ELF) performs well in measuring (anti)aromaticity. A small span of ELF_π bifurcation values (ΔBV(ELF_π)) for C-C bonds is an indicator of aromaticity, the ΔBV(ELF_π)s (range from 0.232 to 0.334, as shown in Figure 1) in the radicals 1-rad.1, 2-rad.1 and 3-rad.1 are close to that of the compounds (0.108–0.235), which were proved to be aromatic by our previous work [31], indicating the better electron delocalization and their aromaticity. In addition, the ΔBV(ELF_π) of the radical 2-rad.2 is small (0.142), indicating its aromaticity. In contrast, the ΔBV(ELF_π) in antiaromatic compound 1-T₁ (0.721) is more than six times of that in aromatic 1-S₀ (0.108), consisting with our previous work (Figure S1) [31]. The intermediate ΔBV(ELF_π) (0.476–0.553) of 3-T₁, 1-rad.2 and 3-rad.2 indicate their non-aromaticity. All these results are in line with those from NICS, AICD, and EDDB analyses.

3.5. Aromaticity Analyses Based on Frontier Molecular Orbitals and Spin Populations

To explore the origin for the aromaticity of the corresponding radicals from compound 2, obtained by oxidation or reduction, we analyzed their frontier molecular orbitals. As shown in Figure 5, the highest singly occupied molecular orbitals (HSOMO) of the radical cations (1-rad.1, 2-rad.1 and 3-rad.1) obtained by oxidation are extremely similar to the HSOMO-1 of the parent complexes in the T₁ state. In the case of compound 2, the HSOMO of 2-rad.1 exhibits an out-of-plane orientation. When compared to the HSOMO-1 of 2-T₁, it becomes evident that this out-of-plane orientation contributes more significantly to its aromaticity. As for radical cations 1-rad.1 and 3-rad.1, they will not be affected by the contribution to out-of-plane antiaromaticity, thus they could keep their aromaticity. Meanwhile, the HSOMO of 1-rad.1 shows a slight clockwise current, which indicates that it contributes a slight contribution for the aromaticity. It is interesting that the radicals obtained by reduction perform different aromaticity and only the radical 2-rad.2 keeps aromaticity whereas the radical anions (1-rad.2 and 3-rad.2) become nonaromatic. Focusing on the radicals 1-rad.2, 2-rad.2 and 3-rad.2, their HSOMOs are similar to the HSOMOs of parent complexes in the T₁ state. For complex 2, the HSOMO of the triplet (T₁) state is oriented in-plane, thereby allowing us to disregard its contribution to out-of-plane aromaticity, in line with our previous work [31]. Similarly, in complex 2-rad.2, the HSOMO also exhibits an in-plane orientation, and this orientation does not reverse its aromaticity. However, the aromaticity change in radical anions 1-rad.2 and 3-rad.2 could contribute to the newly generated significantly paratropic ring current of HSOMOs. All these qualitative analyses are also supported by AICD calculations on these key frontier molecular orbitals (Figure 5).

The studies have demonstrated that the localized spin density on metal center is beneficial to achieve aromaticity for complexes [31,35,36]. In line with the previous findings, aromatic systems (compounds 1-rad.1, 2-T₁, 2-rad.1, 2-rad.2 and 3-rad.1) display localized spin density on the metal center, whereas other anti(non-)aromatic complexes have spin density distributed mainly on the carbon rings. As shown in Figure 6, the spin populations on the osmium center of complexes 1-rad.1, 2-T₁, 2-rad.1, 2-rad.2 and 3-rad.1 are large (45.12–68.41%). In contrast, the spin population on the seven carbon atoms of the eight-membered ring (R_7C) in these complexes are relatively small. Conversely, the atomic spin population in the nonaromatic radical anions 1-rad.2 and 3-rad.2 are mostly distributed on the carbon atoms rather than the metal center, similar to the 1-T₁ and 3-T₁. In summary, the spin density contributed on the metal center plays a crucial rule in their aromaticity.

4. Conclusions

We performed DFT calculations on the osmapentalyne, as well as osmapentalene complexes and their corresponding radicals. It was found that the corresponding radicals of adaptive aromatic complex 2 retain aromaticity regardless of the radicals formed by oxidation or reduction, supported by various aromaticity indices including ∆BL, NICS, AICD, EDDB and ELF. It is necessary to try as many aromatic indices as possible because using a single index is unilateral and may lead to inconsistent judgements [82,83]. The localization of spin electrons on the metal center could be regarded as one of the key reasons to achieve aromaticity. All these findings not only deepen the understanding of the concept of aromaticity, but also help the development of radical chemistry.