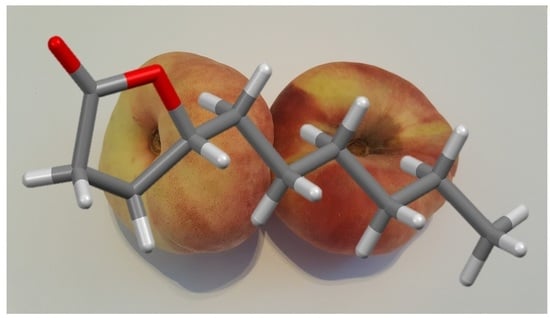
Absolute Configuration of In Situ Crystallized (+)-γ-Decalactone †
Abstract
1. Introduction
2. Materials and Methods
3. Results
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Maher, T.J.; Johnson, D.A. Review of chirality and its importance in pharmacology. Drug Dev. Res. 1991, 24, 149–156. [Google Scholar] [CrossRef]
- Jayakumar, R.; Vadivel, R.; Ananthi, N. Role of Chirality in Drugs. Org. Med. Chem. Int. J. 2018, 5, 555661. [Google Scholar]
- Sheldon, R.A. Chirotechnology: Designing economic chiral syntheses. J. Chem. Technol. Biotechnol. Int. Res. Process Environ. AND Clean Technol. 1996, 67, 1–14. [Google Scholar]
- Bassoli, A.; Borgonovo, G.; Drew, M.G.B.; Merlini, L. Enantiodifferentiation in taste perception of isovanillic derivatives. Tetrahedron Asymmetry 2000, 11, 3177–3186. [Google Scholar] [CrossRef]
- Zawirska-Wojtasiak, R. Chirality and the Nature of Food Authenticity of Aroma. Acta Sci. Pol. Technol. Aliment. 2006, 5, 21–36. [Google Scholar]
- Ulrich, D.; Hoberg, E.; Rapp, A.; Kecke, S. Analysis of strawberry flavour—Discrimination of aroma types by quantification of volatile compounds. Z. Lebensm. Forsch. A 1997, 205, 218–223. [Google Scholar] [CrossRef]
- Guillot, S.; Peytavi, L.; Bureau, S.; Boulanger, R.; Lepoutre, J.-P.; Crouzet, J.; Schorr-Galindo, S. Aroma characterization of various apricot varieties using headspace–solid phase microextraction combined with gas chromatography–mass spectrometry and gas chromatography–olfactometry. Food Chem. 2006, 96, 147–155. [Google Scholar] [CrossRef]
- Ternes, W. Naturwissenschaftliche Grundlagen der Lebensmittelzubereitung. Behr: Santa Ana, CA, USA, 2008. [Google Scholar]
- Parsons, S. Determination of absolute configuration using X-ray diffraction. Tetrahedron Asymmetry 2017, 28, 1304–1313. [Google Scholar] [CrossRef]
- Linden, A. Best practice and pitfalls in absolute structure determination. Tetrahedron Asymmetry 2017, 28, 1314–1320. [Google Scholar] [CrossRef]
- Bhatt, P.M.; Desiraju, G.R. Co-crystal formation and the determination of absolute configuration. CrystEngComm 2008, 10, 1747–1749. [Google Scholar] [CrossRef]
- Boese, R. Special issue on In Situ Crystallization. Z. Krist. Cryst. Mater. 2014, 229, 595–601. [Google Scholar] [CrossRef]
- Veith, M.; Frank, W. Low-temperature x-ray structure techniques for the characterization of thermolabile molecules. Chem. Rev. 1988, 88, 81–92. [Google Scholar] [CrossRef]
- Cremer, D.; Pople, J.A. General definition of ring puckering coordinates. J. Am. Chem. Soc. 1975, 97, 1354–1358. [Google Scholar] [CrossRef]
- Thomas, S.A.; Agbaji, E.B. Molecular conformations of γ-lactone rings from crystal structure data. J. Crystallogr. Spectrosc. Res. 1989, 19, 3–23. [Google Scholar] [CrossRef]
- Torshin, I.Y.; Weber, I.T.; Harrison, R.W. Geometric criteria of hydrogen bonds in proteins and identification of ‘bifurcated’ hydrogen bonds. Protein Eng. Design Sel. 2002, 15, 359–363. [Google Scholar] [CrossRef] [PubMed]
- Wood, P.A.; Allen, F.H.; Pidcock, E. Hydrogen-bond directionality at the donor H atom—Analysis of interaction energies and database statistics. CrystEngComm 2009, 11, 1563–1571. [Google Scholar] [CrossRef]
- Parsons, S.; Flack, H.D.; Wagner, T. Use of intensity quotients and differences in absolute structure refinement. Acta Crystallogr. Sect. B 2013, 69, 249–259. [Google Scholar] [CrossRef] [PubMed]
- Escudero-Adan, E.C.; Benet-Buchholz, J.; Ballester, P. The use of Mo K[alpha] radiation in the assignment of the absolute configuration of light-atom molecules; the importance of high-resolution data. Acta Crystallogr. Sect. B 2014, 70, 660–668. [Google Scholar] [CrossRef] [PubMed]
- Sheldrick, G. SHELXT—Integrated space-group and crystal-structure determination. Acta Crystallogr. Sect. A 2015, C71, 3–8. [Google Scholar] [CrossRef] [PubMed]


| Empirical formula | C10H18O2 |
| Mr | 170.24 |
| (K) | 100(2) |
| (Å) | 1.54178 |
| crystal system | orthorhombic |
| Space group | P212121 |
| 5.0543(6) | |
| 5.3683(6) | |
| 37.143(4) | |
| 1007.8(2) | |
| 4, 1 | |
| ) | 1.122 |
| 0.603 | |
| F(000) | 376 |
| Crystal size (mm) | 1.078 × 0.441 × 0.411 |
| range for data collection (°) | 2.379 to 71.877° |
| Reflections collected/unique | 33819/1902 |
| 0.0389 | |
| observed reflections [] | 1880 |
| 0.84565/0.69343 | |
| data/restraints/parameters | 1902/0/128 |
| Goodness-of-fit on | 1.089 |
| R1 [] | 0.0302 |
| R2 (all data) | 0.0688 |
| Flack x parameter (refined) | −0.025(285) |
| Flack x parameter (from quotients) | −0.019(60) |
| Hooft parameter | −0.03(5) |
| / | 0.106/−0.144 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Patzer, M.; Nöthling, N.; Goddard, R.; Lehmann, C.W. Absolute Configuration of In Situ Crystallized (+)-γ-Decalactone. Chemistry 2021, 3, 578-584. https://doi.org/10.3390/chemistry3020040
Patzer M, Nöthling N, Goddard R, Lehmann CW. Absolute Configuration of In Situ Crystallized (+)-γ-Decalactone. Chemistry. 2021; 3(2):578-584. https://doi.org/10.3390/chemistry3020040
Chicago/Turabian StylePatzer, Michael, Nils Nöthling, Richard Goddard, and Christian W. Lehmann. 2021. "Absolute Configuration of In Situ Crystallized (+)-γ-Decalactone" Chemistry 3, no. 2: 578-584. https://doi.org/10.3390/chemistry3020040
APA StylePatzer, M., Nöthling, N., Goddard, R., & Lehmann, C. W. (2021). Absolute Configuration of In Situ Crystallized (+)-γ-Decalactone. Chemistry, 3(2), 578-584. https://doi.org/10.3390/chemistry3020040