1. Introduction
Fluoroargentates(II) attract interest because of their noticeable similarities with isoelectronic copper(II) oxides; many interesting physical phenomena occur in the latter. One of them is low dimensional magnetism, which plays an important role in superconducting materials. The structural simplicity of these systems also provides important insights into correlation between details of the crystal structure and physical properties, and it provides physicists with a framework to test the available theories. Studying (quasi)-1D antiferromagnets allowed for observation of, for example, spin-Peierls transition [
1], Haldane chains, or spin-ladder systems [
2]. KAgF
3, which adopts a distorted perovskite structure [
3], is an example of such a low-dimensional magnetic system. Magnetic susceptibility measurements indicate substantial magnetic anisotropy in this compound, with strong antiferromagnetic ordering along the crystallographic
b axis, and a weak ferromagnetic one in the
ac plane (
Pnma setting).
KAgF
3 can be regarded as quasi-1D antiferromagnet in which superexchange constant along kinked Ag–F–Ag chains (
Figure 1), J
1D, is about 20 times larger than in the remaining two dimensions [
4,
5]. Importantly, the standard Generalized Gradient Approximation (GGA) approach fails to reproduce the antiferromagnetic ground state of KAgF
3. Due to considerable correlation of silver valence electrons on
d orbitals, it is necessary to introduce Mott-Hubbard correction within the GGA+U framework [
4]. The GGA+U approach permits to reproduce experimental J values for both strong intra-chain and much weaker intra-sheet interactions [
5,
6]. The unique character of KAgF
3 stems from the fact that the intra-chain antiferromagnetic interactions are immensely strong, of the order of 100 meV, thus comparable to those for copper(II) oxides, as well as from substantial magnetic anisotropy.
Interestingly, KAgF
3 exhibits an intriguing phase transition at 230 K which resembles a spin-Peierls transition; it has been preliminarily assigned to a structural order/disorder-type (
Pnma/
Pcma) transition based on X-ray diffraction studies [
4]. Moreover, this compound shows a complex magnetic ordering at temperatures below 66 K [
6], which is subject to ongoing investigation. Analysis of X-ray diffraction experiments suggests that the phase transition present at ca. 230 K might be of the order/disorder type. The low temperature ordered polymorph crystallizes in the
Pnma space group while the high-temperature disordered one is in the
Pcma one, exhibiting tilting of [AgF
4]
2– units around the
a and
c vectors [
4]. Most characteristic structural features (tilting of octahedra, Jahn-Teller effect), as well as magnetic features (antiferromagnetic 1D character) are retained during this transition.
Stability of metal halide perovskites is often represented in terms of Goldschmidt tolerance factor
t (originally developed for oxide systems). Compounds with
t in a range of 0.71–0.90 are prone to adopt orthorhombic symmetry [
7]. It was also found that certain types of perovskites, particularly useful for photonics, exhibit
t in a range of 0.80–1.00 [
7]. KAgF
3 has a tolerance factor equal to 0.77 being indeed orthorhombic. However, dynamic stability of this compound and its possible phase transitions are of interest.
Lattice dynamics studies of related AAgF3 (A—cation) systems have been scarce despite the fact that they host interesting physical phenomena. There are no spectroscopic data published on KAgF3 to date. The goal of the current work is to gain insight into lattice dynamics (phonons) of KAgF3 with both experimental and theoretical tools, assign the bands appearing in the Raman scattering and infrared absorption spectra, as well as further elucidate the nature of the 230 K phase transition. Understanding the impact of disorder on the lattice dynamics is also of interest here.
2. Experimental
KAgF
3 has been prepared using a published synthesis route yielding high purity product (an equimolar mixture of KF and AgF
2 was heated in nickel reactor, under F
2 atmosphere, for four days at 300 °C) [
4]. Infra-red measurements were carried out on Bruker Vertex 80 V vacuum spectrometer using powdered samples placed on HDPE windows. Raman spectra were obtained on a Horiba Jobin Yvon LabRam-HR Raman micro-spectrometer with 632.8 nm He–Ne laser exciting beam. As with other Ag
II compounds, the power of laser beam had to be very low, in order to minimize thermal (or photo-) decomposition [
8,
9]. In this work we have used power of circa 0.3 mW. KAgF
3 can also easily decompose in contact with the atmosphere, so the sample was enclosed inside a sealed quartz capillary. In the case of low-temperature Raman measurements, the capillary was placed in a home-made flow cryostat, described in more detail below, and measured using Horiba Jobin Yvon T64000 Raman spectrometer with 514.5 nm Ar-Kr laser exciting beam, with on-sample power lower than 0.5 mW.
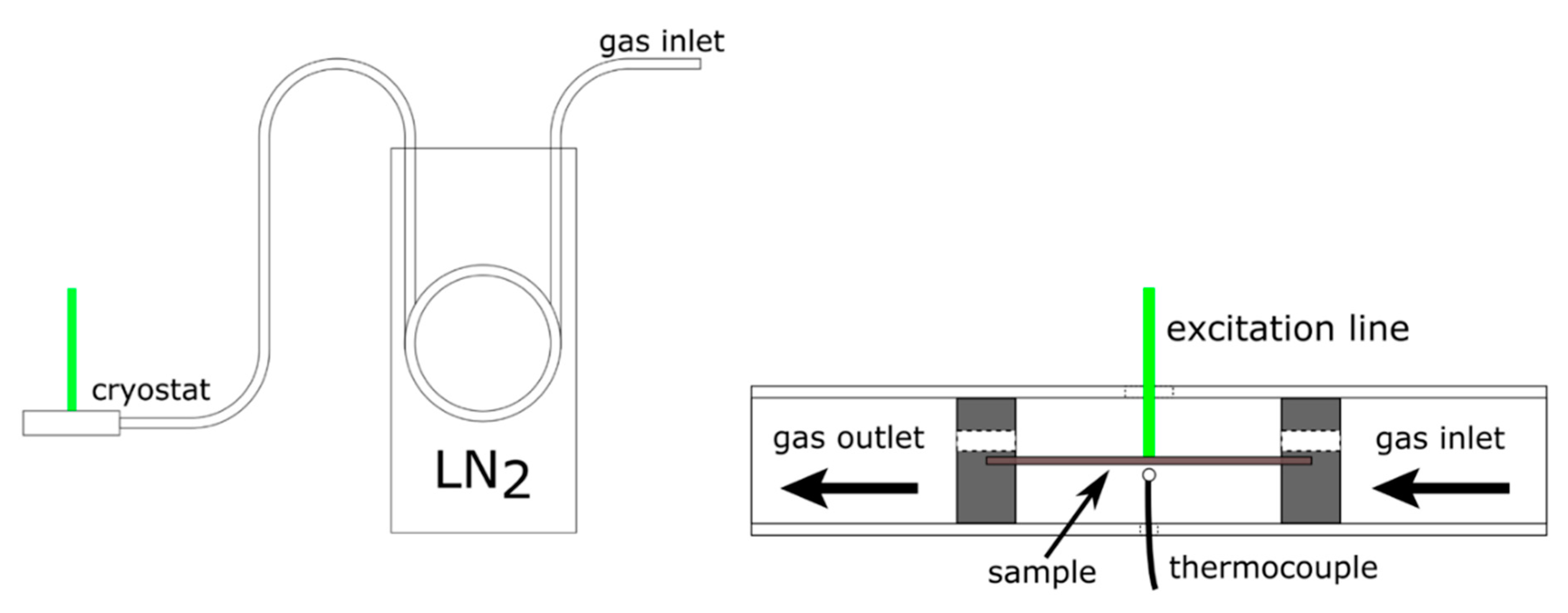
The low-temperature setup was prepared in the following way: 5 N Ar gas bottle was connected to fluorinated ethylene propylene (FEP) coil placed in a 3 L dewar (
Figure 2). The amount of LN2 in the dewar and the flow rate of cool Ar gas were chosen to ensure a near constant temperature in the vicinity of the sample (a maximum of 1–2 K drift was observed during measurements).
The cryostat itself was made from FEP tube with aluminum disks. Holes drilled in the aluminum disks enabled gas flow through the measurement chamber, and retained sample in place. The main body of the cryostat, made from FEP tube, had two holes drilled on opposing sides of the tube—the first one, about 3 mm in diameter to let in the excitation line, the second one, about 1 mm in diameter, to let in a thermocouple. The temperature inside the cryostat was measured using a thermocouple positioned about 1 mm from the capillary with enclosed sample. To ensure that no condensation formed in the hole for the excitation line, a heat gun was used to blow the newly formed ice crystals away from the cryostat. The minimum stable temperature reached in this setup was equal to 123 K, way below the 230 K order/disorder-type phase transition but above the temperature of magnetic ordering (66 K), and we report here spectra measured at 123 K as well as at room temperature.
3. Computational Details
Lattice dynamics was modelled using GGA+U framework using Lichtenstein formalism [
10] with values of U = 5.0 eV and J = 1.0 eV for silver atoms in order to account for strong electron correlation. The Perdew–Burke–Ernzerhof functional revised for solids (PBEsol) [
11] and the projector-augumented-wave method [
12,
13] were used, as implemented in VASP 5.2.12 code [
14,
15,
16]. The cut-off energy of the plane wave basis set was equal to 800 eV with a self-consistent-field convergence criterion of 1 × 10
−7 eV. The k-point mesh spacing used in calculations was equal to 0.16 Å
−1. Ordered, low temperature,
Pnma structure of KAgF
3 was chosen as a starting model for the calculations [
4]. An antiferromagnetic model was assumed in agreement with Zhang et al. (AFM along the chains, FM in planes) [
5].
Phonon dispersion curves and phonon density of states were calculated for a 2 × 2 × 2 supercell with the use of PHONON software package [
17,
18]. Hellmann–Feynman forces were evaluated using series of atomic displacements (0.0018–0.0025 Å) for which single point energies were calculated with the settings mentioned above and harmonic approximation. Furthermore, obtained Γ-point frequencies were compared with values obtained using HSE06 functional [
19]. For that comparison the finite differences method was used, as implemented in VASP core. These calculations were carried for the KAgF
3 unit cell, using less dense k-point mesh spacing of 0.25 Å
−1 to reduce the calculation cost. This implementation yields frequencies in the centre of the first Brillouin zone (Γ point).
4. Results and Discussion
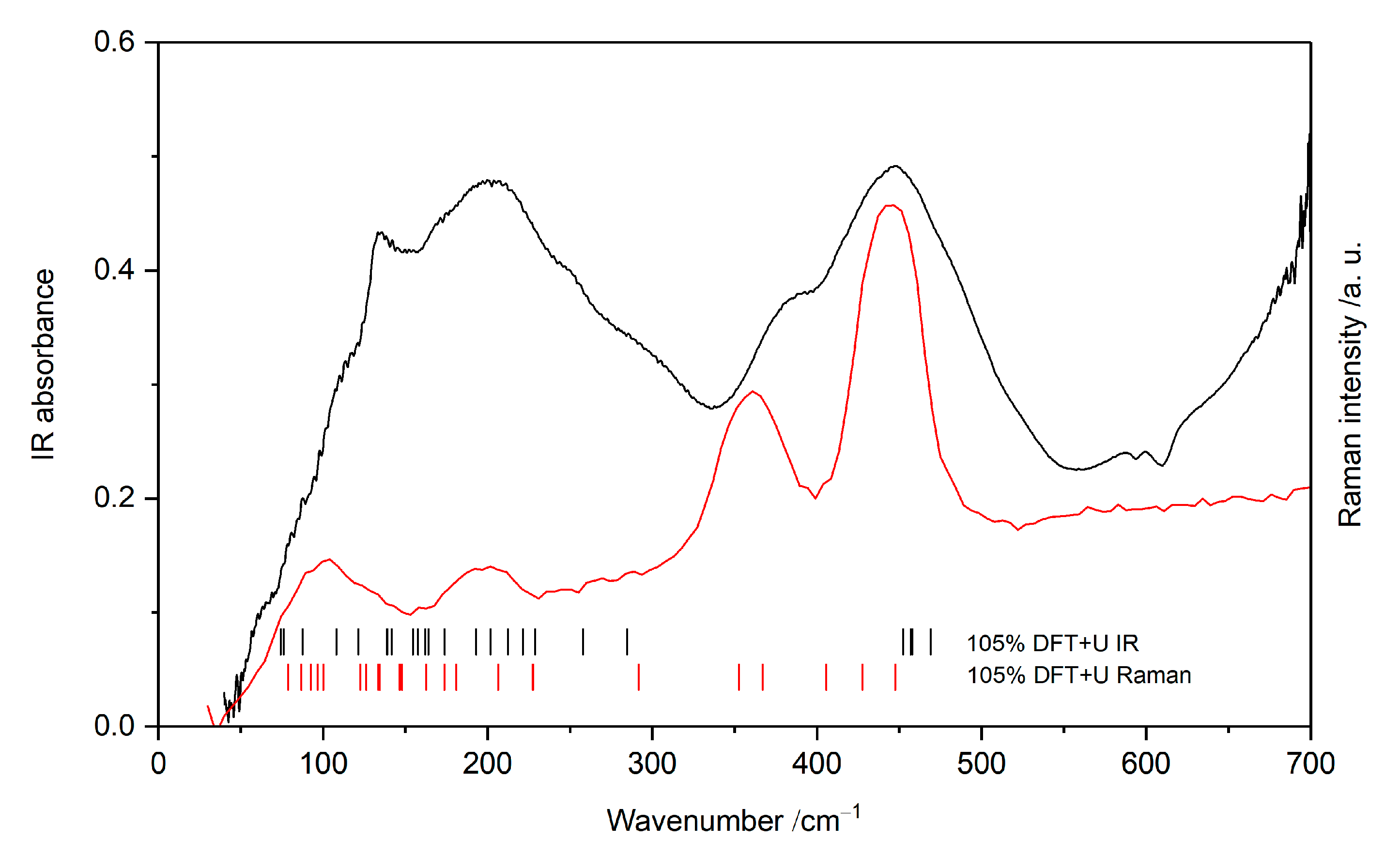
Infrared (
Figure 3) and Raman spectra (
Figure 4) were measured from the instrument lower limit up to 1000 cm
−1. FIR measurement resulted in eight separate bands, while Raman measurement showed seven bands. Low temperature Raman measurement exhibits a slight temperature shift towards higher wavenumbers. A density functional framework was used to gain in-depth understanding of present spectral features.
To set the grounds for analysis of the experimental spectra, we begin our discussion with group theory analysis. According to Group theory analysis there are 60 normal vibrational modes for KAgF3 in Pnma symmetry (Γacoustic = B1u + B2u + B3u; Γoptic = 7Ag + 8Au + 5B1g + 9B1u + 7B2g + 7B2u + 5B3g + 9B3u).
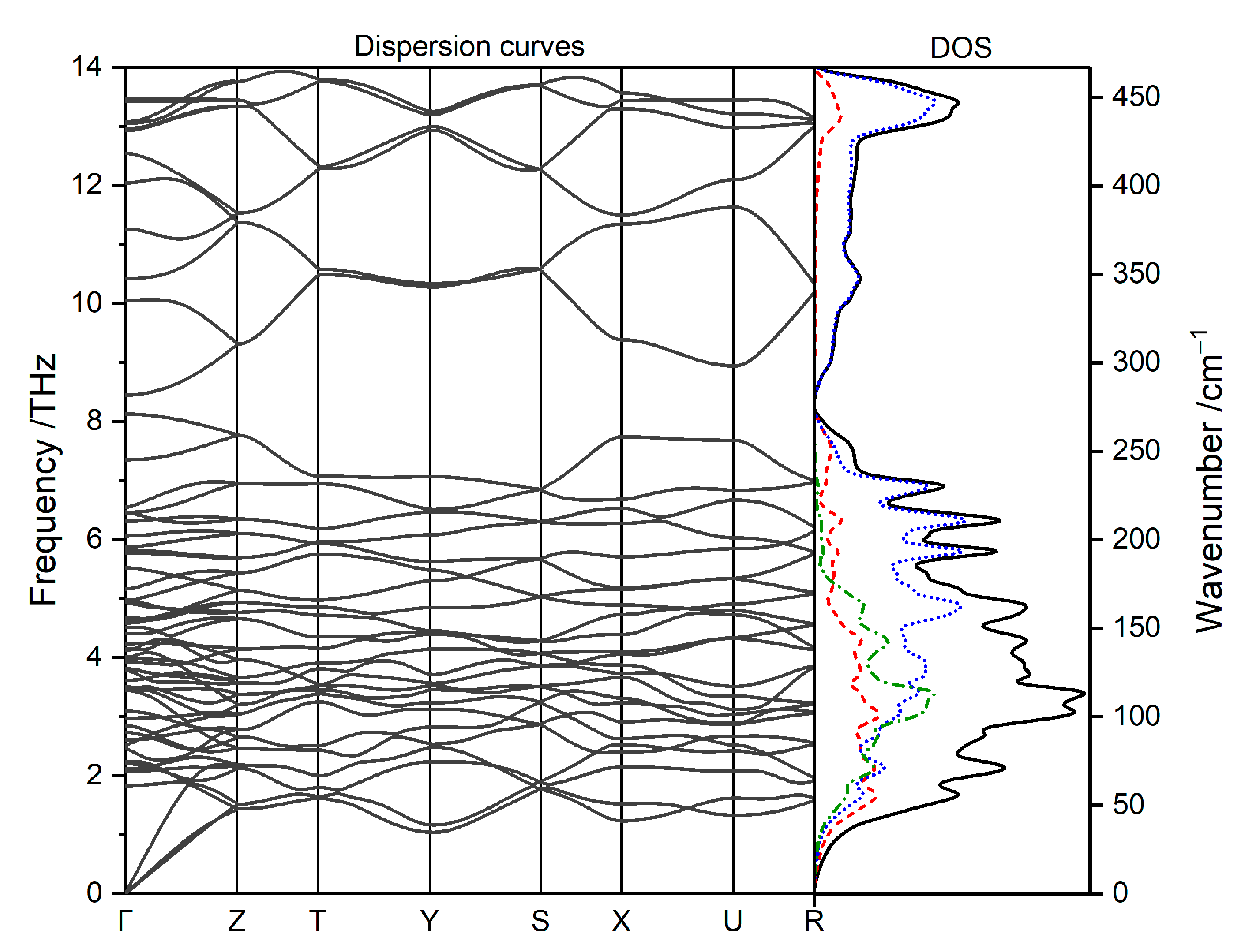
Calculated phonon dispersion curves and phonon density of states are shown in
Figure 5. No imaginary modes are seen; hence, the low-temperature structure is confirmed to be dynamically stable. Partial (atomic) phonon density of states (
Figure 5, right) yields information about atoms involved in oscillations. Up to ca. 175 cm
−1 all atoms contribute comparably to normal modes. The characteristic region of vibrations of potassium atoms is within the energy range 0–225 cm
−1. Silver atoms contribute within slightly broader energy range 0–260 cm
−1 as well as to the highest energy modes between 400 and 450 cm
−1. Fluorine atoms contribute considerably to all phonon modes of the KAgF
3. Their contribution dominates the phonon DOS within the energy range 150–250 cm
−1, which is the characteristic energy region of the F-Ag-F bending vibrations, and the entire higher energy phonon DOS in the range 300–450 cm
−1, which is the characteristic region of the Ag-F stretching vibrations.
The phonon bands belonging to F-Ag-F bending and low-energy lattice modes (below 250 cm−1) show relatively low dispersion. On the other hand, phonon bands stemming from diverse Ag-F stretching modes exhibit substantial dispersion indicating considerable coupling of the stretching vibrations along both the direction of the propagation of the AFM chains (corresponding to Y point in the 1st Brillouin zone) and within the [AgF2] planes (X and Z points in the 1st Brillouin zone).
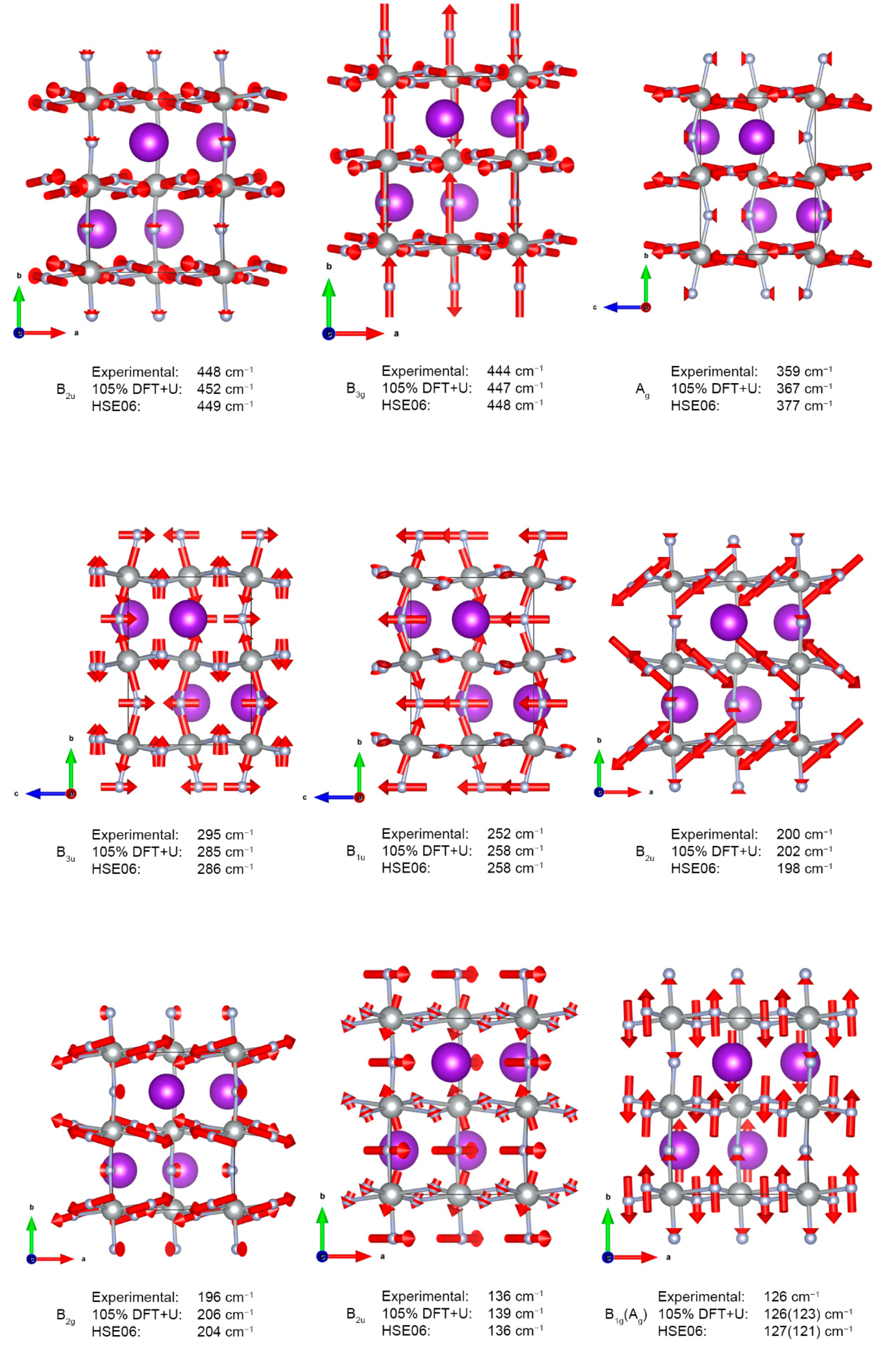
The eigenvectors for selected normal modes assigned to measured IR and Raman spectra are shown in
Figure 6, while the complete list of theoretically predicted Γ-point frequencies is contained in Electronic
Supplementary Material.
The measured room-temperature far infra-red absorption spectrum of KAgF
3 (
Figure 3) consists of eight bands, of which seven were successfully assigned using DFT+U framework while considering both selection rules and calculated absolute values of wavenumbers; we found out that the best match between theoretical and experimental wavenumbers is obtained if the former ones are scaled by the factor of 1.05 (
Table 1 and
Figure 3). The highest frequency band is placed at 448 cm
−1 and was assigned to B
2u stretching within [AgF
2] planes in the
ac plane. The 377 cm
−1 shoulder cannot be assigned to any fundamental mode; it likely originates either from 126 cm
−1 + 252 cm
−1 combination mode (A
1u) or from an unknown impurity. In a lower frequency range, observed bands are rather broad and not fully resolved. One feature centred at 200 cm
−1 may be assigned to [AgF
2] plane buckling vibrations (B
2u). Two shoulders at 295 and 252 cm
−1 are associated with similar plane buckling, as well as bending of the [AgF
+] chain (B
3u and B
1u, respectively). The 136 cm
−1 band of medium strength is also assigned to chain bending (B
2u), but its two shoulders originate from potassium and fluoride lattice modes (both B
2u, at 110
−1 and 59 cm
−1, respectively).
In the measured room-temperature Raman spectrum seven bands are clearly visible. Two bands at 444 cm
−1 and 359 cm
−1 were assigned to Ag-F stretching within chain-forming [AgF
6] octahedra and within [AgF
2] planes (B
2g and A
g, respectively). The weak band at 196 cm
−1 is associated with [AgF
2] intra sheet bending vibrations (B
2g). The lowest lying 126 cm
−1 and 101 cm
−1 features correspond, respectively, to B
1g and/or a nearby A
g, and B
2g lattice modes involving potassium cations. There are two more bands in the spectral region above 800 cm
−1 (not shown); the shoulder at 899 cm
−1 is supposedly the first overtone of the IR-active B
2u mode at 448 cm
−1; the origin of the 949 cm
−1 band currently remains unknown. The assignment presented above is substantially strengthened by the fact that HSE06 hybrid DFT calculations yield identical band assignment without necessity to scale wavenumbers by the 1.05 factor (
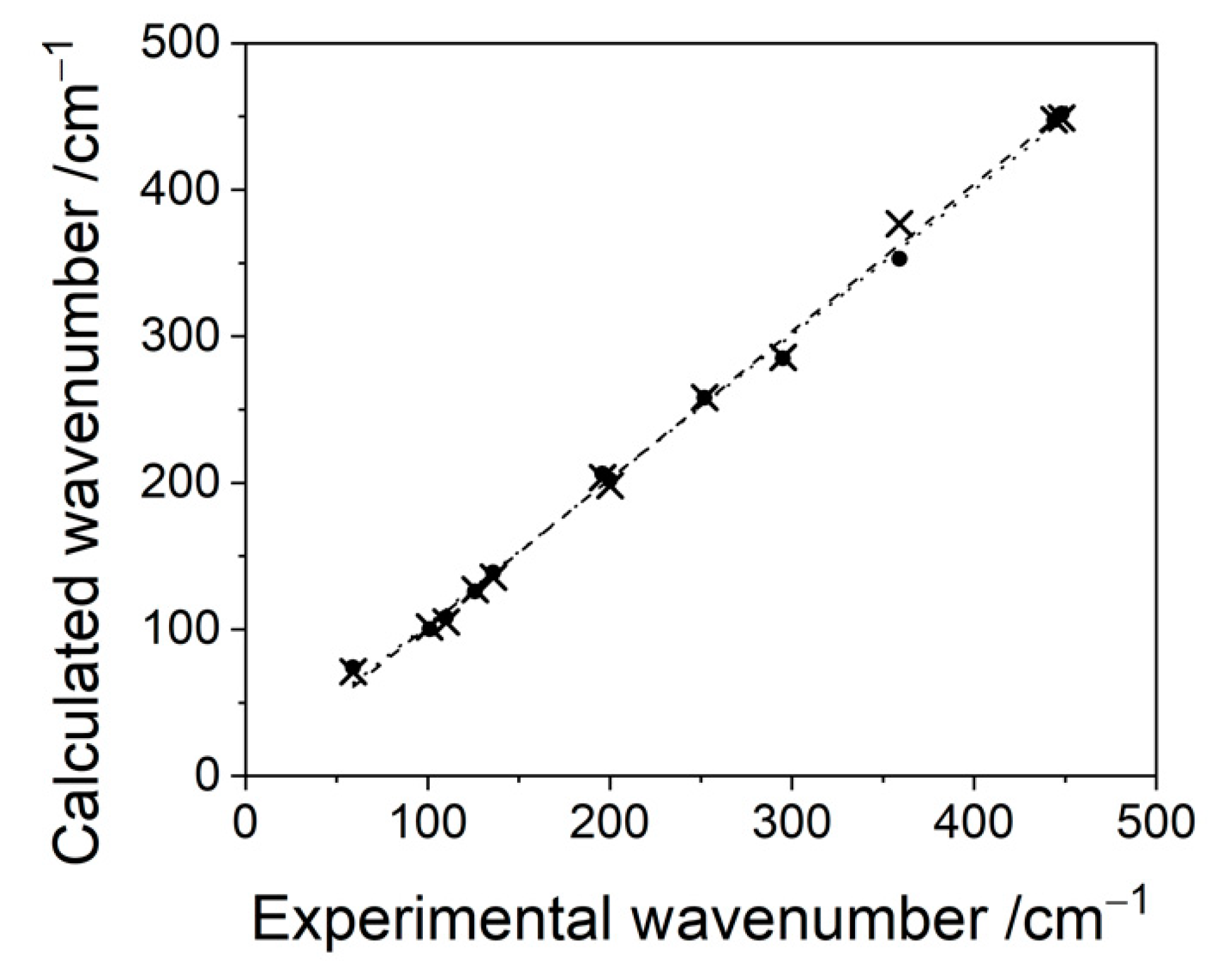
Figure 7). Linear regression with excellent correlation factor of R
2 = 0.997 is obtained for HSE06 dataset.
The measured low-temperature Raman spectra are compared with the room-temperature one in
Figure 4. Both spectra are characteristic of the same four broad main features, while in the low-temperature spectrum these features are shifted to slightly higher energies. All bands that are present at low temperature, are observable also at RT, and the general shape of the spectrum remains unchanged. There is some phonon stiffening observed at low temperatures, with the 444 cm
−1 band now appearing at 464 cm
−1, the 359 cm
−1 one at 380 cm
−1, the 196 cm
−1 one at 217 cm
−1, the 126 cm
−1 one at 127 cm
−1, and the 101 cm
−1 one at 109 cm
−1.
In published work, the disordered room temperature
Pcma structure is derived by dividing the
Pnma unit cell in half and introducing disorder of fluoride anions. The fluorine anions are placed alternately along the [AgF
+] chains with an occupancy of 0.5 [
2]. As this type of phase transition does not substantially alter the network of covalent bonds, the thermal shift observed in Raman spectra can be satisfactorily explained by thermal compressibility of the KAgF
3 lattice. With decreasing temperature, Ag–F bond lengths also decrease, causing energy of associated stretching and bending phonons to rise. The fact that the room temperature vibrational spectra of KAgF
3 may be successfully assigned using the low-temperature
Pnma structure serves as a confirmation of the X-ray diffraction-based hypothesis described above. This is additionally confirmed by comparing Raman spectrum obtained at room temperature and at 123 K (
Figure 4,
Table 2).