Assessing Engineering Students’ Conceptual Understanding of Introductory Quantum Optics
Abstract
:1. Introduction
1.1. Educating the Future Quantum Workforce
- (1)
- The experimental and physical foundations of experiments with heralded photons may directly be leveraged to quantum technology applications within teaching scenarios, e.g., with regard to quantum computing [28], quantum metrology [29], or quantum information [30,31]. This content-specific argument is especially important with regard to undergraduate courses within study programmes for prospective quantum engineers.
- (2)
- Such experiments “provide the simplest method to date for demonstrating the essential mystery of quantum physics” [32] (p. 1), and “elegantly illustrate the fundamental concepts of quantum mechanics such as the wave-particle duality of a single photon, single-photon interference, and the probabilistic nature of quantum measurement” [33] (p. 1). Hence, such quantum optics-based approaches are likely to be conducive to circumvent widespread learning difficulties regarding quantum concepts, as has already been indicated by empirical results presented in Ref. [34].
1.2. Aim of This Study
- (1)
- investigate as to how our concept inventory psychometrically functions on a sample of engineering students,
- (2)
- establish a difficulty scale regarding concepts covered by quantum optics experiments with heralded photons suggested by students’ scores on the concept inventory.
2. Research Background
2.1. Assessment of Students’ Conceptual Understanding of Quantum Optics
2.2. Students’ Conceptions on Quantum Optics Aspects
- a part of the learners interpret single-photon interference via photons that divide and then overlap with themselves [55] (p. 216);
- a part of the respondents equate photons with waves and conclude from this the necessity of observing interference phenomena [55] (p. 221);
- some learners claim that the photons’ localization would fail due to the small size of the photons [55] (p. 227);
- furthermore, a part of the participants believe that quantum anticorrelation at a beam splitter cube would be caused by photons behaving like haptical particles, being either reflected or transmitted [55] (p. 232).
3. The Quantum Optics Concept Inventory for Engineering Students
- (1)
- we updated the existing items (and the corresponding distractors) from Ref. [49], in terms of language, and
- (2)
- beyond the content domains covered in the test of Ref. [49], namely (a) the theoretical and (b) the experimental basics of quantum optics, we developed new items to incorporate the thematic area of the technical basics of quantum optics experiments into the instrument, since we believe this content domain to be relevant to future quantum engineers.
- (1)
- helps to minimize the effect of guessing, and hence, is “useful for gauging the quality of students’ understanding” [59] (p. 3); on the other hand,
- (2)
- it allows for the exploration of learning difficulties regarding the content area under investigation, namely by analyzing incorrect answers that were given confidently [62]. This point is postponed for the future research.
4. Research Questions
- RQ1:
- How does the Quantum Optics Concept Inventory function on a sample of engineering students?
- RQ2:
- What difficulty scale of quantum optics content aspects is suggested by students’ scores on the concept inventory?
5. Methods
5.1. Test Administration and Sample
5.2. Intervention
- (1)
- (2)
5.3. Data Analysis
5.3.1. Analysis Carried out to Answer RQ1
- the skewness and kurtosis of the items do not exceed the range of to ,
- the items are locally independent, and
- the uni-dimensionality of the construct can be assumed.
5.3.2. Analysis Carried out to Answer RQ2
6. Results
6.1. Psychometric Characterization
6.2. Difficulty Scale of Quantum Optics Concepts
7. Discussion
7.1. Discussion of RQ1
7.2. Discussion of RQ2
- (1)
- The item difficulties of the items 12 ( logits), and 13 ( logits) both lay well above average—hence, single-photon interference obviously poses conceptual difficulties to the study participants. This observation is in accordance with prior research, and can be enriched by findings from various qualitative studies exploring student difficulties on the interference of single quanta [33,55].
- (2)
- Item 14, focusing on the anticorrelation effect of single-photon states at an optical beam splitter cube, has an item difficulty of logits. Hence, the concept that photons are either transmitted or reflected at a beam splitter cube seems to be difficult for students. This observation fits well with results presented in an earlier contribution [51], where the authors used a micro-intervention to explore students’ understanding of single-photons’ anticorrelation, using the technique of probing acceptance. Beyond that, this observation is particularly striking against the background of the finding described above regarding single-photon interference: while items 12 and 13 address the wave nature of photons, item 14 can be associated with a particle nature of photons. Hence, the three items represent one of the fundamental issues of quantum physics: wave-particle duality.
8. Conclusions
- aimed at engineering university students, and
- designed to introduce these students to quantum physics and modern quantum technologies via quantum optics experiments with heralded photons.
8.1. Limitations
8.1.1. Limitations of this Study
8.1.2. Limitations Related to the Quantum Optics Concept Inventory
- (i)
- intelligible wording,
- (ii)
- coverage of overarching concepts of quantum optics in the heralded photon realm, rather than addressing all the details of a topic,
- (iii)
- subject-specific correctness.
8.2. Outlook
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. The Quantum Optics Concept Inventory
- (a)
- is employed in the Michelson interferometer, because it can be used to split an incident ray of light into two partial beams.
- (b)
- is made out of two merged prisms, where one of them is responsible for the transmitted beam and one for the reflected beam.
- *(c)
- separates incident rays of light or superimposes two rays of light.
- (a)
- two mirrors can be used to deflect the laser on this line.
- *(b)
- two apertures can be used to define two points laying on this line.
- (c)
- two lenses can be used to focus the laser beam on this line.
- *(a)
- light is emitted by the crystal.
- (b)
- the laser beam is divided in two beams.
- (c)
- diffraction leads to laser light forming a cone after passing the crystal.
- (a)
- electrons are bigger than photons, leading to technical difficulties.
- (b)
- conducting the double-slit experiment one after the other with single electrons does not lead to an interference pattern.
- *(c)
- experiments with single electrons require a vacuum.
- *(a)
- the intensity of the light falling on the eye in the single-photon regime ist too low.
- (b)
- the eye can only perceive light at specific wavelengths, but not those of single photons.
- (c)
- the photons are too small to be resolved with human eyes.
- (a)
- the more photons are emitted.
- (b)
- the longer the wavelength of the emitted light.
- *(c)
- the shorter the wavelength of the emitted light.
- (a)
- half the wavelength of the incident laser light.
- (b)
- the same wavelength of the incident light of the laser.
- *(c)
- double the wavelength of the incident light of the laser.
- (a)
- count the number of registered photons within a certain time interval.
- *(b)
- lead to electron avalanches when some kind of energy portion is registered.
- (c)
- point to the detection of a single-photon with each click.
- *(a)
- mark simultaneous clicks at two or more detectors.
- (b)
- represent a measure of the detectors’ dark count rate.
- (c)
- can each be associated with the detection of a single-photon state.
- (a)
- to show that photons are physical entities with finite size.
- (b)
- in order to experiment with two single-photons simultaneously.
- *(c)
- for single-photon detections.
- *(a)
- an interference pattern with minima and maxima can be observed.
- (b)
- two well-defined detection zones can be observed.
- (c)
- two well-defined detection zones and a zero-order maximum can be observed.
- (a)
- the single-photon follows a specific path, regardless of whether I observe this path or not.
- (b)
- the current position of a photon between source and detector is not indeterminate in principle, but unknown to the experimenter.
- *(c)
- the photon behaves like a particle and like a wave. It is none of them.
- *(a)
- No one can say with certainty at which output port of the beam splitter cube a single-photon will be detected.
- (b)
- A single-photon state is divided at the beam splitter cube.
- (c)
- In the interferometer, the single-photon state is found in a superposition state of both, particle and wave.
- (a)
- more coincident events are detected between the outputs of the beam splitter than can be expected at random.
- *(b)
- a single-photon may only be detected once.
- (c)
- a single photon can be in both states, ’reflected’ and ’transmitted’, at the same time.
- *(a)
- exactly two single-photon detectors.
- (b)
- at least one single-photon detector.
- (c)
- at least three single-photon detectors.
- (a)
- spherical entities moving along a wavy path with the speed of light.
- (b)
- elementary energy portions of light surrounded by a wave that is responsible for interference.
- *(c)
- indivisible energy portions of light that are never detected on both output ports of a beam splitter cube simultaneously.
Appendix B. Overview of the Four-Week Program on Introductory Quantum Optics
Appendix B.1. Part I: Foundations of Quantum Optics Experiments with Heralded Photons
- Second, the study participants were introduced to the properties of avalanche photodiodes operating above their breakdown voltage—also referred to as single-photon avalanche diode (SPAD) [95,96]: For example, quantum efficiency, dark count rate, and dead time [68] were covered. SPADs are binary detectors, which means that the “outcome of these APD’s is either ‘off’ (no photons detected) or ‘on,’ i.e., a ‘click,’ indicating the detection of one or more photons” [97] (p. 1).
- Third, the students were introduced to spontaneous parametric downconversion (PDC) [98], a quantum electrodynamic process in which “an incoming pump photon decays, under energy and momentum conservation, into a photon-pair” [99] (p. 351). Here, the students learned that a) PDC is driven by irradiating a nonlinear crystal (e.g., -barium borate) with a pump beam, most often emitted by a laser, and that b) the “spectral properties of PDC states are governed by the phasematching properties of the nonlinear material, and this determines the frequencies of the downconverted photons” [100] (p. 3442). For an comprehensive overview of parametric downconversion, we refer the reader to [101].
Appendix B.2. Part II: Experiments with Heralded Photons
- First, the students learned about heralded single-photon state preparation, which is widely used in quantum optics research [102,103,104,105]). That is, one of the PDC photons is detected and heralds the second photon at a spatially separated detector—hence, simultaneous clicks at two detectors, also referred to as a coincident events, are “taken as preparation and detection of a single photon state” [37] (p. 348).
- Second, heralded single-photon states incident on a 50:50 beam splitter were investigated. In this experiment, the lack of coincident events at the output ports of the beam splitter, i.e., photon antibunching [106], was discussed. This anticorrelation effect is irreconcilable with any classical description of light: “A single photon can only be detected once” [67] (p. 173). These observations were further substantiated on a formal level a) by means of the second-order correlation function , which allows for a judgement of the purity of single-photon states [94], and b) by highlighting the ideas of quantum superposition and quantum random, as has been achieved in [31].
- The investigation and quantum description of Grangier et al.’s experiment from 1986 [67] represents the last step of the course. In this experiment, the students realized that by using only one single-photon state, the anticorrelation at a 50:50 beam splitter appeared simultaneously to the single-photon interference observed in a spatially separated interferometer (e.g., a Michelson interferometer) using the same single-photon state in the same experimental set-up. The students experienced that the “quantum interference phenomenon shown experimentally is a consequence of the interplay of superposition and nonlocality” [64] (p. 17), while the idea of the photon as a localizable particle is not valid. Instead, in this course, the photon was introduced as an elementary field mode excitation in the sense of quantum electrodynamics [83,84,107,108].
- In the outlook, the engineering students were given first insights into quantum technologies 2.0, namely by applying an understanding of heralded photons experiments to the context of quantum cryptography.
References
- Dowling, J.P.; Milburn, G.J. Quantum technology: The second quantum revolution. Philos. Trans. R. Soc. Lond. Ser. A Math. Phys. Eng. Sci. 2003, 361, 1655–1674. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Acín, A.; Bloch, I.; Buhrman, H.; Calarco, T.; Eichler, C.; Eisert, J.; Esteve, D.; Gisin, N.; Glaser, S.J.; Jelezko, F.; et al. The quantum technologies roadmap: A European community view. New J. Phys. 2018, 20, 080201. [Google Scholar] [CrossRef]
- Foti, C.; Anttila, D.; Maniscalco, S.; Chiofalo, M. Quantum physics literacy aimed at K12 and the general public. Universe 2021, 7, 86. [Google Scholar] [CrossRef]
- Venegas-Gomez, A. The quantum ecosystem and its future workforce: A journey through the funding, the hype, the opportunities, and the risks related to the emerging field of quantum technologies. PhotonicsViews 2020, 17, 34–38. [Google Scholar] [CrossRef]
- Asfaw, A.; Blais, A.; Brown, K.R.; Candelaria, J.; Cantwell, C.; Lincoln, D.C.; Combes, J.; Debroy, D.M.; Donohue, J.M.; Economou, S.E.; et al. Building a quantum engineering undergraduate program. IEEE Trans. Educ. 2022, 65, 220–242. [Google Scholar] [CrossRef]
- Kaur, M.; Venegas-Gomez, A. Defining the quantum workforce landscape: A review of global quantum education initiatives. Opt. Eng. 2022, 61, 081806. [Google Scholar] [CrossRef]
- Stephen Binkley, J. et al. [Subcommittee on Quantum Information Science]; Collins, F. et al. [Committee on Science]; Lander, E. et al. [National Science & Technology Council] Quantum Information Science and Technology Workforce Development National Strategic Plan; Technical Report; United States Government: Washington, DC, USA, 2022. Available online: https://www.quantum.gov/scqis-releases-strategic-plan-for-qist-workforce/ (accessed on 15 September 2022).
- National Q-12 Education Partnership. Growing the Quantum Workforce. Available online: https://q12education.org/ (accessed on 15 September 2022).
- Cervantes, B.; Passante, G.; Wilcox, B.R.; Pollock, S.J. An overview of Quantum Information Science courses at US institutions. In Proceedings of the Physics Education Research Conference 2021: 2021 PERC, Virtual, 4–5 August 2021; Bennett, M.B., Frank, B.W., Vieyra, R., Eds.; American Association of Physics Teachers: College Park, MD, USA, 2021; pp. 93–98. [Google Scholar] [CrossRef]
- Quantum Flagship. The Future Is Quantum. Available online: https://qt.eu/ (accessed on 15 September 2022).
- Quantum Technology Education. European Open Portal: Community and Resources. Available online: https://qtedu.eu/ (accessed on 15 September 2022).
- National Quantum Strategy. Available online: https://ised-isde.canada.ca/site/national-quantum-strategy/en (accessed on 15 September 2022).
- Sydney Quantum Academy. Available online: https://www.sydneyquantum.org/ (accessed on 15 September 2022).
- Quantum Technologies. Available online: https://www.psa.gov.in/technology-frontiers/quantum-technologies/346 (accessed on 15 September 2022).
- QEP: The Quantum Engineering Program. 2022. Available online: https://qepsg.org/ (accessed on 15 September 2022).
- Hughes, C.; Finke, D.; German, D.A.; Merzbacher, C.; Vora, P.M.; Lewandowski, H.J. Assessing the needs of the quantum industry. IEEE Trans. Educ. 2022. [Google Scholar] [CrossRef]
- Aiello, C.D.; Awschalom, D.D.; Bernien, H.; Brower, T.; Brown, K.R.; Brun, T.A.; Caram, J.R.; Chitambar, E.; Felice, R.D.; Edmonds, K.M.; et al. Achieving a quantum smart workforce. Quant. Sci. Technol. 2021, 6, 030501. [Google Scholar] [CrossRef]
- Fox, M.F.J.; Zwickl, B.M.; Lewandowski, H.J. Preparing for the quantum revolution: What is the role of higher education? Phys. Rev. Phys. Educ. Res. 2020, 16, 020131. [Google Scholar] [CrossRef]
- Gerke, F.; Müller, R.; Bitzenbauer, P.; Ubben, M.; Weber, K.A. Requirements for future quantum workforce—A Delphi study. J. Phys. Conf. Ser. 2022, 2297, 012017. [Google Scholar] [CrossRef]
- Greinert, F.; Müller, R.; Bitzenbauer, P.; Ubben, M.S.; Weber, K.A. The future quantum workforce: Competences, requirements and forecasts. arXiv 2022, arXiv:2208.08249. [Google Scholar] [CrossRef]
- Greinert, F.; Müller, R. Competence Framework for Quantum Technologies: Methodology and Version History; European Commission: Brussels, Belgium, 2021. [Google Scholar] [CrossRef]
- Moody, G.; Sorger, V.J.; Blumenthal, D.J.; Juodawlkis, P.W.; Loh, W.; Sorace-Agaskar, C.; Jones, A.E.; Balram, K.C.; Matthews, J.C.F.; Laing, A.; et al. 2022 Roadmap on integrated quantum photonics. J. Phys. Photonics 2022, 4, 012501. [Google Scholar] [CrossRef]
- Leibniz Universität Hannover. Quantum Engineering (Master of Science). Available online: https://tinyurl.com/bdftkpec (accessed on 15 September 2022).
- Rainò, G.; Novotny, L.; Frimmer, M. Quantum engineers in high demand. Nat. Mater. 2021, 20, 1449. [Google Scholar] [CrossRef]
- Universität Stuttgart. Photonic Engineering. Master of Science. Available online: https://www.uni-stuttgart.de/en/study/study-programs/Photonic-Engineering-M.Sc-00001./ (accessed on 15 September 2022).
- Princeton UniversityElectrical and Computer Engineering. Redesigned Quantum Optics Course Brings Applications to the Heart of the Experience. Available online: https://tinyurl.com/4uw4j7nn (accessed on 15 September 2022).
- Duarte, F. Quantum Optics for Engineers; CRC Press: Boca Raton, FL, USA, 2017. [Google Scholar] [CrossRef]
- O’Brien, J.L. Optical Quantum Computing. Science 2007, 318, 1567–1570. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- von Helversen, M.; Böhm, J.; Schmidt, M.; Gschrey, M.; Schulze, J.H.; Strittmatter, A.; Rodt, S.; Beyer, J.; Heindel, T.; Reitzenstein, S. Quantum metrology of solid-state single-photon sources using photon-number-resolving detectors. New J. Phys. 2019, 21, 035007. [Google Scholar] [CrossRef] [Green Version]
- Förtsch, M.; Fürst, J.U.; Wittmann, C.; Strekalov, D.; Aiello, A.; Chekhova, M.V.; Silberhorn, C.; Leuchs, G.; Marquardt, C. A versatile source of single photons for quantum information processing. Nat. Commun. 2013, 4, 1818. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bronner, P.; Strunz, A.; Silberhorn, C.; Meyn, J.P. Demonstrating quantum random with single photons. Eur. J. Phys. 2009, 30, 1189–1200. [Google Scholar] [CrossRef]
- Pearson, B.J.; Jackson, D.P. A hands-on introduction to single photons and quantum mechanics for undergraduates. Am. J. Phys. 2010, 78, 1422. [Google Scholar] [CrossRef]
- Marshman, E.; Singh, C. Investigating and improving student understanding of quantum mechanics in the context of single photon interference. Phys. Rev. Phys. Educ. Res. 2017, 13, 010117. [Google Scholar] [CrossRef] [Green Version]
- Bitzenbauer, P. Effect of an introductory quantum physics course using experiments with heralded photons on preuniversity students’ conceptions about quantum physics. Phys. Rev. Phys. Educ. Res. 2021, 17, 020103. [Google Scholar] [CrossRef]
- Galvez, E.J.; Holbrow, C.H.; Pysher, M.J.; Martin, J.W.; Courtemanche, N.; Heilig, L.; Spencer, J. Interference with correlated photons: Five quantum mechanics experiments for undergraduates. Am. J. Phys. 2005, 73, 127–140. [Google Scholar] [CrossRef] [Green Version]
- Thorn, J.J.; Neel, M.S.; Donato, V.W.; Bergreen, G.S.; Davies, R.E.; Beck, M. Observing the quantum behavior of light in an undergraduate laboratory. Am. J. Phys. 2004, 72, 1210–1219. [Google Scholar] [CrossRef] [Green Version]
- Bronner, P.; Strunz, A.; Silberhorn, C.; Meyn, J.P. Interactive screen experiments with single photons. Eur. J. Phys. 2009, 30, 345–353. [Google Scholar] [CrossRef]
- Bitzenbauer, P. Practitioners’ views on new teaching material for introducing quantum optics in secondary schools. Phys. Educ. 2021, 56, 055008. [Google Scholar] [CrossRef]
- Scholz, R.; Friege, G.; Weber, K.A. Undergraduate quantum optics: Experimental steps to quantum physics. Eur. J. Phys. 2018, 39, 055301. [Google Scholar] [CrossRef]
- Bitzenbauer, P. Quantum physics education research over the last two decades: A bibliometric analysis. Educ. Sci. 2021, 11, 699. [Google Scholar] [CrossRef]
- Krijtenburg-Lewerissa, K.; Pol, H.; Brinkman, A.; van Joolingen, W. Insights into teaching quantum mechanics in secondary and lower undergraduate education. Phys. Rev. Phys. Educ. Res. 2017, 13, 010109. [Google Scholar] [CrossRef]
- Wuttiprom, S.; Sharma, M.D.; Johnston, I.D.; Chitaree, R.; Soankwan, C. Development and use of a conceptual survey in introductory quantum physics. Int. J. Sci. Educ. 2009, 31, 631–654. [Google Scholar] [CrossRef]
- McKagan, S.B.; Perkins, K.K.; Wieman, C.E. Design and validation of the Quantum Mechanics Conceptual Survey. Phys. Rev. Phys. Educ. Res. 2010, 6, 020121. [Google Scholar] [CrossRef] [Green Version]
- di Uccio, U.S.; Colantonio, A.; Galano, S.; Marzoli, I.; Trani, F.; Testa, I. Design and validation of a two-tier questionnaire on basic aspects in quantum mechanics. Phys. Rev. Phys. Educ. Res. 2019, 15, 010137. [Google Scholar] [CrossRef] [Green Version]
- Müller, R.; Wiesner, H. Teaching quantum mechanics on an introductory level. Am. J. Phys. 2002, 70, 200–209. [Google Scholar] [CrossRef] [Green Version]
- Cataloglu, E.; Robinett, R.W. Testing the development of student conceptual and visualization understanding in quantum mechanics through the undergraduate career. Am. J. Phys. 2002, 70, 238–251. [Google Scholar] [CrossRef]
- Goldhaber, S.; Pollock, S.; Dubson, M.; Beale, P.; Perkins, K.; Sabella, M.; Henderson, C.; Singh, C. Transforming upper-division quantum mechanics: Learning goals and assessment. AIP Conf. Proc. 2009, 1179, 145–148. [Google Scholar] [CrossRef] [Green Version]
- Marshman, E.; Singh, C. Validation and administration of a conceptual survey on the formalism and postulates of quantum mechanics. Phys. Rev. Phys. Educ. Res. 2019, 15, 020128. [Google Scholar] [CrossRef] [Green Version]
- Bitzenbauer, P. Development of a test instrument to investigate secondary school students’ declarative knowledge of quantum optics. Eur. J. Sci. Math. Educ. 2021, 9, 57–79. [Google Scholar] [CrossRef]
- Singh, C.; Marshman, E. Review of student difficulties in upper-level quantum mechanics. Phys. Rev. Phys. Educ. Res. 2015, 11, 020117. [Google Scholar] [CrossRef] [Green Version]
- Bitzenbauer, P.; Meyn, J.P. A new teaching concept on quantum physics in secondary schools. Phys. Educ. 2020, 55, 055031. [Google Scholar] [CrossRef]
- Bitzenbauer, P.; Meyn, J.P. Fostering students’ conceptions about the quantum world—Results of an interview study. Prog. Sci. Educ. 2021, 4, 40–51. [Google Scholar] [CrossRef]
- Ubben, M.S.; Bitzenbauer, P. Two cognitive dimensions of students’ mental models in science: Fidelity of gestalt and functional fidelity. Educ. Sci. 2022, 12, 163. [Google Scholar] [CrossRef]
- Waitzmann, M.; Scholz, R.; Weßnigk, S. Wirkung eines Realexperiments auf quantenphysikalische Argumentation. Unsicherheit als Element von naturwissenschaftsbezogenen Bildungsprozessen. In Proceedings of the Jahrestagung der Gesellschaft für Didaktik der Chemie und Physik, Online, 13–16 September 2021; Universität Duisburg-Essen: Duisburg, Germany, 2022; p. 42. [Google Scholar]
- Bitzenbauer, P. Quantenoptik an Schulen. Studie im Mixed-Methods Design zur Evaluation des Erlanger Unterrichtskonzepts zur Quantenoptik; Logos Verlag: Berlin, Germany, 2020. [Google Scholar] [CrossRef]
- Ireson, G. The quantum understanding of pre-university physics students. Phys. Educ. 2000, 35, 15–21. [Google Scholar] [CrossRef]
- Ireson, G. A multivariate analysis of undergraduate physics students’ conceptions of quantum phenomena. Eur. J. Phys. 1999, 20, 193–199. [Google Scholar] [CrossRef]
- Donhauser, A.; Bitzenbauer, P.; Meyn, J.P. Von Schnee- und Elektronenlawinen: Entwicklung eines Erklärvideos zu Einzelphotonendetektoren. PhyDid B—Didaktik der Physik—Beiträge zur DPG-Frühjahrstagung 2020, Bonn, 235–240. Available online: https://ojs.dpg-physik.de/index.php/phydid-b/article/view/1021 (accessed on 15 September 2022).
- Aslanides, J.S.; Savage, C.M. Relativity concept inventory: Development, analysis, and results. Phys. Rev. Phys. Educ. Res. 2013, 9, 010118. [Google Scholar] [CrossRef] [Green Version]
- Veith, J.M.; Bitzenbauer, P.; Girnat, B. Assessing learners’ conceptual understanding of introductory group theory using the CI2GT: Development and analysis of a concept inventory. Educ. Sci. 2022, 12, 376. [Google Scholar] [CrossRef]
- Urlacher, M.A.; Brown, S.A.; Steif, P.S.; Bornasal, F.B. Practicing civil engineers’ understanding of statics concept inventory questions. In Proceedings of the 2015 ASEE Annual Conference and Exposition, Seattle, WA, USA, 14–17 June 2015; pp. 26.1236.1–26.1236.12. [Google Scholar] [CrossRef] [Green Version]
- Hasan, S.; Bagayoko, D.; Kelley, E.L. Misconceptions and the Certainty of Response Index (CRI). Phys. Educ. 1999, 34, 294–299. [Google Scholar] [CrossRef]
- Bitzenbauer, P.; Meyn, J.P. Inhaltsvalidität eines Testinstruments zur Erfassung deklarativen Wissens zur Quantenoptik. PhyDid B—Didaktik der Physik—Beiträge zur DPG-Frühjahrstagung 2020, Bonn, 149–156. Available online: https://ojs.dpg-physik.de/index.php/phydid-b/article/view/1022 (accessed on 15 September 2022).
- Scholz, R.; Wessnigk, S.; Weber, K.A. A classical to quantum transition via key experiments. Eur. J. Phys. 2020, 41, 055304. [Google Scholar] [CrossRef]
- Kohnle, A.; Rizzoli, A. Interactive simulations for quantum key distribution. Eur. J. Phys. 2017, 38, 035403. [Google Scholar] [CrossRef] [Green Version]
- Bloom, Y.; Fields, I.; Maslennikov, A.; Rozenman, G.G. Quantum cryptography—A simplified undergraduate experiment and simulation. Physics 2022, 4, 104–123. [Google Scholar] [CrossRef]
- Grangier, P.; Roger, G.; Aspect, A. Experimental evidence for a photon anticorrelation effect on a beam splitter: A new light on single-photon interferences. Europhys. Lett. 1986, 1, 173–179. [Google Scholar] [CrossRef] [Green Version]
- Silberhorn, C. Detecting quantum light. Contemp. Phys. 2007, 48, 143–156. [Google Scholar] [CrossRef]
- Kline, T. Psychological Testing: A Practical Approach to Design and Evaluation; SAGE Publications, Inc.: Thousand Oaks, CA, USA, 2005. [Google Scholar]
- Jorion, N.; Gane, B.D.; James, K.; Schroeder, L.; DiBello, L.V.; Pellegrino, J.W. An analytic framework for evaluating the validity of concept inventory claims. J. Eng. Educ. 2015, 104, 454–496. [Google Scholar] [CrossRef]
- Taber, K.S. The Use of Cronbach’s alpha when developing and reporting research instruments in science education. Res. Sci. Educ. 2018, 48, 1273–1296. [Google Scholar] [CrossRef] [Green Version]
- Cantó-Cerdán, M.; Cacho-Martínez, P.; Lara-Lacárcel, F.; García-Muñoz, Á. Rasch analysis for development and reduction of Symptom Questionnaire for Visual Dysfunctions (SQVD). Sci. Rep. 2021, 11, 14855. [Google Scholar] [CrossRef] [PubMed]
- Winter-Hölzl, A.; Wäschle, K.; Wittwer, J.; Watermann, R.; Nückles, M. Entwicklung und Validierung eines Tests zur Erfassung des Genrewissens Studierender und Promovierender der Bildungswissenschaften. Zeit. Pädag. 2015, 61, 185–202. [Google Scholar] [CrossRef]
- Planinic, M.; Boone, W.J.; Susac, A.; Ivanjek, L. Rasch analysis in physics education research: Why measurement matters. Phys. Rev. Phys. Educ. Res. 2019, 15, 020111. [Google Scholar] [CrossRef] [Green Version]
- Rizopoulos, D. Package ’ltm’. 18 February 2022. Available online: https://cran.r-project.org/web/packages/ltm/ltm.pdf (accessed on 15 September 2022).
- Robitzsch, A.; Kiefer, A.; Wu, M. Package ’TAM’. 28 August 2022. Available online: https://cran.r-project.org/web/packages/TAM/TAM.pdf (accessed on 15 September 2022).
- Mair, P.; Hatzinger, R.; Maier, M.J.; Rusch, T.; Debelak, R. Package ’eRm’. 15 February 2022. Available online: https://cran.r-project.org/web/packages/eRm/eRm.pdf (accessed on 15 September 2022).
- Matejak Cvenic, K.; Planinic, M.; Susac, A.; Ivanjek, L.; Jelicic, K.; Hopf, M. Development and validation of the Conceptual Survey on Wave Optics. Phys. Rev. Phys. Educ. Res. 2022, 18, 010103. [Google Scholar] [CrossRef]
- Chen, W.-H.; Thissen, D. Local Dependence indexes for item pairs using item response theory. J. Educ. Behav. Stat. 1997, 22, 265–289. [Google Scholar] [CrossRef]
- Christensen, K.B.; Makransky, G.; Horton, M. Critical values for Yen’s Q3: Identification of local dependence in the Rasch model using residual correlations. App. Psych. Meas. 2017, 41, 178–194. [Google Scholar] [CrossRef]
- Jang, E.E.; Roussos, L. An Investigation into the dimensionality of TOEFL using conditional covariance-based nonparametric approach. J. Educ. Meas. 2007, 44, 1–21. [Google Scholar] [CrossRef]
- Susac, A.; Planinic, M.; Klemencic, D.; Milin Sipus, Z. Using the Rasch model to analyze the test of understanding of vectors. Phys. Rev. Phys. Educ. Res. 2018, 14, 023101. [Google Scholar] [CrossRef] [Green Version]
- Jones, D.G.C. Teaching modern physics-misconceptions of the photon that can damage understanding. Phys. Educ. 1991, 26, 93–98. [Google Scholar] [CrossRef]
- Kidd, R.; Ardini, J.; Anton, A. Evolution of the modern photon. Am. J. Phys. 1989, 57, 27–35. [Google Scholar] [CrossRef]
- Nousiainen, M.; Koponen, I.T. Pre-service teachers’ declarative knowledge of wave-particle dualism of electrons and photons: Finding lexicons by using network analysis. Educ. Sci. 2020, 10, 76. [Google Scholar] [CrossRef] [Green Version]
- Hoehn, J.R.; Gifford, J.D.; Finkelstein, N.D. Investigating the dynamics of ontological reasoning across contexts in quantum physics. Phys. Rev. Phys. Educ. Res. 2019, 15, 010124. [Google Scholar] [CrossRef] [Green Version]
- Kalkanis, G.; Hadzidaki, P.; Stavrou, D. An instructional model for a radical conceptual change towards quantum mechanics concepts. Sci. Educ. 2003, 87, 257–280. [Google Scholar] [CrossRef]
- Veith, J.M.; Girnat, B.; Bitzenbauer, P. The role of affective learner characteristics for learning about abstract algebra: A multiple linear regression analysis. EURASIA J. Math. Sci. Technol. Educ. 2022, 18, em2157. [Google Scholar] [CrossRef]
- Rasch, G. On general laws and the meaning of measurement in psychology. In Proceedings of the Fourth Berkeley Symposium on Mathematical Statistics and Probability. Volume 4: Contributions to Biology and Problems of Medicine, Berkeley, CA, USA, 20 June–30 July 1960; Neyman, J., Ed.; University of California Press: Berkeley/Los Angeles, CA, USA, 1961; pp. 321–333. Available online: https://projecteuclid.org/Proceedings/berkeley-symposium-on-mathematical-statistics-and-probability/proceedings-of-the-fourth-berkeley-symposium-on-mathematical-statistics-and/toc/bsmsp/1200512872 (accessed on 15 September 2022).
- Pant, H.A.; Tiffin-Richards, S.P.; Köller, O. Standard-Setting für Kompetenztests im Large-Scale-Assessment. Projekt Standardsetting. Zeit. Pädag. 2010, 56, 175–188. [Google Scholar] [CrossRef]
- Duncan, R.G.; Hmelo-Silver, C.E. Learning progressions: Aligning curriculum, instruction, and assessment. J. Res. Sci. Teach. 2009, 46, 606–609. [Google Scholar] [CrossRef]
- Wright, B.D.; Masters, G.N. Rating Scale Analysis; MESA Press: Chicago, IL, USA, 1982. [Google Scholar]
- Prasad, S.; Scully, M.O.; Martienssen, W. A quantum description of the beam splitter. Opt. Comm. 1987, 62, 139–145. [Google Scholar] [CrossRef]
- Loudon, R. The Quantum Theory of Light; Oxford University Press: Oxford, UK, 2000. [Google Scholar]
- Cova, S.; Longoni, A.; Andreoni, A. Towards picosecond resolution with single-photon avalanche diodes. Rev. Sci. Instr. 1981, 52, 408–412. [Google Scholar] [CrossRef] [Green Version]
- Cova, S.; Ghioni, M.; Lacaita, A.; Samori, C.; Zappa, F. Avalanche photodiodes and quenching circuits for single-photon detection. Appl. Opt. 1996, 35, 1956–1976. [Google Scholar] [CrossRef] [PubMed]
- Zambra, G.; Andreoni, A.; Bondani, M.; Gramegna, M.; Genovese, M.; Brida, G.; Rossi, A.; Paris, M.G.A. Experimental reconstruction of photon statistics without photon counting. Phys. Rev. Lett. 2005, 95, 063602. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hong, C.K.; Mandel, L. Theory of parametric frequency down conversion of light. Phys. Rev. A 1985, 31, 2409–2418. [Google Scholar] [CrossRef] [PubMed]
- Christ, A.; Fedrizzi, A.; Hüel, H.; Jennewein, T.; Silberhorn, C. Parametric down-conversion. In Single-Photon Generation and Detection. Physics and Applications; Migdall, A., Polyakov, S.V., Fan, J., Bienfang, J.C., Eds.; Elsevier: Amsterdam, The Netherlands, 2013; pp. 351–410. [Google Scholar] [CrossRef]
- Christ, A.; Eckstein, A.; Mosley, P.J.; Silberhorn, C. Pure single photon generation by type-I PDC with backward-wave amplification. In Proceedings of the European Conference on Lasers and Electro-Optics and the European Quantum Electronics Conference: CLEO Europe/EQEC 2009 (Abstracts), Munich, Germany, 14–19 June 2009; IEEE: Piscataway, NJ, USA, 2009; p. 917. [Google Scholar] [CrossRef]
- Couteau, C. Spontaneous parametric down-conversion. Contemp. Phys. 2018, 59, 291–304. [Google Scholar] [CrossRef]
- Brida, G.; Degiovanni, I.P.; Genovese, M.; Migdall, A.; Piacentini, F.; Polyakov, S.V.; Ruo Berchera, I. Experimental realization of a low-noise heralded single-photon source. Opt. Express 2011, 19, 1484–1492. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lounis, B.; Orrit, M. Single-photon sources. Rep. Prog. Phys. 2005, 68, 1129–1179. [Google Scholar] [CrossRef]
- Krapick, S.; Herrmann, H.; Quiring, V.; Brecht, B.; Suche, H.; Silberhorn, C. An efficient integrated two-color source for heralded single photons. New J. Phys. 2013, 15, 033010. [Google Scholar] [CrossRef] [Green Version]
- Genovese, M.; Gramegna, M.; Brida, G.; Bondani, M.; Zambra, G.; Andreoni, A.; Rossi, A.R.; Paris, M.G.A. Measuring the photon distribution with ON/OFF photodetectors. Laser Phys. 2006, 16, 385–392. [Google Scholar] [CrossRef] [Green Version]
- Kimble, H.J.; Dagenais, M.; Mandel, L. Photon antibunching in resonance fluorescence. Phys. Rev. Lett. 1977, 39, 691–695. [Google Scholar] [CrossRef] [Green Version]
- Hobson, A. There are no particles, there are only fields. Am. J. Phys. 2013, 81, 211–223. [Google Scholar] [CrossRef] [Green Version]
- Strnad, J. Photons in introductory quantum physics. Am. J. Phys. 1986, 54, 650–652. [Google Scholar] [CrossRef]

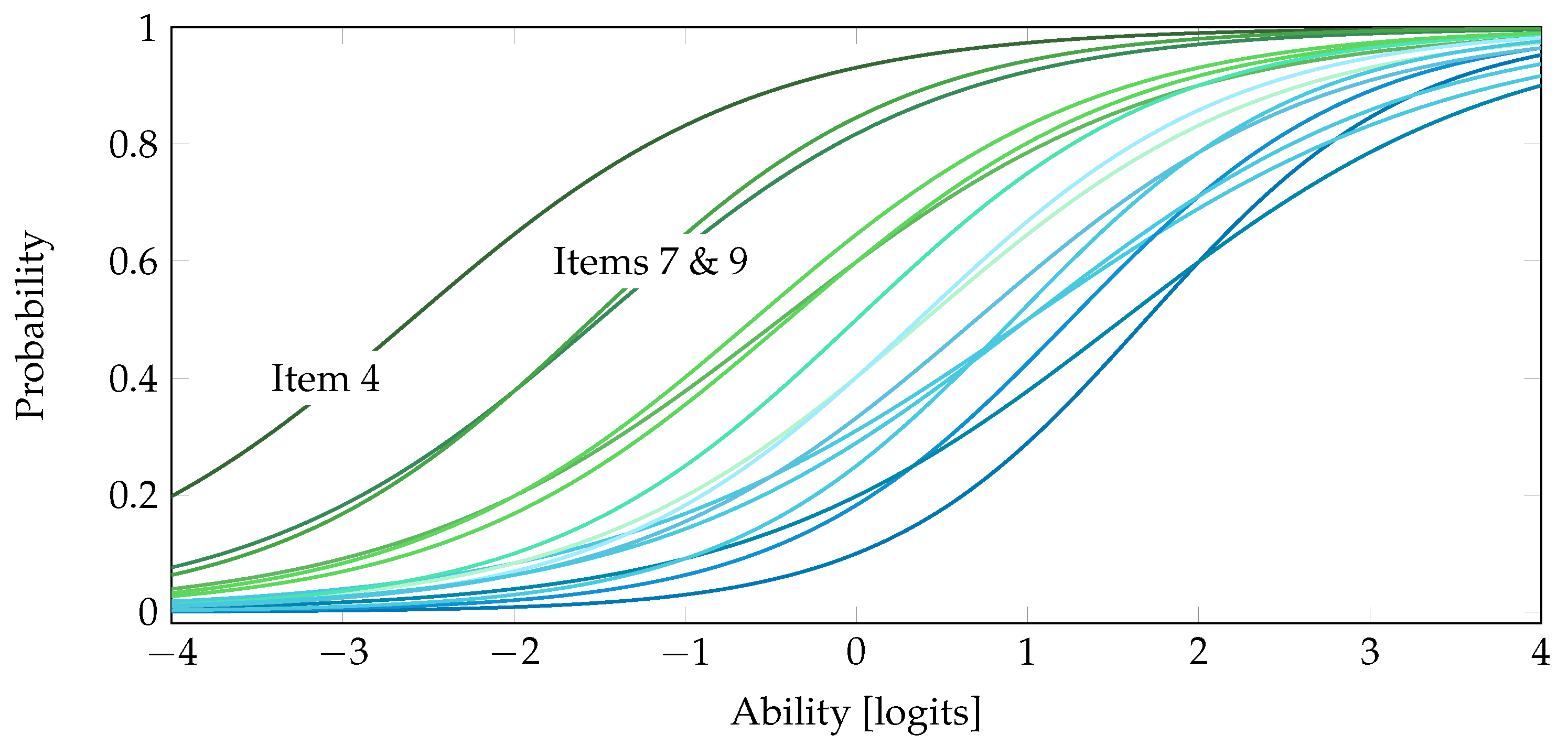

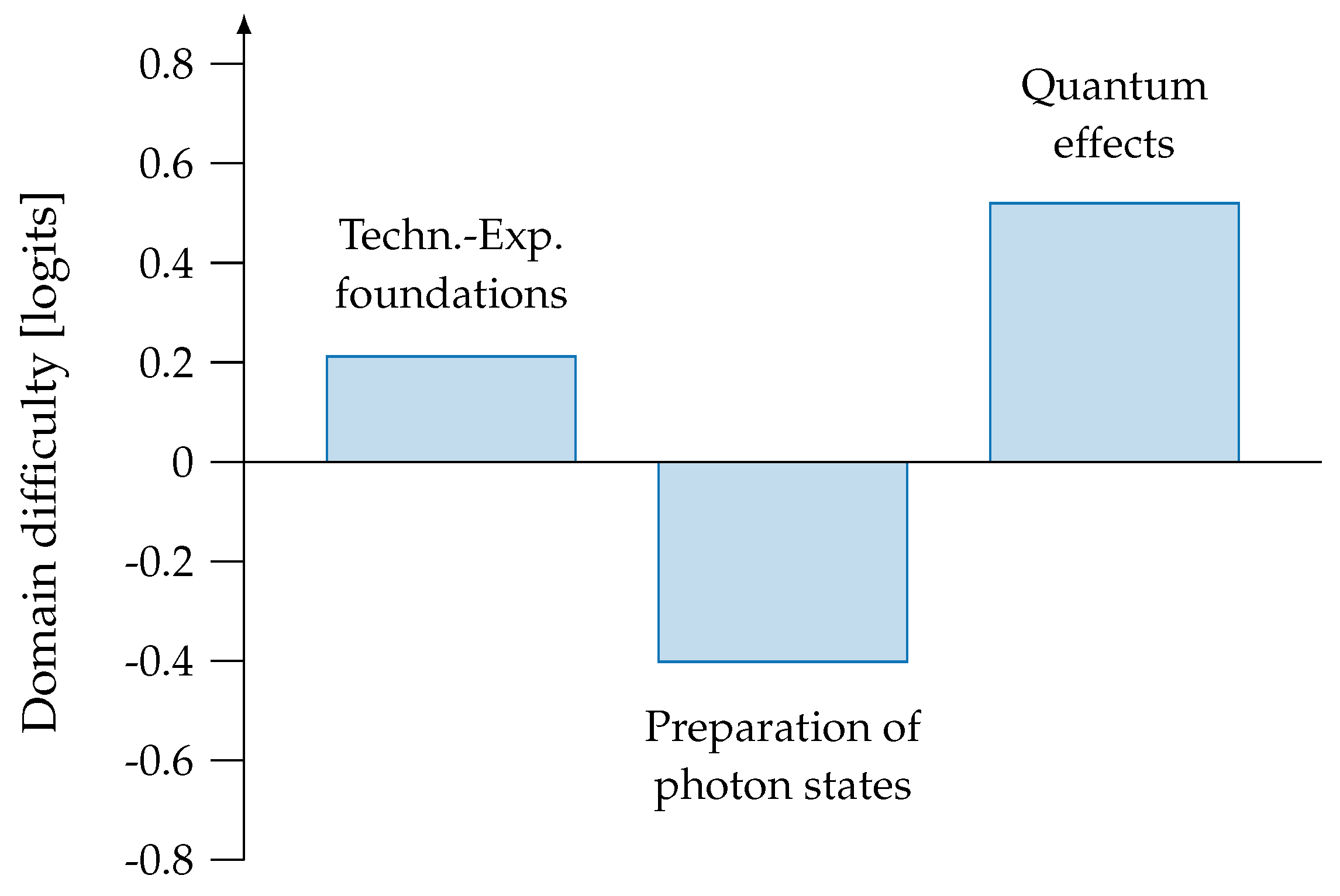
| Content Domain | No. of Items | Topics Covered (Related Items) |
|---|---|---|
| Technical-experimental foundations | 5 | Set-up/adjustment of quantum optical experiment (items 1, 2, and 4), Single-photon detection (items 5 and 8) |
| Preparation of single-photon states | 6 | Parametric downconversion and energy conservation (items 3, 6, and 7), Coincidence technique (items 9, 10, and 15) |
| Quantum effects | 5 | Interference of single quanta (items 11, 12, and 13), Anticorrelation of single-photon states (items 14 and 16) |
| Item No. | Difficulty | Point-Biserial | |
|---|---|---|---|
| 1 | 0.15 | 0.35 | 0.73 |
| 2 | 0.37 | 0.30 | 0.73 |
| 3 | 0.24 | 0.46 | 0.72 |
| 4 | 0.91 | 0.22 | 0.74 |
| 5 | 0.43 | 0.48 | 0.72 |
| 6 | 0.63 | 0.39 | 0.73 |
| 7 | 0.82 | 0.33 | 0.73 |
| 8 | 0.37 | 0.29 | 0.74 |
| 9 | 0.80 | 0.21 | 0.74 |
| 10 | 0.59 | 0.20 | 0.75 |
| 11 | 0.54 | 0.34 | 0.73 |
| 12 | 0.20 | 0.34 | 0.73 |
| 13 | 0.35 | 0.44 | 0.72 |
| 14 | 0.32 | 0.31 | 0.73 |
| 15 | 0.35 | 0.34 | 0.73 |
| 16 | 0.61 | 0.36 | 0.73 |
| Item No. | Skewness | Kurtosis | Difficulty | SE | Infit | Outfit |
|---|---|---|---|---|---|---|
| 1 | 1.99 | 2.00 | 2.096 | 0.206 | 0.929 | 0.916 |
| 2 | 0.54 | −1.72 | 0.648 | 0.156 | 1.056 | 1.042 |
| 3 | 1.22 | −0.51 | 1.393 | 0.174 | 0.902 | 0.772 |
| 4 | −2.83 | 6.07 | −2.697 | 0.247 | 0.985 | 0.873 |
| 5 | 0.30 | −1.93 | 0.364 | 0.152 | 0.902 | 0.889 |
| 6 | −0.54 | −1.72 | −0.652 | 0.155 | 0.951 | 1.139 |
| 7 | −1.63 | 0.67 | −1.787 | 0.189 | 0.956 | 0.952 |
| 8 | 0.54 | −1.72 | 0.648 | 0.156 | 1.079 | 1.048 |
| 9 | −1.48 | 0.20 | −1.649 | 0.183 | 1.080 | 1.109 |
| 10 | −0.38 | −1.87 | −0.462 | 0.153 | 1.148 | 1.197 |
| 11 | −0.15 | −2.00 | −0.184 | 0.151 | 1.022 | 1.002 |
| 12 | 1.48 | 0.20 | 1.647 | 0.183 | 0.987 | 0.907 |
| 13 | 0.63 | −1.62 | 0.745 | 0.157 | 0.924 | 0.931 |
| 14 | 0.80 | −1.37 | 0.948 | 0.161 | 1.028 | 1.003 |
| 15 | 0.63 | −1.62 | 0.745 | 0.157 | 1.017 | 0.973 |
| 16 | −0.46 | −1.81 | −0.556 | 0.154 | 1.005 | 0.967 |
| Analysis | Excellent | Good | Average | Poor | QOCI | ||
|---|---|---|---|---|---|---|---|
| Classical Test theory | |||||||
| Item Statistics | |||||||
| Difficulty | – | – (3) | – | – (3) | good | ||
| Discrimination | >0.2 | >0.1 | >0 | >−0.2 | excellent | ||
| Total score reliability | |||||||
| of total score | >0.9 | >0.8 | >0.65 | >0.5 | average | ||
| -with-item-deleted | All items less than overall | (3) | (6) | (9) | good | ||
| Item Response Theory | |||||||
| Individual item measures | |||||||
| Infit MNSQ | – | – | – | – | excellent | ||
| Outfit MNSQ | – | – | – | – | excellent | ||
| All items fit the model | (2) | (4) | (6) | (8) | excellent | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bitzenbauer, P.; Veith, J.M.; Girnat, B.; Meyn, J.-P. Assessing Engineering Students’ Conceptual Understanding of Introductory Quantum Optics. Physics 2022, 4, 1180-1201. https://doi.org/10.3390/physics4040077
Bitzenbauer P, Veith JM, Girnat B, Meyn J-P. Assessing Engineering Students’ Conceptual Understanding of Introductory Quantum Optics. Physics. 2022; 4(4):1180-1201. https://doi.org/10.3390/physics4040077
Chicago/Turabian StyleBitzenbauer, Philipp, Joaquin M. Veith, Boris Girnat, and Jan-Peter Meyn. 2022. "Assessing Engineering Students’ Conceptual Understanding of Introductory Quantum Optics" Physics 4, no. 4: 1180-1201. https://doi.org/10.3390/physics4040077
APA StyleBitzenbauer, P., Veith, J. M., Girnat, B., & Meyn, J.-P. (2022). Assessing Engineering Students’ Conceptual Understanding of Introductory Quantum Optics. Physics, 4(4), 1180-1201. https://doi.org/10.3390/physics4040077





