Shell Model Applications in Nuclear Astrophysics †
Abstract
:1. Introduction
2. Weak Interaction Processes in Supernovae
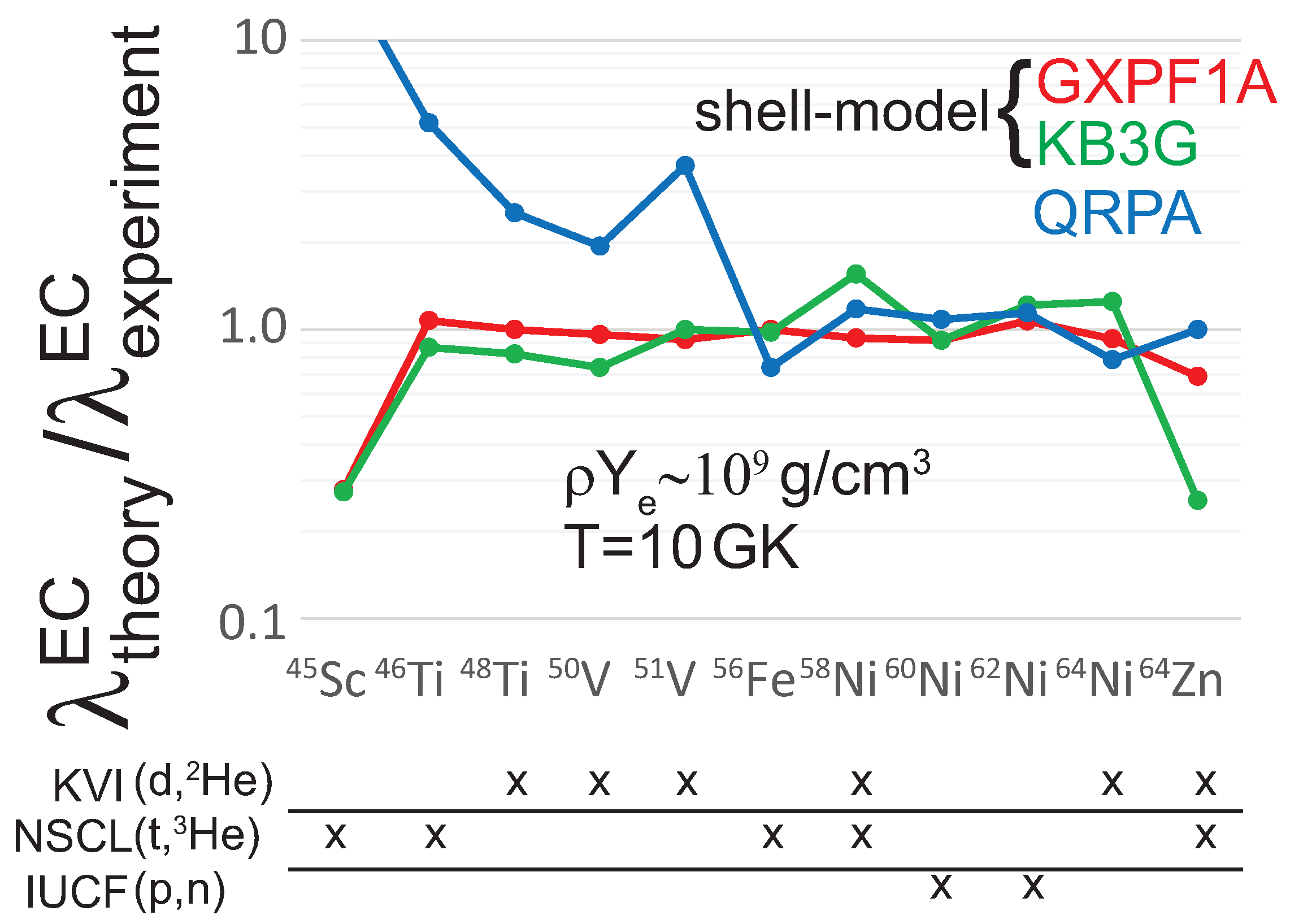
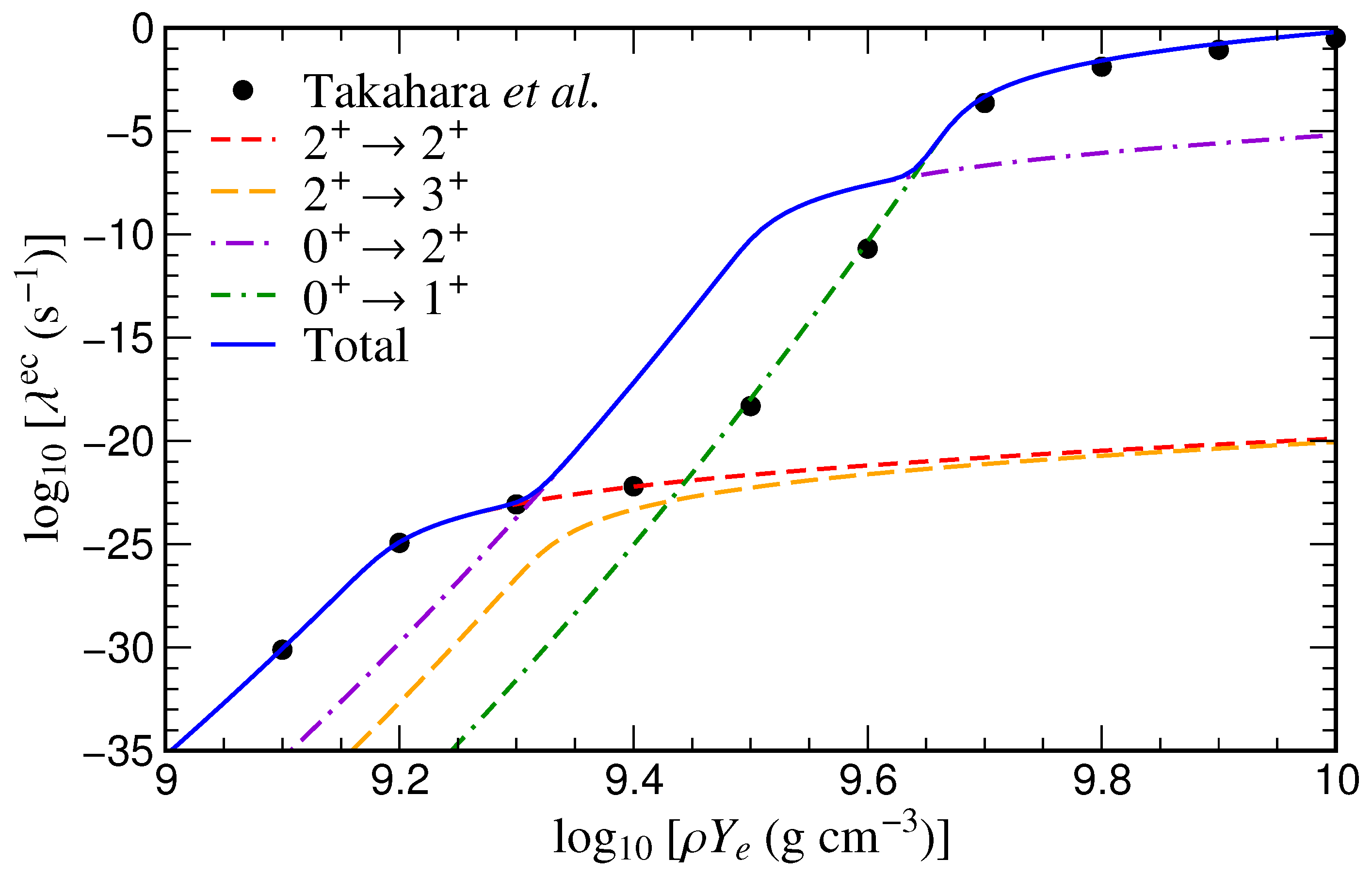
2.1. Electron Capture on Nuclei
2.2. Neutrino–Nucleus Scattering
3. r-Process Nucleosynthesis
4. Summary
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- The National Research Council. Nuclear Physics: Exploring the Heart of Matter; The National Academies Press: Washington, DC, USA, 2013; Available online: https://nap.nationalacademies.org/read/13438/ (accessed on 15 May 2022).
- Caurier, E.; Martinez-Pinedo, G.; Nowacki, F.; Poves, A.; Zuker, A.P. The shell model as a unified view of nuclear structure. Rev. Mod. Phys. 2005, 77, 427–488. [Google Scholar] [CrossRef] [Green Version]
- Coraggio, L.; Covello, A.; Gargano, A.; Itaco, N.; Kuo, T.T.S. Shell-model calculations and realistic effective interactions. Prog. Part. Nucl. Phys. 2009, 62, 135–182. [Google Scholar] [CrossRef] [Green Version]
- Langanke, K.; Martínez-Pinedo, G. Nuclear weak-interaction processes in stars. Rev. Mod. Phys. 2003, 75, 819–862. [Google Scholar] [CrossRef] [Green Version]
- Grawe, H.; Langanke, K.; Martinez-Pinedo, G. Nuclear structure and astrophysics. Rep. Prog. Phys. 2007, 70, 1525–1582. [Google Scholar] [CrossRef]
- Johnson, C.W.; Koonin, S.E.; Lang, G.H.; Ormand, W.E. Monte Carlo methods foer the nuclear shell model. Phys. Rev. Lett. 1992, 69, 3157–3160. [Google Scholar] [CrossRef] [Green Version]
- Koonin, S.E.; Dean, D.J.; Langanke, K. Shell model Monte Carlo methods. Phys. Rep. 1997, 278, 1–77. [Google Scholar] [CrossRef] [Green Version]
- Oda, T.; Hino, M.; Muto, K.; Takahara, T.; Sato, K. Rate tables for the weak processes of sd-shell nuclei in stellar matter. At. Data Nucl. Data Tables 1994, 56, 231–403. [Google Scholar] [CrossRef]
- Dean, D.J.; Langanke, K.; Chatterjee, L.; Radha, P.B.; Strayer, M.R. Electron capture on iron group nuclei. Phys. Rev. C 1998, 58, 536–544. [Google Scholar] [CrossRef] [Green Version]
- Langanke, K.; Martinez-Pinedo, G. Rate tables for the weak processes of pf-shell nuclei in stellar environments. At. Data Nucl. Data Tables 2001, 79, 1–46. [Google Scholar] [CrossRef]
- Martínez-Pinedo, G.; Lam, Y.H.; Langanke, K.; Zegers, R.G.T.; Sullivan, C. Astrophysical weak-interaction rates for selected A = 20 and A = 24 nuclei. Phys. Rev. C 2014, 89, 045806. [Google Scholar] [CrossRef] [Green Version]
- Heger, A.; Langanke, K.; Martinez-Pinedo, G.; Woosley, S.E. Presupernova collapse models with improved weak-interaction rates. Phys. Rev. Lett. 2001, 86, 1678–1681. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Heger, A.; Woosley, S.E.; Martinez-Pinedo, G.; Langanke, K. Presupernova evolution with improved rates for weak interactions. Astrophys. J. 2001, 560, 307–325. [Google Scholar] [CrossRef] [Green Version]
- Nomoto, K. Evolution of 8–10 M⊙ stars toward electron capture supernovale: II. Collapse of an O + Ne + Mg core. Astrophys. J. 1987, 322, 206–214. [Google Scholar] [CrossRef]
- Kirsebom, O.S.; Jones, S.; Strömberg, D.F.; Martínez-Pinedo, G.; Langake, K.; Röpke, F.K.; Brown, B.A.; Eronen, T.; Fynbo, H.O.U.; Hukkanen, M.; et al. Discovery of an exceptionally strong β-decay transition of 20F and the fate of intermediate-mass stars. Phys. Rev. Lett. 2019, 123, 262701. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Brachwitz, F.; Dean, D.J.; Hix, W.R.; Iwamoto, K.; Langanke, K.; Martinez-Pinedo, G.; Nomoto, F.; Strayer, M.R.; Thieleman, F.-K.; Umeda, H. The role of electron captures in Chandrasekhar-mass models for type Ia supernovae. Astrophys. J. 2000, 536, 934–947. [Google Scholar] [CrossRef] [Green Version]
- Langanke, K.; Martínez-Pinedo, G.; Sampaio, J.M.; Dean, D.J.; Hix, W.R.; Messer, O.E.B.; Mezzacappa, A.; Liebendörfer, M.; Janka, H.-T.; Rampp, M. Electron capture rates on nuclei and implications for stellar core collapse. Phys. Rev. Lett. 2003, 90, 241102. [Google Scholar] [CrossRef]
- Hix, W.R.; Messer, O.E.B.; Mezzacappa, A.; Liebendörfer, M.; Sampaio, J.; Langanke, K.; Dean, D.J.; Martínez-Pinedo, G. The consequences of nuclear electron capture in core collapse supernovae. Phys. Rev. Lett. 2003, 91, 210102. [Google Scholar] [CrossRef]
- Janka, H.-T.; Langanke, K.; Marek, A.; Martínez-Pinedo, G.; Mueller, B. Theory of core-collapse supernovae. Phys. Rep. 2007, 442, 38–74. [Google Scholar] [CrossRef] [Green Version]
- Martinez-Pinedo, G.; Langanke, K. Shell-model half-lives for the N = 82 nuclei and their implications for the r-process. Phys. Rev. Lett. 1992, 83, 4502–4505. [Google Scholar] [CrossRef] [Green Version]
- Suzuki, T.; Yoshida, T.; Kajino, T.; Otsuka, T. β decays of isotones with neutron magic number of N = 126 and r-process nucleosynthesis. Phys. Rev. C 2012, 85, 015802. [Google Scholar] [CrossRef] [Green Version]
- Zhi, Q.; Caurier, E.; Cuenca-García, J.J.; Langanke, K.; Martíz-Pinedo, G.; Sieja, K. Shell-model half-lives including first-forbidden contributions for r-process waiting-point nuclei. Phys. Rev. C 2013, 87, 025803. [Google Scholar] [CrossRef]
- Larsen, A.C.; Goriely, S.; Bernstein, L.A.; Bleuel, D.L.; Bracco, A.; Brown, B.A.; Camera, F.; Eriksen, T.K.; Frauendorf, S.; Giacoppo, F.; et al. Upbend and M1 scissors mode in neutron-rich nuclei—Consequences for r-process (n, γ) reaction rates. Acta Phys. Pol. B 2015, 46, 509–512. [Google Scholar] [CrossRef] [Green Version]
- Larsen, A.C.; Spyrou, A.; Liddick, S.N.; Guttormsen, M. Novel techniques for constraining neutron-capture rates relevant for r-process heavy-element nucleosynthesis. Prog. Part. Nucl. Phys. 2019, 107, 69–108. [Google Scholar] [CrossRef] [Green Version]
- Nakada, H.; Alhassid, Y. Total and parity-projected level densities of iron-region nuclei in the auxiliary fields Monte Carlo shell model. Phys. Rev. Lett. 1997, 79, 2939–2942. [Google Scholar] [CrossRef] [Green Version]
- Langanke, K. Shell model Monte Carlo level densities for nuclei around A ∼ 50. Phys. Lett. 1998, 438, 235–241. [Google Scholar] [CrossRef]
- Bethe, H.A. Supernova mechanisms. Rev. Mod. Phys. 1990, 62, 801–867. [Google Scholar] [CrossRef]
- Bethe, H.A.; Brown, G.E.; Applegate, J.; Lattimer, J.M. Equation of state in the gravitational collapse of stars. Nucl. Phys. A 1979, 324, 487–533. [Google Scholar] [CrossRef]
- Langanke, K.; Dean, D.J.; Radha, P.B.; Alhassid, Y.; Koonin, S.E. Shell model Monte Carlo studies of fp-shell nuclei. Phys. Rev. C 1995, 52, 718–725. [Google Scholar] [CrossRef] [Green Version]
- Martínez-Pinedo, G.; Poves, A.; Caurier, E.; Zuker, A.P. Effective gA in the pf-shell. Phys. Rev. C 1996, 53, R2602–R2605. [Google Scholar] [CrossRef] [Green Version]
- Caurier, E.; Langanke, K.; Martínez-Pinedo, G.; Nowacki, F. Shell-Model calculations of stellar weak interaction rates. I. Gamow-Teller distributions and spectra of nuclei in the mass range A = 45 − 65. Nucl. Phys. A 1999, 653, 439–452. [Google Scholar] [CrossRef] [Green Version]
- Vetterli, M.C.; Jackson, K.P.; Celler, A.; Engel, J.; Yen, S. The 70,72Ge(n, p)70,72Ga reactions: Suppression of Gamow–Teller strength near N = 40. Phys. Rev. C 1992, 45, 997–1004. [Google Scholar] [CrossRef] [PubMed]
- Frekers, D.; Alanssari, M. Charge-exchange reactions and the quest for resolution. Eur. Phys. J. A 2018, 54, 177. [Google Scholar] [CrossRef]
- Fuller, G.M.; Fowler, W.A.; Newman, M.J. Stellar weak-interaction rates for sd-shell nuclei. I. Nuclear matrix element systematics with application to 26Al and selected nuclei of importance to the supernova problem. Astrophys. J. Suppl. Ser. 1980, 42, 447–473. [Google Scholar] [CrossRef] [Green Version]
- Fuller, G.M.; Fowler, W.A.; Newman, M.J. Stellar weak interaction rates for intermediate mass nuclei. III. Rate tables for the free nucleons and nuclei with A = 21 to A = 60. Astrophys. J. Suppl. Ser. 1982, 48, 279–320. [Google Scholar] [CrossRef]
- Cole, A.L.; Anderson, T.S.; Zegers, R.G.T.; Sam, M.; Austin, B.; Brown, A.; Valdez, L.; Gupta, S.; Hitt, G.W.; Fawwaz, O. Gamow–Teller strengths and electron-capture rates for pf-shell nuclei of relevance for late stellar evolution. Phys. Rev. C. 2012, 86, 015809. [Google Scholar] [CrossRef] [Green Version]
- Poves, A.; Sánchez-Solano, J.; Caurier, E.; Nowacki, F. Shell model study of the isobaric chains A = 50, A = 51 and A = 52. Nucl. Phys. A. 2001, 694, 157–198. [Google Scholar] [CrossRef] [Green Version]
- Honma, M.; Otsuka, T.; Brown, B.A.; Mizusaki, T. New effective interaction for pf-shell nuclei and its implications for the stability of the N = Z = 28 closed core. Phys. Rev. C 2004, 69, 034335. [Google Scholar] [CrossRef] [Green Version]
- Langanke, K.; Martinez-Pinedo, G.; Zegers, R.M.T. Stellar electron capture. Rep. Prog. Phys. 2021, 84, 066301. [Google Scholar] [CrossRef]
- Kirsebom, O.S.; Hukkanen, M.; Kankainen, A.; Trzaska, W.H.; Ströberg, D.F.; Martínz-Pinedo, G.; Andersen, K.; Bodewits, E.; Canete, L.; Cederkäl, J. Measurement of the 2+→0+ ground-state transition in the β decay of 20F. Phys. Rev. C 2019, 100, 065805. [Google Scholar] [CrossRef] [Green Version]
- Jones, S.; Röpke, F.K.; Pakmor, R.; Seitenzahl, I.R.; Ohlmann, S.T.; Edelmann, P.V.F. Do electron-capture supernovae make neutron stars? First multidimensional hydrodynamic simulations of the oxygen deflagration. Astron. Astrophys. 2016, 593, A72. [Google Scholar] [CrossRef]
- Zha, S.; Leung, S.C.; Suzuki, T.; Nomoto, K. Evolution of ONeMg core in super-AGB stars toward electron-capture supernovae: Effects of updated electron-capture rate. Astrophys. J. 2019, 886, 22. [Google Scholar] [CrossRef]
- Strömberg, D.F.; Martínez-Pinedo, G.; Nowacki, F. Forbidden electron capture on 24Na and 27Al in degenerate oxygen-neon stellar cores. Phys. Rev. C 2022, 105, 025803. [Google Scholar] [CrossRef]
- Takahara, M.; Hino, M.; Oda, T.; Muto, K.; Wolters, A.A.; Glaudemans, P.W.M.; Sato, K. Microscopic calculation of the rates of electron captures which induce the collapse of the O+Ne+Mg cores. Nucl. Phys. A 1989, 504, 167–192. [Google Scholar] [CrossRef]
- Fuller, G.M. Neutron shell blocking of electron capture during gravitational collapse. Astrophys. J. 1982, 252, 741–764. [Google Scholar] [CrossRef]
- Langanke, K.; Kolbe, E.; Dean, D.J. Unblocking of the Gamow–Teller strength in stellar electron capture on neutron-rich germanium isotopes. Phys. Rev. C 2001, 63, 032801(R). [Google Scholar] [CrossRef] [Green Version]
- Fischer, T.; Langanke, K.; Martínez-Pinedo, G. Neutrino-pair emission from nuclear de-excitation in core-collapse supernova simulations. Phys. Rev. C 2013, 88, 065804. [Google Scholar] [CrossRef] [Green Version]
- Grewe, E.-W.; Bäumer, C.; Dohmann, H.; Frekers, D.; Harakeh, M.N.; Hollstein, S.; Johansson, H.; Popescu, L.; Rakers, S.; Savran, D.; et al. The (d,2He) reaction on 76Se and the double-β-decay matrix elements for A = 76. Phys. Rev. C 2008, 78, 044301. [Google Scholar] [CrossRef]
- Zhi, Q.; Langanke, K.; Martínez-Pinedo, G.; Nowacki, F.; Sieja, K. The 76Se Gamow–Teller strength distribution and its importance for stellar electron capture rates. Nucl. Phys. A 2011, 859, 172–184. [Google Scholar] [CrossRef]
- Dean, D.J.; Ressell, M.T.; Hjorth-Jensen, M.; Koonin, S.E.; Langake, K.; Zuker, A.P. Shell-model Monte Carlo studies of neutron-rich nuclei in the 1s-0d-1p-0f shells. Phys. Rev. C 1999, 59, 2474–2486. [Google Scholar] [CrossRef] [Green Version]
- Caurier, E.; Langanke, K.; Martínez-Pinedo, G.; Nowacki, F.; Vogel, P. Shell model description of isotope shifts in calcium. Phys. Lett. B 2001, 522, 240–244. [Google Scholar] [CrossRef] [Green Version]
- Sullivan, C.; Connor, E.O.; Zegers, R.G.T.; Grubb, T.; Austin, S.M. The sensitivity of core-collapse supernovae to nuclear electron capture. Astrophys. J. 2016, 816, 44. [Google Scholar] [CrossRef]
- Titus, R.; Ney, E.M.; Zegers, R.G.T.; Bazin, D.; Belarge, J.; Bender, P.C.; Brown, B.A.; Campbell, C.M.; Elman, B.; Engel, J.; et al. Constraints for stellar electron-capture rates on 86Kr via the 86Kr(t,3He + γ)86Br reaction and the implications for core-collapse supernovae. Phys. Rev. C 2019, 100, 045805. [Google Scholar] [CrossRef] [Green Version]
- Zamora, J.C.; Zegers, R.G.T.; Austin, S.M.; Bazin, D.; Brown, B.A.; Bender, P.C.; Crawford, H.L.; Engel, J.; Falduto, A.; Gade, A.; et al. Experimental constraint on stellar electron-capture rates from the 88Sr(t,3He + γ)88Rb reaction at 115 MeV/u. Phys. Rev. C 2019, 100, 032801. [Google Scholar] [CrossRef] [Green Version]
- Dzhioev, A.A.; Langanke, K.; Martínez-Pinedo, G.; Vdovin, A.I.; Stoyanov, C. Unblocking of stellar electron captures for neutron-rich N = 50 nuclei at finite temperatures. Phys. Rev. C 2020, 101, 025805. [Google Scholar] [CrossRef] [Green Version]
- Litvinova, E.; Robin, C. Impact of complex many-body correlations on electron capture in thermally excited nuclei around 78Ni. Phys. Rev. C 2021, 103, 024326. [Google Scholar] [CrossRef]
- Juodagalvis, A.; Langanke, K.; Hix, W.R.; Martínez-Pinedo, G.; Sampaio, J.M. Improved estimate of stellar electron capture rates on nuclei. Nucl. Phys. A 2010, 848, 454–478. [Google Scholar] [CrossRef] [Green Version]
- Langanke, K.; Martínez-Pinedo, G. Shell-model calculations of stellar weak interaction rates: II. Weak rates for nuclei in the mass range A = 45 − 65 in supernovae environments. Nucl. Phys. A 2000, 673, 481–508. [Google Scholar] [CrossRef] [Green Version]
- Iwamoto, K.; Brachwitz, F.; Nomoto, K.; Kishimoto, N.; Umeda, H.; Hix, W.R.; Thielemann, F.-K. Nucleosynthesis in Chandrasekhar mass models for type Ia supernovae and constraints on progenitor systems and burning-front propagation. Astrophys. J. Suppl. Ser. 1999, 125, 439–462. [Google Scholar] [CrossRef]
- Fuller, G.M.; Meyer, B.S. High-temperature neutrino-nucleus processes in stellar collapse. Astrophys. J. 1991, 376, 701–716. [Google Scholar] [CrossRef] [Green Version]
- Bruenn, S.W.; Haxton, W.C. Neutrino-nucleus interactions in core-collapse supernovae. Astrophys. J. 1991, 376, 678–700. [Google Scholar] [CrossRef]
- Langanke, K.; Martínez-Pinedo, G.; Müller, B.; Janka, H.-T.; Marek, A.; Hix, W.R.; Juodagalvis, A.; Sampaio, J.M. Effects of inelastic neutrino-nucleus scattering on supernova dynamics and radiated neutrino spectra. Phys. Rev. Lett. 2008, 100, 011101. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Donnelly, T.W.; Peccei, R.D. Neutral current effects in nuclei. Phys. Rep. 1979, 50, 1–85. [Google Scholar] [CrossRef]
- Langanke, K.; Martinez-Pinedo, G.; von Neumann-Cosel, P.; Richter, A. Supernova inelastic neutrino-nucleus cross sections from high-resolution electron scattering experiments and shell-model calculations". Phys. Rev. Lett. 2004, 93, 202501. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Von Neumann-Cosel, P.; Poves, A.; Retamosa, J.; Richter, A. Magnetic dipole response in nuclei at the N = 28 shell closure: A new look. Phys. Lett. B 1998, 443, 1–6. [Google Scholar] [CrossRef]
- Juodagalvis, A.; Langanke, K.; Martínez-Pinedo, G.; Hix, W.R.; Dean, D.J.; Sampaioc, J.M. Neutral-current neutrino-nucleus cross sections for A ∼ 50–65 nuclei. Nucl. Phys. A 2005, 747, 87–108. [Google Scholar] [CrossRef] [Green Version]
- Woosley, S.E.; Hartmann, D.H.; Hofmann, R.D.; Haxton, W.C. The ν-process. Astrophys. J. 1990, 356, 272–301. [Google Scholar] [CrossRef]
- Heger, A.; Kolbe, E.; Haxton, W.C.; Langanke, K.; Martínez-Pinedo, G.; Woosley, S.E. Neutrino nucleosynthesis. Phys. Lett. B 2005, 606, 258–264. [Google Scholar] [CrossRef] [Green Version]
- Byelikov, A.; Adachi, T.; Fujita, H.; Fujita, K.; Fujita, Y.; Hatanaka, K.; Heger, A.; Kalmykov, Y.; Kawase, K.; Langanke, K. Gamov–Teller strength in the exotic, odd-odd nuclei 138La and 180Ta and its relevance for neutrino nucleosynthesis. Phys. Rev. Lett. 2007, 98, 082501. [Google Scholar] [CrossRef]
- Sieverding, A.; Martínez-Pinedo, G.; Huther, L.; Langanke, K.; Heger, A. The ν-process in the light of an improved understanding of supernova neutrino spectra. Astrophys. J. 2018, 865, 143. [Google Scholar] [CrossRef] [Green Version]
- Sieverding, A.; Langanke, K.; Martínez-Pinedo, G.; Bollig, R.; Janka, H.-T.; Heger, A. The ν-process with fully time-dependent supernova neutrino emission spectra. Astrophys. J. 2019, 876, 151. [Google Scholar] [CrossRef] [Green Version]
- Kolbe, E.; Langanke, K.; Thielemann, F.-K.; Vogel, P. Inclusive 12C(νμ, μ)12N reaction in the continuum random phase approximation. Phys. Rev C 1995, 52, 3437–3441. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kolbe, E.; Langanke, K.; Martínez-Pinedo, G.; Vogel, P. Neutrino–nucleus reactions and nuclear structure. J. Phys. G Nucl. Part. Phys. 2003, 29, 2569–2596. [Google Scholar] [CrossRef] [Green Version]
- Balasi, K.G.; Langanke, K.; Martínez-Pinedo, G. Neutrino–nucleus reactions and their role for supernova dynamics and nucleosynthesis. Prog. Part. Nucl. Phys. 2015, 85, 33–81. [Google Scholar] [CrossRef] [Green Version]
- Fröhlich, C.; Martínez-Pinedo, G.; Liebendörfer, M.; Thielemann, F.-K.; Bravo, E.; Hix, W.R.; Langanke, K.; Zinner, N.T. Neutrino-induced nucleosynthesis of A > 64 nuclei: The νp-process. Phys. Rev. Lett. 2006, 96, 142502. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Pruet, J.; Woosley, S.E.; Buras, R.; Janka, H.-T.; Hoffman, R.D. Nucleosynthesis in the hot convective bubble in core-collapse supernovae. Astrophys. J. 2006, 623, 325–336. [Google Scholar] [CrossRef] [Green Version]
- Wanajo, S. The rp-process in neutrino-driven winds. Astrophys. J. 2006, 647, 1323–1340. [Google Scholar] [CrossRef] [Green Version]
- Burbidge, E.M.; Burbidge, G.R.; Fowler, W.A.; Hoyle, F. Synthesis of the elements in stars. Rev. Mod. Phys. 1957, 29, 547–650. [Google Scholar] [CrossRef] [Green Version]
- Thielemann, F.-K.; Arcones, A.; Käppeli, R.; Liebendörfer, M.; Rauscher, T.; Winteler, C.; Fröhlich, C.; Dillmann, I.; Fischer, T.; Martinez-Pinedo, G.; et al. What are the astrophysical sites for the r-process and the production of heavy elements? Prog. Part. Nucl. Phys. 2011, 66, 346–353. [Google Scholar] [CrossRef]
- Cowan, J.J.; Thielemann, F.-K.; Truran, J.W. The R-process and nucleochronology. Phys. Rep. 1991, 208, 267–394. [Google Scholar] [CrossRef]
- Abbot, B.P.; et al. [LIGO Scientific Collaboration and Virgo Collaboration; Fermi GBM; INTEGRAL; IceCube Collaboration; AstroSat Cadmium ZincTelluride Imager Team; IPN Collaboration; The Insight-HXMT Collaboration; ANTARES Collaboration; The Swift Collaboration; AGILE Team; The 1M2H Team; The Dark Energy Camera GW-EM Collaboration and the DES Collaboration; The DLT40 Collaboration, GRAWITA: GRAvitational Wave Inaf TeAm; The Fermi Large Area Telescope Collaboration; ATCA: Australia Telescope CompactArray; ASKAP: Australian SKA Pathfinder; Las Cumbres Observatory Group; OzGrav; DWF(Deeper, Wider, Faster Program); AST3, and CAASTRO Collaborations; The VINROUGE Collaboration; MASTER Collaboration; J-GEM, GROWTH, JAGWAR, Caltech-NRAO, TTU-NRAO, and NuSTAR Collaborations; Pan-STARRS; TheMAXITeam; TZACConsortium; KU Collaboration; NordicOptical Telescope; ePESSTO; GROND; Texas Tech University; SALT Group; TOROS: Transient Robotic Observatory of the SouthCollaboration; The BOOTES Collaboration; MWA: Murchison Widefield Array; The CALET Collaboration; IKI-GW Follow-upCollaboration; H.E.S.S. Collaboration; LOFAR Collaboration; LWA: Long Wavelength Array; HAWC Collaboration; The Pierre Auger Collaboration; ALMA Collaboration; Euro VLBI Team; Pi of the Sky Collaboration; The Chandra Team at McGill University; DFN:Desert Fireball Network; ATLAS; High Time Resolution Universe Survey; RIMAS and RATIR; SKA South Africa/MeerKAT] Multi-messenger observations of a binary neutron star merger. Astrophys. J. Lett. 2017, 848, L12. [Google Scholar] [CrossRef]
- Cowperthwaite, P.S.; Berger, E.; Villar, V.A.; Metzger, B.D.; Nicholl, M.; Chornock, R.; Blanchard, P.K.; Fong, W.; Margutti, R.; Soares-Santos, M.; et al. The electromagnetic counterpart of the binary neutron star merger LIGO/Virgo GW170817. II. UV, optical, and near-infrared light curves and comparison to kilonova models. Astrophys. J. Lett. 2017, 848, L17. [Google Scholar] [CrossRef]
- Metzger, B.D.; Martńez-Pinedo, G.; Darbha, S.; Quataert, E.; Arcones, A.; Kasen, D.; Thomas, R.; Nugent, P.; Panov, I.V.; Zinner, N.T. Electromagnetic counterparts of compact object mergers powered by the radioactive decay of r-process nuclei. Mon. Not. R. Astron. Soc. 2010, 406, 2650–2662. [Google Scholar] [CrossRef]
- Cowan, J.J.; Sneden, C.; Lawler, J.E.; Aprahamian, A.; Wiescher, M.; Langake, K.; Martínez-Pinedo, G.; Thielemann, F.-K. Origin of the heaviest elements: The rapid neutron-capture process. Process. Rev. Mod. Phys. 2021, 93, 015002. [Google Scholar] [CrossRef]
- Wu, J.; Nishimura, S.; Lorusso, G.; Möller, P.; Ideguchi, E.; Regan, P.-H.; Simpson, G.S.; Söderström, P.A.; Waller, P.M.; Watanable, H.; et al. 94 β-decay half-lives of neutron-rich 55Cs to 67Ho: Experimental feedback and evaluation of the r-process rare-earth peak formation. Phys. Rev. Lett. 2017, 118, 072701. [Google Scholar]
- Lorusso, G.; Nishimura, S.; Xu, Z.Y.; Jungclaus, A.; Shimizu, Y.; Simpson, G.S.; Söderström, P.-A.; Watanabe, H.; Browne, F.; Doornenbal, P.; et al. β-decay half-lives of 110 neutron-rich nuclei across the N = 82 shell gap: Implications for the mechanism and universality of the astrophysical r process. Phys. Rev. Lett. 2015, 114, 192501. [Google Scholar] [CrossRef] [Green Version]
- Möller, P.; Nix, J.R.; Kratz, K.-L. Nuclear properties for astrophysical and radioactive-ion-beam applications. At. Nucl. Data Tables 1997, 66, 131–343. [Google Scholar] [CrossRef] [Green Version]
- Borzov, I.N.; Goriely, S. Weak interaction rates of neutron-rich nuclei and the r-process nucleosynthesis. Phys. Rev. C 2000, 62, 035501. [Google Scholar] [CrossRef]
- Borzov, I.N. Gamow–Teller and first-forbidden decays near the r-process paths at N = 50, 82, and 126. Phys. Rev. C 2003, 67, 025802. [Google Scholar] [CrossRef]
- Marketin, T.; Huther, L.; Martinez-Pinedo, G. Large-scale evaluation of β-decay rates of r-process nuclei with the inclusion of first-forbidden transitions. Phys. Rev. C 2016, 93, 025805. [Google Scholar] [CrossRef] [Green Version]
- Mustonen, M.T.; Engel, G. Global description of β− decay in even-even nuclei with the axially-deformed Skyrme finite-amplitude method. Phys. Rev. C 2016, 93, 014304. [Google Scholar] [CrossRef] [Green Version]
- Shafer, T.; Engel, J.; Frölich, C.; Mclaughlin, G.C.; Mumpower, M.; Surman, R. β decay of deformed r-process nuclei near A = 80 and A = 160, including odd-A and odd-odd nuclei, with the Skyrme finite-amplitude method. Phys. Rev. C 2016, 94, 055802. [Google Scholar] [CrossRef] [Green Version]
- Ney, E.M.; Engel, J.; Li, T.; Schunck, N. Global description of β− decay with the axially deformed Skyrme finite-amplitude method: Extension to odd-mass and odd-odd nuclei. Phys. Rev. C 2020, 102, 034326. [Google Scholar] [CrossRef]
- Hosmer, P.T.; Schatz, H.; Aprahamian, A.; Arndt, O.; Clement, R.R.C.; Estrade, A.; Kratz, K.-L.; Liddick, S.N.; Mantica, P.F.; Mueller, W.F.; et al. Half-life of the doubly magic r-process nucleus 78Ni. Phys. Rev. Lett. 2005, 94, 112501. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- De Jesús Mendoza-Temis, J.; Wu, M.-R.; Langanke, K.; Martínez-Pinedo, G.; Bauswein, A.; Janka, H.-T. Nuclear robustness of the r process in neutron-star mergers. Phys. Rev. C 2015, 92, 055805. [Google Scholar] [CrossRef] [Green Version]
- Petermann, I.; Langanke, K.; Martínez-Pinedo, G.; Panov, I.V.; Reinhard, P.-G.; Thielemann, F.-K. Have superheavy elements been produced in nature? Eur. Phys. J. A 2012, 48, 122. [Google Scholar] [CrossRef] [Green Version]
- Wu, M.-R.; Barnes, J.; Martínez-Pinedo, G.; Metzger, B.D. Fingerprints of heavy-element nucleosynthesis in the late-time lightcurves of kilonovae. Phys. Rev. Lett. 2019, 122, 062701. [Google Scholar] [CrossRef] [Green Version]
- Pfeiffer, B.; Kratz, K.-L.; Thielemann, F.-K.; Walters, W.B. Nuclear structure studies for the astrophysical r-process. Nucl. Phys. A 2001, 693, 282–324. [Google Scholar] [CrossRef]
- Dillmann, I.; Kratz, K.-L.; Wöhr, A.; Arndt, O.; Brown, B.A.; Hoff, P.; Hjorth-Jensen, M.; Köster, U.; Ostrowski, A.N.; Pfeiffer, D.; et al. N = 82 shell quenching of the classical r-process "waiting-point" nucleus 130Cd. Phys. Rev. Lett. 2003, 91, 162503. [Google Scholar] [CrossRef] [Green Version]
- Fogelberg, B.; Gausemel, H.; Mezilev, K.A.; Hoff, P.; Mach, H.; Sanchez-Vega, M.; Lindroth, A.; Ramström, E.; Genevey, J.; Pinston, J.A.; et al. Decays of 131In, 131Sn, and the position of the h11/2 neutron hole state. Phys. Rev. C 2004, 70, 034312. [Google Scholar] [CrossRef]
- Alhassid, Y.; Liu, S.; Nakada, H. Particle-number reprojection in the shell model Monte Carlo method: Application to nuclear level densities. Phys. Rev. Lett. 1999, 83, 4265–4268. [Google Scholar] [CrossRef] [Green Version]
- Alhassid, Y.; Liu, S.; Nakada, H. Spin projection in the shell model Monte Carlo method and the spin distribution of nuclear level densities. Phys. Rev. Lett. 2007, 99, 162504. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Langanke, K. Shell Model Monte Carlo studies of pairing correlations and level densities in medium-mass nuclei. Nucl. Phys. A 2006, 778, 233–246. [Google Scholar] [CrossRef]
- Alhassid, Y.; Bertsch, G.F.; Liu, S.; Nakada, H. Parity dependence of nuclear level densities. Phys. Rev. Lett. 2000, 84, 4313–4316. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mocelj, D.; Rauscher, T.; Martínez-Pinedo, G.; Langanke, K.; Pacearescu, L.; Faessler, A.; Thielemann, F.-K.; Alhassid, Y. Large-scale prediction of the parity distribution in the nuclear level density and application to astrophysical reaction rates. Phys. Rev. C 2007, 75, 045805. [Google Scholar] [CrossRef] [Green Version]
- Rauscher, T.; Thielemann, F.-K. Astrophysical reaction rates from statistical model calculations. At. Nucl. Data Tables 2000, 75, 1–351. [Google Scholar] [CrossRef] [Green Version]
- Rauscher, T.; Thielemann, F.-K. Tables of nuclear cross sections and reaction rates: An addendum to the paper “Astrophysical reaction rates from statistical model calculations”. At. Nucl. Data Tables 2001, 79, 47–64. [Google Scholar] [CrossRef] [Green Version]
- Rauscher, T. The path to improved reaction rates for astrophysics. Int. J. Mod. Phys. E 2011, 20, 1071–1169. [Google Scholar] [CrossRef] [Green Version]
- Goriely, S.; Hilaire, S.; Koning, A.J. Improved microscopic nuclear level densities within the Hartree-Fock-Bogoliubov plus combinatorial method. Phys. Rev. C 2008, 78, 064307. [Google Scholar] [CrossRef]
- Koning, A.J.; Hilaire, S.; Goriely, S. Global and local level density models. Nucl. Phys. A 2008, 810, 13–76. [Google Scholar] [CrossRef]
- Goriely, S.; Hilaire, S.; Girod, M. Latest development of the combinatorial model of nuclear level densities. J. Phys. Conf. Ser. 2012, 337, 012027. [Google Scholar] [CrossRef] [Green Version]
- Guttormsen, M.; Chankova, R.; Agvaanluvsan, U.; Algin, E.; Bernstein, L.A.; Ingebretsen, F.; Lönnroth, T.; Messelt, S.; Mitchell, G.E.; Rekstad, J.; et al. Radiative strength functions in 93–98Mo. Phys. Rev. C 2005, 71, 044307. [Google Scholar] [CrossRef] [Green Version]
- Larsen, A.C.; Chankova, R.; Guttormsen, M.; Ingebretsen, F.; Messelt, S.; Rekstad, J.; Siem, S.; Syed, N.U.H.; Øegård, S.W.; Lönnroth, T.; et al. Microcanonical entropies and radiative strength functions of 50,51V. Phys. Rev. C 2006, 73, 064301. [Google Scholar] [CrossRef] [Green Version]
- Goriely, S. Radiative neutron captures by neutron-rich nuclei and the r-process nucleosynthesis. Phys. Lett. B 1998, 436, 10–18. [Google Scholar] [CrossRef]
- Larsen, A.C.; Goriely, S. Impact of a low-energy enhancement in the γ-ray strength function on the neutron-capture cross section. Phys. Rev. C 2010, 82, 014318. [Google Scholar] [CrossRef] [Green Version]
- Litvinova, E.; Ring, P.; Tselyaev, V.; Langanke, K. Relativistic quasiparticle time blocking approximation. II. Pygmy dipole resonance in neutron-rich nuclei. Phys. Rev. C 2009, 79, 054312. [Google Scholar] [CrossRef] [Green Version]
- Sieja, K. Electric and magnetic dipole strength at low energy. Phys. Rev. Lett. 2017, 119, 052502. [Google Scholar] [CrossRef]
- Sieja, K. Shell-model study of the M1 dipole strength at low energy in the A > 100 nuclei. Phys. Rev. C 2018, 98, 064312. [Google Scholar] [CrossRef]
- Loens, H.P.; Langanke, K.; Martínez-Pinedo, G.; Sieja, K. M1 strength functions from large-scale shell model calculations and their effect on astrophysical neutron capture cross-sections. Eur. Phys. J. A 2012, 48, 34. [Google Scholar] [CrossRef]
- Bohle, D.; Richter, A.; Steffen, W.; Dieperink, A.E.L.; Lo Ludice, N.; Palumbo, F.; Scholten, O. New magnetic dipole excitation mode studied in the heavy deformed nucleus 156Gd by inelastic electron scattering. Phys. Lett. 1984, 137, 27–31. [Google Scholar] [CrossRef] [Green Version]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Martínez-Pinedo, G.; Langanke, K. Shell Model Applications in Nuclear Astrophysics. Physics 2022, 4, 677-689. https://doi.org/10.3390/physics4020046
Martínez-Pinedo G, Langanke K. Shell Model Applications in Nuclear Astrophysics. Physics. 2022; 4(2):677-689. https://doi.org/10.3390/physics4020046
Chicago/Turabian StyleMartínez-Pinedo, Gabriel, and Karlheinz Langanke. 2022. "Shell Model Applications in Nuclear Astrophysics" Physics 4, no. 2: 677-689. https://doi.org/10.3390/physics4020046
APA StyleMartínez-Pinedo, G., & Langanke, K. (2022). Shell Model Applications in Nuclear Astrophysics. Physics, 4(2), 677-689. https://doi.org/10.3390/physics4020046





