Magnetic Resonance Velocimetry Measurement of Viscous Flows through Porous Media: Comparison with Simulation and Voxel Size Study
Abstract
:1. Introduction
Fundamentals of the Data Reconstruction in MRV
2. Materials and Methods
2.1. Experimental Setup
2.1.1. MRV Protocols
2.1.2. MRV Data Processing
2.2. CFD Setup
3. Results and Discussion
3.1. MRV Velocity Field
3.2. Comparison to CFD Results
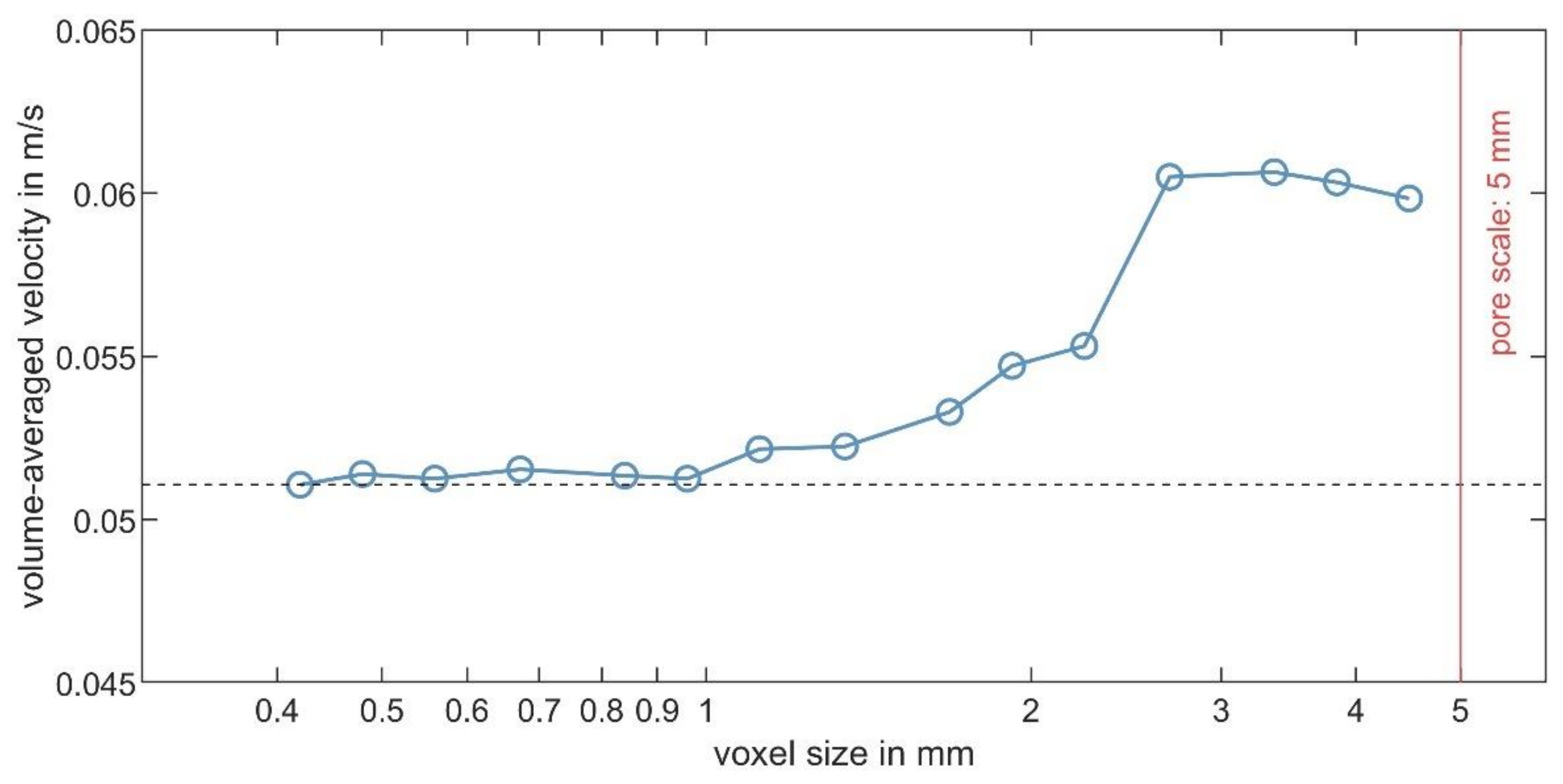
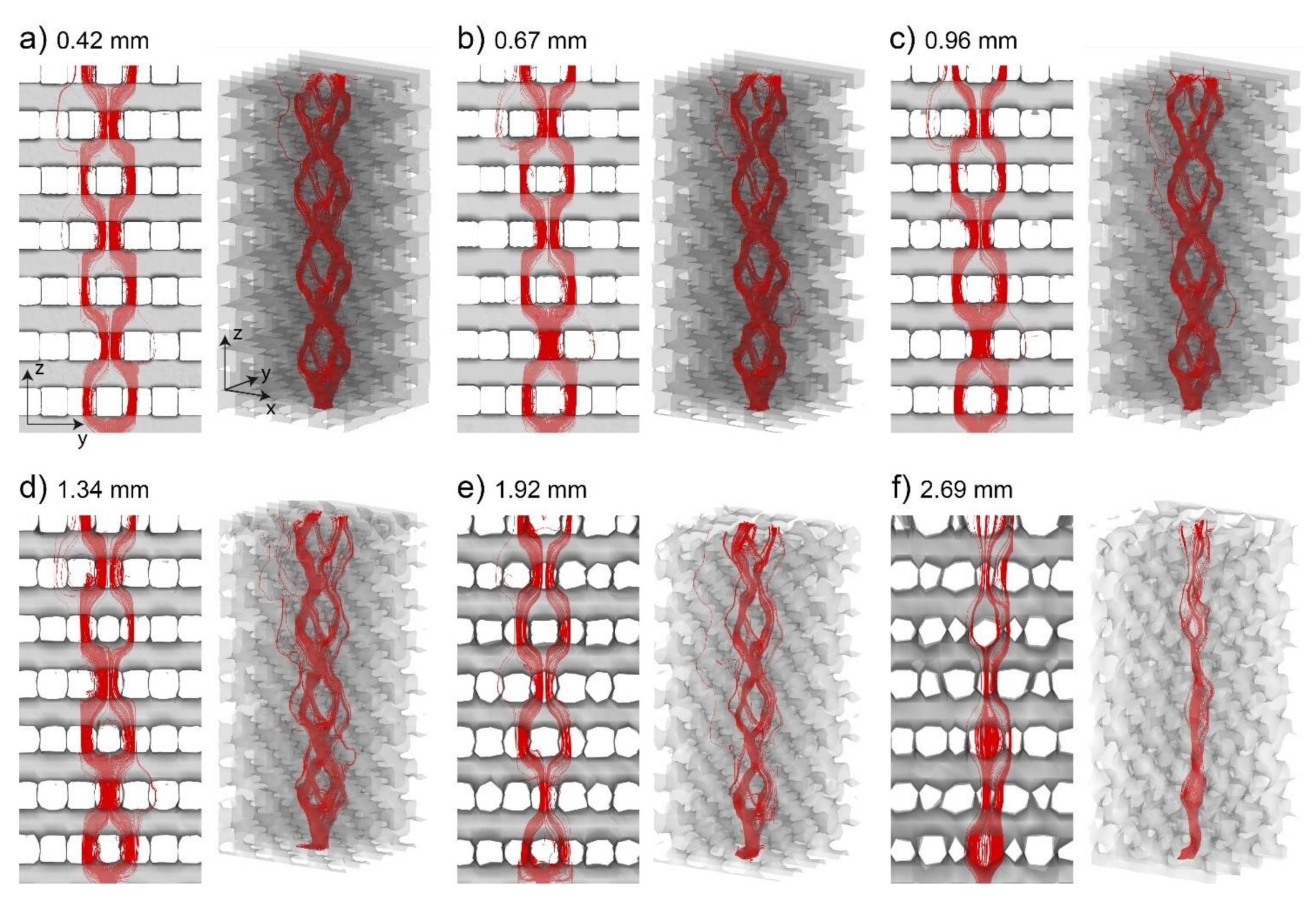
3.3. Study on Voxel Size
3.3.1. Influence of the Voxel Size on Flow Quantification
3.3.2. Influence of the Voxel Size on the Local Velocity Distribution
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Vafai, K. (Ed.) Porous Media: Applications in Biological Systems and Biotechnology; CRC Press/Taylor & Francis Group: Boca Raton, FL, USA, 2010. [Google Scholar] [CrossRef]
- Vafai, K. (Ed.) Handbook of Porous Media; CRC Press/Taylor & Francis Group: Boca Raton, FL, USA, 2015. [Google Scholar] [CrossRef]
- Dullien, F.A.L. Porous Media: Fluid Transport and Pore Structure; Academic Press, Inc.: San Diego, CA, USA, 1992. [Google Scholar] [CrossRef]
- Nield, D.A.; Bejan, A. Convection in Porous Media; Springer: New York, NY, USA, 2013. [Google Scholar] [CrossRef]
- Northrup, M.A.; Kulp, T.J.; Angel, S.M. Fluorescent particle image velocimetry: Application to flow measurement in refractive index-matched porous media. Appl. Opt. 1991, 30, 3034–3040. [Google Scholar] [CrossRef] [PubMed]
- Saleh, S.; Thovert, J.F.; Adler, P.M. Measurement of two-dimensional velocity fields in porous media by particle image displacement velocimetry. Exp. Fluids 1992, 12, 210–212. [Google Scholar] [CrossRef]
- Gladden, L.F.; Mitchell, J. Measuring adsorption, diffusion and flow in chemical engineering: Applications of magnetic resonance to porous media. New J. Phys. 2011, 13, 035001. [Google Scholar] [CrossRef]
- Kutsovsky, Y.E.; Scriven, L.E.; Davis, H.T.; Hammer, B.E. NMR imaging of velocity profiles and velocity distributions in bead packs. Phys. Fluids 1996, 8, 863–871. [Google Scholar] [CrossRef]
- Manz, B.; Alexander, P.; Gladden, L.F. Correlations between dispersion and structure in porous media probed by nuclear magnetic resonance. Phys. Fluids 1999, 11, 259–267. [Google Scholar] [CrossRef]
- Ogawa, K.; Matsuka, T.; Hirai, S.; Okazaki, K. Three-dimensional velocity measurement of complex interstitial flows through water-saturated porous media by the tagging method in the MRI technique. Meas. Sci. Technol. 2001, 12, 172–180. [Google Scholar] [CrossRef]
- Sankey, M.; Holland, D.; Sederman, A.; Gladden, L. Magnetic resonance velocity imaging of liquid and gas two-phase flow in packed beds. J. Magn. Reson. 2009, 196, 142–148. [Google Scholar] [CrossRef] [PubMed]
- Wu, A.-X.; Liu, C.; Yin, S.-H.; Xue, Z.-L.; Chen, X. Pore structure and liquid flow velocity distribution in water-saturated porous media probed by MRI. Trans. Nonferrous Met. Soc. China 2016, 26, 1403–1409. [Google Scholar] [CrossRef]
- Lovreglio, P.; Das, S.; Buist, K.A.; Peters, E.A.J.F.; Pel, L.; Kuipers, H. Experimental and numerical investigation of structure and hydrodynamics in packed beds of spherical particles. AIChE J. 2018, 64, 1896–1907. [Google Scholar] [CrossRef]
- Romanenko, K.; Xiao, D.; Balcom, B.J. Velocity field measurements in sedimentary rock cores by magnetization prepared 3D SPRITE. J. Magn. Reson. 2012, 223, 120–128. [Google Scholar] [CrossRef] [PubMed]
- Onstad, A.J.; Elkins, C.J.; Medina, F.; Wicker, R.B.; Eaton, J.K. Full-field measurements of flow through a scaled metal foam replica. Exp. Fluids 2011, 50, 1571–1585. [Google Scholar] [CrossRef]
- Sadeghi, M.; Mirdrikvand, M.; Pesch, G.R.; Dreher, W.; Thöming, J. Full-field analysis of gas flow within open-cell foams: Comparison of micro-computed tomography-based CFD simulations with experimental magnetic resonance flow mapping data. Exp. Fluids 2020, 61, 124. [Google Scholar] [CrossRef]
- Yang, X.; Scheibe, T.D.; Richmond, M.; Perkins, W.A.; Vogt, S.; Codd, S.L.; Seymour, J.; McKinley, M.I. Direct numerical simulation of pore-scale flow in a bead pack: Comparison with magnetic resonance imaging observations. Adv. Water Resour. 2013, 54, 228–241. [Google Scholar] [CrossRef] [Green Version]
- Meinicke, S.; Möller, C.-O.; Dietrich, B.; Schlüter, M.; Wetzel, T. Experimental and numerical investigation of single-phase hydrodynamics in glass sponges by means of combined µPIV measurements and CFD simulation. Chem. Eng. Sci. 2017, 160, 131–143. [Google Scholar] [CrossRef]
- Munro, B.; Becker, S.; Uth, M.F.; Preußer, N.; Herwig, H. Fabrication and Characterization of Deformable Porous Matrices with Controlled Pore Characteristics. Transp. Porous Med. 2015, 107, 79–94. [Google Scholar] [CrossRef]
- Nishimura, D.G.; Jackson, J.I.; Pauly, J.M. On the nature and reduction of the displacement artifact in flow images. Magn. Reson. Med. 1991, 22, 481–492. [Google Scholar] [CrossRef]
- Schmidt, S.; Flassbeck, S.; Bachert, P.; Ladd, M.E.; Schmitter, S. Velocity encoding and velocity compensation for multi-spoke RF excitation. Magn. Reson. Imaging 2020, 66, 69–85. [Google Scholar] [CrossRef] [PubMed]
- Bruschewski, M.; Freudenhammer, D.; Buchenberg, W.B.; Schiffer, H.-P.; Grundmann, S. Estimation of the measurement uncertainty in magnetic resonance velocimetry based on statistical models. Exp. Fluids 2016, 57, 83. [Google Scholar] [CrossRef]
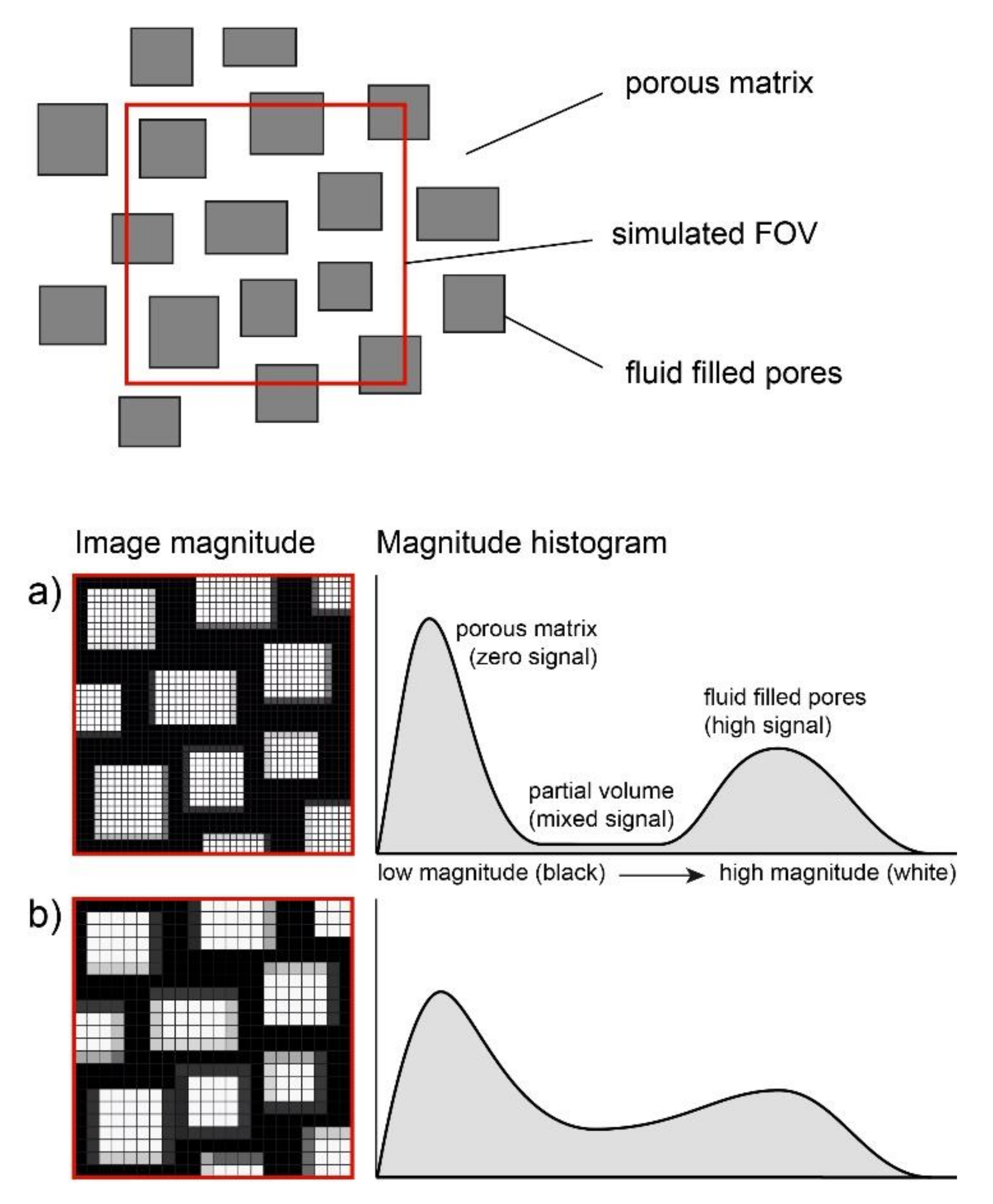

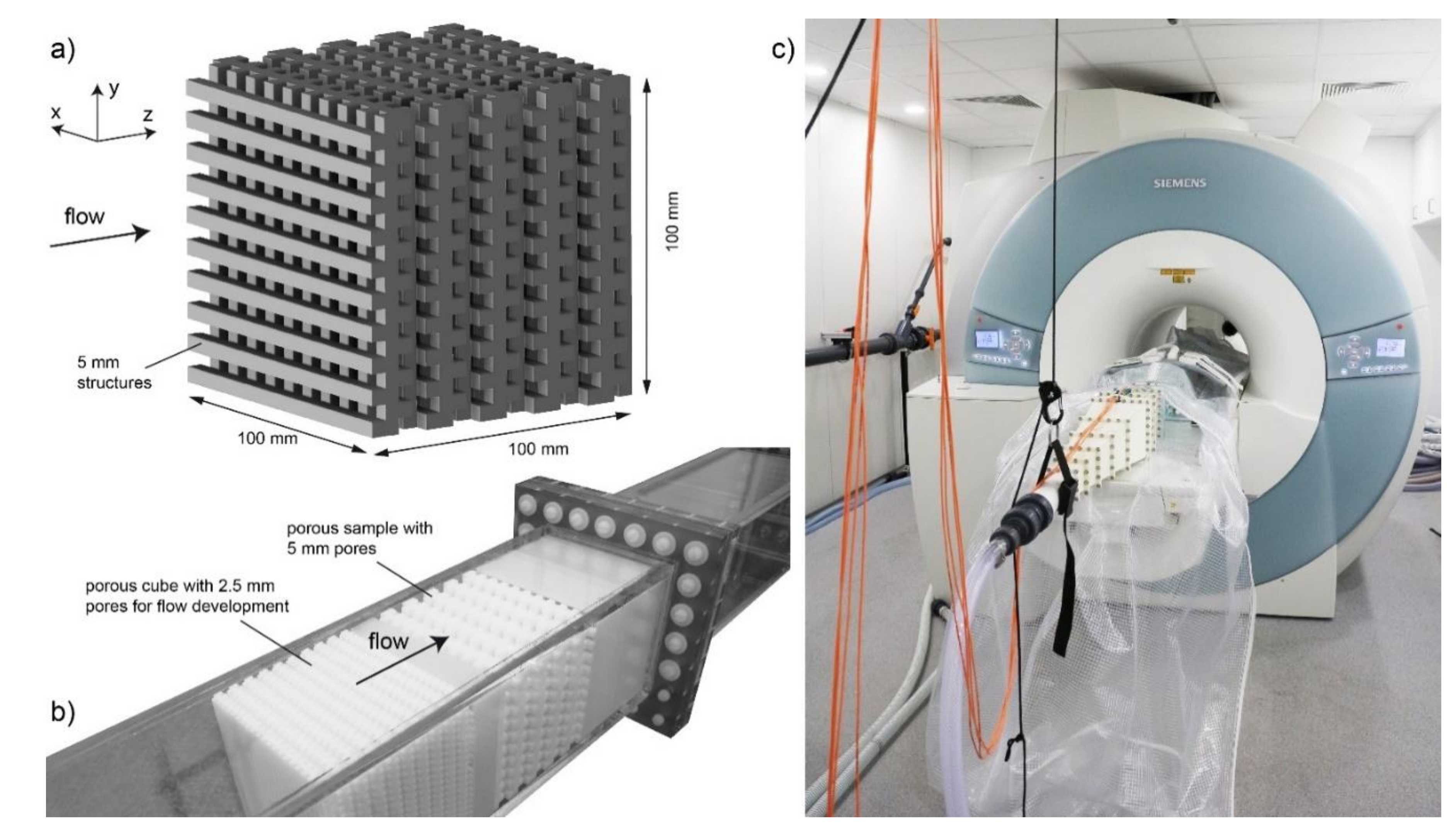


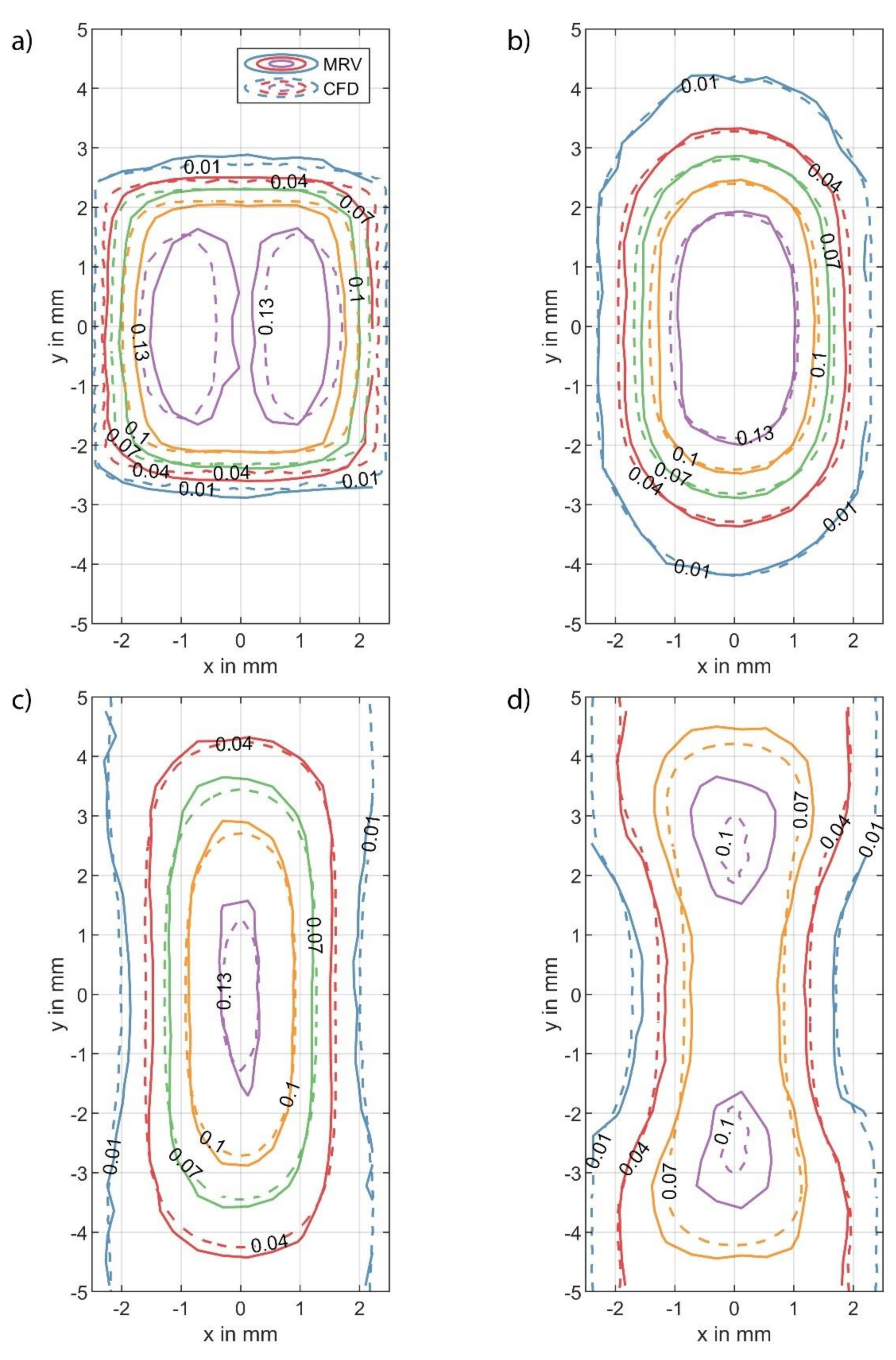
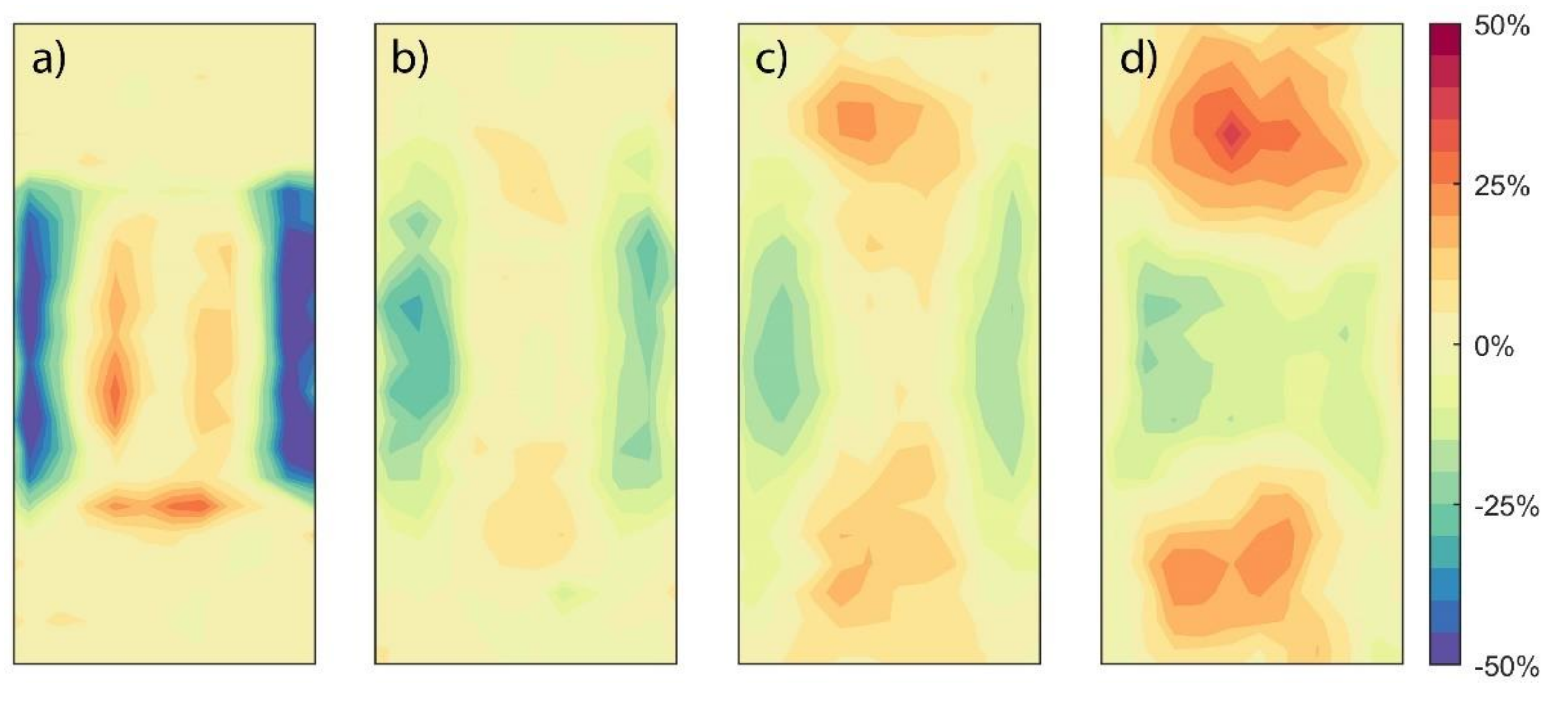

| No. of Voxels (x,y,z) | Voxel Size (mm) | Encoding Direction | TE (ms) | TR (ms) | Flip Angle (°) | BW (Hz/pixel) | VENC (m/s) | Averages | Total Time (h) |
|---|---|---|---|---|---|---|---|---|---|
| 256 × 256 × 256 | 0.42 × 0.42 × 0.42 | RO PE SS | 5.1 | 8.6 | 20 | 407 | 0.2 | 9 | 6 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bruschewski, M.; Flint, S.; Becker, S. Magnetic Resonance Velocimetry Measurement of Viscous Flows through Porous Media: Comparison with Simulation and Voxel Size Study. Physics 2021, 3, 1254-1267. https://doi.org/10.3390/physics3040079
Bruschewski M, Flint S, Becker S. Magnetic Resonance Velocimetry Measurement of Viscous Flows through Porous Media: Comparison with Simulation and Voxel Size Study. Physics. 2021; 3(4):1254-1267. https://doi.org/10.3390/physics3040079
Chicago/Turabian StyleBruschewski, Martin, Sam Flint, and Sid Becker. 2021. "Magnetic Resonance Velocimetry Measurement of Viscous Flows through Porous Media: Comparison with Simulation and Voxel Size Study" Physics 3, no. 4: 1254-1267. https://doi.org/10.3390/physics3040079
APA StyleBruschewski, M., Flint, S., & Becker, S. (2021). Magnetic Resonance Velocimetry Measurement of Viscous Flows through Porous Media: Comparison with Simulation and Voxel Size Study. Physics, 3(4), 1254-1267. https://doi.org/10.3390/physics3040079





