Quality Parameters and the Modeling of the Microwave Drying Kinetics of Basil ‘Nufar’ (Ocimum basilicum L.) Leaves
Abstract
1. Introduction
2. Materials and Methods
2.1. Chemicals
2.2. Sample Preparation
2.3. Drying Experiment
2.4. Quality Attributes
2.5. Mathematical Model
2.6. Statistical Analysis
3. Results
3.1. Microwave Drying
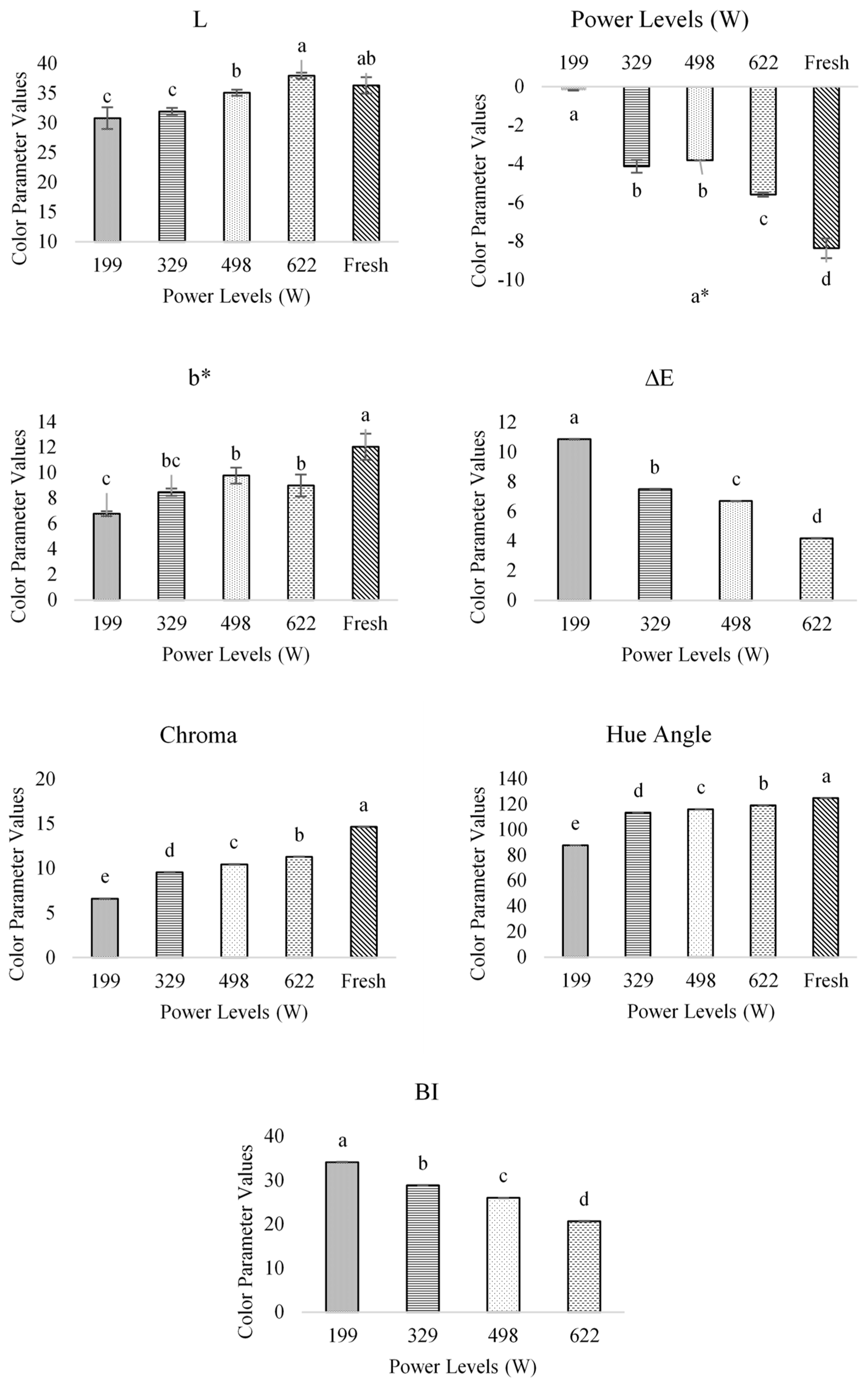
3.2. Color
3.3. Total Phenolic Content
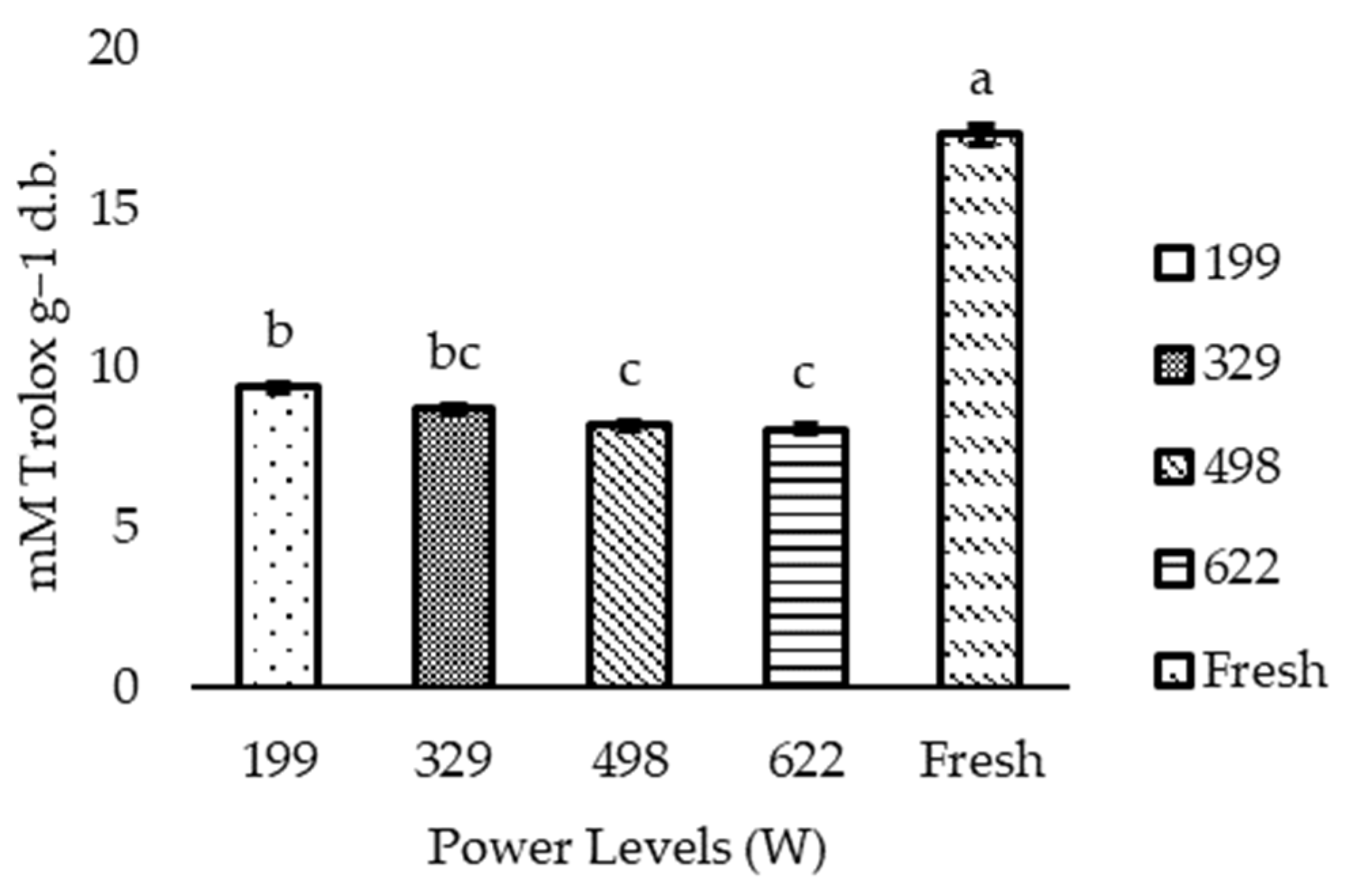
3.4. Antioxidant Capacity
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- da Silva, W.M.F.; Kringel, D.H.; de Souza, E.J.D.; da Rosa Zavareze, E.; Dias, A.R.G. Basil Essential Oil: Methods of Extraction, Chemical Composition, Biological Activities, and Food Applications. Food Bioprocess Tech. 2022, 15, 1–27. [Google Scholar] [CrossRef]
- Duke, J.A. Handbook of Medicinal Herbs; CRC Press: Boca Raton, FL, USA, 2002. [Google Scholar]
- Marwat, S.K.; Fazal-Ur-Rehman; Khan, M.S.; Ghulam, S.; Anwar, N.; Mustafa, G.; Usman, K. Phytochemical constituents and pharmacological activities of sweet Basil-Ocimum basilicum L. (Lamiaceae). Asian J. Chem. 2011, 23, 3773–3782. [Google Scholar]
- Shalaby, S.M.; Darwesh, M.; Ghoname, M.S.; Salah, S.E.; Nehela, Y.; Fetouh, M.I. The effect of drying sweet basil in an indirect solar dryer integrated with phase change material on essential oil valuable components. Energy Rep. 2020, 6, 43–50. [Google Scholar] [CrossRef]
- Ibrahim, A.; Elsebaee, I.; Amer, A.; Aboelasaad, G.; El-Bediwy, A.; El-Kholy, M. Development and evaluation of a hybrid smart solar dryer. J. Food Sci. 2023, 88, 3859–3878. [Google Scholar] [CrossRef] [PubMed]
- Mbegbu, N.N.; Nwajinka, C.O.; Amaefule, D.O. Thin layer drying models and characteristics of scent leaves (Ocimum gratissimum) and lemon basil leaves (Ocimum africanum). Heliyon 2021, 7, e05945. [Google Scholar] [CrossRef] [PubMed]
- Sęczyk, Ł.; Ozdemir, F.A.; Kołodziej, B. In vitro bioaccessibility and activity of basil (Ocimum basilicum L.) phytochemicals as affected by cultivar and postharvest preservation method—Convection drying, freezing, and freeze-drying. Food Chem. 2022, 382, 132363. [Google Scholar] [CrossRef] [PubMed]
- Bušić, A.; Vojvodić, A.; Komes, D.; Akkermans, C.; Belščak-Cvitanović, A.; Stolk, M.; Hofland, G. Comparative evaluation of CO2 drying as an alternative drying technique of basil (Ocimum basilicum L.)—The effect on bioactive and sensory properties. Food Res. Int. 2014, 64, 34–42. [Google Scholar] [CrossRef]
- Kusuma, H.S.; Izzah, D.N.; Linggajati, I.W.L. Microwave-assisted drying of Ocimum sanctum leaves: Analysis of moisture content, drying kinetic model, and techno-economics. Appl. Food Res. 2023, 3, 100337. [Google Scholar] [CrossRef]
- Delfiya, D.S.A.; Prashob, K.; Murali, S.; Alfiya, P.V.; Samuel, M.P.; Pandiselvam, R. Drying kinetics of food materials in infrared radiation drying: A review. J. Food Process Eng. 2022, 45, e13810. [Google Scholar] [CrossRef]
- Altay, K.; Hayaloglu, A.A.; Dirim, S.N. Determination of the drying kinetics and energy efficiency of purple basil (Ocimum basilicum L.) leaves using different drying methods. Heat Mass Transf. 2019, 55, 2173–2184. [Google Scholar] [CrossRef]
- Amini, G.; Salehi, F.; Rasouli, M. Drying kinetics of basil seed mucilage in an infrared dryer: Application of GA-ANN and ANFIS for the prediction of drying time and moisture ratio. J. Food Process. Preserv. 2021, 45, e15258. [Google Scholar] [CrossRef]
- Aksüt, B.; Dinçer, E.; Saraçoğlu, O.; Polatci, H. Kurutma yöntemi ve sıcaklık değerlerinin mor reyhanın kuruma kinetiği ve renk kalitesi üzerine etkisi. Anadolu J. Agric. Sci. 2022, 38, 187–198. [Google Scholar] [CrossRef]
- Phoungchandang, S.; Kongpim, P. Modeling Using a New Thin-Layer Drying Model and Drying Characteristics of Sweet Basil (Ocimum Baslicum Linn.) Using Tray and Heat Pump-Assisted Dehumidified Drying. J. Food Process. Eng. 2012, 35, 851–862. [Google Scholar] [CrossRef]
- Chandrasekaran, S.; Ramanathan, S.; Basak, T. Microwave food processing—A review. Food Res. Int. 2013, 52, 243–261. [Google Scholar] [CrossRef]
- Pham, N.; Khan, M.; Karim, M. A mathematical model for predicting the transport process and quality changes during intermittent microwave convective drying. Food Chem. 2020, 325, 126932. [Google Scholar] [CrossRef] [PubMed]
- Maftoonazad, N.; Dehghani, M.R.; Ramaswamy, H.S. Hybrid microwave-hot air tunnel drying of onion slices: Drying kinetics, energy efficiency, product rehydration, color, and flavor characteristics. Dry. Technol. 2022, 40, 966–986. [Google Scholar] [CrossRef]
- Sarkar, T.; Salauddin, M.; Hazra, S.K.; Chakraborty, R. Artificial neural network modelling approach of drying kinetics evolution for hot air oven, microwave, microwave convective and freeze dried pineapple. SN Appl. Sci. 2020, 2, 1621. [Google Scholar] [CrossRef]
- Trystram, G. Modelling of food and food processes. J. Food Eng. 2012, 110, 269–277. [Google Scholar] [CrossRef]
- Inyang, U.E.; Oboh, I.O.; Etuk, B.R. Kinetic Models for Drying Techniques—Food Materials. Adv. Chem. Eng. Sci. 2018, 08, 27–48. [Google Scholar] [CrossRef]
- Castro, A.M.; Mayorga, E.Y.; Moreno, F.L. Mathematical modelling of convective drying of fruits: A review. J. Food Eng. 2018, 223, 152–167. [Google Scholar] [CrossRef]
- Thamkaew, G.; Sjöholm, I.; Galindo, F.G. A review of drying methods for improving the quality of dried herbs. Crit. Rev. Food Sci. Nutr. 2021, 61, 1763–1786. [Google Scholar] [CrossRef] [PubMed]
- Imaizumi, T.; Jitareerat, P.; Laohakunjit, N.; Kaisangsri, N. Effect of microwave drying on drying characteristics, volatile compounds and color of holy basil (Ocimum tenuiflorum L.). Agric. Nat. Resour. 2021, 55, 1–6. [Google Scholar] [CrossRef]
- Arslan, A.; Soysal, Y.; Keskin, M. Mathematical Modeling, Moisture Diffusion and Color Quality in Intermittent Microwave Drying of Organic and Conventional Sweet Red Peppers. AgriEngineering 2020, 2, 393–407. [Google Scholar] [CrossRef]
- Rusu, M.E.; Fizeșan, I.; Pop, A.; Gheldiu, A.-M.; Mocan, A.; Crișan, G.; Vlase, L.; Loghin, F.; Popa, D.-S.; Tomuta, I. Enhanced Recovery of Antioxidant Compounds from Hazelnut (Corylus avellana L.) Involucre Based on Extraction Optimization: Phytochemical Profile and Biological Activities. Antioxidants 2019, 8, 460. [Google Scholar] [CrossRef]
- Romano, R.; De Luca, L.; Aiello, A.; Pagano, R.; Di Pierro, P.; Pizzolongo, F.; Masi, P. Basil (Ocimum basilicum L.) Leaves as a Source of Bioactive Compounds. Foods 2022, 11, 3212. [Google Scholar] [CrossRef] [PubMed]
- Crank, J. The Mathematics of Diffusion, 2nd ed.; Oxford University Press: Oxford, UK, 1975. [Google Scholar]
- Bergman, T.L. Fundamentals of Heat and Mass Transfer; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- de Aquino Brito Lima-Corrêa, R.; dos Santos Andrade, M.; da Silva, M.F.d.G.F.; Freire, J.T.; Ferreira, M.d.C. Thin-layer and vibrofluidized drying of basil leaves (Ocimum basilicum L.): Analysis of drying homogeneity and influence of drying conditions on the composition of essential oil and leaf colour. J. Appl. Res. Med. Aromat. Plants 2017, 7, 54–63. [Google Scholar] [CrossRef]
- Nesvadba, P. Thermal properties of unfrozen foods. In Engineering Properties of Foods; CRC Press: Boca Raton, FL, USA, 2014; pp. 171–196. [Google Scholar]
- Canabarro, N.I.; Mazutti, M.A.; do Carmo Ferreira, M. Drying of olive (Olea europaea L.) leaves on a conveyor belt for supercritical extraction of bioactive compounds: Mathematical modeling of drying/extraction operations and analysis of extracts. Ind. Crops Prod. 2019, 136, 140–151. [Google Scholar] [CrossRef]
- Kayisoglu, S. Drying Kinetics and Changes in Color Parameters When Drying Green Pea (Pisum Sativum L.) in Microwave. Lat. Am. Appl. Res. 2020, 50, 235–240. [Google Scholar] [CrossRef]
- Al-Harahsheh, M.; Al-Muhtaseb, A.H.; Magee, T.R.A. Microwave drying kinetics of tomato pomace: Effect of osmotic dehydration. Chem. Eng. Process. Process Intensif. 2009, 48, 524–531. [Google Scholar] [CrossRef]
- Evin, D. Thin layer drying kinetics of Gundelia tournefortii L. Food Bioprod. Process. 2012, 90, 323–332. [Google Scholar] [CrossRef]
- Zhou, X.; Wang, S. Recent developments in radio frequency drying of food and agricultural products: A review. Dry. Technol. 2019, 37, 271–286. [Google Scholar] [CrossRef]
- Efremov, G.; Kudra, T. Calculation of the Effective Diffusion Coefficients by Applying a Quasi-Stationary Equation for Drying Kinetics. Dry. Technol. 2004, 22, 2273–2279. [Google Scholar] [CrossRef]
- Lin, S.; Zhao, Y.; Chen, Q.; Jiang, X. Microwave drying optimization and kinetic modeling of fly ash from municipal solid waste incineration. Adv. Powder Technol. 2022, 33, 103720. [Google Scholar] [CrossRef]
- Chaves, R.P.F.; de Araújo, A.L.; Lopes, A.S.; Pena, R.d.S. Convective Drying of Purple Basil (Ocimum basilicum L.) Leaves and Stability of Chlorophyll and Phenolic Compounds during the Process. Plants 2022, 12, 127. [Google Scholar] [CrossRef] [PubMed]
- Karimi, S.; Layeghinia, N.; Abbasi, H. Microwave pretreatment followed by associated microwave-hot air drying of Gundelia tournefortii L.: Drying kinetics, energy consumption and quality characteristics. Heat Mass Transf. 2021, 57, 133–146. [Google Scholar] [CrossRef]
- Śledź, M.; Nowacka, M.; Wiktor, A.; Witrowa-Rajchert, D. Selected chemical and physico-chemical properties of microwave-convective dried herbs. Food Bioprod. Process. 2013, 91, 421–428. [Google Scholar] [CrossRef]
- Demirhan, E.; Özbek, B. Color Change Kinetics of Microwave-Dried Basil. Dry. Technol. 2009, 27, 156–166. [Google Scholar] [CrossRef]
- Di Cesare, L.F.; Forni, E.; Viscardi, D.; Nani, R.C. Changes in the Chemical Composition of Basil Caused by Different Drying Procedures. J. Agric. Food Chem. 2003, 51, 3575–3581. [Google Scholar] [CrossRef]
- Yilmaz, A.; Alibas, I. The impact of drying methods on quality parameters of purple basil leaves. J. Food Process. Preserv. 2021, 45, e15638. [Google Scholar] [CrossRef]
- Filip, S.; Vidović, S.; Vladić, J.; Pavlić, B.; Adamović, D.; Zeković, Z. Chemical composition and antioxidant properties of Ocimum basilicum L. extracts obtained by supercritical carbon dioxide extraction: Drug exhausting method. J. Supercrit. Fluids 2016, 109, 20–25. [Google Scholar] [CrossRef]
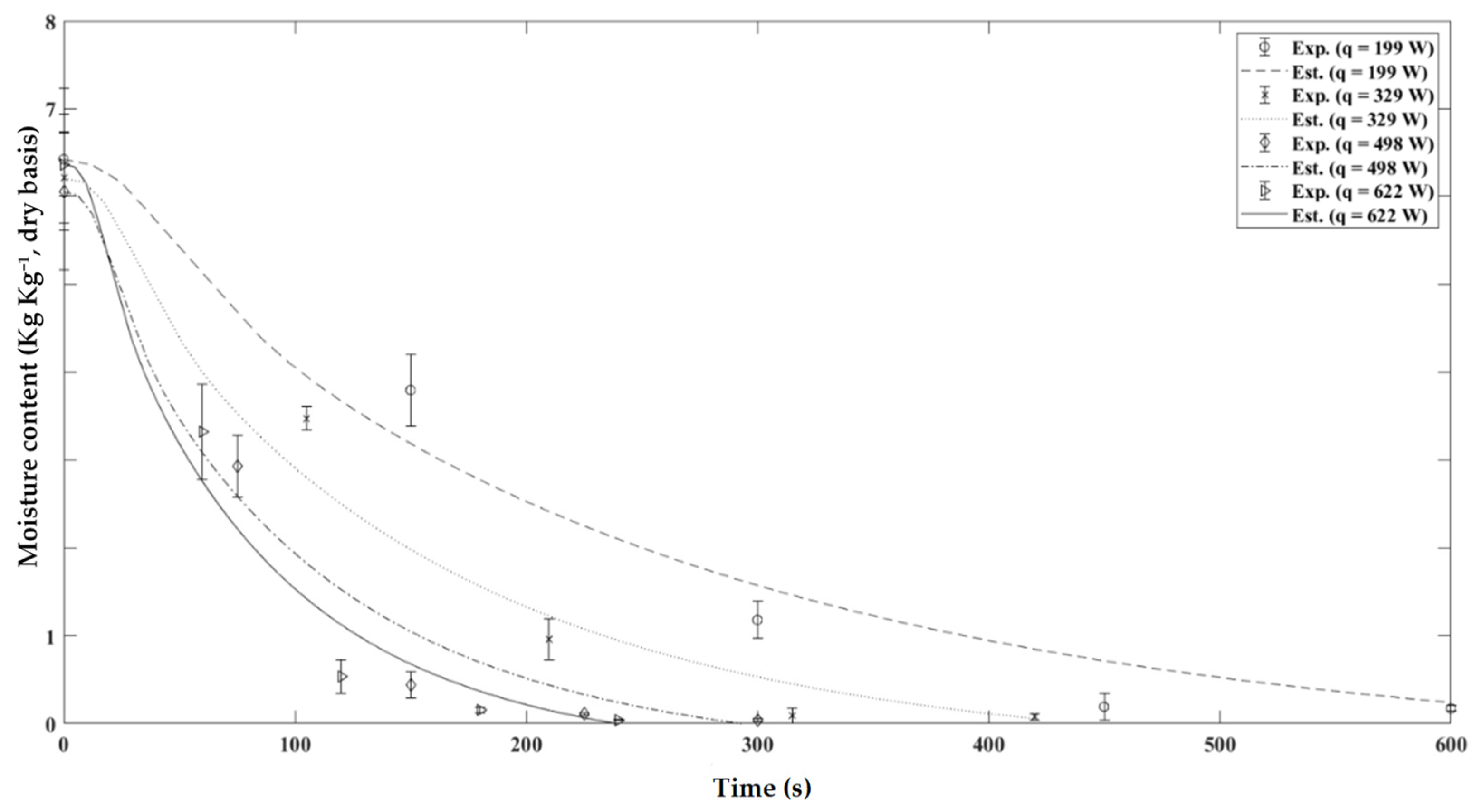


| q (W) | D (m2 s−1) | R2adj | RMSE |
|---|---|---|---|
| 199 | 8.253 × 10−11 | 0.974 | 0.439 |
| 329 | 1.265 × 10−10 | 0.976 | 0.411 |
| 498 | 2.125 × 10−10 | 0.982 | 0.346 |
| 622 | 2.468 × 10−10 | 0.976 | 0.429 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
López-Hernández, M.; Montealegre, M.Á.; Criollo, J.; Váquiro, H.A.; Sandoval-Aldana, A. Quality Parameters and the Modeling of the Microwave Drying Kinetics of Basil ‘Nufar’ (Ocimum basilicum L.) Leaves. AgriEngineering 2024, 6, 2417-2428. https://doi.org/10.3390/agriengineering6030141
López-Hernández M, Montealegre MÁ, Criollo J, Váquiro HA, Sandoval-Aldana A. Quality Parameters and the Modeling of the Microwave Drying Kinetics of Basil ‘Nufar’ (Ocimum basilicum L.) Leaves. AgriEngineering. 2024; 6(3):2417-2428. https://doi.org/10.3390/agriengineering6030141
Chicago/Turabian StyleLópez-Hernández, Martha, Miguel Ángel Montealegre, Jenifer Criollo, Henry Alexander Váquiro, and Angélica Sandoval-Aldana. 2024. "Quality Parameters and the Modeling of the Microwave Drying Kinetics of Basil ‘Nufar’ (Ocimum basilicum L.) Leaves" AgriEngineering 6, no. 3: 2417-2428. https://doi.org/10.3390/agriengineering6030141
APA StyleLópez-Hernández, M., Montealegre, M. Á., Criollo, J., Váquiro, H. A., & Sandoval-Aldana, A. (2024). Quality Parameters and the Modeling of the Microwave Drying Kinetics of Basil ‘Nufar’ (Ocimum basilicum L.) Leaves. AgriEngineering, 6(3), 2417-2428. https://doi.org/10.3390/agriengineering6030141






