Non-Linearity Flux of Fractional Transport Density Equation in Traffic Flow with Solutions
Abstract
1. Introduction
2. Fractional Derivative
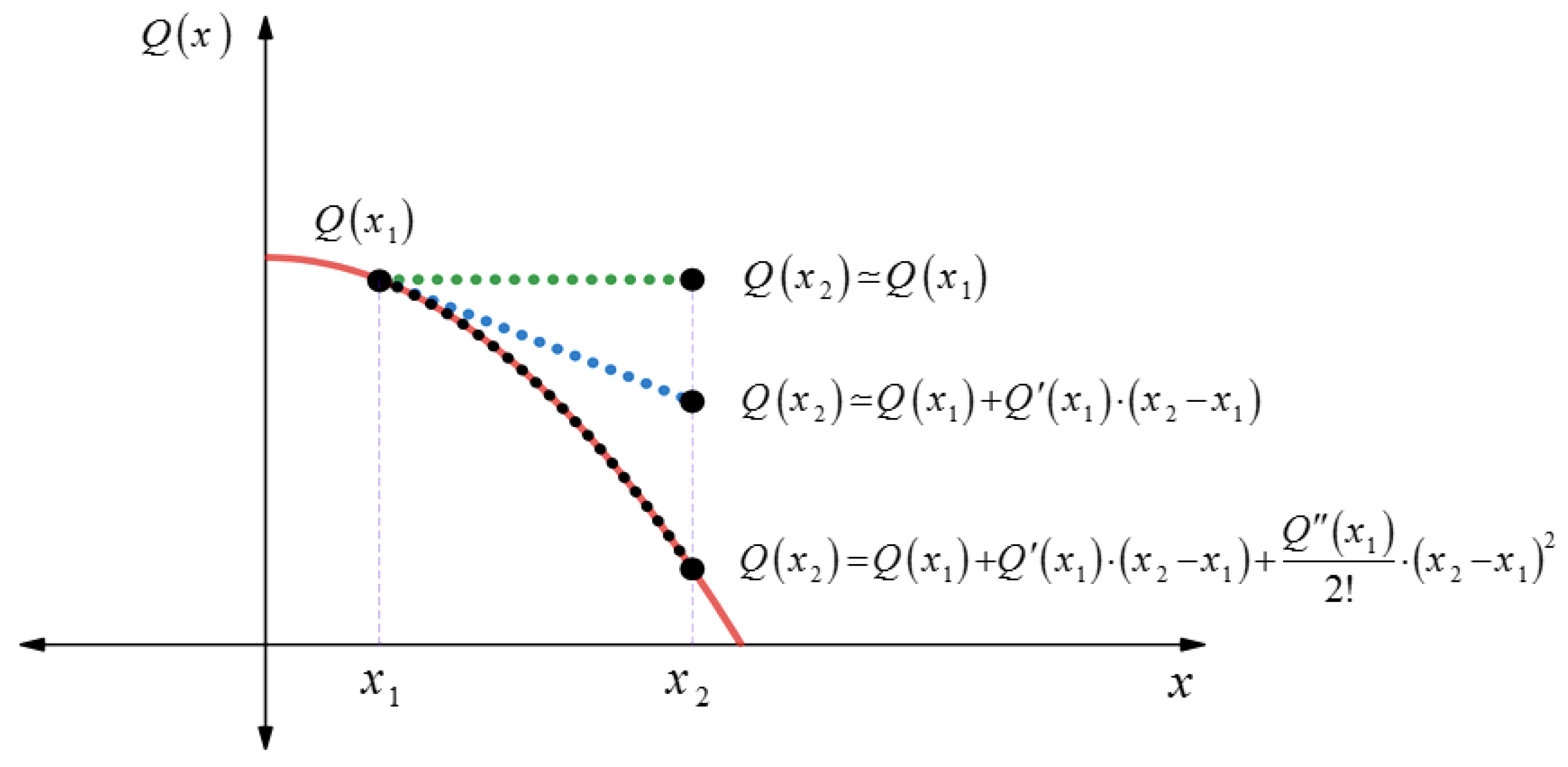
3. Truncating Series
- is continuous on .
4. Derivation and Validation
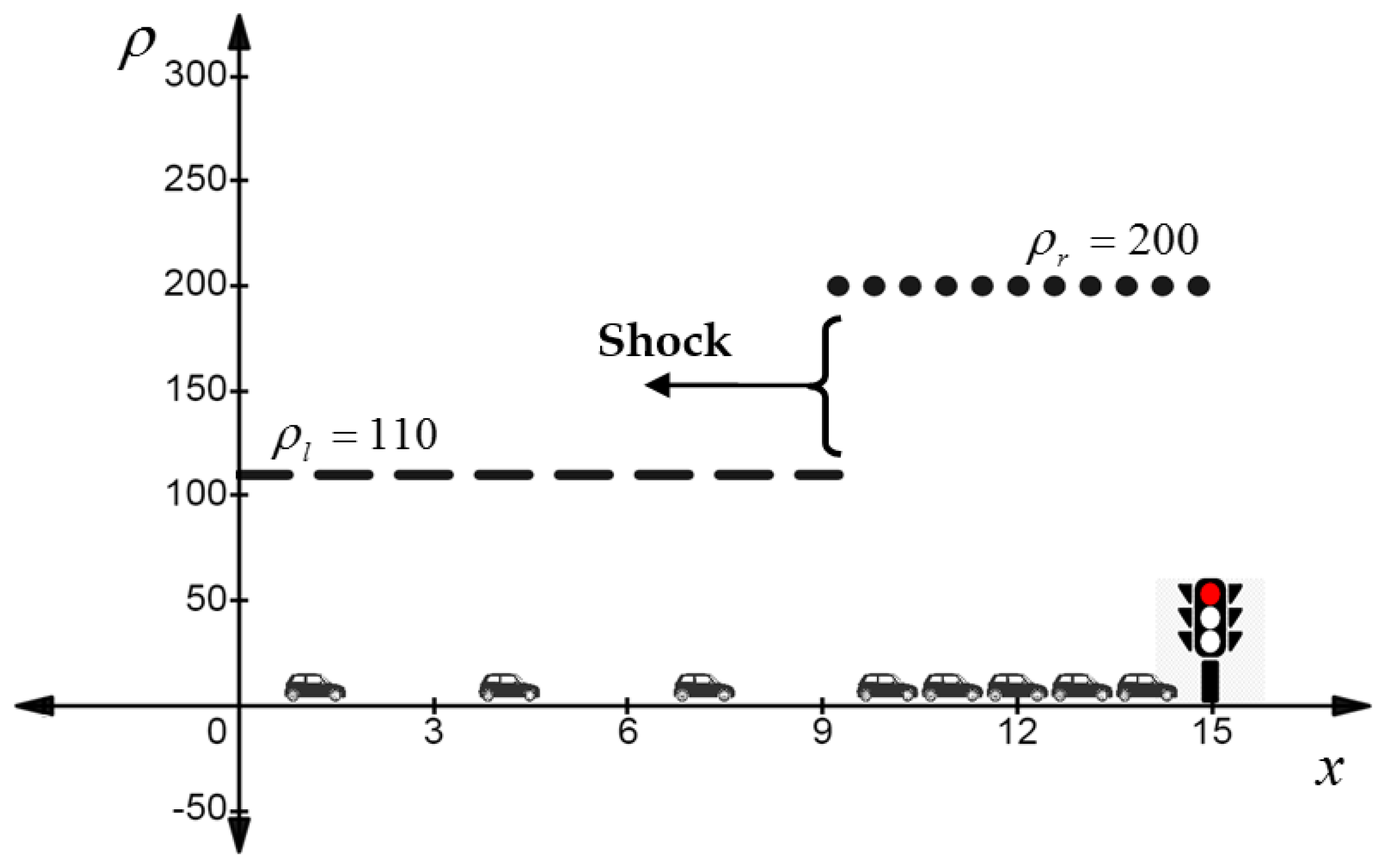
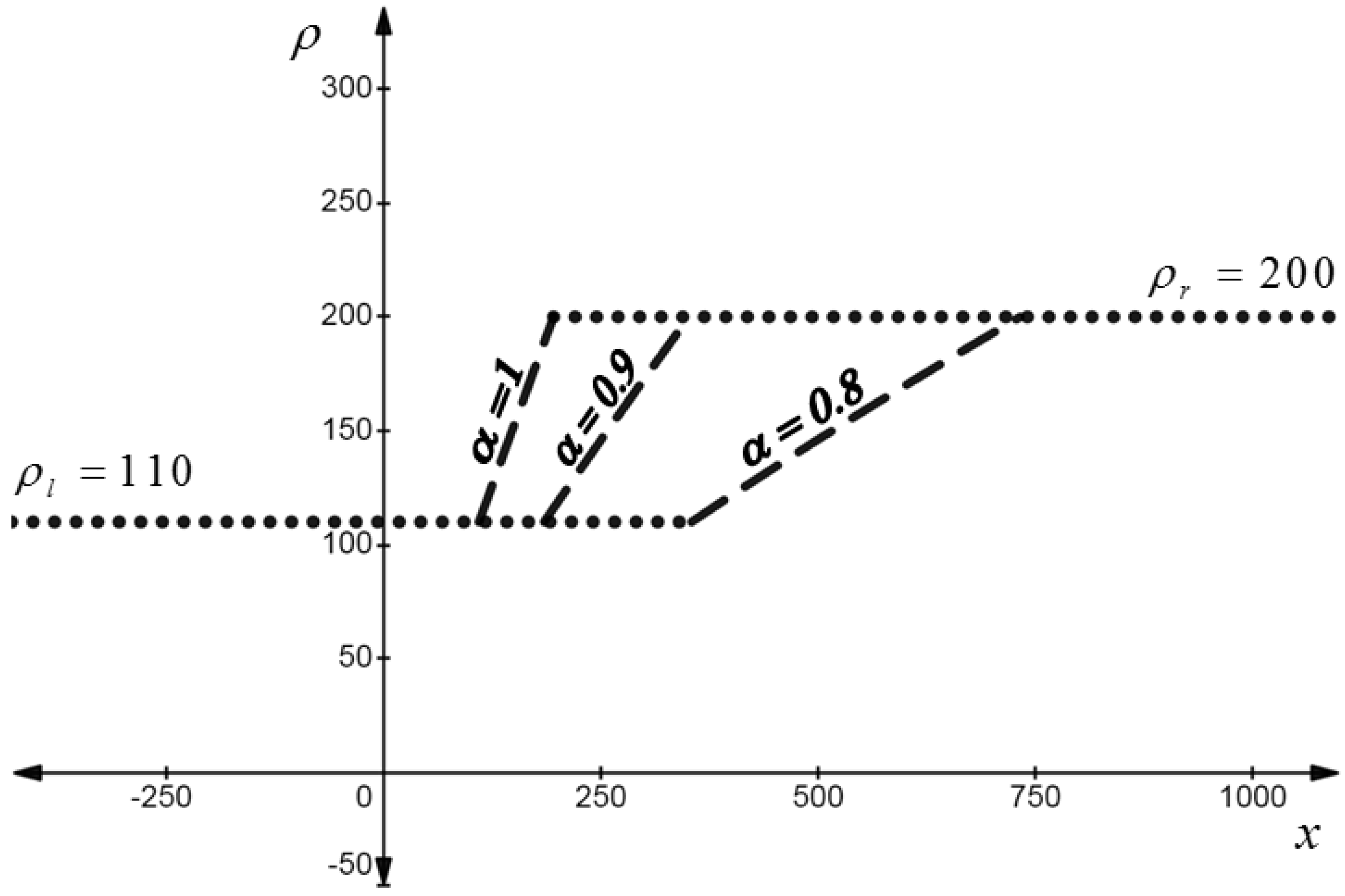
5. Characteristics Method
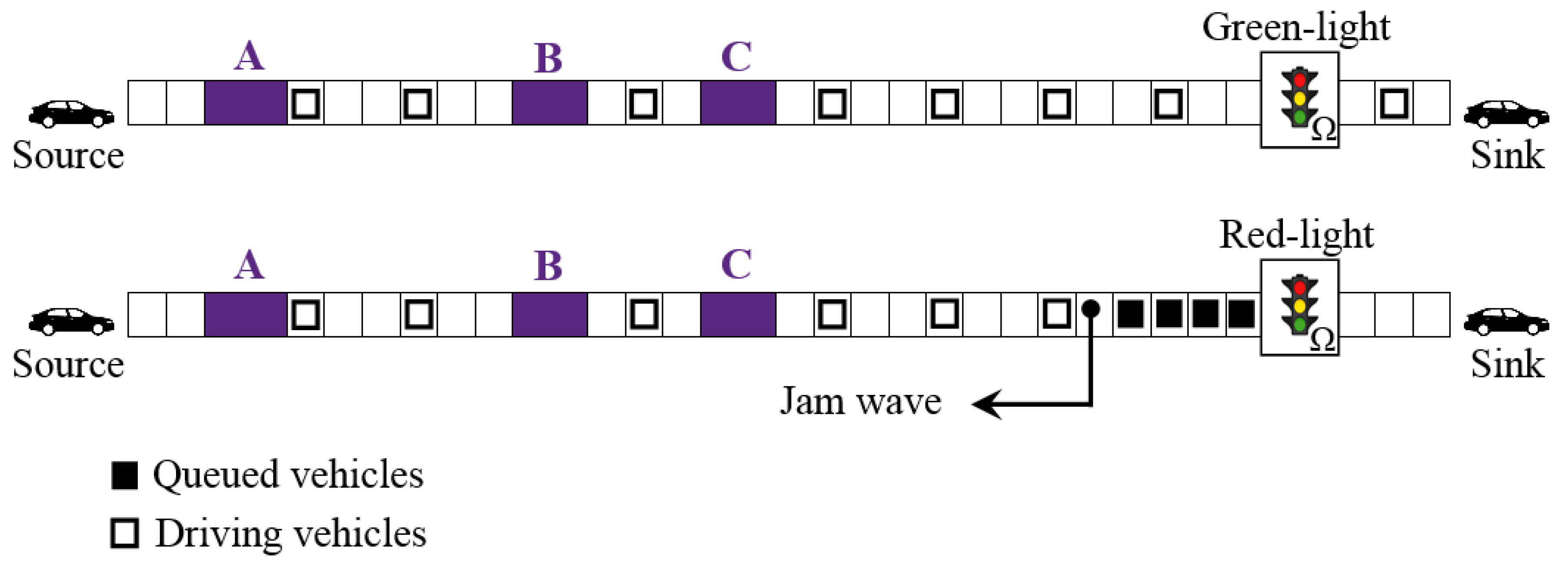
6. Discussion
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Erramilli, A.; Willinger, W.; Pruthi, P. Fractal traffic flows in high-speed communications networks. Fractals 1994, 2, 409–412. [Google Scholar] [CrossRef]
- Chen, W.; Sun, H.; Zhang, X.; Korošak, D. Anomalous diffusion modeling by fractal and fractional derivatives. Comput. Math. Appl. 2010, 59, 1754–1758. [Google Scholar] [CrossRef]
- Magin, R.L. Fractional Calculus in Bioengineering; Begell House: Danbury, CT, USA, 2006. [Google Scholar]
- Hadid, S.B.; Ibrahim, R.W.; Altulea, D.; Momani, S. Solvability and stability of a fractional dynamical system of the growth of COVID19 with approximate solution by fractional Chebyshev polynomials. Adv. Differ. Equ. 2020, 2020, 338. [Google Scholar] [CrossRef]
- Iqbal, N.; Akgul, A.; Shah, R.; Bariq, A.; Mossa Al-Sawalha, M.; Ali, A. On solutions of fractional-order gas dynamics equation by effective techniques. J. Funct. Spaces 2022, 2022, 3341754. [Google Scholar] [CrossRef]
- Mainardi, F. Fractional Calculus: Some Basic Problems in Continuum and Statistical Mechanics. In Fractals and Fractional Calculus in Continuum Mechanics; Springer: New York, NY, USA, 1997. [Google Scholar]
- Laskin, N. Fractional market dynamics. Phys. A Stat. Mech. Its Appl. 2000, 287, 482–492. [Google Scholar] [CrossRef]
- Bagley, R.L.; Calico, R.A. Fractional order state equations for the control of viscoelastically damped structures. J. Guid. Control Dyn. 1991, 14, 304–311. [Google Scholar] [CrossRef]
- Ali, F.; Sheikh, N.A. Introductory Chapter: Fluid Flow Problems. In Fluid Flow Problems; IntechOpen: London, UK, 2018. [Google Scholar]
- Lighthill, M.J.; Whitham, G.B. On kinematic waves-II. A theory of traffic flow on long crowded roads. Proc. R. Soc. London Ser. A Math. Phys. Sci. 1955, 229, 317–345. [Google Scholar]
- Richards, P.I. Shock waves on the highway. Oper. Res. 1956, 4, 42–51. [Google Scholar] [CrossRef]
- Mason, A. Advanced Differential Equations; ED-Tech: London, UK, 2019. [Google Scholar]
- Peitgen, H.-O.; Jurgens, H.; Saupe, D. Chaos and Fractals. In New Frontiers of Science; Springer: Berlin/Heidelberg, Germany, 1992. [Google Scholar]
- Boris, S.K. Complex dynamics of traffic management. In Encyclopedia of Complexity and Systems Science; Springer: New York, NY, USA, 2009. [Google Scholar]
- May, A.D. Traffic Flow Fundamentals; Prentice Hall: Englewood Cliffs, NJ, USA, 1990. [Google Scholar]
- Shang, P.; Wan, M.; Kama, S. Fractal nature of highway traffic data. Comput. Math. Appl. 2007, 54, 107–116. [Google Scholar] [CrossRef][Green Version]
- Wang, L.F.; Yang, X.J.; Baleanu, D.; Cattani, C.; Zhao, Y. Fractal dynamical model of vehicular traffic flow within the local fractional conservation laws. Abstr. Appl. Anal. 2014, 2014, 1–5. [Google Scholar] [CrossRef]
- Kumar, D.; Tchier, F.; Singh, J.; Baleanu, D. An efficient computational technique for fractal vehicular traffic flow. Entropy 2018, 20, 259. [Google Scholar] [CrossRef]
- Jassim, H.K. On approximate methods for fractal vehicular traffic flow. TWMS J. App. Eng. Math. 2017, 7, 58–65. [Google Scholar]
- Wheatcraft, S.W.; Meerschaert, M.M. Fractional conservation of mass. Adv. Water Resour. 2008, 31, 1377–1381. [Google Scholar] [CrossRef]
- Almeida, R. A Caputo fractional derivative of a function with respect to another function. Commun. Nonlinear Sci. Numer. Simul. 2017, 44, 460–481. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional Differential Equations; Academic Press: San Diego, CA, USA, 1999; ISBN 0-1255-8840-2. [Google Scholar]
- Abu-Shady, M.; Kaabar, K.A. A Generalized definition of the fractional derivative with applications. Math. Probl. Eng. 2021, 2021, 1–9. [Google Scholar] [CrossRef]
- Martínez, F.; Kaabar, K.A. A Novel theoretical investigation of the Abu-Shady–Kaabar fractional derivative as a modeling tool for science and engineering. Comput. Math. Methods Med. 2022, 2022, 1–8. [Google Scholar] [CrossRef] [PubMed]
- Usero, D. Fractional Taylor Series for Caputo Fractional Derivatives: Construction of Numerical Schemes. 2008. Preprint. Available online: http://www.fdi.ucm.es/profesor/lvazquez/calcfrac/docs/paper_Usero.pdf (accessed on 2 June 2022).
- Vargas, A.M. Finite difference method for solving fractional differential equations at irregular meshes. Math. Comput. Simul. 2022, 193, 204–216. [Google Scholar] [CrossRef]
- Olayode, I.O.; Tartibu, L.K.; Campisi, T. Stability analysis and prediction of traffic flow of trucks at road intersections based on heterogenous optimal velocity and artificial neural network model. Smart Cities 2022, 5, 1092–1114. [Google Scholar] [CrossRef]
- Ostoja-Starzewski, M.; Li, J.; Joumaa, H.; Demmie, P. From fractal media to continuum mechanics. ZAMM 2014, 94, 373–401. [Google Scholar] [CrossRef]
- Cai, W.; Chen, W.; Wang, F. Three-dimensional Hausdorff derivative diffusion model for isotropic/anisotropic fractal porous media. Therm. Sci. 2018, 22, S1–S6. [Google Scholar] [CrossRef]
- Tarasov, V. Anisotropic fractal media by vector calculus in non-integer dimensional space. J. Math. Phys. 2014, 55, 083510. [Google Scholar] [CrossRef]
- El-Nabulsi, R.A.; Anukool, W. Quantum dots and cuboid quantum wells in fractal dimensions with position-dependent masses. Appl. Phys. A 2021, 127, 856. [Google Scholar] [CrossRef]
- Balankin, A.S. A continuum framework for mechanics of fractal materials I: From fractional space to continuum with fractal metric. Eur. J. Phys. B 2015, 88, 90. [Google Scholar] [CrossRef]
- El-Nabulsi, R.A.; Anukool, W. Fractal dimension modeling of seismology and earthquakes dynamics. Acta Mech. 2022, 233, 2107–2122. [Google Scholar] [CrossRef]
- Tarasov, V.E. Fractional Dynamics: Applications of Fractional Calculus to Dynamics of Particles, Fields, and Media; Springer: Berlin, Germany, 2010. [Google Scholar]
- Li, J.; Ostoja-Starzewski, M. Comment on hydrodynamics of fractal continuum flow and map of fluid flow in fractal porous medium into fractal continuum flow. Phys. Rev. E 2013, 88, 057001. [Google Scholar] [CrossRef] [PubMed]
- Tarasov, V.E. Fractional systems and fractional Bogoliubov hierarchy equations. Phys. Rev. E 2005, 71, 0111102. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Wang, L.F.; Zeng, S.D.; Zhao, Y. Local fractional Laplace variational iteration method for fractal vehicular traffic flow. Adv. Theor. Math. Phys. 2014, 2014, 649318. [Google Scholar] [CrossRef]
- Wu, G.C. A fractional characteristic method for solving fractional partial differential equations. Appl. Math. Lett. 2011, 24, 1046–1050. [Google Scholar] [CrossRef]
- Dubey, V.P.; Kumar, D.; Alshehri, H.M.; Dubey, S.; Singh, J. Computational analysis of local fractional LWR model occurring in a fractal vehicular traffic flow. Fractal Fract. 2022, 6, 426. [Google Scholar] [CrossRef]
- Dafermos, C. Hyperbolic Conservation Laws in Continuum Physics; Springer: Berlin, Germany, 2000. [Google Scholar]
- Glimm, J.G. Solutions in the large for nonlinear hyperbolic systems of equations. Commun. Pure Appl. Math. 1965, 18, 697–715. [Google Scholar] [CrossRef]
- Daganzo, C.F.; Geroliminis, N. An analytical approximation for the macroscopic fundamental diagram of urban traffic. Transp. Res. Part B Methodol. 2008, 42, 771–781. [Google Scholar] [CrossRef]
- Greenshields, B.D. A study of traffic capacity. In Proceedings of the Fourteenth Annual Meeting of the Highway Research Board, Washington, DC, USA, 6–7 December 1934; pp. 448–477. Available online: http://pubsindex.trb.org/view.aspx?id=120649 (accessed on 8 March 2022).
- Singh, N.; Kumar, K.; Goswami, P.; Jafari, H. Analytical method to solve the local fractional vehicular traffic flow model. Math. Methods Appl. Sci. 2022, 45, 3983–4001. [Google Scholar] [CrossRef]

| Time (h) | Fractional Order | Shock Location (km) | Shock Speed (km/h) |
|---|---|---|---|
| A | B | C | |
|---|---|---|---|
| 59.78 | 47.75 | 41.75 | |
| 69.80 | 55.80 | 48.81 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Soliby, R.M.; Jamaian, S.S. Non-Linearity Flux of Fractional Transport Density Equation in Traffic Flow with Solutions. Smart Cities 2022, 5, 1655-1669. https://doi.org/10.3390/smartcities5040084
Soliby RM, Jamaian SS. Non-Linearity Flux of Fractional Transport Density Equation in Traffic Flow with Solutions. Smart Cities. 2022; 5(4):1655-1669. https://doi.org/10.3390/smartcities5040084
Chicago/Turabian StyleSoliby, Rfaat Moner, and Siti Suhana Jamaian. 2022. "Non-Linearity Flux of Fractional Transport Density Equation in Traffic Flow with Solutions" Smart Cities 5, no. 4: 1655-1669. https://doi.org/10.3390/smartcities5040084
APA StyleSoliby, R. M., & Jamaian, S. S. (2022). Non-Linearity Flux of Fractional Transport Density Equation in Traffic Flow with Solutions. Smart Cities, 5(4), 1655-1669. https://doi.org/10.3390/smartcities5040084





