Cellulose-Based Acoustic Absorber with Macro-Controlled Properties
Abstract
1. Introduction
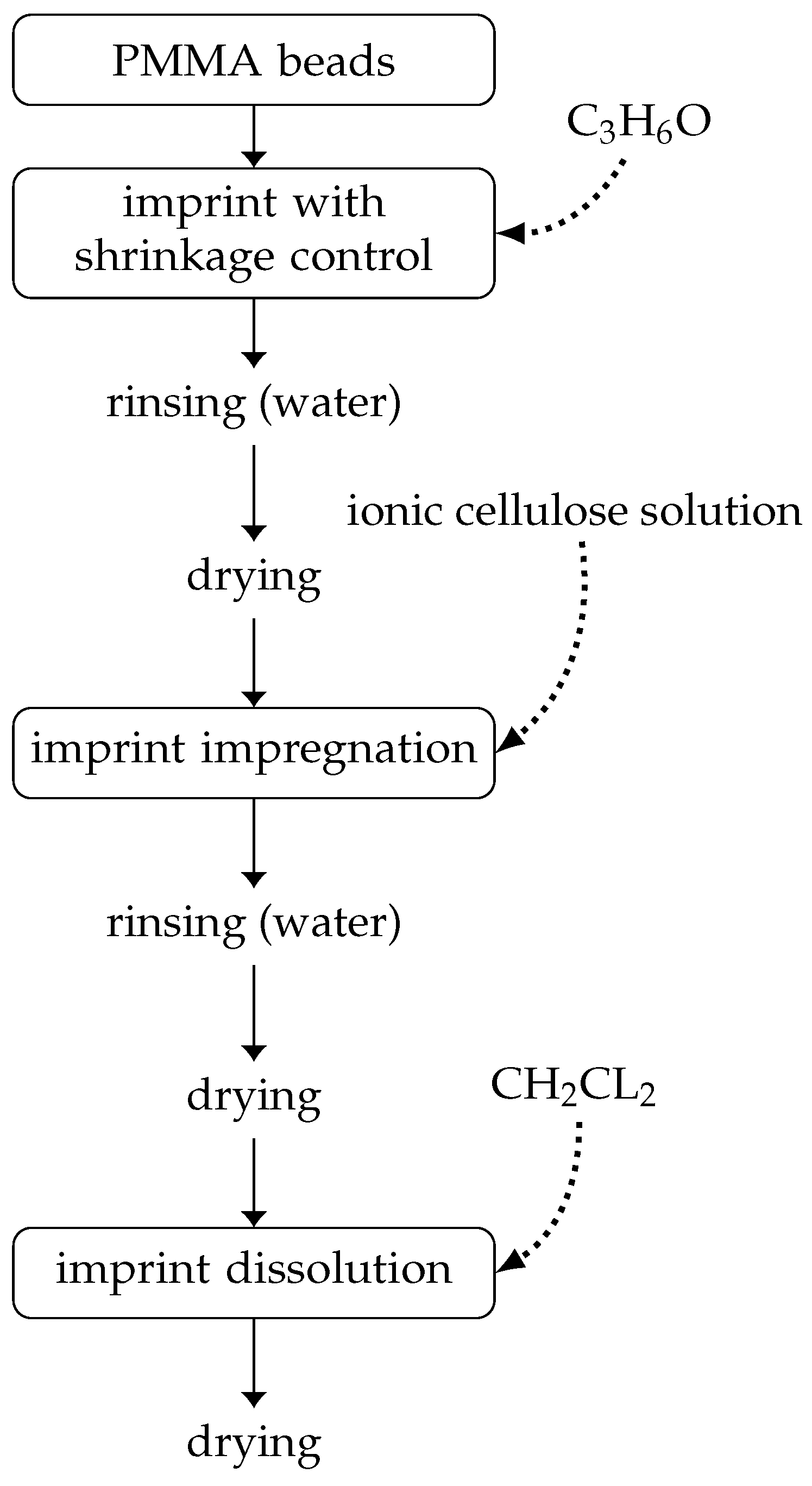
2. Materials and Methods
3. Results
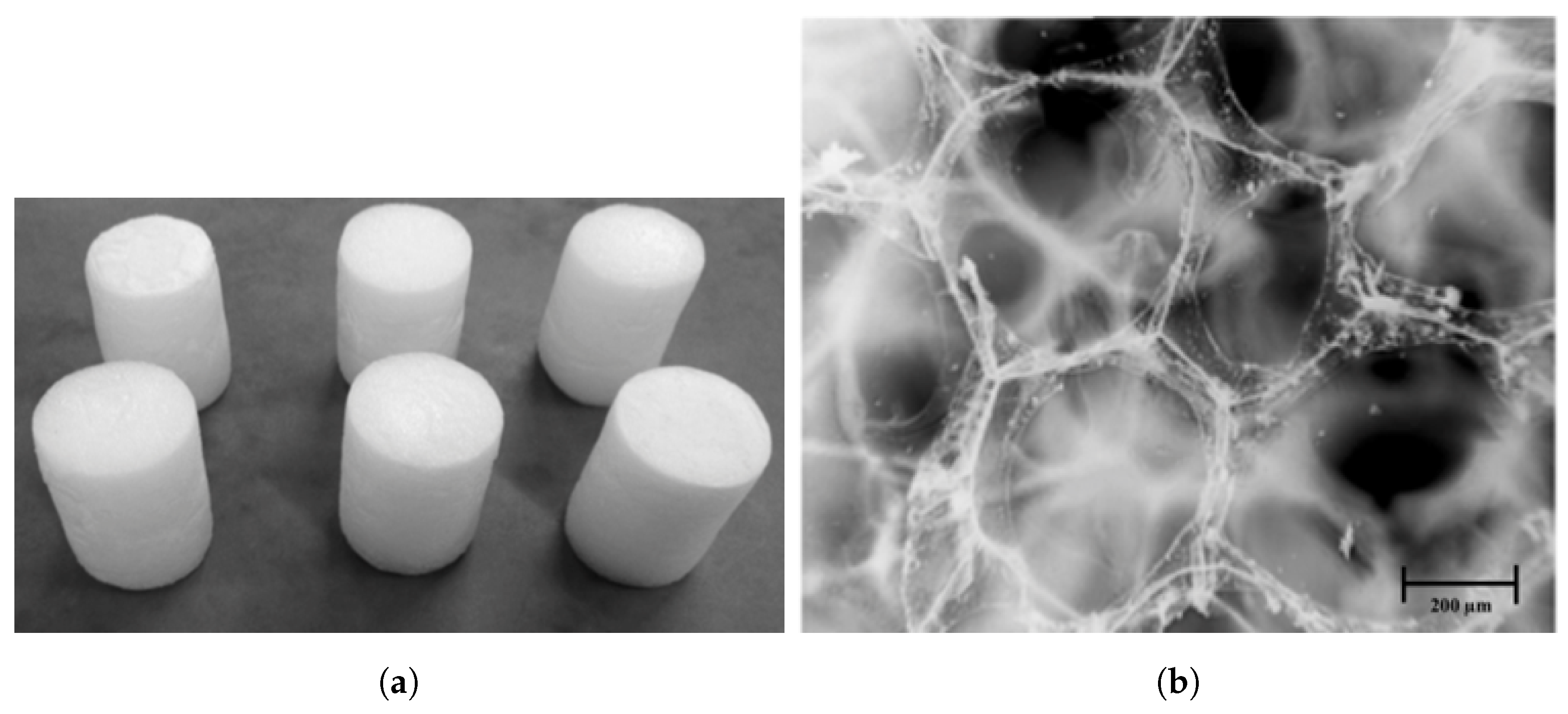
3.1. Basic Control of Organic Frame
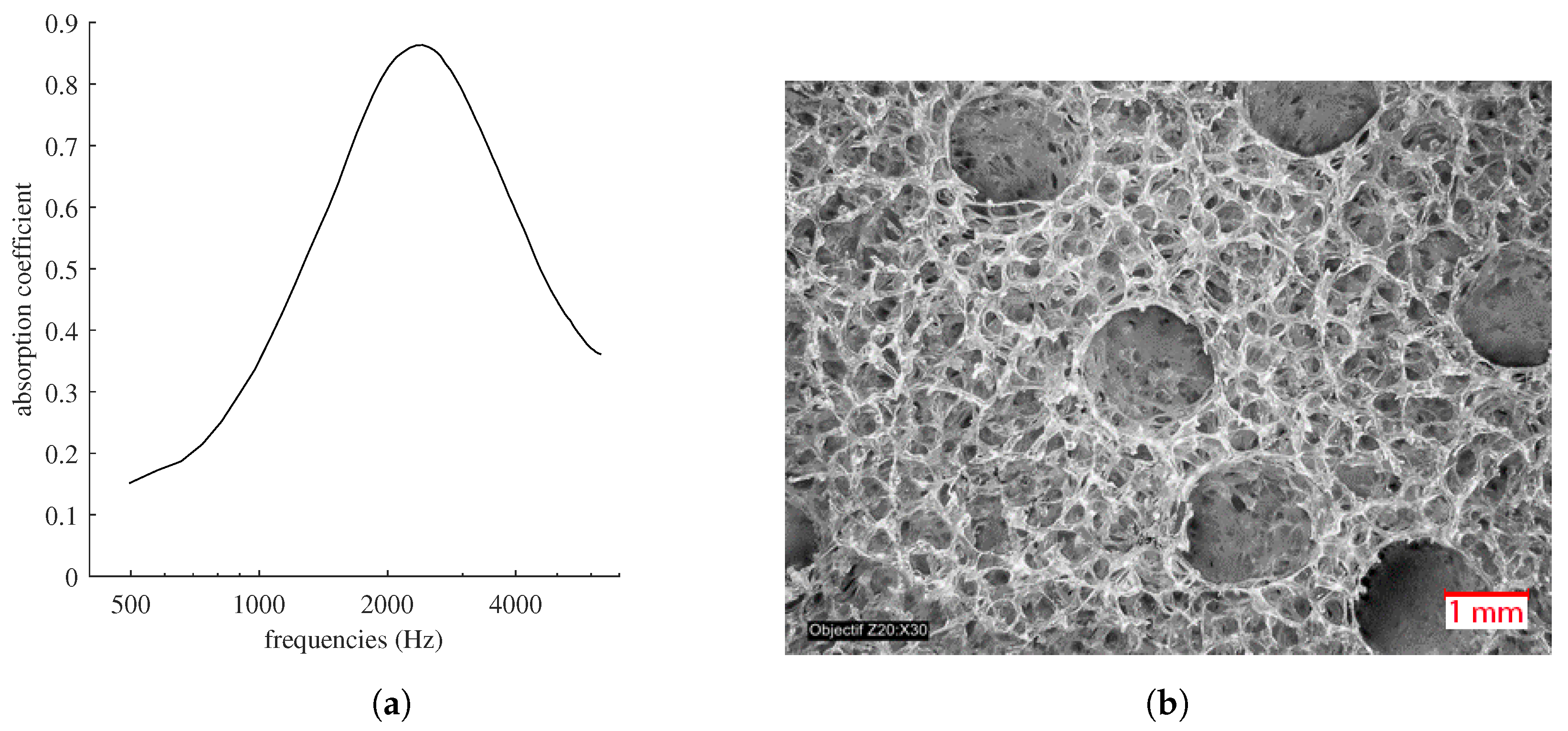
3.2. Double Porosity
3.3. Gradient-Porous Biomaterials
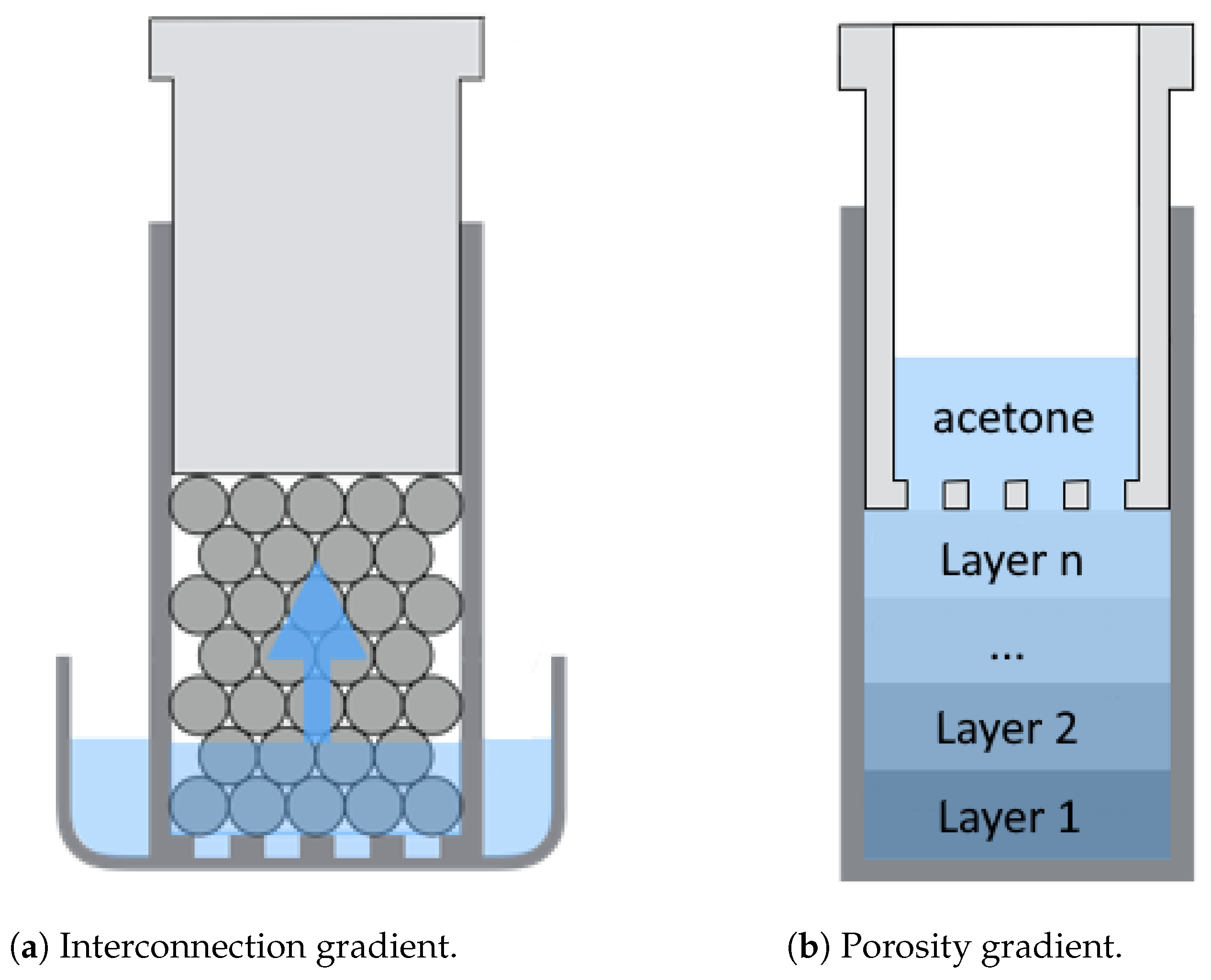
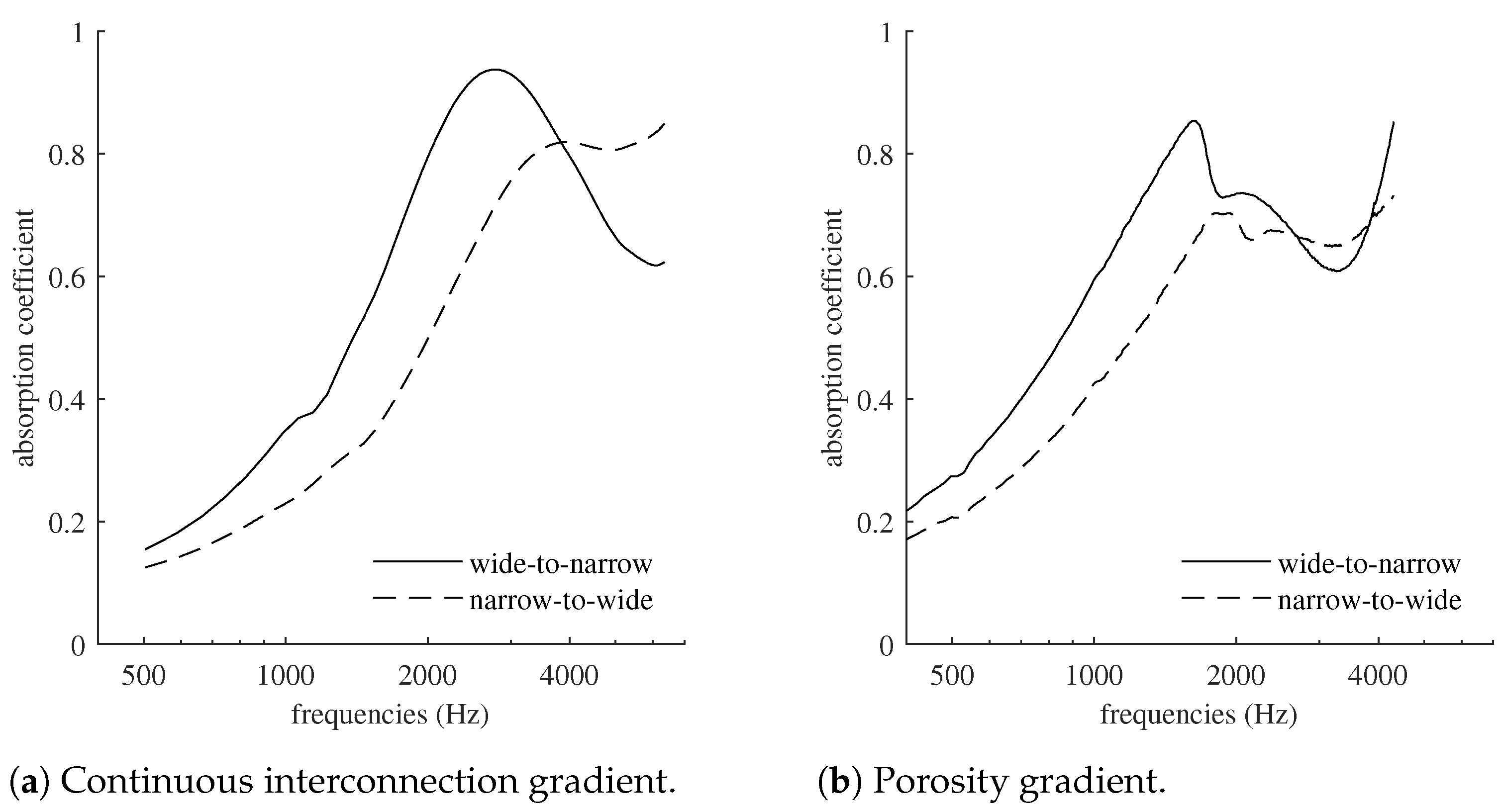
3.3.1. Continuous Interconnection Gradient
3.3.2. Porosity Gradient
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Al-Qararah, A.M.; Ekman, A.; Hjelt, T.; Ketoja, J.A.; Kiiskinen, H.; Koponen, A.; Timonen, J. A unique microstructure of the fiber networks deposited from foam–fiber suspensions. Colloids Surfaces Physicochem. Eng. Asp. 2015, 482, 544–553. [Google Scholar] [CrossRef]
- Ferreira, F.V.; Otoni, C.G.; Kevin, J.; Barud, H.S.; Lona, L.M.; Cranston, E.D.; Rojas, O.J. Porous nanocellulose gels and foams: Breakthrough status in the development of scaffolds for tissue engineering. Mater. Today 2020, 37, 126–141. [Google Scholar] [CrossRef]
- Zieliński, T.G.; Dauchez, N.; Boutin, T.; Leturia, M.; Wilkinson, A.; Chevillotte, F.; Bécot, F.X.; Venegas, R. Taking advantage of a 3D printing imperfection in the development of sound-absorbing materials. Appl. Acoust. 2022, 197, 108941. [Google Scholar] [CrossRef]
- Xue, Y.; Nobles, L.P.; Sharma, B.; Bolton, J.S. Designing hybrid aerogel-3D printed absorbers for simultaneous low frequency and broadband noise control. Mater. Des. 2024, 242, 113026. [Google Scholar] [CrossRef]
- Arjunan, A.; Baroutaji, A.; Robinson, J.; Vance, A.; Arafat, A. Acoustic metamaterials for sound absorption and insulation in buildings. Build. Environ. 2024, 251, 111250. [Google Scholar] [CrossRef]
- Godbold, O.; Soar, R.; Buswell, R. Implications of solid freeform fabrication on acoustic absorbers. Rapid Prototyp. J. 2007, 13, 298–303. [Google Scholar] [CrossRef]
- Setaki, F.; Tian, F.; Turrin, M.; Tenpierik, M.; Nijs, L.; Van Timmeren, A. 3D-printed sound absorbers: Compact and customisable at broadband frequencies. Archit. Struct. Constr. 2023, 3, 205–215. [Google Scholar] [CrossRef]
- Cardone, L.; De Rosa, S.; Petrone, G.; Catapane, G.; Squillace, A.; Landolfi, L.; Detry, A. Acoustic characteristics evaluation of an innovative metamaterial obtained through 3D printing technique. In Proceedings of the Aeronautics and Astronautics: AIDAA XXVII International Congress, Padova, Italia, 4–7 December 2023; Materials Research Forum LLC: Millersville PA, USA, 2023; Volume 37, p. 325. [Google Scholar]
- Cingolani, M.; Fratoni, G.; Barbaresi, L.; D’orazio, D.; Hamilton, B.; Garai, M. A trial acoustic improvement in a lecture Hall with MPP sound absorbers and FDTD acoustic simulations. Appl. Sci. 2021, 11, 2445. [Google Scholar] [CrossRef]
- Fusaro, G.; Barbaresi, L.; Cingolani, M.; Garai, M.; Ida, E.; Prato, A.; Schiavi, A. Investigation of the impact of additive manufacturing techniques on the acoustic performance of a coiled-up resonator. J. Acoust. Soc. Am. 2023, 153, 2921. [Google Scholar] [CrossRef]
- Li, X.; Chua, J.W.; Yu, X.; Li, Z.; Zhao, M.; Wang, Z.; Zhai, W. 3D-Printed Lattice Structures for Sound Absorption: Current Progress, Mechanisms and Models, Structural-Property Relationships, and Future Outlook. Adv. Sci. 2024, 11, 2305232. [Google Scholar] [CrossRef]
- Jagadeesh, P.; Puttegowda, M.; Rangappa, S.M.; Alexey, K.; Gorbatyuk, S.; Khan, A.; Doddamani, M.; Siengchin, S. A comprehensive review on 3D printing advancements in polymer composites: Technologies, materials, and applications. Int. J. Adv. Manuf. Technol. 2022, 121, 127–169. [Google Scholar] [CrossRef]
- Li, Y.; Ren, X.; Zhu, L.; Li, C. Biomass 3D printing: Principles, materials, post-processing and applications. Polymers 2023, 15, 2692. [Google Scholar] [CrossRef] [PubMed]
- Zheng, Z.; Li, Y.; Yang, W. Absorption properties of natural fiber-reinforced sandwich structures based on the fabric structures. J. Reinf. Plast. Compos. 2013, 32, 1561–1568. [Google Scholar] [CrossRef]
- Tiuc, A.E.; Vermeşan, H.; Gabor, T.; Vasile, O. Improved sound absorption properties of polyurethane foam mixed with textile waste. Energy Procedia 2016, 85, 559–565. [Google Scholar] [CrossRef]
- Zhu, X.; Kim, B.J.; Wang, Q.; Wu, Q. Recent Advances in the Sound Insulation Properties of Bio-based Materials. BioResources 2014, 9, 1764–1786. [Google Scholar] [CrossRef]
- Oldham, D.J.; Egan, C.A.; Cookson, R.D. Sustainable acoustic absorbers from the biomass. Appl. Acoust. 2011, 72, 350–363. [Google Scholar] [CrossRef]
- Del Rey, R.; Alba, J.; Arenas, J.P.; Sanchis, V.J. An empirical modelling of porous sound absorbing materials made of recycled foam. Appl. Acoust. 2012, 73, 604–609. [Google Scholar]
- Trematerra, A.; Lombardi, I. Acoustic Properties of Cellulose. In Proceedings of the IOP Conference Series: Materials Science and Engineering, Hyderabad, India, 13–14 July 2017; IOP Publishing: Bristol, UK, 2017; Volume 225, p. 012082. [Google Scholar]
- Arenas, J.P.; Rebolledo, J.; Rey Tormos, R.M.d.; Alba Fernández, J. Sound absorption properties of unbleached cellulose loose-fill insulation material. BioResources 2014, 9, 6227–6240. [Google Scholar] [CrossRef]
- Seciureanu, M.; Nastac, S.M.; Guiman, M.V.; Nechita, P. Cellulose Fibers-Based Porous Lightweight Foams for Noise Insulation. Polymers 2023, 15, 3796. [Google Scholar] [CrossRef]
- Nastac, S.M.; Nechita, P.; Guiman, M.V.; Roman, M.; Rosca, I.C. Applications of Xylan Derivatives to Improve the Functional Properties of Cellulose Foams for Noise Insulation. Polymers 2023, 15, 4648. [Google Scholar] [CrossRef]
- Muchlisinalahuddin, M.; Dahlan, H.; Mahardika, M.; Rusli, M. Cellulose-based Material for Sound Absorption and Its Application—A Short Review. In Proceedings of the BIO Web of Conferences, Bogor, Indonesia, 21–22 September 2023; EDP Sciences: Les Ulis, France, 2023; Volume 77, p. 01003. [Google Scholar]
- Miranda-Valdez, I.Y.; Coffeng, S.; Zhou, Y.; Viitanen, L.; Hu, X.; Jannuzzi, L.; Puisto, A.; Kostiainen, M.A.; Mäkinen, T.; Koivisto, J.; et al. Foam-formed biocomposites based on cellulose products and lignin. Cellulose 2023, 30, 2253–2266. [Google Scholar] [CrossRef]
- Taiwo, E.M.; Yahya, K.; Haron, Z. Potential of using natural fiber for building acoustic absorber: A review. In Proceedings of the Journal of Physics: Conference Series, Selangor, Malaysia, 7 March 2019; IOP Publishing: Bristol, UK, 2019; Volume 1262, p. 012017. [Google Scholar]
- Mahasaranon, S.; Horoshenkov, K.V.; Khan, A.; Benkreira, H. The effect of continuous pore stratification on the acoustic absorption in open cell foams. J. Appl. Phys. 2012, 111, 084901. [Google Scholar] [CrossRef]
- Geslain, A.; Groby, J.P.; Dazel, O.; Mahasaranon, S.; Horoshenkov, K.; Khan, A. An application of the Peano series expansion to predict sound propagation in materials with continuous pore stratification. J. Acoust. Soc. Am. 2012, 132, 208–215. [Google Scholar] [CrossRef] [PubMed]
- Descamps, M.; Duhoo, T.; Monchau, F.; Lu, J.; Hardouin, P.; Hornez, J.; Leriche, A. Manufacture of macroporous β-tricalcium phosphate bioceramics. J. Eur. Ceram. Soc. 2008, 28, 149–157. [Google Scholar] [CrossRef]
- Joly, N.; Granet, R.; Krausz, P. Crosslinking of cellulose by olefin metathesis. J. Carbohydr. Chem. 2003, 22, 47–55. [Google Scholar] [CrossRef]
- Panneton, R.; Gros, E. A missing mass method to measure the open porosity of porous solids. Acta Acust. United Acust. 2005, 91, 342–348. [Google Scholar]
- ISO 10534-2; Acoustics—Determination of Sound Absorption Coefficient and Impedance in Impedance Tubes. International Organization for Standardization: Geneva, Switzerland, 2001.
- Sambucci, M.; Sibai, A.; Fattore, L.; Martufi, R.; Lucibello, S.; Valente, M. Finite element multi-physics analysis and experimental testing for hollow brick solutions with lightweight and eco-sustainable cement mix. J. Compos. Sci. 2022, 6, 107. [Google Scholar] [CrossRef]
- Atalla, Y.; Panneton, R. Inverse acoustical characterization of open cell porous media using impedance tube measurements. Can. Acoust. 2005, 33, 11–24. [Google Scholar]
- Allard, J.; Allard, J. Sound propagation in cylindrical tubes and porous materials having cylindrical pores. In Propagation of Sound in Porous Media: Modelling Sound Absorbing Materials; John Wiley & Sons: Hoboken, NJ, USA, 1993; pp. 48–78. [Google Scholar]
- Sgard, F.C.; Olny, X.; Atalla, N.; Castel, F. On the use of perforations to improve the sound absorption of porous materials. Appl. Acoust. 2005, 66, 625–651. [Google Scholar] [CrossRef]
- Liu, X.; Ma, X.; Yu, C.; Xin, F. Sound absorption of porous materials perforated with holes having gradually varying radii. Aerosp. Sci. Technol. 2022, 120, 107229. [Google Scholar] [CrossRef]
- Doutres, O.; Atalla, N. Sound absorption properties of functionally graded polyurethane foam. In Proceedings of the INTER-NOISE and NOISE-CON Congress and Conference Proceedings, New York, NY, USA, 19–22 August 2012; Institute of Noise Control Engineering: Wakefield, MA, USA, 2012; Volume 2012, pp. 679–688. [Google Scholar]
- Sacristan, C.; Dupont, T.; Sicot, O.; Leclaire, P.; Verdière, K.; Panneton, R.; Gong, X.L. A mixture approach to the acoustic properties of a macroscopically inhomogeneous porous aluminum in the equivalent fluid approximation. J. Acoust. Soc. Am. 2016, 140, 2847–2855. [Google Scholar] [CrossRef]
- Verdejo, R.; Stämpfli, R.; Alvarez-Lainez, M.; Mourad, S.; Rodriguez-Perez, M.; Brühwiler, P.; Shaffer, M. Enhanced acoustic damping in flexible polyurethane foams filled with carbon nanotubes. Compos. Sci. Technol. 2009, 69, 1564–1569. [Google Scholar] [CrossRef]



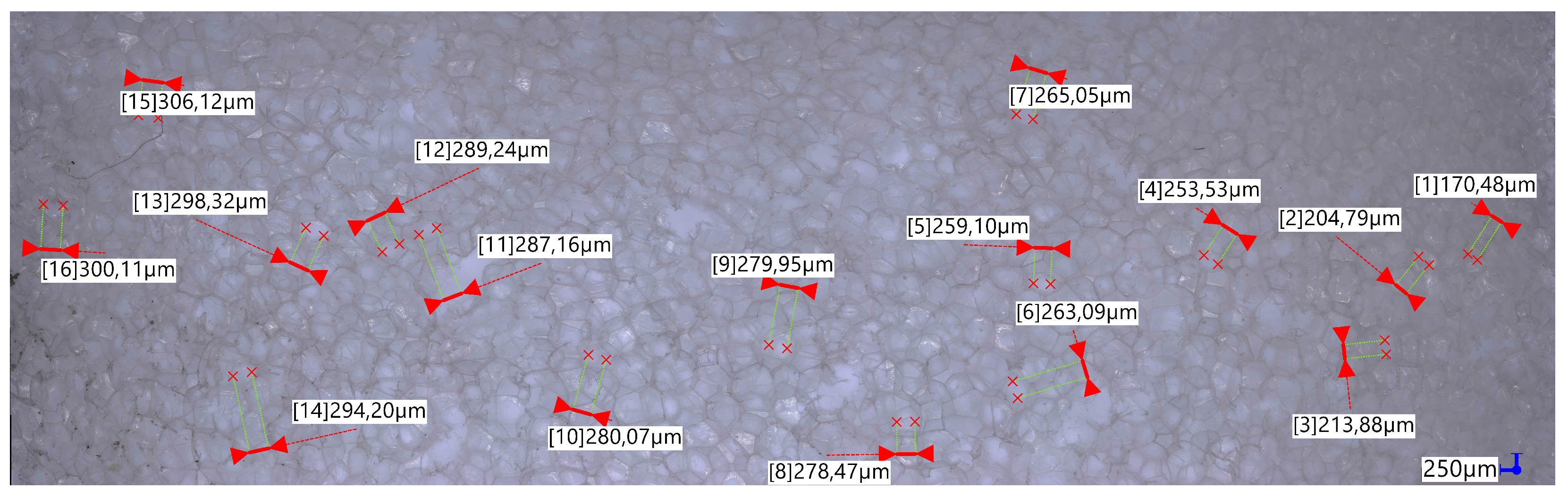
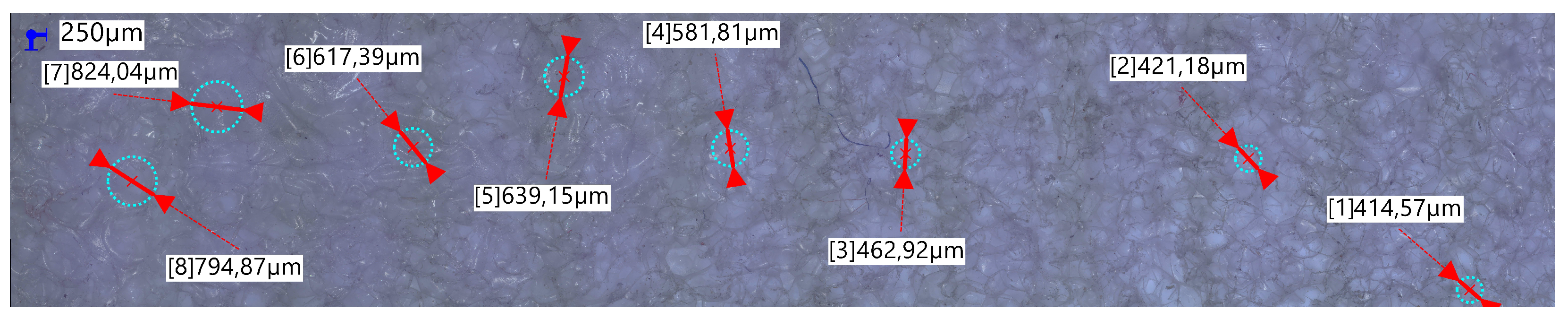
| Sample | m (g) | h (mm) | d (mm) | () | () | (%) | (kg/m3) |
|---|---|---|---|---|---|---|---|
| 1 | 0.5492 | 35.57 | 28.09 | 22.04 | 0.38 | 0.983 | 24.9 |
| 2 | 0.5720 | 35.25 | 28.13 | 21.91 | 0.39 | 0.982 | 26.1 |
| 3 | 0.5894 | 34.86 | 27.65 | 20.93 | 0.41 | 0.981 | 28.2 |
| 4 | 0.4612 | 35.12 | 25.79 | 18.35 | 0.32 | 0.983 | 25.1 |
| 5 | 0.5479 | 34.83 | 27.62 | 20.87 | 0.38 | 0.982 | 26.2 |
| 6 | 0.5792 | 35.78 | 27.57 | 21.36 | 0.40 | 0.981 | 27.1 |
| Sample | D (mm) | m (g) | h (mm) | d (mm) | (%) | (kg/m3) |
|---|---|---|---|---|---|---|
| A | 0.2–0.4 | 0.17 | 18.1 | 22.04 | 9 | 24.6 |
| B | 0.5–0.6 | 0.22 | 20.3 | 21.91 | 5 | 28.7 |
| C | 0.5–0.6 | 0.21 | 19.1 | 20.93 | 11 | 31.9 |
| D | 0.6–0.7 | 0.23 | 20.9 | 18.35 | 5 | 41.6 |
| E | 0.7–0.8 | 0.21 | 19.1 | 20.87 | 5.9 | 32.1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lefebvre, J.; Genestie, B.; Leblanc, A. Cellulose-Based Acoustic Absorber with Macro-Controlled Properties. Acoustics 2024, 6, 1088-1099. https://doi.org/10.3390/acoustics6040059
Lefebvre J, Genestie B, Leblanc A. Cellulose-Based Acoustic Absorber with Macro-Controlled Properties. Acoustics. 2024; 6(4):1088-1099. https://doi.org/10.3390/acoustics6040059
Chicago/Turabian StyleLefebvre, Jérôme, Benoit Genestie, and Alexandre Leblanc. 2024. "Cellulose-Based Acoustic Absorber with Macro-Controlled Properties" Acoustics 6, no. 4: 1088-1099. https://doi.org/10.3390/acoustics6040059
APA StyleLefebvre, J., Genestie, B., & Leblanc, A. (2024). Cellulose-Based Acoustic Absorber with Macro-Controlled Properties. Acoustics, 6(4), 1088-1099. https://doi.org/10.3390/acoustics6040059







