An Acoustic Simulation Method of the Japanese Vowels /i/ and /u/ by Using the Boundary Element Method
Abstract
1. Introduction
2. Materials and Methods
2.1. Participants
2.2. Simulation Method
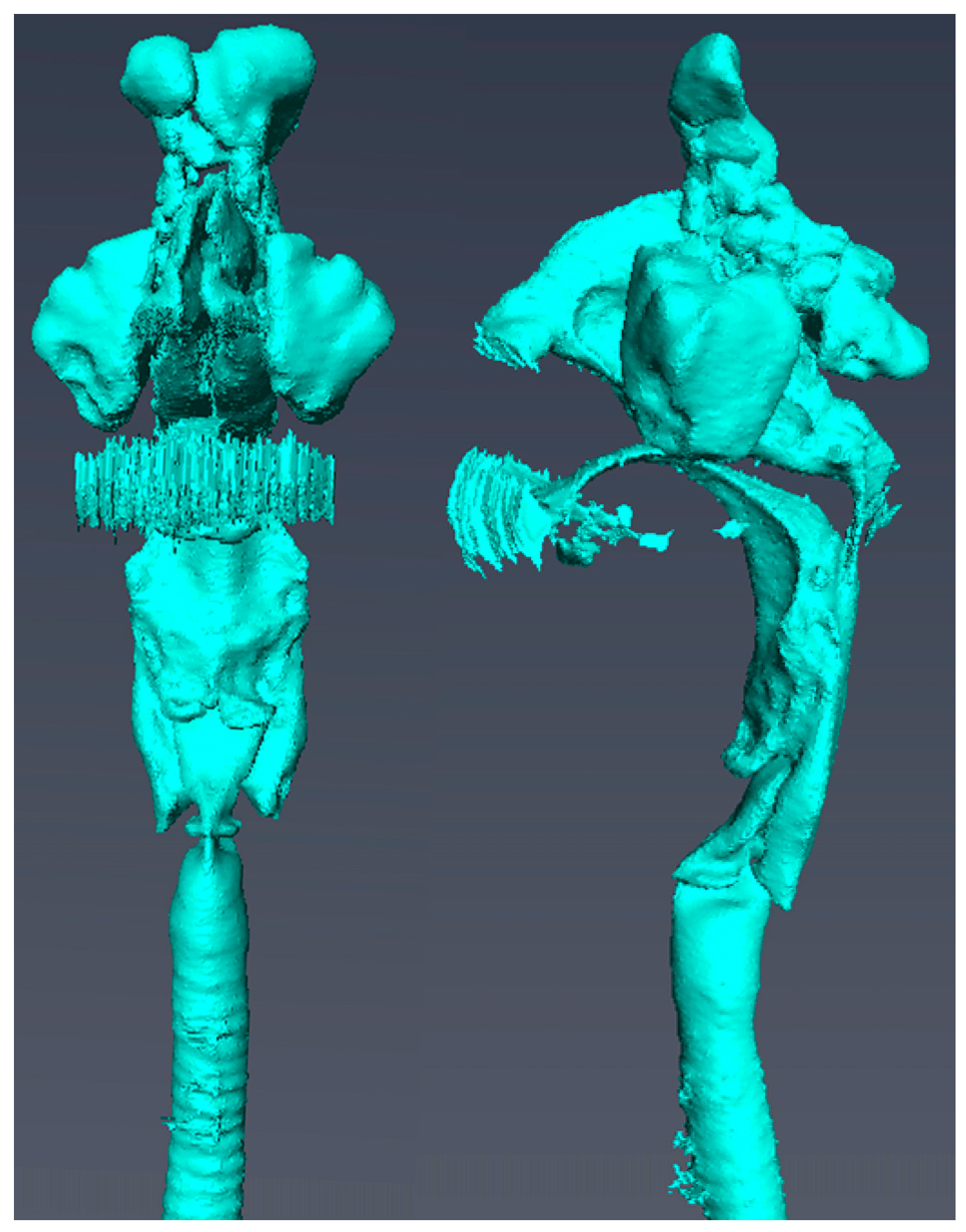
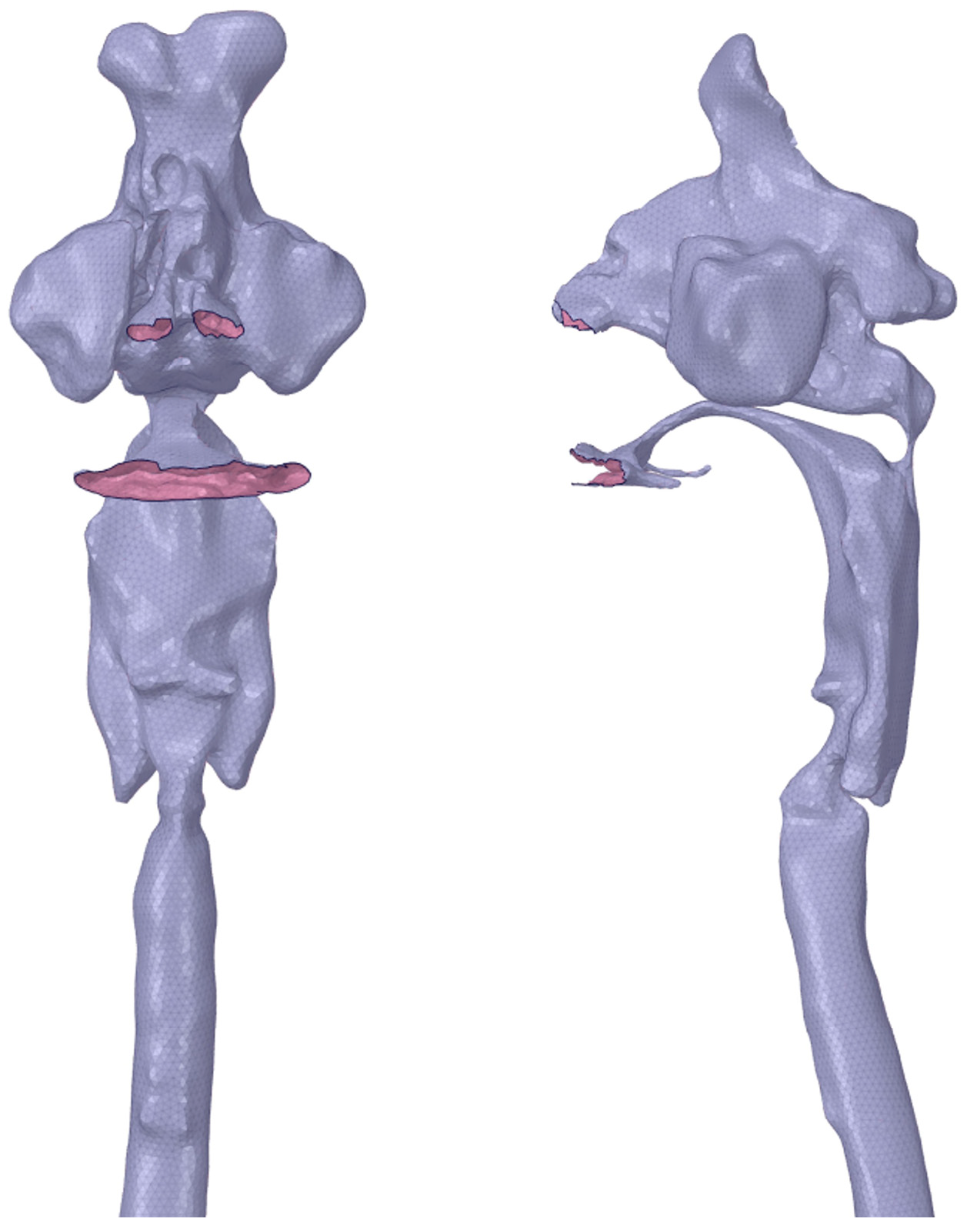
- A vocal tract model including the frontal sinus, ethmoid sinus, sphenoid sinus, maxillary sinus, nasal cavity, oral cavity, pharynx, larynx, and glottis is shown.
- A surface mesh model for analysis is shown. The nostrils, oral cavity, and tracheal bifurcation are opened.
2.3. Validity of the Acoustic Simulation
3. Results
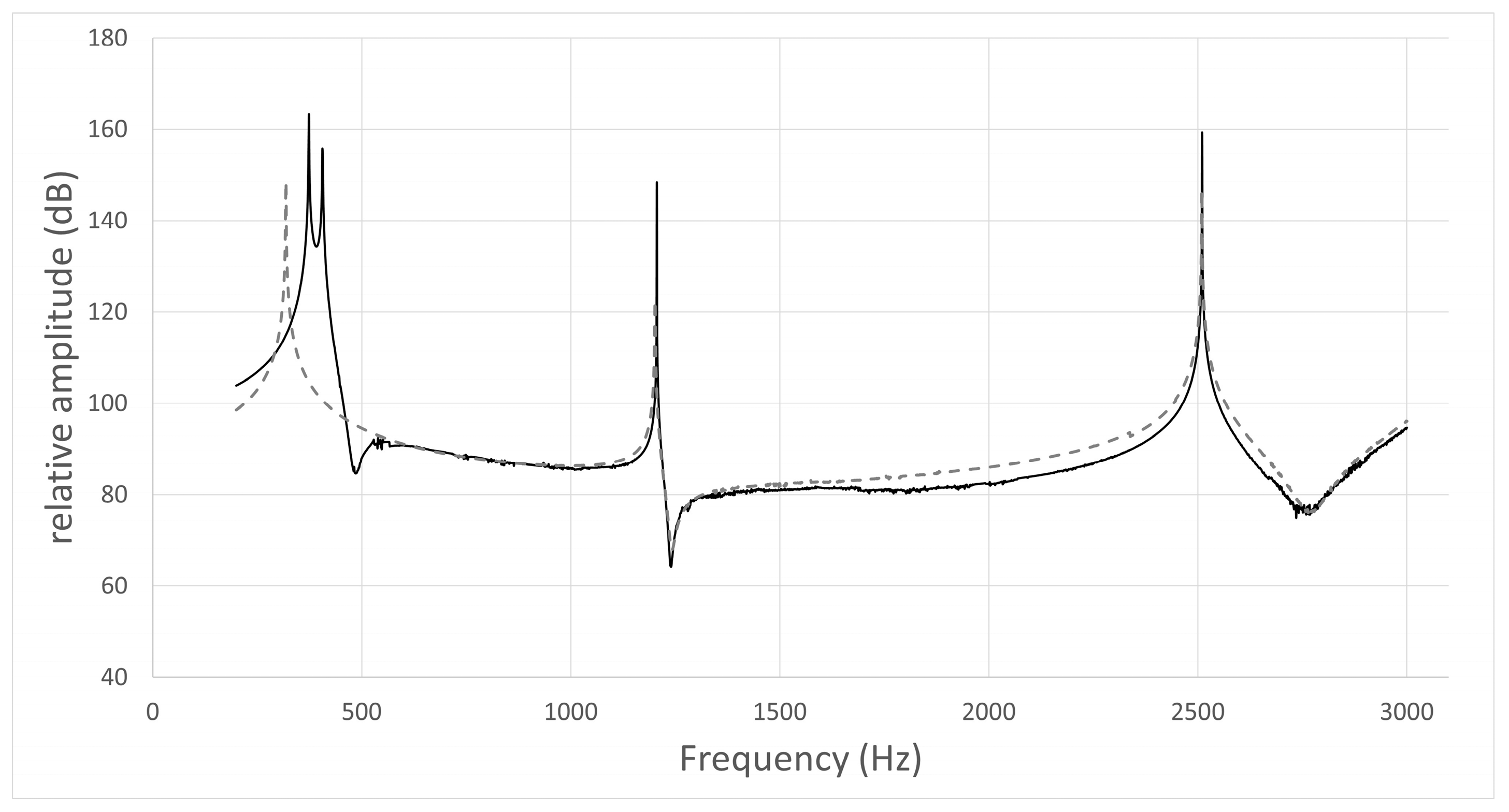
- The simulation result of the model including the nasal cavity and sinuses is indicated by the solid line, and that of the model excluding the nasal cavity and sinuses is indicated by the dotted line. In the former curve, the first peak is F1 (374 Hz), the second is due to nasal coupling for a pole–zero pair (pole: 406 Hz; zero: 486 Hz), the third is a pole–zero pair (pole: 1206 Hz; zero: 1240 Hz), and the fourth is F2 (2510 Hz). In the latter curve, the first peak is F1 (319 Hz), the second is not due to nasal coupling for a pole–zero pair (pole: 1202 Hz; zero: 1242 Hz), and the third is F2 (2509 Hz).
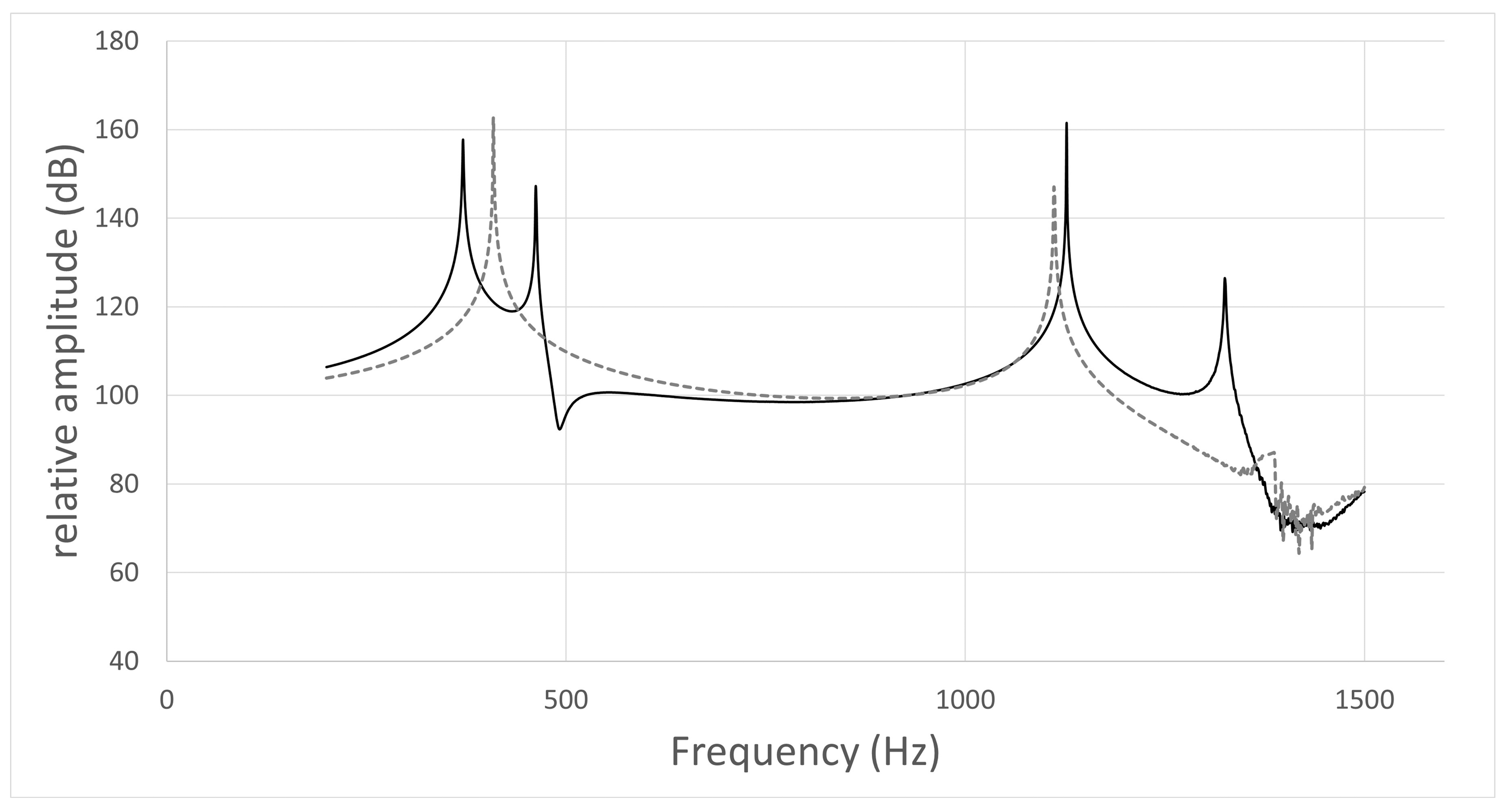
- The simulation result of the model including the nasal cavity and sinuses is indicated by the solid line, and that of the model not including the nasal cavity and sinuses by the dotted line. In the former curve, the first peak is F1 (371 Hz), the second is due to nasal coupling for a pole–zero pair (pole: 462 Hz; zero: 492 Hz), and the third is F2 (1127 Hz). In the latter curve, the first peak is F1 (409 Hz) and the second is F2 (1111 Hz).
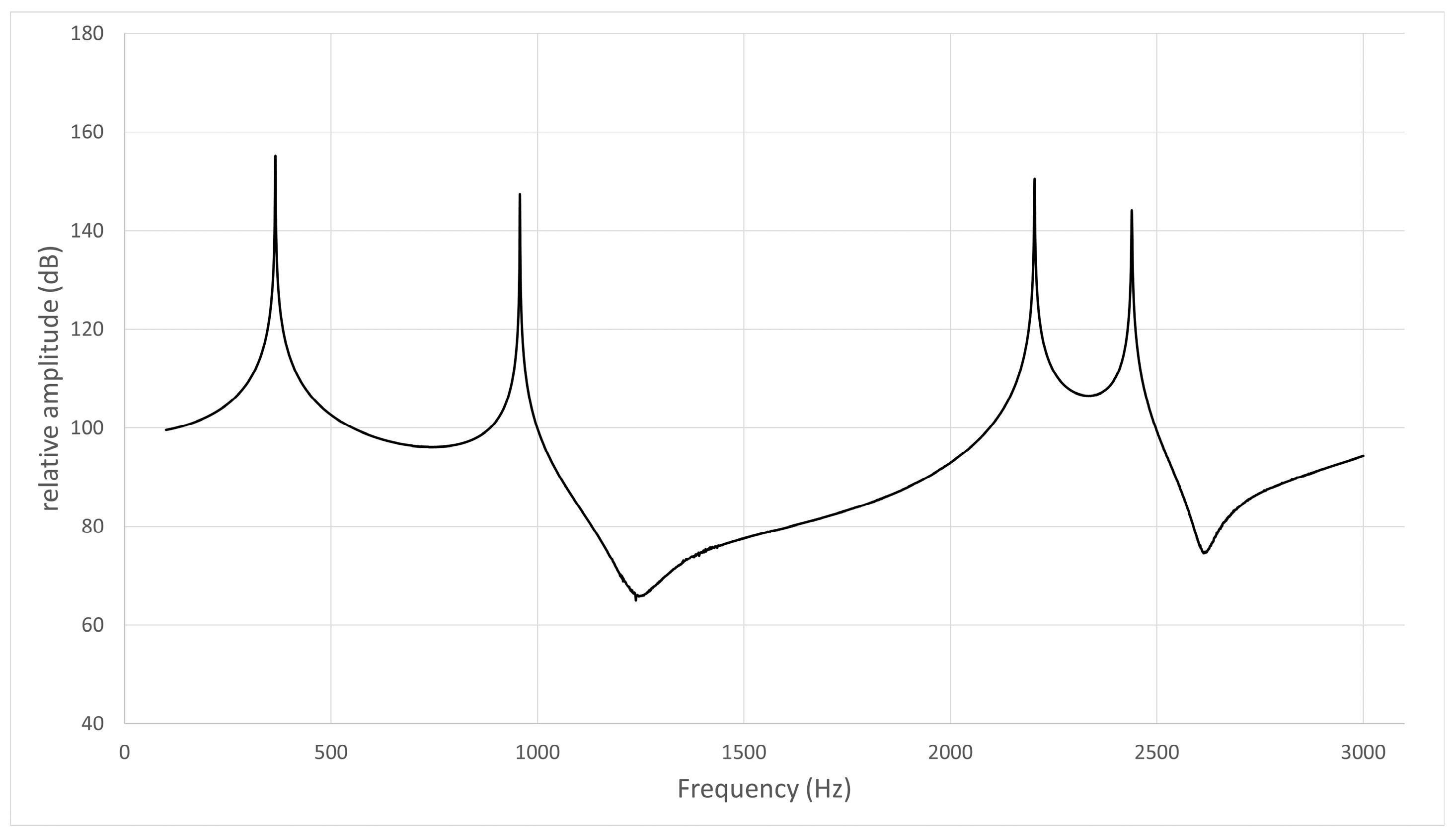
- The frequency response curve obtained from the simulation of the models without the nasal cavity and sinuses is shown. The first peak is F1 (365 Hz), the second is a pole–zero pair (pole: 944 Hz, zero: 1175 Hz) not due to nasal coupling, and the third is F2 (2204 Hz).
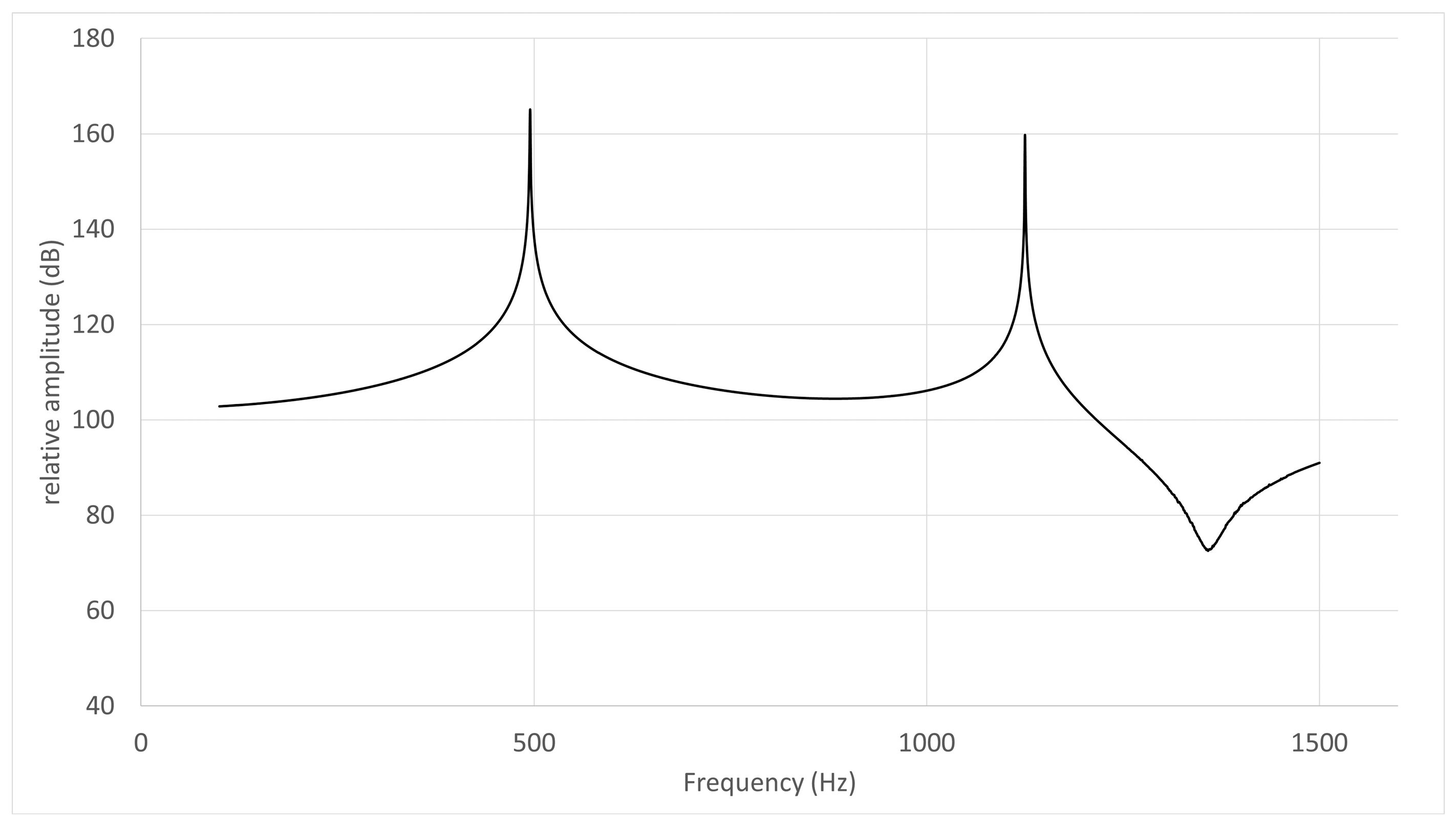
- The frequency response curve obtained from the simulation of the models without the nasal cavity and sinuses is shown. The first peak is F1 (495 Hz) and the second is F2 (1220 Hz).
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Bejdová, S.; Krajíček, V.; Peterka, M. Variability in palatal shape and size in patients with bilateral complete cleft lip and palate assessed using dense surface model construction and 3D geometric morphometrics. J. Craniomaxillofac. Surg. 2012, 40, 201–208. [Google Scholar] [CrossRef] [PubMed]
- Fleischer, M.; Rummel, S.; Stritt, F.; Fischer, J.; Bock, M.; Echternach, M.; Richter, B.; Traser, L. Voice efficiency for different voice qualities combining experimentally derived sound signals and numerical modeling of the vocal tract. Front. Physiol. 2022, 13, 1081622. [Google Scholar] [CrossRef] [PubMed]
- Dabbaghchian, S.; Arnela, M.; Engwall, O.; Guasch, O. Simulation of vowel-vowel utterances using a 3D biomechanical-acoustic model. Int. J. Numer. Method Biomed. Eng. 2021, 37, e3407. [Google Scholar] [CrossRef] [PubMed]
- Marc, A.; Rémi, B.; Saeed, D.; Oriol, G.; Francesc, A.; Xavier, P.; Annemie, V.H.; Olov, E. Influence of lips on the production of vowels based on finite element simulations and experiments. J. Acoust. Soc. Am. 2016, 139, 2852–2859. [Google Scholar]
- Takemoto, H.; Mokhtari, P.; Kitamura, T. Acoustic analysis of the vocal tract during vowel production by finite-difference time-domain method. J. Acoust. Soc. Am. 2010, 128, 3724–3738. [Google Scholar] [CrossRef] [PubMed]
- Tsuji, T.; Tsuchiya, T.; Kagawa, Y. Finite element and boundary element modelling for the acoustic wave transmission in mean flow medium. J. Sound Vib. 2002, 255, 849–866. [Google Scholar] [CrossRef]
- Amelia, J.G.; Helena, D.; Damian, T.M. Diphthong synthesis using the dynamic 3d digital waveguide mesh. IEEE/ACM Trans. Audio Speech Lang Process. 2018, 26, 243–255. [Google Scholar]
- Sakuma, T.; Yasuda, Y. Fast multipole boundary element method for large-scale steady-state sound field analysis. Part I: Setup and validation, Acust. Acta Acust. 2002, 88, 513–525. [Google Scholar]
- Shiraishi, M.; Mishima, K.; Umeda, H. Development of an Acoustic Simulation Method during Phonation of the Japanese Vowel/a/by the Boundary Element Method. J. Voice 2021, 35, 530–544. [Google Scholar] [CrossRef] [PubMed]
- Steffen, M. Six boundary elements per wavelength: Is that enough? J. Comput. Acoust. 2002, 10, 25–51. [Google Scholar]
- Zwikker, C.; Kosten, C.W. Sound Absorbent Material; Elsevier: New York, NY, USA, 1949; pp. 1–22. [Google Scholar]
- Lyzenga, J.; Horst, J.W. Frequency discrimination of bandlimited harmonic complexes related to vowel formants. J. Acoust. Soc. Am. 1995, 98, 1943–1955. [Google Scholar] [CrossRef]
- Eguchi, S. Difference limens for the formant frequencies: Normal adult values and their development in children. J. Am. Audiol. Soc. 1976, 1, 145–149. [Google Scholar]
- Hirahara, T.; Akahane-Yamada, R. Acoustic characteristics of Japanese vowels. In Proceedings of the 18th International Congress on Acoustics, Kyoto, Japan, 4–9 April 2004; pp. 3287–3290. [Google Scholar]
- Hosono, M. National Diagnostic Reference Levels in Japan (2020)—Japan DRLs 2020. Available online: http://www.radher.jp/J-RIME/report/DRL2020_Engver.pdf (accessed on 22 May 2023).
- Chen, M.Y. Acoustic correlates of English and French nasalized vowels. J. Acoust. Soc. Am. 1997, 102, 2360–2370. [Google Scholar] [CrossRef] [PubMed]
- Kenneth, N.S. Acoustic Phonetics; MIT Press: Cambridge, MA, USA, 2000; pp. 135–137. [Google Scholar]
- Havel, M.; Kornes, T.; Weitzberg, E.; Lundberg, J.O.; Sundberg, J. Eliminating paranasal sinus resonance and its effects on acoustic properties of the nasal tract. Logoped. Phoniatr. Vocol. 2016, 41, 33–40. [Google Scholar] [CrossRef] [PubMed]
| F1 | F2 | |||||||
|---|---|---|---|---|---|---|---|---|
| Sex | Subject No. | Number of Elements | Simulation Value (Hz) | Actual Voice (Hz) | Discrimination Threshold (%) | Simulation Value (Hz) | Actual Voice (Hz) | Discrimination Threshold (%) |
| M | 1 | 28,509 | 356 | 351 | 1.4 | 2196 | 2031 | 7.5 |
| 2 | 11,864 | 365 | 342 | 6.3 | 2204 | 2356 | 6.9 | |
| 3 | 11,492 | 317 | 336 | 6.0 | 2347 | 2180 | 7.1 | |
| F | 4 | 21,192 | 374 | 370 | 1.1 | 2510 | 2649 | 5.5 |
| 5 | 8361 | 368 | 358 | 2.7 | 2451 | 2579 | 5.2 | |
| 6 | 22,419 | 401 | 442 | 10.2 | 2775 | 2882 | 3.9 | |
| F1 | F2 | |||||||
|---|---|---|---|---|---|---|---|---|
| Sex | Subject No. | Number of Elements | Simulation Value (Hz) | Actual Voice (Hz) | Discrimination Threshold (%) | Simulation Value (Hz) | Actual Voice (Hz) | Discrimination Threshold (%) |
| M | 1 | 28,982 | 371 | 364 | 1.9 | 1127 | 1198 | 6.3 |
| 2 | 9905 | 445 | 436 | 2.0 | 1102 | 1222 | 11.0 | |
| 3 | 9728 | 381 | 375 | 1.6 | 1121 | 1261 | 12.5 | |
| F | 4 | 20,644 | 449 | 447 | 0.4 | 1403 | 1333 | 5.0 |
| 5 | 8653 | 495 | 464 | 6.3 | 1220 | 1292 | 6.0 | |
| 6 | 22,151 | 432 | 472 | 9.3 | 2072 | 1906 | 8.0 | |
| F1 | F2 | ||||||
|---|---|---|---|---|---|---|---|
| Sex | Subject No. | Length of the Virtual Trachea | Length of the Virtual Trachea | ||||
| 11 cm | 10 cm | 0 cm | 11 cm | 10 cm | 0 cm | ||
| M | 1 | 387 | 410 | 390 | 2279 | 2364 | 2432 |
| 2 | 369 | 379 | 501 | 2298 | 2342 | 2369 | |
| 3 | 317 | 324 | 393 | 2520 | 2663 | 2679 | |
| F | 4 | 416 | 420 | 451 | 2663 | 2840 | 3317 |
| 5 | 370 | 375 | 580 | 2608 | 2782 | 3070 | |
| 6 | 410 | 429 | 633 | 2860 | 2874 | 3350 | |
| F1 | F2 | ||||||
|---|---|---|---|---|---|---|---|
| Sex | Subject No. | Length of the Virtual Trachea | Length of the Virtual Trachea | ||||
| 11 cm | 10 cm | 0 cm | 11 cm | 10 cm | 0 cm | ||
| M | 1 | 380 | 391 | 404 | 1195 | 1230 | 1749 |
| 2 | 483 | 521 | 597 | 1110 | 1193 | 1960 | |
| 3 | 399 | 410 | 519 | 1253 | 1369 | 2451 | |
| F | 4 | 439 | 449 | 1061 | 1339 | 1399 | 2041 |
| 5 | 508 | 523 | 725 | 1265 | 1295 | 1850 | |
| 6 | 438 | 446 | 753 | 2106 | 2120 | 2160 | |
| Sex | Subject No. | /i/ | /u/ |
|---|---|---|---|
| M | 1 | 2.82 | 7.91 |
| F | 4 | 0.45 | 12.01 |
| 6 | 1.44 | 4.12 |
| /i/ | /u/ | |||
|---|---|---|---|---|
| Sex | Subject No. | Pole–Zero 1 (Hz) | Pole–Zero 2 (Hz) | Pole–Zero (Hz) |
| M | 1 | 411; 534 | 1037; 1209 | 462; 492 |
| 2 | - | 944; 1175 | - | |
| 3 | - | 936; 1011 | - | |
| F | 4 | 406; 486 | 1206; 1240 | 613; 654 |
| 5 | - | 1100; 1251 | - | |
| 6 | 449; 518 | 1143; 1213 | 531; 587 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shiraishi, M.; Mishima, K.; Takekawa, M.; Mori, M.; Umeda, H. An Acoustic Simulation Method of the Japanese Vowels /i/ and /u/ by Using the Boundary Element Method. Acoustics 2023, 5, 553-562. https://doi.org/10.3390/acoustics5020033
Shiraishi M, Mishima K, Takekawa M, Mori M, Umeda H. An Acoustic Simulation Method of the Japanese Vowels /i/ and /u/ by Using the Boundary Element Method. Acoustics. 2023; 5(2):553-562. https://doi.org/10.3390/acoustics5020033
Chicago/Turabian StyleShiraishi, Mami, Katsuaki Mishima, Masahiro Takekawa, Masaaki Mori, and Hirotsugu Umeda. 2023. "An Acoustic Simulation Method of the Japanese Vowels /i/ and /u/ by Using the Boundary Element Method" Acoustics 5, no. 2: 553-562. https://doi.org/10.3390/acoustics5020033
APA StyleShiraishi, M., Mishima, K., Takekawa, M., Mori, M., & Umeda, H. (2023). An Acoustic Simulation Method of the Japanese Vowels /i/ and /u/ by Using the Boundary Element Method. Acoustics, 5(2), 553-562. https://doi.org/10.3390/acoustics5020033






