Reviving the Low-Frequency Response of a Rupestrian Church by Means of FDTD Simulation
Abstract
1. Introduction
2. Materials and Methods
2.1. The Church Surveyed
2.2. Acoustic Measurement Methods
2.3. Geometrical Modelling of the Space
2.4. FDTD Simulation
2.5. Material Characterization
3. Results
3.1. On-Site Acoustic Measurements
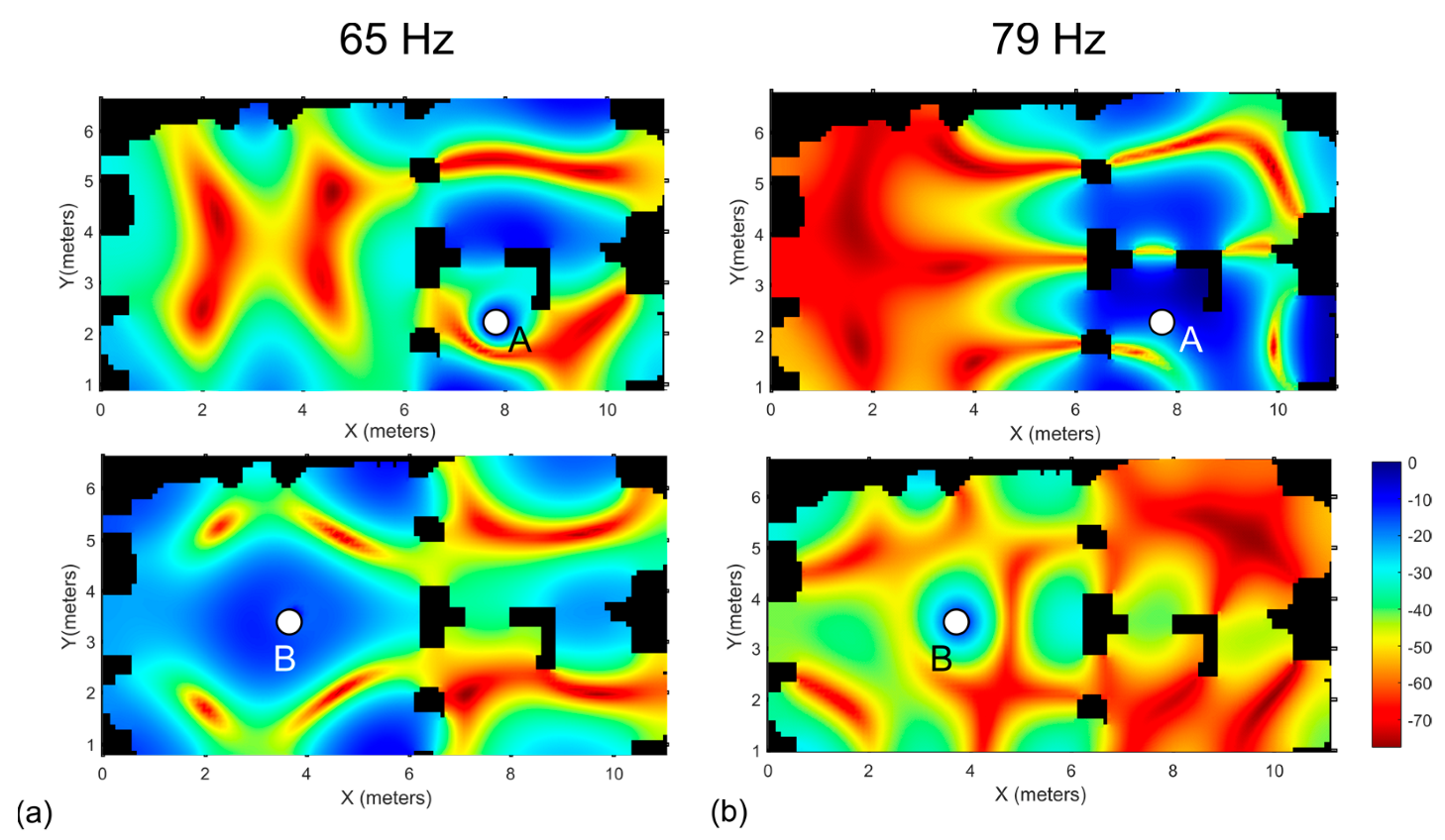
3.2. FDTD Acoustical Simulation of Current State
3.3. FDTD Acoustical Reconstruction of Original State
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- UNESCO World Heritage Centre. Available online: http://whc.unesco.org/ (accessed on 28 March 2023).
- Bixio, R.; De Pascale, A.; Mainetti, M. Census of Rocky Sites in the Mediterranean Area. In The Rupestrian Settlements in the Circum-Mediterranean Area; Crescenzi, C., Caprara, R., Eds.; Publisher DAdsp–UniFi: Florence, Italy, 2012. [Google Scholar]
- Crescenzi, C. Typology of Rupestrian Churches in Cappadocia. In The Rupestrian Settlements in the Circum-Mediterranean Area; Crescenzi, C., Caprara, R., Eds.; Publisher DAdsp–UniFi: Florence, Italy, 2012. [Google Scholar]
- Plummer, W.T. Infrasonic Resonances in Natural Underground Cavities. J. Acoust. Soc. Am. 1969, 46, 1074–1080. [Google Scholar] [CrossRef]
- Dayton, L. Rock art evokes beastly echoes of the past. New Sci. 1992, 1849, 14. [Google Scholar]
- Waller, S.J. Sound and rock art. Nature 1993, 363, 501. [Google Scholar] [CrossRef]
- Jahn, R.; Devereux, P.; Ibison, M. Acoustical resonances of assorted ancient structures. J. Acoust. Soc. Am. 1996, 99, 649–658. [Google Scholar] [CrossRef]
- Abel, J.S.; Rick, J.W.; Huang, P.P.; Kolar, M.A.; Smith, J.O.; Chawning, J.M. On the acoustics of the underground galleries of Ancient Chavin de Huantar. In Proceedings of the Acoustics 08, Paris, France, 29 June 29–4 July 2008; pp. 4165–4170. [Google Scholar]
- Reznikoff, I. Sound resonance in prehistoric times: A study of Paleolithic painted caves and rocks. In Proceedings of the Acoustics 08, Paris, France, 29 June–4 July 2008; pp. 4135–4139. [Google Scholar]
- Fazenda, B.; Scarre, C.; Till, R.; Pasalodos, R.J.; Guerra, M.R.; Tejedor, C.; Peredo, R.O.; Watson, A.; Wyatt, S.; Benito, C.G.; et al. Cave acoustics in prehistory: Exploring the association of Palaeolithic visual motifs and acoustic response. J. Acoust. Soc. Am. 2017, 142, 1332. [Google Scholar] [CrossRef] [PubMed]
- Wolfe, K.; Swanson, D.; Till, R. The frequency spectrum and geometry of the Ħal Saflieni Hypogeum appear tuned. J. Arch. Sci. Rep. 2020, 34, 102623. [Google Scholar] [CrossRef]
- Santos da Rosa, N.; Alvarez Morales, L.; Martorell Briz, X.; Fernández Macías, L.; Díaz-Andreu García, M. The acoustics of aggregation sites: Listening to the rock art landscape of Cuevas de la Araña (Spain). J. Field Archaeol. 2023, 48, 130–143. [Google Scholar] [CrossRef]
- Mattioli, T.; Farina, A.; Armelloni, E.; Hameau, P.; Díaz-Andreu, M. Echoing landscapes: Echolocation and the placement of rock art in the Central Mediterranean. J. Archaeol. 2017, 83, 12–25. [Google Scholar] [CrossRef]
- Till, R. Sound Archaeology: A Study of the Acoustics of Three World Heritage Sites, Spanish Prehistoric Painted Caves, Stonehenge, and Paphos Theatre. Acoustics 2019, 1, 661–692. [Google Scholar] [CrossRef]
- Iannace, G.; Trematerra, A. The acoustics of the caves. Appl. Acoust. 2014, 86, 42–46. [Google Scholar] [CrossRef]
- Małecki, P.; Lipecki, T.; Czopek, D.; Piechowicz, J.; Wiciak, J. Acoustics of Icelandic lava caves. Appl. Acoust. 2022, 197, 108929. [Google Scholar] [CrossRef]
- Iannace, G.; Trematerra, A.; Qandil, A. The acoustics of the catacombs. Arch. Acoust. 2014, 39, 583–590. [Google Scholar] [CrossRef]
- Adeeb, A.H.; Sü, Z.; Henry, A. Characterizing the Indoor Acoustical Climate of the Religious and Secular Rock-Cut Structures of Cappadocia. Int. J. Archit. Herit. 2021, 1–22. [Google Scholar] [CrossRef]
- Martellotta, F.; Cirillo, E. Acoustic characterization of Apulian Rupestrian churches. In Proceedings of the Forum Acusticum 2011, Aalborg, Denmark, 27 June–1 July 2011. Paper 00052. [Google Scholar]
- Kuttruff, H. Room Acoustics, 5th ed.; Spon Press: London, UK, 2009. [Google Scholar]
- Fratoni, G.; Hamilton, B.; D’Orazio, D. Feasibility of a finite-difference time-domain model in large-scale acoustic simulations. J. Acoust. Soc. Am. 2022, 152, 330–341. [Google Scholar] [CrossRef] [PubMed]
- D’Orazio, D.; Fratoni, G.; Rovigatti, A.; Hamilton, B. Numerical simulations of Italian opera houses using geometrical and wave-based acoustics methods. In Proceedings of the 23rd International Congress on Acoustics, Aachen, Germany, 9–13 September 2019; pp. 5994–5996. [Google Scholar]
- Dell’Aquila, F.; Messina, A. Le chiese rupestri di Puglia e Basilicata; Adda Editore: Bari, Italy, 1998. [Google Scholar]
- Müller, S.; Massarani, P. Transfer-function measurement with sweeps. J. Audio Eng. Soc. 2001, 49, 443–471. Available online: http://www.aes.org/e-lib/browse.cfm?elib=10189 (accessed on 28 March 2023).
- ISO 3382-2009; Acoustics–Measurement of Room Acoustic Parameters--Part 1: Performance Spaces. ISO: Geneva, Switzerland, 2009.
- Martellotta, F.; Cirillo, E.; Carbonari, A.; Ricciardi, P. Guidelines for acoustical measurements in churches. Appl. Acoust. 2008, 70, 378–388. [Google Scholar] [CrossRef]
- Botteldooren, D. Finite-difference time-domain simulation of low-frequency room acoustic problems. J. Acoust. Soc. Am. 1995, 98, 3302–3308. [Google Scholar] [CrossRef]
- Southern, A.; Siltanen, S.; Murphy, D.T.; Savioja, L. Room Impulse Response Synthesis and Validation Using a Hybrid Acoustic Model. IEEE Trans Audio Speech Lang Process 2013, 21, 1940–1952. [Google Scholar] [CrossRef]
- Kowalczyk, K. Boundary and Medium Modelling Using Compact Finite Difference Schemes in Simulations of Room Acoustics for Audio and Architectural Design Applications. Ph.D. Dissertation, Queen’s University Belfast, Belfast, UK, 2008. [Google Scholar]
- Kowalczyk, K.; van Walstijn, M. Room acoustics simulation using 3-D compact explicit FDTD schemes. IEEE Trans. Audio Speech Lang. Process. 2011, 19, 34–46. [Google Scholar] [CrossRef]
- Hill, A.J.; Hawksford, M.O.J. Visualization and Analysis Tools for Low-Frequency Propagation in a Generalized 3D Acoustic Space. J. Audio Eng. Soc. 2011, 59, 321–337. Available online: http://www.aes.org/e-lib/browse.cfm?elib=15932 (accessed on 28 March 2023).
- Kowalczyk, K.; van Walstijn, M. Formulation of locally reacting surfaces in FDTD/K-DWM modelling of acoustic spaces. Acta Acust. United Acust. 2008, 94, 891–906. [Google Scholar] [CrossRef]
- ISO 10534-2; Acoustics–Determination of Sound Absorption Coefficient and Impedance in Impedance Tubes-Part 2: Transfer-function Method. ISO: Geneva, Switzerland, 1998.
- Rindel, J.H. Modal energy analysis of nearly rectangular rooms at low frequencies. Acta Acust. United Acust. 2015, 101, 1211–1221. [Google Scholar] [CrossRef]
- Prato, A.; Casassa, F.; Schiavi, A. Reverberation time measurements in non-diffuse acoustic field by the modal reverberation time. Appl. Acoust. 2016, 110, 160–169. [Google Scholar] [CrossRef]
- Martellotta, F.; D’Alba, M.; Della Crociata, S. Laboratory measurement of sound absorption of occupied pews and standing audiences. Appl. Acoust. 2011, 72, 341–349. [Google Scholar] [CrossRef]
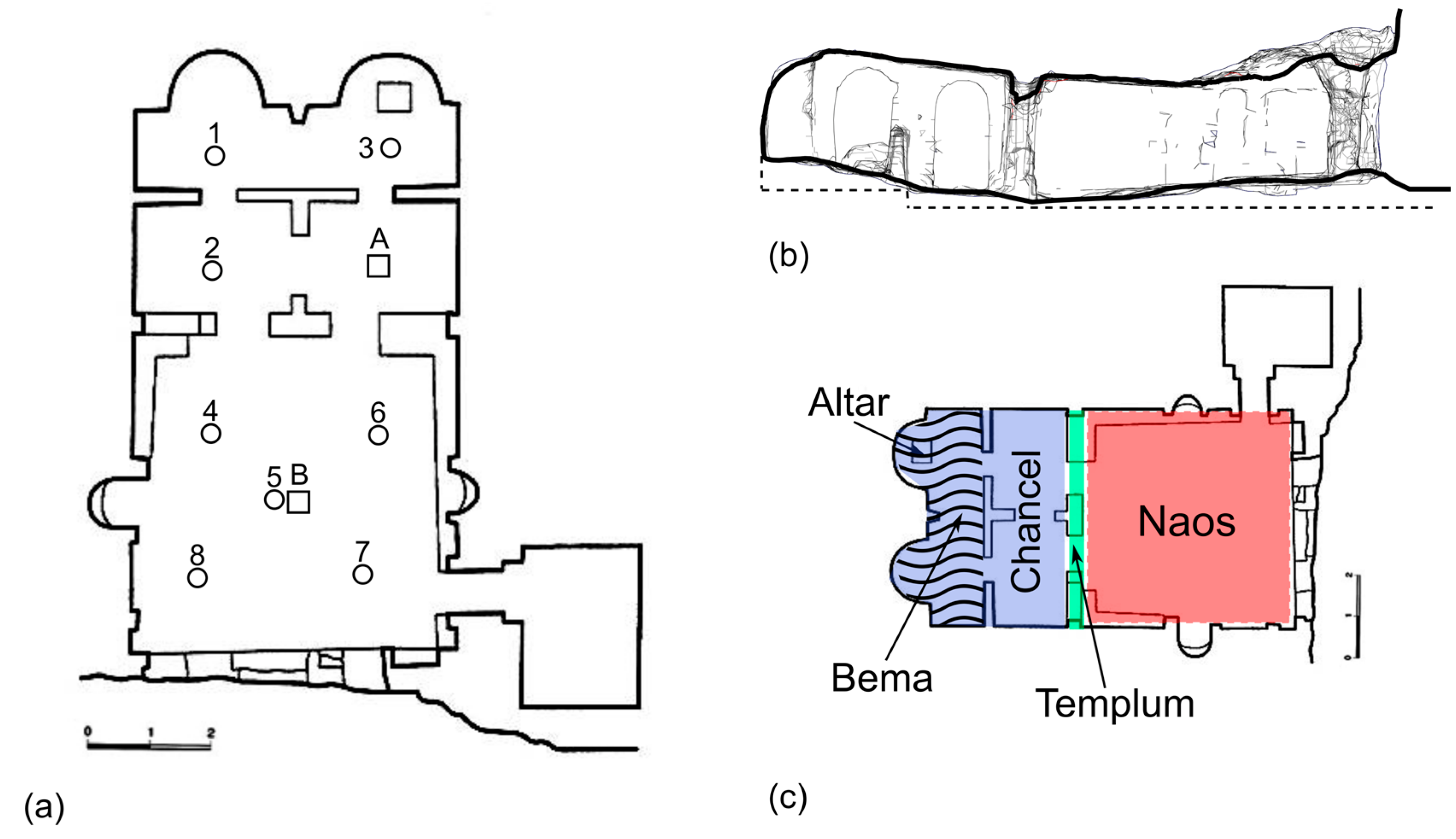











| Volume | 113 m3 |
| Plan surface | 57 m2 |
| Total area | 210 m2 |
| Average height | 2.15 m |
| Surface openings | 5 m2 |
| Floor | Dry ground with large cracks |
| Ceiling/vault | Extremely rough and peeling tuffaceous stone |
| Wall | Strongly degraded tuffaceous stone, with evident signs of erosion and, in some points, covered by frescoed plasters also with evident signs of superficial deterioration |
| Material | 63 Hz | 80 Hz | 100 Hz | 125 Hz | 160 Hz |
|---|---|---|---|---|---|
| Soft limestone (Carparo) | 0.010 | 0.020 | 0.030 | 0.050 | 0.080 |
| Hard limestone | 0.010 | 0.020 | 0.020 | 0.024 | 0.037 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Martellotta, F.; Liuzzi, S.; Rubino, C. Reviving the Low-Frequency Response of a Rupestrian Church by Means of FDTD Simulation. Acoustics 2023, 5, 396-413. https://doi.org/10.3390/acoustics5020023
Martellotta F, Liuzzi S, Rubino C. Reviving the Low-Frequency Response of a Rupestrian Church by Means of FDTD Simulation. Acoustics. 2023; 5(2):396-413. https://doi.org/10.3390/acoustics5020023
Chicago/Turabian StyleMartellotta, Francesco, Stefania Liuzzi, and Chiara Rubino. 2023. "Reviving the Low-Frequency Response of a Rupestrian Church by Means of FDTD Simulation" Acoustics 5, no. 2: 396-413. https://doi.org/10.3390/acoustics5020023
APA StyleMartellotta, F., Liuzzi, S., & Rubino, C. (2023). Reviving the Low-Frequency Response of a Rupestrian Church by Means of FDTD Simulation. Acoustics, 5(2), 396-413. https://doi.org/10.3390/acoustics5020023









