The Proscenium of Opera Houses as a Disappeared Intangible Heritage: A Virtual Reconstruction of the 1840s Original Design of the Alighieri Theatre in Ravenna
Abstract
1. Introduction
- When the orchestra plays in the pit, it is also possible to dim the lights in the cavea, increasing the focusing of audience on the scenes. During the 19th Century, before the Festspielhaus opening—some show companies, such as the Meiningen Company, dimmed the auditorium during the performance [21]. Wagner’s main goal in Festspielhaus was to prevent the audience reading the libretto during the drama, enhancing the visual and the listening experience of the audience: for this reason, the sunken pit was intended to cover the lights used by musicians [22].
- In the 19th Century, orchestras were changing, by adding more brass and, consequently, more strings and woodwinds were needed to balance the tone [23]. Consequently, the sound level of the orchestra was louder than the soloist and the pit was needed also to decrease the orchestral sound strength [24,25].
- Recitativo gradually disappeared during the 19th-Century opera, so soloist and conductor did have more need for proximity, which allows—from the point of view of the soloist—to follow the continuo accompaniment, often played by the conductor himself.
2. Methodology
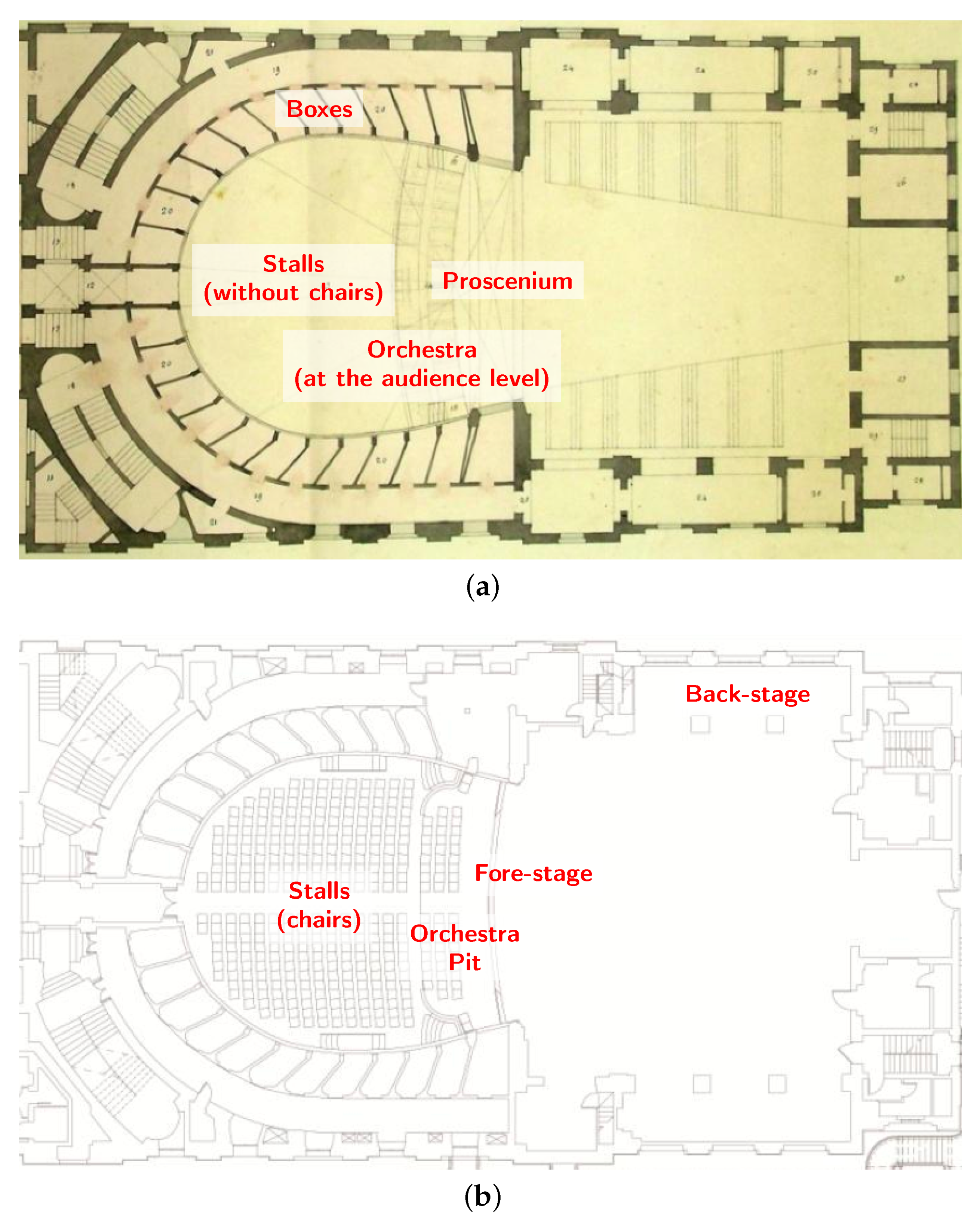
2.1. The Alighieri Theatre in Ravenna
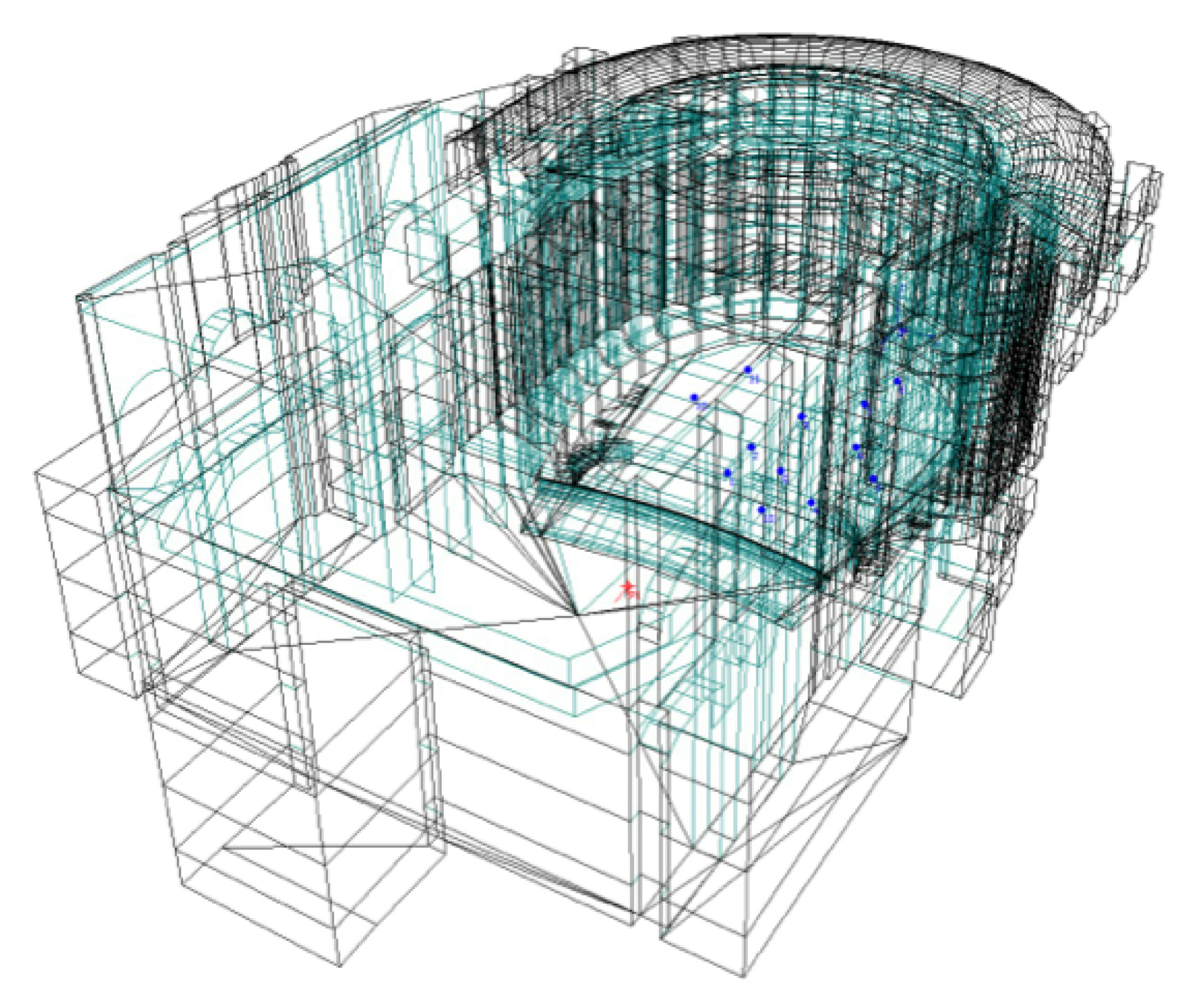
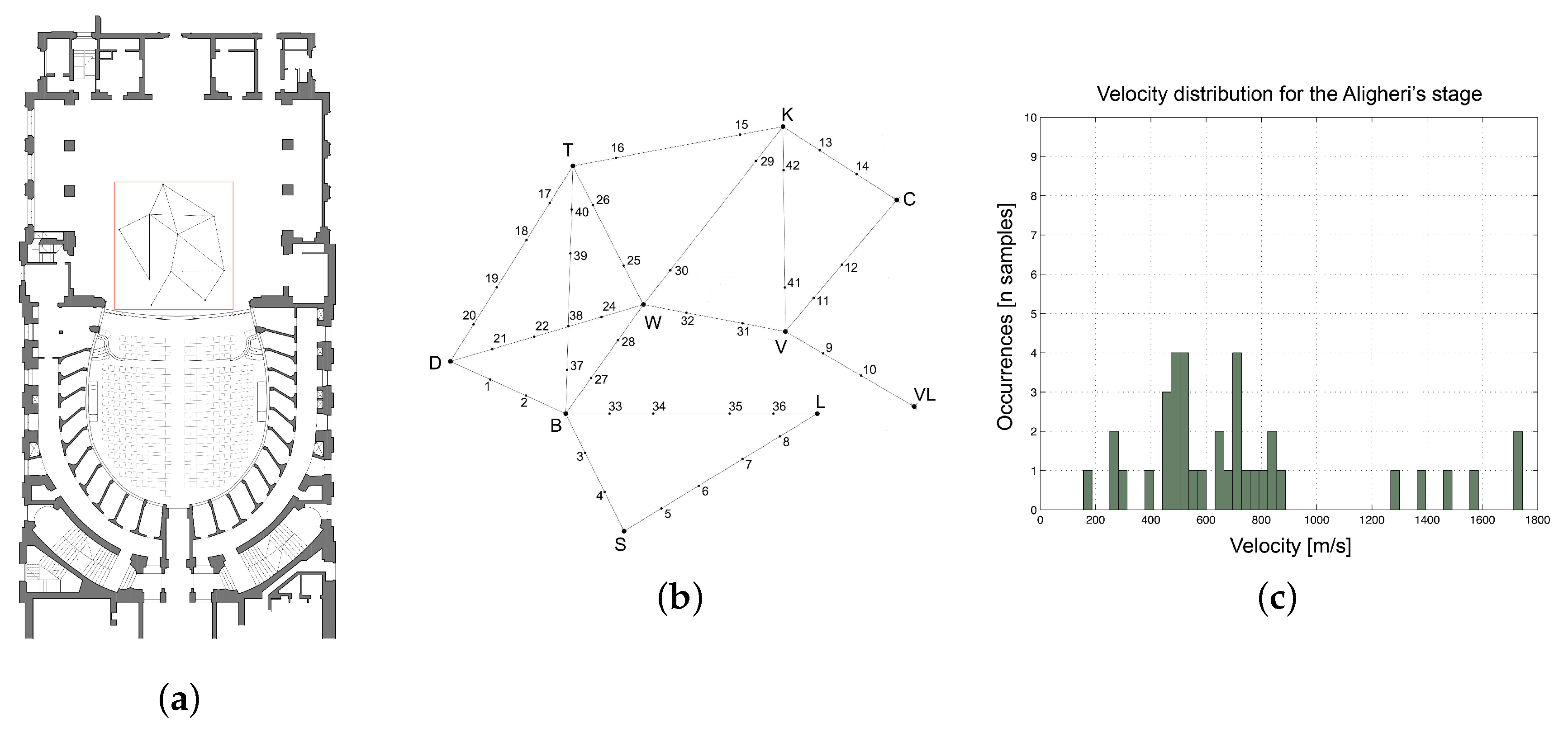
2.2. Measurements and Simulation Set-Up
2.3. Assumptions and Numerical Calibration
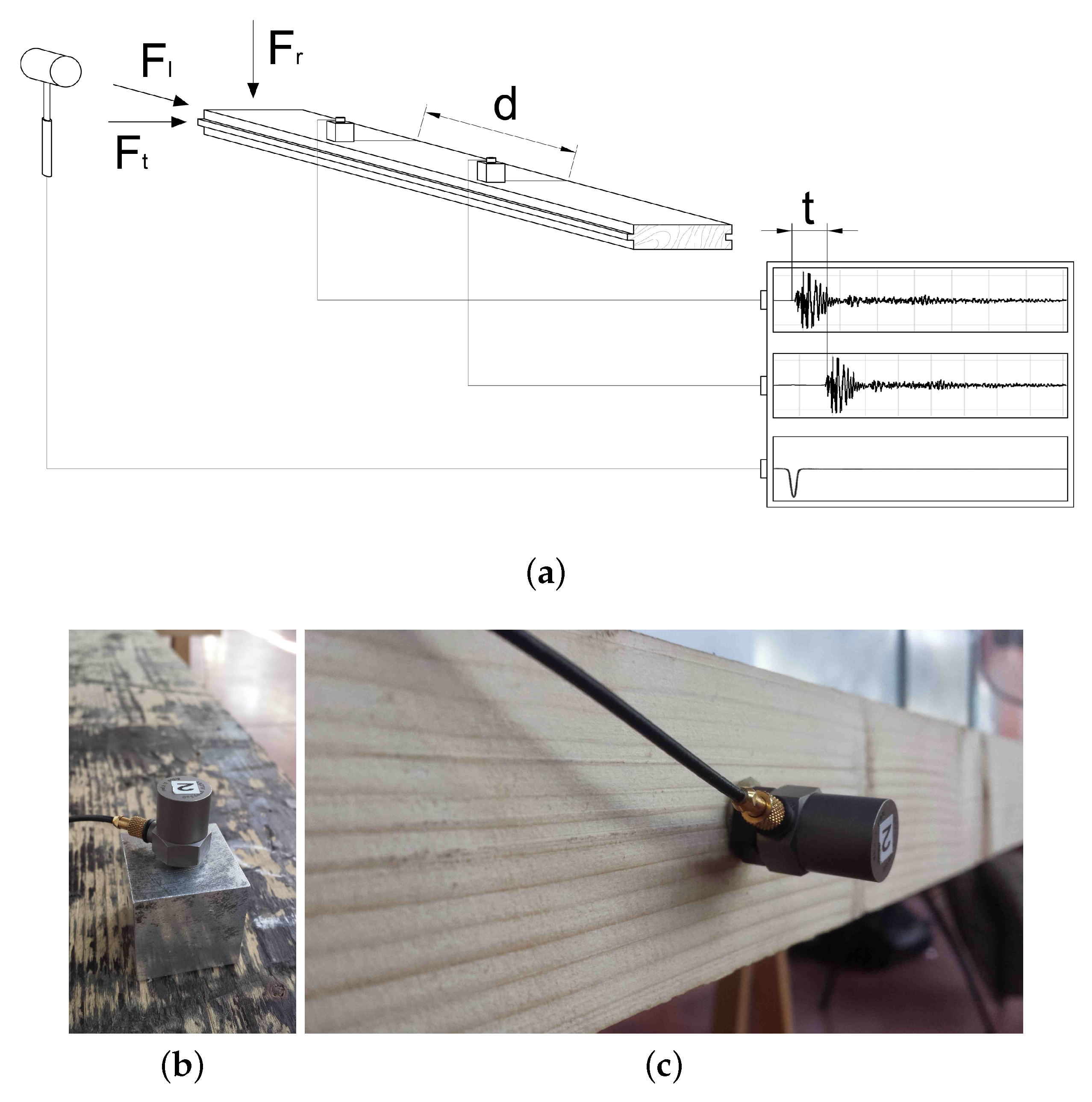
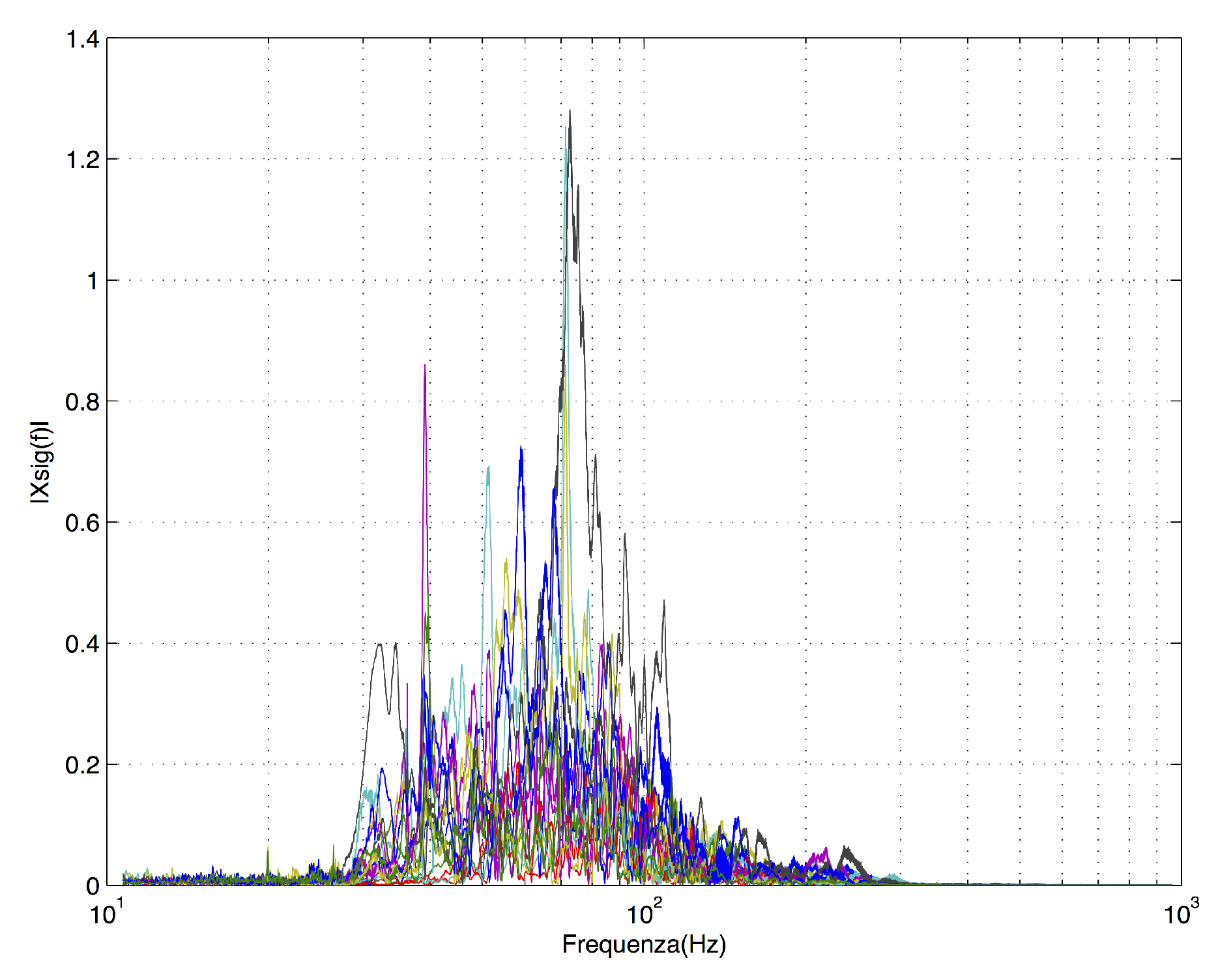
2.4. Vibroacoustic Measurements of the Historical Wooden Stage
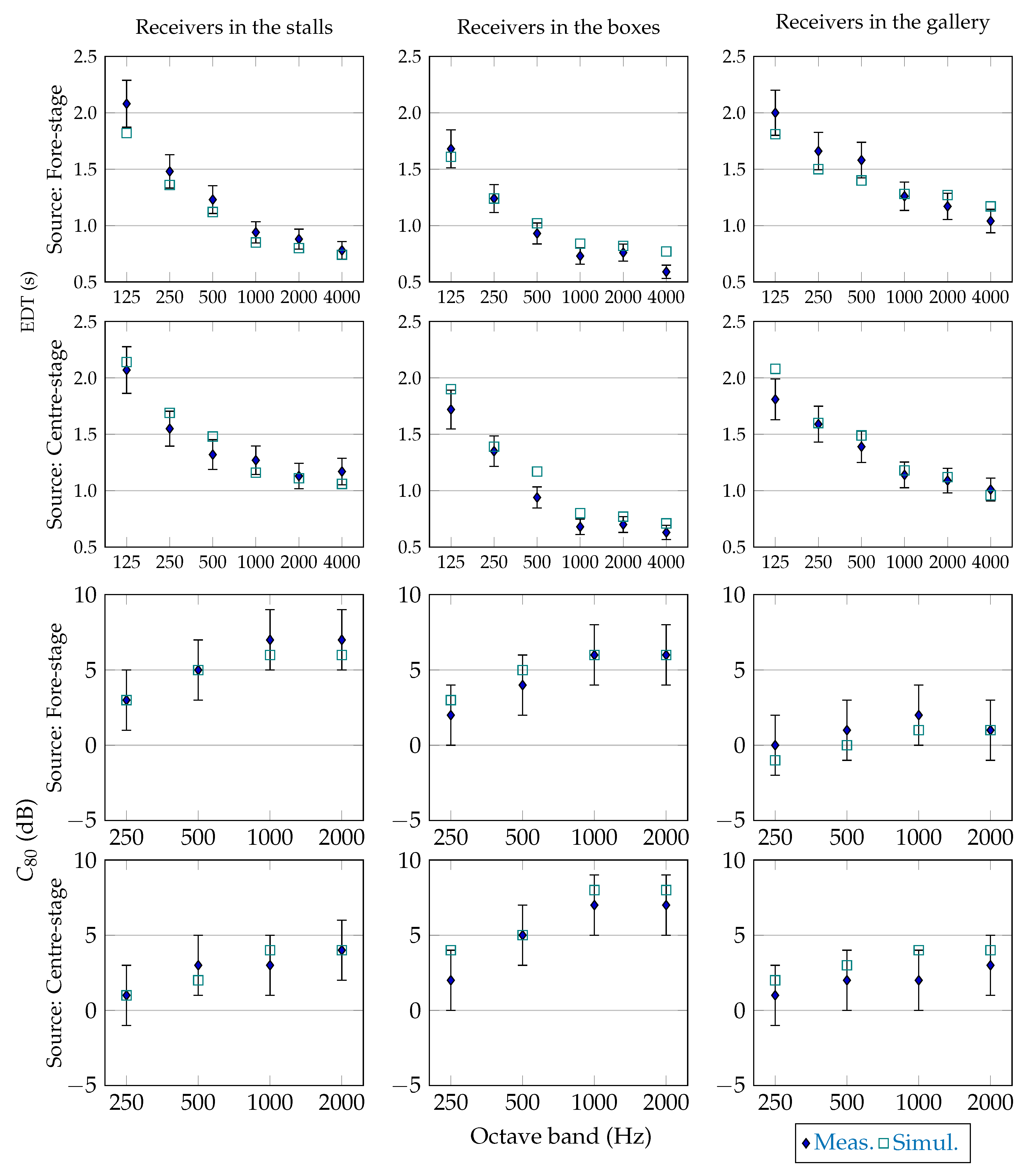
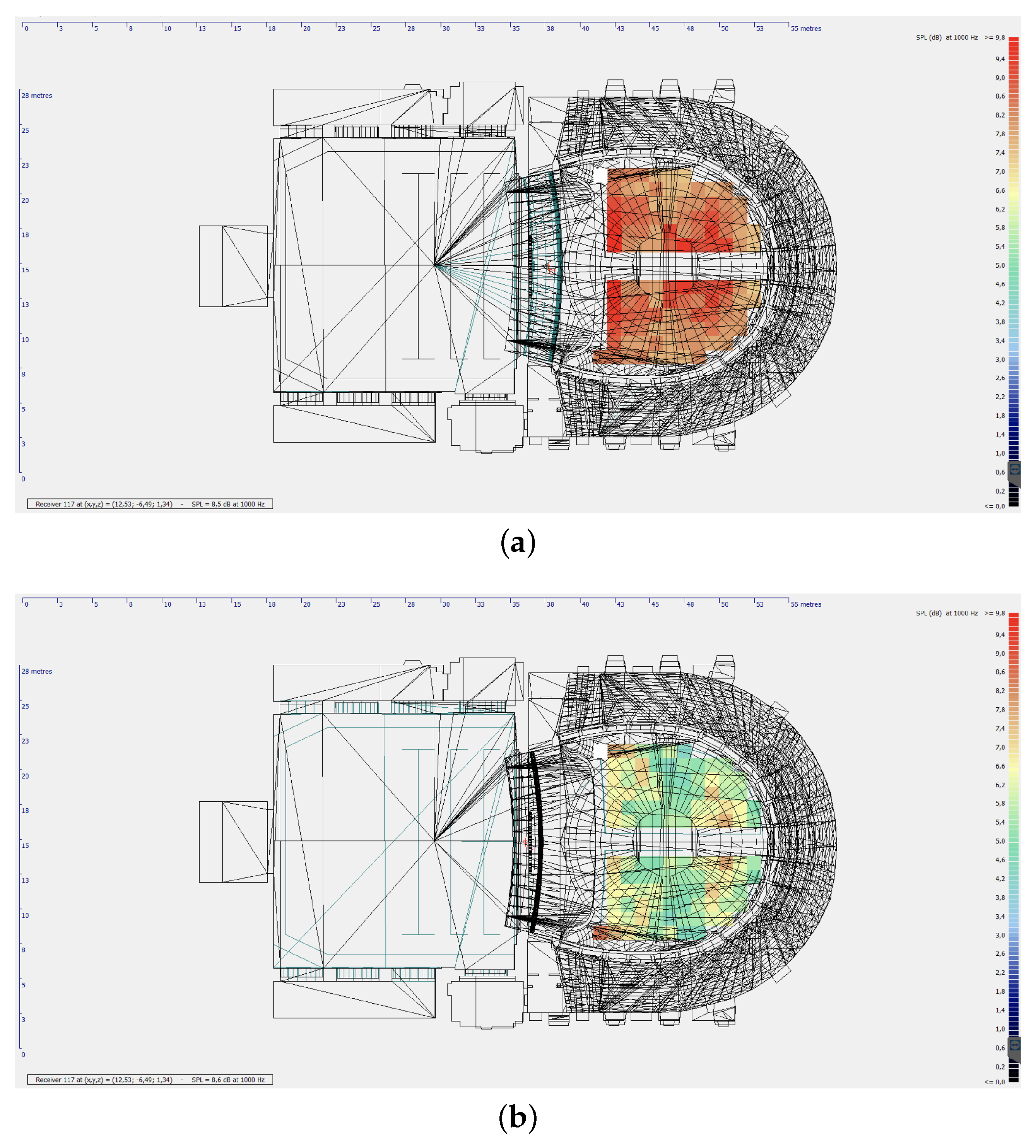
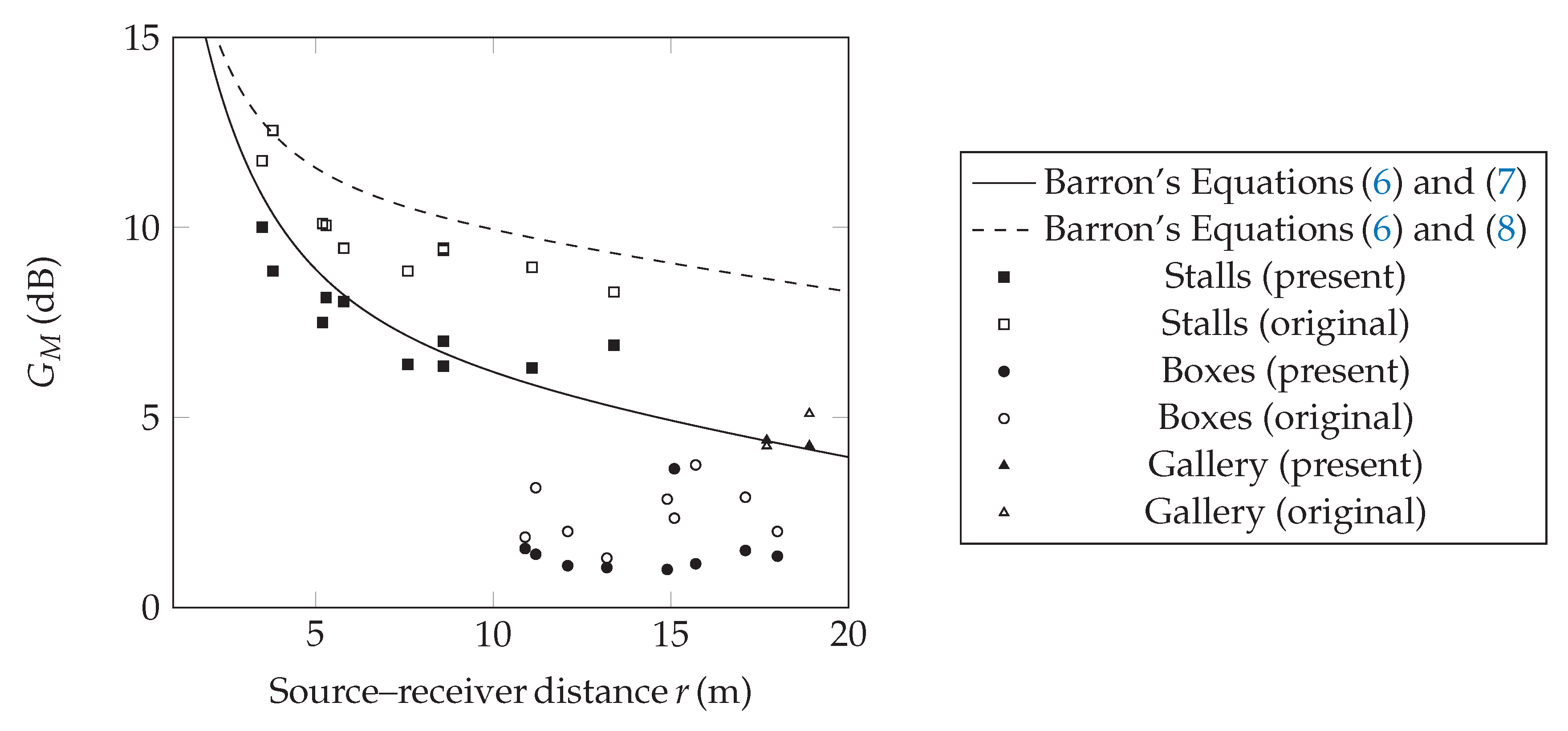
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References and Note
- Vecco, M. A definition of Cultural Heritage: From the tangible to the intangible. J. Cult. Herit. 2010, 11, 321–324. [Google Scholar] [CrossRef]
- Đórđévić, Z. Intangible tangibility: Acoustical heritage in architecture. Struct. Integr. Life 2016, 16, 59–66. [Google Scholar]
- Fausti, P.; Pompoli, R.; Prodi, N. Acoustics of opera houses: A cultural heritage. J. Acoust. Soc. Am. 1999, 105, 929. [Google Scholar] [CrossRef]
- Tronchin, L.; Farina, A. Acoustics of the Former Teatro—La Fenice—In Venice. J. Audio Eng. Soc. 1997, 45, 1051–1062. [Google Scholar]
- Iannace, G.; Ianniello, C.; Maffei, L.; Romano, R. Objective measurement of the listening condition in the old Italian opera house “Teatro di San Carlo”. J. Sound Vib. 2000, 232, 239–249. [Google Scholar] [CrossRef]
- Farina, A. Acoustic quality of theatres: Correlations between experimental measures and subjective evaluations. Appl. Acoust. 2001, 62, 899–916. [Google Scholar] [CrossRef]
- Cammarata, G.; Fichera, A.; Pagano, A.; Rizzo, G. Acoustical prediction in some Italian theatres. Acoust. Res. Lett. Online 2001, 2, 61–66. [Google Scholar] [CrossRef]
- Astolfi, A.; Bortolotto, A.; Filippi, A.; Masoero, M.; Pisani, R. Acoustical characterisation of small Italian opera house. In Proceedings of the Forum Acusticum, Budapest, Hungary, 29 August–2 September 2005; pp. 2301–2306. [Google Scholar]
- Pompoli, R. (Ed.) Proc. Teatri d’Opera dell’Unità d’Italia (Proceedings Opera houses of the Italian Unification); Acoustical Society of Italy (AIA): Venezia, Italy, 2011. (In Italian) [Google Scholar]
- Garai, M.; Morandi, F.; D’Orazio, D.; De Cesaris, S.; Loreti, L. Acoustic measurements in eleven Italian opera houses: Correlations between room criteria and considerations on the local evolution of a typology. Build. Environ. 2015, 94, 900–912. [Google Scholar] [CrossRef]
- Prodi, L.; Pompoli, R.; Martellotta, F.; Sato, S. Acoustics of Italian Historical Opera Houses. J. Acoust. Soc. Am. 2015, 138, 769–781. [Google Scholar] [CrossRef]
- Weinzierl, S.; Sanvito, P.; Schultz, F.; Büttner, C. The acoustics of renaissance theatres in Italy. Acta Acust. 2015, 101, 632–641. [Google Scholar] [CrossRef]
- Pompoli, R.; Prodi, N. Guidelines for acoustical measurements inside historical opera houses: Procedures and validation. J. Sound Vib. 2000, 232, 281–301. [Google Scholar] [CrossRef]
- ISO 3382-1: Acoustics—Measurement of Room Acoustic Parameters. Part 1: Performance Spaces; ISO: Geneva, Switzerland, 2009.
- Pisani, R.; Duretto, F. Il restauro ed i problemi di acustica dei teatri storici (The restoration and the acoustics problems in historical theatres). In Proceedings of the XXVII National Conference of the Italian Acoustics Association (AIA), Genova, Italy, 26–28 May 1999. [Google Scholar]
- Prodi, N.; Pompoli, R. Acoustics in the restoration of Italian historical opera houses: A review. J. Cult. Herit. 2016, 21, 915–921. [Google Scholar] [CrossRef]
- Prodi, N. From Tangible to Intangible Heritage inside Italian Historical Opera Houses. Heritage 2019, 2, 826–835. [Google Scholar] [CrossRef]
- D’Orazio, D.; Nannini, S. Towards Italian Opera Houses: A Review of Acoustic Design in Pre-Sabine Scholars. Acoustics 2019, 1, 252–280. [Google Scholar] [CrossRef]
- Wagner, R. The Opera House at Bayreuth. In Selected from His Writings and Translated; Wagner, R., Ed.; H. Holt and Company: New York, NY, USA, 1875; pp. 255–288. [Google Scholar]
- Ledoux, C.N. Architecture Considérée Sous le Rapport de l’Art des Moeurs et de la Législation; Porroneau: Paris, France, 1804. [Google Scholar]
- Carnegy, P. Wagner and the Art of the Theatre; Yale University Press: New Haven, CT, USA, 2006. [Google Scholar]
- Müller, K. Die Demokratisierung der Akustik (The democratization of acoustics). In Das Richard Wagner Festspielhaus Bayreuth; Kiesel, M., Ed.; Nettpress: Düsseldorf, Germany, 2007; pp. 174–197. [Google Scholar]
- Stefani, D. L’orchestra del Teatro alla Scala nella prima metá dell’800 Organizzazione, Funzioni, Gerarchie. Ph.D. Thesis, Politecnico di Milano, Milan, Italy, 2015. [Google Scholar]
- Lautenbach, M.R.; Vercammen, M.L.S.; Lorenz-Kierakiewitz, K.H. Acoustic Aspects of Stage and Orchestra Pit in Opera Houses. In Proceedings of the 38th German Acoustical Society (DAGA), Darmstadt, Germany, 19–20 March 2012. [Google Scholar]
- Vercammen, M.L.S.; Lautenbach, M.R. Stage and Pit Acoustics in Opera Houses. In Proceedings of the International Symposium on Music and Room Acoustics (ISMRA), La Plata, Argentine, 11–13 September 2016. [Google Scholar]
- Disposizione scenica per l’opera “Otello”, dramma lirico in quattro atti, versi di Arrigo Boito, musica di Giuseppe Verdi, compilata e regolata secondo la messa in scena del Teatro alla Scala Milano, Ricordi, 1887. (In Italian)
- Reggiani, L. Il Teatro Alighieri di Ravenna: Rilevazioni Acustiche e Modelli Numerici per la Conservazione, la Ricerca Musicologica e l’Auralizzazione di Futuri Allestimenti. Master’s Thesis, University of Bologna, Bologna, Italy, 2011. [Google Scholar]
- Rinaldi, M. Vibro-Acoustic Measurements of Wooden Stages in Historical Italian Theatres. Master’s Thesis, University of Bologna, Bologna, Italy, 2015. [Google Scholar]
- Vorländer, M. Computer simulations in room acoustics: Concepts and uncertainties. J. Acoust. Soc. Am. 2013, 133, 1203–1213. [Google Scholar] [CrossRef] [PubMed]
- Vissilantonopoulos, S.; Mourjopoulos, J. Virtual acoustic reconstruction of ritual and public spaces of ancient Greece. Acta Acust. United Acust. 2001, 87, 604–609. [Google Scholar]
- Katz, B.F.G.; Wetherill, E. Fogg art museum lecture room: A calibrated recreation of the birthplace of room acoustics. In Proceedings of the Forum Acusticum, Budapest, Hungary, 29 August–2 September 2005. [Google Scholar]
- Weinzierl, S.; Rosenheinrich, H.; Blickensdorff, J.; Horn, M.; Lindau, A. The acoustics of the concert hall in Leipzig’s Gewandhaus. History, reconstruction and auralization. In Proceedings of the Fortschritte des Akustik, Berlin, Germany, 15–18 September 2010. [Google Scholar]
- Takala, J.; Kylliïnen, M. Comparison of modelled performance of a vanished building with historical information on its acoustics. In Proceedings of the Forum Acusticum, Krakow, Poland, 7–12 September 2014. [Google Scholar]
- Sü Gül, Z.; Xiang, N.; Çaliskan, M. Investigations on sound energy decays and flows in a monumental mosque. J. Acoust. Soc. Am. 2016, 140, 344–355. [Google Scholar] [CrossRef]
- Sender, M.; Planells, R.; Perello, R.; Segura, J.; Gimenez, A. Virtual acoustic reconstruction of a lost church: Application to an Order of Saint Jerome monastery in Alzira, Spain. J. Build. Perf. Simul. 2017, 11, 369–390. [Google Scholar] [CrossRef]
- Suárez, R.; Alonso, A.; Sendra, J.J.A. Virtual acoustic environment reconstruction of the hypostyle mosque of Cordoba. Appl. Acoust. 2018, 140, 214–224. [Google Scholar] [CrossRef]
- D’Orazio, D.; De Cesaris, S.; Morandi, F.; Garai, M. The aesthetics of the Bayreuth Festspielhaus explained by means of acoustic measurements and simulations. J. Cult. Herit. 2018, 34, 151–158. [Google Scholar] [CrossRef]
- Bo, E.; Shtrepi, L.; Pelegrin Garcia, D.; Barbato, G.; Aletta, F.; Astolfi, A. The accuracy of predicted acoustical parameters in ancient open-air theaters: A case study in Syrscusae. Appl. Sci. 2018, 8, 1393. [Google Scholar] [CrossRef]
- Postma, B.N.J.; Dubouilh, S.; Katz, B.F.G. An archeoacoustic study of the history of the Palais du Trocadero. J. Acoust. Soc. Am. 2019, 145, 2810–2821. [Google Scholar] [CrossRef] [PubMed]
- Đórđévić, Z.; Novković, D.; Andrić, U. Archaeoacoustic Examination of Lazarica Church. Acoustics 2019, 1, 423–438. [Google Scholar] [CrossRef]
- Meduna, T.; Meduna, G. Il Teatro La Fenice in Venezia… (On the La Fenice Theatre…); Antonelli: Venezia, Italy, 1849. (In Italian) [Google Scholar]
- Cocchi, A.; Garai, M.; Tronchin, L. Influenza di cavità risonanti poste sotto la fossa orchestrale: Il caso del teatro Alighieri di Ravenna (The Influence of Resonating Cavities under the Orchestra Pit: The Case of the Alighieri Theatre in Ravenna); Nardini Editore: Firenze, Italy, 1997; pp. 135–153. (In Italian) [Google Scholar]
- Sarasini, F. Il teatro Alighieri di Ravenna. Storia e restauri dalla fondazione ad oggi. Ravenna Studi e Ricerche 2005, 12, 165–199. (In Italian) [Google Scholar]
- D’Orazio, D.; De Cesaris, S.; Guidorzi, P.; Barbaresi, L.; Garai, M.; Magalotti, R. Room acoustic measurements using a high SPL. In Proceedings of the 140th Audio Engineering Society Convention (AES), Paris, France, 4–7 June 2016. [Google Scholar]
- Guidorzi, P.; Barbaresi, L.; D’Orazio, D.; Garai, M. Impulse responses measured with MLS or Swept–Sine signals applied to architectural acoustics: An in-depth analysis of the two methods and some case studies of measurements inside theaters. Energy Procedia 2015, 78, 1611–1616. [Google Scholar] [CrossRef]
- Christensen, C.L.; Koutsouris, G.; Gil, G. ODEON Room Acoustics Software, Version 12, User Manual; ODEON A/S: Lyngby, Denmark, 2013. [Google Scholar]
- Postma, B.N.J.; Katz, B.F.G. Perceptive and objective evaluation of calibrated room acoustic simulation auralizations. J. Acoust. Soc. Am. 2016, 140, 4326–4337. [Google Scholar] [CrossRef] [PubMed]
- Vorländer, M. Auralization: Fundamentals of Acoustics, Modelling, Simulation, Algorithms and Acoustic Virtual Reality; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Cox, T.J.; D’Antonio, P. Acoustic Absorbers and Diffusers: Theory, Design and Application, 3rd ed.; CRC Press: Boca Raton, FL, USA, 2004. [Google Scholar]
- Koutsouris, G.; Norgaard, A.K.; Christensen, C.L. Discretisation of curved surfaces and choice of simulation parameters in acoustic modeling of religious spaces. In Proceedings of the 23rd International Congress on Sound and Vibration (ICSV), Athens, Greece, 10–14 July 2016. [Google Scholar]
- Nakanishi, S.; Sakagami, K.; Daido, M.; Morimoto, M. Acoustic properties of a cavity backed stage floor: A theoretical model. Appl. Acoust. 1999, 57, 17–27. [Google Scholar] [CrossRef]
- Augusztinovicz, F. Vibroacoustic analysis of the stage floor of a concert hall—A case study. Appl. Acoust. 2012, 73, 648–658. [Google Scholar] [CrossRef]
- Guettler, K.; Askenfelt, A.; Buen, A. Double basses on the stage floor: Tuning fork-tabletop effect, or not? J. Acoust. Soc. Am. 2012, 1, 795–806. [Google Scholar] [CrossRef] [PubMed]
- Beranek, L.; Johnson, F.R.; Shultz, T.J.; Walters, B.G. Acoustics of Philharmonic Hall, New York, during its first season. J. Acoust. Soc. Am. 1964, 36, 1247–1262. [Google Scholar] [CrossRef]
- Cremer, L.; Heckl, M.; Petersson, B.A.T. Structure-Borne Sound—Structural Vibrations and Sound Radiation at Audio Frequencies, 3rd ed.; Springer: Berlin/Heidelberg, Germany, 2005. [Google Scholar]
- De Cesaris, S.; Morandi, F.; Loreti, L.; D’Orazio, D.; Garai, M. Notes about the early to late transition in Italian theatres. In Proceedings of the 22nd International Congress on Sound and Vibration (ICSV), Florence, Italy, 12–16 June 2015. [Google Scholar]
- Barron, M.; Lee, L.J. Energy relations in concert auditoriums. J. Acoust. Soc. Am. 1988, 84, 618–628. [Google Scholar] [CrossRef]
- D’Orazio, D.; Fratoni, G.; Garai, M. Acoustics of a chamber hall inside a former church by means of sound energy distribution. Can. Acoust. 2017, 45, 7–16. [Google Scholar]
- Garai, M.; De Cesaris, S.; Morandi, F.; D’Orazio, D. Sound energy distribution energy distribution in Italian Historical Theatres. In Proceedings of the 22nd International Congress on Acoustics (ICA), Buenos Aires, Argentine, 5–9 September 2016. [Google Scholar]
- D’Orazio, D.; De Cesaris, S.; Garai, M. Recordings of Italian opera orchestra and soloists in a silent room. Proc. Mtgs. Acoust. 2016, 28, 015014. [Google Scholar]
| aria | solo vocal piece with instrumental accompaniment |
| libretto | text written for and set to music in an opera |
| recitativo | style of delivery in which a singer is allowed to adopt the rhythms of ordinary speech |
| continuo | (or bass continuo) a part for keyboard consisting of a succession of bass notes with figures that indicate the required chords |
| Room under Study | Calibration Reference | Calibration Approach |
|---|---|---|
| Public/Ritual Spaces [30] | Architectural drawings | Collected historical records. |
| Fogg Art Museum [31] | impulse response measurement (1973) and reverberation time data (1912) | Calibrated model using reverberation time data and photo, reconstruction former room states by modifying model and final comparison of original state to Sabine’s data. |
| Concert Hall, Gewandhaus [32] | Historical reverberation time data (1933) | Material properties based on detailed construction documentation, seating material determined from photo inspection, adjusted to reverberation time data. |
| Acheron Necromancy, Olympia echo hall, Temple of Zeus [30] | Archival plans | Material properties based on common materials of the time. |
| Finnish concert halls [33] | Reviews of acoustical qualities (2014) | Reproduce acoustical characteristics by adjusting material and scattering properties. |
| Súleymaniye Mosque [34] | impulse response measurement | Calibrated model based on the field test results. |
| Santa Maria de la Murta [35] | Archival plans and images | Material properties determined from historical images and similar studies. |
| Mosque, Cordoba [36] | Previous studies and archival materials | Calibration based on the current model results. |
| Festspielhaus, Bayreuth [37] | Archival plans and sections | Material properties determined from historical images and similar studies. |
| Greek Theatre of Syracusae [38] | Impulse response measurement and previous studies | Material properties based on detailed construction documentation and photo inspection. |
| Palais du Trocadero [39] | Historical documentations and historical acoustic data (1911, 1906, 1907) | Historic photos of the space, documents concerning the construction and design and acoustic data. |
| Lazarica Church [40] | Impulse response measurement | Calibrated model using on-site measurement results. |
| Present work | Original plans and sections | Material properties based on acoustic and vibro-acoustic measurements of the current status of the theatre. |
| Configuration | Volume (m) | Number of Surfaces | Number of Late Rays |
|---|---|---|---|
| Original | 10,455 | 7680 | 10,584 |
| Present | 10,455 | 7676 | 10,564 |
| Materials | Surface % | Absorption | s | Ref. | |||||
|---|---|---|---|---|---|---|---|---|---|
| 125 Hz | 250 Hz | 500 Hz | 1000 Hz | 2000 Hz | 4000 Hz | ||||
| Marble | 1 | 0.02 | 0.02 | 0.03 | 0.05 | 0.05 | 0.05 | 0.05 | [48] |
| Plaster (Fly Tower) | 23 | 0.12 | 0.14 | 0.15 | 0.15 | 0.16 | 0.16 | 0.05 | adapted [49] |
| Plaster (Hall) | 16 | 0.08 | 0.10 | 0.10 | 0.10 | 0.10 | 0.07 | 0.05 | adapted [49] |
| Boxes | 33 | 0.20 | 0.22 | 0.30 | 0.33 | 0.33 | 0.33 | 0.50 | adapted [49] |
| Wood (Stage) | 10 | 0.18 | 0.12 | 0.10 | 0.09 | 0.08 | 0.07 | 0.05 | adapted [48] |
| Wood (Hall) | 3 | 0.04 | 0.04 | 0.07 | 0.06 | 0.06 | 0.07 | 0.05 | [48] |
| Stage grid | 4 | 0.15 | 0.15 | 0.25 | 0.40 | 0.50 | 0.50 | 0.20 | adapted [10] |
| Wings | 6 | 0.15 | 0.20 | 0.20 | 0.40 | 0.40 | 0.42 | 0.05 | adapted [48] |
| Velvet | 1 | 0.15 | 0.35 | 0.55 | 0.77 | 0.70 | 0.60 | 0.05 | adapted [49] |
| Wooden chairs | 1 | 0.12 | 0.11 | 0.10 | 0.09 | 0.08 | 0.07 | 0.70 | [48] |
| Seats | 2 | 0.40 | 0.55 | 0.70 | 0.80 | 0.80 | 0.70 | 0.70 | adapted [49] |
| Beams | z | R | |||||||
|---|---|---|---|---|---|---|---|---|---|
| kg/m | m/s | m/s | m/s | m/s | GPa | GPa | 10 kg/(m s) | m/(kg s) | |
| not-aged | 390 | 3000 | 920 | 900 | 260 | 3.4 | 0.34 | 1170 | 7.57 |
| two-year aged | 400 | 4570 | 870 | 1000 | 340 | 8.1 | 0.30 | 1828 | 11.2 |
| historical | 450 | 5300 | 540 | 800 | 230 | 12.8 | 0.13 | 2385 | 11.85 |
| (dB) | (dB) | STI | ||
|---|---|---|---|---|
| Present (without proscenium) | Stalls | 7.6 | 6.0 | 0.65 |
| Boxes | 1.5 | 2.9 | 0.63 | |
| Gallery | 4.4 | −1.4 | 0.56 | |
| Original (with proscenium) | Stalls | 10.0 | 1.2 | 0.61 |
| Boxes | 2.5 | 0.8 | 0.57 | |
| Gallery | 4.7 | −2.6 | 0.55 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
D’Orazio, D.; Rovigatti, A.; Garai, M. The Proscenium of Opera Houses as a Disappeared Intangible Heritage: A Virtual Reconstruction of the 1840s Original Design of the Alighieri Theatre in Ravenna. Acoustics 2019, 1, 694-710. https://doi.org/10.3390/acoustics1030041
D’Orazio D, Rovigatti A, Garai M. The Proscenium of Opera Houses as a Disappeared Intangible Heritage: A Virtual Reconstruction of the 1840s Original Design of the Alighieri Theatre in Ravenna. Acoustics. 2019; 1(3):694-710. https://doi.org/10.3390/acoustics1030041
Chicago/Turabian StyleD’Orazio, Dario, Anna Rovigatti, and Massimo Garai. 2019. "The Proscenium of Opera Houses as a Disappeared Intangible Heritage: A Virtual Reconstruction of the 1840s Original Design of the Alighieri Theatre in Ravenna" Acoustics 1, no. 3: 694-710. https://doi.org/10.3390/acoustics1030041
APA StyleD’Orazio, D., Rovigatti, A., & Garai, M. (2019). The Proscenium of Opera Houses as a Disappeared Intangible Heritage: A Virtual Reconstruction of the 1840s Original Design of the Alighieri Theatre in Ravenna. Acoustics, 1(3), 694-710. https://doi.org/10.3390/acoustics1030041







