Shape Coexistence in Odd-Z Isotopes from Fluorine to Potassium
Abstract
1. Introduction
2. Deformed Relativistic Hartree–Bogoliubov Theory in Continuum
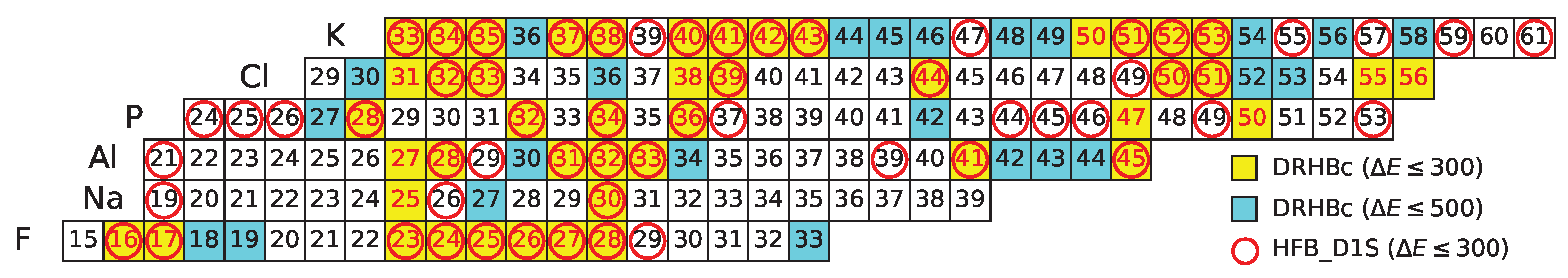
3. Results
4. Summary
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wood, J.L.; Heyde, K.; Nazarewicz, W.; Huyse, M.; Van Duppen, P. Coexistence in even-mass nuclei. Phys. Rep. 1992, 215, 101–201. [Google Scholar] [CrossRef]
- Gade, A.; Liddick, S. Shape coexistence in neutron-rich nuclei. J. Phys. G 2016, 43, 024001. [Google Scholar] [CrossRef]
- Garrett, P.E.; Zielińska, M.; Clément, E. An experimental view on shape coexistence in nuclei. Prog. Part. Nucl. Phys. 2022, 124, 103931. [Google Scholar] [CrossRef]
- Choi, Y.B.; Lee, C.H.; Mun, M.H.; Kim, Y. Bubble nuclei with shape coexistence in even-even isotopes of Hf to Hg. Phys. Rev. C 2022, 105, 024306. [Google Scholar] [CrossRef]
- Bonatsos, D.; Martinou, A.; Peroulis, S.K.; Mertzimekis, T.J.; Minkov, N. Shape Coexistence in Even–Even Nuclei: A Theoretical Overview. Atoms 2023, 11, 117. [Google Scholar] [CrossRef]
- Leoni, S.; Fornal, B.; Bracco, A.; Tsunoda, Y.; Otsuka, T. Multifaceted character of shape coexistence phenomena in atomic nuclei. Prog. Part. Nucl. Phys. 2024, 139, 104119. [Google Scholar] [CrossRef]
- Brown, B.A. Islands of insight in the nuclear chart. Physics 2010, 3, 104. [Google Scholar] [CrossRef]
- Scheit, H. Spectroscopy in and around the Island of inversion. J. Phys. Conf. Ser. 2011, 312, 092010. [Google Scholar] [CrossRef]
- Caurier, E.; Nowacki, F.; Poves, A. Merging of the islands of inversion at N = 20 and N = 28. Phys. Rev. C 2014, 90, 014302. [Google Scholar] [CrossRef]
- Miyagi, T.; Stroberg, S.R.; Holt, J.D.; Shimizu, N. Ab initio multishell valence-space Hamiltonians and the island of inversion. Phys. Rev. C 2020, 102, 034320. [Google Scholar] [CrossRef]
- Jeong, S.; Papakonstantinou, P.; Ishiyama, H.; Kim, Y. A brief overview of RAON physics. JKPS 2018, 73, 516. [Google Scholar] [CrossRef]
- In, E.J.; Kim, Y.; Papakonstantinou, P.; Hong, S.W. Shape coexistence in isotopes from oxygen to calcium. JKPS 2020, 77, 966. [Google Scholar] [CrossRef]
- Zhao, P.W.; Li, Z.P.; Yao, J.M.; Meng, J. New parametrization for the nuclear covariant energy density functional with a point-coupling interaction. Phys. Rev. C 2010, 82, 054319. [Google Scholar] [CrossRef]
- Zhou, S.G.; Meng, J.; Ring, P.; Zhao, E.G. Neutron halo in deformed nuclei. Phys. Rev. C 2010, 82, 011301. [Google Scholar] [CrossRef]
- Li, L.; Meng, J.; Ring, P.; Zhao, E.G.; Zhou, S.G. Deformed relativistic Hartree-Bogoliubov theory in continuum. Phys. Rev. C 2012, 85, 024312. [Google Scholar] [CrossRef]
- Meng, J.; Zhou, S.G. Halos in medium-heavy and heavy nuclei with covariant density functional theory in continuum. J. Phys. G 2015, 42, 093101. [Google Scholar] [CrossRef]
- Zhang, K.; Cheoun, M.K.; Choi, Y.B.; Chong, P.S.; Dong, J.; Geng, L.; Ha, E.; He, X.; Heo, C.; Ho, M.C.; et al. Deformed relativistic Hartree-Bogoliubov theory in continuum with a point-coupling functional: Examples of even-even Nd isotopes. Phys. Rev. C 2020, 102, 024314. [Google Scholar] [CrossRef]
- Zhang, K.; Cheoun, M.K.; Choi, Y.B.; Chong, P.S.; Dong, J.; Dong, Z.; Du, X.; Geng, L.; Ha, E.; He, X.T.; et al. Nuclear mass table in deformed relativistic Hartree–Bogoliubov theory in continuum, I: Even–even nuclei. At. Data Nucl. Data Tables 2022, 144, 101488. [Google Scholar] [CrossRef]
- Pan, C.; Cheoun, M.K.; Choi, Y.B.; Dong, J.; Du, X.; Fan, X.H.; Gao, W.; Geng, L.; Ha, E.; He, X.T.; et al. Deformed relativistic Hartree-Bogoliubov theory in continuum with a point-coupling functional. II. Examples of odd Nd isotopes. Phys. Rev. C 2022, 106, 014316. [Google Scholar] [CrossRef]
- Guo, P.; Cao, X.; Chen, K.; Chen, Z.; Cheoun, M.K.; Choi, Y.B.; Lam, P.C.; Deng, W.; Dong, J.; Du, P.; et al. Nuclear mass table in deformed relativistic Hartree–Bogoliubov theory in continuum, II: Even-Z nuclei. At. Data Nucl. Data Tables 2024, 158, 101661. [Google Scholar] [CrossRef]
- Heyde, K.; Van Isacker, P.; Waroquier, M.; Wood, J.L.; Meyer, R.A. Coexistence in odd-mass nuclei. Phys. Rep. 1983, 102, 291. [Google Scholar] [CrossRef]
- Aggarwal, M. Shape coexistence in excited odd-Z proton emitters Eu131-136. Phys. Rev. C 2014, 90, 064322. [Google Scholar] [CrossRef]
- Sun, Q.K.; Sun, T.T.; Zhang, W.; Zhang, S.S.; Chen, C. Possible shape coexistence in odd-A Ne isotopes and the impurity effects of Λ hyperons. Chin. Phys. C 2022, 46, 074106. [Google Scholar] [CrossRef]
- Meng, J. Relativistic Density Functional for Nuclear Structure; World Scientific: Singapore, 2016. [Google Scholar]
- Kucharek, H.; Ring, P.; Schuk, P.; Bengtsson, R.; Girod, M. Pairing properties of nuclear matter from the gogny force. Phys. Lett. B 1989, 216, 249. [Google Scholar] [CrossRef]
- Available online: https://www-phynu.cea.fr/science_en_ligne/carte_potentiels_microscopiques/carte_potentiel_nucleaire_eng.htm (accessed on 4 February 2025).
- Abusara, H.; Ahmad, S. Shape evolution in Kr, Zr, and Sr isotopic chains in covariant density functional theory. Phys. Rev. C 2017, 96, 064303. [Google Scholar] [CrossRef]
- Martinou, A.; Bonatsos, D.; Mertzimekis, T.J.; Karakatsanis, K.E.; Assimakis, I.E.; Peroulis, S.K.; Sarantopoulou, S.; Minkov, N. The islands of shape coexistence within the Elliott and the proxy-SU(3) Models. Eur. Phys. J. A 2021, 57, 84. [Google Scholar] [CrossRef]
- Martinou, A.; Bonatsos, D.; Peroulis, S.K.; Karakatsanis, K.E.; Mertzimekis, T.J.; Minkov, N. Islands of Shape Coexistence: Theoretical Predictions and Experimental Evidence. Symmetry 2023, 15, 29. [Google Scholar] [CrossRef]

| Isotopes | (MeV) | Isotopes | (MeV) | ||
|---|---|---|---|---|---|
| 16F | −0.12, 0.20 | 0.09 | 31Cl | −0.17, 0.09 | 0.27 |
| 17F | −0.08, 0.10 | 0.03 | 32Cl | −0.10, 0.08 | 0.12 |
| 23F | −0.17, 0.19 | 0.16 | 33Cl | −0.07, 0.06 | 0.08 |
| 24F | −0.13, 0.13 | 0.13 | 38Cl | −0.09, 0.10 | 0.11 |
| 25F | −0.08, 0.09 | 0.04 | 39Cl | −0.09, 0.12 | 0.05 |
| 26F | −0.12, 0.13 | 0.02 | 44Cl | −0.22, 0.27 | 0.17 |
| 27F | −0.08, 0.10 | 0.02 | 50Cl | −0.25, 0.06 | 0.23 |
| 28F | −0.09, 0.09 | 0.02 | 51Cl | −0.21, 0.10 | 0.21 |
| 25Na | −0.26, 0.31 | 0.12 | 55Cl | −0.10, 0.08 | 0.27 |
| 30Na | −0.08, 0.10 | 0.10 | 56Cl | −0.06, 0.06 | 0.06 |
| 27Al | −0.27, 0.18 | 0.18 | 33K | −0.09, 0.06 | 0.12 |
| 28Al | −0.19, 0.16 | 0.21 | 34K | −0.06, 0.05 | 0.07 |
| 31Al | −0.16, 0.16 | 0.27 | 35K | −0.05, 0.05 | 0.04 |
| 32Al | −0.11, 0.11 | 0.25 | 37K | −0.08, 0.04 | 0.21 |
| 33Al | −0.04, 0.06 | 0.12 | 38K | −0.07, 0.05 | 0.23 |
| 41Al | −0.36, 0.34 | 0.24 | 40K | −0.07, 0.06 | 0.23 |
| 45Al | −0.26, 0.19 | 0.29 | 41K | −0.06, 0.04 | 0.09 |
| 28P | −0.20, 0.16 | 0.20 | 42K | −0.08, 0.07 | 0.18 |
| 32P | −0.10, 0.08 | 0.15 | 43K | −0.10, 0.06 | 0.28 |
| 34P | −0.06, 0.05 | 0.08 | 50K | −0.10, 0.07 | 0.20 |
| 36P | −0.07, 0.10 | 0.02 | 51K | −0.08, 0.04 | 0.10 |
| 47P | −0.25, 0.13 | 0.13 | 52K | −0.05, 0.03 | 0.03 |
| 50P | −0.05, 0.08 | 0.07 | 53K | −0.07, 0.03 | 0.20 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mun, M.-H.; Papakonstantinou, P.; Kim, Y. Shape Coexistence in Odd-Z Isotopes from Fluorine to Potassium. Particles 2025, 8, 32. https://doi.org/10.3390/particles8010032
Mun M-H, Papakonstantinou P, Kim Y. Shape Coexistence in Odd-Z Isotopes from Fluorine to Potassium. Particles. 2025; 8(1):32. https://doi.org/10.3390/particles8010032
Chicago/Turabian StyleMun, Myeong-Hwan, Panagiota Papakonstantinou, and Youngman Kim. 2025. "Shape Coexistence in Odd-Z Isotopes from Fluorine to Potassium" Particles 8, no. 1: 32. https://doi.org/10.3390/particles8010032
APA StyleMun, M.-H., Papakonstantinou, P., & Kim, Y. (2025). Shape Coexistence in Odd-Z Isotopes from Fluorine to Potassium. Particles, 8(1), 32. https://doi.org/10.3390/particles8010032






