Determining the Ground State for Superheavy Nuclei from the Deformed Relativistic Hartree–Bogoliubov Theory in Continuum
Abstract
1. Introduction
2. Theoretical Framework
3. Numerical Details
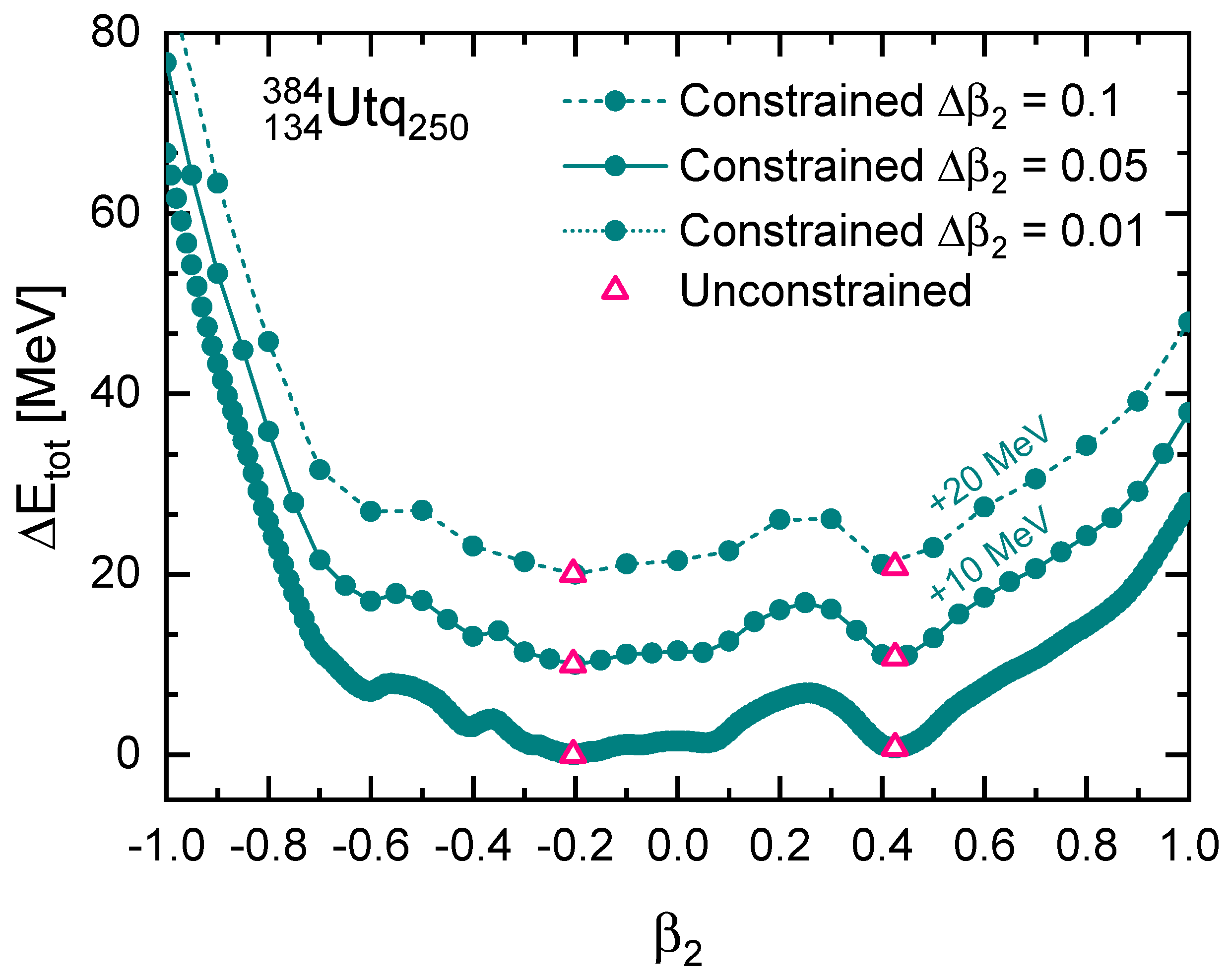
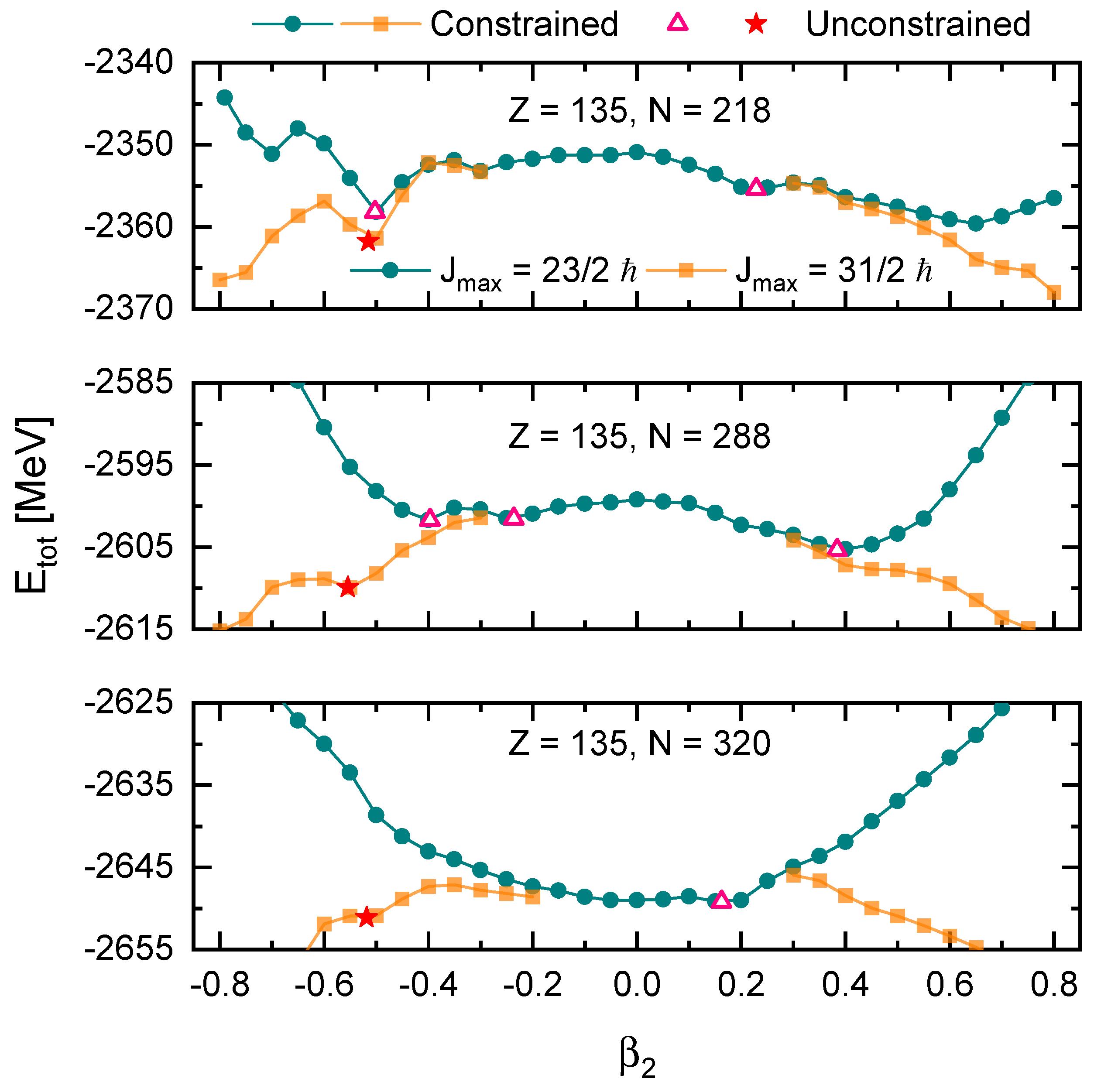
4. Results and Discussion
- Choose the initial deformation step size with to obtain a smooth enough PEC with acceptable computational costs.
- Check the total energies for large deformation with by calculations with .
- Perform unconstrained calculations in the vicinity of local minima in PEC. The configuration with the lowest total energy is the ground state.
5. Summary
- Choose the initial deformation step size with to obtain a smooth enough PEC with acceptable computational costs.
- Check the total energies for large deformation with by calculations with .
- Perform unconstrained calculations in the vicinity of local minima in PEC. The configuration with the lowest total energy is the ground state.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lunney, D.; Pearson, J.M.; Thibault, C. Recent trends in the determination of nuclear masses. Rev. Mod. Phys. 2003, 75, 1021–1082. [Google Scholar] [CrossRef]
- Blaum, K. High-accuracy mass spectrometry with stored ions. Phys. Rep. 2006, 425, 1–78. [Google Scholar] [CrossRef]
- Mumpower, M.; Surman, R.; McLaughlin, G.; Aprahamian, A. The impact of individual nuclear properties on r-process nucleosynthesis. Prog. Part. Nucl. Phys. 2016, 86, 86–126. [Google Scholar] [CrossRef]
- Jiang, X.F.; Wu, X.H.; Zhao, P.W. Sensitivity Study of r-process Abundances to Nuclear Masses. Astrophys. J. 2021, 915, 29. [Google Scholar] [CrossRef]
- Baldo, M.; Burgio, G.F.; Schulze, H.J.; Taranto, G. Nucleon effective masses within the Brueckner-Hartree-Fock theory: Impact on stellar neutrino emission. Phys. Rev. C 2014, 89, 048801. [Google Scholar] [CrossRef]
- Hofmann, S.; Münzenberg, G. The discovery of the heaviest elements. Rev. Mod. Phys. 2000, 72, 733–767. [Google Scholar] [CrossRef]
- Oganessian, Y.T.; Utyonkov, V.K. Super-heavy element research. Rep. Prog. Phys. 2015, 78, 036301. [Google Scholar] [CrossRef] [PubMed]
- Giuliani, S.A.; Matheson, Z.; Nazarewicz, W.; Olsen, E.; Reinhard, P.G.; Sadhukhan, J.; Schuetrumpf, B.; Schunck, N.; Schwerdtfeger, P. Colloquium: Superheavy elements: Oganesson and beyond. Rev. Mod. Phys. 2019, 91, 011001. [Google Scholar] [CrossRef]
- Smits, O.R.; Düllmann, C.E.; Indelicato, P.; Nazarewicz, W.; Schwerdtfeger, P. The quest for superheavy elements and the limit of the periodic table. Nat. Rev. Phys. 2024, 6, 86–98. [Google Scholar] [CrossRef]
- Gregorich, K. Simulation of recoil trajectories in gas-filled magnetic separators. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2013, 711, 47–59. [Google Scholar] [CrossRef]
- Zhang, Z.Y.; Gan, Z.G.; Yang, H.B.; Ma, L.; Huang, M.H.; Yang, C.L.; Zhang, M.M.; Tian, Y.L.; Wang, Y.S.; Sun, M.D.; et al. New Isotope 220Np: Probing the Robustness of the N = 126 Shell Closure in Neptunium. Phys. Rev. Lett. 2019, 122, 192503. [Google Scholar] [CrossRef] [PubMed]
- Ma, L.; Zhang, Z.Y.; Gan, Z.G.; Zhou, X.H.; Yang, H.B.; Huang, M.H.; Yang, C.L.; Zhang, M.M.; Tian, Y.L.; Wang, Y.S.; et al. Short-Lived α-Emitting Isotope 222Np and the Stability of the N = 126 Magic Shell. Phys. Rev. Lett. 2020, 125, 032502. [Google Scholar] [CrossRef] [PubMed]
- Myers, W.D.; Swiatecki, W.J. Nuclear masses and deformations. Nucl. Phys. 1966, 81, 1–60. [Google Scholar] [CrossRef]
- Viola, V.; Seaborg, G. Nuclear systematics of the heavy elements—II Lifetimes for alpha, beta and spontaneous fission decay. J. Inorg. Nucl. Chem. 1966, 28, 741–761. [Google Scholar] [CrossRef]
- Oganessian, Y.T.; Abdullin, F.S.; Alexander, C.; Binder, J.; Boll, R.A.; Dmitriev, S.N.; Ezold, J.; Felker, K.; Gostic, J.M.; Grzywacz, R.K.; et al. Production and Decay of the Heaviest Nuclei 293,294117 and 294118. Phys. Rev. Lett. 2012, 109, 162501. [Google Scholar] [CrossRef]
- Aboussir, Y.; Pearson, J.; Dutta, A.; Tondeur, F. Nuclear mass formula via an approximation to the Hartree–Fock method. At. Data Nucl. Data Tables 1995, 61, 127–176. [Google Scholar] [CrossRef]
- Wang, N.; Liu, M.; Wu, X.; Meng, J. Surface diffuseness correction in global mass formula. Phys. Lett. B 2014, 734, 215–219. [Google Scholar] [CrossRef]
- Möller, P.; Sierk, A.; Ichikawa, T.; Sagawa, H. Nuclear ground-state masses and deformations: FRDM(2012). At. Data Nucl. Data Tables 2016, 109–110, 1–204. [Google Scholar] [CrossRef]
- Samyn, M.; Goriely, S.; Heenen, P.H.; Pearson, J.; Tondeur, F. A Hartree–Fock–Bogoliubov mass formula. Nucl. Phys. A 2002, 700, 142–156. [Google Scholar] [CrossRef]
- Goriely, S.; Chamel, N.; Pearson, J.M. Skyrme-Hartree-Fock-Bogoliubov Nuclear Mass Formulas: Crossing the 0.6 MeV Accuracy Threshold with Microscopically Deduced Pairing. Phys. Rev. Lett. 2009, 102, 152503. [Google Scholar] [CrossRef] [PubMed]
- Goriely, S.; Hilaire, S.; Girod, M.; Péru, S. First Gogny-Hartree-Fock-Bogoliubov Nuclear Mass Model. Phys. Rev. Lett. 2009, 102, 242501. [Google Scholar] [CrossRef]
- Lalazissis, G.; Raman, S.; Ring, P. Ground-state properties of even–even nuclei in the relativistic mean-field theory. At. Data Nucl. Data Tables 1999, 71, 1–40. [Google Scholar] [CrossRef]
- Geng, L.; Toki, H.; Meng, J. Masses, Deformations and Charge Radii—Nuclear Ground-State Properties in the Relativistic Mean Field Model. Prog. Theor. Phys. 2005, 113, 785–800. [Google Scholar] [CrossRef]
- Afanasjev, A.; Agbemava, S.; Ray, D.; Ring, P. Nuclear landscape in covariant density functional theory. Phys. Lett. B 2013, 726, 680–684. [Google Scholar] [CrossRef]
- Agbemava, S.E.; Afanasjev, A.V.; Ray, D.; Ring, P. Global performance of covariant energy density functionals: Ground state observables of even–even nuclei and the estimate of theoretical uncertainties. Phys. Rev. C 2014, 89, 054320. [Google Scholar] [CrossRef]
- Afanasjev, A.V.; Agbemava, S.E.; Ray, D.; Ring, P. Neutron drip line: Single-particle degrees of freedom and pairing properties as sources of theoretical uncertainties. Phys. Rev. C 2015, 91, 014324. [Google Scholar] [CrossRef]
- Xia, X.; Lim, Y.; Zhao, P.; Liang, H.; Qu, X.; Chen, Y.; Liu, H.; Zhang, L.; Zhang, S.; Kim, Y.; et al. The limits of the nuclear landscape explored by the relativistic continuum Hartree–Bogoliubov theory. At. Data Nucl. Data Tables 2018, 121–122, 1–215. [Google Scholar] [CrossRef]
- Zhang, K.; Cheoun, M.K.; Choi, Y.B.; Chong, P.S.; Dong, J.; Dong, Z.; Du, X.; Geng, L.; Ha, E.; He, X.T.; et al. Nuclear mass table in deformed relativistic Hartree–Bogoliubov theory in continuum, I: Even–even nuclei. At. Data Nucl. Data Tables 2022, 144, 101488. [Google Scholar] [CrossRef]
- Guo, P.; Cao, X.; Chen, K.; Chen, Z.; Cheoun, M.K.; Choi, Y.B.; Lam, P.C.; Deng, W.; Dong, J.; Du, P.; et al. Nuclear mass table in deformed relativistic Hartree–Bogoliubov theory in continuum, II: Even-Z nuclei. At. Data Nucl. Data Tables 2024, 158, 101661. [Google Scholar] [CrossRef]
- Geng, J.; Long, W.H. Relativistic Hartree-Fock-Bogoliubov model for axially deformed nuclei. Phys. Rev. C 2022, 105, 034329. [Google Scholar] [CrossRef]
- Geng, J.; Zhao, P.W.; Niu, Y.F.; Long, W.H. A coherent microscopic picture for the exotic structure of 11Be. Phys. Lett. B 2024, 858, 139036. [Google Scholar] [CrossRef]
- Li, L.; Meng, J.; Ring, P.; Zhao, E.G.; Zhou, S.G. Deformed relativistic Hartree–Bogoliubov theory in continuum. Phys. Rev. C 2012, 85, 024312. [Google Scholar] [CrossRef]
- Zhang, K.; Cheoun, M.K.; Choi, Y.B.; Chong, P.S.; Dong, J.; Geng, L.; Ha, E.; He, X.; Heo, C.; Ho, M.C.; et al. Deformed relativistic Hartree–Bogoliubov theory in continuum with a point-coupling functional: Examples of even–even Nd isotopes. Phys. Rev. C 2020, 102, 024314. [Google Scholar] [CrossRef]
- Zhao, P.W.; Li, Z.P.; Yao, J.M.; Meng, J. New parametrization for the nuclear covariant energy density functional with a point-coupling interaction. Phys. Rev. C 2010, 82, 054319. [Google Scholar] [CrossRef]
- Zhang, K.; He, X.; Meng, J.; Pan, C.; Shen, C.; Wang, C.; Zhang, S. Predictive power for superheavy nuclear mass and possible stability beyond the neutron drip line in deformed relativistic Hartree–Bogoliubov theory in continuum. Phys. Rev. C 2021, 104, L021301. [Google Scholar] [CrossRef]
- DRHBc Mass Table Collaboration. Available online: http://drhbctable.jcnp.org (accessed on 20 December 2024).
- Pan, C.; Cheoun, M.K.; Choi, Y.B.; Dong, J.; Du, X.; Fan, X.H.; Gao, W.; Geng, L.; Ha, E.; He, X.T.; et al. Deformed relativistic Hartree–Bogoliubov theory in continuum with a point-coupling functional. II. Examples of odd Nd isotopes. Phys. Rev. C 2022, 106, 014316. [Google Scholar] [CrossRef]
- Dechargé, J.; Berger, J.F.; Dietrich, K.; Weiss, M. Superheavy and hyperheavy nuclei in the form of bubbles or semi-bubbles. Phys. Lett. B 1999, 451, 275–282. [Google Scholar] [CrossRef]
- Bender, M.; Nazarewicz, W.; Reinhard, P.G. Shell stabilization of super- and hyperheavy nuclei without magic gaps. Phys. Lett. B 2001, 515, 42–48. [Google Scholar] [CrossRef]
- Afanasjev, A.; Agbemava, S.; Gyawali, A. Hyperheavy nuclei: Existence and stability. Phys. Lett. B 2018, 782, 533–540. [Google Scholar] [CrossRef]
- Zhou, S.G.; Meng, J.; Ring, P.; Zhao, E.G. Neutron halo in deformed nuclei. Phys. Rev. C 2010, 82, 011301. [Google Scholar] [CrossRef]
- Zhang, W.; Meng, J.; Zhang, S.; Geng, L.; Toki, H. Magic numbers for superheavy nuclei in relativistic continuum Hartree–Bogoliubov theory. Nucl. Phys. A 2005, 753, 106–135. [Google Scholar] [CrossRef]
- Brodziński, W.; Skalski, J. Predictions for superheavy elements beyond Z = 126. Phys. Rev. C 2013, 88, 044307. [Google Scholar] [CrossRef]
- Zhou, S.G.; Meng, J.; Ring, P. Spherical relativistic Hartree theory in a Woods–Saxon basis. Phys. Rev. C 2003, 68, 034323. [Google Scholar] [CrossRef]
- Shen, S.; Liang, H.; Long, W.; Meng, J.; Ring, P. Towards an ab initio covariant density functional for nuclear structure. Prog. Part. Nucl. Phys. 2019, 109, 103713. [Google Scholar] [CrossRef]
- Wang, S.; Zhao, Q.; Ring, P.; Meng, J. Nuclear matter in relativistic Brueckner-Hartree-Fock theory with Bonn potential in the full Dirac space. Phys. Rev. C 2021, 103, 054319. [Google Scholar] [CrossRef]
- Wang, S.; Tong, H.; Wang, C.; Zhao, Q.; Ring, P.; Meng, J. Tensor-force effects on nuclear matter in relativistic ab initio theory. Sci. Bull. 2024, 69, 2166–2169. [Google Scholar] [CrossRef] [PubMed]
- Nikšić, T.; Paar, N.; Reinhard, P.G.; Vretenar, D. Optimizing relativistic energy density functionals: Covariance analysis. J. Phys. G Nucl. Part. Phys. 2015, 42, 034008. [Google Scholar] [CrossRef][Green Version]
- Agbemava, S.E.; Afanasjev, A.V.; Taninah, A. Propagation of statistical uncertainties in covariant density functional theory: Ground state observables and single-particle properties. Phys. Rev. C 2019, 99, 014318. [Google Scholar] [CrossRef]
- Wu, X.H.; Pan, C.; Zhang, K.Y.; Hu, J. Nuclear mass predictions of the relativistic continuum Hartree–Bogoliubov theory with the kernel ridge regression. Phys. Rev. C 2024, 109, 024310. [Google Scholar] [CrossRef]
- Guo, Y.Y.; Yu, T.; Wu, X.H.; Pan, C.; Zhang, K.Y. Nuclear mass predictions of the relativistic continuum Hartree–Bogoliubov theory with the kernel ridge regression. II. Odd-even effects. Phys. Rev. C 2024, 110, 064310. [Google Scholar] [CrossRef]
- Chat, J. Recommendations for the Naming of Elements of Atomic Numbers Greater than 100. Pure Appl. Chem. 1979, 51, 381–384. [Google Scholar] [CrossRef]
- Yang, Y.L.; Wang, Y.K.; Zhao, P.W.; Li, Z.P. Nuclear landscape in a mapped collective Hamiltonian from covariant density functional theory. Phys. Rev. C 2021, 104, 054312. [Google Scholar] [CrossRef]



| A | N | (MeV) | (MeV) | (MeV) | |
|---|---|---|---|---|---|
| (Utq) | |||||
| 352 | 218 | 2360.01 | 2363.23 | 6.714 | −0.511 |
| 388 | 254 | 2516.95 | 2518.84 | 6.492 | 0.461 |
| 422 | 288 | 2600.77 | 2603.71 | 6.170 | −0.563 |
| 454 | 320 | 2639.96 | 2642.49 | 5.821 | −0.518 |
| (Utp) | |||||
| 353 | 218 | 2361.76 | 2364.88 | 6.700 | −0.515 |
| 423 | 288 | 2609.93 | 2612.71 | 6.177 | −0.554 |
| 455 | 320 | 2651.20 | 2653.72 | 5.832 | −0.529 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, S.; Guo, P.; Pan, C. Determining the Ground State for Superheavy Nuclei from the Deformed Relativistic Hartree–Bogoliubov Theory in Continuum. Particles 2024, 7, 1139-1149. https://doi.org/10.3390/particles7040070
Wang S, Guo P, Pan C. Determining the Ground State for Superheavy Nuclei from the Deformed Relativistic Hartree–Bogoliubov Theory in Continuum. Particles. 2024; 7(4):1139-1149. https://doi.org/10.3390/particles7040070
Chicago/Turabian StyleWang, Sibo, Peng Guo, and Cong Pan. 2024. "Determining the Ground State for Superheavy Nuclei from the Deformed Relativistic Hartree–Bogoliubov Theory in Continuum" Particles 7, no. 4: 1139-1149. https://doi.org/10.3390/particles7040070
APA StyleWang, S., Guo, P., & Pan, C. (2024). Determining the Ground State for Superheavy Nuclei from the Deformed Relativistic Hartree–Bogoliubov Theory in Continuum. Particles, 7(4), 1139-1149. https://doi.org/10.3390/particles7040070






