Giant Halo in 66Ca Within Relativistic Continuum Hartree–Bogoliubov Theory Combined with Lipkin–Nogami Method
Abstract
1. Introduction
2. Method
2.1. The Relativistic Continuum Hartree–Bogoliubov Theory
2.2. The Lipkin–Nogami Method
3. Numerical Details
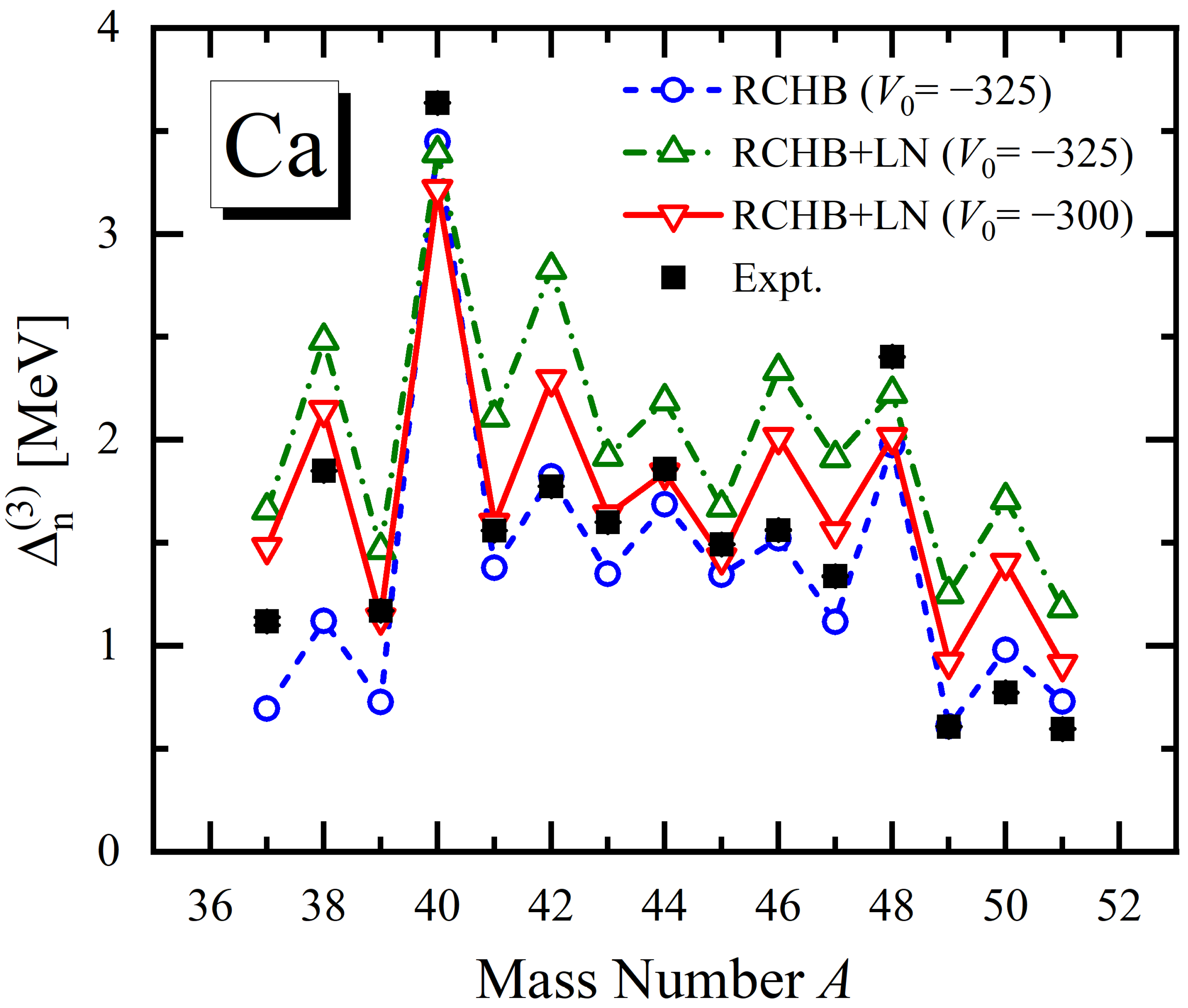
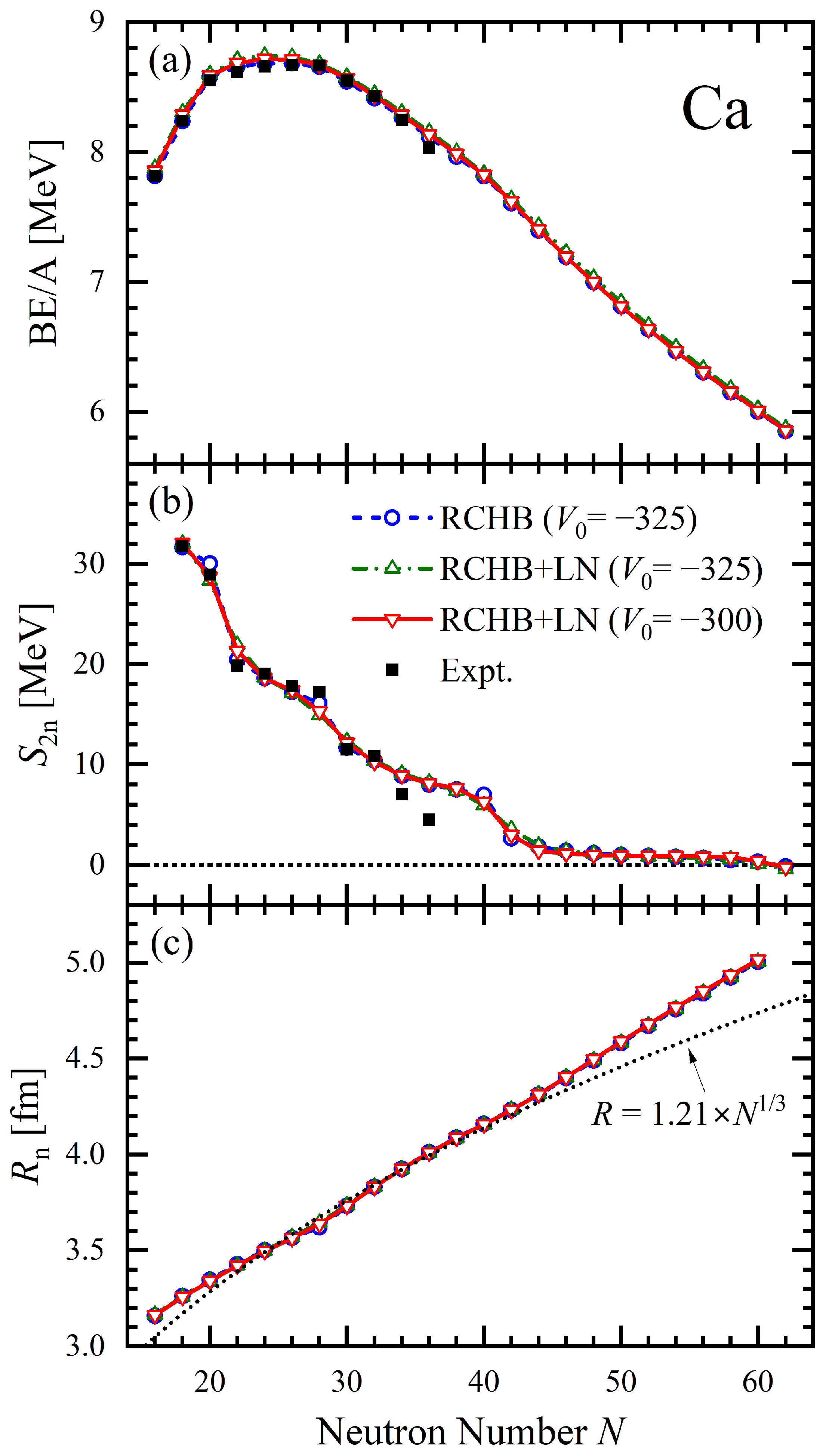
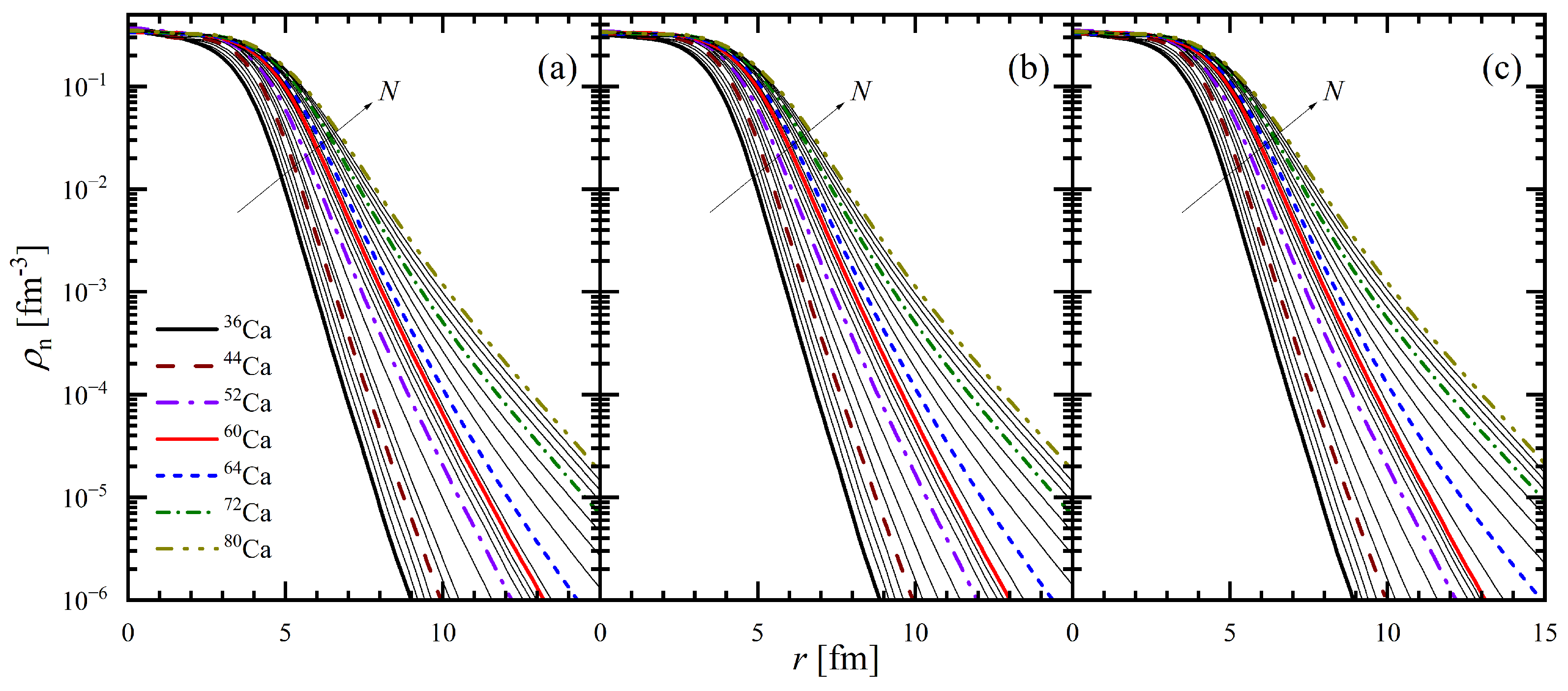
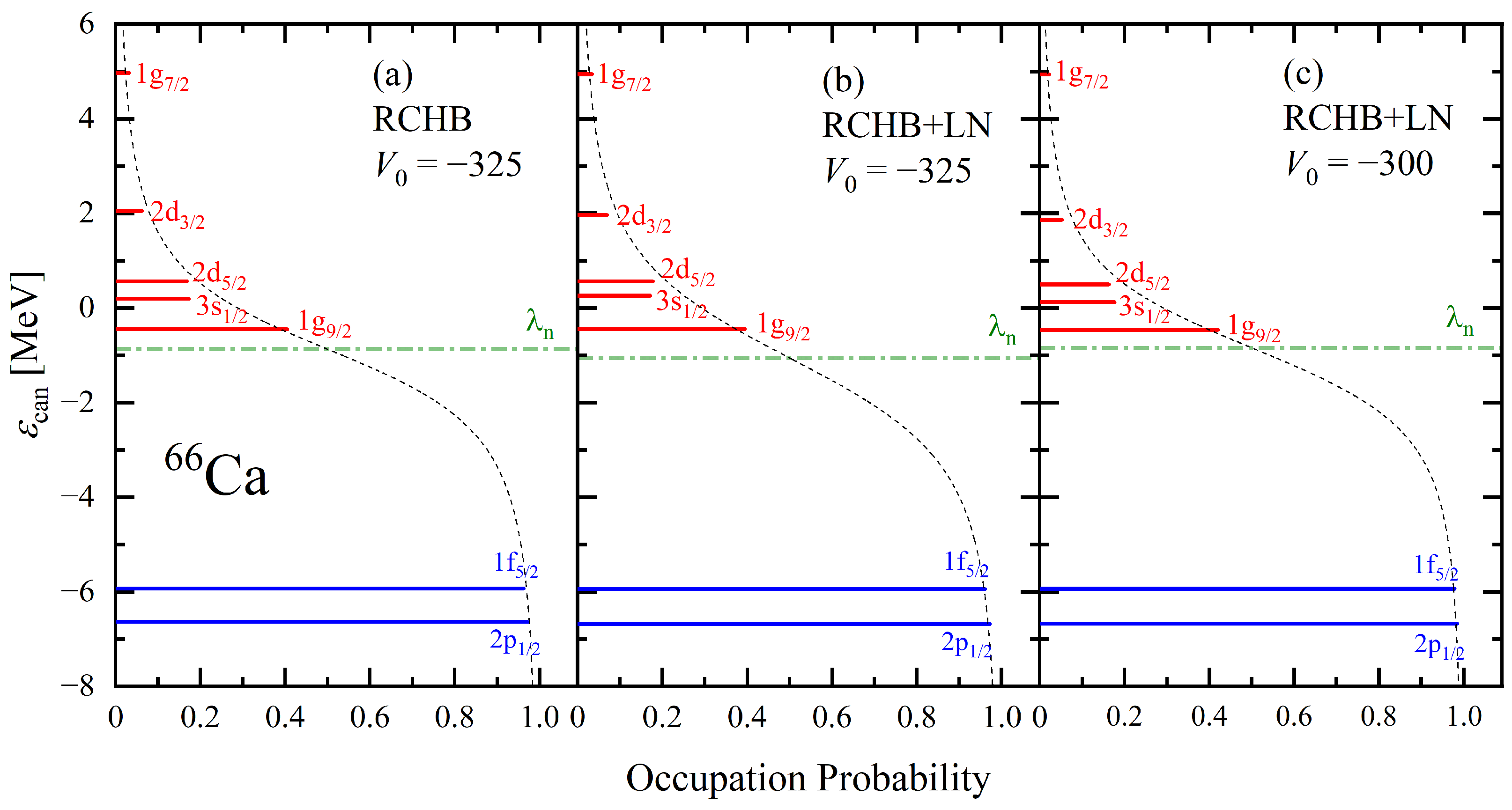
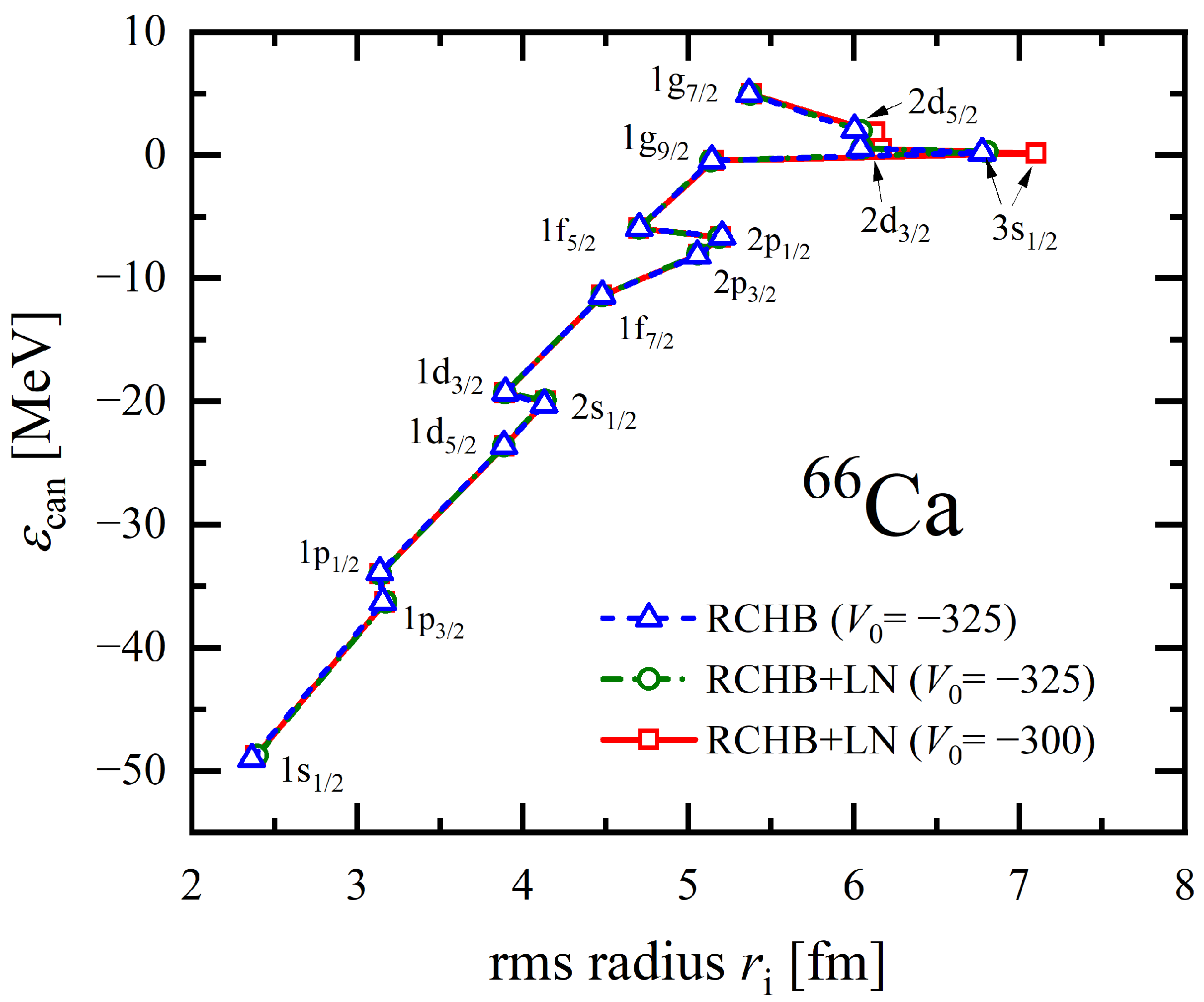
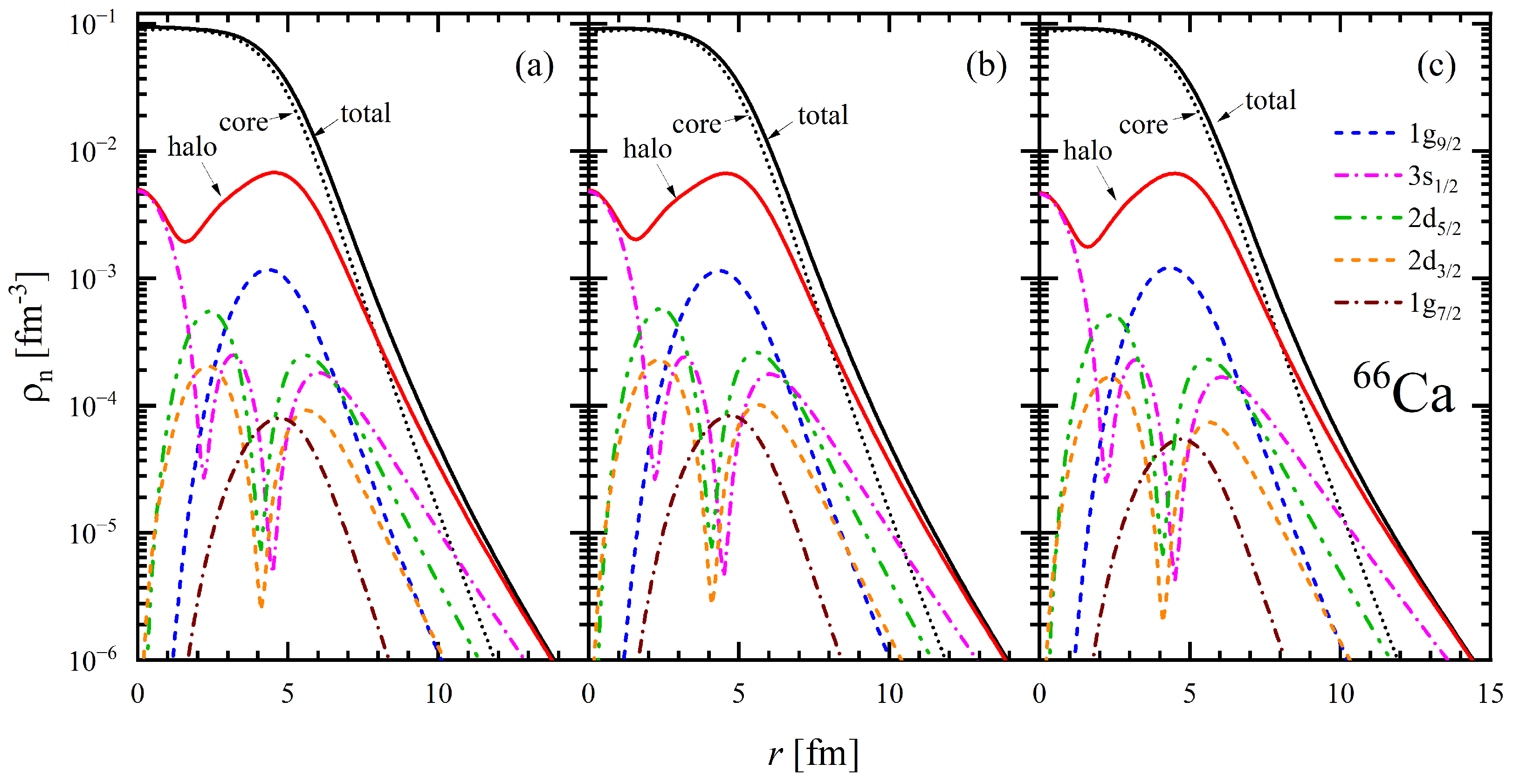
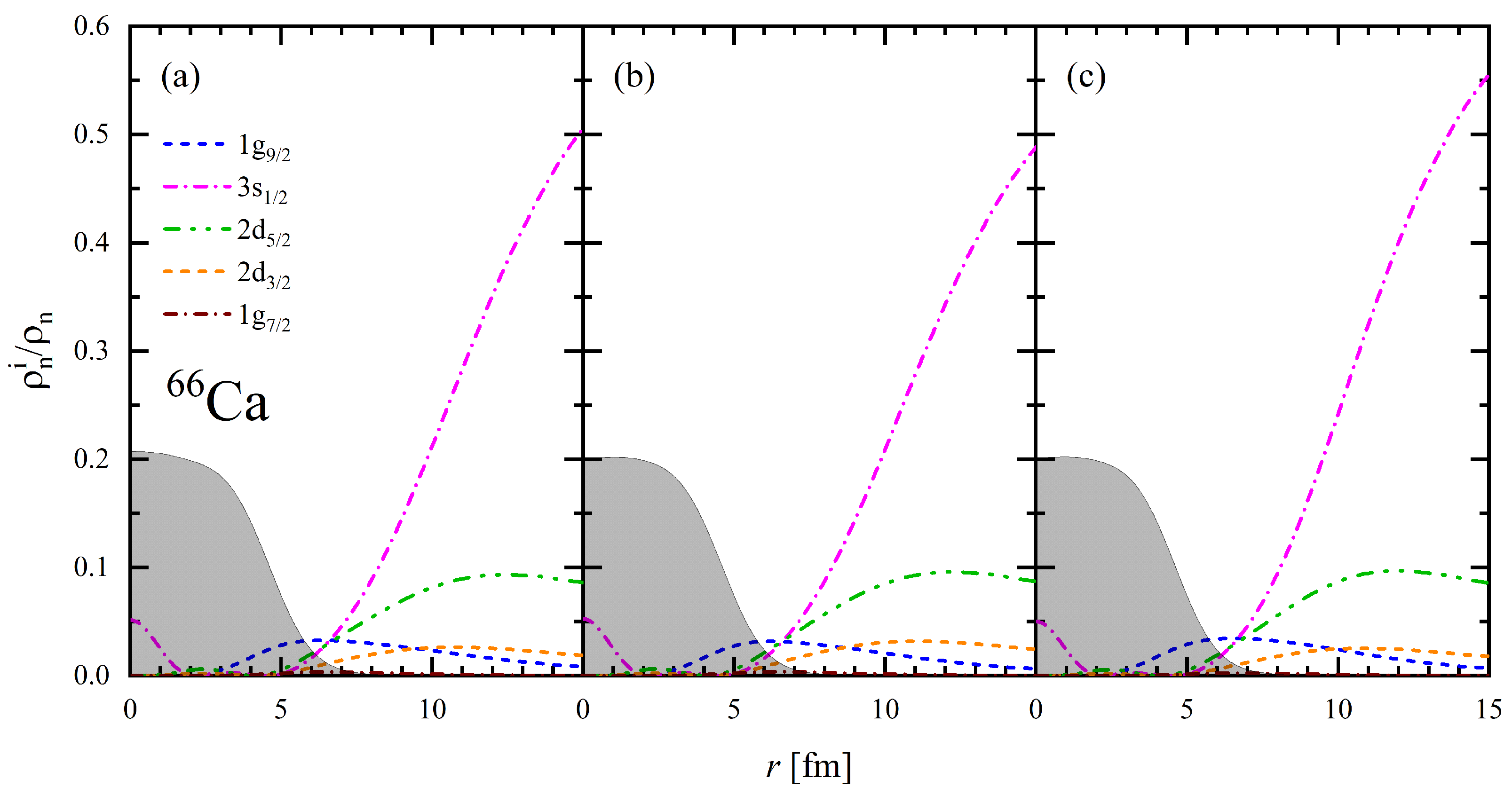
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Vretenar, D.; Afanasjev, A.; Lalazissis, G.; Ring, P. Relativistic Hartree-Bogoliubov theory: Static and dynamic aspects of exotic nuclear structure. Phys. Rep. 2005, 409, 101–259. [Google Scholar] [CrossRef]
- Gade, A.; Glasmacher, T. In-beam nuclear spectroscopy of bound states with fast exotic ion beams. Prog. Part. Nucl. Phys. 2008, 60, 161–224. [Google Scholar] [CrossRef]
- Otsuka, T.; Gade, A.; Sorlin, O.; Suzuki, T.; Utsuno, Y. Evolution of shell structure in exotic nuclei. Rev. Mod. Phys. 2020, 92, 015002. [Google Scholar] [CrossRef]
- Yamaguchi, T.; Koura, H.; Litvinov, Y.; Wang, M. Masses of exotic nuclei. Prog. Part. Nucl. Phys. 2021, 120, 103882. [Google Scholar] [CrossRef]
- Tanihata, I.; Hamagaki, H.; Hashimoto, O.; Shida, Y.; Yoshikawa, N.; Sugimoto, K.; Yamakawa, O.; Kobayashi, T.; Takahashi, N. Measurements of Interaction Cross Sections and Nuclear Radii in the Light p-Shell Region. Phys. Rev. Lett. 1985, 55, 2676–2679. [Google Scholar] [CrossRef]
- Zhang, K.Y.; Papakonstantinou, P.; Mun, M.H.; Kim, Y.; Yan, H.; Sun, X.X. Collapse of the N=28 shell closure in the newly discovered 39Na nucleus and the development of deformed halos towards the neutron dripline. Phys. Rev. C 2023, 107, l041303. [Google Scholar] [CrossRef]
- Kobayashi, T.; Yamakawa, O.; Omata, K.; Sugimoto, K.; Shimoda, T.; Takahashi, N.; Tanihata, I. Projectile Fragmentation of the Extremely Neutron-Rich Nucleus 11Li at 0.79 GeV/nucleon. Phys. Rev. Lett. 1988, 60, 2599–2602. [Google Scholar] [CrossRef] [PubMed]
- Orr, N.A.; Anantaraman, N.; Austin, S.M.; Bertulani, C.A.; Hanold, K.; Kelley, J.H.; Morrissey, D.J.; Sherrill, B.M.; Souliotis, G.A.; Thoennessen, M.; et al. Momentum distributions of 9Li fragments following the breakup of 11Li. Phys. Rev. Lett. 1992, 69, 2050–2053. [Google Scholar] [CrossRef]
- Ieki, K.; Sackett, D.; Galonsky, A.; Bertulani, C.A.; Kruse, J.J.; Lynch, W.G.; Morrissey, D.J.; Orr, N.A.; Schulz, H.; Sherrill, B.M.; et al. Coulomb dissociation of 11Li. Phys. Rev. Lett. 1993, 70, 730–733. [Google Scholar] [CrossRef]
- Meng, J.; Ring, P. Relativistic Hartree-Bogoliubov Description of the Neutron Halo in 11Li. Phys. Rev. Lett. 1996, 77, 3963–3966. [Google Scholar] [CrossRef] [PubMed]
- Meng, J. Relativistic continuum Hartree-Bogoliubov theory with both zero range and finite range Gogny force and their application. Nucl. Phys. A 1998, 635, 3–42. [Google Scholar] [CrossRef]
- Meng, J.; Ring, P. Giant Halo at the Neutron Drip Line. Phys. Rev. Lett. 1998, 80, 460–463. [Google Scholar] [CrossRef]
- Meng, J.; Toki, H.; Zeng, J.Y.; Zhang, S.Q.; Zhou, S.G. Giant halo at the neutron drip line in Ca isotopes in relativistic continuum Hartree-Bogoliubov theory. Phys. Rev. C 2002, 65, 041302. [Google Scholar] [CrossRef]
- Terasaki, J.; Zhang, S.Q.; Zhou, S.G.; Meng, J. Giant halos in relativistic and nonrelativistic approaches. Phys. Rev. C 2006, 74, 054318. [Google Scholar] [CrossRef]
- Sandulescu, N.; Geng, L.S.; Toki, H.; Hillhouse, G.C. Pairing correlations and resonant states in the relativistic mean field theory. Phys. Rev. C 2003, 68, 054323. [Google Scholar] [CrossRef]
- Zhang, S.S.; Xu, X.D.; Peng, J.P. Hints of giant halo in Zr isotopes by resonant RMF+ACCC+BCS approach. Eur. Phys. J. A 2012, 48. [Google Scholar] [CrossRef]
- Ding, K.M.; Shi, M.; Guo, J.Y.; Niu, Z.M.; Liang, H. Resonant-continuum relativistic mean-field plus BCS in complex momentum representation. Phys. Rev. C 2018, 98, 014316. [Google Scholar] [CrossRef]
- Cao, X.N.; Ding, K.M.; Shi, M.; Liu, Q.; Guo, J.Y. Exploration of the exotic structure in Ce isotopes by the relativistic point-coupling model combined with complex momentum representation. Phys. Rev. C 2020, 102, 044313. [Google Scholar] [CrossRef]
- Long, W.H.; Ring, P.; Meng, J.; Van Giai, N.; Bertulani, C.A. Nuclear halo structure and pseudospin symmetry. Phys. Rev. C 2010, 81, 031302. [Google Scholar] [CrossRef]
- Grasso, M.; Yoshida, S.; Sandulescu, N.; Van Giai, N. Giant neutron halos in the non-relativistic mean field approach. Phys. Rev. C 2006, 74, 064317. [Google Scholar] [CrossRef]
- Zhang, Y.; Matsuo, M.; Meng, J. Pair correlation of giant halo nuclei in continuum Skyrme-Hartree-Fock-Bogoliubov theory. Phys. Rev. C 2012, 86, 054318. [Google Scholar] [CrossRef]
- Qu, X.Y.; Zhang, Y. Canonical states in continuum Skyrme Hartree-Fock-Bogoliubov theory with Green’s function method. Phys. Rev. C 2019, 99, 014314. [Google Scholar] [CrossRef]
- Sun, T.T. Green’s function method in covariant density functional theory. Sci. Sin.-Phys. Mech. Astron. 2015, 46, 012006. (In Chinese) [Google Scholar] [CrossRef]
- Qu, X.Y.; Tong, H.; Zhang, S.Q. Canonical states in relativistic continuum theory with the Green’s function method: Neutrons in continuum of zirconium giant-halo nuclei. Phys. Rev. C 2022, 105, 014326. [Google Scholar] [CrossRef]
- Ring, P.; Schuck, P. The Nuclear Many-Body Problem; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Sheikh, J.A.; Dobaczewski, J.; Ring, P.; Robledo, L.M.; Yannouleas, C. Symmetry restoration in mean-field approaches. J. Phys. G Nucl. Part. Phys. 2021, 48, 123001. [Google Scholar] [CrossRef]
- Afanasjev, A.; Ring, P.; König, J. Cranked relativistic Hartree–Bogoliubov theory: Formalism and application to the superdeformed bands in the A∼190 region. Nucl. Phys. A 2000, 676, 196–244. [Google Scholar] [CrossRef]
- Lipkin, H.J. Collective motion in many-particle systems. Ann. Phys. 1960, 9, 272–291. [Google Scholar] [CrossRef]
- Nogami, Y. Improved Superconductivity Approximation for the Pairing Interaction in Nuclei. Phys. Rev. 1964, 134, B313–B321. [Google Scholar] [CrossRef]
- Pradhan, H.; Nogami, Y.; Law, J. Study of approximations in the nuclear pairing-force problem. Nucl. Phys. A 1973, 201, 357–368. [Google Scholar] [CrossRef]
- Bennour, L.; Heenen, P.H.; Bonche, P.; Dobaczewski, J.; Flocard, H. Charge distributions of 208Pb, 206Pb, and 205Tl and the mean-field approximation. Phys. Rev. C 1989, 40, 2834–2839. [Google Scholar] [CrossRef]
- Shi, H.; Wang, X.B.; Dong, G.X.; Wang, H. Abnormal odd-even staggering behavior around 132Sn studied by density functional theory. Chin. Phys. C 2020, 44, 094108. [Google Scholar] [CrossRef]
- Raj, R.; Rustgi, M.L. Modified Tamm-Dancoff approximation calculations using Lipkin-Nogami approach. Phys. Rev. C 1982, 26, 243–248. [Google Scholar] [CrossRef]
- Raj, R.; Rustgi, M.L. Odd Ni isotopes in the Lipkin-Nogami approach. Phys. Rev. C 1983, 28, 935–937. [Google Scholar] [CrossRef]
- Terasaki, J.; Heenen, P.H.; Bonche, P.; Dobaczewski, J.; Flocard, H. Superdeformed rotational bands with density dependent pairing interactions. Nucl. Phys. A 1995, 593, 1–20. [Google Scholar] [CrossRef]
- Afanasjev, A.V.; König, J.; Ring, P. Cranked relativistic Hartree-Bogoliubov theory: Superdeformed bands in the A∼190 region. Phys. Rev. C 1999, 60, 051303. [Google Scholar] [CrossRef]
- Hung, N.Q.; Dang, N.D. Canonical and microcanonical ensemble descriptions of thermal pairing within BCS and quasiparticle random-phase approximation. Phys. Rev. C 2010, 81, 057302. [Google Scholar] [CrossRef]
- Saleh Yousef, M.; Elsharkawy, H.; Rashed, N. Matrix elements of the two-neutrino double beta decay of 76Ge using deformed BCS and Lipkin–Nogami approaches. Nucl. Phys. A 2018, 975, 97–106. [Google Scholar] [CrossRef]
- Meng, J.; Toki, H.; Zhou, S.; Zhang, S.; Long, W.; Geng, L. Relativistic continuum Hartree Bogoliubov theory for ground-state properties of exotic nuclei. Prog. Part. Nucl. Phys. 2006, 57, 470–563. [Google Scholar] [CrossRef]
- Meng, J.; Zhou, S.G. Halos in medium-heavy and heavy nuclei with covariant density functional theory in continuum. J. Phys. G Nucl. Part. Phys. 2015, 42, 093101. [Google Scholar] [CrossRef]
- Kucharek, H.; Ring, P. Relativistic field theory of superfluidity in nuclei. Z. Phys. A 1991, 339, 23–35. [Google Scholar] [CrossRef]
- Zhou, S.G.; Meng, J.; Ring, P. Spherical relativistic Hartree theory in a Woods-Saxon basis. Phys. Rev. C 2003, 68, 034323. [Google Scholar] [CrossRef]
- Zhou, S.G.; Meng, J.; Ring, P.; Zhao, E.G. Neutron halo in deformed nuclei. Phys. Rev. C 2010, 82, 011301. [Google Scholar] [CrossRef]
- Sun, X.X.; Zhao, J.; Zhou, S.G. Shrunk halo and quenched shell gap at N=16 in 22C: Inversion of sd states and deformation effects. Phys. Lett. B 2018, 785, 530–535. [Google Scholar] [CrossRef]
- Zhang, K.; He, X.; Meng, J.; Pan, C.; Shen, C.; Wang, C.; Zhang, S. Predictive power for superheavy nuclear mass and possible stability beyond the neutron drip line in deformed relativistic Hartree-Bogoliubov theory in continuum. Phys. Rev. C 2021, 104, l021301. [Google Scholar] [CrossRef]
- Choi, Y.B.; Lee, C.H.; Mun, M.H.; Kim, Y. Bubble nuclei with shape coexistence in even-even isotopes of Hf to Hg. Phys. Rev. C 2022, 105, 024306. [Google Scholar] [CrossRef]
- Guo, P.; Pan, C.; Zhao, Y.C.; Du, X.K.; Zhang, S.Q. Prolate-shape dominance in atomic nuclei within the deformed relativistic Hartree-Bogoliubov theory in continuum. Phys. Rev. C 2023, 108, 014319. [Google Scholar] [CrossRef]
- Mun, M.H.; Kim, S.; Cheoun, M.K.; So, W.; Choi, S.; Ha, E. Odd-even shape staggering and kink structure of charge radii of Hg isotopes by the deformed relativistic Hartree–Bogoliubov theory in continuum. Phys. Lett. B 2023, 847, 138298. [Google Scholar] [CrossRef]
- Nikšić, T.; Vretenar, D.; Ring, P. Beyond the relativistic mean-field approximation. II. Configuration mixing of mean-field wave functions projected on angular momentum and particle number. Phys. Rev. C 2006, 74, 064309. [Google Scholar] [CrossRef]
- Zhang, K.; Cheoun, M.K.; Choi, Y.B.; Chong, P.S.; Dong, J.; Dong, Z.; Du, X.; Geng, L.; Ha, E.; He, X.T.; et al. Nuclear mass table in deformed relativistic Hartree–Bogoliubov theory in continuum, I: Even–even nuclei. At. Data Nucl. Data Tables 2022, 144, 101488. [Google Scholar] [CrossRef]
- Zhao, P.W.; Li, Z.P.; Yao, J.M.; Meng, J. New parametrization for the nuclear covariant energy density functional with a point-coupling interaction. Phys. Rev. C 2010, 82, 054319. [Google Scholar] [CrossRef]
- Zhang, K.; Cheoun, M.K.; Choi, Y.B.; Chong, P.S.; Dong, J.; Geng, L.; Ha, E.; He, X.; Heo, C.; Ho, M.C.; et al. Deformed relativistic Hartree-Bogoliubov theory in continuum with a point-coupling functional: Examples of even-even Nd isotopes. Phys. Rev. C 2020, 102, 024314. [Google Scholar] [CrossRef]
- Pan, C.; Cheoun, M.K.; Choi, Y.B.; Dong, J.; Du, X.; Fan, X.H.; Gao, W.; Geng, L.; Ha, E.; He, X.T.; et al. Deformed relativistic Hartree-Bogoliubov theory in continuum with a point-coupling functional. II. Examples of odd Nd isotopes. Phys. Rev. C 2022, 106, 014316. [Google Scholar] [CrossRef]
- Guo, P.; Cao, X.; Chen, K.; Chen, Z.; Cheoun, M.K.; Choi, Y.B.; Lam, P.C.; Deng, W.; Dong, J.; Du, P.; et al. Nuclear mass table in deformed relativistic Hartree–Bogoliubov theory in continuum, II: Even-Z nuclei. At. Data Nucl. Data Tables 2024, 158, 101661. [Google Scholar] [CrossRef]
- Wang, M.; Huang, W.; Kondev, F.; Audi, G.; Naimi, S. The AME 2020 atomic mass evaluation (II). Tables, graphs and references. Chin. Phys. C 2021, 45, 030003. [Google Scholar] [CrossRef]
- Centelles, M.; Roca-Maza, X.; Viñas, X.; Warda, M. Origin of the neutron skin thickness of 208Pb in nuclear mean-field models. Phys. Rev. C 2010, 82, 054314. [Google Scholar] [CrossRef]
- Roca-Maza, X.; Centelles, M.; Viñas, X.; Warda, M. Neutron Skin of 208Pb, Nuclear Symmetry Energy, and the Parity Radius Experiment. Phys. Rev. Lett. 2011, 106, 252501. [Google Scholar] [CrossRef] [PubMed]
- Warda, M.; Centelles, M.; Viñas, X.; Roca-Maza, X. Influence of the single-particle structure on the nuclear surface and the neutron skin. Phys. Rev. C 2014, 89, 064302. [Google Scholar] [CrossRef]
- Fayans, S.; Tolokonnikov, S.; Trykov, E.; Zawischa, D. Nuclear isotope shifts within the local energy-density functional approach. Nucl. Phys. A 2000, 676, 49–119. [Google Scholar] [CrossRef]
- Reinhard, P.G.; Nazarewicz, W. Toward a global description of nuclear charge radii: Exploring the Fayans energy density functional. Phys. Rev. C 2017, 95, 064328. [Google Scholar] [CrossRef]
- Miller, A.J.; Minamisono, K.; Klose, A.; Garand, D.; Kujawa, C.; Lantis, J.D.; Liu, Y.; Maaß, B.; Mantica, P.F.; Nazarewicz, W.; et al. Proton superfluidity and charge radii in proton-rich calcium isotopes. Nat. Phys. 2019, 15, 432–436. [Google Scholar] [CrossRef]
- An, R.; Geng, L.S.; Zhang, S.S. Novel ansatz for charge radii in density functional theories. Phys. Rev. C 2020, 102, 024307. [Google Scholar] [CrossRef]
- Yang, D.; Rong, Y.T.; An, R.; Shi, R.X. Potential signature of new magicity from universal aspects of nuclear charge radii. arXiv 2024, arXiv:2411.03076. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, C.; Guo, P.; Jiang, X. Giant Halo in 66Ca Within Relativistic Continuum Hartree–Bogoliubov Theory Combined with Lipkin–Nogami Method. Particles 2024, 7, 1128-1138. https://doi.org/10.3390/particles7040069
Zhou C, Guo P, Jiang X. Giant Halo in 66Ca Within Relativistic Continuum Hartree–Bogoliubov Theory Combined with Lipkin–Nogami Method. Particles. 2024; 7(4):1128-1138. https://doi.org/10.3390/particles7040069
Chicago/Turabian StyleZhou, Chang, Peng Guo, and Xiaofei Jiang. 2024. "Giant Halo in 66Ca Within Relativistic Continuum Hartree–Bogoliubov Theory Combined with Lipkin–Nogami Method" Particles 7, no. 4: 1128-1138. https://doi.org/10.3390/particles7040069
APA StyleZhou, C., Guo, P., & Jiang, X. (2024). Giant Halo in 66Ca Within Relativistic Continuum Hartree–Bogoliubov Theory Combined with Lipkin–Nogami Method. Particles, 7(4), 1128-1138. https://doi.org/10.3390/particles7040069





