Experimental Study of Cold Dense Nuclear Matter

Abstract

The fundamental theory of nuclear interactions, Quantum Chromodynamics (QCD), operates in terms of quarks and gluons at higher resolution. At low resolution the relevant degrees of freedom are nucleons. Two-nucleon Short-Range Correlations (SRC) help to interconnect these two descriptions. SRCs are temporary fluctuations of strongly interacting close pairs of nucleons. The distance between the two nucleons is comparable to their radii and their relative momenta are larger than the fermi sea level. According to the electron scattering experiments held in the last decade, SRCs have far-reaching impacts on many-body systems, the nucleon-nucleon interactions, and nuclear substructure. The modern experiments with ion beams and cryogenic liquid hydrogen target make it possible to study properties of the nuclear fragments after quasi-elastic knockout of a single nucleon or an SRC pair. Here we review the status and perspectives of the SRC program in so-called inverse kinematics at JINR (Dubna, Russia). The first SRC experiment at the BM@N spectrometer (2018) with 4 GeV/c/nucleon carbon beam has shown that detection of an intact 11B nucleus after interaction selects out the quasi-elastic knockout reaction with minimal contribution of initial- and final-state interactions. Also, 25 events of SRC-breakups showed agreement in SRC properties as known from electron beam experiments. The analysis of the second measurement of SRC at BM@N held in 2022 with an improved setup is currently ongoing. The SRC project at JINR moved to a new experimental area in 2023, where the next measurement is being planned in terms of experimental setup and physics goals.

1. Introduction

Short-Range Correlations (SRCs) are local nuclear density fluctuations created by close-proximity nucleon pairs [1,2,3]. The two nucleons of SRC pairs come as close as a nucleon radius for a very short time, so that they partly overlap. Considering an SRC pair in momentum space, the individual momenta of both nucleons are opposite and high in absolute value compared to the corresponding Fermi momentum. The local density of SRC pairs is around 2–5 times higher than the average nuclear density (0.17 nucleons/fm³) and comparable to that of a neutron star. Studying SRCs inside various nuclei allows for investigation of cold dense nuclear matter, complementing the approach of heavy ion collisions focusing on hot dense nuclear matter studies.

The first experiments at JINR (Dubna, Russia) started in 1957 initiated interest for the short-range structure of nuclei. They used a 675 MeV proton beam impinging on nuclear targets and showed significant amount of forward-going secondary deuterons with momenta up to 1.5 GeV/c [4,5]. The observed process looked kinematically similar to the elastic pd scattering, which lead to a conclusion, that the beam protons knock out quasi-deuterons from nuclei. There has been a series of experiments (e.g., [6]) observing quasi-elastic knockout of light ions and later mesons from nuclear targets at high momentum transfer, which inspired interest to the nature of short-range correlations within nuclei and nucleon-nucleon interactions at short distances. In 1971 A. M. Baldin suggested [7] the idea of scaling invariance for relativistic ion collisions. Spectra of high-energy secondary particles in collisions with relativistic ions characterize the local properties of nuclear matter rather than these of a particular reaction. The cumulative reactions, when scattering happens off a nucleon cluster comprised by several nucleons, have been thoroughly investigated both in theory and experiment. D. I. Blokhintsev suggested [8] that nucleons can form instant compact clusters within nuclei, which cause fluctuations of local nuclear density. The “fluctuons” are defined as compressed atomic nuclei of lighter mass inside a larger nucleus. The experiments studying elastic backward scattering of protons off light ions at high momentum transfer (e.g., [9]) can be described using fluctuon-based approach. The idea of fluctuons has been developed into two types of models: the first assumes the nuclear density fluctuations are comprised by multi-quark configurations [10], and the second considers few-nucleon correlations [11]. Deep inelastic electron scattering at high Bjorken x > 1 [12] showed that the local nuclear density fluctuations are a property of nuclei and are not dynamically created by the probe.

The first SRC experiment [13,14] used 6, 8, and 9 GeV proton beam scattered on a carbon target at an angle of around 90° in the center of mass frame. The cross section $\frac{d\sigma }{dt}$ in this case falls as $s^{-10}$ [15] (where s and t are the Mandelstam variables), which increases the probability of interactions with protons having large momentum along the beam direction. The two protons in the final state: the scattered and the knocked-out ones were detected, and it was searched for a second nucleon emitted in the direction of the proton momentum before the interaction (missing) p_miss = p_p − q, where p_p is the detected proton momentum, q is the momentum transfer. For the most cases of high momentum protons in the final state there was a partner neutron emitted in the direction opposite to p_miss indicating a strong correlation between them. The low-momentum neutrons have an isotropic momentum distribution relative to p_miss. The same effect, when knocked-out high-momentum protons almost always have a correlated neutron partner, was later observed with electron beams [16] too. The agreement between the experimental results with electron and proton probes, which have significantly different reaction mechanisms, supported the following data interpretation: carbon nucleus contains proton-neutron SRC pairs, where the relative nucleon momentum is high. Similar measurements [17] on Al, Fe, and Pb targets demonstrated the dominance of neutron-proton pairs over neutron-neutron and proton-proton ones even for heavy asymmetric nuclei.

An accessory analysis aiming at estimation of relative event rates with high and low p_miss [18] was performed using CLAS data of (e, e’ p) and (e, e’ n) reactions on C, Al, Fe, and Pb targets with a 5 GeV electron beam. The ratio of the number of neutrons to protons in the final state with low p_miss increased with the mass of the target nucleus approximately as the ratio of neutrons to protons (N/Z). The same ratio for the high p_miss events did not depend on the nucleus mass, which is consistent with np-dominance. This resulted in the fraction of high p_miss protons increasing with N/Z.

One interesting consequence of the np-dominance effect is the kinetic energy-sharing inversion. Within the independent particle model in case of neutron-rich nuclei the average kinetic energy for neutrons will be higher than that for protons. However, considering the SRC neutron-proton pairs, there will be equal number of neutrons and protons with high kinetic energy even in asymmetric nuclei such as ²⁰⁸Pb, where N/Z = 128/82 ≈ 1.5. If approximately 20% of nucleons in a nucleus belong to SRC pairs, the fraction of protons with high kinetic energy will be 20/82 ≈ 0.25, and the fraction of energetic neutrons will be only 20/128 ≈ 0.16.

The dominance of neutron-proton SRC pairs was experimentally observed for relative nucleon momenta within SRC pairs from 300 to 600 MeV/c. The neutron-proton SRC pairs have basically quantum numbers of a deuteron, therefore, the shape of the S- and D-components of the deuteron wave-function (see Figure 1) have direct consequences for the properties of SRC pairs. The S-component corresponding to spin-0 has a minimum at 400 MeV/c, and the range between 300 and 600 MeV/c is dominated by the tensor D-component for spin-1 nucleon pairs. The proton-proton and neutron-neutron pairs with the total spin of 1 are strongly suppressed due to Pauli blocking, which results into the observed np-dominance of SRC. However, for the nucleon momentum range above 600 MeV/c we expect the ratio of proton-proton to proton-neutron SRC pairs to increase.

The ratio between the reaction yields for (e, e’ pp) and (e, e’ p) on carbon target as a function of the measured missing momentum was recently compared to various calculations using different nucleon-nucleon potentials [19]. There is a reasonable agreement between the data and AV18 potential. The relative reaction yield of (e, e’ pp) compared to (e, e’ p) is small for p_miss around 400 MeV/c, which is consistent with the previous measurements, and increases to 0.16 for p_miss of 800 MeV/c. This is consistent with simple pair counting after considering the effects of the detector acceptance. The same increase was observed for calculations using very different nucleon-nucleon potentials, ranging from the relatively hard AV18 potential to the far softer N2LO(1.0) and N2LO(1.2) potentials. The probability of creating SRC pairs was lower for the softer potentials, but the pp to np pair fraction was very similar. Potentials without a tensor component, such as the AV4’ potential, exhibited very different behavior and did not agree with the measured ratio of proton-proton to neutron-proton pairs.

This result was confirmed by comparing the data from electron scattering on a carbon target between three event types: (e, e’ pp), (e, e’ pn), and (e, e’ p) for the same data set [20]. It was shown that the (e, e’ pn)-to-(e, e’ p) ratio after corrections for experimental effects including efficiency and acceptance was consistent with unity for the values of nucleon momentum before the interaction in the range from 300 to 1000 MeV/c. Also, the acceptance-corrected (e, e’ pp) to (e, e’ p) ratio at the largest missing momenta approached the scalar limit of 0.33.

The definition of an SRC pair in the momentum space is the following: a pair with a high relative momentum larger than the Fermi momentum for the given nucleus and a small momentum of the center-of-mass. The center-of-mass momentum was measured using an electron probe in the (e, e’ pp) reaction by reconstructing the total momentum of the proton–proton pair before the interaction with the virtual photon. The total pair momentum was measured [21] in the two directions perpendicular to the momentum transfer. In [17] it was found that the motion of the SRC pair perpendicular to the momentum transfer can be described by a gauss distribution with a standard deviation of 140–170 MeV/c. The SRC center-of-mass momentum was also measured with hadron probe in inverse kinematics [22], and here the SRC pair balances the A-2 residual nucleus in the center-of-mass frame of the incident nucleus. The values for the standard deviation of the SRC pairs center-of-mass momentum agree between the three different types of measurements. The width of the distribution increases from σ ≈ 100 MeV/c for ⁴He to about 140 MeV/c for ¹²C and about 150 MeV/c for Al, Fe, and Pb. The value of the width for the center-of-mass momentum distribution of the SRC pairs can help identify which nucleons form SRC pairs, because SRC pairs are formed from two mean-field nucleons at a short distance, so that the total momentum of the SRC pair should equal to the summed momenta of those two nucleons. The measured values are consistent with the idea that the SRC paired nucleons are just two random nucleons originating from a realistic nucleon momentum distribution or a Fermi gas distribution. A slightly less consistent explanations would be the two nucleons in a relative S state and possible nucleon-nucleon pairs from shell model orbits [23].

The distance between nucleons in the SRC state is smaller than the nucleon radius, which means that the interaction between the correlated nucleons is much more intense than the total interaction with the remaining A-2 nucleons. This leads to the idea that the detailed characteristics of the SRC pairs are universal, i.e., independent of the nucleus type/mass. Calculations of two-nucleon position and momentum densities for different nuclei and a set of potentials show that at short distances (≤1 fm) and high momenta those distributions are nucleus-independent. Therefore, the SRC pairs can be modeled as a nucleus-independent scaling coefficient (contact) multiplied by a universal position- or momentum-space density. This approach is the base for the Generalized Contact Formalism (GCF) [24,25]. The following momenta values characterizing the SRC pair: the SRC center-of-mass motion described by a gauss with the standard deviation of ~150 MeV/c, the relative momentum of the SRC nucleon p_miss > 300 MeV/c, and the momentum transfer needed to break up an SRC pair above 1 GeV/c, have different scales. The GCF model describes scattering from SRC pairs very well [19].

The choice of nucleon–nucleon potential defines the shape of the two-body densities, but the ratios of scaling coefficients representing the amount of SRC pairs are independent from the particular potential type. The calculations [24] show that the contact ratios relative to deuterium (for s = 1 neutron-proton pairs) and to ⁴He (for s = 0 proton-proton pairs) are independent of nucleon-nucleon potential for nuclei from A = 3 to 40. The proton–neutron contacts are the same for both effective field theories and phenomenological potentials (N2LO(1.0), N2LO(1.2), and AV18) in both position and momentum space.

Thus, the two-nucleon distributions show significant scale and scheme dependence at small distances or high momentum. But the extracted ratios of scaling coefficients for nuclei A to deuterium or ⁴He are scale- and scheme-independent. This is true for both short distances and high momenta. Therefore, the formation of SRC pairs within the nucleus, which determines their abundances, is a long-range (mean-field) phenomenon.

Since the phenomenon of short-range nucleonic correlations stems from the basic properties of nuclear matter, it is essential to study the effects of short-range correlations using different probes, nuclei, and reactions to be able to focus on the ground state properties and get rid of reaction effects and other processes smearing the picture. The state-of-the-art world-wide effort aiming to reveal the nature of SRC is using largely electron, but also real photon, and hadron beams around the world: Thomas Jefferson National Accelerator Facility, GSI Helmholts Zentrum fuer Schwerionenforschung GmbH and FAIR, and Joint Institute for Nuclear Research (NICA). The experiments, which are going on and planned at these facilities, complement each other and aim at refining our understanding of the details of nuclear matter at short distances and high momenta.

In the following we aim to focus on the JINR program of SRC measurements in inverse kinematics, which is characterized by the usage of high-momentum beam of carbon-12 ions and a liquid hydrogen target. Inverse kinematic experiments have a unique possibility to study the nuclear fragment(s) after interaction.

2. Upgrade of the SRC Experimental Setup at JINR

The first and second JINR SRC measurement in inverse kinematics were performed at the BM@N spectrometer with a 48 GeV/c and 44.4 GeV/c momentum carbon beam from the Nuclotron accelerator incident on a proton target [26] in 2018 and 2022, respectively. The baseline configuration of the BM@N setup was modified with the two-arm spectrometer for registration of the scattered and knocked-out protons at an angle of 30° with respect to the beam, which corresponds to back-to-back elastic (pp, pp) scattering in the center-of-mass system. The automodel behavior [15] of the elastic (pp, pp) cross section near 90° was used to increase the probability of interactions with high-momentum nuclear protons. The details and the results of the 2018 pilot measurement can be found in [22]. Inspired by the first successful measurement, which served as a proof of concept and allowed accessing ground state properties of 12C in a quasi-free unperturbed single-step reaction ¹²C (p, 2p) ¹¹B as well as measuring properties of SRC pairs, the second measurement was conducted in 2022. It aimed at measuring the absolute cross sections, quenching, and attenuation at high momentum transfer for the cases of quasi-elastic single proton knockout reaction. And for the SRC studies the main objectives were to achieve higher statistics, detect the recoil partner, and perform multi-fragment reconstruction with the idea to study fragmentation patterns and get a clue on the production mechanism of SRC pairs.

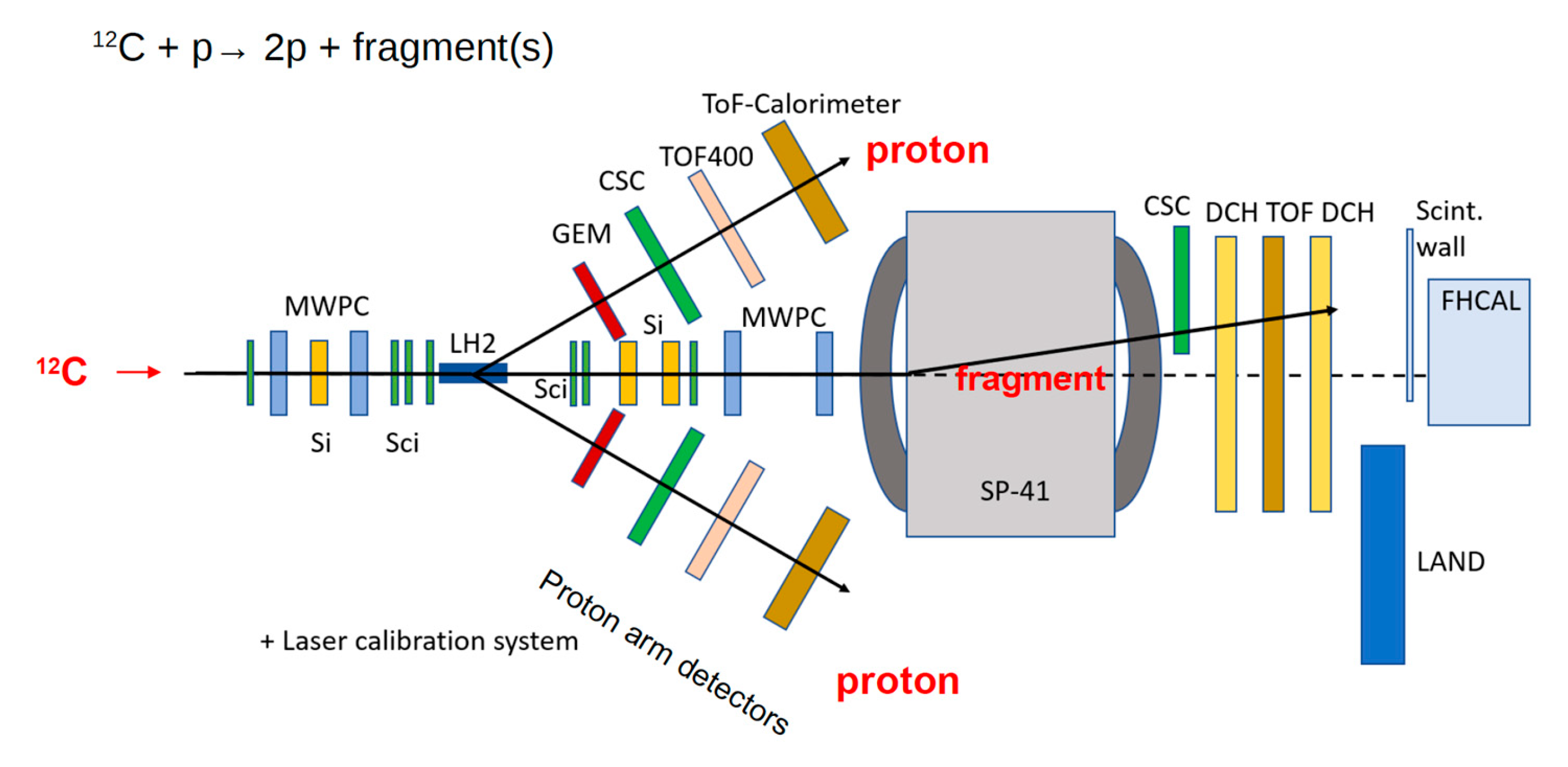
To achieve these goals, several aspects of the experimental setup were improved compared to 2018. The schematic view of the experimental setup in 2022 is shown in Figure 2. Two new start time scintillator beam counters and three scintillator counters for charge measurements (Sci in Figure 1) were designed and produced to achieve a better time and amplitude resolutions. Each counter was read out by two PMTs, and the light signal was transported from the scintillator to the PMT window by a Plexiglas light guide (compared to a single PMT for each counter and air light guides in 2018). The new compact cryogenic liquid hydrogen target [18] was designed, developed, and manufactured at JINR. Two pairs of double-sided Si detectors, where each second detector was rotated by 90 degrees with respect to the beam direction, were used for coordinate measurements of the fragments downstream the target. The two-arm spectrometer was improved with new detectors compared to 2018. Each arm contained a GEM (Gas Electron Multiplier) [27] and CSC (Cathode-Strip Chamber) coordinate planes as well as a time-of-flight TOF400 detector based on MRPC (Multi-gap Resistive Plate Chambers) technology. The new large-area Tof-Calorimeter, with a width of 1.5 m and height of 2 m, was assembled at JINR and located at each arm. The Tof-Calorimeter consisted of a scintillator array providing timing information and three layers of LAND [28] modules providing statistical proton/pion separation. Downstream, the analyzing magnet a CSC was added for tracking of light fragments with large turning angles. The scintillator wall provided charge information for each fragment in the final state. A new laser calibration system with optical fibers going to all scintillator detectors was used to perform time calibration without beam. The main physics trigger was formed based on the signals from the scintillator beam counters and the scintillator layer of the Tof-Calorimeter on both arms.

3. Discussion

The data analysis of the 2022 experiment is currently ongoing. The analysis results will be used to refine the physics program for the next SRC measurement at JINR planned at the HyperNIS experimental area. The engineering work has been started to accommodate for the new advanced SRC experimental setup.

The SRC project at JINR is motivated by a combination of the following factors: unique high-energy ion beams provided by the Nuclotron-Booster accelerator facility (inverse kinematics), and a possibility of a complete exclusive measurements in inverse kinematics at the BM@N and HyperNIS spectrometers. A special interest is the availability of polarized deuteron beams with high energies up to 6 GeV/nucleon and high intensities. The quasi-elastic proton knockout measurements at high energies, large momentum transfer, large missing momentum, and a possibility to detect the recoil partner allow investigating the following aspects of SRC:

-

Formation process of SRC pairs and their spin structure.

-

Probing SRC pairs gives access to the short-range part of nucleon–nucleon interaction which is a key in any modern theory of nuclei and denser nuclear systems. SRC studies at JINR can provide valuable input for constraining the nucleon–nucleon potential in high-resolution scale using precision measurements of both static and dynamic SRC properties [19,20]. A more challenging task is the search for three-nucleon correlations.

-

Understanding neutron stars, supernova, and perhaps even isotope production and abundances in the universe requires microscopic understanding of nuclear interaction in a cold, dense, neutron-rich environment. SRC studies potentially provide a lab on Earth to do so. SRC local density is equivalent to what is predicted in neutron-star cores, and it is possible to access multi-nucleon forces in the nuclear environment. A future goal is to expedite the link between SRC properties to density and asymmetry dependence, and constraints of the equation of state. These efforts require active theory participation combining ab-initio theory, studies of the equation of state, and astrophysics.

-

The nucleus at high densities is not well understood from first principles. SRCs can embody an ideal environment to address such questions. Their macroscopic structure in high-resolution scale, e.g., when nucleons overlap, does require to consider and include non-nucleonic degrees of freedom in experiment and theory with relativistic descriptions. That poses challenges even to advanced nuclear physics beyond the scale-limited scope of effective theories.

The few-body system of SRC can be understood as a lab to study cold dense nuclear matter across scales in terms of resolution, densities, and asymmetry. JINR has unique high-energy ion beams, making it possible to perform exclusive measurements in inverse kinematics to study SRCs. The SRC project at JINR successfully started with two measurements at the BM@N experimental area in 2018 and 2022, and is being continued. The physics program for the upcoming SRC experiments is being developed based on the results from 2018 and 2022 and state of the art of the SRC field both in experiment and theory. The SRC project at JINR naturally complements the world-wide efforts on SRC studies.