Precision Storage Rings for Electric Dipole Moment Searches: A Tool En Route to Physics Beyond-the-Standard-Model
Abstract
1. Introduction
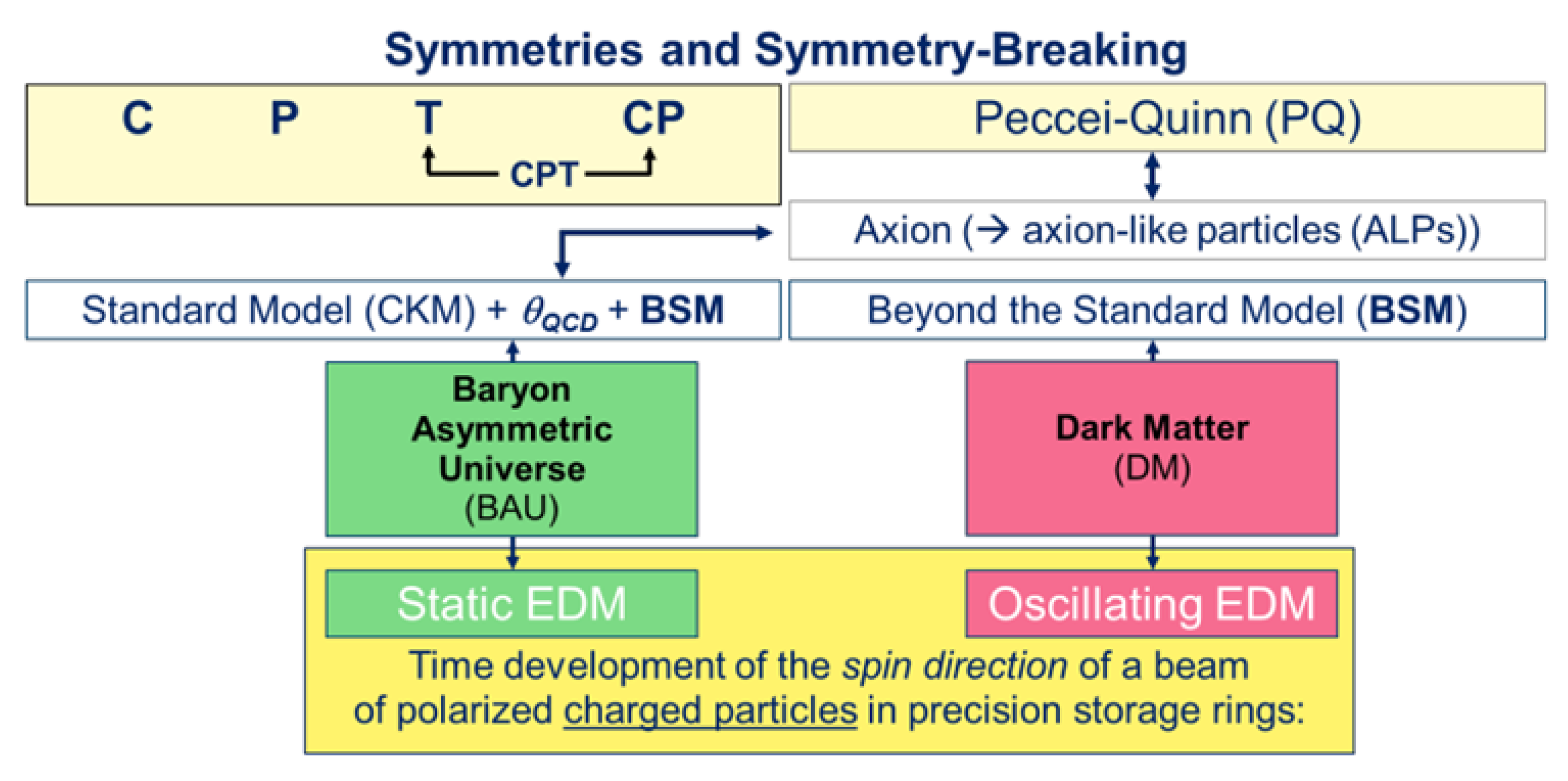
1.1. Scientific Background
- Reason for the Baryon-asymmetric Universe (BAU) (Why is there matter and no antimatter?) [3]
1.1.1. The Ambition to Solve the Matter–Antimatter Asymmetry
- Baryon number violation—a change in the difference between the number of baryons and antibaryons at the particle level,
- Charge conjugation (C) and charge-parity (CP) violation—a different behaviour of matter and antimatter—and
- Thermal non-equilibrium (i.e., effective during a phase transition of the Universe)—to prevent a subsequent compensation of the asymmetry between matter and antimatter.
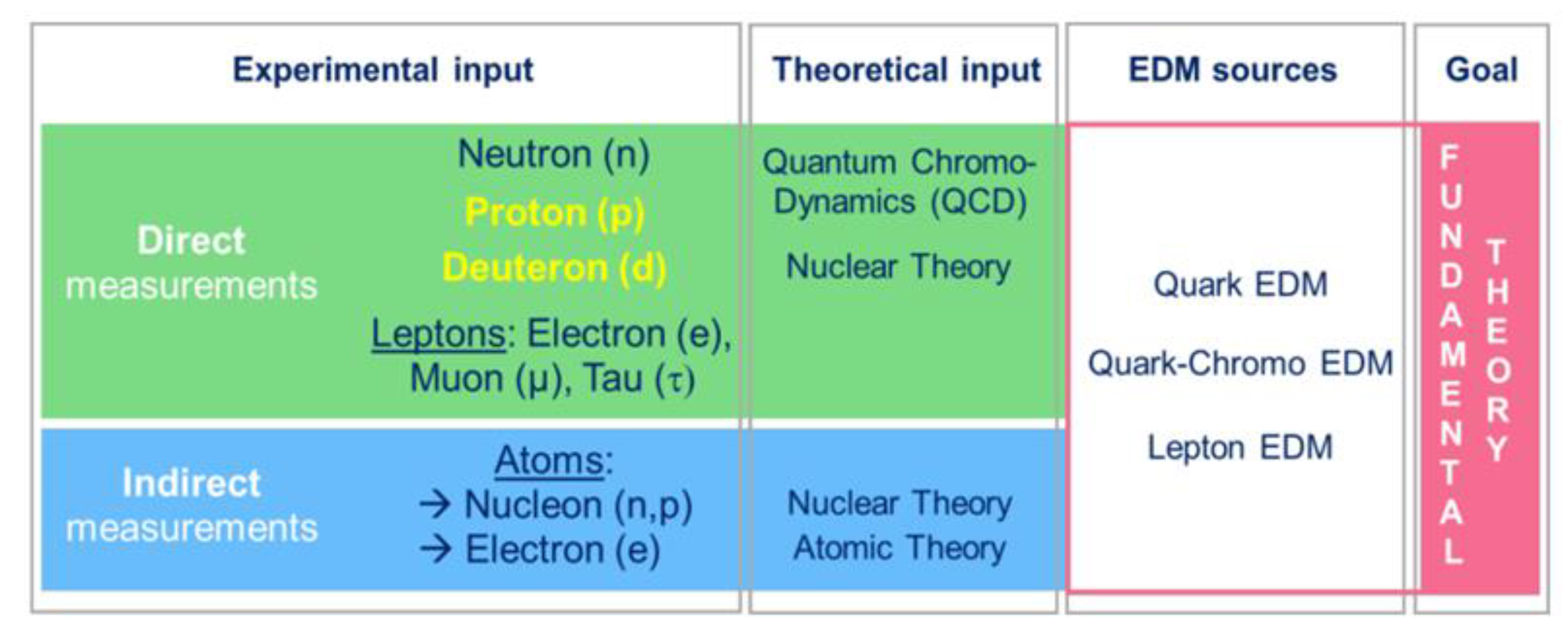
- Direct observation of the EDM effect of the system (proton, deuteron, and possibly other light ions such as 3He) under investigation, i.e., no need to extract particle EDM from measurement in a complex system;
- Virtually no limit to the number of particles used in the investigation, i.e., no limitation in the achievable statistical accuracy;
- There are theoretical relations between EDMs for protons and deuterons (see, e.g., [21]), which will serve as consistency checks for theory.
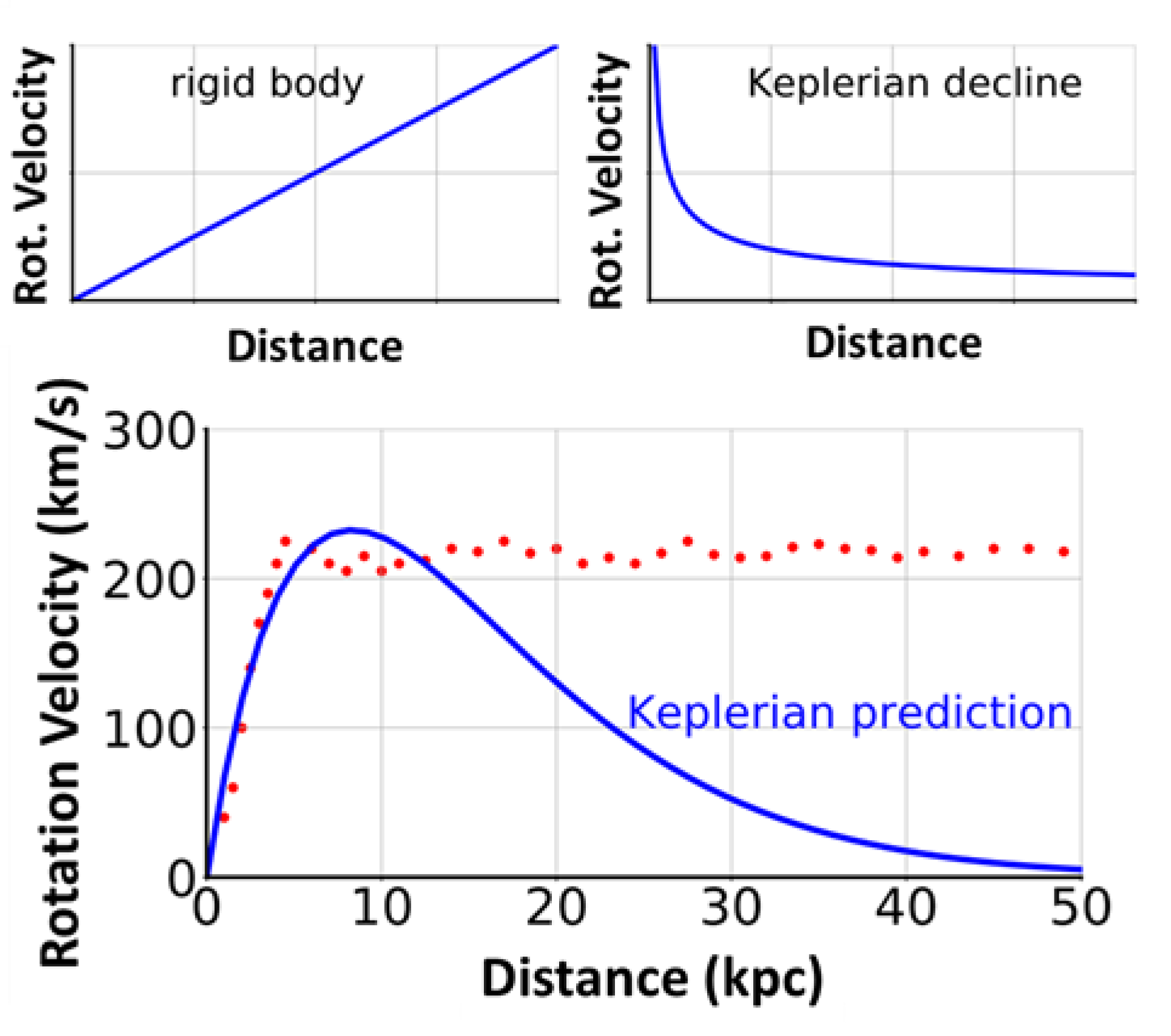
1.1.2. The Intent to Understand the Nature of Dark Matter
- Direct production of DM particles at accelerators;
- Detection of galactic DM particles through interactions with SM matter; and
- Detection of DM as coherently oscillating waves.
1.2. State-of-the-Art Knowledge
- 2.
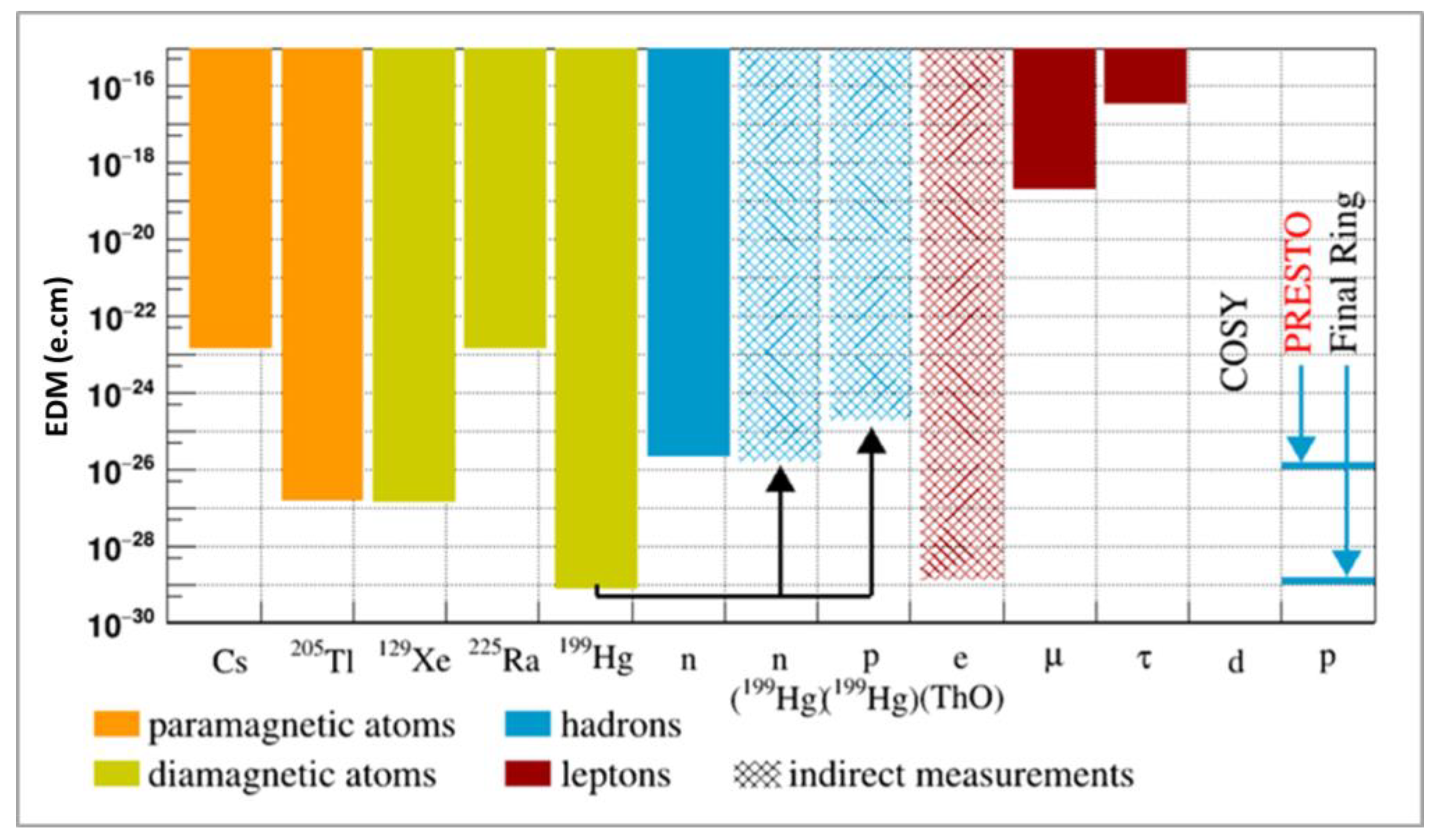
- EDM results from different systems will be needed to pin down the (set of) EDM sources.
2. Methodology of Charged-Particle EDM Searches
- Since the spin s of a particle is linked to a magnetic moment µ = (G + 1) (q/m) s, where G is the magnetic anomaly, q the charge, and m the mass, the particles will precess in the electric and/or magnetic field of the storage ring, which are necessary to keep them on a closed orbit. The total angular precession frequency of the spin is given by ds/dt = Ωs × s, where Ωs has components due to the magnetic and electric dipole moments of the particle.
- For any particle and energy, the electric and magnetic fields bending the beam can be chosen such that the angular rotation of the spin vector s from turn to turn vanishes, the spin is then said to “be frozen”, and, e.g., an initial longitudinal polarization of the particle beam is maintained.
- For particles with G > 0, such as protons, the above frozen-spin condition can be fulfilled with electric fields only by operating the ring at a specific energy, the so-called “magic” energy.
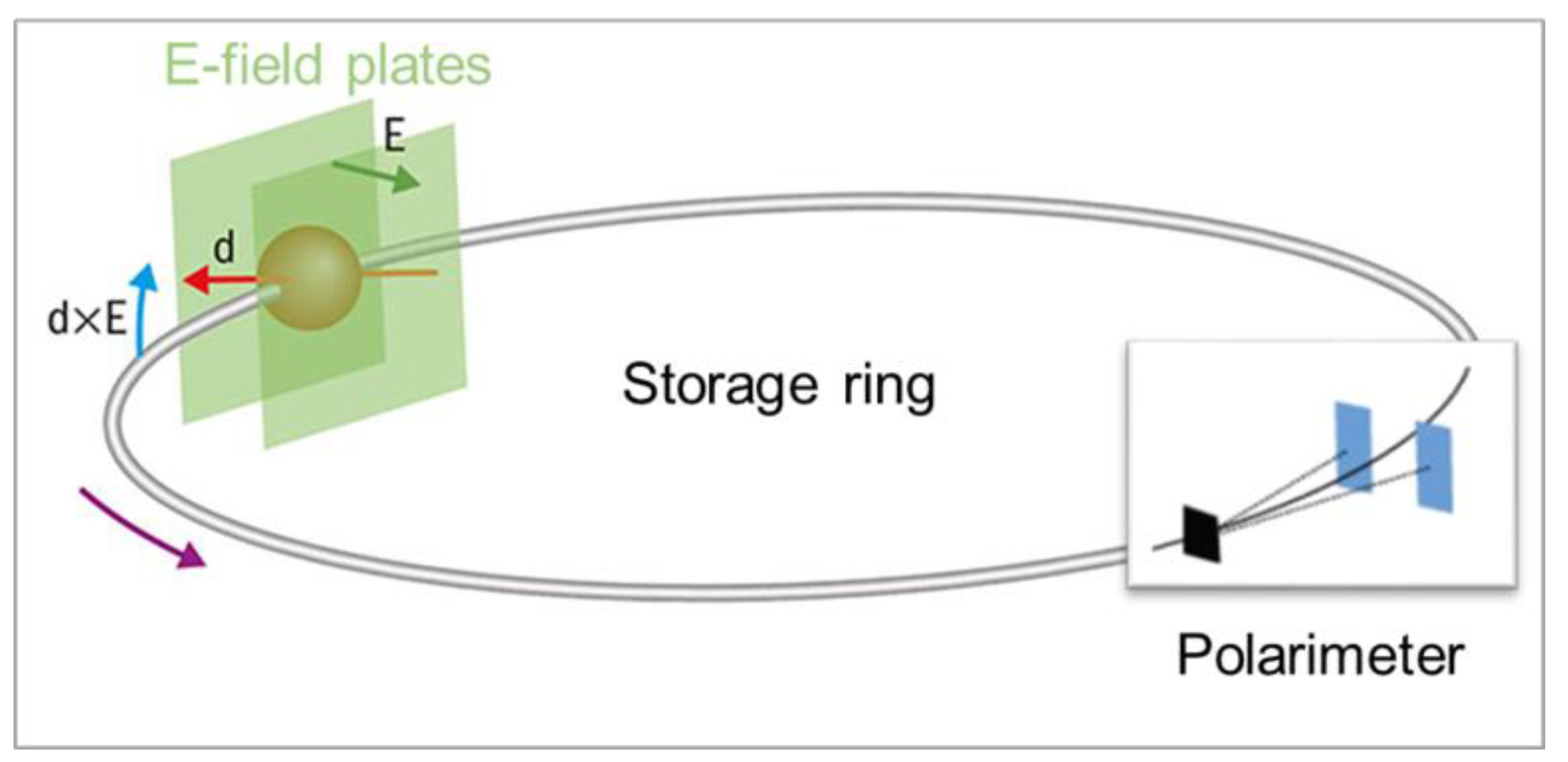
- The interaction between electric field and EDM will produce a torque d × E that rotates the spin out of the ring plane, as schematically shown in Figure 5.
- Inject a polarized charged particle beam with its spin vectors pointing in the direction of the momentum vector into a storage ring fulfilling the “frozen spin” condition. Thus, when the particles possess only a magnetic dipole moment (MDM), the beam will remain polarized in longitudinal direction.
- Let the particles interact with an electric field that couples to the possible EDM.
- Observe the rotation of the beam polarization out of the storage ring plane due to a non-zero EDM as a function of time. This can be observed with a dedicated detector system inside the ring, called a polarimeter.
- Switch off the external magnetic field: B = 0 (i.e., use an all-electric storage ring and shield all external magnetic fields);
- Arrange that bunches are polarized in the longitudinal direction during the duration of the measurement by choosing a “magic momentum”; this is possible for particles with a positive magnetic anomaly (G > 0), e.g., for the proton for a momentum of p = 700.7 MeV/c (corresponding to a kinetic energy of 232.8 MeV).
- Beam characteristics: storage time of the intensive beams, spin coherence time of the beam polarization and beam emittance; need of phase space cooling;
- Hardware: electric bends, storage ring vacuum; need of cryogenics, instrumentation for beam and spin manipulation, monitoring and control, polarimetry/polarimeter, injection scheme into the storage ring, and stability of power supplies;
- Other: alignment and metrology of ring elements, systematics investigations, and beam and spin tracking simulations.
3. Strategy towards Realization
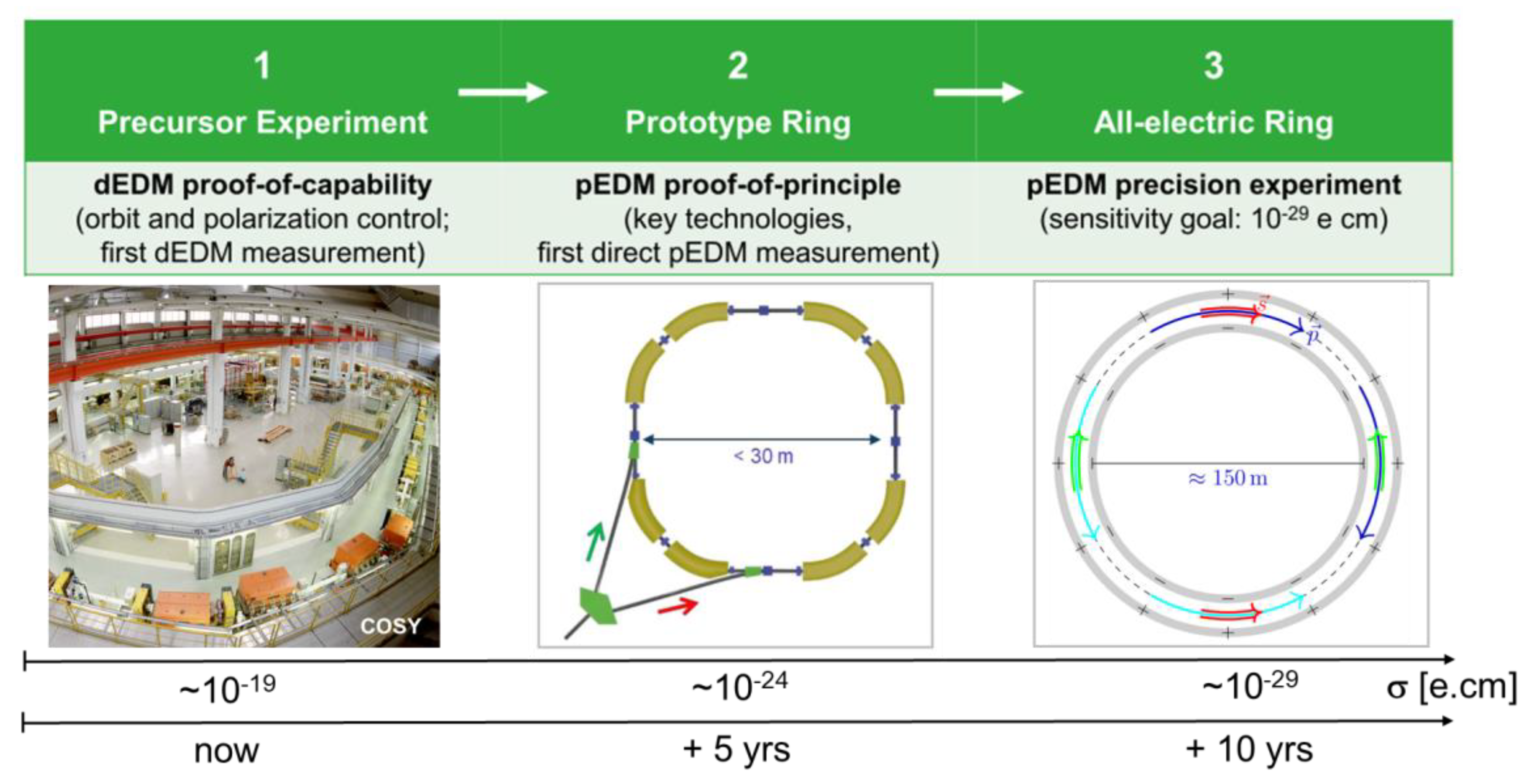
3.1. Stage 1: R&D and Measurements at COSY as “Proof-of-Capability”
- Development and implementation of techniques to preserve, manipulate, and observe the polarization of polarized beam bunches in a storage ring (optimization of the spin coherence time, use of a radio-frequency Wien filter, including the pilot-bunch method, design and set-up of an in-beam polarimeter), since they are essential prerequisites for a successful measurement of electric dipole moments of charged particles.
- A Rogowski beam-position monitor has been developed, optimized, installed into COSY, and used in the deuteron EDM (dEDM) measurements.
- A new polarimeter to determine the polarization direction of a circulating polarized beam, based on LYSO scintillators with a silicon photosensor readout, has been developed, optimized, installed into COSY, and used in the dEDM measurements [35].
- A radio-frequency Wien filter has been designed, built, and installed in COSY [36]. In a magnetic (B-field) storage ring such as COSY, the effect of a possible EDM on the particle spin (i.e., on the polarization of an ensemble of polarized particles) will exactly cancel, since the torque due to EDM changes sign—this is why the final EDM storage ring will be an all-electric ring with E-fields only. However, with the concept of such a Wien filter, it is possible in principle to observe the EDM effect (at reduced sensitivity). This device has been exploited for the so-called “precursor experiments” [37] with a polarized deuteron beam.
- In order to study the influence of the RF WF on the polarization (and possibly the orbit), two beam-bunches were injected into the COSY ring, and the RF WF was upgraded such that it could be switched on/off when one of the two bunches passes through the device. After successful tests, this so-called “pilot bunch” method was exploited in the second dEDM precursor experiment.
Scientific Results
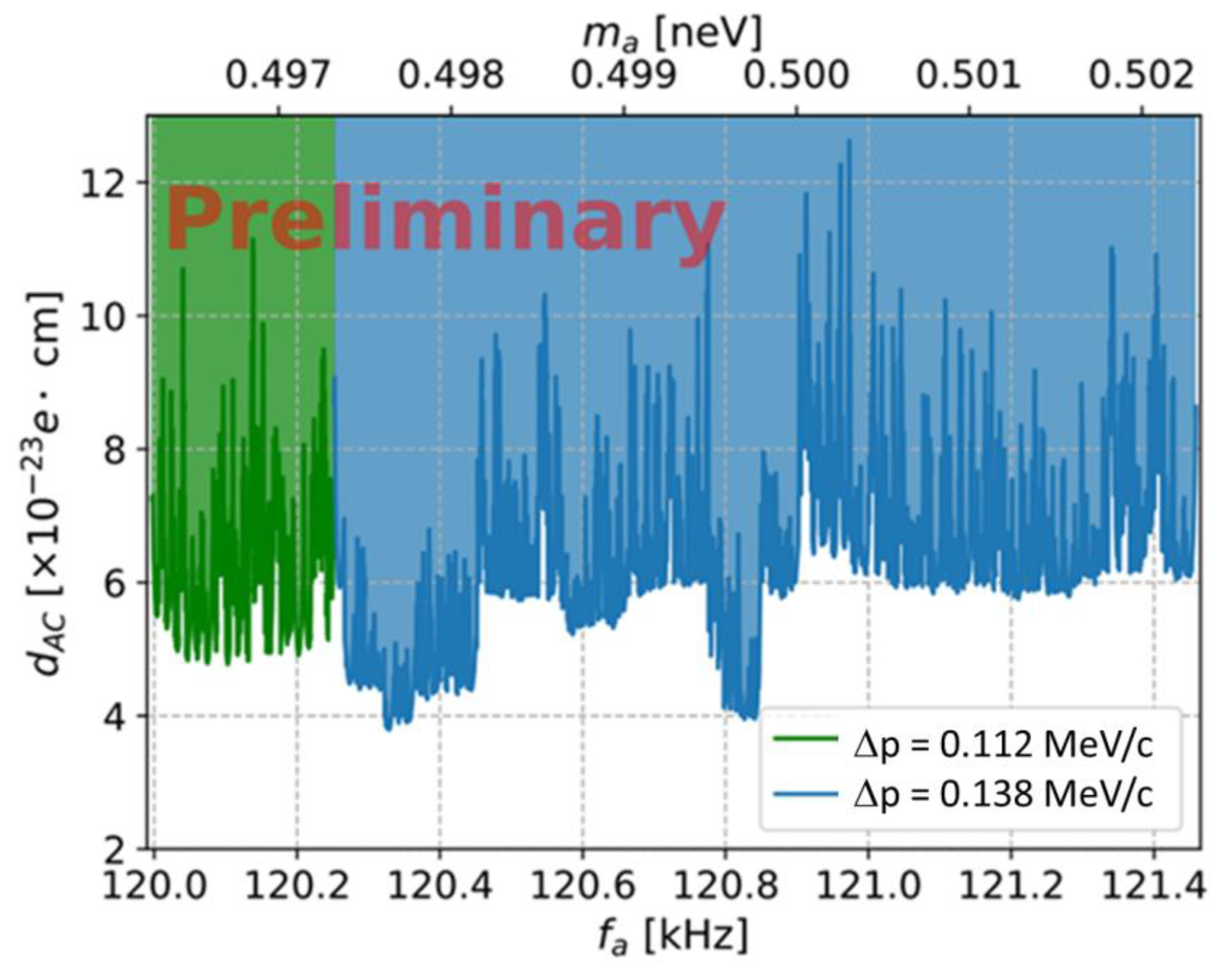
- A successful measurement of an oscillating electric dipole moment (limit) for a polarized deuteron beam has been performed in COSY—this was not anticipated originally, but it demonstrates that small changes of the beam polarization can be observed reliably [38]. A preliminary exclusion plot for the size of the oscillating EDM is given in Figure 7.
- A proof-of-capability of measuring a limit for a deuteron electric dipole moment in a magnetic storage ring (COSY) has also been achieved; the EDM result has not been finalized up to now due to very complex investigations required to quantify the systematic uncertainty.
3.2. Stage 2: A Prototype Storage Ring (PSR) for EDM Searches
- as an all-electric ring for CW/CCW operation, but not at the magic momentum; and
- in the “frozen spin” mode after complementing the ring with B-fields; this will allow to perform a first competitive proton (pEDM) experiment with a sensitivity similar to the neutron EDM, i.e., about 10−26 e·cm.
3.3. Stage 3: Design and Implementation of the Final Precision Storage Ring
4. Summary and Outlook
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Allen, R.E.; Lidström, S. Life, the Universe, and everything—42 fundamental questions. Phys. Scr. 2017, 92, 12501. [Google Scholar] [CrossRef]
- Alarcon, R.; Alexander, J.; Anastassopoulos, V.; Aoki, T.; Baartman, R.; Baeßler, S.; Bartoszek, L.; Beck, D.H.; Bedeschi, F.; Berger, R.; et al. Electric dipole moments and the search for new physics. arXiv 2022, arXiv:2203.08103. [Google Scholar] [CrossRef]
- Canetti, L.; Drewes, M.; Shaposhnikov, M. Matter and Antimatter in the Universe. New J. Phys. 2012, 14, 95012. [Google Scholar] [CrossRef]
- Chadha-Day, F.; Ellis, J.; Marsh, D.J. Axion dark matter: What is it and why now? arXiv 2021, arXiv:2105.01406v2. [Google Scholar] [CrossRef] [PubMed]
- Boveia, A.; Berkat, M.; Chen, T.Y.; Desai, A.; Doglioni, C.; Drlica-Wagner, A.; Gardner, S.; Gori, S.; Greaves, J.; Harding, P.; et al. Snowmass 2021 Dark Matter Complementarity Report. arXiv 2022, arXiv:2211.07027v2. 17. [Google Scholar] [CrossRef]
- Ramsey, N.F. Electric-dipole moments of elementary particles. Rep. Prog. Phys. 1982, 45, 95. [Google Scholar] [CrossRef]
- Leach, P.; Paliathanasis, A. (Eds.) Noether’s Theorem and Symmetry; MDPI: Basel, Switzerland, 2020; ISBN 978-3-03928-234-0/978-3-03928-235-7. [Google Scholar] [CrossRef]
- Blum, A.S.; de Velasco, A. The genesis of the CPT theorem. Eur. Phys. J. H 2022, 47, 5. [Google Scholar] [CrossRef]
- Roussy, T.S.; Caldwell, L.; Wright, T.; Cairncross, W.B.; Shagam, Y.; Ng, K.B.; Schlossberger, N.; Park, S.Y.; Wang, A.; Ye, J.; et al. A new bound on the electron’s electric dipole moment. arXiv 2022, arXiv:2212.11841v3. [Google Scholar] [CrossRef]
- Abel, C.; Afach, S.; Ayres, N.J.; Baker, C.A.; Ban, G.; Bison, G.; Bodek, K.; Bondar, V.; Burghoff, M.; Chanel, E.; et al. Measurement of the Permanent Electric Dipole Moment of the Neutron. Phys. Rev. Lett. 2020, 124, 81803. [Google Scholar] [CrossRef]
- Peccei, R.D. The Strong CP Problem and Axions; Lecture Notes in Physics; Springer: Berlin/Heidelberg, Germany, 2008; Volume 741, pp. 3–17. [Google Scholar] [CrossRef]
- Ringwald, A. Axions and Axion-Like Particles. arXiv 2014, arXiv:1407.0546v1. [Google Scholar] [CrossRef]
- Kim, O.; Semertzidis, Y.K. New method of probing an oscillating EDM induced by axionlike dark matter using an RF Wien Filter in storage rings. Phys. Rev. D 2021, 104, 96006. [Google Scholar] [CrossRef]
- Anastassopoulos, V.; Andrianov, S.; Baartman, R.; Baessler, S.; Bai, M.; Benante, J.; Berz, M.; Blaskiewicz, M.; Bowcock, T.; Brown, K.; et al. A storage ring experiment to detect a proton electric dipole moment. Rev. Sci. Instr. 2016, 87, 115116. [Google Scholar] [CrossRef] [PubMed]
- Sakharov, A.D. Violation of CP invariance, C asymmetry, and baryon asymmetry of the universe. Sov. Phys. Usp. 1991, 34, 392. [Google Scholar] [CrossRef]
- Mohanmurthy, P.; Winger, J.A. Estimation of CP violating EDMs from known mechanisms in the SM. arXiv 2020, arXiv:2009.00852v3. 66. [Google Scholar] [CrossRef]
- Fukuyama, T. Searching for New Physics beyond the Standard Model in Electric Dipole Moment. Int. J. Mod. Phys. A 2012, 27, 1230015. [Google Scholar] [CrossRef]
- Kirch, K.; Schmidt-Wellenburg, P. Search for electric dipole moments. EPJ Web Conf. 2020, 234, 1007. [Google Scholar] [CrossRef]
- Ema, Y.; Gao, T.; Pospelov, M. Improved indirect limits on muon EDM. arXiv 2021, arXiv:2108.05398. [Google Scholar]
- Bernreuther, W.; Chen, L.; Nachtmann, O. Electric dipole moment of the tau lepton revisited. Phys. Rev. D 2021, 103, 96011. [Google Scholar] [CrossRef]
- Abusaif, F.; Aggarwal, A.; Aksentev, A.; Alberdi-Esuain, B.; Andres, A.; Atanasov, A.; Barion, L.; Basile, S.; Berz, C.; Böhme, C.; et al. Storage Ring to Search for Electric Dipole Moments of Charged Particles: Feasibility Study; CERN Yellow Reports: Monographs; CERN: Meyrin, Switzerland, 2021; Volume 3. [Google Scholar] [CrossRef]
- Trimble, V. Existence and Nature of Dark Matter in the Universe. Ann. Rev. Astron. Astrophys. 1987, 25, 425–472. [Google Scholar] [CrossRef]
- Zwicky, F. Die Rotverschiebung von extragalaktischen Nebeln. Helv. Phys. Acta 1933, 6, 110–127. [Google Scholar]
- Johnson, J., Jr. Zwicky: The Outcast Genius Who Unmasked the Universe; Harvard University Press: Cambridge, MA, USA, 2019; ISBN 9780674979673. [Google Scholar]
- Bahcall, N.A. Dark matter universe. Proc. Natl. Acad. Sci. USA 2015, 112, 12243–12245. [Google Scholar] [CrossRef] [PubMed]
- Rajendran, S. New Directions in the Search for Dark Matter. arXiv 2022, arXiv:2204.03085v1.84. [Google Scholar] [CrossRef]
- Chupp, T.E.; Fierlinger, P.; Ramsey-Musolf, M.J.; Singh, J.T. Electric dipole moments of atoms, molecules, nuclei, and particles. Rev. Mod. Phys. 2019, 91, 15001. [Google Scholar] [CrossRef]
- Lee, S.Y. The Thomas–BMT equation. In Spin Dynamics and Snakes in Synchrotrons; World Scientific: Singapore, 1997; pp. 9–24. [Google Scholar]
- Fukuyama, T.; Silenko, A.J. Derivation of Generalized Thomas-Bargmann-Michel-Telegdi Equation for a Particle with Electric Dipole Moment. Int. J. Mod. Phys. A 2013, 28, 1350147. [Google Scholar] [CrossRef]
- Wilkin, C. The legacy of the experimental hadron physics programme at COSY. Eur. Phys. J. A 2017, 53, 114. [Google Scholar] [CrossRef]
- Annual Report 2018, Institut für Kernphysik COSY, Jül-4418, ISSN 0944-2952 (Forschungszentrum Jülich). pp. 15–21. Available online: https://www.fz-juelich.de/de/ikp/service/downloads (accessed on 25 February 2023).
- Annual Report 2019, Institut für Kernphysik COSY, Jül-4423, ISSN 0944-2952 (Forschungszentrum Jülich). pp. 8–13. Available online: https://www.fz-juelich.de/de/ikp/service/downloads (accessed on 25 February 2023).
- Annual Report 2020, Institut für Kernphysik COSY, Jül-4427, ISSN 0944-2952 (Forschungszentrum Jülich). pp. 7–13. Available online: https://www.fz-juelich.de/de/ikp/service/downloads (accessed on 25 February 2023).
- Annual Report 2021, Institut für Kernphysik COSY, Jül-4429, ISSN 0944-2952 (Forschungszentrum Jülich). pp. 6–11. Available online: https://www.fz-juelich.de/de/ikp/service/downloads (accessed on 25 February 2023).
- Müller, F.; Javakhishvili, O.; Shergelashvili, D.; Keshelashvili, I.; Mchedlishvili, D.; Abusaif, F.; Aggarwal, A.; Barion, L.; Basile, S.; Böker, J.; et al. A new beam polarimeter at COSY to search for electric dipole moments of charged particles. J. Instrum. 2020, 15, P12005. [Google Scholar] [CrossRef]
- Slim, J.; Nikolaev, N.N.; Rathmann, F.; Wirzba, A.; Nass, A.; Hejny, V.; Pretz, J.; Soltner, H.; Abusaif, F.; Aggarwal, A.; et al. (JEDI Collaboration), First detection of collective oscillations of a stored deuteron beam with an amplitude close to the quantum limit. Phys. Rev. Accel. Beams 2021, 24, 124601. [Google Scholar] [CrossRef]
- Lehrach, A.; Lorentz, B.; Morse, W.; Nikolaev, N.; Rathmann, F. Precursor Experiments to Search for Permanent Electric Dipole Moments (EDMs) of Protons and Deuterons at COSY. arXiv 2012, arXiv:1201.5773. [Google Scholar]
- Karanth, S.; Stephenson, E.J.; Chang, S.P.; Hejny, V.; Park, S.; Pretz, J.; Semertzidis, Y.; Wrońska, A.; Abusaif, F.; Aksentev, A.; et al. First Search for Axion-Like Particles in a Storage Ring Using a Polarized Deuteron Beam. arXiv 2022, arXiv:2208.07293v2. [Google Scholar] [CrossRef]
- Hacıömeroğlu, S.; Semertzidis, Y.K. Hybrid ring design in the storage-ring proton electric dipole moment experiment. Phys. Rev. Accel. Beams 2019, 22, 34001. [Google Scholar] [CrossRef]
- Omarov, Z.; Davoudiasl, H.; Hacıömeroğlu, S.; Lebedev, V.; Morse, W.M.; Semertzidis, Y.K.; Silenko, A.J.; Stephenson, E.J.; Suleiman, R. Comprehensive symmetric-hybrid ring design for a proton EDM experiment at below 10−29 e⋅cm. Phys. Rev. D 2022, 105, 32001. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ströher, H.; Schmidt, S.M.; Lenisa, P.; Pretz, J. Precision Storage Rings for Electric Dipole Moment Searches: A Tool En Route to Physics Beyond-the-Standard-Model. Particles 2023, 6, 385-398. https://doi.org/10.3390/particles6010020
Ströher H, Schmidt SM, Lenisa P, Pretz J. Precision Storage Rings for Electric Dipole Moment Searches: A Tool En Route to Physics Beyond-the-Standard-Model. Particles. 2023; 6(1):385-398. https://doi.org/10.3390/particles6010020
Chicago/Turabian StyleStröher, Hans, Sebastian M. Schmidt, Paolo Lenisa, and Jörg Pretz. 2023. "Precision Storage Rings for Electric Dipole Moment Searches: A Tool En Route to Physics Beyond-the-Standard-Model" Particles 6, no. 1: 385-398. https://doi.org/10.3390/particles6010020
APA StyleStröher, H., Schmidt, S. M., Lenisa, P., & Pretz, J. (2023). Precision Storage Rings for Electric Dipole Moment Searches: A Tool En Route to Physics Beyond-the-Standard-Model. Particles, 6(1), 385-398. https://doi.org/10.3390/particles6010020






