Charge Asymmetry of Muons Generated in a Muon Generator from Ultra-Dense Hydrogen D(0) and p(0)
Abstract
1. Introduction
2. Background
2.1. Theory of Ultra-Dense Hydrogen H(0)
2.2. Muon-Induced Fusion
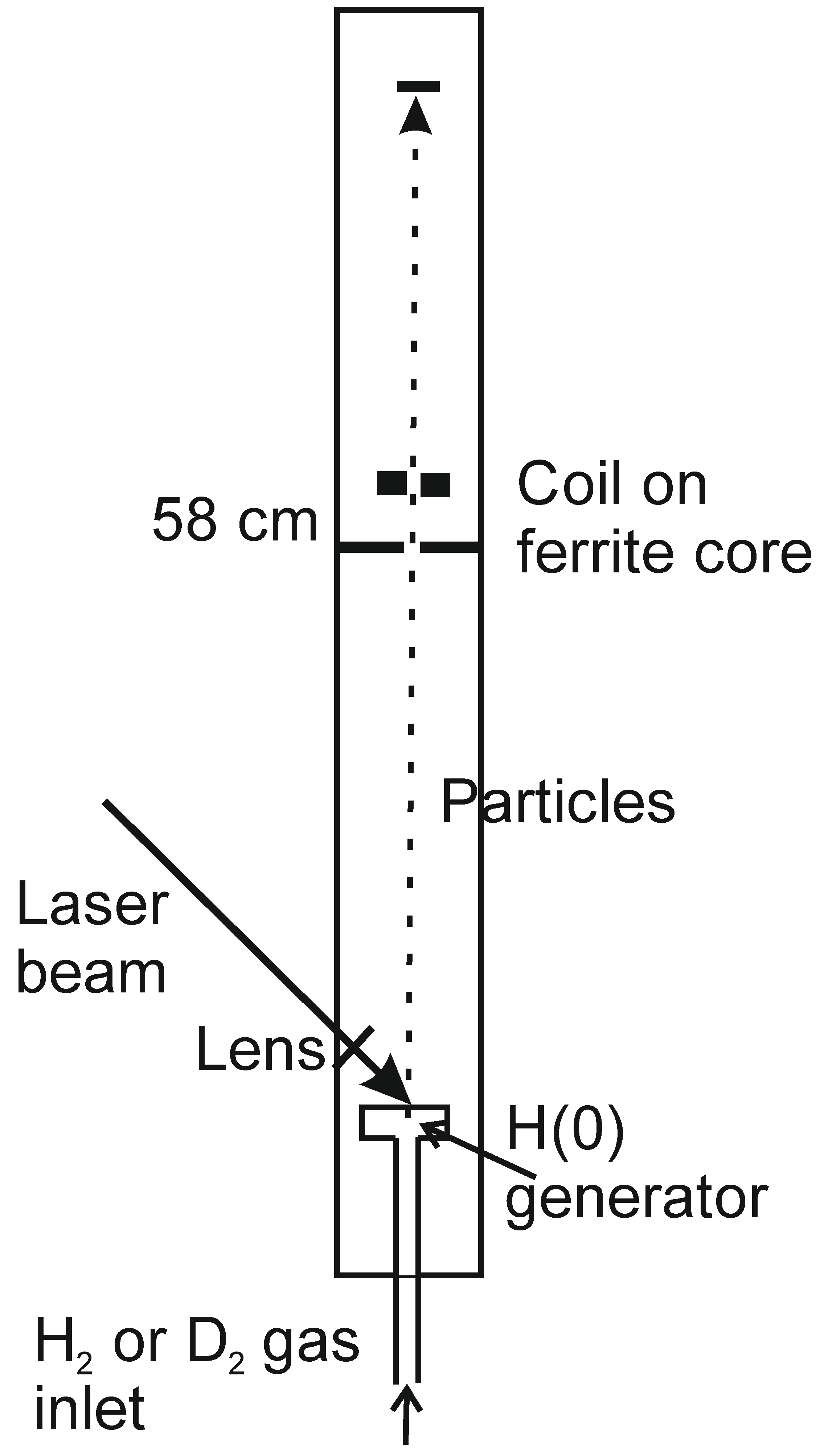
3. Experimental
4. The Nuclear Reaction Process
4.1. Kaon and Pion Decay
4.2. Formation and Detection of Muonium
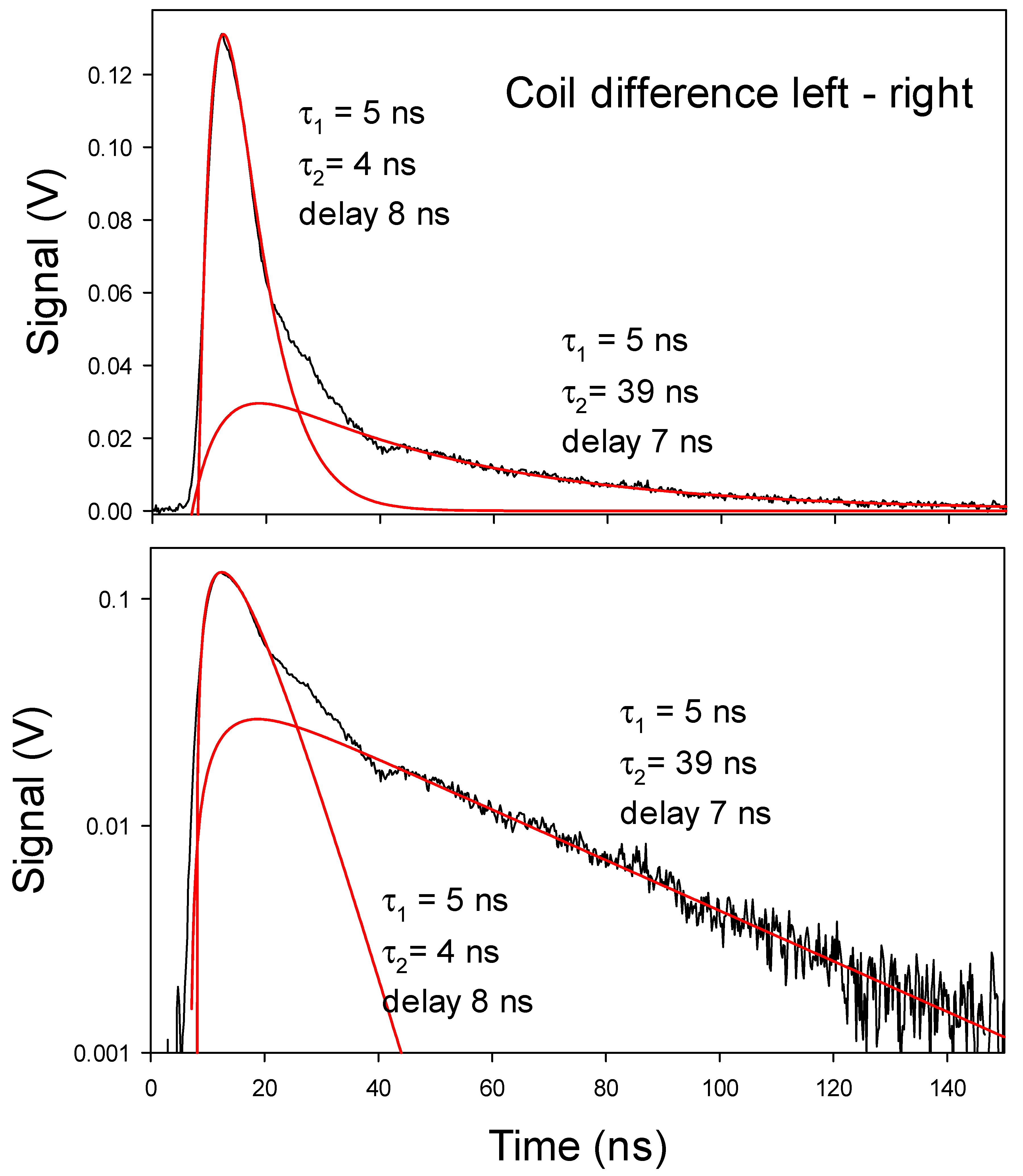
5. Discussion of Charge Asymmetry
6. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Holmlid, L.; Zeiner-Gundersen, S. Ultradense protium p(0) and deuterium D(0) and their relation to ordinary Rydberg matter: A review. Phys. Scr. 2019, 94, 075005. [Google Scholar] [CrossRef]
- Holmlid, L.; Kotarba, A.; Stelmachowski, P. Production of ultra-dense hydrogen H(0): A novel nuclear fuel. Int. J. Hydrogen Energy 2021, 46, 18466–18480. [Google Scholar] [CrossRef]
- Babaev, E.; Sudbø, A.; Ashcroft, N.W. A superconductor to superfluid phase transition in liquid metallic hydrogen. Nature 2004, 431, 666. [Google Scholar] [CrossRef] [PubMed]
- Andersson, P.U.; Holmlid, L. Superfluid ultra-dense deuterium D(-1) at room temperature. Phys. Lett. A 2011, 375, 1344–1347. [Google Scholar] [CrossRef]
- Andersson, P.U.; Holmlid, L.; Fuelling, S. Search for superconductivity in ultra-dense deuterium D(−1) at room temperature: Depletion of D(−1) at field strength >0.05 T. J. Supercond. Nov. Magn. 2012, 25, 873–882. [Google Scholar] [CrossRef]
- Holmlid, L.; Kotzias, B. Phase transition temperatures of 405-725 K in superfluid ultra-dense hydrogen clusters on metal surfaces. AIP Adv. 2016, 6, 45111. [Google Scholar] [CrossRef]
- Holmlid, L. Two-collector timing of 3-14 MeV/u particles from laser-induced processes in ultra-dense deuterium. Int. J. Mod. Phys. E 2013, 22, 1350089. [Google Scholar] [CrossRef]
- Olofson, F.; Holmlid, L. Electron-positron pair production observed from laser-induced processes in ultra-dense deuterium D(-1). Laser Part. Beams 2014, 32, 537–548. [Google Scholar] [CrossRef]
- Holmlid, L. Heat generation above break-even from laser-induced fusion in ultra-dense deuterium. AIP Adv. 2015, 5, 087129. [Google Scholar] [CrossRef]
- Holmlid, L. MeV particles in a decay chain process from laser-induced processes in ultra-dense deuterium D(0). Int. J. Mod. Phys. E 2015, 24, 1550026. [Google Scholar] [CrossRef]
- Holmlid, L. Nuclear particle decay in a multi-MeV beam ejected by pulsed-laser impact on ultra-dense hydrogen H(0). Int. J. Mod. Phys. E 2015, 24, 1550080. [Google Scholar] [CrossRef]
- Holmlid, L. Mesons from laser-induced processes in ultra-dense hydrogen H(0). PLoS ONE 2017, 12, e0169895. [Google Scholar] [CrossRef]
- Holmlid, L.; Olafsson, S. Decay of muons generated by laser-induced processes in ultra-dense hydrogen H(0). Heliyon 2019, 5, e01864. [Google Scholar] [CrossRef]
- Holmlid, L. Laser-induced nuclear processes in ultra-dense hydrogen take place in small non-superfluid HN(0) clusters. J. Clust. Sci. 2018, 30, 235–242. [Google Scholar] [CrossRef]
- Holmlid, L. Energy production by laser-induced annihilation in ultradense hydrogen H(0). Int. J. Hydrogen Energy 2021, 46, 14592–14595. [Google Scholar] [CrossRef]
- Nordling, C.; Österman, J. Physics Handbook; Studentlitteratur: Lund, Sweden, 1988. [Google Scholar]
- Burcham, W.E.; Jobes, M. Nuclear and Particle Physics; Pearson: Harlow, UK, 1995. [Google Scholar]
- Workman, R.L.; Burkert, V.D.; Crede, V.; Klempt, E.; Thoma, U.; Tiator, L.; Agashe, K.; Aielli, G.; Allanach, B.C.; Amsler, C.; et al. The review of particle physics 2022. Prog. Theor. Exp. Phys. 2022, 2022, 083C01. [Google Scholar] [CrossRef]
- Holmlid, L. Apparatus for Generating Muons with Intended Use in A Fusion Reactor. Swedish Patent nr. SE539684C2, 31 October 2017. [Google Scholar]
- Holmlid, L. Existing source for muon-catalyzed nuclear fusion can give MW thermal fusion generator. Fusion Sci. Technol. 2018, 75, 208–217. [Google Scholar] [CrossRef]
- Balin, D.V.; Ganzha, V.A.; Kozlov, S.M.; Maev, E.M.; Petrov, G.E.; Soroka, M.A.; Schapkin, G.N.; Semenchuk, G.G.; Trofimov, V.A.; Vasiliev, A.A.; et al. High precision study of muon catalyzed fusion in D2 and HD gas. Phys. Part. Nucl. 2011, 42, 185–214. [Google Scholar] [CrossRef]
- Holmlid, L. Muon-catalyzed fusion and annihilation energy generation will supersede non-sustainable T + D nuclear fusion. Energy Sustain. Soc. 2022, 12, 1–8. [Google Scholar] [CrossRef]
- Chatrchyan, S.; Khachatryan, V.; Sirunyan, A.M.; Tumasyan, A.; Adam, W.; Bergauer, T.; Dragicevic, M.; Erö, J.; Fabjan, C.; Friedl, M.; et al. Measurement of the muon charge asymmetry in pp → W + X production at √s = 7 TeV and an improved determination of light parton distribution functions. Phys. Rev. D 2014, 90, 032004. [Google Scholar] [CrossRef]
- Gorringe, T.; Hertzog, D. Precision muon physics. Prog. Part. Nucl. Phys. 2015, 84, 73–123. [Google Scholar] [CrossRef]
- Bogomilov, M.; Karadzhov, Y.; Kolev, D.; Russinov, I.; Tsenov, R.; Vankova-Kirilova, G.; Wang, L.; Xu, F.Y.; Zheng, S.X.; Bertoni, R.; et al. The MICE Muon Beam on ISIS and the beam-line instrumentation of the Muon Ionization Cooling Experiment. J. Instrum. 2012, 7, P05009–P05009. [Google Scholar] [CrossRef]
- Bogomilov, M.; Tsenov, R.; Vankova-Kirilova, G.; Song, Y.; Tang, J.; Li, Z.; Bertoni, R.; Bonesini, M.; Chignoli, F.; Mazza, R.; et al. Lattice design and expected performance of the Muon Ionization Cooling Experiment demonstration of ionization cooling. Phys. Rev. Accel. Beams 2017, 20, 063501. [Google Scholar] [CrossRef]
- Morenzoni, E.; Kottmann, F.; Maden, D.; Matthias, B.; Meyberg, M.; Prokscha, T.; Wutzke, T.; Zimmermann, U. Generation of very slow polarized positive muons. Phys. Rev. Lett. 1994, 72, 2793–2796. [Google Scholar] [CrossRef] [PubMed]
- Holmlid, L.; Olafsson, S. Spontaneous ejection of high-energy particles from ultra-dense deuterium D(0). Int. J. Hydrogen Energy 2015, 40, 10559–10567. [Google Scholar] [CrossRef]
- Holmlid, L.; Olafsson, S. Charged particle energy spectra from laser-induced processes: Nuclear fusion in ultra-dense deuterium D(0). Int. J. Hydrogen Energy 2016, 41, 1080–1088. [Google Scholar] [CrossRef]
- Alvarez, L.W.; Bradner, H.; Crawford, J.F.S.; Falk-Vairant, P.; Good, M.L.; Gow, J.D.; Rosenfeld, A.H.; Solmitz, F.; Stevenson, M.L.; Ticho, H.K.; et al. Catalysis of nuclear reactions by μMesons. Phys. Rev. 1957, 105, 1127–1128. [Google Scholar] [CrossRef]
- Jackson, J.D. Catalysis of nuclear reactions between hydrogen isotopes by μMesons. Phys. Rev. 1957, 106, 330–339. [Google Scholar] [CrossRef]
- Filipowicz, M.; Bystritsky, V.M.; Gerasimov, V.V.; Wozniak, J. Kinetics of muon catalyzed fusion processes in solid H/D mixture. Eur. Phys. J. D 2008, 47, 157–170. [Google Scholar] [CrossRef]
- Friar, J.L.; Gibson, B.F.; Jean, H.C.; Payne, G.L. Nuclear transition rates in μ-catalyzed P-D fusion. Phys. Rev. Lett. 1991, 66, 1827. [Google Scholar] [CrossRef]
- Kelly, R.S. Muon Catalyzed Fusion. An investigation of reactor design. Ph.D. Thesis, Department of Physics, Imperial College, London, UK, 2018. [Google Scholar]
- Holmlid, L. Controlling the process of muon formation for muon-catalyzed fusion: Method of non-destructive average muon sign detection. EPJ Tech. Instrum. 2021, 8, 1–14. [Google Scholar] [CrossRef]
- Blokland, W. Beam Current Monitors. 2009. Available online: https://uspas.fnal.gov/materials/09UNM/BeamCurrentMonitors.pdf.2009 (accessed on 3 May 2021).
- Holmlid, L.; Olafsson, S. Laser-induced annihilation: Relativistic particles from ultra-dense hydrogen H(0). High Energy Density Phys. 2021, 40, 100942. [Google Scholar] [CrossRef]
- Klempt, E.; Batty, C.; Richard, J.-M. The antinucleon–nucleon interaction at low energy: Annihilation dynamics. Phys. Rep. 2005, 413, 197–317. [Google Scholar] [CrossRef]
- Groom, D.E.; Mokhov, N.V.; Striganov, S.I. Muon stopping power and range tables 10 MeV–100 TeV. At. Data Nucl. Data Tables 2001, 78, 183–356. [Google Scholar] [CrossRef]
- Otani, M.; Fukao, Y.; Futatsukawa, K.; Kawamura, N.; Matoba, S.; Mibe, T.; Miyake, Y.; Shimomura, K.; Yamazaki, T.; Hasegawa, K.; et al. Negative muonium ion production with a C12A7 electride film. J. Phys. Conf. Ser. 2019, 1350, 012067. [Google Scholar] [CrossRef]
- Measday, D. The nuclear physics of muon capture. Phys. Rep. 2001, 354, 243–409. [Google Scholar] [CrossRef]
- Holmlid, L. Novel hydrogen nuclear fuels and their nuclear reactions. Energies 2022. submitted. [Google Scholar]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Holmlid, L. Charge Asymmetry of Muons Generated in a Muon Generator from Ultra-Dense Hydrogen D(0) and p(0). Particles 2023, 6, 188-197. https://doi.org/10.3390/particles6010010
Holmlid L. Charge Asymmetry of Muons Generated in a Muon Generator from Ultra-Dense Hydrogen D(0) and p(0). Particles. 2023; 6(1):188-197. https://doi.org/10.3390/particles6010010
Chicago/Turabian StyleHolmlid, Leif. 2023. "Charge Asymmetry of Muons Generated in a Muon Generator from Ultra-Dense Hydrogen D(0) and p(0)" Particles 6, no. 1: 188-197. https://doi.org/10.3390/particles6010010
APA StyleHolmlid, L. (2023). Charge Asymmetry of Muons Generated in a Muon Generator from Ultra-Dense Hydrogen D(0) and p(0). Particles, 6(1), 188-197. https://doi.org/10.3390/particles6010010





