CBM Performance for Λ Hyperon Directed Flow Measurements in Au + Au Collisions at 12A GeV/c

Abstract

We present the current status of the performance studies of $\mathsf{\Lambda }$ hyperon directed flow measurement with the CBM experiment at the future FAIR facility in Darmstadt. Kalman Filter mathematics is used to reconstruct $\mathsf{\Lambda }\to p{\pi }^{-}$ weak decay kinematics, while the Particle Finder Simple package is used to optimize criteria for $\mathsf{\Lambda }$ hyperon candidate selection. Directed flow of $\mathsf{\Lambda }$ hyperons is studied as a function of rapidity, transverse momentum and collision centrality. The effects on flow measurement due to non-uniformity of the CBM detector response in the azimuthal angle, transverse momentum and rapidity are corrected using the QnTools analysis framework.

1. Introduction

The main goal of the Compressed Baryonic Matter (CBM) experiment at the future Facility for Antiproton and Ion Research (FAIR) in Darmstadt is to study highly compressed baryonic matter produced by heavy-ion collisions. The SIS-100 accelerator will provide heavy-ion beams with momentum of $3.3–12\mathit{A}$ GeV/$\mathit{c}$ and an interaction rate of up to ${10}^7$ Hz. This will allow investigation of the QCD matter at temperatures up to approximately 120 MeV and net baryon densities 5–6 times the normal nuclear density. Experimental studies under these conditions are also important for understanding the properties of the neutron stars and evolution of the neutron star mergers [1].

Strange quarks are produced at the early stage of the collision of heavy ions. They provide information about the equation of state of the QCD matter in the high baryon density phase where the yield of strange quarks is expected to be comparable with that of light quarks [2,3]. According to hadronic transport models, (multi)-strange hyperons are produced in sequential collisions involving kaons and $\mathsf{\Lambda }$s and, therefore, are sensitive to the density of the hot matter created in the nuclei overlap. This sensitivity is largest at beam energies close to the production threshold in elementary collisions, and it is expected to shed light on the compressibility of nuclear matter [4,5].

The measurement of produced particles’ anisotropic flow is important for understanding the dynamics and evolution of the QCD matter created in the collision. The collective flow of strange hadrons is driven by the pressure gradients in the early dense phase of the collision evolution and, as such, allows the study of the dynamics of the strange quark production. In particular, an important aspect to observe is the directed flow, which is caused by the interaction of produced particles in the nuclei overlap region with the nuclei spectator fragments and is sensitive to the equation of state of created matter. Directed flow of strange hadrons ($\mathsf{\Lambda }$ and kaons) in the collision energy range of a few GeV was measured by experiments at RHIC [6,7], AGS [8,9] and SIS-18 [10] and is a part of the physics program of experiments at the future FAIR and NICA facilities. Due to the high interaction rate, the CBM experiment will allow multi-differential analysis of the anisotropic flow of rarely produced multi-strange particles such as $\mathsf{\Xi }$, $\mathsf{\Omega }$ and hypernuclei. The main goal is to develop and optimize the measurement procedure for multi-strange particle flow. In the current work, we validate the procedure with $\mathsf{\Lambda }$ hyperons as the most abundantly produced and we study possible biases due to the CBM reconstruction algorithms.

2. CBM Experiment and Simulation Setup

The CBM is a multipurpose fixed-target experiment which will be capable to measure the production of hadrons, electrons and muons in proton-ion and heavy-ion collisions over the full FAIR beam momentum range [5]. The main subsystems of the CBM experiment relevant to the measurement of the $\mathsf{\Lambda }$ hyperon flow are the Silicon Tracking System (STS) and Micro-Vertex Detector (MVD) for charged hadron tracking, the Time Of Flight (TOF) detector for charged particle identification and the Projectile Spectator Detector (PSD) for centrality and reaction plane estimation. The MVD and STS are located inside the dipole magnet with a magnetic field bending power of 1 Tm and allow the reconstruction of strange hyperon decay products with a momentum resolution of $\mathsf{\Delta }p/p\sim 1.5–2\%$ and decay vertex resolution of $\mathsf{\Delta }z\sim 50–100$m [11]. The z axis of the CBM coordinate system is directed along the beam line, while the magnetic field is directed along the y axis.

A sample of 5M Au + Au collisions at $p_{beam}$ = 12$\mathit{A}$ GeV/$\mathit{c}$ was generated with the DCM-QGSM-SMM model [12,13], which includes coalescence, fragmentation of nuclei recoil and hypernuclei production. In particular, modeling of fragmentation is important for centrality and reaction plane determination and flow studies [14]. The GEANT4 [15,16] package was used to transport generated particles through the CBM detector material. The resulting signals were used to reconstruct collision products using the CBMROOT [17] software.

Centrality was estimated using track multiplicity following the procedure described in [18] and implemented in [19]. For multiplicity calculation, the reconstructed tracks with vertex ${\chi }_{vertex}^2<3$, number of hits $N_{hits}\ge 4$, reduced track ${\chi }_{track}^2/NDF<3$ and pseudorapidity range $0.2<\eta <6$ were used.

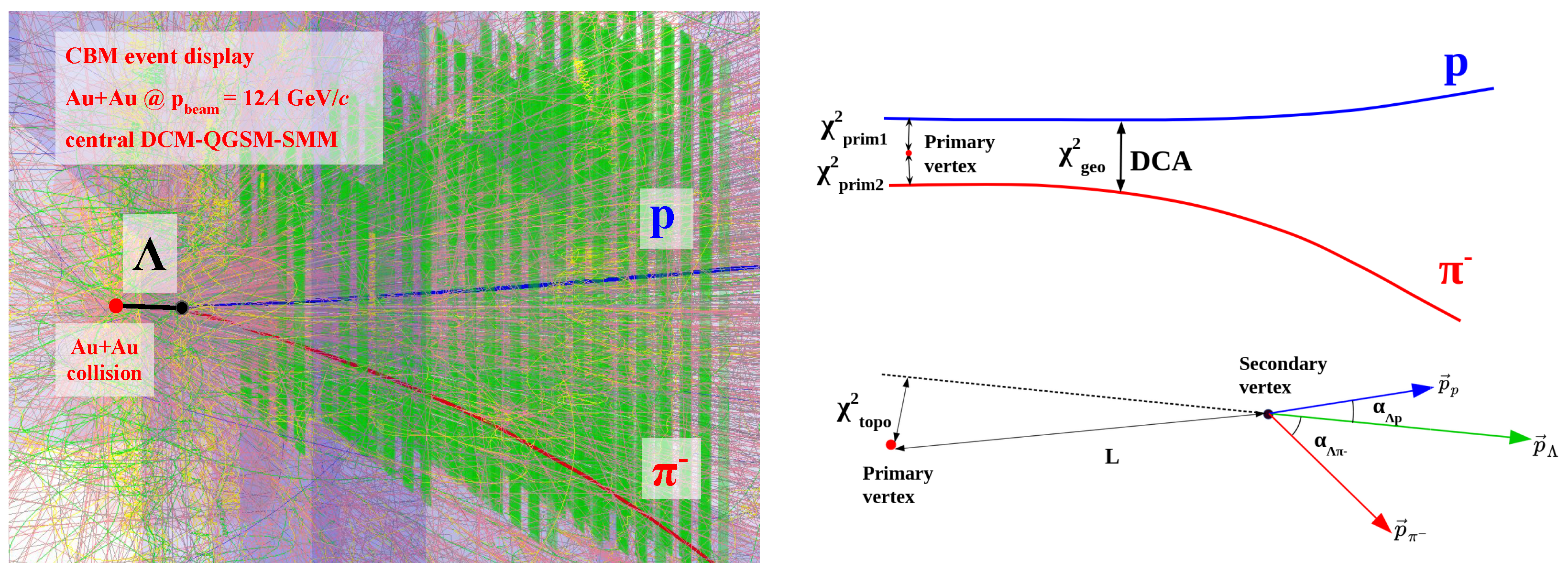
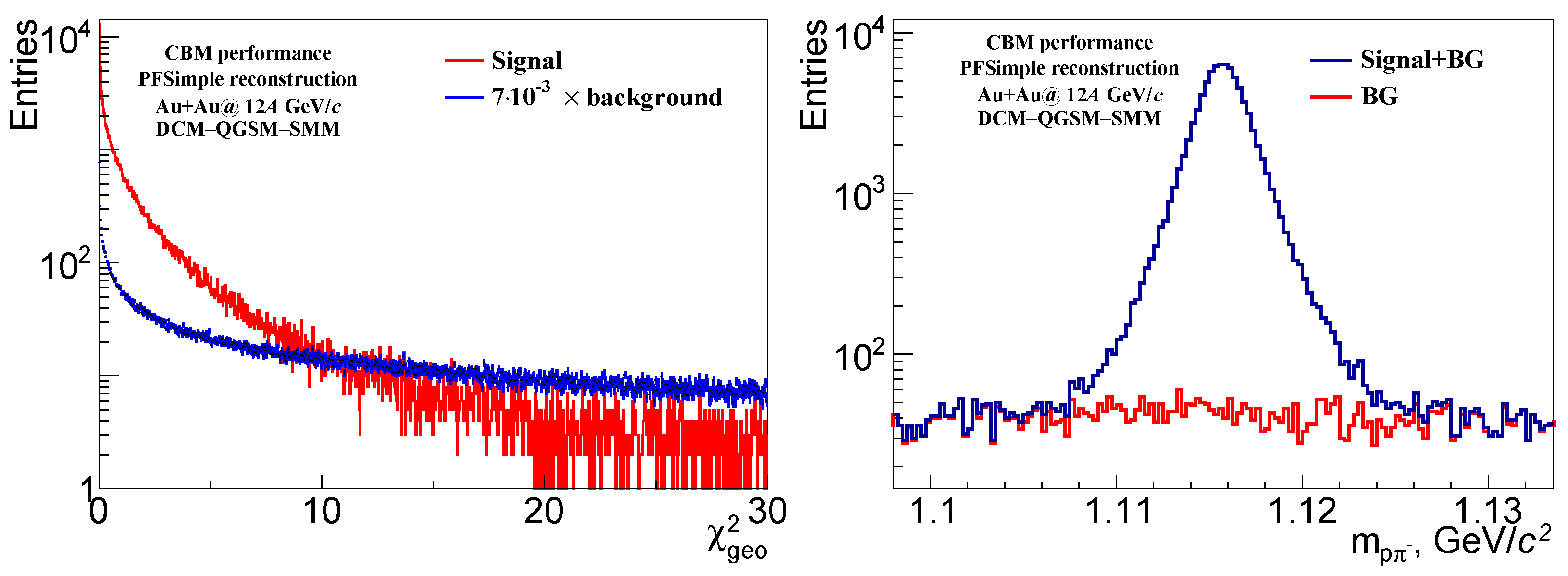
3. $\mathsf{\Lambda }$ Hyperon Reconstruction

The kinematics of the strange neutral hadrons, such as $\mathsf{\Lambda }$ hyperons, can only be reconstructed in CBM via their weak decay products. Figure 1 (left) shows the CBM event display as an illustration of the multi-particle environment, which complicates the hyperon decay reconstruction. The event display is shown for one of the central (impact parameter $b=0.3fm$) Au + Au collisions at beam momentum 12$\mathit{A}$ GeV/$\mathit{c}$ generated with the DCM-QGSM-SMM event generator. The red (blue) line represents the trajectory of the daughter pion (proton) originating from the $\mathsf{\Lambda }$ weak decay. The red dot indicates the vertex of the the Au + Au collision and the black dot represents the decay vertex, which are called the primary and secondary vertex, respectively. The black line represents the straight line trajectory of the $\mathsf{\Lambda }$ hyperon before decay.

The following information is used for the $\mathsf{\Lambda }$ decay reconstruction: the primary vertex position; the reconstructed parameters of the daughter tracks, such as charge, momentum and covariance matrix calculated at the first hit position of the daughter track; and the particle type hypothesis. To construct the $\mathsf{\Lambda }$ candidates, each reconstructed negatively charged track (${\pi }^{-}$ candidate) is combined with each positively charged track (proton candidate), with particle type used as track preselection. For the presented analysis, the particle types were taken from matching the reconstructed tracks with true particle trajectories in GEANT4 simulations.

Figure 1 (right) illustrates the parameters which determine the decay topology and are used for the $\mathsf{\Lambda }$ candidate selection optimization. The parameters are:

• $DCA$—a distance of the closest approach between two daughter tracks (in cm);
• $cos{\alpha }_{\mathsf{\Lambda }p}$—a cosine of the angle between the $\mathsf{\Lambda }$ candidate and proton momenta;
• $L/\mathsf{\Delta }L$ —a distance (L) between the primary and secondary vertices divided by its error ($\mathsf{\Delta }L$);
• ${\chi }_{prim,p}^2\left({\chi }_{prim,{\pi }^{-}}^2\right)$—a square of the distance between the proton (negatively charge pion) track and the primary vertex position divided by its error;
• ${\chi }_{geo}^2$—a square of the distance between the daughter proton and negatively charged pion divided by its error;
• ${\chi }_{topo}^2$—a square of the distance between the $\mathsf{\Lambda }$ candidate trajectory and primary vertex divided by its error.

The ${\chi }_{prim,p}^2$, ${\chi }_{prim,{\pi }^{-}}^2$, ${\chi }_{geo}^2$ and ${\chi }_{topo}^2$ are calculated as the matrix convolution of the respective distance $\mathsf{\Delta }\mathbf{r}$ with elements of the corresponding inverted covariance matrix $C_{ij}^{-1}$:

$${\chi }^2=\left(C^{-1}\mathsf{\Delta }\mathbf{r}\right)\mathsf{\Delta }\mathbf{r}=C_{ij}^{-1}\mathsf{\Delta }{r}_i\mathsf{\Delta }{r}_j.$$

(1)

The Kalman Filter implementation of the decay parameter calculation is described in [20] and the corresponding mathematics is implemented in the Kalman Filter Particle (KFParticle) package [21,22]. The KFParticle mathematics is interfaced via the Particle Finder Simple (PFSimple) code [23] for optimized and efficient $\mathsf{\Lambda }$ hyperon reconstruction and background rejection. Numerical values of the $\mathsf{\Lambda }$ candidate selection criteria are listed in

Table 1. Numerical values of the $\mathsf{\Lambda }$ candidate selection criteria.

Parameter	${\mathit{\chi }}_{\mathit{p}\mathit{r}\mathit{i}\mathit{m}\mathit{,}\mathit{p}}^2$	${\mathit{\chi }}_{\mathit{p}\mathit{r}\mathit{i}\mathit{m}\mathit{,}{\mathit{\pi }}^{\mathit{-}}}^2$	DCA (cm)	$\mathit{L}\mathbf{/}\mathsf{\Delta }\mathit{L}$	${\mathit{\chi }}_{\mathit{g}\mathit{e}\mathit{o}}^2$	$\mathbf{cos}{\mathit{\alpha }}_{\mathbf{\Lambda }\mathit{p}}$	${\mathit{\chi }}_{\mathit{t}\mathit{o}\mathit{p}\mathit{o}}^2$
Selection criteria	>26	>110	<0.15	>4	<11	>0.99825	<29

.

Figure 2 (left) shows as an example the distribution of the ${\chi }_{geo}^2$ for the $\mathsf{\Lambda }$ candidates constructed from daughters of the true $\mathsf{\Lambda }$ decay (signal) and combinatorial background normalized to the integral of the signal.

Figure 2 (right) and Figure 3 illustrate the performance of PFSimple code. The signal to background ratio calculated within five standard deviations from the invariant mass peak is around 30 and the reconstruction efficiency in the midrapidity region is $\epsilon \approx 70\%$ for the decay channel $\mathsf{\Lambda }\to p{\pi }^{-}$.

4. Anisotropic Flow Measurement Technique

Anisotropic flow is characterized by coefficients in a Fourier decomposition of the produced particles’ azimuthal distribution $\rho \left(\phi \right)$ relative to the reaction (symmetry) plane:

$$\rho (\phi -{\mathsf{\Psi }}_{RP})\sim 1+2\sum _{n=1}^{\infty }{v}_{n}cos\left(n(\phi -{\mathsf{\Psi }}_{RP})\right),$$

(2)

where n is the harmonic number, $\phi$ is the azimuthal angle of the particle and ${\mathsf{\Psi }}_{RP}$ is the reaction (symmetry) plane angle. The flow coefficients $v_n$ can be calculated as follows:

$$v_n=\langle cos\left(n(\phi -{\mathsf{\Psi }}_{RP})\right)\rangle .$$

(3)

The first harmonic coefficient $v_1$ represents the directed flow.

The reaction plane angle in Equation (3) is not known. A data-driven procedure for the reaction plane estimation and corresponding resolution correction extraction using the spectators’ energy registered with the PSD [24] is discussed in [14]. The focus of the current work is on the CBM performance for $\mathsf{\Lambda }$ hyperon directed flow measurements, and we used the reaction plane angle ${\mathsf{\Psi }}_{RP}$ from the event generator, assuming that it can be reconstructed reliably with the PSD. Signal and background $\mathsf{\Lambda }$ candidates reconstructed with PFSimple were separated by matching their daughters to the true particle trajectories in GEANT4 simulations.

Non-uniformity of the $\mathsf{\Lambda }$ reconstruction in the azimuthal angle ${\phi }_{\mathsf{\Lambda }}$, transverse momentum $p_{T,\mathsf{\Lambda }}$ and rapidity $y_{\mathsf{\Lambda }}$ may bias the measurement of the directed flow. To study detector biases in the $\mathsf{\Lambda }$ directed flow measurement, we use two independent estimates from projections in the x and y direction of the laboratory frame:

$$v_{1x}=2\langle cos{\phi }_{\mathsf{\Lambda }}cos{\mathsf{\Psi }}_{RP}\rangle ,v_{1y}=2\langle sin{\phi }_{\mathsf{\Lambda }}sin{\mathsf{\Psi }}_{RP}\rangle .$$

(4)

Effects of the azimuthal non-uniformity can be corrected with the procedure described in [25]. For this, a ${\mathbf{q}}_1=(cos{\phi }_{\mathsf{\Lambda }},sin{\phi }_{\mathsf{\Lambda }})$ vector is introduced, which is corrected via three main consecutive steps:

• Recenter the ${\mathbf{q}}_1$ distribution by subtracting the corresponding average values;
• Twist the ${\mathbf{q}}_1$ vector distribution;
• Rescale the ${\mathbf{q}}_1$ vector distribution along x and y directions.

Figure 4 illustrates the transformation of the ${\mathbf{q}}_1$ vector distribution after different correction steps.

The correction procedure is implemented in the QnTools [26] and QnAnalysis [27] software packages.

Figure 3 illustrates the asymmetric CBM detector response in $p_{T,\mathsf{\Lambda }}-y_{\mathsf{\Lambda }}$, $p_{T,\mathsf{\Lambda }}-{\phi }_{\mathsf{\Lambda }}$ and $y_{\mathsf{\Lambda }}-{\phi }_{\mathsf{\Lambda }}$, which provides input for the QnTools package. Non-uniformity of the $\mathsf{\Lambda }$ reconstruction in $p_{T,\mathsf{\Lambda }}$ and $y_{\mathsf{\Lambda }}$ is taken into account by weighting the $\mathsf{\Lambda }$ candidates in the $v_1$ calculation with the inverse value of efficiency for a given $p_{T,\mathsf{\Lambda }}$ and $y_{\mathsf{\Lambda }}$ region.

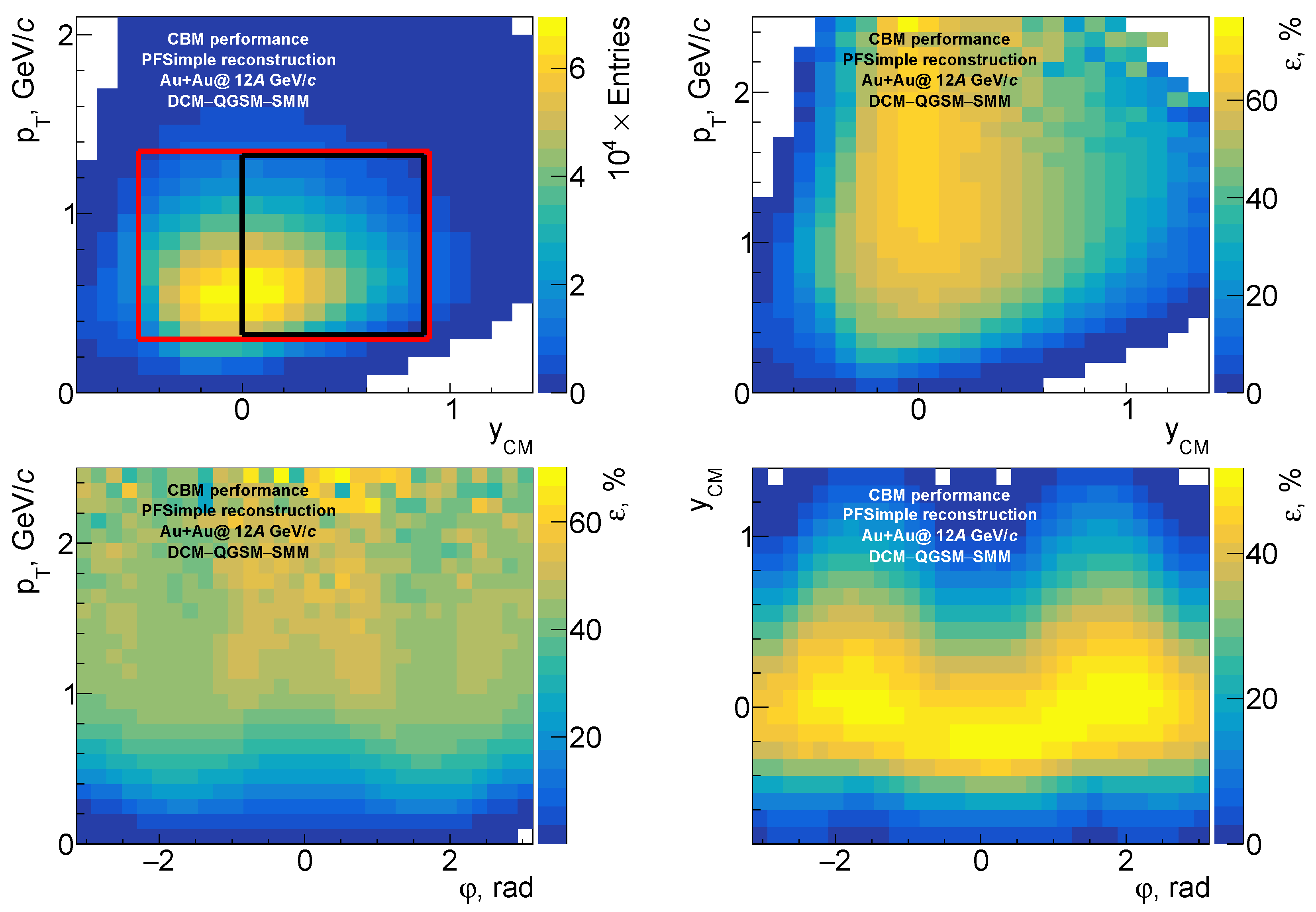
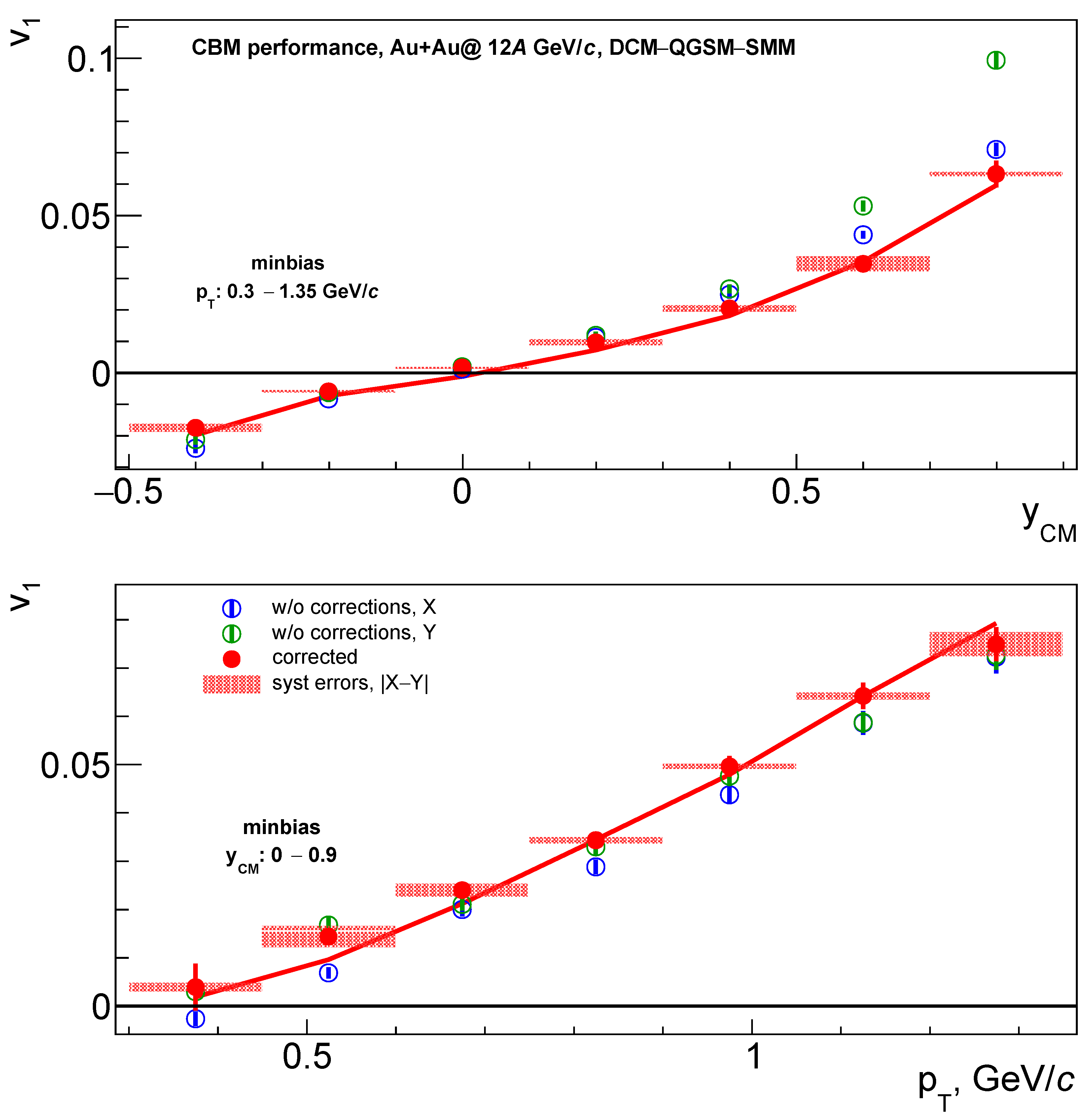
5. Results

Figure 5 shows the CBM performance for the $\mathsf{\Lambda }$ hyperon directed flow measurement. $v_1$ is presented as a function of $\mathsf{\Lambda }$ hyperon’s rapidity, transverse momentum and centrality. The integration region over the kinematical phase space is indicated in Figure 3 (top left) by red (black) boxes for the calculation of $v_1$ vs. y ($p_T$ and centrality). Blue and green open circles in Figure 5 show, respectively, the uncorrected $v_{1,x}$ and $v_{1,y}$ defined by Equation (4). Red full circles show an average value of $v_{1,x}$ and $v_{1,y}$ after applying both azimuthal non-uniformity corrections and ($p_T-y$)-dependent efficiency weights. The red solid line shows the Monte Carlo true value of the $\mathsf{\Lambda }$ directed flow. The lighter red-shaded areas show the systematical uncertainty estimated from the remaining difference between $v_{1x}$ and $v_{1y}$ after all corrections are applied.

A positive slope of $\mathsf{\Lambda }$$v_1\left(y\right)$ is reproduced, with $v_1$ being consistent within the statistical precision with the Monte Carlo input values (see Figure 5, top). The $p_T$ dependence of $v_1$ is shown in the middle panel of Figure 5. There is a small discrepancy between evaluated and true values of $v_1$ at small $p_T\sim 0.5$ GeV/c, which requires further investigation with higher statistics. Figure 5 (bottom) illustrates $v_1$ dependence on centrality. The sign change of $v_1$ with centrality around $50\%$ is reproduced. Peripheral collisions with centrality >70% are not considered in this analysis because of the very poor statistics of the produced $\mathsf{\Lambda }$ hyperons.

6. Conclusions

In summary, the performance of the CBM experiment for the measurements of the directed flow of $\mathsf{\Lambda }$ hyperons is presented. After applying corrections for azimuthal non-uniformity and $p_T$ and rapidity-dependent efficiency weights, the Monte Carlo input is reproduced within the statistical precision. The future plans are to use the data-driven procedure for the reaction plane estimation with the PSD, deploy the invariant mass fit method for signal–background separation in the hyperon reconstruction and perform multi-differential $p_T$, y and centrality analysis.