Chirally Improved Quark Pauli Blocking in Nuclear Matter and Applications to Quark Deconfinement in Neutron Stars
Abstract
1. Introduction
2. Quark Exchange in Nuclear Matter
2.1. Quark Substructure Effect on the Self-Energy of the Nucleons
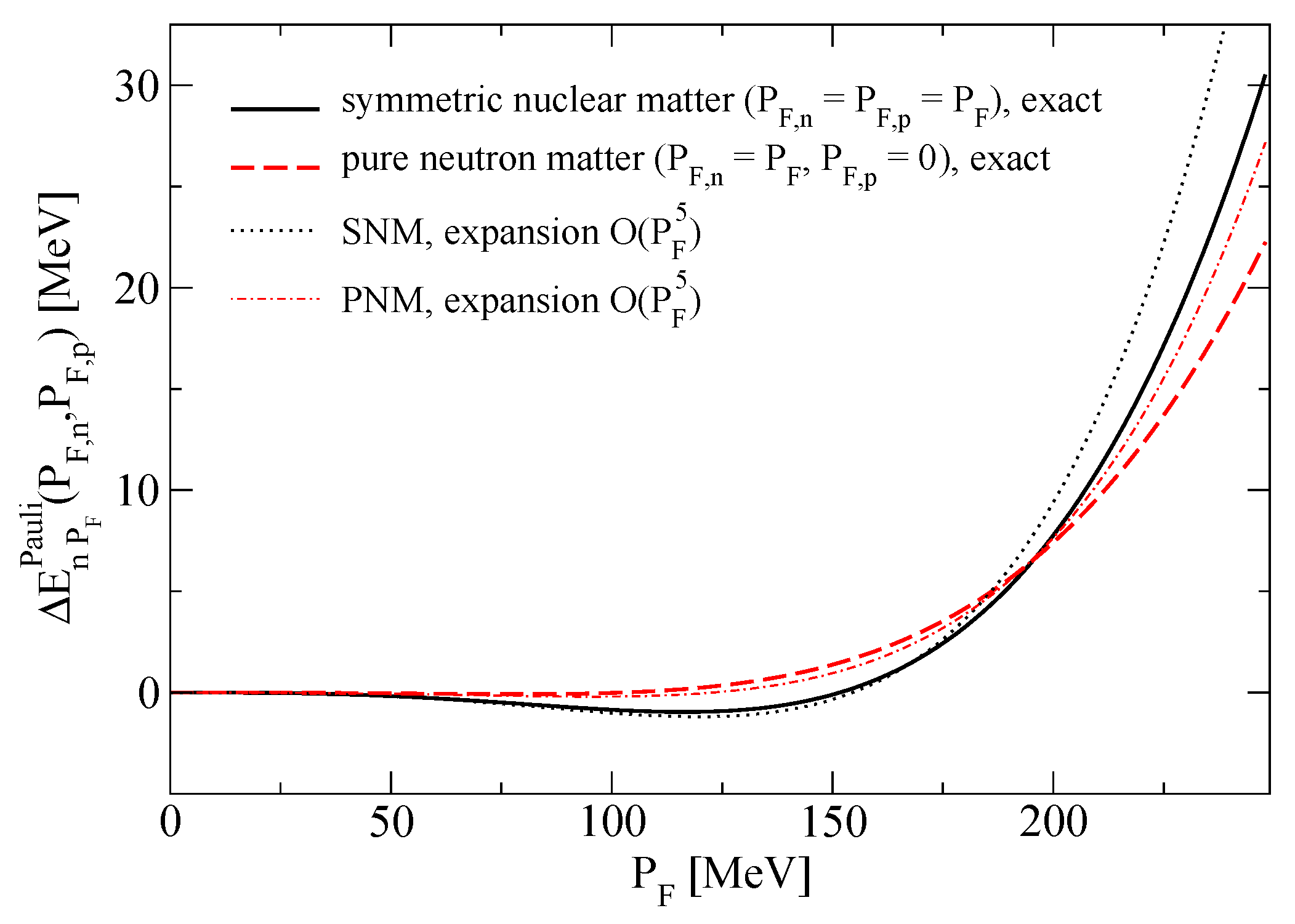
- Symmetric nuclear matter (SNM), for which andThe Pauli shift for protons is obtained using the symmetry relation that is encoded in the coefficients of Table 1. With these coefficients and the low-density expansion (A29) up to fifth order in the Fermi momentum, we obtainThis energy shift can be identified with a shift in the chemical potential and thus be used to derive a contribution to the equation of state, see [7].In order to give numerical results for the Pauli shift (4), we adopt the values MeV and fm according to [20], which reproduce quite well the single nucleon properties. With the relation , the Pauli blocking shift can be given as a function of the nuclear matter densitywith MeV fm3 and MeV fm5. As has been discussed already in [7], this density dependent energy shift is in good agreement with the repulsive part of the Skyrme Hartree–Fock shift in nuclear matter obtained by Vautherin and Brink [8].
- Pure neutron matter (PNM), for which , andInserting the coefficients from Table 1 and the low-density expansion (A29) up to fifth order in the Fermi momentum, we obtainInserting the relation between Fermi momentum and density for PNM, we obtain the energy shift in the form (5) with the coefficients MeV fm3 and MeV fm5.
2.2. Chiral Improvement of the Quark Pauli Blocking
3. Equation of State of Cold, Dense Matter with Deconfinement Transition
3.1. Relativistic Mean Field Model with Quark Exchange Contribution
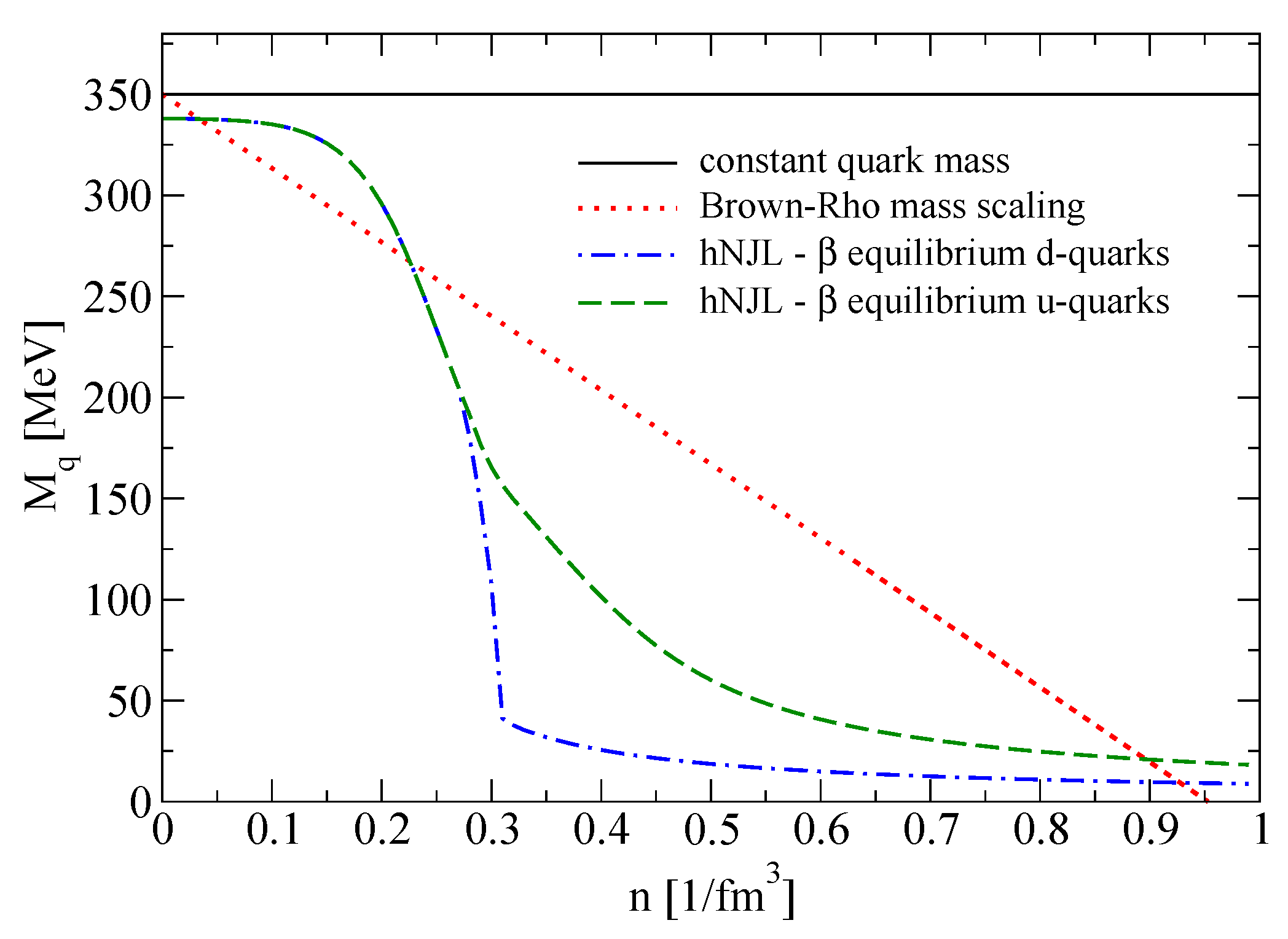
3.2. NJL Model with Higher Order Quark Interactions
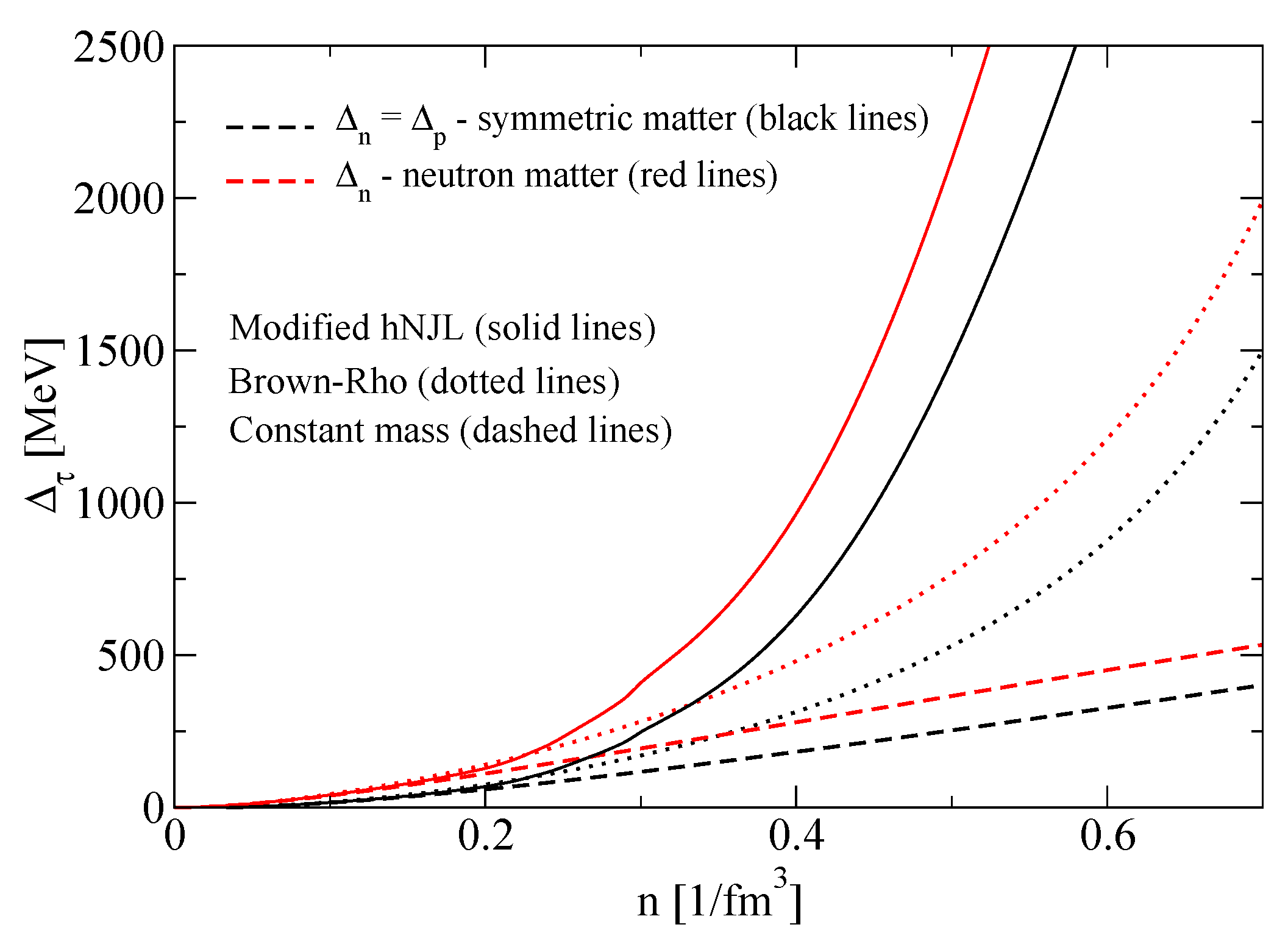
3.3. Quark Deconfinement Phase Transition
4. Results
4.1. Parameterization of the Model
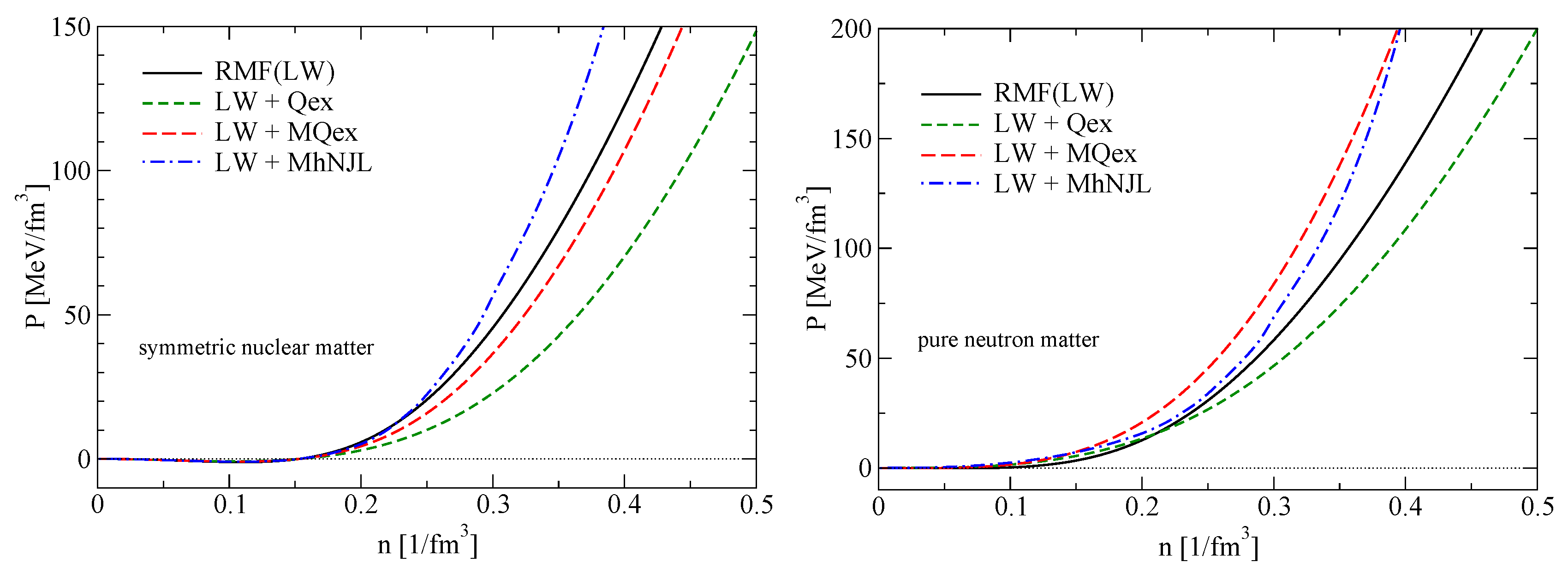
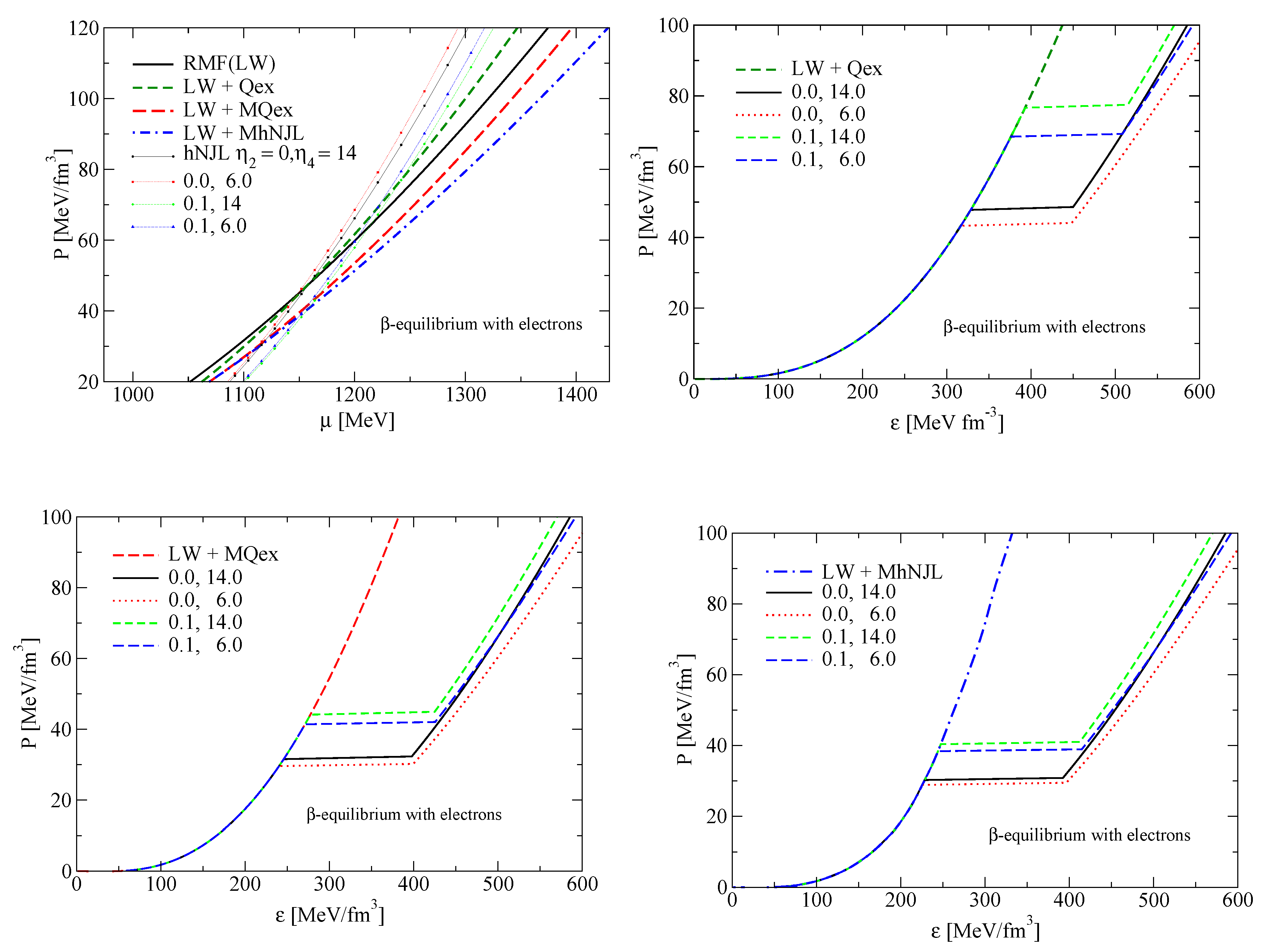
4.2. Equation of State
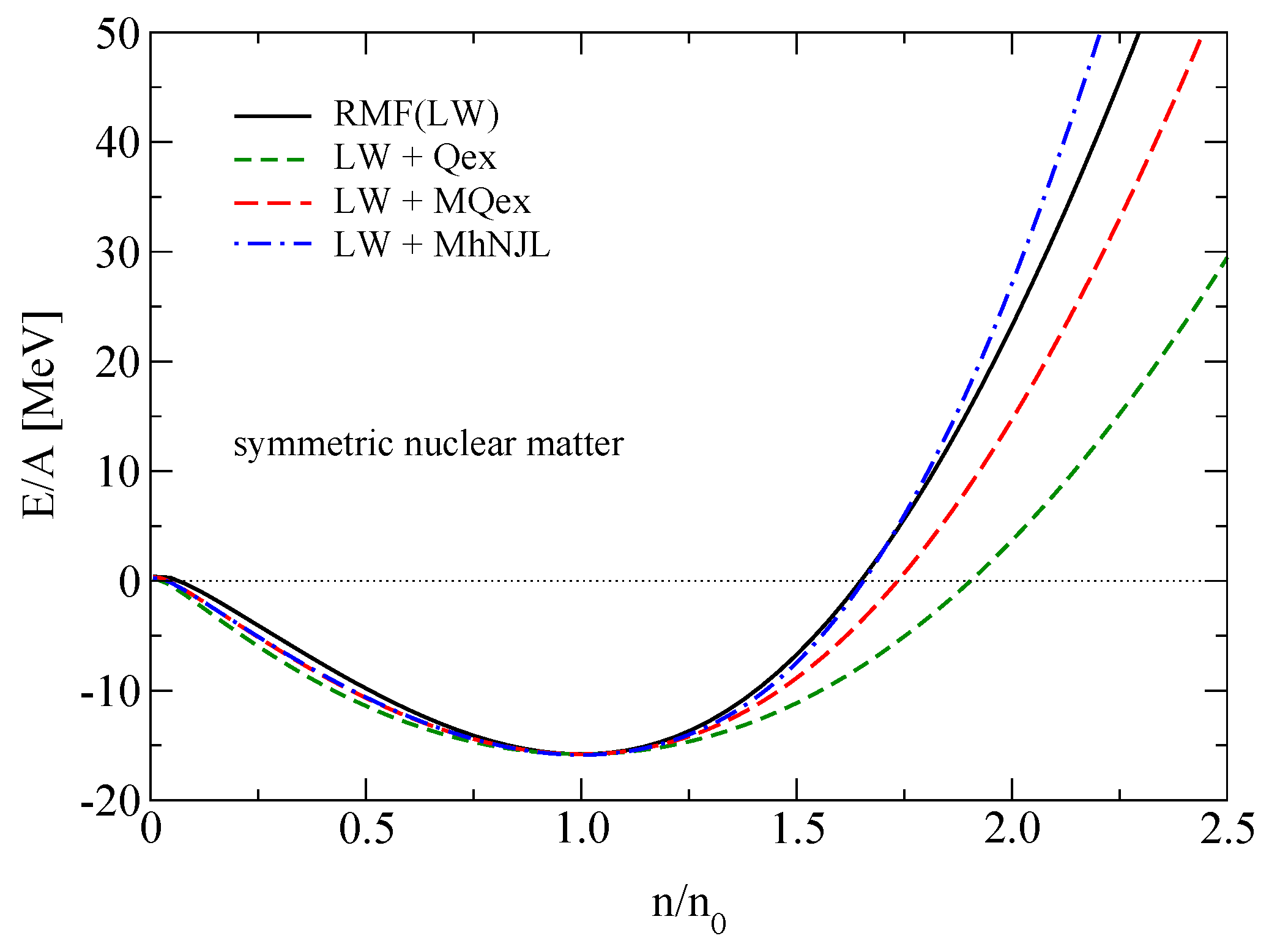
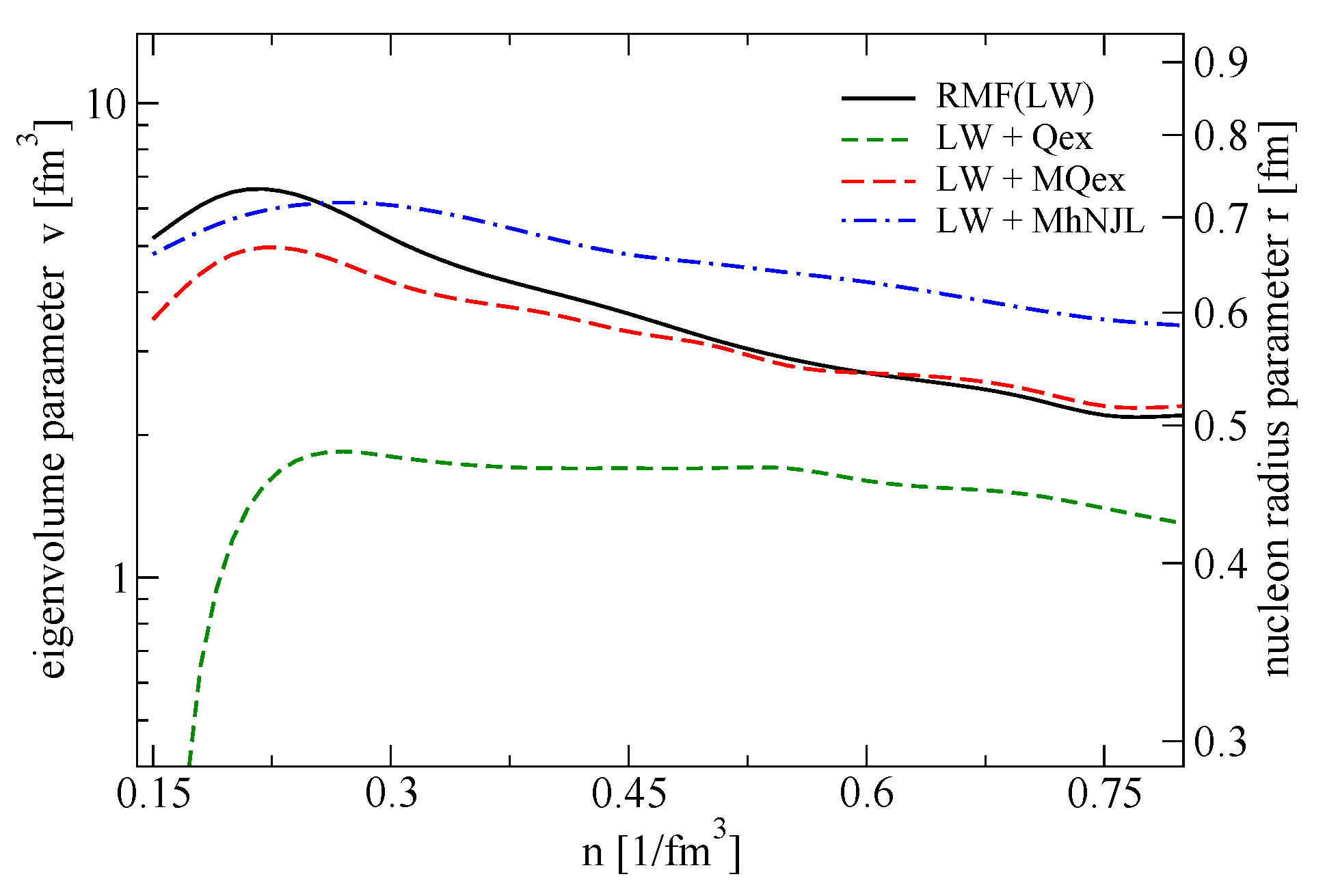
4.3. Comparison with Nucleonic Excluded Volume
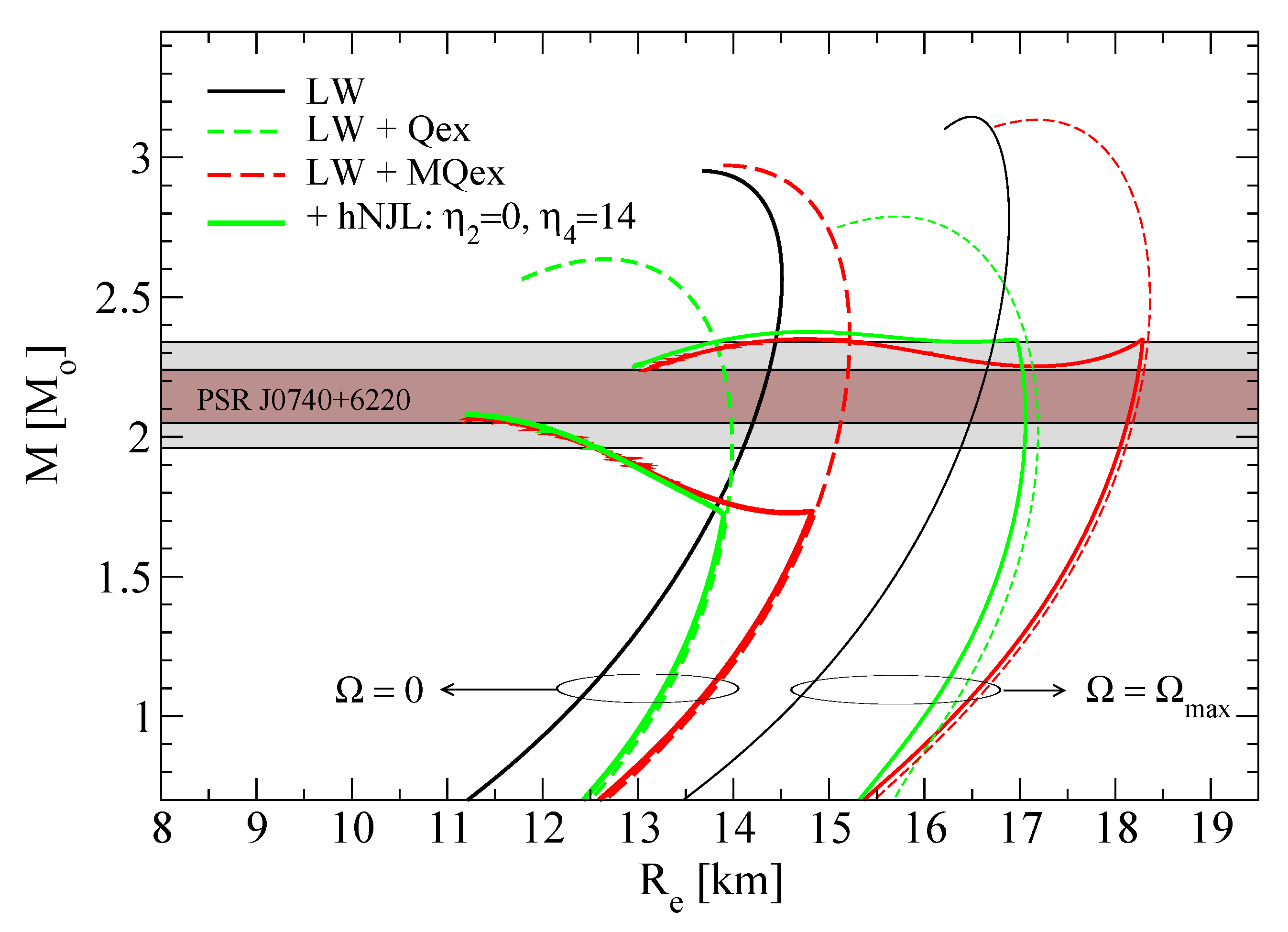
4.4. Applications for Neutron Stars
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Pauli Quenching for Nucleons in Nuclear Matter-a Quark Substructure Effect
| 31/243 | −31/243 | ||
| 14/243 | −17/243 | ||
| 14/243 | −17/243 | ||
| 22/243 | −25/243 | ||
| 1/3 | −10/27 |

References
- Ebeling, W.; Blaschke, D.; Redmer, R.; Reinholz, H.; Röpke, G. The Influence of Pauli blocking effects on the properties of dense hydrogen. J. Phys. A 2009, 42, 214033. [Google Scholar] [CrossRef]
- Carnahan, N.F.; Starling, K.E. Equation of State for Nonattracting Rigid Spheres. J. Chem. Phys. 1969, 51, 635–636. [Google Scholar] [CrossRef]
- Mansoori, G.A.; Carnahan, N.F.; Starling, K.E.; Leland, T.W.J. Equilibrium Thermodynamic Properties of the Mixture of Hard Spheres. J. Chem. Phys. 1971, 54, 1523–1525. [Google Scholar] [CrossRef]
- Sagun, V.; Bugaev, K.; Ivanytskyi, A.; Yakimenko, I.; Nikonov, E.; Taranenko, A.; Greiner, C.; Blaschke, D.; Zinovjev, G. Hadron Resonance Gas Model with Induced Surface Tension. Eur. Phys. J. A 2018, 54, 100. [Google Scholar] [CrossRef]
- Röpke, G.; Blaschke, D.; Döppner, T.; Lin, C.; Kraeft, W.D.; Redmer, R.; Reinholz, H. Ionization potential depression and Pauli blocking in degenerate plasmas at extreme densities. Phys. Rev. E 2019, 99, 033201. [Google Scholar] [CrossRef] [PubMed]
- Röpke, G. Parametrization of light nuclei quasiparticle energy shifts and composition of warm and dense nuclear matter. Nucl. Phys. A 2011, 867, 66–80. [Google Scholar] [CrossRef]
- Röpke, G.; Blaschke, D.; Schulz, H. Pauli Quenching Effects in a Simple String Model of Quark/Nuclear Matter. Phys. Rev. 1986, D34, 3499–3513. [Google Scholar] [CrossRef]
- Vautherin, D.; Brink, D. Hartree-Fock calculations with Skyrme’s interaction. 1. Spherical nuclei. Phys. Rev. C 1972, 5, 626–647. [Google Scholar] [CrossRef]
- Bugaev, K.A.; Ivanytskyi, A.I.; Sagun, V.V.; Grinyuk, B.E.; Savchenko, D.O.; Zinovjev, G.M.; Taranenko, A.V. Hard-core Radius of Nucleons within the Induced Surface Tension Approach. Universe 2019, 5, 63. [Google Scholar] [CrossRef]
- Bugaev, K.A.; Vitiuk, O.V.; Grinyuk, B.E.; Sagun, V.V.; Yakovenko, N.S.; Ivanytskyi, O.I.; Zabrodin, E.E. Second virial coefficients of light nuclear clusters and their chemical freeze-out in nuclear collisions. arXiv 2020, arXiv:2005.01555. [Google Scholar]
- Vovchenko, V.; Motornenko, A.; Gorenstein, M.I.; Stoecker, H. Beth-Uhlenbeck approach for repulsive interactions between baryons in a hadron gas. Phys. Rev. C 2018, 97, 035202. [Google Scholar] [CrossRef]
- Typel, S. Variations on the excluded-volume mechanism. Eur. Phys. J. A 2016, 52, 16. [Google Scholar] [CrossRef]
- Röpke, G.; Münchow, L.; Schulz, H. Particle clustering and Mott transitions in nuclear matter at finite temperature. Nucl. Phys. A 1982, 379, 536–552. [Google Scholar] [CrossRef]
- Röpke, G.; Schmidt, M.; Münchow, L.; Schulz, H. Particle clustering and Mott transition in nuclear matter at finite temperature (II). Nucl. Phys. A 1983, 399, 587–602. [Google Scholar] [CrossRef]
- Typel, S.; Röpke, G.; Klähn, T.; Blaschke, D.; Wolter, H. Composition and thermodynamics of nuclear matter with light clusters. Phys. Rev. C 2010, 81, 015803. [Google Scholar] [CrossRef]
- Natowitz, J.B.; Röpke, G.; Typel, S.; Blaschke, D.; Bonasera, A.; Hagel, K.; Wada, R. Symmetry energy of dilute warm nuclear matter. Phys. Rev. Lett. 2010, 104, 202501. [Google Scholar] [CrossRef]
- Sumiyoshi, K.; Röpke, G. Appearance of light clusters in post-bounce evolution of core-collapse supernovae. Phys. Rev. C 2008, 77, 055804. [Google Scholar] [CrossRef]
- Röpke, G. Light p-shell nuclei with cluster structures (4 ≤ A ≤ 16) in nuclear matter. arXiv 2020, arXiv:2004.09773. [Google Scholar]
- Lattimer, J.M.; Swesty, F. A Generalized equation of state for hot, dense matter. Nucl. Phys. A 1991, 535, 331–376. [Google Scholar] [CrossRef]
- Blaschke, D.; Röpke, G. Pauli Quenching for Hadrons in Nuclear Matter: A Quark Substructure Effect; Report JINR-E2-88-77; JINR Dubna: Dubna, Russia, 1988. [Google Scholar]
- Brown, G.; Rho, M. Scaling effective Lagrangians in a dense medium. Phys. Rev. Lett. 1991, 66, 2720–2723. [Google Scholar] [CrossRef]
- Benic, S. Heavy hybrid stars from multi-quark interactions. Eur. Phys. J. A 2014, 50, 111. [Google Scholar] [CrossRef][Green Version]
- Chin, S.; Walecka, J. An Equation of State for Nuclear and Higher-Density Matter Based on a Relativistic Mean-Field Theory. Phys. Lett. B 1974, 52, 24–28. [Google Scholar] [CrossRef]
- Walecka, J. A Theory of highly condensed matter. Ann. Phys. 1974, 83, 491–529. [Google Scholar] [CrossRef]
- Kashiwa, K.; Kouno, H.; Sakaguchi, T.; Matsuzaki, M.; Yahiro, M. Chiral phase transition in an extended NJL model with higher-order multi-quark interactions. Phys. Lett. B 2007, 647, 446–451. [Google Scholar] [CrossRef]
- Danielewicz, P.; Lacey, R.; Lynch, W.G. Determination of the equation of state of dense matter. Science 2002, 298, 1592–1596. [Google Scholar] [CrossRef]
- Ivanytskyi, A.; Bugaev, K.; Sagun, V.; Bravina, L.; Zabrodin, E. Influence of flow constraints on the properties of the critical endpoint of symmetric nuclear matter. Phys. Rev. C 2018, 97, 064905. [Google Scholar] [CrossRef]
- Cromartie, H.T.; Fonseca, E.; Ransom, S.M.; Demorest, P.B.; Arzoumanian, Z.; Blumer, H.; Ferdman, R.D. Relativistic Shapiro delay measurements of an extremely massive millisecond pulsar. Nat. Astron. 2019, 4, 72–76. [Google Scholar] [CrossRef]
- Gerlach, U.H. Equation of State at Supranuclear Densities and the Existence of a Third Family of Superdense Stars. Phys. Rev. 1968, 172, 1325–1330. [Google Scholar] [CrossRef]
- Alvarez-Castillo, D.; Benic, S.; Blaschke, D.; Han, S.; Typel, S. Neutron star mass limit at 2M⊙ supports the existence of a CEP. Eur. Phys. J. A 2016, 52, 232. [Google Scholar] [CrossRef]
- Blaschke, D.; Alvarez-Castillo, D.E.; Benic, S. Mass-radius constraints for compact stars and a critical endpoint. arXiv 2013, arXiv:1310.3803. [Google Scholar]
- Blaschke, D.; Ayriyan, A.; Alvarez-Castillo, D.; Grigorian, H. Was GW170817 a canonical neutron star merger? Bayesian analysis with a third family of compact stars. Universe 2020, 6, 81. [Google Scholar] [CrossRef]
- Blaschke, D.; Tovmasian, T.; Kämpfer, B. Predicting Stable Quark Cores in Neutron Stars From a Unified Description of Quark - Hadron Matter. Sov. J. Nucl. Phys. 1990, 52, 675–678. [Google Scholar]
- Chubarian, E.; Grigorian, H.; Poghosyan, G.S.; Blaschke, D. Deconfinement phase transition in rotating nonspherical compact stars. Astron. Astrophys. 2000, 357, 968–976. [Google Scholar]
- Blaschke, D.; Alvarez-Castillo, D.E.; Ayriyan, A.; Grigorian, H.; Largani, N.K.; Weber, F. Astrophysical aspects of general relativistic mass twin stars. In Topics in Strong Gravity; Zen Vasconcellos, C.A., Ed.; World Scientific: Singapore, 2020; pp. 207–256. [Google Scholar] [CrossRef]
- Isgur, N.; Karl, G. P Wave Baryons in the Quark Model. Phys. Rev. 1978, D18, 4187. [Google Scholar] [CrossRef]
- Isgur, N.; Karl, G. Positive Parity Excited Baryons in a Quark Model with Hyperfine Interactions. Phys. Rev. 1979, D19, 2653. [Google Scholar] [CrossRef]
- Isgur, N.; Karl, G. Ground State Baryons in a Quark Model with Hyperfine Interactions. Phys. Rev. 1979, D20, 1191–1194. [Google Scholar] [CrossRef]
- Barnes, T.; Capstick, S.; Kovarik, M.; Swanson, E. N N core interactions and differential cross-sections from one gluon exchange. Phys. Rev. C 1993, 48, 539–552. [Google Scholar] [CrossRef]
- Oka, M.; Yazaki, K. Nuclear Force in a Quark Model. Phys. Lett. 1980, 90B, 41–44. [Google Scholar] [CrossRef]
- Oka, M.; Yazaki, K. Short Range Part of Baryon Baryon Interaction in a Quark Model. 1. Formulation. Prog. Theor. Phys. 1981, 66, 556–571. [Google Scholar] [CrossRef][Green Version]
- Oka, M.; Yazaki, K. Short Range Part of Baryon Baryon Interaction in a Quark Model. 2. Numerical Results for S-Wave. Prog. Theor. Phys. 1981, 66, 572–587. [Google Scholar] [CrossRef]
- Burov, V.V.; Dorkin, S.M.; Lukyanov, V.K.; Titov, A.I. On the Six Quark Structure in the Deuteron Form-factor. Z. Phys. 1982, A306, 149. [Google Scholar] [CrossRef]
- Faessler, A.; Fernandez, F.; Lübeck, G.; Shimizu, K. The Quark Model and the Nature of the Repulsive Core of the Nucleon Nucleon Interaction. Phys. Lett. 1982, 112B, 201–205. [Google Scholar] [CrossRef]
- Faessler, A.; Fernandez, F.; Lübeck, G.; Shimizu, K. The Nucleon Nucleon Interaction and the Role of the (42) Orbital Six Quark Symmetry. Nucl. Phys. 1983, A402, 555–568. [Google Scholar] [CrossRef]
- Oka, M.; Horowitz, C.J. Hadron interaction in the string flipflop model of quark confinement with color, spin and flavor degrees of freedom. 2. Nucleon-nucleon interaction. Phys. Rev. 1985, D31, 2773–2779. [Google Scholar] [CrossRef]
- Barnes, T.; Swanson, E. Kaon-nucleon scattering amplitudes and Z* enhancements from quark Born diagrams. Phys. Rev. C 1994, 49, 1166–1184. [Google Scholar] [CrossRef]
- Oka, M. Hadron interaction in the string flipflop model of quark confinement with color, spin and flavor degrees of freedom. 1. Meson-meson interaction. Phys. Rev. 1985, D31, 2274–2287. [Google Scholar] [CrossRef]
- Barnes, T.; Swanson, E. A Diagrammatic approach to meson meson scattering in the nonrelativistic quark potential model. Phys. Rev. D 1992, 46, 131–159. [Google Scholar] [CrossRef]
- Barnes, T.; Swanson, E.; Weinstein, J.D. I = 3/2 K pi scattering in the nonrelativistic quark potential model. Phys. Rev. D 1992, 46, 4868–4872. [Google Scholar] [CrossRef] [PubMed]
- Blaschke, D.; Röpke, G. Quark exchange contribution to the effective meson meson interaction potential. Phys. Lett. B 1993, 299, 332–337. [Google Scholar] [CrossRef]
- Horowitz, C.; Moniz, E.; Negele, J.W. Hadron structure in a simple model of quark/nuclear matter. Phys. Rev. D 1985, 31, 1689–1699. [Google Scholar] [CrossRef]
- Blaschke, D.; Reinholz, F.; Röpke, G.; Kremp, D. Mott Mechanism and the Hadronic to Quark Matter Phase Transition. Phys. Lett. 1985, 151B, 439–443. [Google Scholar] [CrossRef]
- Schulz, H.; Röpke, G. A Note on the Massive Quark Matter Phase. Z. Phys. 1987, C35, 379–382. [Google Scholar] [CrossRef]
- Röpke, G.; Blaschke, D.; Schulz, H. Quark substructure contribution to the temperature dependent effective nucleon mass. Phys. Lett. 1986, B174, 5–9. [Google Scholar] [CrossRef]
- Storm, M.H.; Watt, A. Clustering in many quark systems: The two nucleon problem. J. Phys. 1985, G11, 217–230. [Google Scholar] [CrossRef]



| n | − | |
| p | − |
| [fm2] | [fm2] | K [MeV] | [MeV] | [km] | |
|---|---|---|---|---|---|
| RMF (LW) | 11.6582 | 15.2883 | 608.874 | 21.58 | 13.22 |
| LW + Qex | 6.11035 | 9.91197 | 331.958 | 32.04 | 13.70 |
| LW + MQex | 8.59170 | 13.29118 | 481.713 | 34.12 | 14.40 |
| LW + MhNJL | 9.25683 | 13.9474 | 582.831 | 31.55 | 14.29 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Blaschke, D.; Grigorian, H.; Röpke, G. Chirally Improved Quark Pauli Blocking in Nuclear Matter and Applications to Quark Deconfinement in Neutron Stars. Particles 2020, 3, 477-499. https://doi.org/10.3390/particles3020033
Blaschke D, Grigorian H, Röpke G. Chirally Improved Quark Pauli Blocking in Nuclear Matter and Applications to Quark Deconfinement in Neutron Stars. Particles. 2020; 3(2):477-499. https://doi.org/10.3390/particles3020033
Chicago/Turabian StyleBlaschke, David, Hovik Grigorian, and Gerd Röpke. 2020. "Chirally Improved Quark Pauli Blocking in Nuclear Matter and Applications to Quark Deconfinement in Neutron Stars" Particles 3, no. 2: 477-499. https://doi.org/10.3390/particles3020033
APA StyleBlaschke, D., Grigorian, H., & Röpke, G. (2020). Chirally Improved Quark Pauli Blocking in Nuclear Matter and Applications to Quark Deconfinement in Neutron Stars. Particles, 3(2), 477-499. https://doi.org/10.3390/particles3020033






