Study of an Optimized Mechanical Oscillator for the Forced Vibration of the Soil Cutting Blade

Abstract

In the nursery sector, the transport and planting of trees must occur with the roots wrapped in a ball of the original earth. The cutting of the original soil can be carried out with a semicircular vibrating blade moved by an oscillator mounted on a self-propelled machine. The oscillator produces an excitation torque supplied to the blade together with the soil cutting torque. The advantage of the vibrating blade is a reduction in the cutting torque of up to 70%. However, to correctly design the oscillator, we need to investigate the link between the maximum displacement of the blade, the maximum oscillation velocity, the cutting velocity, the dry friction, the excitation torque, the elastic torque, the cutting torque, the required power, the required energy, and the excitation frequency. The maximum displacement and velocity ratio need to have the right values to minimize the cutting torque and to avoid the springs reaching the end of stroke; otherwise, vibrations are transmitted to the machine and to the operator. Therefore, starting from the forced oscillation differential equation and using an approximate solution method developed by Den Hartog, along with some experimental data, a mathematical model was constructed to optimize the oscillator design. After construction, it was coupled to blades of various diameters (0.6, 0.9, and 1.2 m) to undergo experimental tests. The soil cutting tests highlighted the achievement of the above objectives and, at the same time, confirmed the validity of the Den Hartog equations used to calculate the phase lag and the maximum displacement, resulting in an average error of 4.4% and a maximum error of 6.4%.

1. Introduction

In many areas of technology, vibrating tools are used to reduce the cutting force in materials. In addition, in the case of soil, intense research and applications have been conducted over the last few decades. The first contribution was by Gunn and Tramontini [1], who found that the total energy required for cutting is approximately equal between the vibrating blade and the static one. Subsequently, Egenmueller [2] demonstrated that if the ratio ${\dot{A}}_0/v_{cut}$ between the peak velocity of the oscillation ${\dot{A}}_0$ and the cutting velocity v_cut of the tool is higher, then the vibration produces a greater reduction in the soil cutting force. In his experiments, he found that the ratio of the cutting force with the vibrating tool to that of the non-vibrating tool was a monotonic function decreasing with respect to the velocity ratio ${\dot{A}}_0/v_{cut}$. With the maximum value of the latter equal to 6, he found that the force ratio was reduced to 0.4. However, his results were conditioned by the maximum linear displacement A₀ of at least 6 mm, but he also demonstrated that higher values of A₀ produce only poor improvements in cutting force reduction. In the following years, other authors substantially confirmed these indications [3,4].

Butson and MacIntyre [5], through experiments in soil tanks, obtained a reduction in the cutting force of 50% with a velocity ratio greater than 1, a frequency of 50 Hz, and an A₀ of 8 mm. Butson and Rackham [6] derived a mathematical model for predicting cutting force by considering all parameters for an effective prediction.

Narayanarao and Verma [7] also developed a mathematical model to predict cutting force, with the results compared with the experimental ones detected on a vibrating tool in harmonic motion.

In the specific potato-digging machine sector, Al-Jubouri and McNulty [8] developed a vibrating digging blade and confirmed the importance of a higher velocity ratio to reduce the cutting force, even if the expended energy increases. They also observed that the vibrating blade reduced potato damage and losses.

Other works [9,10] were carried out in which the authors showed that the oscillations must occur lengthwise, along the direction of motion, to obtain the maximum efficacy in reducing the cutting force. Lateral or vertical vibrations are not very useful.

In all the experiments mentioned, the forced vibrations were harmonic, while Smith et al. [11,12] also tested non-harmonic vibrations such as square waves and sawtooth waves, finding no difference for the purpose of reducing the cutting force.

With a vibrating tool on clay soil, Niyamapa and Salokhe [13] ascertained that the vibration resulted in a 41–45% increase in power requirement compared with the non-oscillating tool, but the vibration produced a greater breaking up of the soil.

Szabo et al. [14] conducted experiments with an oscillating tool, pushing the velocity ratio beyond the limit of 6, concluding that a ratio of cutting forces, with and without vibration, equal to 0.3 can be obtained by a employing velocity ratio ${\dot{A}}_0/v_{cut}$ that is equal to or greater than 17.

Shahgoli et al. [15] developed a vibrating ripper for hard compacted soil, achieving a force reduction of 50% with a frequency of 4.9 Hz and an amplitude of 60–69 mm. They then studied [16] a dynamic simulation model for an oscillating subsoiler for small tractors. Finally, they identified [17] the optimal frequency, reaching a cutting force ratio of 0.36.

Tang et al. [18] conducted comparison tests between a static, a rotating, and a vibrating subsoiler, concluding that the latter is more effective in reducing the cutting force. Tests by Shchukin et al. [19] on a vibrating subsoiler compared with a static one showed that the vibration improved the soil structure. Razzaghi and Sohrabi [20] demonstrated a new method of analysis of the interaction between a vibrating tool and the soil based on polar coordinates.

Rao et al. [21,22,23,24,25] developed optimized design algorithms for a vibratory tillage cultivator. Keppler et al. [26] simulated the effect of tillage cultivator vibrations on cutting force using the discrete element method (DEM). Biris et al. [27] found that the influence of vibration during soil cutting is equivalent to an additional force to overcome the frictional resistance.

Experimenting on a vibratory tillage cultivator, Dzhabborov et al. [28] determined that an increase in frequency improves both the reduction in cutting force and the structure of the soil. Wang et al. [29] also confirmed that a frequency increase produces a reduction in the cutting force, but an increase in the required power.

In summary, the best result, with regard to a cutting force ratio equal to 0.3, is obtained by reaching a velocity ratio ${\dot{A}}_0/v_{cut}$ equal to or greater than 17, and with a maximum linear displacement A₀ of at least 6 mm.

This knowledge was used some years ago [30] to experimentally analyze the motion of the 0.9 m diameter vibrating cutting blade of a tree digger machine. These machines are used to cut the hemispherical clod of soil (Figure 1) that encloses the root system of trees so that they can be transported and planted in orchards or gardens after growing in a tree nursery. Alternatively, the tree digger machine which forms the clod of soil using a series of spades can be used. The spades are mounted on a frame arranged around the tree and driven into the soil with hydraulic cylinders without the aid of vibrations. With no vibrations, digging is slower. However, the tree digger machine with spades is still interesting for digging large trees.

The study and experimentation at the time highlighted the shortcomings of the mechanical oscillator used for the forced vibration of the blade. In fact, with this oscillator, it was found that the maximum linear displacement A₀ was limited to approximately 5 mm instead of 6, while it allowed a correct velocity ratio ${\dot{A}}_0/v_{cut}$ equal to 17.

With the use of the tree digger machine extended to root ball diameters greater than 0.9 m (i.e., 1.2 m), the mechanical oscillator then showed two further limitations. The first was that the blade vibrated with maximum linear displacement values equal to half of the optimal value of at least 6 mm. The second problem was a dangerous transmission of vibrations to the machine frame and therefore to the operator [31,32,33].

Since the vibrating blades cut the soil and encounter dry friction without sticking, the motion dynamics can be described with the approximate solution proposed by Den Hartog [34]. This author also demonstrated the excellent correlation of this approximate solution with the exact solution provided by the differential equation of motion obtained by representing the dry friction force with a Fourier series.

Therefore, in this work, mathematical modeling based on Den Hartog equations providing the maximum displacement and the phase lag will be performed. The values of these quantities will allow for the design of a new mechanical oscillator able to increase the maximum linear displacement to the optimal values for a maximum reduction in cutting force and which will not transmit the vibrations to the frame of the machine, especially for a larger diameter (i.e., equal to 1.2 m).

Three different blades (D = 0.6, 0.9 and 1.2 m) will be mounted on this mechanical oscillator, and experimental soil cutting tests will be performed for a validation of the mathematical model based on Den Hartog’s equations. In fact, it must be considered that the application of Den Hartog’s theory on agricultural soil requires the assumption of simplifying the hypotheses on dry friction represented by Coulomb’s law. Finally, the mathematical model equations will be used to calculate the cutting and oscillation power and energy, thus defining the best excitation frequency value.

2. Materials and Methods

2.1. The Mechanical Oscillator and the Vibrating Blade

Figure 2 shows the vibrating system, consisting of a semicircular blade for cutting the soil and an oscillator, with a set of five gear wheels inside. The central wheel (in black) is moved by an external hydraulic motor placed on the left. The motion is transmitted, via the two intermediate gear wheels (1) to the outermost gear wheels, which each carry an eccentric mass (2).

During the rotation of the gear wheels, when the two masses are at an angle of π/2 with respect to how they appear in Figure 2, they produce a maximum excitation torque T_em, while in the position of Figure 2, they are in opposition and therefore their centrifugal forces are balanced. Therefore, the excitation torque varies according to harmonic law and induces the entire gear housing (3) to an oscillating motion, which is transmitted to the shaft (4) and therefore to the horizontal butterfly bush (5) and to the blade (6). Note that the splined shaft to the left of the horizontal butterfly bush (5) is not connected to the central gear wheel (in black) but is connected to the gear housing (3). Therefore, the latter transmits the oscillation to the splined shaft and hence to the blade (6) via the shaft (4). The horizontal butterfly bush has a system of springs (7) supported by a vertical butterfly bush (8). However, this bush is not fixed but can rotate because it is controlled by a worm screw (9) when the operator wants to impart the rotating cutting movement to the blade.

2.2. The Differential Equation of the Forced Vibration with Coulomb Friction

Figure 3 shows the dynamic diagram of Den Hartog’s system [34], here modified to replace linear motion with angular motion, given that the vibrating blade is in rotation while cutting the soil. The blade is then subjected to excitation torque due to the two rotating eccentric masses (Figure 2 (2)): $T_{em}·\cos \left(\omega t+\varphi \right)$. In this expression, the phase angle ϕ is introduced, as per Den Hartog, who, recognizing the fact that there is a delay in the motion of the blade, explained that the phase angle introduced in the definition of the excitation torque has no meaning and is only included with the purpose of writing the boundary conditions for the solution to the equation of dynamics in a simple way.

Figure 3 also shows the presence of dry friction torque T_F, which, following Coulomb’s law, is variable according to a rectangular wave (Figure 4) in phase with the sine wave of the angular velocity $\dot{\alpha }$ of the blade oscillation. Mostaghel [35] proposed representing this rectangular wave with a hyperbolic function: $T_F·\tanh \left(1000·\dot{\alpha }\right)$, where $\dot{\alpha }$ is the angular velocity of the blade oscillation, while α represents the angular displacement of the blade. Finally, Figure 3 shows the presence of return springs, which exert an elastic torque equal to k·α, where k is the torsional spring rate. If J denotes the moment of inertia of the gear housing/bush-blade system (Figure 2), the differential equation of dynamics is

$$J·\ddot{\alpha }+k·\alpha +T_F=T_{em}·\cos \left(\omega t+\varphi \right)$$

(1)

where J (moment of inertia), k (torsional springs rate), and T_em (maximum excitation torque) are constants to be correlated with the geometric and dynamic characteristics of the system.

The moment of inertia J was calculated using AutoCAD 2016 software after drawing the oscillating parts of the system. The quantity k was calculated with the following expression:

$$k=z·k_l·b_S^2$$

(2)

where z is the number of springs (z = 4); k_l represents the linear spring rate of the single helicoidal spring; and b_S denotes the lever arm of the springs.

The maximum excitation torque T_em was calculated with the following expression:

$$T_{em}=n·m·{\omega }^2·y_G·b_G$$

(3)

where ω is the angular velocity of the eccentric masses and, hence, the angular frequency of the excitation torque; n stands for the number of eccentric masses (2 masses in Figure 2, but they can be 4 or 6); m denotes the eccentric mass value; y_G represents the mass eccentricity; and b_G refers to the lever arm of the masses (i.e., the distance between the eccentric mass shaft and the blade shaft).

The amplitude of Coulomb torque T_F is

$$T_F=dry friction force·lever arm=2·\mu ·G·\frac{D}{\pi }$$

(4)

where D/π represents the lever arm of the dry friction force and D is the diameter of the hemispherical root ball. The dry friction force is the product of the external friction coefficient μ and the force G acting on the blade when it is at maximum depth (i.e., halfway through the cutting stroke of the soil ball). Thus, the force G, applied to the upper side of the blade, is due to the mass of soil that has already been cut when the blade is at maximum depth. Therefore, this mass corresponds to half of the root ball, which in turn is half of the sphere of diameter D. Since half of the tree weighs on the half root ball, and since it can be said, empirically, that the tree weighs as much as the root ball, the normal force G on the upper side of the blade is: $G=2·\frac{1}{4}·\rho ·\frac{\pi }{6}{D}^3·g$, where ρ is the soil density, D represents the diameter of the blade and is therefore the diameter of the root ball, and g denotes the gravity acceleration. Furthermore, in Equation (4), the coefficient 2 appears because the reaction of the cut soil is equal and opposite to G on the lower side of the blade. Ultimately, the friction torque is

$$T_F=\mu ·\rho ·\frac{D^4}{6}·g$$

(5)

2.3. Den Hartog Solution Applied to the Vibrating Blade

Den Hartog [34] rewrote the differential Equation (1) after introducing the following abbreviations, adapted here to the case of angular instead of linear motion:

The static displacement under maximum excitation torque $a=T_{em}/k$

The static displacement under maximum friction torque ${\alpha }_f=T_F/k$

The natural frequency ${\omega }_n=\sqrt{\frac{k}{J}}$

$$\ddot{\alpha }+{\omega }_n^2·\left(\alpha -{\alpha }_f\right)= a·{\omega }_n^2·\cos \left(\omega t+\varphi \right)$$

(6)

With the following boundary conditions:

• $t=0 \to \alpha ={\alpha }_0 \to \dot{\alpha }=0$
• $t=\mathsf{\pi }/\omega \to \alpha =-{\alpha }_0 \to \dot{\alpha }=0$

Den Hartog obtained a steady-state solution, and from it, he proposed the equations for the “magnification factor” ${\alpha }_0/a$ and the “phase angle” ϕ:

$$\frac{{\alpha }_0}{a}=\sqrt{V^2-{\left(\frac{T_F}{T_{em}}\right)}^2{U}^2}$$

(7)

$$\varphi =\arccos \left(\frac{{\alpha }_0}{a}·\frac{1}{V}\right)$$

(8)

where

$V=\frac{{\beta }^2}{{\beta }^2-1}$ is the “response function”;

$U=\frac{\beta ·\sin \left(\beta ·\pi \right)}{1+\cos \left(\beta ·\pi \right)}$ is the “Coulomb damping function”;

$\beta =\frac{{\omega }_n}{\omega }$ is the “frequency ratio”.

2.4. The Vibrating Blade While Cutting the Soil

When the operator activates the screw gear (9) (Figure 2), it rotates the vibrating blade to cut the soil. The reaction of the soil induces the cutting torque T_cut on the blade, which forces the springs on one side of the vertical bush (Figure 2) to compress, while those on the opposite side lengthen. This static compression results in $\left(T_{cut}/k\right)b_S$. Thus, the coil springs of one side result in a total linear compression, which is the sum of the previous static compression and the maximum linear displacement of the springs: $\left(T_{cut}/k\right)b_S+{\alpha }_0·b_S,$ where α₀ is the maximum angular displacement, k represents the torsional spring rate and b_S denotes the lever arm of the springs.

To prevent vibration from being transmitted to the tree digger machine frame and therefore also to the operator, the total linear compression of the springs must be less than the total space S_t between the coils of the springs:

$$S_t\ge \left(\frac{T_{cut}}{k}+{\alpha }_0\right)·b_S$$

(9)

2.5. Soil Cutting Time and Energy Required

After setting the optimal velocity ratio ${\dot{A}}_0/v_{cut}$ between the maximum oscillation velocity of the blade ${\dot{A}}_0=\dot{{\alpha }_0}·D/2={\alpha }_0·\omega ·D/2$ and the cutting velocity $v_{cut}=(\pi ·D/2)/t_{cut}$, the soil cutting time can be calculated, given that the blade runs in a semicircle of diameter D during cutting. As a previous work [14] had established that this velocity ratio ${\dot{A}}_0/v_{cut}$ must be at least equal to 17, the soil cutting time can be obtained with the following expression:

$$t_{cut}=\frac{17·\pi }{{\alpha }_0·\omega }$$

(10)

The power required for the oscillation is given by the following equation:

$$P_o={\left(T_{em}\right)}_{eff}·{\left({\dot{\alpha }}_0\right)}_{eff}·\cos \left(\psi \right)=\frac{1}{2}·T_{em}·{\alpha }_0·\omega ·\cos \left(\varphi -\frac{\pi }{2}\right)$$

(11)

where the excitation torque T_em and the angular velocity of the oscillation of the blade ${\dot{\alpha }}_0$ are the effective values, equivalent to the maximum values multiplied by $\sqrt{2}/2$; the maximum angular velocity of oscillation is ${\dot{\alpha }}_0={\alpha }_0·\omega$; and the phase lag ψ is the angle between the excitation torque T_em and the angular velocity ${\dot{\alpha }}_0$. As this velocity is ahead by π/2 in comparison with the angular displacement ${\alpha }_0$, and this is behind by $\varphi$ in comparison with T_em, the result is: $\psi =\varphi -\pi /2$.

To obtain the total power P_t, this oscillating power P_o must be added to the soil cutting power P_cut, that is,

$$P_{cut}=\frac{T_{cut·}\pi }{t_{cut}}$$

(12)

The oscillating power P_o is supplied by the external hydraulic motor to the central gear wheel (in black) of the housing (3) (Figure 2), while the cutting power P_cut is supplied by another external hydraulic motor to the worm screw (9).

The theoretical total energy W_tot (i.e., net of transmission efficiencies) required during the vibratory cutting operation is the sum of the oscillating energy W_o and the cutting energy W_cut. These energies can be obtained by multiplying the power by the cutting time t_cut. Equations (10)–(12) combined give the following total energy equation:

$$W_t=W_o+W_{cut}=P_o·t_{cut}+P_{cut}·t_{cut}=\frac{1}{2}·T_{em}·17\pi ·\cos \left(\varphi -\frac{\pi }{2}\right)+T_{cut}·\pi$$

(13)

2.6. Experimental Evaluation of Cutting Torque and Maximum Displacement

To measure the cutting torque T_cut, four weighting platforms linked to a data logger were used. They were placed under the two tracks of the tree digger machine, two on the right and two on the left. When the blade of the tree digger machine cut the soil, for balance, an equal and opposite reaction torque manifested itself as T_cut = F·C, where C was the center distance between the two tracks and è F was the force that was measured by the weighting platforms.

To measure the maximum angular displacement of the blade α₀ while cutting the soil, a laser Doppler vibrometer was used that measured the maximum linear displacement x_G0 of the gear housing (Figure 2). If d is the diameter of the gear housing, the experimental value of α₀ is ${\alpha }_0=x_{G0}/d$.

The cutting tests were performed using a tree digger machine with the new oscillator coupled with the three different blades with diameters D of 0.6, 0.9, and 1.2 m, respectively. The cutting operation, repeated five times, was carried out on typical tree-nursery soil, that is, a medium-textured soil with an average moisture content equal to 21.5%, an average external friction coefficient μ with the steel blade of 0.52, and an average soil density ρ of 1598 kg/m³.

The moisture content of the soil was measured in the laboratory by weighing the samples before and after drying in an oven at 408 K (135 °C) for 2 hours. The test was replicated five times. The soil density ρ was obtained by measuring the mass and volume of the samples. The coefficient of dynamic external friction μ was measured using an adjustable inclined steel plane, on which the cubic clod of soil was placed, and above which there was an accelerometer. After having started the motion of the soil clod by raising the angle of the inclined plane, and having measured both the plane angle γ and the acceleration a, the dynamic friction coefficient μ was calculated with the dynamics equation: $\mu =\tan \beta -a/(g·\cos \beta )$. The test was replicated five times.

3. Results and Discussion

Through Equations (2), (3), (5), (7)–(9), along with the following Equation (14), which provides the cutting torque T_cut detected in previous tests [11] with the old oscillator:

$$T_{cut}=2478·D-4272·D^2+6385·D^3$$

(14)

A different oscillator was studied and built that satisfied the conditions for minimizing the cutting torque (i.e., a maximum linear displacement $A_0\ge 6 \mathrm{mm}$ and a velocity ratio $\left(\dot{{\alpha }_0}·D/2\right)/v_{cut}\ge 17)$ and which did not transmit vibrations to the frame or to the operator, even for the bigger blade diameter (i.e., D = 1.2 m). In

Table 1. Geometric and dynamic features of the oscillators.

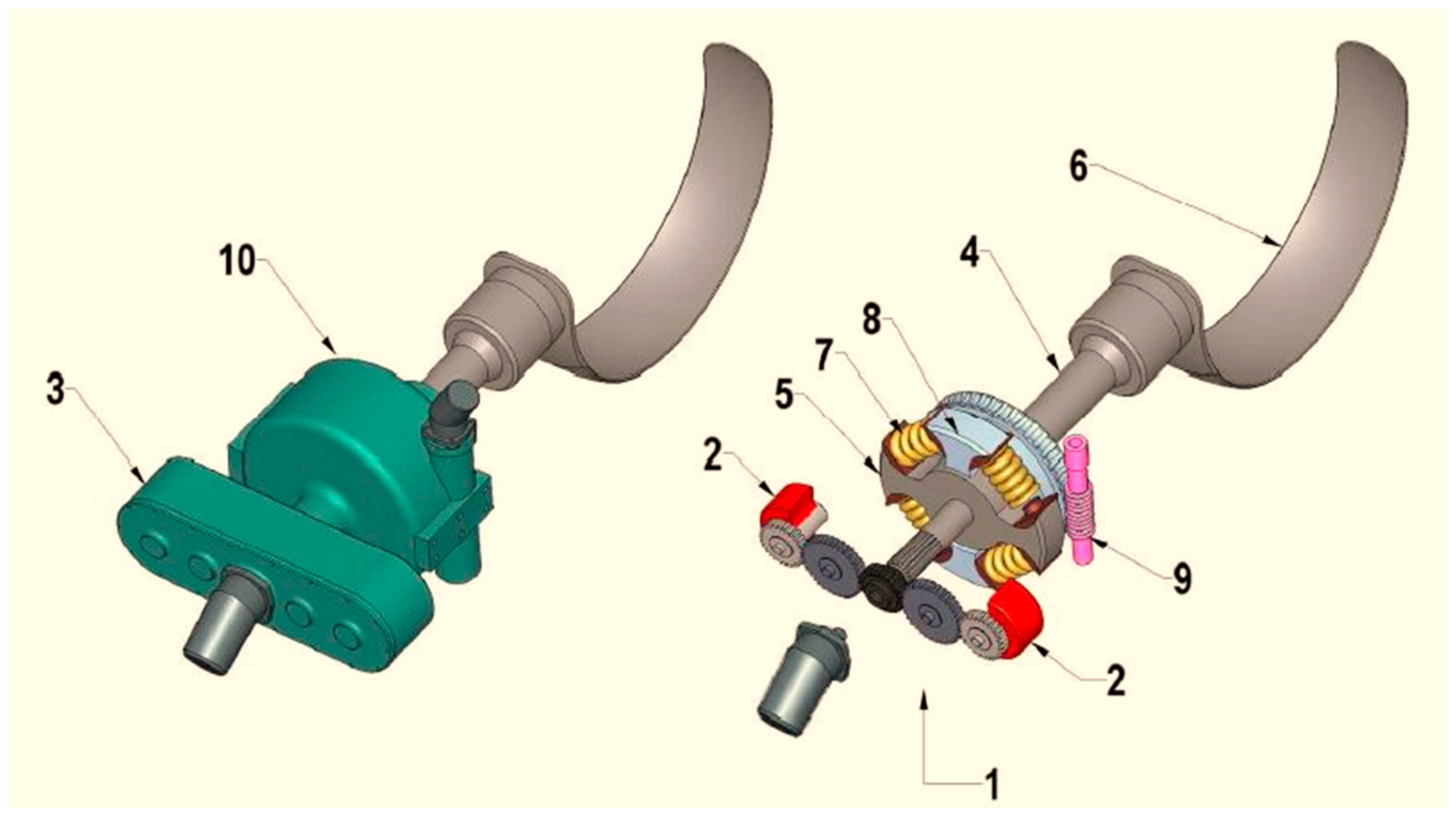
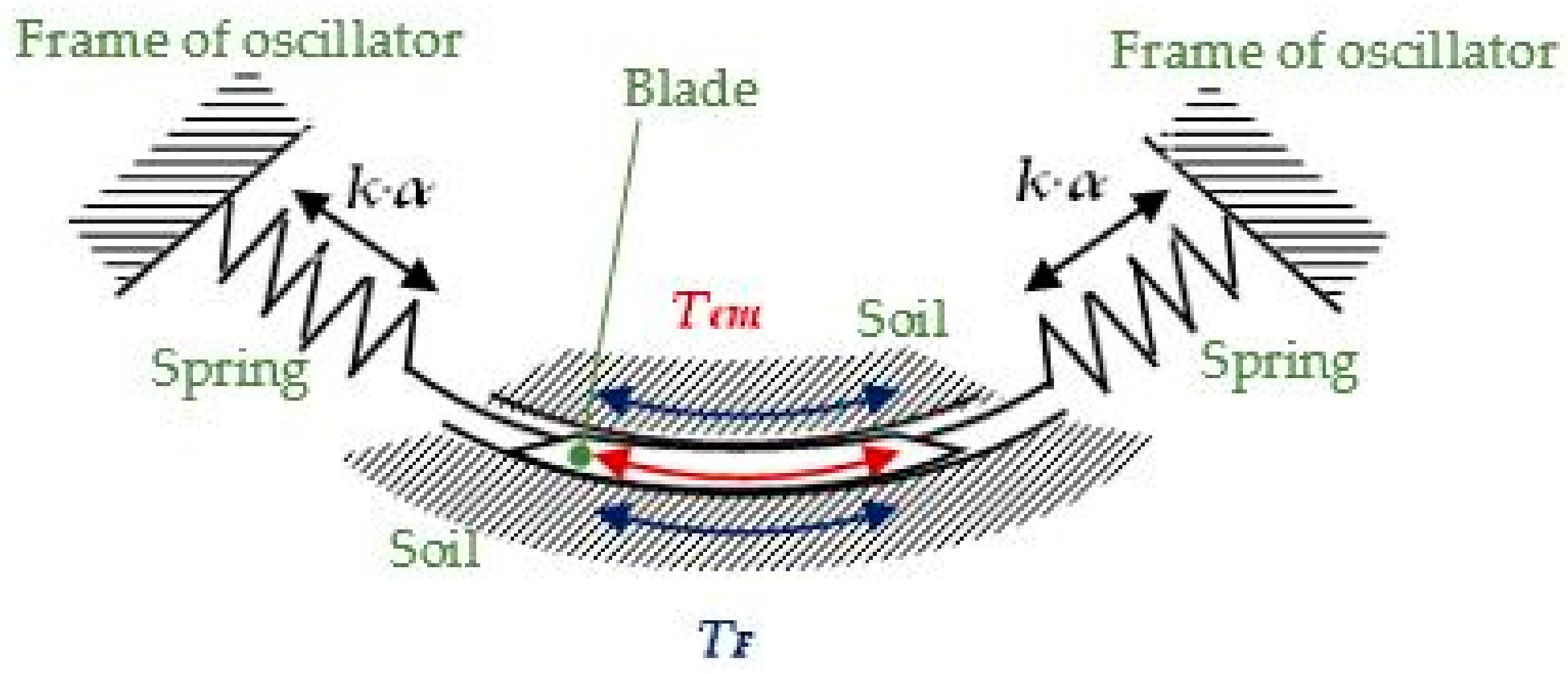
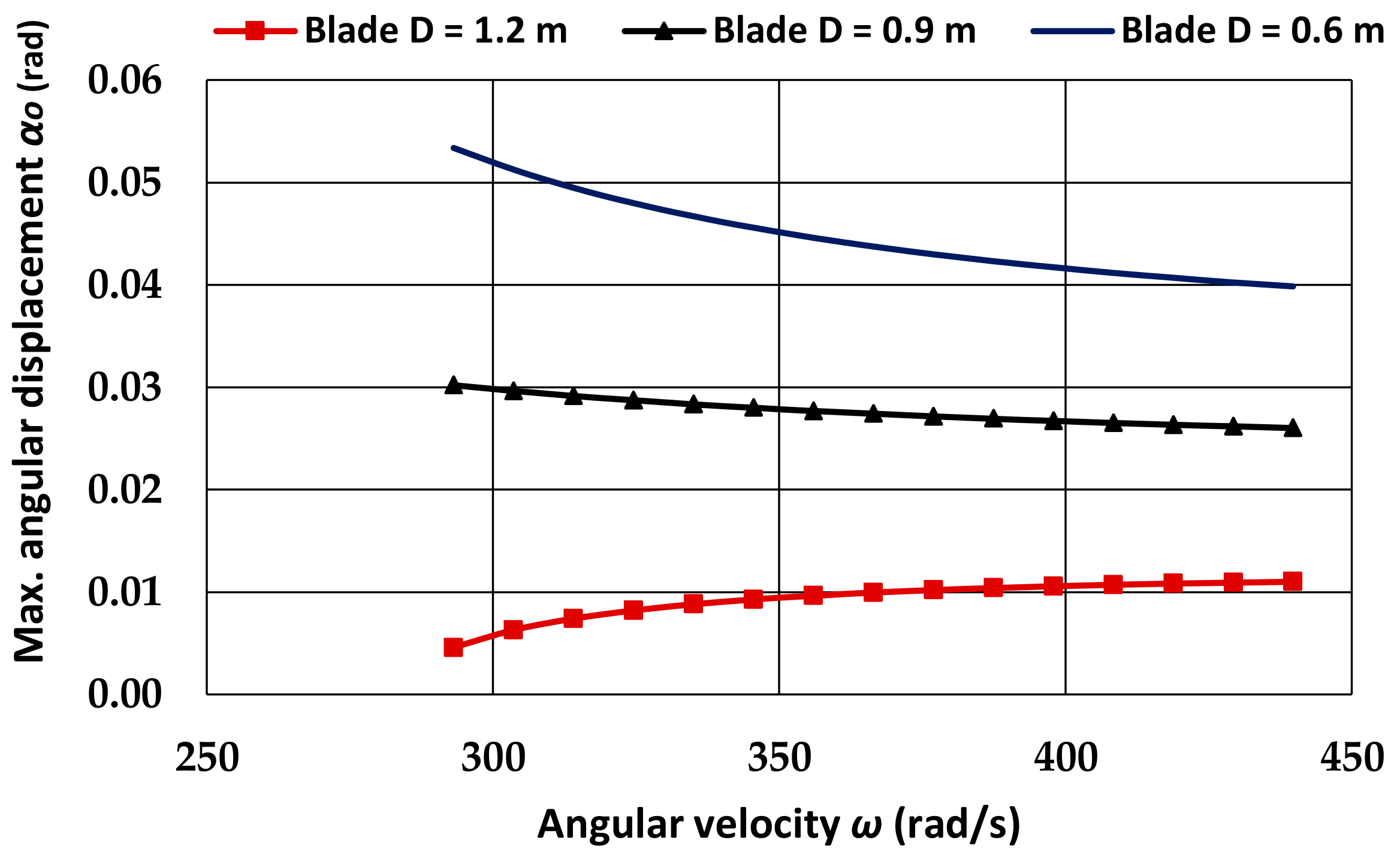
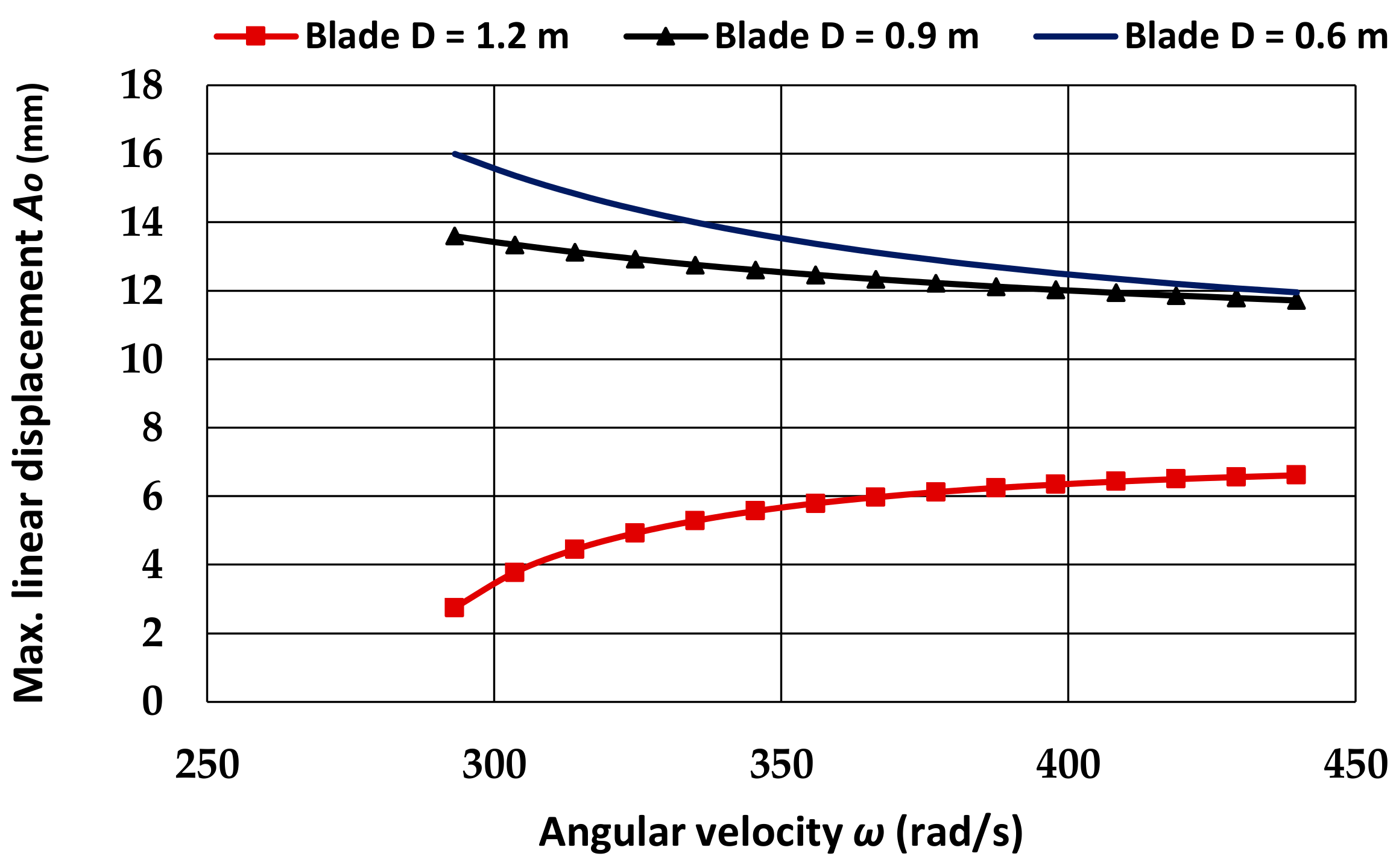
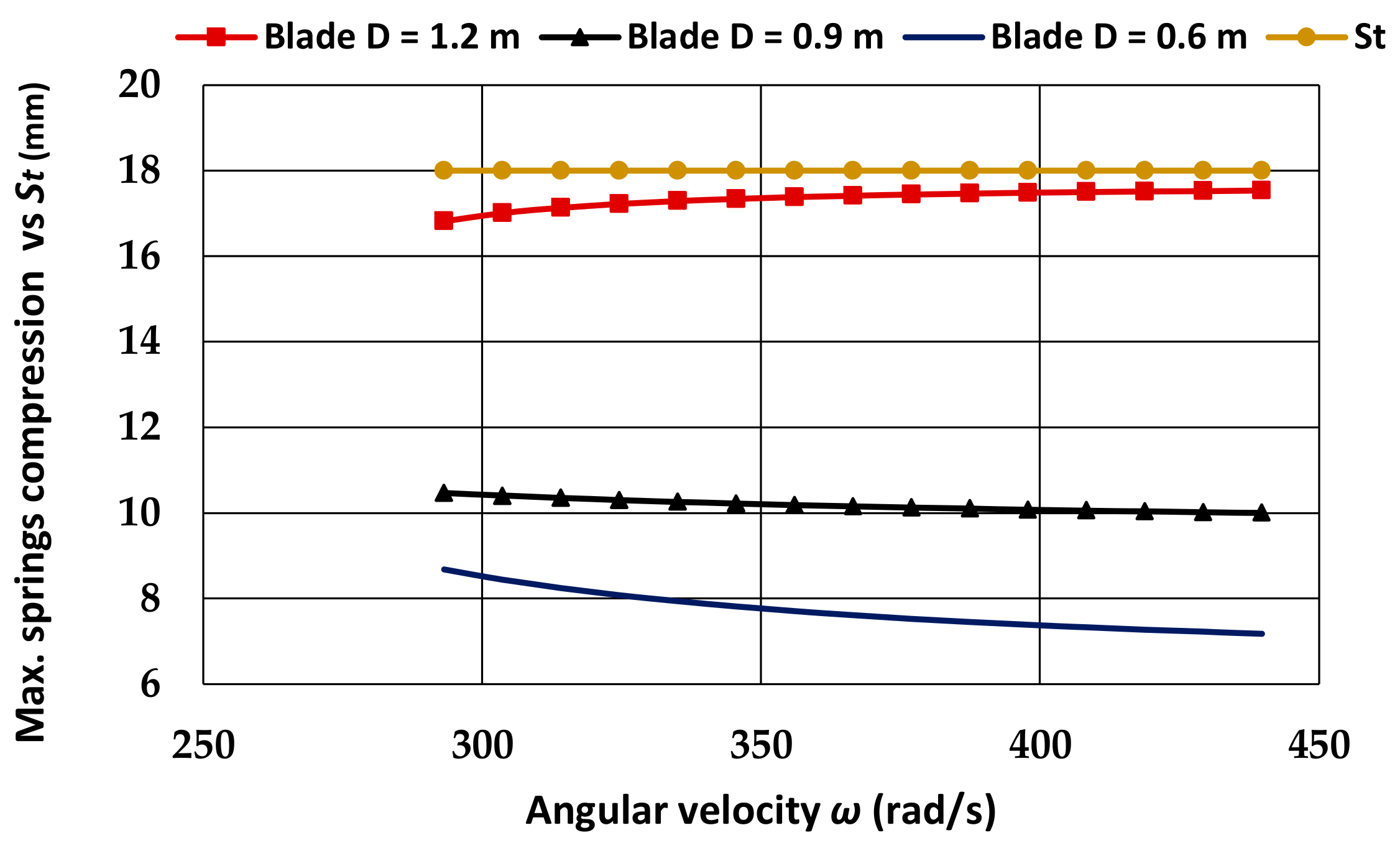
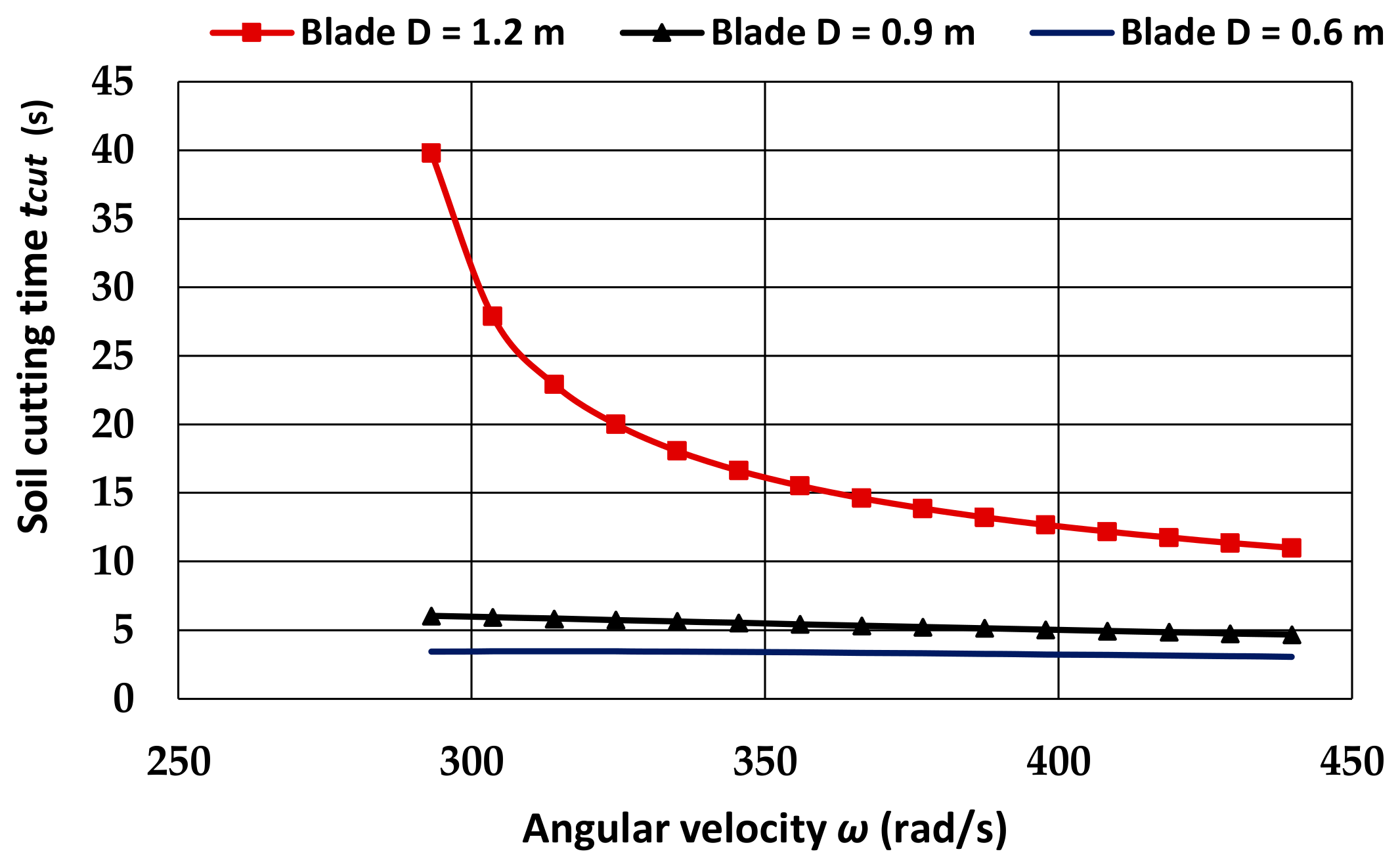
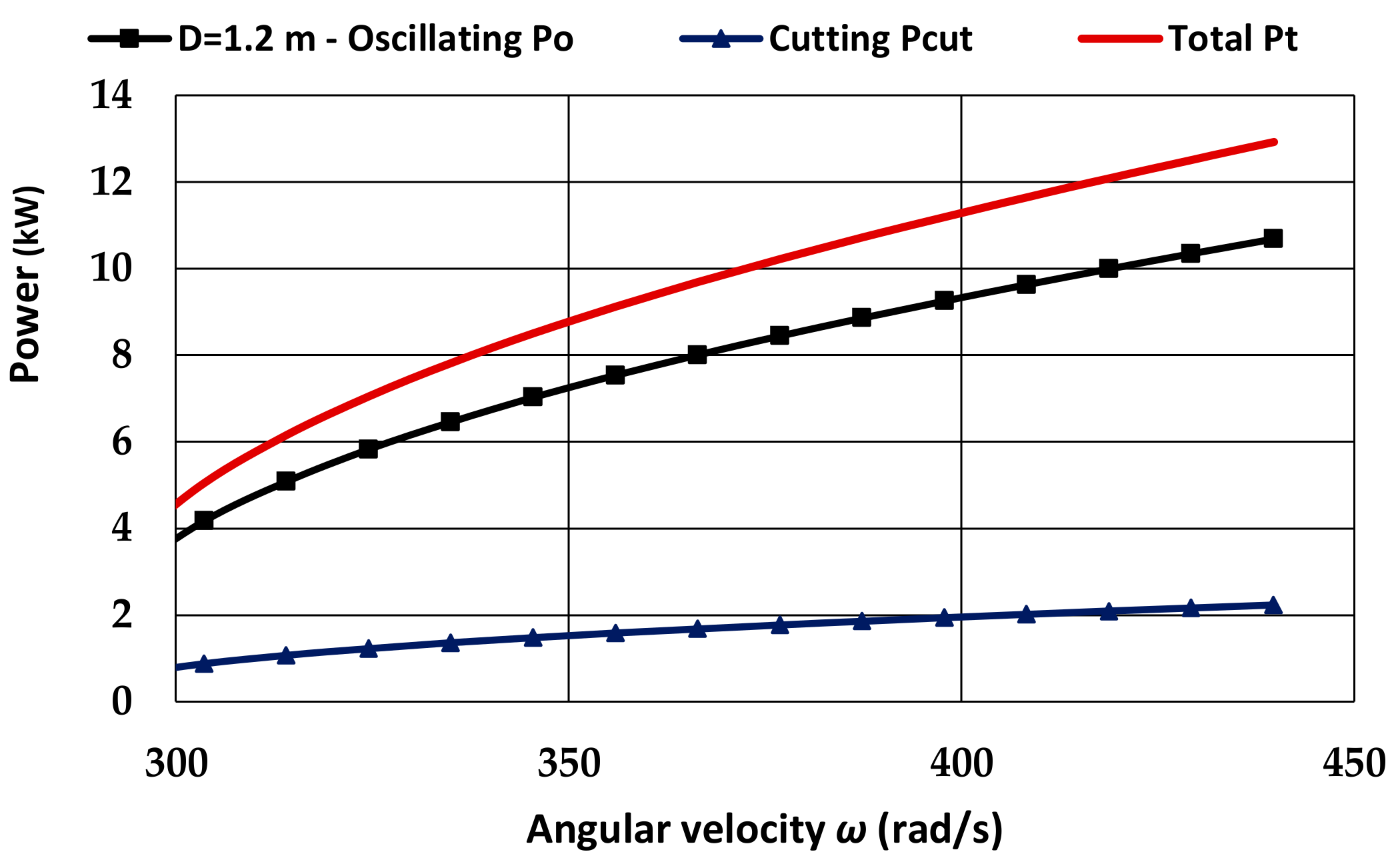
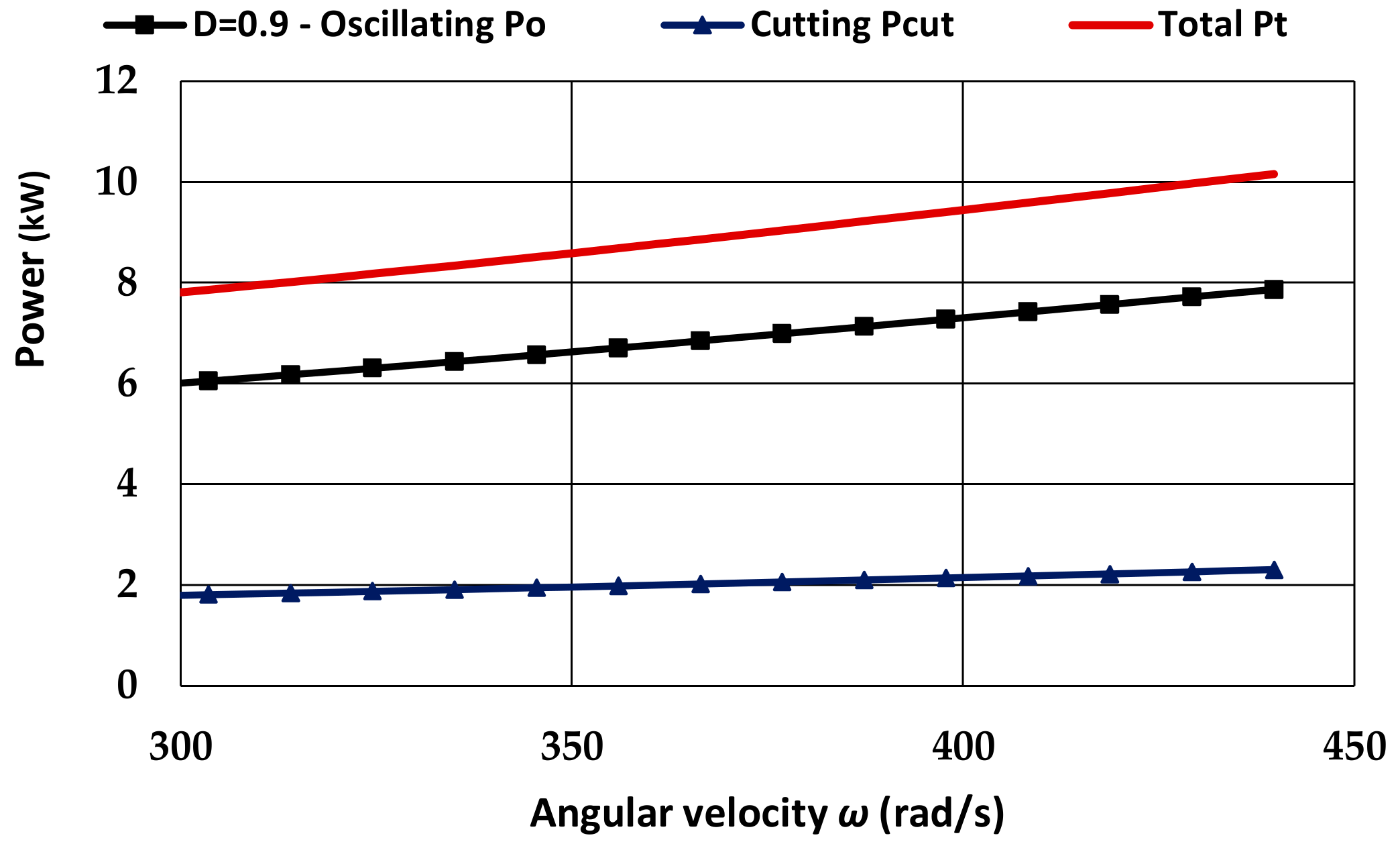
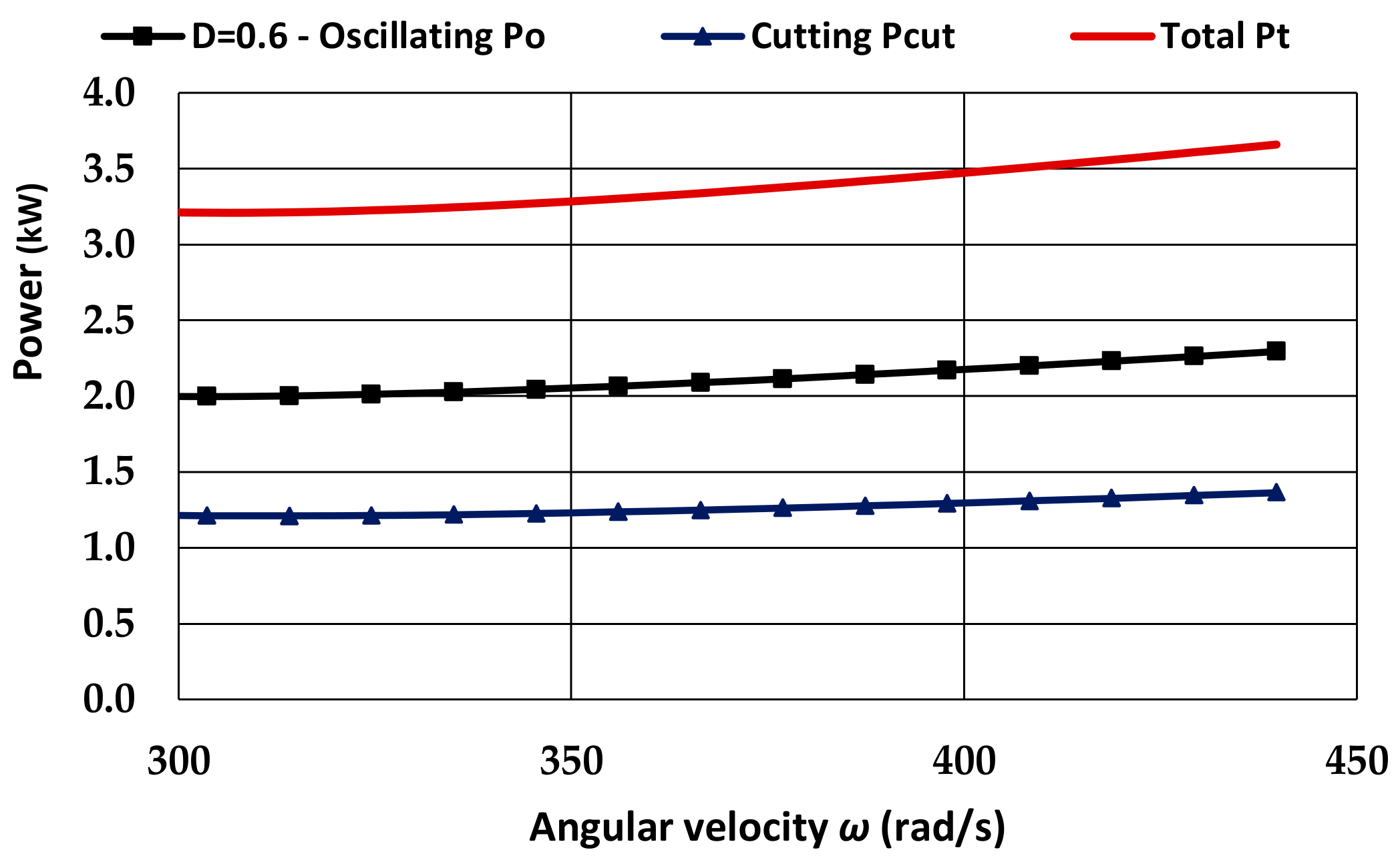
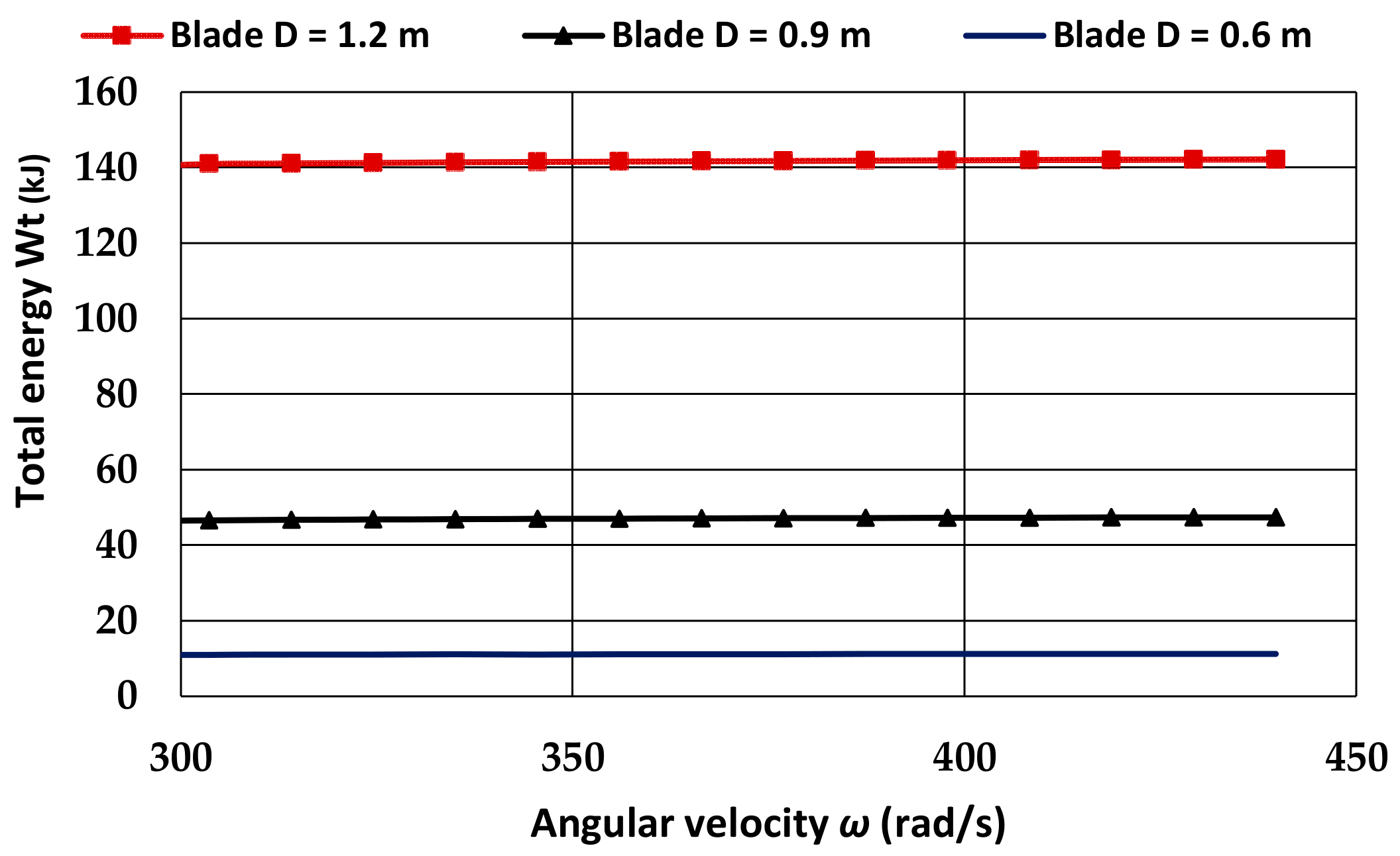
Quantity	Symbol	Oscillator Old	Oscillator New
Linear spring rate	kl (N m−1)	572,000	1,140,000
Number of springs	z	4	4
Lever arm of the springs	bS (m)	0.087	0.108
Torsional springs rate	k (N m rad−1)	17,318	53,227
Number of masses	n	4	4
Mass	m (kg)	1.27	2.41
Eccentricity	yG (m)	0.0212	0.028
Mass lever arm	bG (m)	0.163	0.200
Moment of inertia	JO (kg m2)	0.38	1.30

, the geometric and dynamic data of this new oscillator are shown together with those of the old oscillator.

Table 2. Geometric and dynamic features of the blades and systems (blades + oscillator).

Quantity	Symbol	Values
Blade Diameter	D (m)	0.6	0.9	1.2
Moment of inertia (blade)	JB (kg m2)	0.32	1.02	3.40
Moment of inertia (oscillator)	JO (kg m2)	1.30	1.30	1.30
Moment of inertia (system b+o)	J (kg m2)	1.62	2.32	4.70
Natural frequency	ωn (rad s−1)	181.2	151.4	106.4

shows the data relating to the blades coupled with the new oscillator.

Through the data of Table 1 and Table 2, using Equations (2), (3), (5), (7), and (8), the values of the maximum angular displacement α₀ and, respectively, of the phase angle ϕ were obtained (Figure 5 and Figure 6) with respect to the frequency ω of the excitation torque T_em.

Furthermore, through the expression $A_0={\alpha }_0·D/2$, the maximum linear displacement, reported in Figure 7, was derived. This figure shows that, for blade diameters of 0.6 and 0.9 m, the displacement A₀ fully satisfies the condition: $A_0\ge 6 \mathrm{mm}$, while for the 1.2 m blade diameter, the excitation frequency ω must be greater than 360 rad s⁻¹.

Considering the values of the cutting torque T_cut supplied by Equation (14), the inequality (9) was used to construct the graph in Figure 8, which indicates that, for all the diameters of the blade and for all the frequencies analyzed, the oscillator does not carry the springs to pack with $S_t=\left(\frac{T_{cut}}{k}+{\alpha }_0\right)·b_S$, and it therefore does not present the dangerous condition in which there is a transmission of vibrations to the machine and the operator.

Using Equation (10), the diagram in Figure 9 was created, which shows the soil cutting time t_cut as a function of the excitation frequency ω. For the two blades of 0.6 and 0.9 m, the times t_cut are very short, signaling an excellent machine performance, while for the 1.2 m blade and for ω < 360 rad s⁻¹, the time t_cut is greater than 15 seconds, rapidly rising to values that greatly reduce the productivity of the machine—one more reason to operate with ω > 360 rad s⁻¹.

Figure 10, Figure 11 and Figure 12 show the theoretical powers (i.e., net of transmission efficiencies) required for the operation, respectively, with the three blade diameters: 1.2, 0.9, and 0.6 m. Three curves are shown in each diagram: the power required to push the blade into the soil while cutting, calculated with Equation (12); the power required for the oscillation, calculated with Equation (11); and the total power, the sum of the previous two. For example, it is interesting to note that the power required to push the blade for cutting the soil is approximately four times less than that required by the oscillation in the case of the 0.9 and 1.2 m blades. Furthermore, going from a blade diameter of 0.6 m to 1.2 m, the total power increases by approximately three times.

Finally, Figure 13 shows the theoretical total energy W_t (i.e., net of transmission efficiencies) required for the operation, respectively, for blade diameters of 1.2, 0.9, and 0.6 m. The total energy was calculated with Equation (13), and is the sum of the energy required to push the blade into the soil while cutting W_cut and the energy required for the oscillation W_o. From Equation (13), the energy W_cut is constant as a function of ω. By contrast, the energy required for the oscillation W_o appears nearly constant vs. ω. In fact [36], it is $W_o=P_o·t_{cut}=\frac{1}{2}·T_{em}·17\pi ·\cos \left(\varphi -\frac{\pi }{2}\right)$, where the excitation torque T_em depends directly on ω², while cos(ϕ − π/2) is almost inversely proportional to ω² in the range of ω values considered. Therefore, the total energy required is also substantially invariant with respect to ω.

The choice of the best excitation frequency ω value must be made between the minimum limit of 360 rad s⁻¹ to have the maximum linear displacement of the blade $A_0\ge 6 \mathrm{mm}$ (Figure 7) and the maximum limit of 420 rad s⁻¹, which depends on the resistance of the blade and the oscillator materials. Considering that the total energy required practically does not depend on ω, the optimal value between these limits depends only on two factors: (1) the cutting time t_cut, which must be minimized, maximizing ω (Figure 9); and (2) the total power required P_t, to be minimized by minimizing ω (Figure 10, Figure 11 and Figure 12). Since even in the most onerous condition with the 1.2 m blade diameter, the difference in total theoretical power P_t required between 360 and 420 rad s⁻¹ is just +3.2 kW, it is advisable to operate at maximum ω, given that in this way, a reduction of 25% in the cutting time t_cut is obtained.

After having obtained these positive results using the mathematical modeling equations described in Section 2.2, Section 2.3, Section 2.4 and Section 2.5, experimental tests were carried out to determine the cutting torque and the maximum displacement with the new oscillator coupled to the three blades, with diameters of 0.6, 0.9, and 1.2 m.

Table 3. Experimental values of the cutting torque T_cut using the tree digger machine with the new oscillator operating at an excitation frequency of ω = 420 rad s⁻¹.

Quantity	Symbol	Values
Blade Diameter	D (m)	0.6	0.9	1.2
Cutting torque	Tcut (N m)	1268	3190	6324
Standard deviation	S.D. (N m)	89	182	286
Friction torque	TF (N m)	176	892	2819

shows the experimental results obtained regarding the cutting torque. The friction torque calculated via Equation (5), with the experimental values of the external friction coefficient and the density of the soil (Section 2.6), is also shown.

The comparison between the cutting torque obtained and that of the previous tests [11], considering that the soil was the same with the only difference being in the moisture content (21.5% now and 20.2% then), appears interesting. Therefore, using Equation (14) of the previous tests, the values of the cutting torque are similar for the diameters of 0.6 m and 0.9 m, while a difference of –19% appears for D = 1.2 m. In fact, the previous tests were carried out with the old oscillator (Table 1), which produced, with D = 1.2 m, A₀ < 6 mm, and which transmitted vibrations to the machine frame. Thus, the lower cutting torque with the new oscillator is evidence of its optimized design based on Den Hartog’s equations.

The results of the tests on the cutting torque T_cut with the new oscillator as a function of the blade diameter D can be summarized through the following regression equation:

$$T_{cut}=6733.3·D^2-3693.3·D+1060$$

(15)

The maximum angular displacements α₀ calculated with the Den Hartog Equation (7) and those measured with the procedure seen in Section 2.6 are compared in

Table 4. Calculated and measured maximum displacements using the tree digger machine with the new oscillator operating at the excitation frequency ω = 420 rad s⁻¹.

	Quantity	Symbol	Values
	Blade Diameter	D (m)	0.6	0.9	1.2
Calculated	Max. angular displacement	α0 (rad)	0.0407	0.0264	0.0108
	Max. linear displacement	A0 (mm)	12.2	11.9	6.5
Measured	Max. angular displacement	α0 (rad)	0.0396	0.0253	0.0101
	Max. linear displacement	A0 (mm)	11.9	11.4	6.1

. For all diameters, the experimental value of α₀ is lower, with a difference of 2.7%, 4.2% and 6.4%, respectively, for D equal to 0.6, 0.9, and 1.2 m. Consequently, the experimental values are lower than those also calculated for the maximum linear displacements. These differences can be explained by the damping due to the lubricant inside the oscillator and probably also by an underestimation of the friction torque T_F of Equation (5). However, these errors are acceptable, and seem not to be attributable to the approximate theory of Den Hartog.

4. Conclusions

The tree digger machine uses a semicircular vibrating blade to cut a clod of soil containing the roots of trees grown in tree nurseries, which must then be transported and planted in orchards or gardens. In a previous work [30], the mechanical oscillator coupled to the vibrating blade was analyzed, and its non-optimal performance was highlighted, especially if coupled to large blades of 0.9 m and 1.2 m in diameter. Two facts emerged: (1) the oscillator did not allow the blade to reach the optimum value of maximum linear displacement to reduce the cutting torque as much as possible, and (2) for D = 1.2 m, the oscillator transmitted vibrations to the machine and therefore to the operator.

To understand the origin of these negative phenomena and to realize a better mechanical oscillator, the approximate solution method of the differential equation of forced vibration under dry friction proposed by Den Hartog [34] was applied. The equations with regard to the linear oscillating motion proposed by this author were adapted to the oscillating angular motion of the blade, leading to the correct design of the new oscillator so that it can cut root balls with diameters as large as 1.2 m without the problems of the old oscillator.

More specifically, using the modified equations of Den Hartog, a simulation of the behavior of the system, composed of the blade and the new oscillator, allowed for the calculation of the displacement; the relationship between the maximum oscillation velocity of the blade and the cutting velocity; the required power; and the required energy. The simulation highlighted that the new oscillator, to overcome the problems of the old one, had to have a substantial tripling of the torsional spring rate k, partly obtained by doubling the linear spring rate k_l and partly with the 50% increase in the lever arm of the springs b_s. Furthermore, the equations highlighted that the oscillator also had to have a substantial tripling of the maximum excitation torque. Based on Equation (3), this need imposed a substantial doubling of the eccentric masses and an increase in both the eccentricity of the masses and their lever arm, respectively, by approximately 30%. Finally, the simulation showed that the optimal value of the excitation frequency ω is the maximum value allowed by the resistance of the blade and the oscillator materials (i.e., 420 rad s⁻¹).

After the construction of this optimized oscillator, experimental tests were carried out in cutting the soil. The results substantially confirmed what was predicted by the mathematical modeling based on Den Hartog’s equations with a maximum error of 6.4%.

In future, to compete with the tree digger machine with spades in digging the largest trees, there is a need to make blades with diameters greater than 1.2 m (even up to 1.8 m), for which the problem of transmitting vibrations to the machine and to the operator will probably re-occur due to reaching the end of stroke of the springs during the cut. A possible solution to eliminate this risk is the adoption of springs in the oscillator with an elastic force dependent on the square of the displacement $F=k·x^2$; however, this will make the oscillator anharmonic. Therefore, a differential equation of vibrating motion solution distinct from that of Den Hartog will have to be studied.