Vibration Transmission across Seismically Damaged Beam-to-Column Junctions of Reinforced Concrete Using Statistical Energy Analysis
Abstract
1. Introduction

2. Materials and Methods
2.1. Beam-to-Column Junctions
2.2. Finite Element Modelling
2.3. Monte Carlo Simulation for ESEA
2.4. Experimental Statistical Energy Analysis
2.5. T-Junctions—Wave Approach: Bending Waves Only
3. Results and Discussion
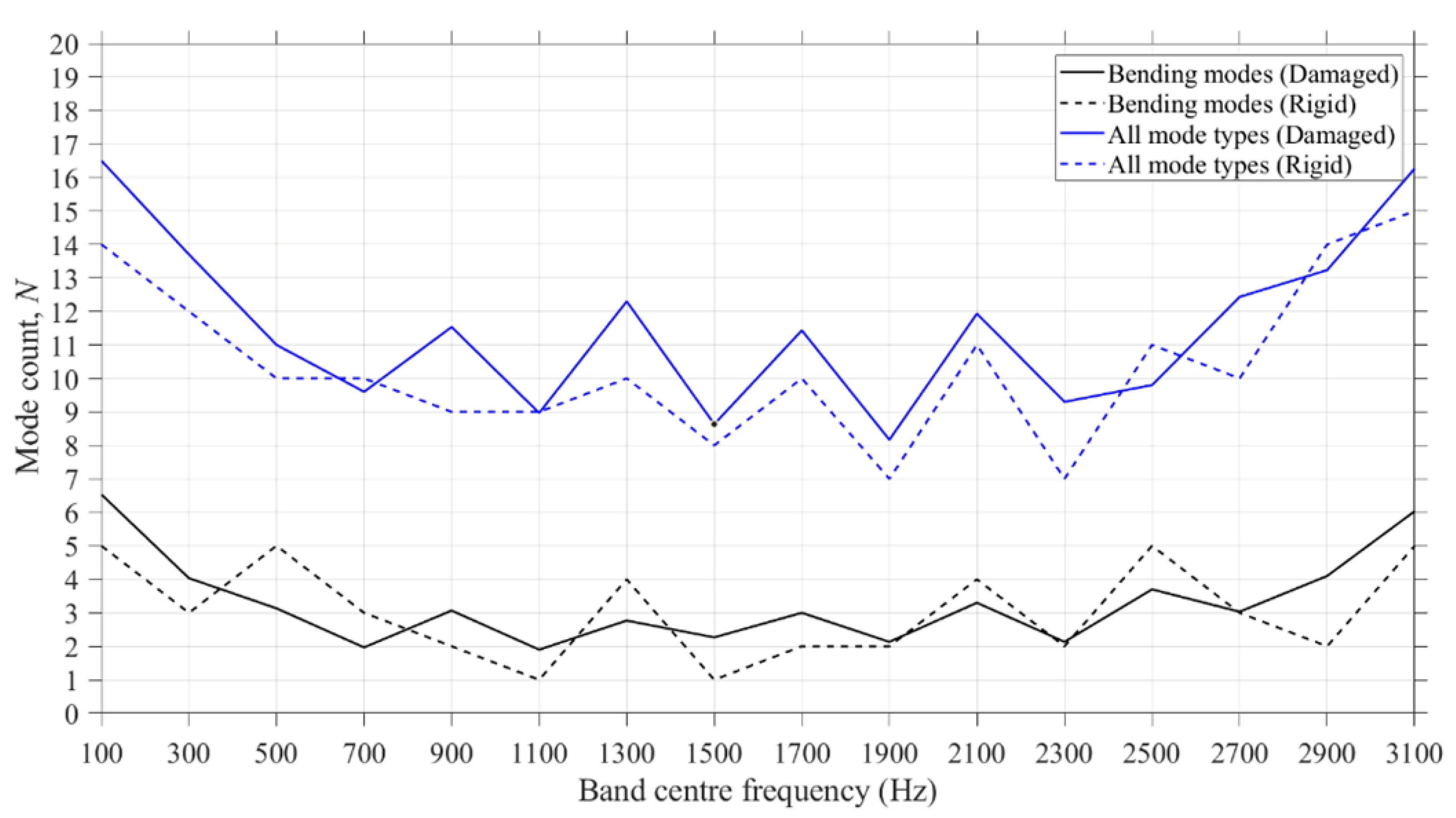
3.1. Mode Count and Modal Overlap
3.2. Comparison of Coupling Loss Factors from FEM ESEA and Wave Approach for the Undamaged, Rigid T-Junction
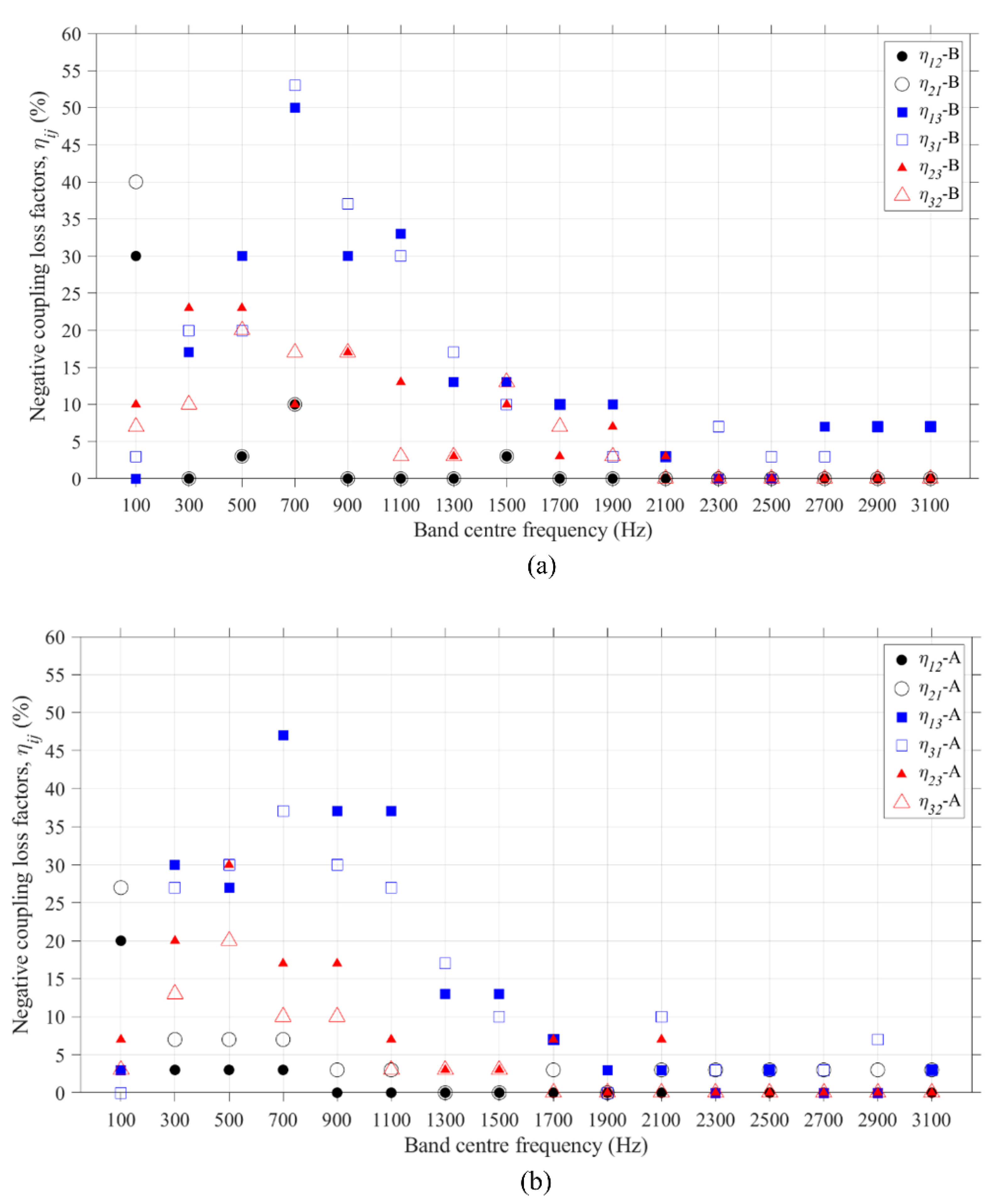
3.3. Coupling Loss Factors from FEM ESEA for Damaged and Rigid T-Junctions
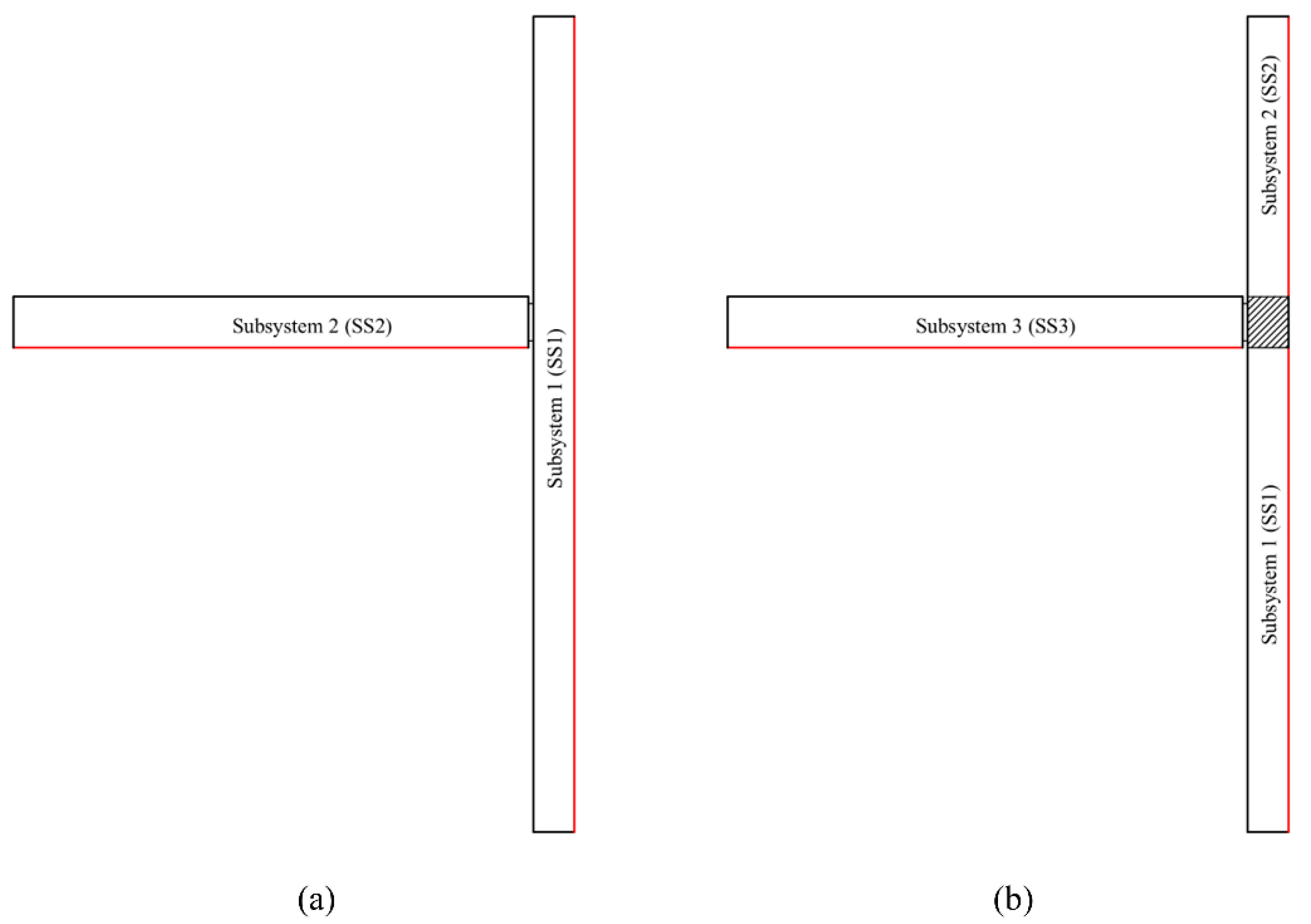
3.3.1. Two Subsystems
3.3.2. Three Subsystems
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Huo, R.; Agapiou, A.; Bocos-Bintintant, V.; Brown, L.J.; Burns, C.; Creaser, C.S.; Devenport, N.A.; Gao-Lau, B.; Guallar-Hoyas, C.; Hildebrand, L.; et al. The trapped human experiment. J. Breath Res. 2011, 5, 046006. [Google Scholar] [CrossRef]
- Macintyre, A.G.; Barbera, J.A.; Smith, E.R. Surviving collapsed structure entrapment after earthquakes: A “Time-to-Rescue” analysis. Prehospital Disaster Med. 2006, 21, 4–19. [Google Scholar] [CrossRef] [PubMed]
- Macintyre, A.G.; Barbera, J.A.; Petinaux, B.P. Survival interval in earthquake entrapments: Research findings reinforced during the 2010 Haiti earthquake Response. Disaster Med. Public Health Prep. 2011, 5, 13–22. [Google Scholar] [CrossRef] [PubMed]
- Bäckström, C.J.; Christofferson, N. Urban Search and Rescue—An Evaluation of Technical Search Equipment and Methods; Lund University: Lund, Sweden, 2006. [Google Scholar]
- Filippoupolitis, M.; Hopkins, C. Experimental validation of finite element models representing stacked concrete beams with unbonded surface contacts. Eng. Struct. 2021, 227, 111421. [Google Scholar] [CrossRef]
- NFPA 1670:2017; Standard on Operations and Training for Technical Search and Rescue Incidents. National Fire Protection Association: Quincy, MA, USA.
- O’Connell, J. Collapse Operations for First Responders, 1st ed.; Penn Well Corporation: Tulsa, OK, USA, 2012. [Google Scholar]
- Schweier, C.; Markus, M. Classification of collapsed buildings for fast damage and loss assessment. Bull. Earthq. Eng. 2006, 4, 177–192. [Google Scholar] [CrossRef]
- Sezen, H.; Whittaker, A.S.; Elwood, K.J.; Mosalam, K.M. Performance of reinforced concrete buildings during the August 17, 1999 Kocaeli, Turkey earthquake, and seismic design and construction practice in Turkey. Eng. Struct. 2003, 25, 103–114. [Google Scholar] [CrossRef]
- Lyon, R.H.; DeJong, R.J. Theory and Application of Statistical Energy Analysis, 2nd ed.; Butterworth-Heinemann: Newton, MA, USA, 1995. [Google Scholar]
- Hopkins, C. Vibration transmission between coupled plates using finite element methods and statistical energy analysis. Part 2: The effect of window apertures in masonry flanking walls. Appl. Acoust. 2003, 64, 975–997. [Google Scholar] [CrossRef]
- Hopkins, C. Statistical energy analysis of coupled plate systems with low modal density and low modal overlap. J. Sound Vib. 2002, 251, 193–214. [Google Scholar] [CrossRef]
- Hopkins, C. Experimental statistical energy analysis of coupled plates with wave conversion at the junction. J. Sound Vib. 2009, 322, 155–166. [Google Scholar] [CrossRef]
- Marshal, J.D.; Lang, A.F.; Baldridge, S.M.; Popp, D.R. Recipe for disaster: Construction methods, materials, and building performance in the January 2010 Haiti earthquake. Earthq. Spectra 2011, 27, 323–343. [Google Scholar] [CrossRef]
- Abaqus 6.14 Documentation and User Manual; Dassault Systèmes Simulia Corporation: Providence, RI, USA, 2014.
- Filippoupolitis, M.; Hopkins, C. Experimental validation of finite element models for reinforced concrete beams with discontinuities that form dowelled-type joints. Vibration 2021, 4, 537–550. [Google Scholar] [CrossRef]
- Atalla, N.; Sgard, F. Finite Element and Boundary Methods on Structural Acoustics and Vibration; CRC Press: Boca Raton, FL, USA; Taylor & Francis Group: Abingdon, UK, 2015. [Google Scholar]
- Hopkins, C. Sound Insulation; Butterworth-Heinemann: Oxford, UK, 2007. [Google Scholar]
- Bies, D.A.; Hamid, S. In situ determination of loss and coupling loss factors by the power injection method. J. Sound Vib. 1980, 70, 187–204. [Google Scholar] [CrossRef]
- Woodhouse, J. An introduction to statistical energy analysis of structural vibration. Appl. Acoust. 1981, 14, 455–469. [Google Scholar] [CrossRef]
- Hodges, C.H.; Nash, P.; Woodhouse, J. Measurement of coupling loss factors by matrix fitting: An investigation of numerical procedures. Appl. Acoust. 1987, 22, 47–69. [Google Scholar] [CrossRef]
- De las Heras, M.F.; Manguán, M.C.; Millán, E.R.; de las Heras, L.F.; Hidalgo, F.S. Determination of SEA loss factors by Monte Carlo Filtering. J. Sound Vib. 2020, 479, 115348. [Google Scholar] [CrossRef]
- Poblet-Puig, J. Estimation of the coupling loss factors of structural junctions with in-plane waves by means of the inverse statistical energy analysis problem. J. Sound Vib. 2021, 493, 115850. [Google Scholar]
- Lalor, N. Practical Considerations for the Measurement of Internal and Coupling Loss Factors on Complex Structures, ISVR Technical Report No. 182. June 1990.
- Jang, H.; Hopkins, C. Prediction of sound transmission in long spaces using ray tracing and experimental Statistical Energy Analysis. Appl. Acoust. 2018, 130, 15–33. [Google Scholar]
- Cremer, L.; Heckl, M.; Ungar, E.E. Structure-Borne Sound; Springer: New York, NY, USA, 1973. [Google Scholar]
- Wang, X.; Hopkins, C. Bending, longitudinal and torsional wave transmission on Euler-Bernoulli and Timoshenko beams with high propagation losses. J. Acoust. Soc. Am. 2016, 140, 2312–2332. [Google Scholar] [PubMed]
- Craik, R.J.M. Sound Transmission Through Buildings: Using Statistical Energy Analysis; Gower Publishing Limited: Hampshire, UK, 1996. [Google Scholar]
- Fahy, F.J.; Mohammed, A.D. A study of uncertainty in applications of SEA to coupled beam and plate systems. Part 1: Computational experiments. J. Sound Vib. 1992, 158, 45–67. [Google Scholar] [CrossRef]
- Renji, K. On the number of modes required for statistical energy analysis-based calculations. J. Sound Vib. 2004, 269, 1128–1132. [Google Scholar] [CrossRef]
- Wang, X.; Hopkins, C. Experimental and numerical validation of Advanced Statistical Energy Analysis to incorporate tunneling mechanisms for vibration transmission across a grillage of beams. Acta Acust. 2022, 6, 51. [Google Scholar] [CrossRef]

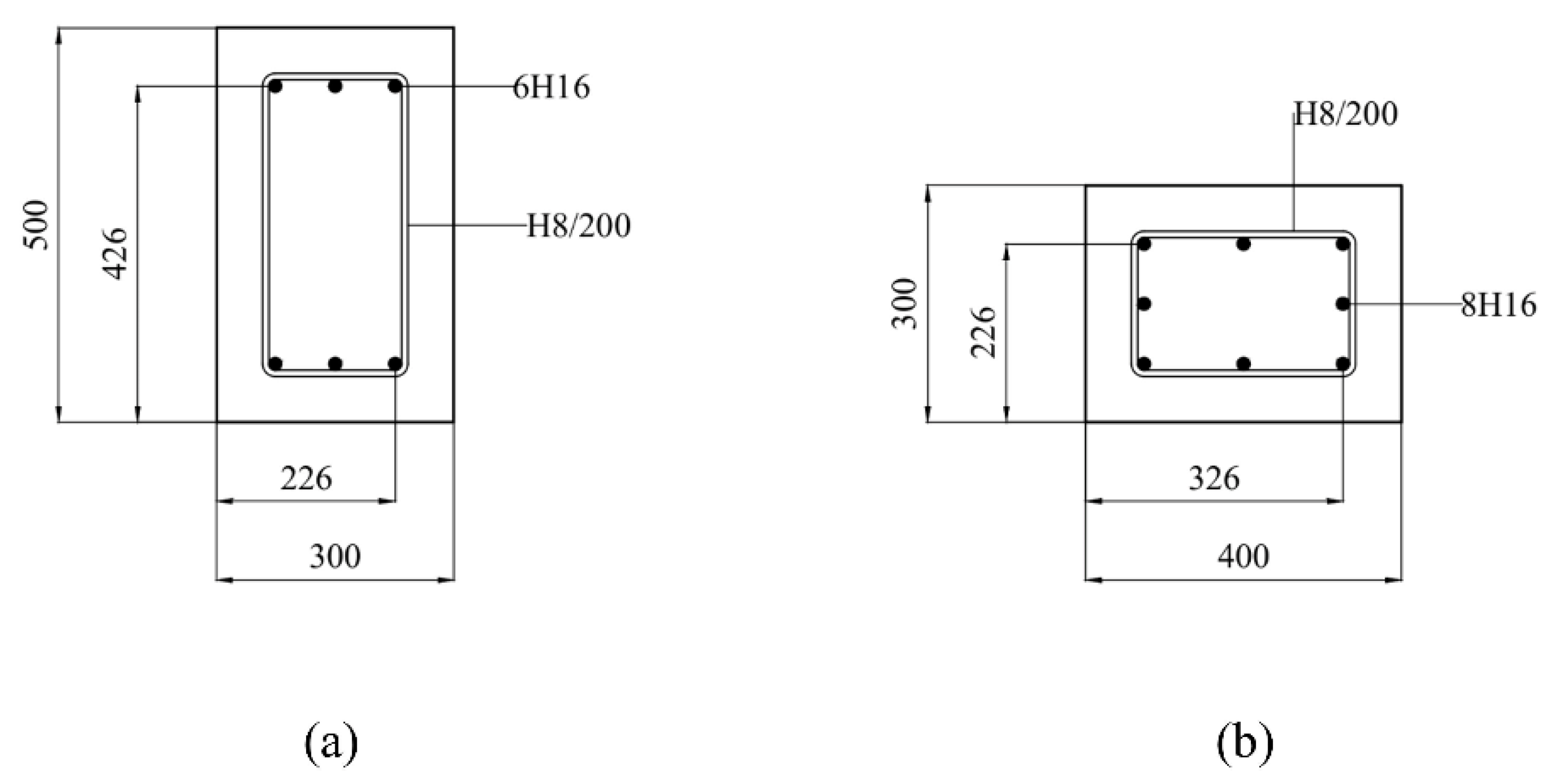






| Material | Density, ρ [kg/m3] | Young’s Modulus, E [N/m2] | Poisson’s Ratio, ν [–] |
|---|---|---|---|
| Concrete | 2287 | 34.7 × 109 | 0.2 |
| Steel | 7800 | 200 × 109 | 0.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Filippoupolitis, M.; Hopkins, C. Vibration Transmission across Seismically Damaged Beam-to-Column Junctions of Reinforced Concrete Using Statistical Energy Analysis. Vibration 2023, 6, 149-164. https://doi.org/10.3390/vibration6010011
Filippoupolitis M, Hopkins C. Vibration Transmission across Seismically Damaged Beam-to-Column Junctions of Reinforced Concrete Using Statistical Energy Analysis. Vibration. 2023; 6(1):149-164. https://doi.org/10.3390/vibration6010011
Chicago/Turabian StyleFilippoupolitis, Marios, and Carl Hopkins. 2023. "Vibration Transmission across Seismically Damaged Beam-to-Column Junctions of Reinforced Concrete Using Statistical Energy Analysis" Vibration 6, no. 1: 149-164. https://doi.org/10.3390/vibration6010011
APA StyleFilippoupolitis, M., & Hopkins, C. (2023). Vibration Transmission across Seismically Damaged Beam-to-Column Junctions of Reinforced Concrete Using Statistical Energy Analysis. Vibration, 6(1), 149-164. https://doi.org/10.3390/vibration6010011






