Fully Coupled Vibrations of Cable-Harnessed Beams with a Non-Periodic Wrapping Pattern
Abstract
:1. Introduction
2. Mathematical Modeling
2.1. Derivation of Strain and Kinetic Energies
- The cable will be in pre-tension during harnessing and remains in tension during structural vibrations.
- The cable pre-tension, T, results in a pre-compressive load on the beam.
- The cable remains attached to the beam during vibrations; friction between the beam and cable is ignored.
- The cable strains are the same as that of the top fiber of the beam.
2.2. Solution to the Coupled Partial Differential Equations
3. Results and Discussion
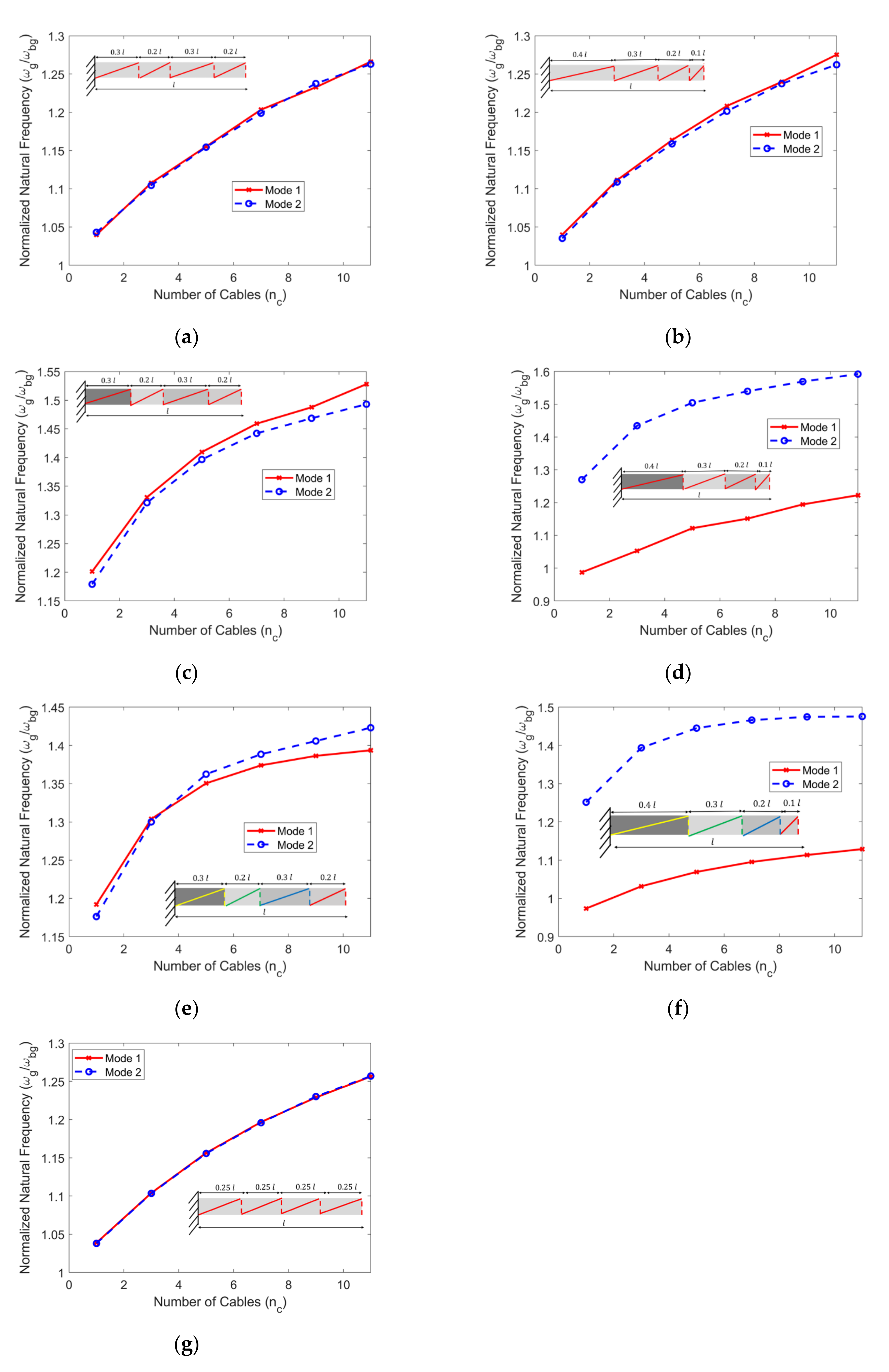
3.1. Sensitivity Analysis for Homogenous and Non-Homogenous Cable-Harnessed Structures
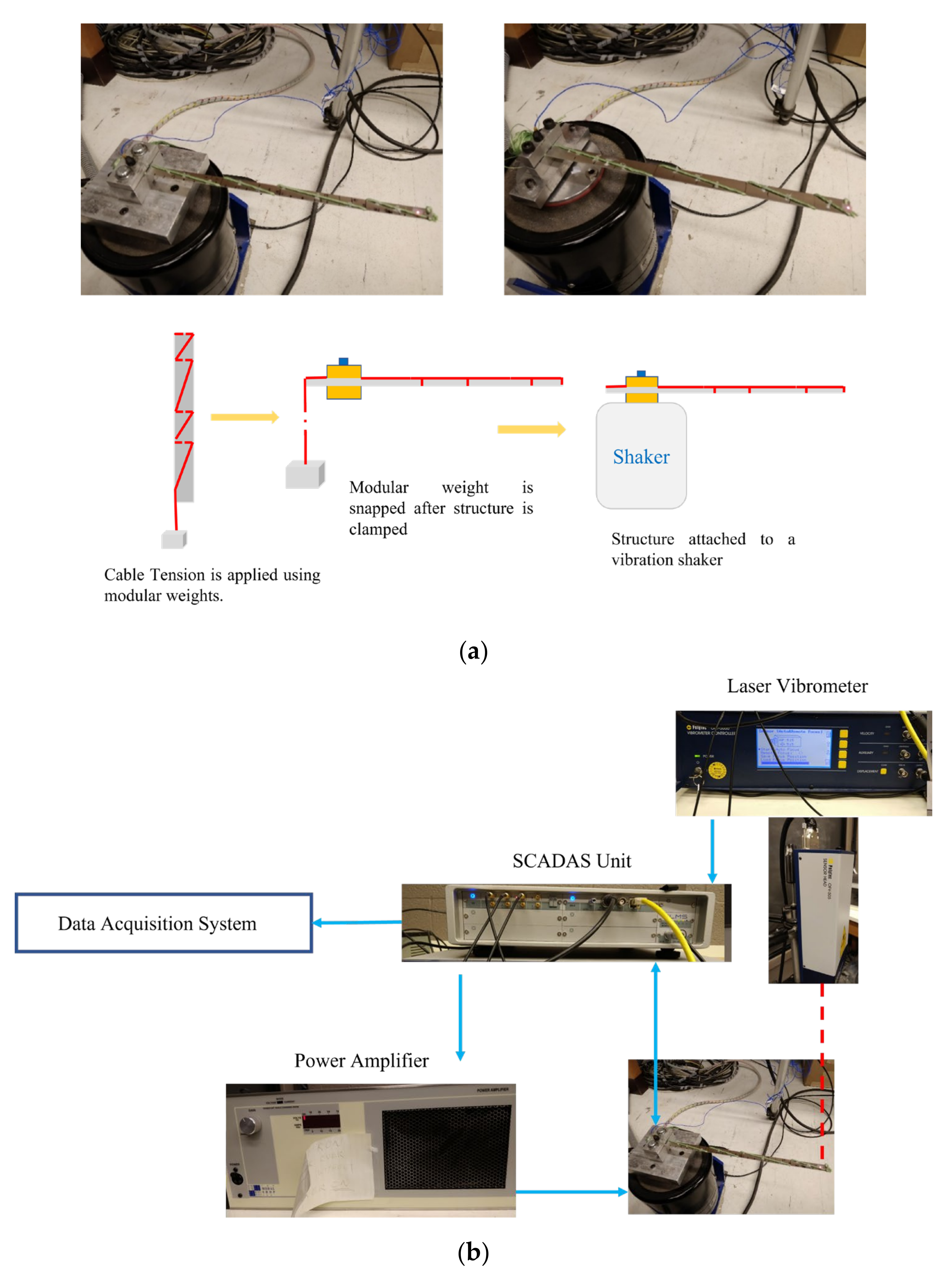
3.2. Experimental Setup
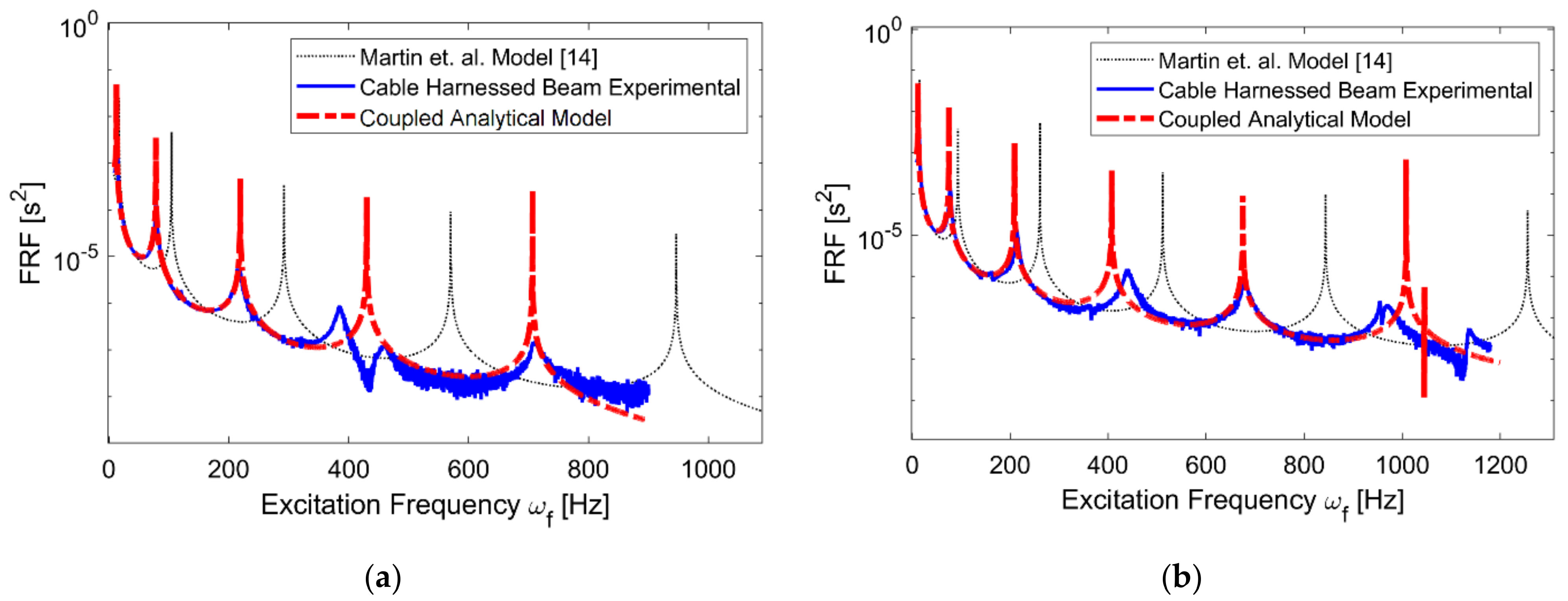
3.3. FRF Comparison between Theory and Experiment
3.4. Curvature Analysis and Mismatched Modes
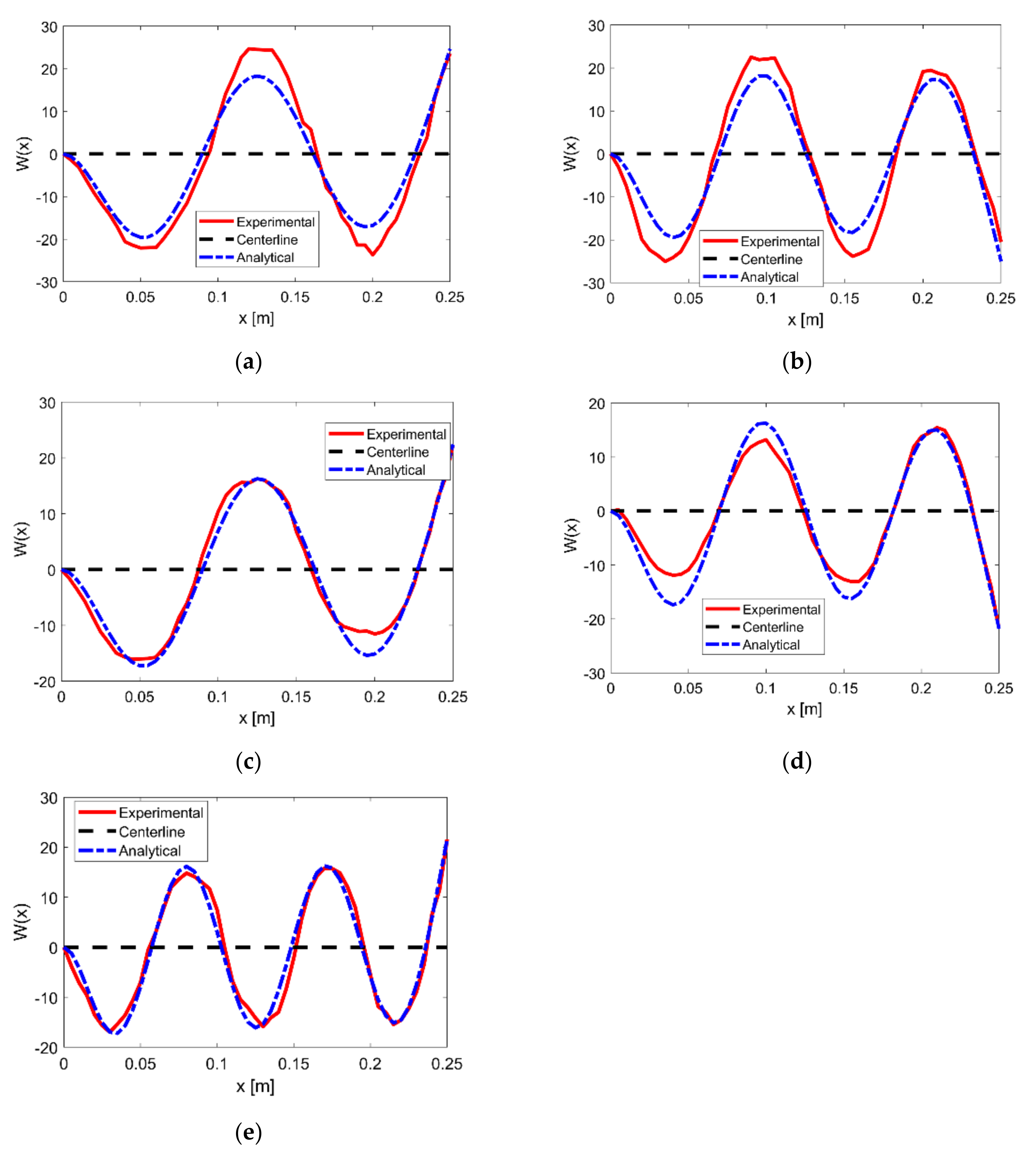
3.5. In-Plane-Bending- and Torsion-Dominant Experimental Mode Shapes
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Cross-sectional area of the beam and the cable | |
| Width of the beam | |
| Thickness of the beam | |
| Strain energy coefficients for Euler–Bernoulli based model of ith fundamental element | |
| Strain energy coefficients for the constant coefficient model of ith fundamental element | |
| Kinetic energy coefficients of ith fundamental element | |
| Kinetic energy coefficients of the constant coefficient model of ith fundamental element | |
| Length of the beam | |
| Number of cables | |
| Radius of the cable | |
| Pre-tension of the cables | |
| Base excitation | |
| , | Actuation and sensing location |
| , | y and z coordinates of the cable where strains are evaluated |
| Wrapping angle of ith element | |
| Excitation frequency | |
| Natural frequency of the mode for cable-harnessed beam | |
| Natural frequency of the mode for beam with no cable | |
| Length of ith fundamental element | |
| Beam and cable densities of ith fundamental element | |
| Beam and cable Young’s modulus of ith fundamental element | |
| Beam and cable shear modulus of ith fundamental element | |
| Lumped-mass effect due to the horizontal part of the cable in the axial, in-plane bending, out-of-plane bending and torsional directions of ith fundamental element | |
| Displacement components at all points of the body of the beam | |
| Velocity components at all points of the body of the beam |
Appendix A
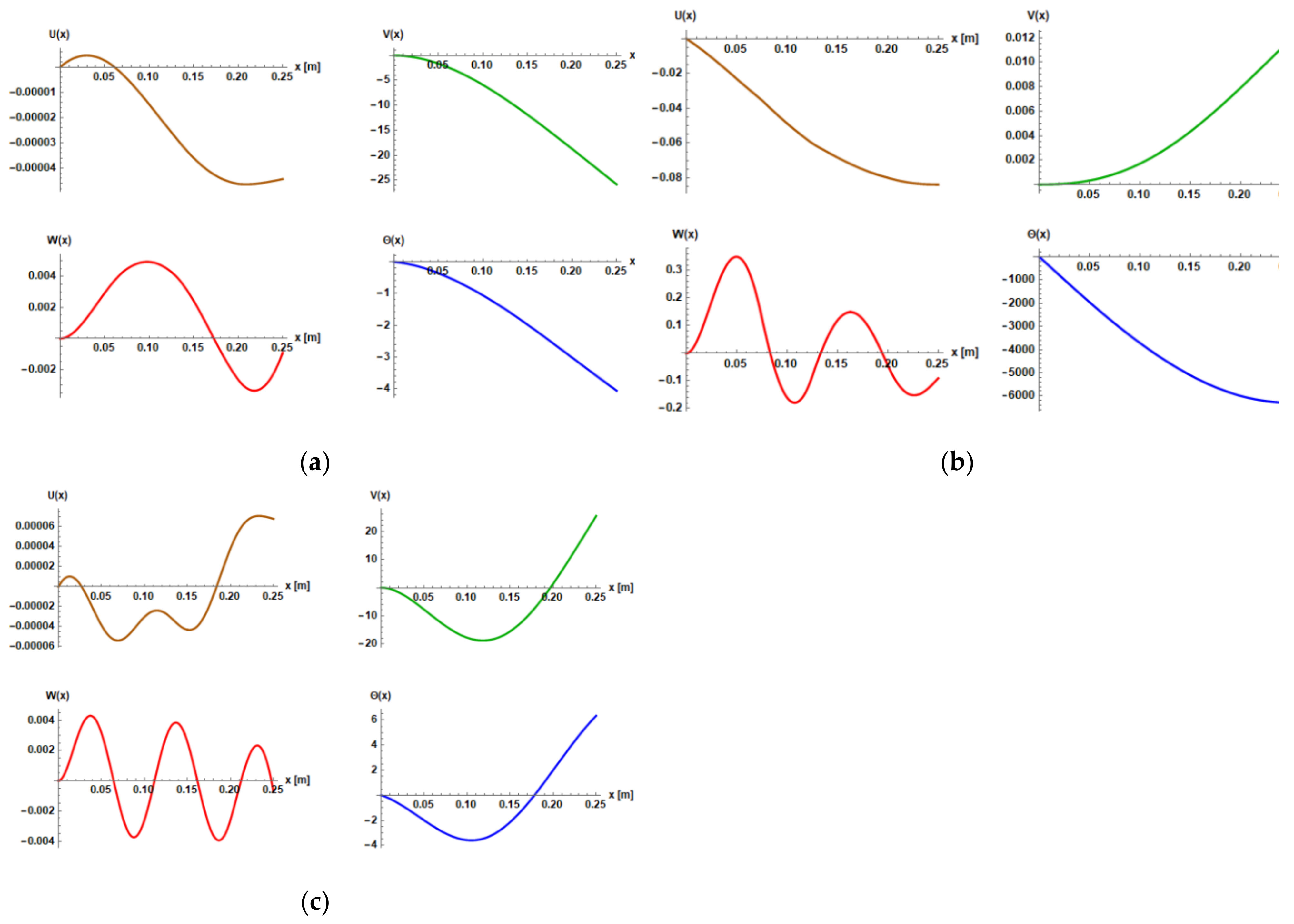


References
- Babuska, V.; Coombs, D.M.; Goodding, J.C.; Ardelean, E.V.; Robertson, L.M.; Lane, S.A. Modeling and experimental validation of space structures with wiring harnesses. J. Spacecr. Rockets 2010, 47, 1038–1052. [Google Scholar] [CrossRef]
- Robertson, L.; Lane, S.; Ingram, B.; Hansen, E.; Babuska, V.; Goodding, J.; Mimovich, M.; Mehle, G.; Coombs, D.; Ardelean, E. Cable effects on the dynamics of large precision structures. In Proceedings of the 48th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Honolulu, HI, USA, 23–26 April 2007; p. 2389. [Google Scholar]
- Goodding, J.C.; Ardelean, E.V.; Babuska, V.; Robertson, L.M., III; Lane, S.A. Experimental techniques and structural parameter estimation studies of spacecraft cables. J. Spacecr. Rockets 2011, 48, 942–957. [Google Scholar] [CrossRef]
- Coombs, D.M.; Goodding, J.C.; Babuška, V.; Ardelean, E.V.; Robertson, L.M.; Lane, S.A. Dynamic modeling and experimental validation of a cable-loaded panel. J. Spacecr. Rockets 2011, 48, 958–974. [Google Scholar] [CrossRef]
- Kauffman, J.L.; Lesieutre, G.A.; Babuška, V. Damping models for shear beams with applications to spacecraft wiring harnesses. J. Spacecr. Rockets 2014, 51, 16–22. [Google Scholar] [CrossRef]
- Kauffman, J.L.; Lesieutre, G.A. Damping models for timoshenko beams with applications to spacecraft wiring harnesses. In Proceedings of the 54th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Boston, MA, USA, 8–11 April 2013; p. 1890. [Google Scholar]
- Spak, K. Modeling Cable Harness Effects on Space Structures. Ph.D. Thesis, Virginia Tech, Blacksburg, VA, USA, 2014. [Google Scholar]
- Spak, K.S.; Agnes, G.S.; Inman, D.J. Bakeout effects on dynamic response of spaceflight cables. J. Spacecr. Rockets 2014, 51, 1721–1734. [Google Scholar] [CrossRef]
- Martin, B.; Salehian, A. Mass and stiffness effects of harnessing cables on structural dynamics: Continuum modeling. AIAA J. 2016, 54, 2881–2904. [Google Scholar] [CrossRef]
- Martin, B.; Salehian, A. Homogenization modeling of periodically wrapped string-harnessed beam structures: Experimental validation. AIAA J. 2016, 54, 3965–3980. [Google Scholar] [CrossRef]
- Agrawal, P.; Salehian, A. Damping Mechanisms in Cable-Harnessed Structures for Space Applications: Analytical Modeling. J. Vib. Acoust. 2020, 143, 021001. [Google Scholar] [CrossRef]
- Yerrapragada, K.; Salehian, A. Coupled dynamics of cable-harnessed structures: Experimental validation. J. Vib. Acoust. Trans. ASME 2019, 141, 061001. [Google Scholar] [CrossRef] [Green Version]
- Yerrapragada, K.; Salehian, A. Analytical Study of Coupling Effects for Vibrations of Cable-Harnessed Beam Structures. J. Vib. Acoust. 2019, 141, 031001. [Google Scholar] [CrossRef] [Green Version]
- Martin, B.; Salehian, A. Continuum Modeling of Nonperiodic String-Harnessed Structures: Perturbation Theory and Experiments. AIAA J. 2019, 57, 1736–1751. [Google Scholar] [CrossRef]
- Banerjee, J.R. Frequency equation and mode shape formulae for composite Timoshenko beams. Compos. Struct. 2001, 51, 381–388. [Google Scholar] [CrossRef]
- Amoozgar, M.; Bodaghi, M.; Ajaj, M.R. The effect of non-conservative compressive force on the vibration of rotating composite blades. Vibration 2020, 3, 478–490. [Google Scholar] [CrossRef]
- Wang, Q.; Wang, Z.; Fan, B. Coupled bending and torsional vibration characteristics analysis of inhomogeneous wind turbine tower with variable cross section under elastic constraint. Appl. Math. Model. 2021, 93, 188–205. [Google Scholar] [CrossRef]
- Mei, C. Effect of material coupling on wave vibration of composite Timoshenko beams. J. Vib. Acoust. Trans. ASME 2005, 127, 333–340. [Google Scholar] [CrossRef]
- Yerrapragada, K.; Salehian, A. Multi-Dimensional Vibrations of Cable-Harnessed Beam Structures with Periodic Pattern: Modeling and Experiment. Shock. Vib. 2022, 2022, 7343582. [Google Scholar]
- Yerrapragada, K. Coupled Dynamics of Cable-Harnessed Structures: Analytical Modeling and Experimental Validation. Ph.D. Thesis, University of Waterloo, Waterloo, ON, Canada, 2019. [Google Scholar]
- Stoykov, S.; Ribeiro, P. Vibration analysis of rotating 3D beams by the p-version finite element method. Finite Elem. Anal. Des. 2013, 65, 76–88. [Google Scholar] [CrossRef]
- Stoykov, S.; Ribeiro, P. Nonlinear forced vibrations and static deformations of 3D beams with rectangular cross section: The influence of warping, shear deformation and longitudinal displacements. Int. J. Mech. Sci. 2010, 52, 1505–1521. [Google Scholar] [CrossRef]
- Rao, S.S. Vibration of Continuous Systems; John Wiley & Sons: Hoboken, NJ, USA, 2019. [Google Scholar]
- Hagedorn, P.; DasGupta, A. Vibrations and Waves in Continuous Mechanical Systems; John Wiley & Sons: West Sussex, UK, 2007. [Google Scholar]
- Karami, M.A.; Inman, D.J. Analytical modeling and experimental verification of the vibrations of the zigzag microstructure for energy harvesting. J. Vib. Acoust. 2011, 133, 11002. [Google Scholar] [CrossRef]
- Ansari, M.H.; Karami, M.A. Energy harvesting from heartbeat using piezoelectric beams with fan-folded configuration and added tip mass. In ASME Smart Materials, Adaptive Structures and Intelligent Systems Conference; ASME: Colorado Springs, CO, USA, 2015; Volume 57304, p. V002T07A020. [Google Scholar]
- Ansari, M.H.; Karami, M.A. Modeling and experimental verification of a fan-folded vibration energy harvester for leadless pacemakers. J. Appl. Phys. 2016, 119, 94506. [Google Scholar] [CrossRef]
- Erturk, A.; Inman, D.J. An experimentally validated bimorph cantilever model for piezoelectric energy harvesting from base excitations. Smart Mater. Struct. 2009, 18, 25009. [Google Scholar] [CrossRef]
- Erturk, A.; Inman, D.J. Piezoelectric Energy Harvesting; John Wiley & Sons: West Sussex, UK, 2011. [Google Scholar]


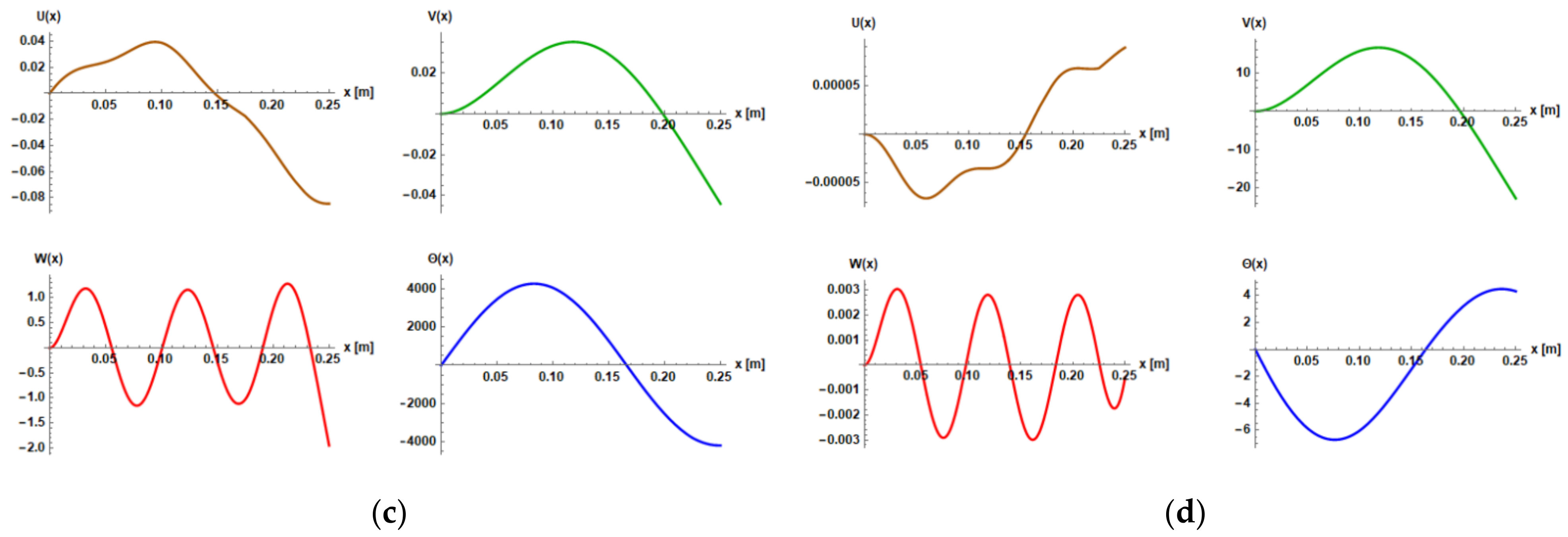
 represents position of lumped-mass section.
represents position of lumped-mass section.
 represents position of lumped-mass section.
represents position of lumped-mass section.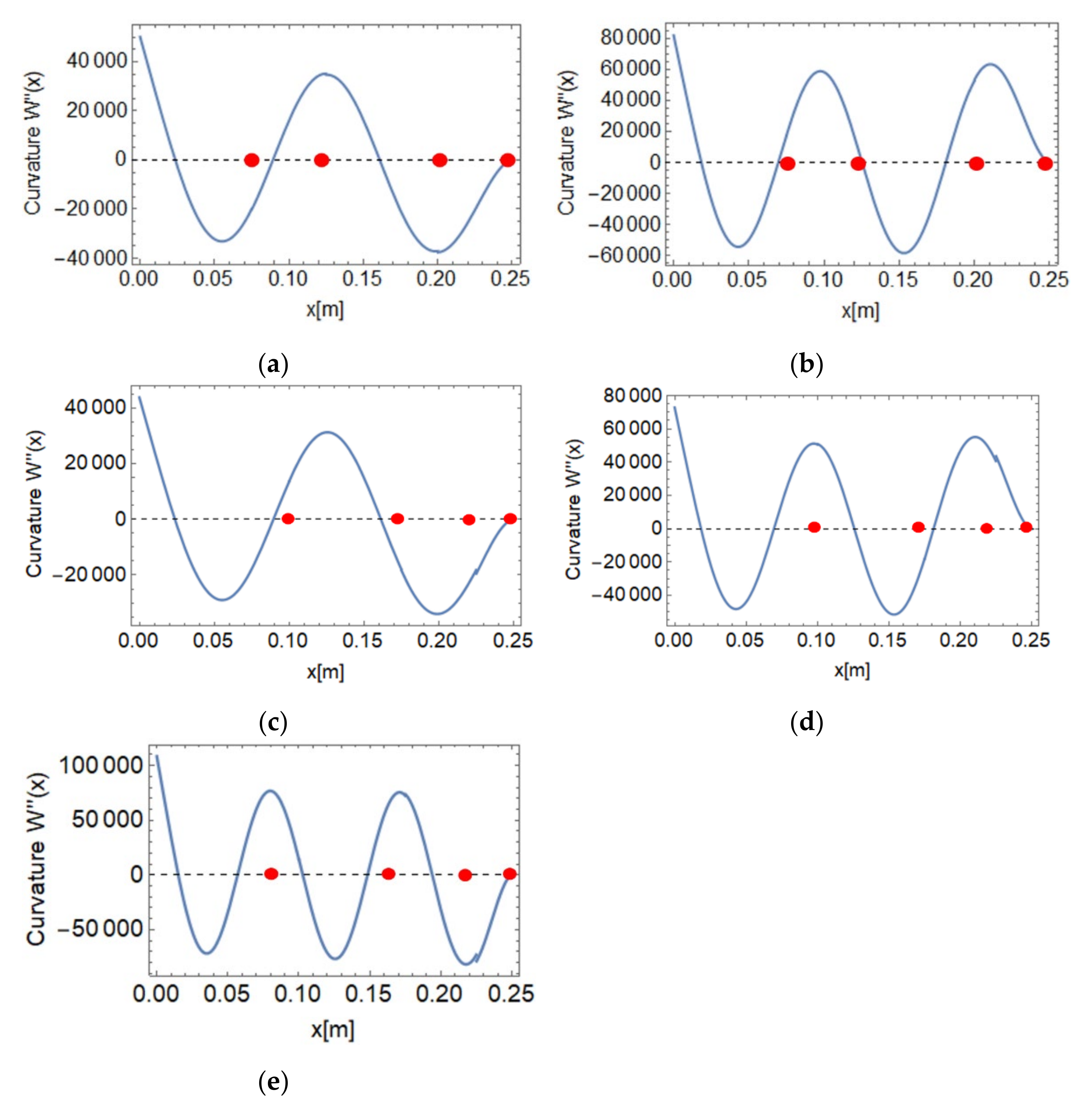

| Parameters | System 1 | System 2 | System 3 |
|---|---|---|---|
| Beam length () [mm] | 250 | 250 | 250 |
| Beam width () [mm] | 10 | 10 | 10 |
| Beam thickness () [mm] | 0.782 | 0.782 | 0.782 |
| No. of fundamental elements | 4 | 4 | 4 |
| Cable radius () [mm] | 0.21 | 0.21 | 0.21 |
| Pre-tension of cables () [N] | 4 | 4 | 4 |
| Density of each beam element [kg/m3] | |||
| 2768 | 2768 | 2768 | |
| 2768 | 7850 | 7850 | |
| 2768 | 1100 | 1100 | |
| 2768 | 8790 | 8790 | |
| Young’s Modulus of each beam element [GPa] | |||
| 68.9 | 68.9 | 68.9 | |
| 68.9 | 210 | 210 | |
| 68.9 | 5 | 5 | |
| 68.9 | 121 | 121 | |
| Shear Modulus of each beam element [GPa] | |||
| 25.7 | 25.7 | 25.7 | |
| 25.7 | 81 | 81 | |
| 25.7 | 1.8 | 1.8 | |
| 25.7 | 43.2 | 43.2 | |
| Density of each cable element [kg/m3] | |||
| 1400 | 1400 | 1400 | |
| 1400 | 1400 | 7850 | |
| 1400 | 1400 | 1400 | |
| 1400 | 1400 | 8790 | |
| Young’s Modulus of each cable element [GPa] | |||
| 128.04 | 128.04 | 128.04 | |
| 128.04 | 128.04 | 210 | |
| 128.04 | 128.04 | 128.04 | |
| 128.04 | 128.04 | 121 |
| System Parameters | Sample 1 | Sample 2 |
|---|---|---|
| Width () [mm] | 10 | 13.1 |
| Thickness () [mm] | 0.782 | 0.782 |
| Length () [mm] | 250 | 250 |
| Number of Cables | 9 | 8 |
| Cable pre-tension () [N] | 14 | 14 |
| Number of fundamental elements () | 4 | 4 |
| Beam density () [kg/m3] | 2768 | 2768 |
| Beam modulus of elasticity () [GPa] | 68.9 | 68.9 |
| Cable radius () [mm] | 0.21 | 0.21 |
| Beam shear modulus () [GPa] | 25.7 | 25.7 |
| Cable density () [kg/m3] | 1400 | 1400 |
| Cable modulus of elasticity () [GPa] | 128.04 | 128.04 |
| Mode No | Experiment [Hz] | Coupled [Hz] | Decoupled [Hz] | % Coupled and Experiment | % Decoupled and Experiment |
|---|---|---|---|---|---|
| 1 | 13.2 (OP) | 12.5 | 16.6 | −5.5% | 26.1% |
| 2 | 78.6 (OP) | 78.2 | 104.2 | −0.5% | 32.5% |
| 3 | 121.0 (IP) | 140.3 | - | 15.9% | - |
| 4 | 215.7 (OP) | 218.9 | 291.9 | 1.5% | 35.3% |
| 5 | 384.8 (OP) | 429.8 | 570.0 | 11.7% | 48.1% |
| 6 | 459.5 (Torsion) | 447.1 | - | −2.7% | - |
| 7 | 709.1 (OP) | 706.4 | 946.3 | −0.4% | 33.4% |
| 8 | 752.8 (IP) | 879.9 | - | 16.9% | - |
| Mode No | Experiment [Hz] | Coupled [Hz] | Decoupled [Hz] | % Coupled and Experiment | % Decoupled and Experiment |
|---|---|---|---|---|---|
| 1 | 13.7 (OP) | 11.9 | 14.9 | −13.1% | 8.9% |
| 2 | 76.9 (OP) | 74.7 | 93.3 | −2.8% | 21.3% |
| 3 | 151.5 (IP) | 179.7 | - | 18.6% | - |
| 4 | 213.4 (OP) | 208.8 | 260.7 | −2.2% | 22.1% |
| 5 | 363.2 (Torsion) | 347.0 | - | −4.5% | - |
| 6 | 438.9 (OP) | 407.4 | 511.5 | −7.2% | 16.5% |
| 7 | 679.0 (OP) | 675.2 | 843.4 | −0.6% | 24.2% |
| 8 | 965.7 (OP) | 1008.1 | 1256.0 | 4.4% | 30.1% |
| 9 | 1137.2 (Torsion) | 1045.3 | - | −8.1% | - |
| 10 | 952.0 (IP) | 1123.5 | - | 18.0% | - |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yerrapragada, K.; Martin, B.; Agrawal, P.; Salehian, A. Fully Coupled Vibrations of Cable-Harnessed Beams with a Non-Periodic Wrapping Pattern. Vibration 2022, 5, 238-263. https://doi.org/10.3390/vibration5020015
Yerrapragada K, Martin B, Agrawal P, Salehian A. Fully Coupled Vibrations of Cable-Harnessed Beams with a Non-Periodic Wrapping Pattern. Vibration. 2022; 5(2):238-263. https://doi.org/10.3390/vibration5020015
Chicago/Turabian StyleYerrapragada, Karthik, Blake Martin, Pranav Agrawal, and Armaghan Salehian. 2022. "Fully Coupled Vibrations of Cable-Harnessed Beams with a Non-Periodic Wrapping Pattern" Vibration 5, no. 2: 238-263. https://doi.org/10.3390/vibration5020015
APA StyleYerrapragada, K., Martin, B., Agrawal, P., & Salehian, A. (2022). Fully Coupled Vibrations of Cable-Harnessed Beams with a Non-Periodic Wrapping Pattern. Vibration, 5(2), 238-263. https://doi.org/10.3390/vibration5020015






