Investigating the Relationship between Coupling Forces and Hand-Transmitted Vibration under Varying Excitation Levels
Abstract
:1. Introduction
2. Methodology
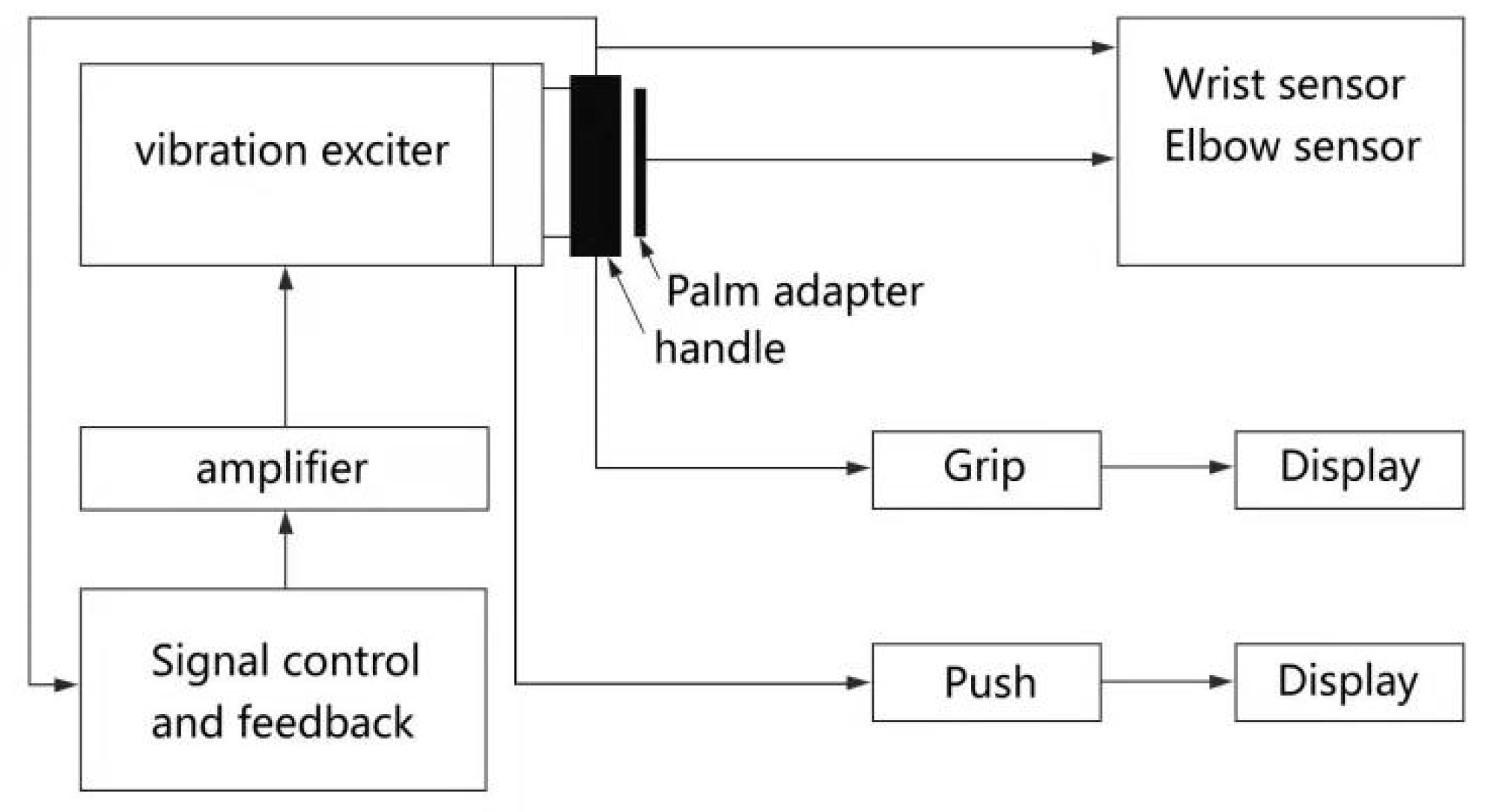
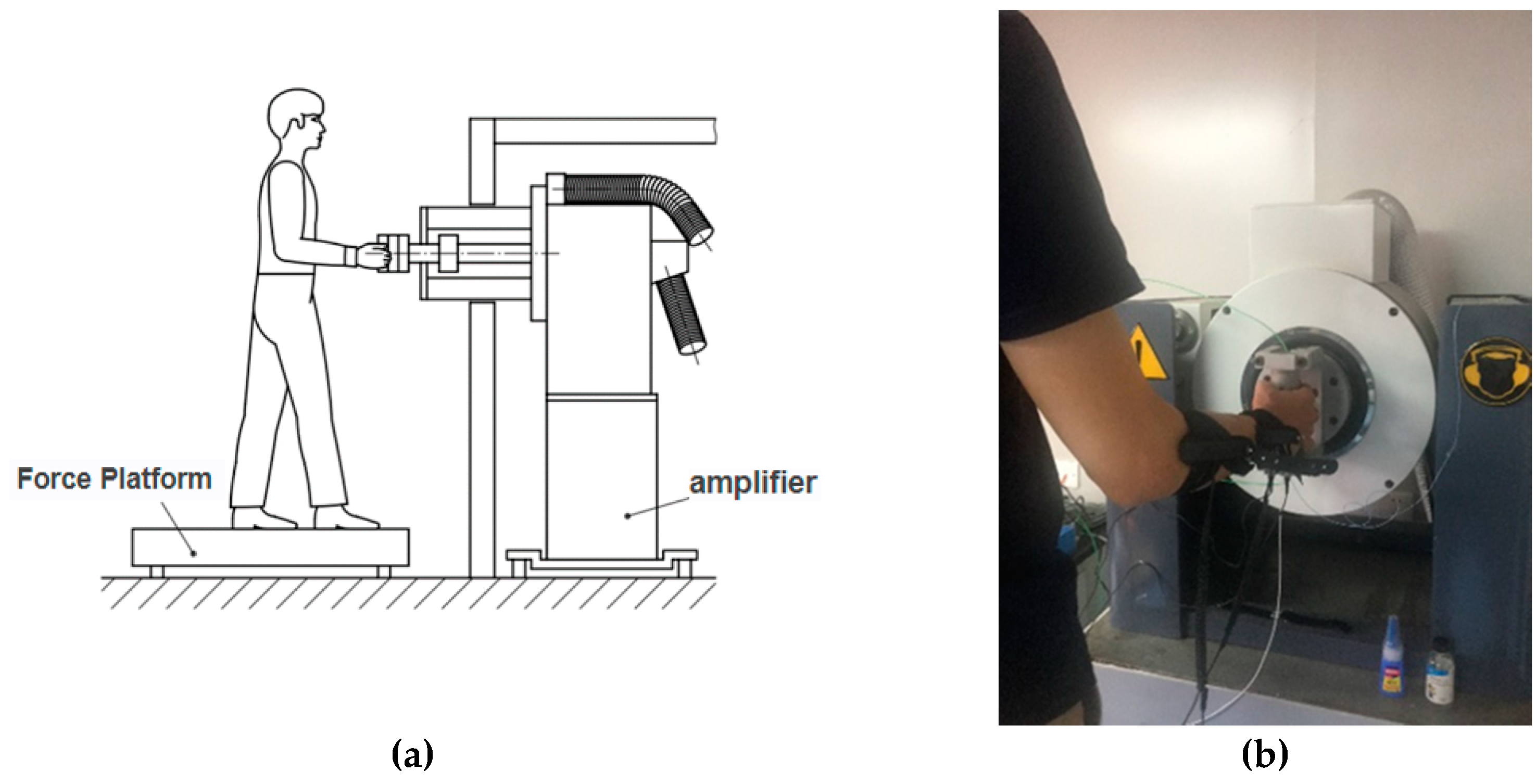
2.1. Experimental Setup
2.2. Test Matrix
2.3. Test Subjects
2.4. Data Collection and Analysis
3. Results
3.1. Transmission of Vibration to the Wrist and Elbow
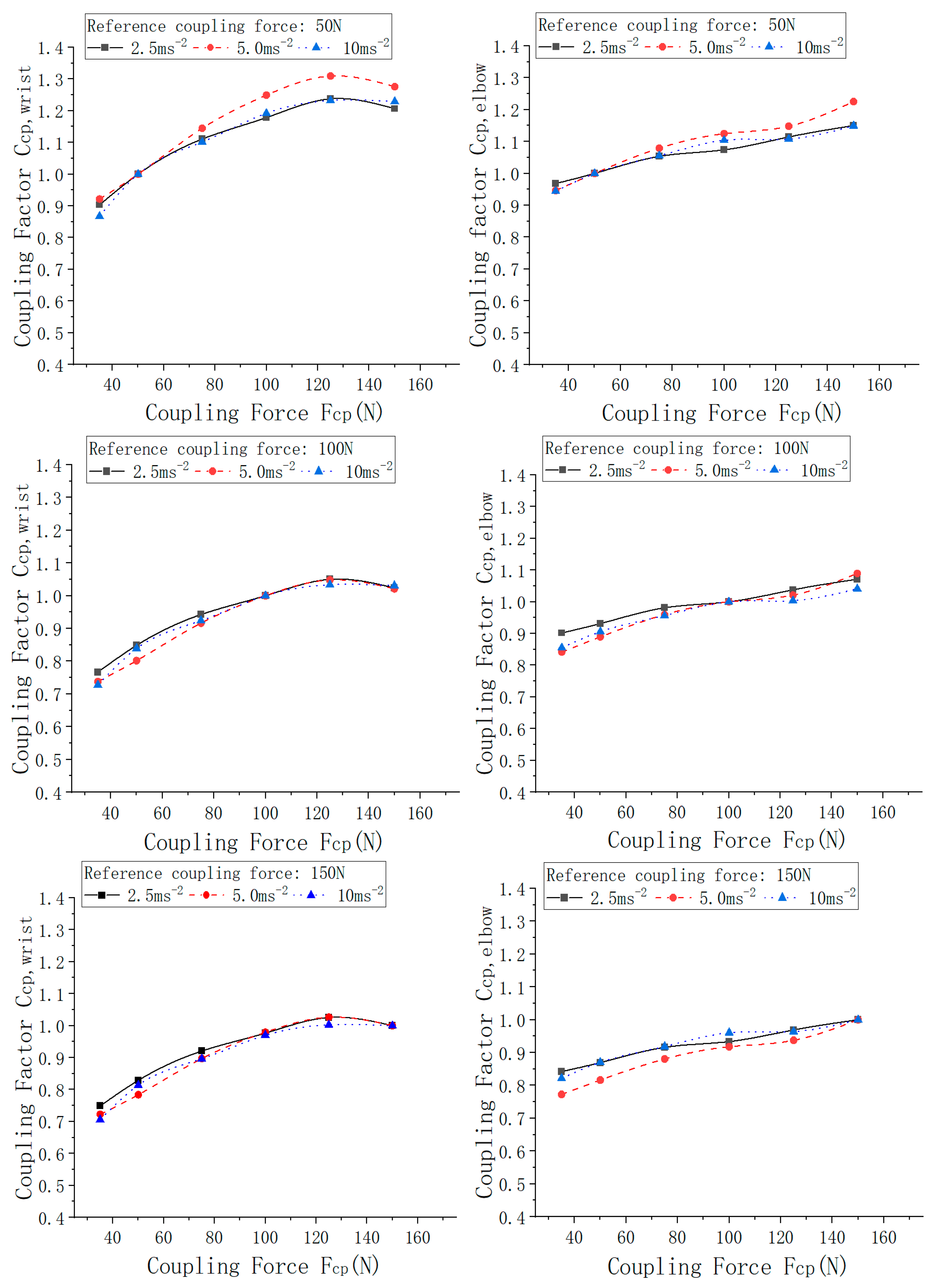
3.2. Coupling Factor at the Wrist and Elbow
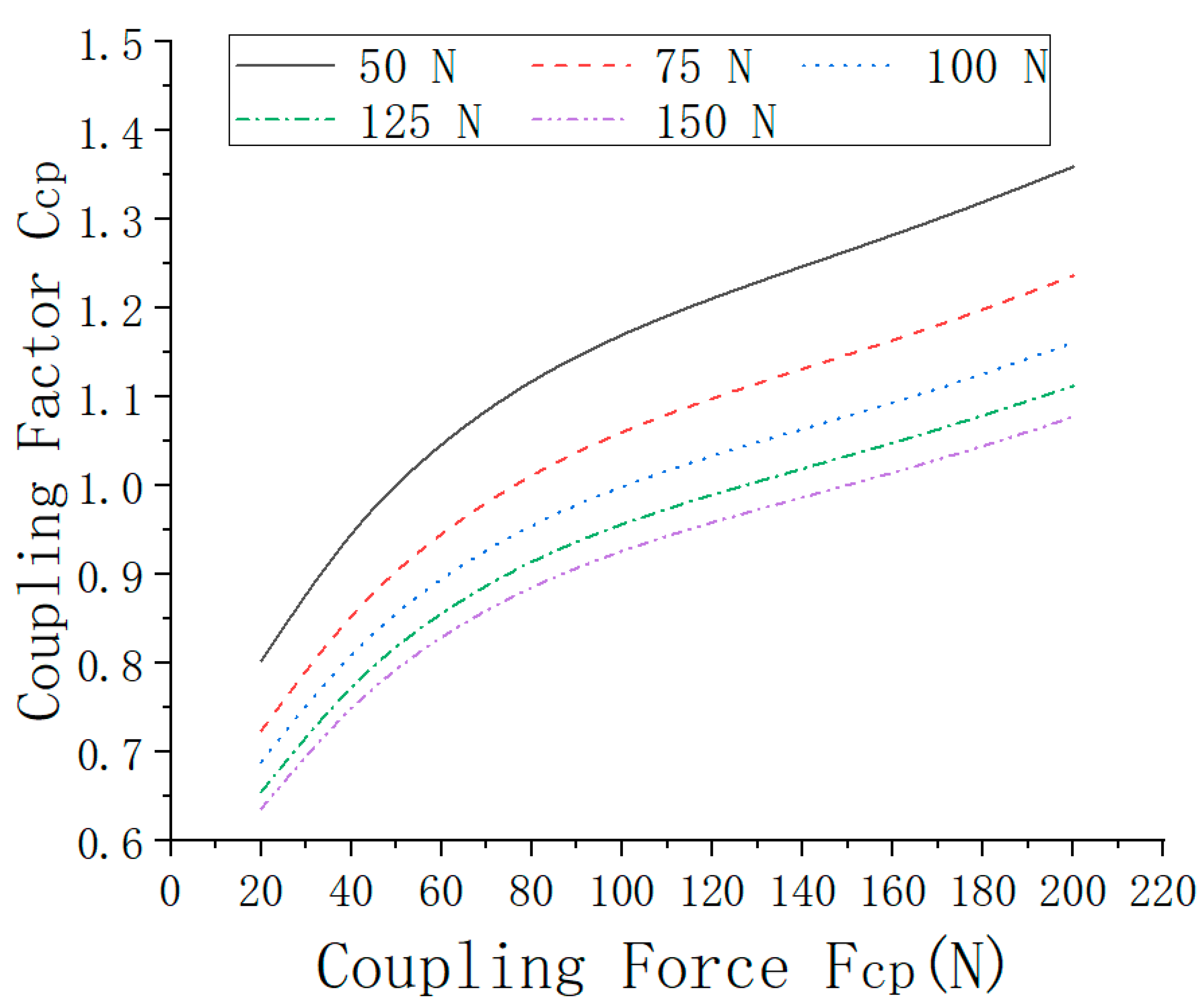
3.3. Comparison with the ISO/TS 15230–2 Relationships from Total Vibration Ratios at the Various Excitation Levels
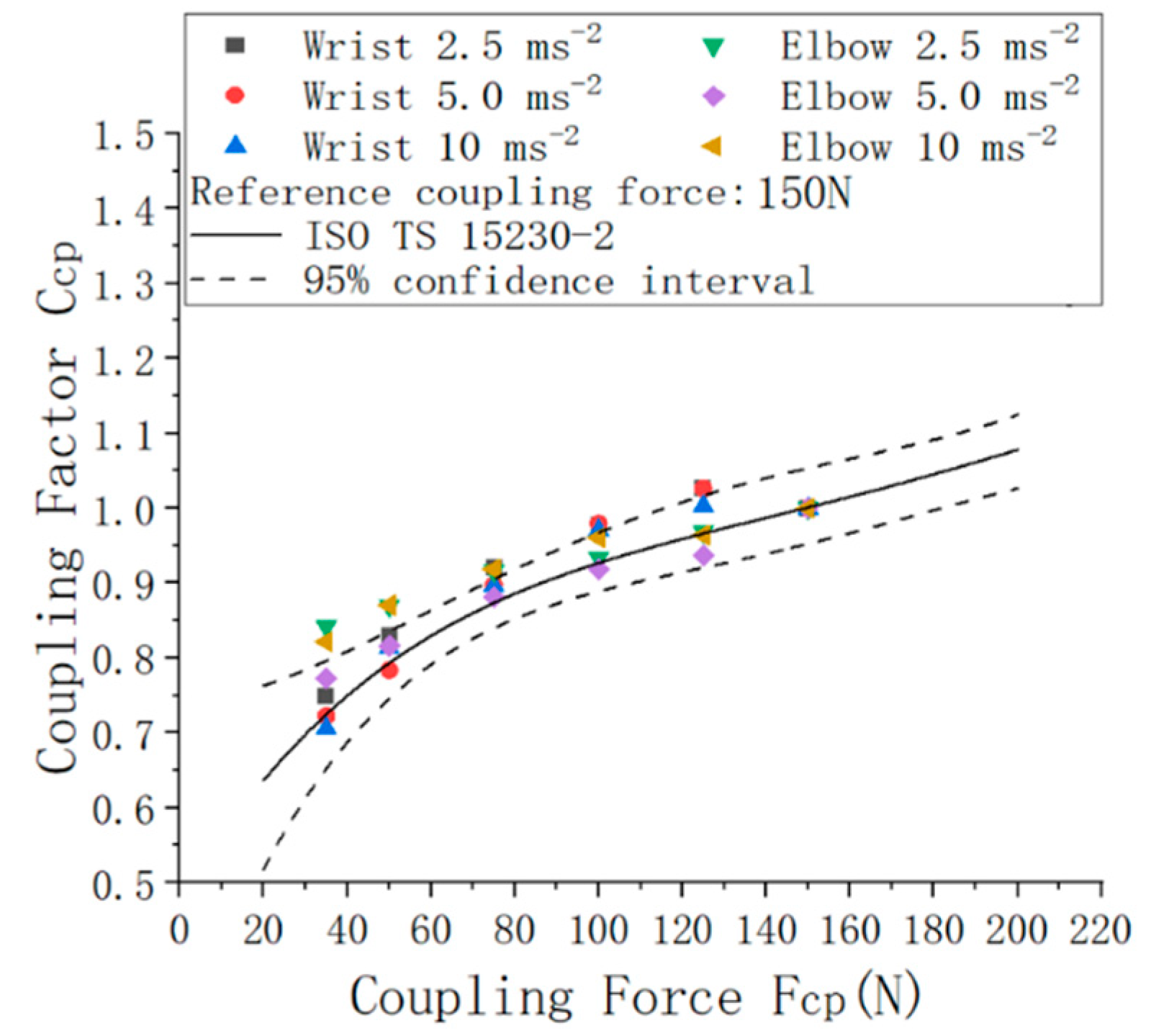
3.4. Overall Measured Coupling Factors and Comparison with ISO/TS 15230–2
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Griffin, M.J. Handbook of Human Vibration; Academic Press: London, UK, 1990. [Google Scholar]
- ISO 5349-1: 2001, Mechanical Vibration—Measurement and Evaluation of Human Exposure to Hand-transmitted Vibration—Part 1: General Requirements. International Organization for Standardization. Available online: https://www.iso.org/standard/32355.html (accessed on 18 November 2021).
- Aldien, Y.; Marcotte, P.; Rakheja, S.; Boileau, P.-É. Influence of hand forces and handle size on power absorption of the human hand–arm exposed to zh-axis vibration. J. Sound Vib. 2006, 290, 1015–1039. [Google Scholar] [CrossRef]
- Adewusi, S.; Rakheja, S.; Marcotte, P.; Boutin, J. Vibration transmissibility characteristics of the human hand–arm system under different postures, hand forces and excitation levels. J. Sound Vib. 2010, 329, 2953–2971. [Google Scholar] [CrossRef]
- Dong, R.G.; Welcome, D.E.; McDowell, T.W.; Wu, J.Z.; Schopper, A.W. Frequency weighting derived from power absorption of fingers–hand–arm system under zh-axis vibration. J. Biomech. 2006, 39, 2311–2324. [Google Scholar] [CrossRef] [PubMed]
- Marcotte, P.; Aldien, Y.; Boileau, P.-É.; Rakheja, S.; Boutin, J. Effect of handle size and hand–handle contact force on the biodynamic response of the hand–arm system under zh-axis vibration. J. Sound Vib. 2005, 283, 1071–1091. [Google Scholar] [CrossRef]
- Pan, D.; Xu, X.S.; Welcome, D.E.; McDowell, T.W.; Warren, C.; Wu, J.; Dong, R.G. The relationships between hand coupling force and vibration biodynamic responses of the hand-arm system. Ergon. 2018, 61, 818–830. [Google Scholar] [CrossRef] [PubMed]
- Xu, X.S.; Dong, R.G.; Welcome, D.E.; Warren, C.; McDowell, T.W.; Wu, J.Z. Vibrations transmitted from human hands to upper arm, shoulder, back, neck, and head. Int. J. Ind. Ergon. 2017, 62, 1–12. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Riedel, S. Consideration of grip and push forces for the assessment of vibration exposure. Central Eur. J. Public Health 1995, 3, 139–141. [Google Scholar]
- Saha, S.; Kalra, P. A review on hand-arm vibration exposure and vibration transmissibility from power hand tools to hand-arm system. Int. J. Hum. Factors Ergon. 2016, 4, 10–46. [Google Scholar] [CrossRef]
- ISO/TS 15230-2: (Technical Specification awaiting publication) Mechanical Vibration and Shock- Coupling Forces at the Man-machine Interface for Hand-transmitted Vibration-Part 2: Guidelines for the Evaluation of Coupling Forces. International Organization for Standardization. Available online: https://www.iso.org/standard/77517.html (accessed on 10 February 2022).
- ISO 15230-1: 2021, Mechanical Vibration and Shock—Coupling Forces at the Man-machine Interface for Hand-transmitted Vibration—Part 1: Measurement and Evaluation. International Organization for Standardization. Available online: https://www.iso.org/standard/77516.html (accessed on 18 November 2021).
- Dupuis, H.; Scheffer, M. Wirkung von Greif- und Andruckkraft unter Schwingungsbelastung—Teilprojekt II; Forschungsbericht Hand-Arm-Schwingungen III; Schriftenreihe des Hauptverbandes der gewerblichen Berufsgenossenschaften; Hauptverbandes der gewerblichen Berufsgenossenschaften: Sankt Augustin, Germany, 1992; pp. 1–164. [Google Scholar]
- Hartung, E.; Dupuis, H.; Scheffer, M. Effects of grip and push forces on the acute response of the hand-arm system under vibrating conditions. Int. Arch. Occup. Environ. Health 1993, 64, 463–467. [Google Scholar] [CrossRef] [PubMed]
- ISO 21420: 2020, Protective Gloves—General Requirements and Test Methods. International Organization for Standardization. Available online: https://www.iso.org/standard/69030.html (accessed on 18 November 2021).



| Coefficient | A | B | C | D | E | |
|---|---|---|---|---|---|---|
| Coupling Force (N) | ||||||
| 50 | 0.606 | 1.15 × 10–2 | –8.846 × 10–5 | 3.482 × 10–7 | –4.961 × 10–10 | |
| 75 | 0.5483 | 1.021 × 10–2 | –7.55 × 10–5 | 2.838 × 10–7 | –3.775 × 10–10 | |
| 100 | 0.5218 | 9.755 × 10–3 | –7.503 × 10–5 | 2.932 × 10–7 | –4.101 × 10–10 | |
| 125 | 0.4906 | 9.636 × 10–3 | –7.561 × 10–5 | 3.024 × 10–7 | –4.376 × 10–10 | |
| 150 | 0.48 | 9.132 × 10–3 | –7.017 × 10–5 | 2.732 × 10–7 | –3.796 × 10–10 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lin, H.; Boileau, P.-E.; Jiang, Y.; Yan, M.; Xiao, B. Investigating the Relationship between Coupling Forces and Hand-Transmitted Vibration under Varying Excitation Levels. Vibration 2022, 5, 141-152. https://doi.org/10.3390/vibration5010009
Lin H, Boileau P-E, Jiang Y, Yan M, Xiao B. Investigating the Relationship between Coupling Forces and Hand-Transmitted Vibration under Varying Excitation Levels. Vibration. 2022; 5(1):141-152. https://doi.org/10.3390/vibration5010009
Chicago/Turabian StyleLin, Hansheng, Paul-Emile Boileau, Yongjian Jiang, Maosheng Yan, and Bin Xiao. 2022. "Investigating the Relationship between Coupling Forces and Hand-Transmitted Vibration under Varying Excitation Levels" Vibration 5, no. 1: 141-152. https://doi.org/10.3390/vibration5010009
APA StyleLin, H., Boileau, P.-E., Jiang, Y., Yan, M., & Xiao, B. (2022). Investigating the Relationship between Coupling Forces and Hand-Transmitted Vibration under Varying Excitation Levels. Vibration, 5(1), 141-152. https://doi.org/10.3390/vibration5010009










