Experimental Evaluation of the Driving Parameters in Human–Structure Interaction
Abstract
:1. Introduction
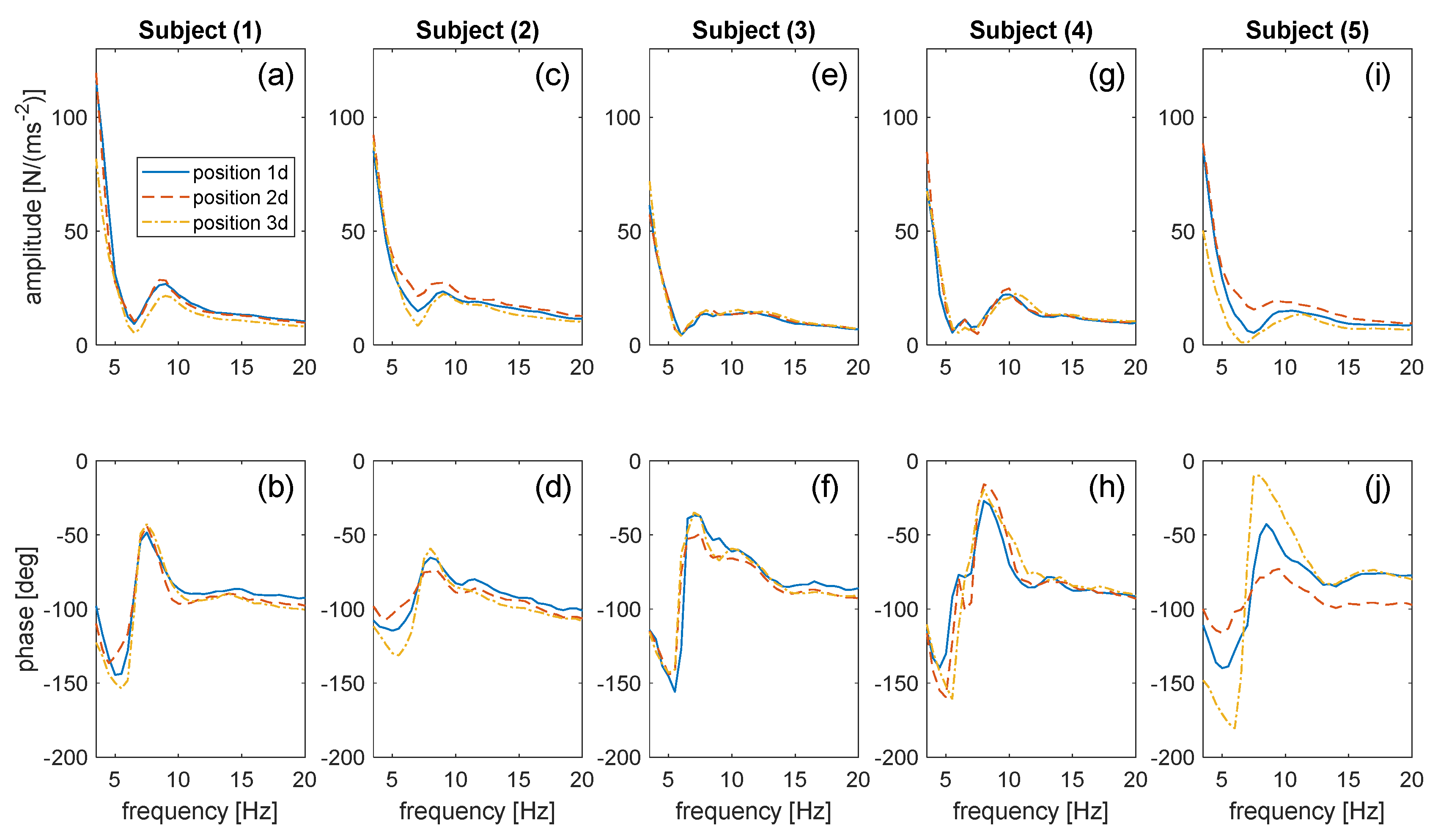
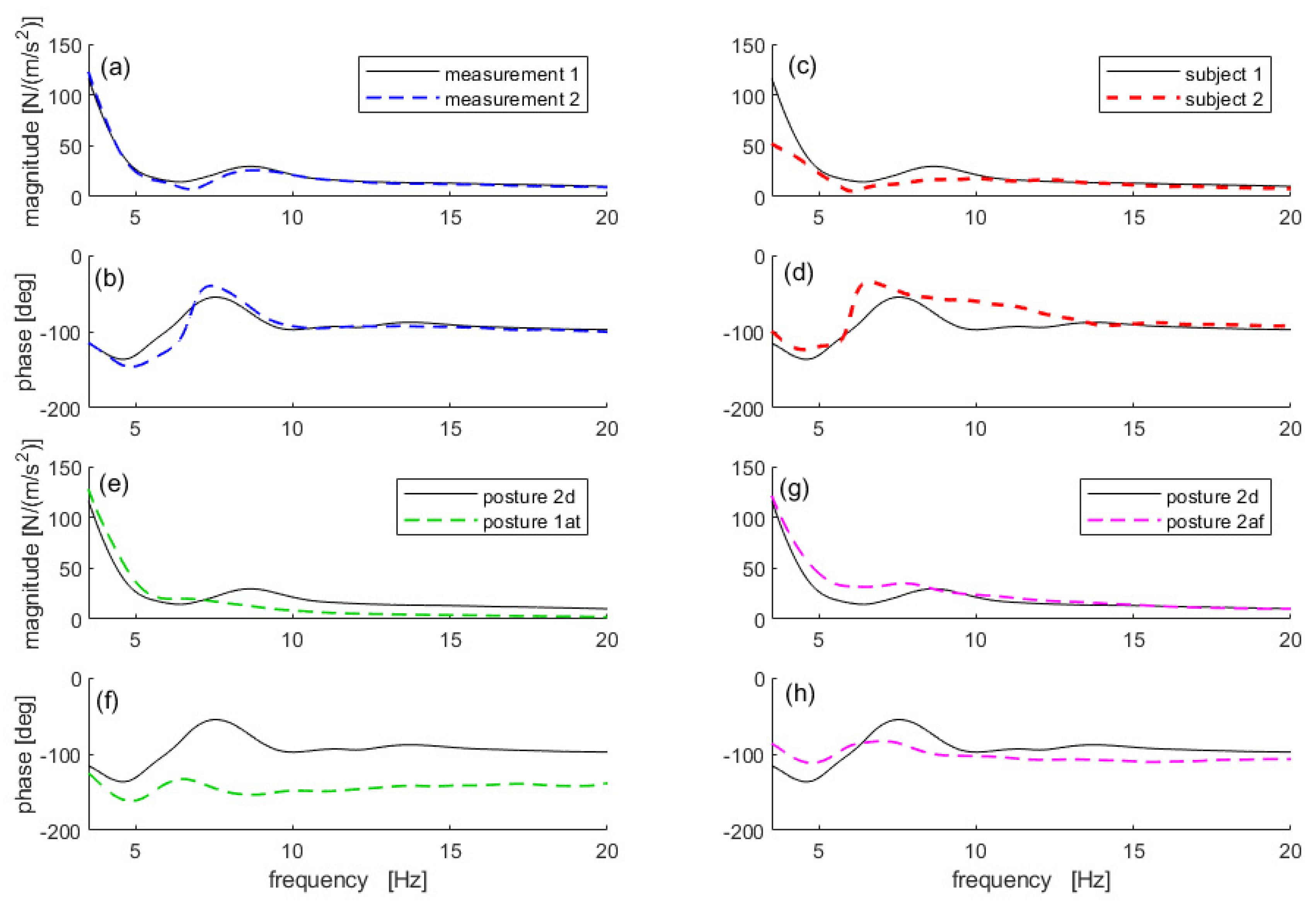
- Intra-subject variability: the AM curve of the same person in a given posture is affected by a natural dispersion;
- Inter-subject variability: the AM curves of different people in the same posture are different;
2. Passive Pedestrian Contribution
2.1. Motion Analysis
2.2. Number and Types of Tests
2.3. Measurement of AM Curves
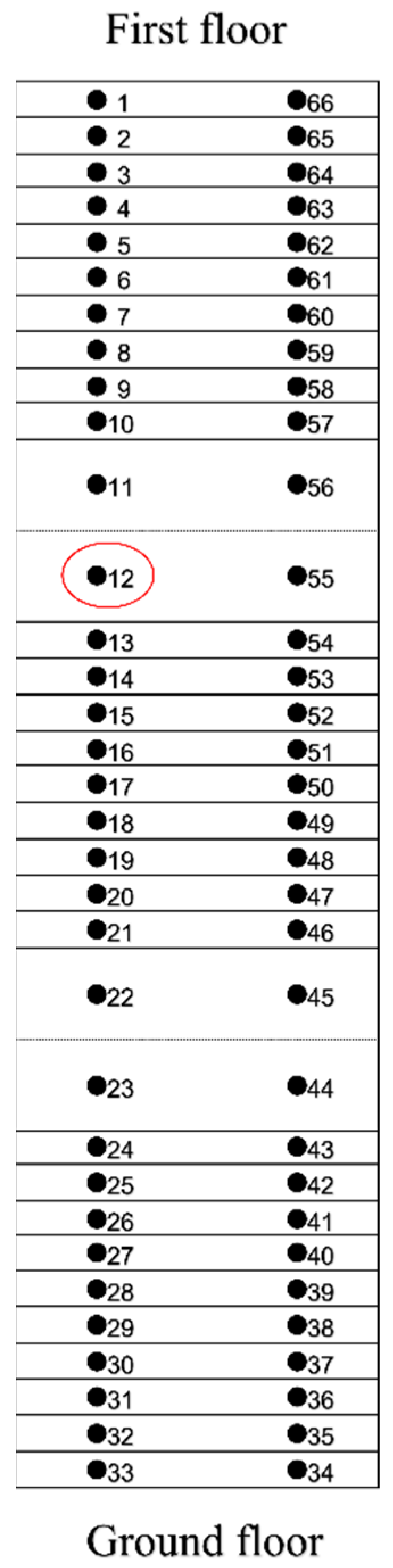
2.3.1. Set-Up Description
2.3.2. Description of the Test
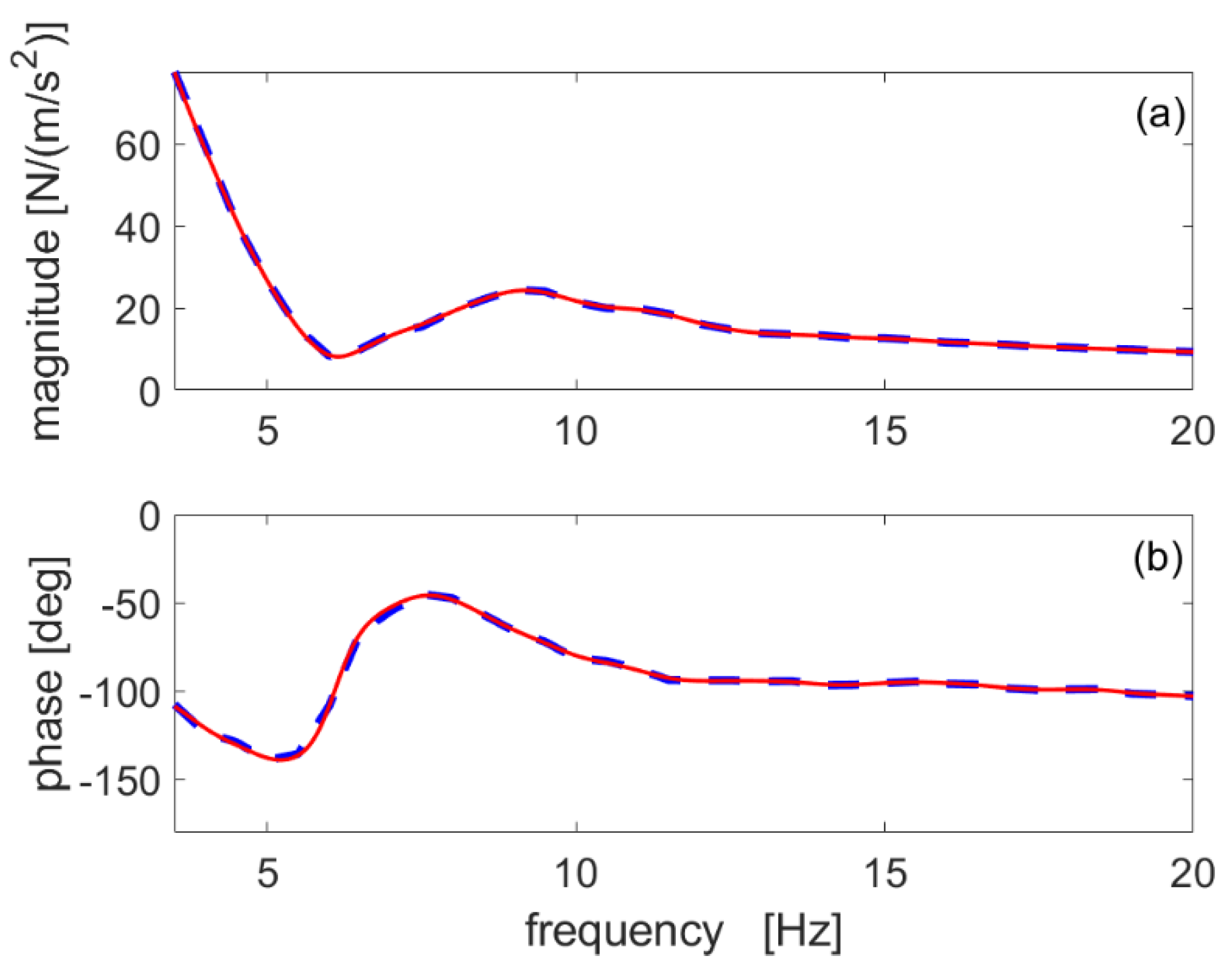
2.3.3. Post-Processing of Data
3. Reference Structure
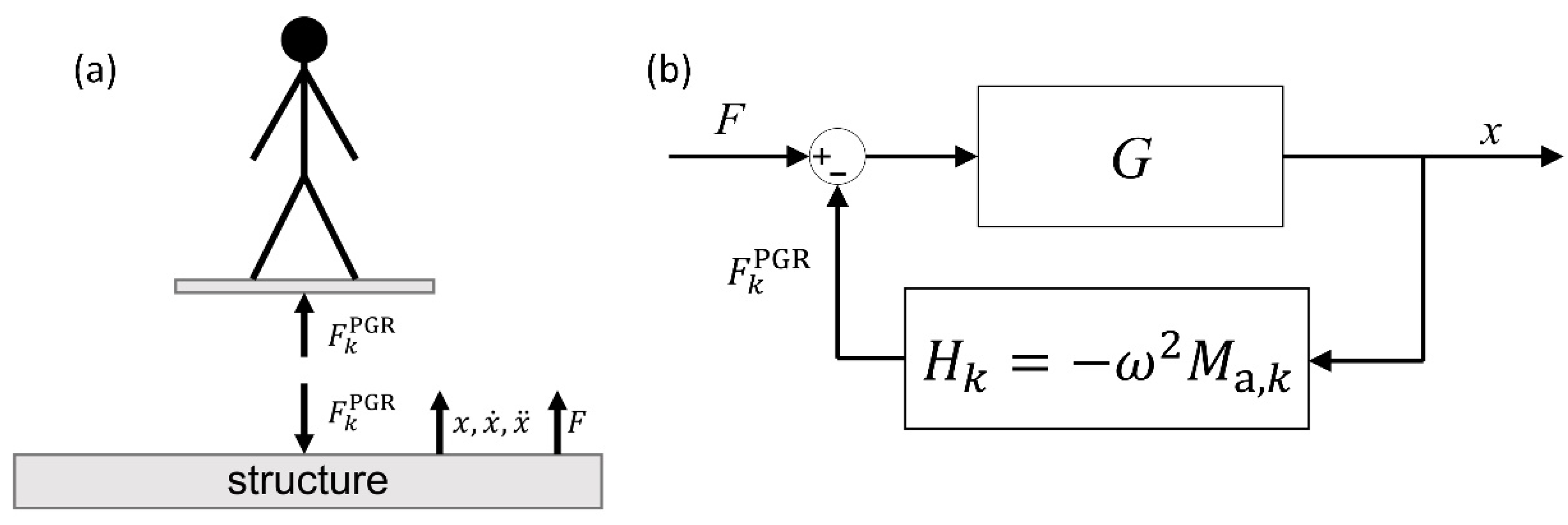
4. Pedestrian–Structure Coupling
4.1. Analytical Model and Its Implementation
4.2. Statistical Treatment
5. Tests and Results
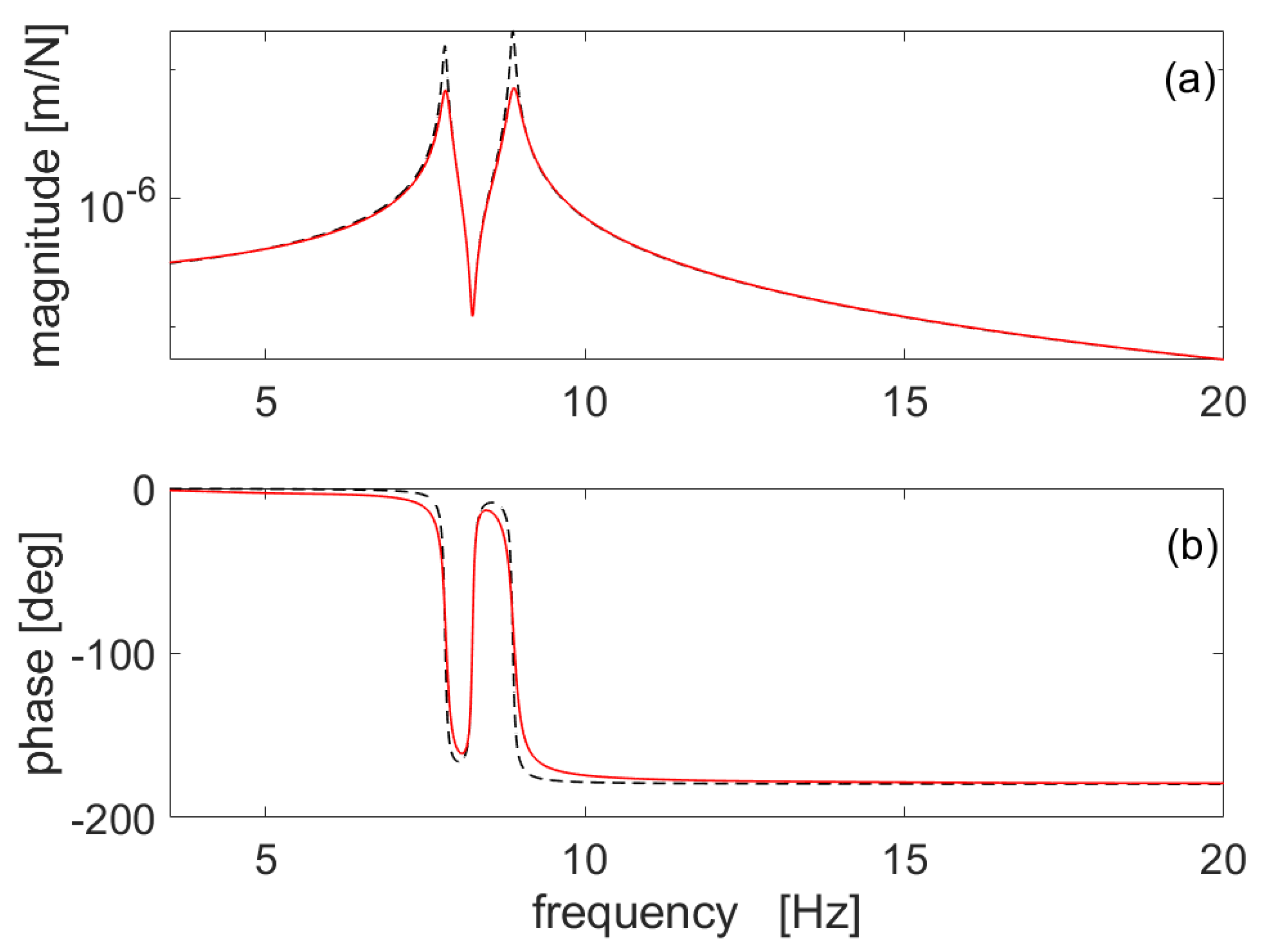
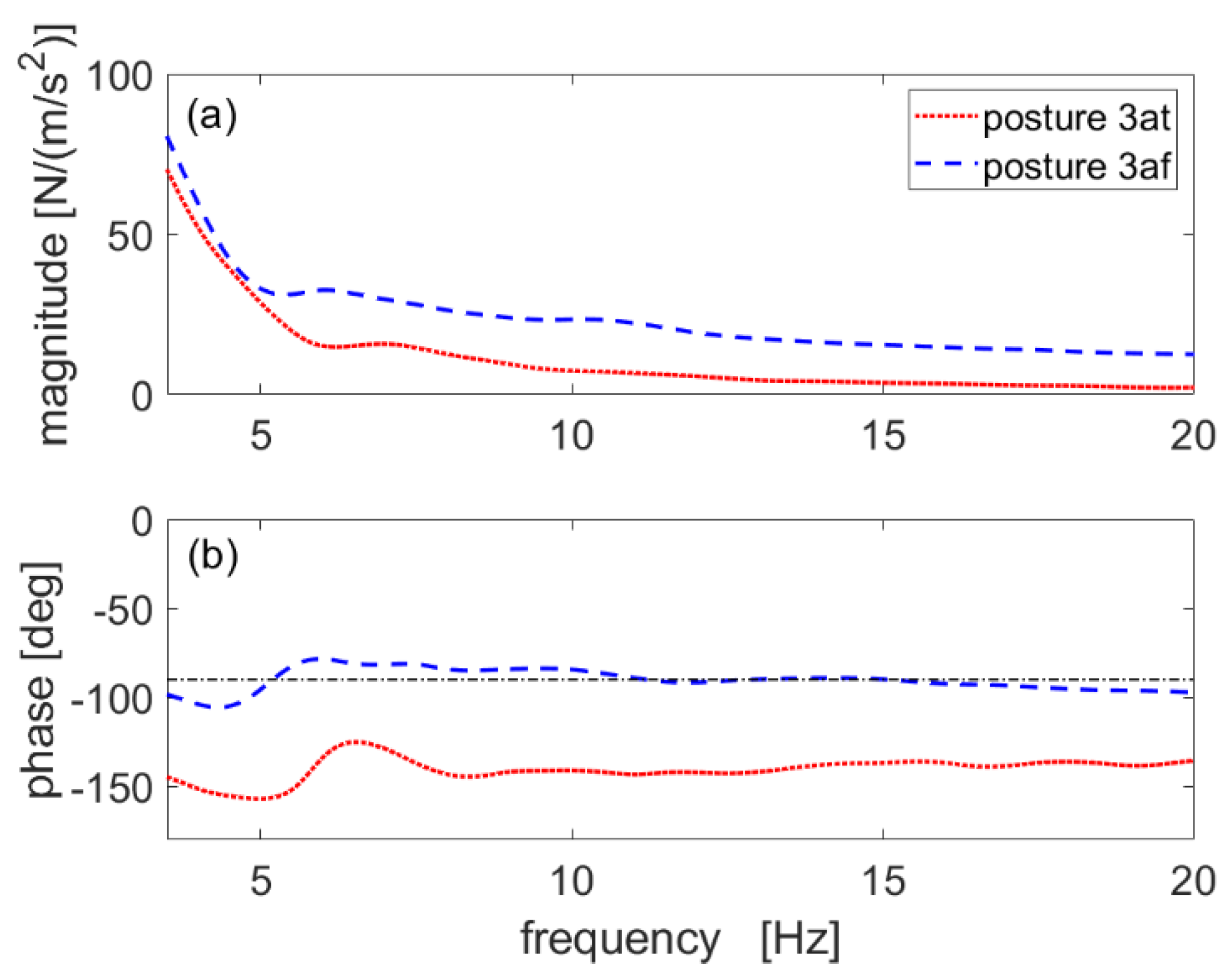
5.1. Passive HSI Effect
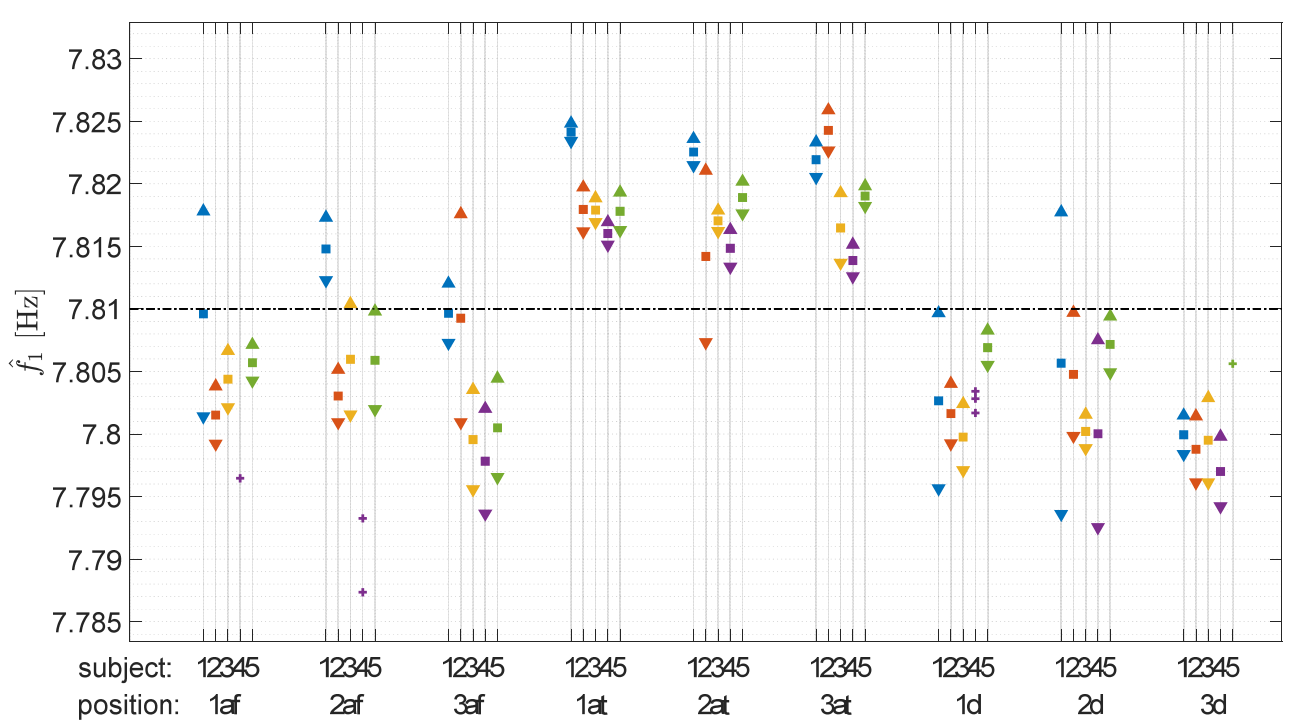
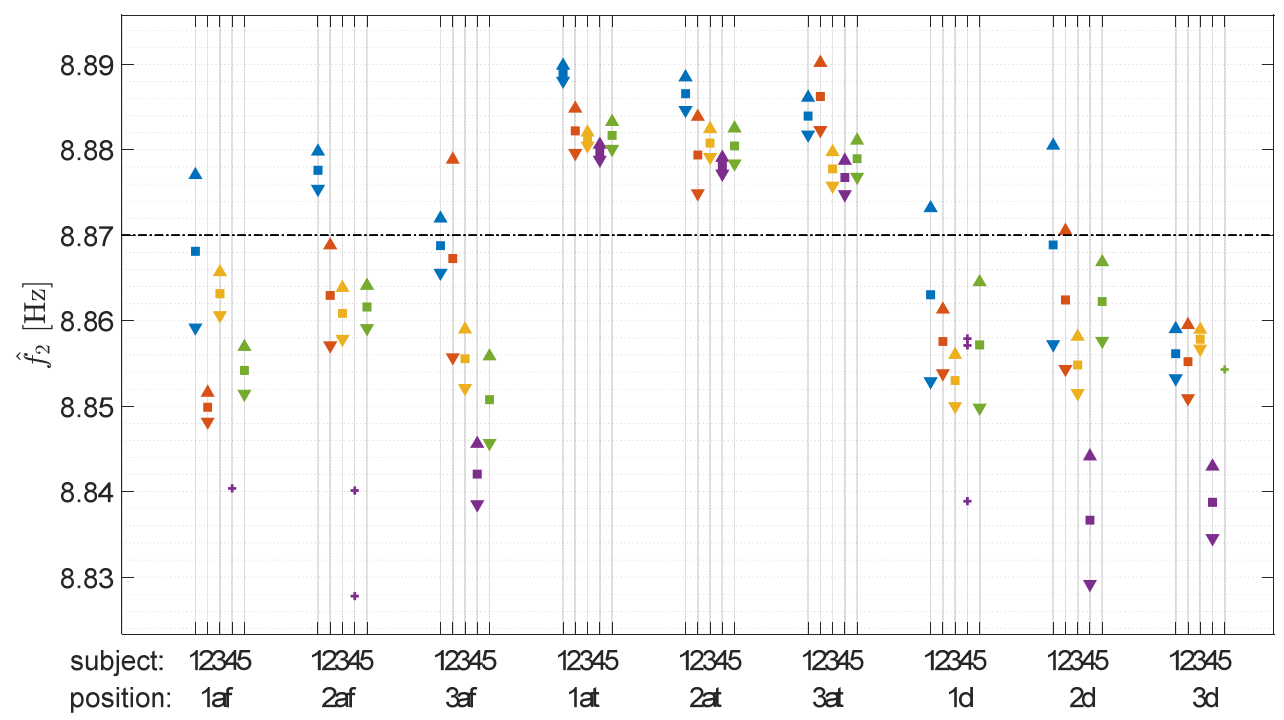
5.1.1. Change in Eigenfrequencies
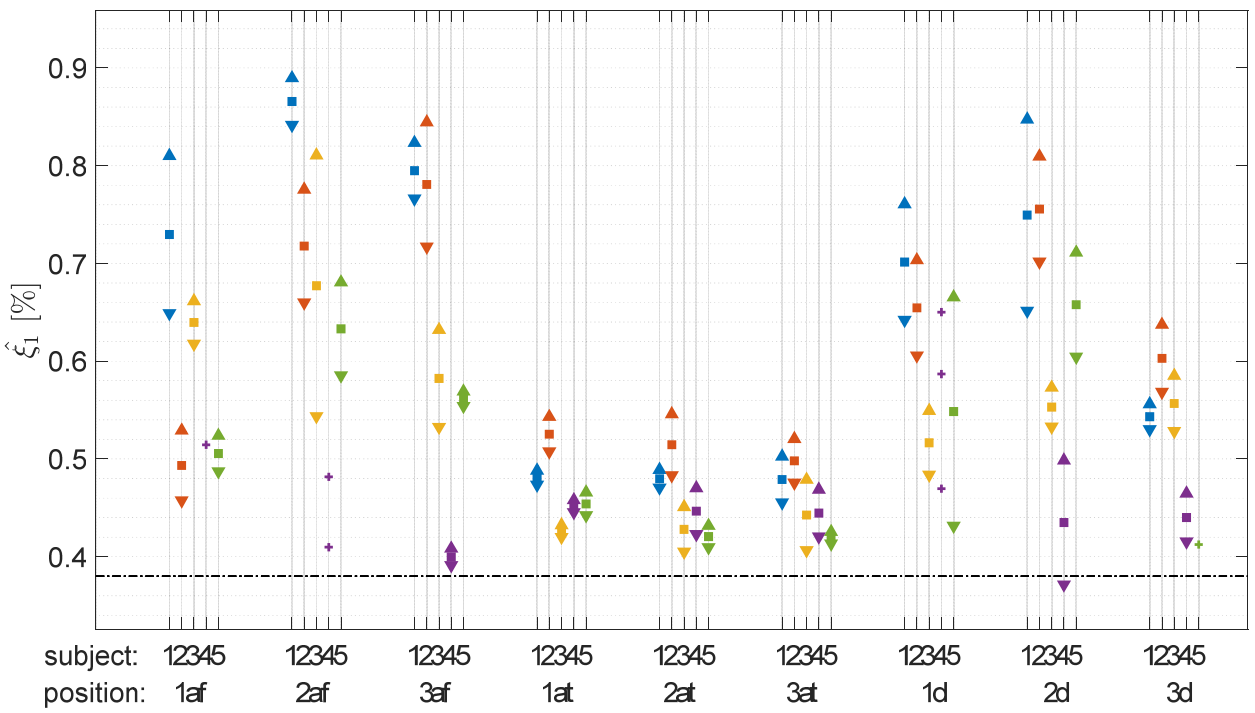
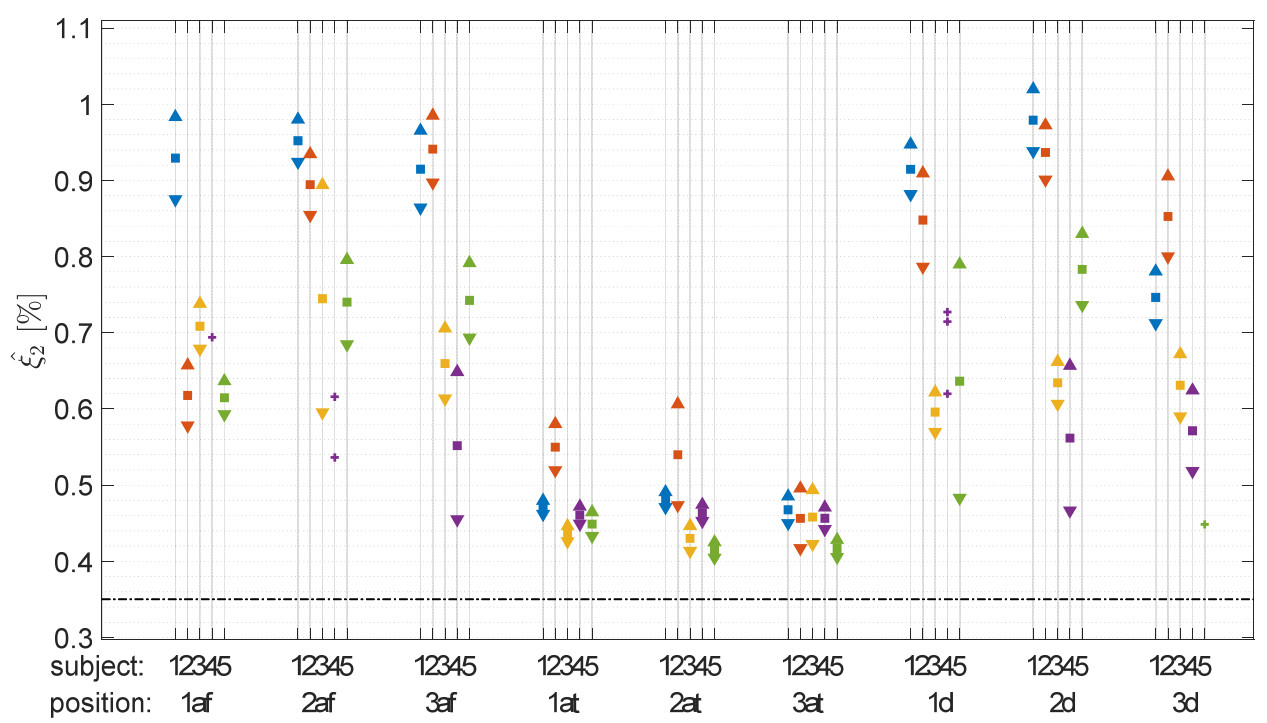
5.1.2. Change in Non-Dimensional Damping Ratio
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A


References
- Figueiredo, F.P.; da Silva, J.G.S.; de Lima, L.R.O.; da S. Vellasco, P.C.G.; de Andrade, S.A.L. A parametric study of composite footbridges under pedestrian walking loads. Eng. Struct. 2008, 30, 605–615. [Google Scholar] [CrossRef]
- Ingólfsson, E.T.; Georgakis, C.T. A stochastic load model for pedestrian-induced lateral forces on footbridges. Eng. Struct. 2011, 33, 3454–3470. [Google Scholar] [CrossRef]
- Piccardo, G.; Tubino, F. Equivalent spectral model and maximum dynamic response for the serviceability analysis of footbridges. Eng. Struct. 2012, 40, 445–456. [Google Scholar] [CrossRef]
- Venuti, F.; Bruno, L.; Bellomo, N. Crowd dynamics on a moving platform: Mathematical modelling and application to lively footbridges. Math. Comput. Model. 2007, 45, 252–269. [Google Scholar] [CrossRef]
- Tubino, F.; Carassale, L.; Piccardo, G. Human-Induced Vibrations on Two Lively Footbridges in Milan. J. Bridge Eng. 2015, 21, C4015002. [Google Scholar] [CrossRef]
- Toso, M.A.; Gomes, H.M.; Da Silva, F.T.; Pimentel, R.L. Experimentally fitted biodynamic models for pedestrian-structure interaction in walking situations. Mech. Syst. Signal Process. 2016, 72–73, 590–606. [Google Scholar] [CrossRef]
- Caprani, C.C.; Ahmadi, E. Formulation of human-structure interaction system models for vertical vibration. J. Sound Vib. 2016, 377, 346–367. [Google Scholar] [CrossRef]
- Setareh, M.; Ph, D.; Asce, M. Vibrations due to Walking in a Long-Cantilevered Office Building Structure. J. Perform. Constr. Facil. 2012, 26, 255–270. [Google Scholar] [CrossRef]
- Bedon, C.; Fasan, M. Reliability of Field Experiments, Analytical Methods and Pedestrian’s Perception Scales for the Vibration Serviceability Assessment of an In-Service Glass Walkway. Appl. Sci. 2019, 9, 1936. [Google Scholar] [CrossRef] [Green Version]
- Busca, G.; Cappellini, A.; Manzoni, S.; Tarabini, M.; Vanali, M. Quantification of changes in modal parameters due to the presence of passive people on a slender structure. J. Sound Vib. 2014, 333, 5641–5652. [Google Scholar] [CrossRef]
- Cappellini, A.; Manzoni, S.; Vanali, M.; Cigada, A. Evaluation of the dynamic behaviour of steel staircases damped by the presence of people. Eng. Struct. 2016, 115, 165–178. [Google Scholar] [CrossRef]
- Vanali, M.; Berardengo, M.; Manzoni, S. Numerical Model for Human Induced Vibrations. In Proceedings of the International Modal Analysis Conference, IMAC XXXV, Garden Grove, CA, USA, 30 January–2 February 2017; pp. 179–186. [Google Scholar]
- Berardengo, M.; Drago, L.; Manzoni, S.; Vanali, M. An approach to predict human–structure interaction in the case of staircases. Arch. Appl. Mech. 2019, 89, 2167–2191. [Google Scholar] [CrossRef]
- Reynolds, P.; Pavic, A. Changes of modal properties of a stadium structure occupied by a crowd. In Proceedings of the 22nd IMAC Conference and Exposition 2004 (IMAC XXII), Dearborn, MI, USA, 26–29 January 2004. [Google Scholar]
- Sachse, R.; Pavic, A.; Reynolds, P. Parametric study of modal properties of damped two-degree-of-freedom crowd-structure dynamic systems. J. Sound Vib. 2004, 274, 461–480. [Google Scholar] [CrossRef]
- Cappellini, A.; Cattaneo, A.; Manzoni, S.; Matteo Scaccabarozzi, M.V. Effects of People Occupancy on the Modal Properties of a Stadium Grandstand. In Proceedings of the XXXIII IMAC, Orlando, FL, USA, 1–4 February 2015. [Google Scholar]
- SÉTRA Technical Guide Footbridges Assessment of Vibrational Behaviour of Footbridges under Pedestrian Loading; The Technical Department for Transport, Roads and Bridges Engineering and Road Safety: Bagneux, France, 2006.
- International Organization for Standardization. ISO 10137; Bases for Design of Structures-Serviceability of Buildings and Walk ways against Vibrations. International Organization for Standardization: Geneva, Switzerland, 2007.
- EN1990; Eurocode Basis of Structural Design. European Committee for Standardization: Brussels, Belgium, 2002.
- Murray, T.M.; Allen, D.E.; Ungar, E.E. Floor Vibrations due to Human Activity. In Steel Design Guide Series; American Institute of Steel Construction (AISC): Chicago, IL, USA, 1997. [Google Scholar]
- ISO 2631-2; Evaluation of Human Exposure to Whole-Body Vibration. Part 2: Continuous and Shock-Induced Vibration in Buildings (1–80 Hz). International Standards Organisation: Geneva, Switzerland, 1989.
- Research Fund for Coal and Steel. HiVoSS: Design of Footbridges; Rheinisch-Westfaelische Technische Hochschule Aachen: Aachen, Germany, 2008. [Google Scholar]
- Van Nimmen, K.; Lombaert, G.; De Roeck, G.; Van den Broeck, P. Vibration serviceability of footbridges: Evaluation of the current codes of practice. Eng. Struct. 2014, 59, 448–461. [Google Scholar] [CrossRef]
- Tubino, F. Probabilistic assessment of the dynamic interaction between multiple pedestrians and vertical vibrations of footbridges. J. Sound Vib. 2018, 417, 80–96. [Google Scholar] [CrossRef]
- Ortiz-Lasprilla, A.R.; Caicedo, J.M. Comparing Closed Loop Control Models andMass-Spring-Damper Models for Human Structure Interaction Problems. In Dynamics of Civil Structures, Volume 2; Springer: Cham, Switzerland, 2015. [Google Scholar] [CrossRef]
- Alexander, N.A. Theoretical treatment of crowd–structure interaction dynamics. Proc. Inst. Civ. Eng. Struct. Build. 2006, 159, 329–338. [Google Scholar] [CrossRef]
- Sim, J.; Blakeborough, A.; Williams, M. Modelling of joint crowd-structure system using equivalent reduced-DOF system. Shock Vib. 2007, 14, 261–270. [Google Scholar] [CrossRef]
- Živanovic, S.; Pavic, A.; Reynolds, P. Probability-based prediction of multi-mode vibration response to walking excitation. Eng. Struct. 2007, 29, 942–954. [Google Scholar] [CrossRef]
- Sachse, R.; Pavic, A.; Reynolds, P. Human-structure dynamic interaction in civil engineering dynamics: A literature review. Shock Vib. Dig. 2003, 35, 3–18. [Google Scholar] [CrossRef]
- Živanović, S.; Pavic, A.; Reynolds, P. Vibration serviceability of footbridges under human-induced excitation: A literature review. J. Sound Vib. 2005, 279, 1–74. [Google Scholar] [CrossRef] [Green Version]
- Racic, V.; Pavic, A.; Brownjohn, J.M.W. Experimental identification and analytical modelling of human walking forces: Literature review. J. Sound Vib. 2009, 326, 1–49. [Google Scholar] [CrossRef]
- Van Nimmen, K.; Lombaert, G.; De Roeck, G.; Van den Broeck, P. The impact of vertical human-structure interaction on the response of footbridges to pedestrian excitation. J. Sound Vib. 2017, 402, 104–121. [Google Scholar] [CrossRef] [Green Version]
- Shahabpoor, E.; Pavic, A.; Racic, V. Structural vibration serviceability: New design framework featuring human-structure interaction. Eng. Struct. 2017, 136, 295–311. [Google Scholar] [CrossRef]
- Venuti, F.; Racic, V.; Corbetta, A. Modelling framework for dynamic interaction between multiple pedestrians and vertical vibrations of footbridges. J. Sound Vib. 2016, 379, 245–263. [Google Scholar] [CrossRef] [Green Version]
- Lucà, F.; Berardengo, M.; Manzoni, S.; Vanali, M.; Drago, L. Human-structure interaction: Convolution-based estimation of human-induced vibrations using experimental data. Mech. Syst. Signal Process. 2022, 167, 108511. [Google Scholar] [CrossRef]
- Matsumoto, Y.; Griffin, M.J. Dynamic Response of the Standing Human Body Exposed To Vertical Vibration: Influence of Posture and Vibration Magnitude. J. Sound Vib. 1998, 212, 85–107. [Google Scholar] [CrossRef]
- Ewins, D.J. Modal Testing: Theory, Practice and Application, 2nd ed.; Wiley: Hoboken, NJ, USA, 2000; ISBN 978-0-863-80218-8. [Google Scholar]
- Dallard, P.; Flint, A.; Le Bourva, S.; Low, A.; Smith, R.M.R.; Willford, M. The London Millennium Footbridge. Struct. Eng. 2001, 79, 17–35. [Google Scholar]
- Mashaly, E.S.; Ebrahim, T.M.; Abou-Elfath, H.; Ebrahim, O.A. Evaluating the vertical vibration response of footbridges using a response spectrum approach. Alex. Eng. J. 2013, 52, 419–424. [Google Scholar] [CrossRef] [Green Version]
- Racic, V.; Brownjohn, J.M.W. Stochastic model of near-periodic vertical loads due to humans walking. Adv. Eng. Inform. 2011, 25, 259–275. [Google Scholar] [CrossRef]
- Matsumoto, Y.; Griffin, M.J. Mathematical models for the apparent masses of standing subjects exposed to vertical whole-body vibration. J. Sound Vib. 2003, 260, 431–451. [Google Scholar] [CrossRef]
- Tarabini, M.; Solbiati, S.; Saggin, B.; Scaccabarozzi, D. Setup for the Measurement of Apparent Mass Matrix of Standing Subjects. IEEE Trans. Instrum. Meas. 2016, 65, 1856–1864. [Google Scholar] [CrossRef]
- Brandt, A. Noise and Vibration Analysis: Signal Analysis and Experimental Procedures; Wiley: New York, NY, USA, 2011; ISBN 9780470746448. [Google Scholar]
- Brandt, A.; Berardengo, M.; Manzoni, S.; Cigada, A. Scaling of mode shapes from operational modal analysis using harmonic forces. J. Sound Vib. 2017, 407, 128–143. [Google Scholar] [CrossRef]
- Brandt, A.; Berardengo, M.; Manzoni, S.; Vanali, M.; Cigada, A. Global scaling of operational modal analysis modes with the OMAH method. Mech. Syst. Signal Process. 2019, 117, 52–64. [Google Scholar] [CrossRef] [Green Version]
- Peeters, B.; Van Der Auweraer, H.; Guillaume, P.; Leuridan, J. The PolyMAX frequency-domain method: A new standard for modal parameter estimation? Shock Vib. 2004, 11, 395–409. [Google Scholar] [CrossRef]
- Woodbury, M.A. Inverting Modified Matrices; Statistical Research Group, Princeton University: Princeton, NJ, USA, 1950. [Google Scholar]
- JCGM 100:2008; Evaluation of Measurement Data—Guide to the Expression of Uncertainty in Measurement. Bureau International des Poids et Mesures: Sevres, France, 2008.



| Subject | Gender | Height (cm) | Mass (kg) |
|---|---|---|---|
| 1 | male | 175 | 85 |
| 2 | male | 185 | 90 |
| 3 | female | 165 | 55 |
| 4 | male | 185 | 80 |
| 5 | male | 180 | 70 |
| Direction | Foot-Step Contact | Position Labels | ||
|---|---|---|---|---|
| ascent | full | 1af | 2af | 3af |
| ascent | tip | 1at | 2at | 3at |
| descent | full | 1d | 2d | 3d |
| Mode | ||
|---|---|---|
| 1st | 0.38 | 7.81 |
| 2nd | 0.35 | 8.87 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lucà, F.; Berardengo, M.; Manzoni, S.; Scaccabarozzi, D.; Vanali, M.; Drago, L. Experimental Evaluation of the Driving Parameters in Human–Structure Interaction. Vibration 2022, 5, 121-140. https://doi.org/10.3390/vibration5010008
Lucà F, Berardengo M, Manzoni S, Scaccabarozzi D, Vanali M, Drago L. Experimental Evaluation of the Driving Parameters in Human–Structure Interaction. Vibration. 2022; 5(1):121-140. https://doi.org/10.3390/vibration5010008
Chicago/Turabian StyleLucà, Francescantonio, Marta Berardengo, Stefano Manzoni, Diego Scaccabarozzi, Marcello Vanali, and Loris Drago. 2022. "Experimental Evaluation of the Driving Parameters in Human–Structure Interaction" Vibration 5, no. 1: 121-140. https://doi.org/10.3390/vibration5010008
APA StyleLucà, F., Berardengo, M., Manzoni, S., Scaccabarozzi, D., Vanali, M., & Drago, L. (2022). Experimental Evaluation of the Driving Parameters in Human–Structure Interaction. Vibration, 5(1), 121-140. https://doi.org/10.3390/vibration5010008






