Fixture for the High-Frequency Transfer Path Analysis for Relative Comparison of Fluids of an Electric Motor

Abstract

In a previous paper, it was determined that coolant fluids can have a significant influence on the acoustic emission of an electric motor. There is no appliance that can investigate and compare the transfer path analysis of isolated fluids. For this case, a new fixture is introduced and its suitability is evaluated. Furthermore, the appliance is used to measure and compare different media and their frequency response functions. The results indicate that the fixture and its repeatability are suitable to measure and compare fluids and media. A comparison of different media shows how the air medium has the lowest amplitudes for the transfer path analysis, in relation to incompressible fluids. Furthermore, differences in the transfer path analysis between both considered gearbox oils are marginal. While the utilization of air as a coolant medium is not always possible, due to thermal issues, the presented fixture can help find an acoustically suitable coolant medium for future applications in electric motors. Additionally, it is possible to investigate the influence of temperature and pressure conditions on the transfer path analysis of fluids.

1. Introduction

Coolant fluids are essential for the high-performance electric motors of the future. Because of the operating principle of electric motors, the structural excitation is different compared to internal combustion engines. In fact, the excitation frequency is higher. Therefore, there is a need to consider surrounding systems and components for their influence on acoustic radiation [1,2,3].

The acoustic radiation caused by the electromagnetic forces propagates into adjacent media. Sound propagation describes the propagation of waves in a defined field. In the case of oscillation in solids and fluids, we speak of mechanical waves. Waves in the air with a frequency between 16 Hz and 20 kHz are referred to as sound [4,5].

Furthermore, a differentiation can be made between transverse waves and longitudinal waves. With transverse waves, the direction of oscillation is perpendicular to the direction of propagation. With longitudinal waves, the direction of oscillation is parallel to the direction of propagation. While both types of waves are possible in solids, only longitudinal waves occur in fluids [6,7].

A frequency response function (FRF) describes the relationship between a system excitation and a system response of a dynamic system. In the context of this paper, transfer functions are used to represent the relationship between the force excitation and the surface acceleration in the measurements of the fixture [8,9].

A previous paper has shown that the coolant fluid of the electric motor has a significant influence on the acoustic radiation of the electric machine [10]. Therefore, a detailed investigation into the propagation of the coolant fluid is needed. Because an electric motor is complex in its function and geometric shape, the interpretation of results can become quite difficult. A simplified and isolated test setup facilitates the interpretation of the obtained results. A fixture for an isolated understanding of transfer paths of fluids is not known and, therefore, is required.

For this purpose, the authors introduce a novel fixture to perform a transfer path analysis on media and fluids [11]. It enables the investigation of fluids for a frequency up to 10 kHz and a relative comparison of different media. Additionally, it is prepared to consider pressure and temperature conditions. Overall the fixture is designated to be simulated and validated within the FEA.

The suitability of this fixture is evaluated in this paper. Afterward, a relative comparison between different media is performed.

2. Materials and Methods

In this paper, a novel fixture is presented [11]. This fixture is able to excite a fluid with vibration and detect the resulting reaction. This chapter describes how this fixture works in detail and how it is put into operation.

The fixture describes a cylindrical base body made of aluminum. The base body exhibits an axial length of 50 mm and an inner diameter of 30 mm. A cylindrical cavity is defined. The two cylindrical end surfaces of the cavity are each realized with a membrane. The membrane consists of spring steel and has a thickness of 0.5 mm. The pressing of the membranes to the base body is ensured with one aluminum clamping ring and eight screws. A groove is defined as the contact between a diaphragm and the base body. An elastomer sealing ring is inserted into this groove to ensure the tightness of the system. As the diaphragm is pressed to the base body—in addition to the static pressing—there is no dynamic load on the sealing ring; the sealing ring does not contribute to the dynamic vibration behaviour. The fixture is depicted in Figure 1.

The filling of the cylindrical cavity in the base body is made possible via two inflow openings. These inflow openings are positioned radially. These openings are each closed with a screw plug, which is provided with a rubber sealing ring. During assembly, all screw connections are tightened with a defined torque.

Before a membrane is mounted, it is glued with a so-called force cup for the high-frequency miniature shaker. With the help of a jig, the force cup is positioned orthogonally in the center of the circle on the membrane and glued with a two-component adhesive. After gluing and assembling the fixture, the high-frequency miniature shaker can be placed on the force cup.

The high-frequency miniature shaker accelerates its mass in a frequency spectrum from 500 to 10,000 Hz. In addition, the high-frequency miniature shaker is able to record its generated force. This recorded force is used to calculate a frequency response function (FRF) from the quotient of the resulting surface acceleration and the oscillating force excitation. The surface acceleration is detected on the second, opposing steel membrane with a laser Doppler vibrometer over a circular area. Each measuring point of the laser vibrometer is measured nine times and then averaged. The measurement of the FRF is completed five times and then averaged. The force excitation is carried out with a constant voltage of 3 V and corresponds to a force excitation of 0.7 N_rms.

$$H\left(f\right)=\frac{Acceleration}{Force}=\frac{a\left(f\right)}{F\left(f\right)}$$

(1)

To perform the measurements, the entire test assembly is mounted without fluid. The test setup is depicted in Figure 2. Next, one inflow opening is closed with a screw plug. Then the defined volume of fluid is filled in. Finally, excess air is removed and the second screw plug is mounted. The volume of the fluid is defined in such a way that no compression of the fluid takes place through the screw connection. In previous investigations, it was found that this procedure delivers improved repeatability.

Within the scope of this work, a relative comparison of the media is carried out. For this purpose, the device is filled with different media and the FRFs are measured. The repeatability of the fixture is also proven.

3. Results

After the “Materials and Methods” have been described in the previous chapter, the results are presented in this chapter. First, the repeatability of the two filling methods is reviewed for the water medium in a representative comparison. Then the repeatability for the transmission fluid used is presented. Eventually, a relative comparison of different fluids and media follows.

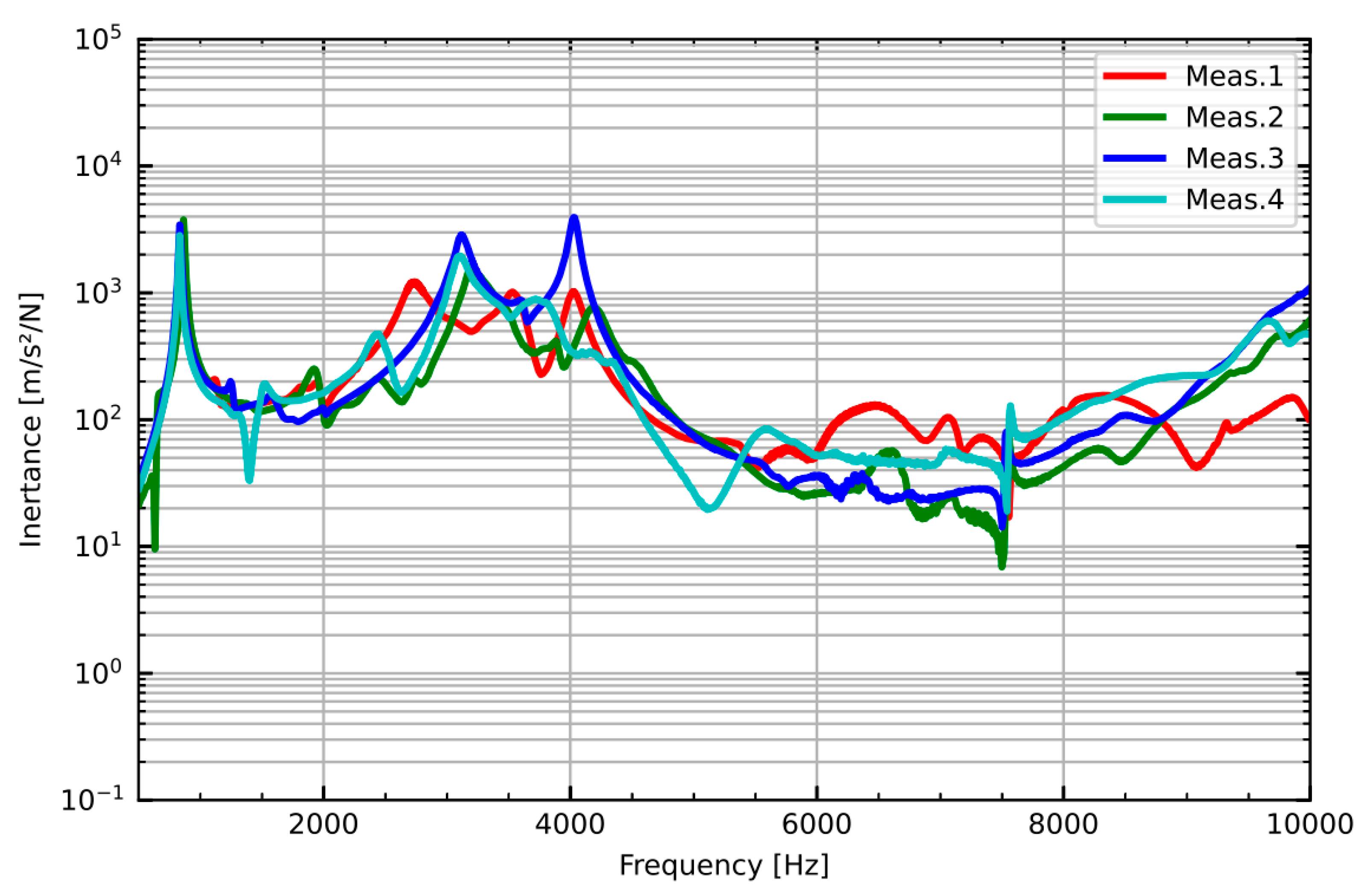
In Figure 3, the transfer functions for four repetitions of the measurement are shown, over a frequency spectrum from 500 to 10,000 Hz.

The illustration of “pouring filling” shows good repeatability up to a frequency of about 4000 Hz. The resonances at 800 Hz and at 2100 Hz have a good agreement between the individual repetitions. In the frequency range between 1200 Hz and 2000 Hz, a shift of the resonance frequency takes place. From the frequency of 4000 Hz, the dispersion of the repeatability increases. Both the amplitude values and the resonance frequencies change between the individual repetitions. A uniform correlation is not evident. The resonances in the frequency range from 7500 Hz to 8000 Hz, showing fluctuations between the individual repetitions.

Figure 4 shows the transfer functions for four repetitions of the measurement of “immersion filling”. The resonance at 800 Hz is repeatable in its frequency and amplitude level. Between 1000 Hz and 3000 Hz the growth function is similar. However, there are a number of resonances in the spectrum, which are shifted in their frequency. Above the frequency of 4000 Hz, the dispersion of the amplitude height and the resonance frequency is high. A uniform correlation is not recognizable. The resonance at 7500 Hz varies in its amplitude height. The resonance frequency also fluctuates.

The comparison between the two methods shows similar deviations for frequencies above 4000 Hz. “Immersion filling” also shows deviations in the frequency range below 4000 Hz. For “pouring filling”, deviations below the frequency of 4000 Hz are smaller in comparison to “immersion Filling”. Additionally, the handling of the fixture with “pouring filling” is easier than in comparison with the “immersion filling”. Because of the smaller deviations below 4000 Hz and the easier handling of the fixture, the “pouring method” is chosen as the suitable method to be used for future measurements.

Figure 5 illustrates the five repetitions of the FRF measurement for transmission fluid as a medium. Over the entire frequency spectrum considered, the repeatability for the gear oil proves to be considerably better compared to the water medium, in Figure 3 and Figure 4.

Over the complete range of the frequency spectrum, the deviation between the measurements of the transmission fluid is visibly smaller than in water media. The overall level of the amplitude of the FRFs is identical to the levels in water media. The course of the FRFs of transmission fluid is identical to the FRFs of water media. Due to the smaller deviation of the FRFs of the transmission fluid, more resonances are identifiable and detailed, for example, at 2300, 9000, and 9500 Hz. This improved deviation can be due to the increased viscosity of transmission fluid over the viscosity of water. An increased deviation is observed around 3800 and 6250 Hz. This could be due to structural resonances or other side effects in the excitation of the structure.

After the results for the two filling methods for water and the repeatability for the used transmission fluid have been presented, the results for the relative comparison of different media are finally illustrated. For this purpose, Figure 6 shows the averaged FRFs for the air media, water, transmission fluid 1, and transmission fluid 2. Air was added because it provides a different phase of matter in comparison to liquid matter. This way a change in the FRFs can be provoked.

From the relative comparison of the FRFs, it can be seen that compressible air medium has the lowest amplitude height up to a frequency of 5500 Hz. The FRF for air shows weakly damped resonances at 1200 Hz and 1800 Hz. Between 3000 Hz and 4000 Hz, multiple individual small resonances are evident.

Compared to air, the resonances of water media and transmission fluids are shifted in their resonance frequencies and strongly increased in their amplitude heights. In certain frequencies, differences by a factor of 1000 can be seen. The resonances for the incompressible media show higher damping in the resonances than is the case with the air medium.

The resonance frequencies between water and the two transmission fluids are slightly different for the resonances at 800 Hz, 2200 Hz, and 3100 Hz. The amplitude height of water is also slightly lower, in comparison to both transmission fluids.

In the frequency range from 3500 to 5500 Hz there are visible differences between the water media and the two transmission fluids. The resonance frequencies are shifted. In addition, the amplitude height drops for the water medium.

In the frequency range between 5500 and 7000 Hz, the air medium excels the incompressible media with two resonances at 5800 and 6600 Hz. The damping in the resonances is lower.

Above a frequency of 7000 Hz, amplitude values for the incompressible media are higher than for the air medium. The damping is also higher. The FRF of the air medium shows an anti-resonance and two resonance peaks above 7000 Hz. These resonances are weakly damped. Only the resonance at 7600 HZ is visible for all media, both in the resonance frequency and in the amplitude height. This could be caused by a structural resonance of the fixture.

4. Discussion

Summarizing the comparison between the media shows that the incompressible media have a similar curve shape. In addition, the incompressible media have higher amplitude values, compared to compressible air. Furthermore, incompressible media result in increased damping. Further, investigations are needed to determine how the viscosity and the compression modulus relate to the results shown.

“Pouring filling” for filling the fixture was found to be a suitable method. It represents a good compromise between the repeatability of the measurements and the handling of the fixture. Particularly for the two oils considered, the repeatability is drastically improved.

Relative comparison of the investigated media shows that incompressible media have increased transmission and propagation of excitation behaviour. The additional input of mass and incompressibility into the overall structural system also leads to increased damping of the structural system. Further detailed investigations are necessary to quantify in detail which mechanism is responsible for this behaviour of the structural system.

In addition, the mode shapes of the membrane can also be investigated. The resonance modes in the membrane can be identified and a possible change in resonance frequencies and modes can be considered. This can provide an additional understanding of the influence of the media on the device.

5. Conclusions

In the present work, a novel fixture for the transfer path analysis of fluids was evaluated and a relative comparison of fluids and media was completed. The FRFs for different media are shown in a frequency range up to 10 kHz. The relative comparison of different media is also possible. The results indicate that the fixture is suitable for its objective.

“Pouring filling” as the filling method of the device has been found to be a suitable method. This represents a good compromise between the repeatability of the measurements and the handling of the assembly of the test device. Particularly for the two oils considered, the repeatability improves. Future work should include further detailed investigations into repeatability. It is planned to quantify the repeatability and investigate possibilities and measures to improve the repeatability.

The relative comparison of the investigated media shows that incompressible media have increased transmission behaviour. The additional input of mass and incompressibility into the overall system also leads to increased damping of the system. Further detailed investigations are necessary to quantify which mechanism is responsible for this system behaviour. In addition, the mode shapes should be investigated. The resonance modes in the membrane can be identified and, consequently, a possible change of resonance frequencies and modes can be considered. This can provide a more in-depth understanding of the influence of the device on the media. Further studies are planned to investigate and analyse the viscosity and other material characteristics of the fluids for their possible influence on the transfer behaviour.

An absolute determination of the media parameters can be attained using FEA. Therefore, the fixture with the media needs to be modelled and validated in FEA.

Furthermore, the fixture can be preconditioned in the future. It is interesting to know how temperature and pressure affect the transfer function of the media. For this purpose, the fixture is already equipped with two inlet openings.

6. Patents

Schnell, M.; Strauch, U.; Urban, F. Verfahren zur Charakterisierung von dynamischen Eigenschaften eines Fluids; Daimler AG. Anmeldenr. 10 2019 007 910.8, Deutschland. 09.07.2020. Deutschland. Veröffentlichungsnr. DE 10 2019 007 910 A1.