Continuous Evaluation of Track Modulus from a Moving Railcar Using ANN-Based Techniques
Abstract
1. Introduction
2. The Stiffness Measurement System and Numerical Simulations
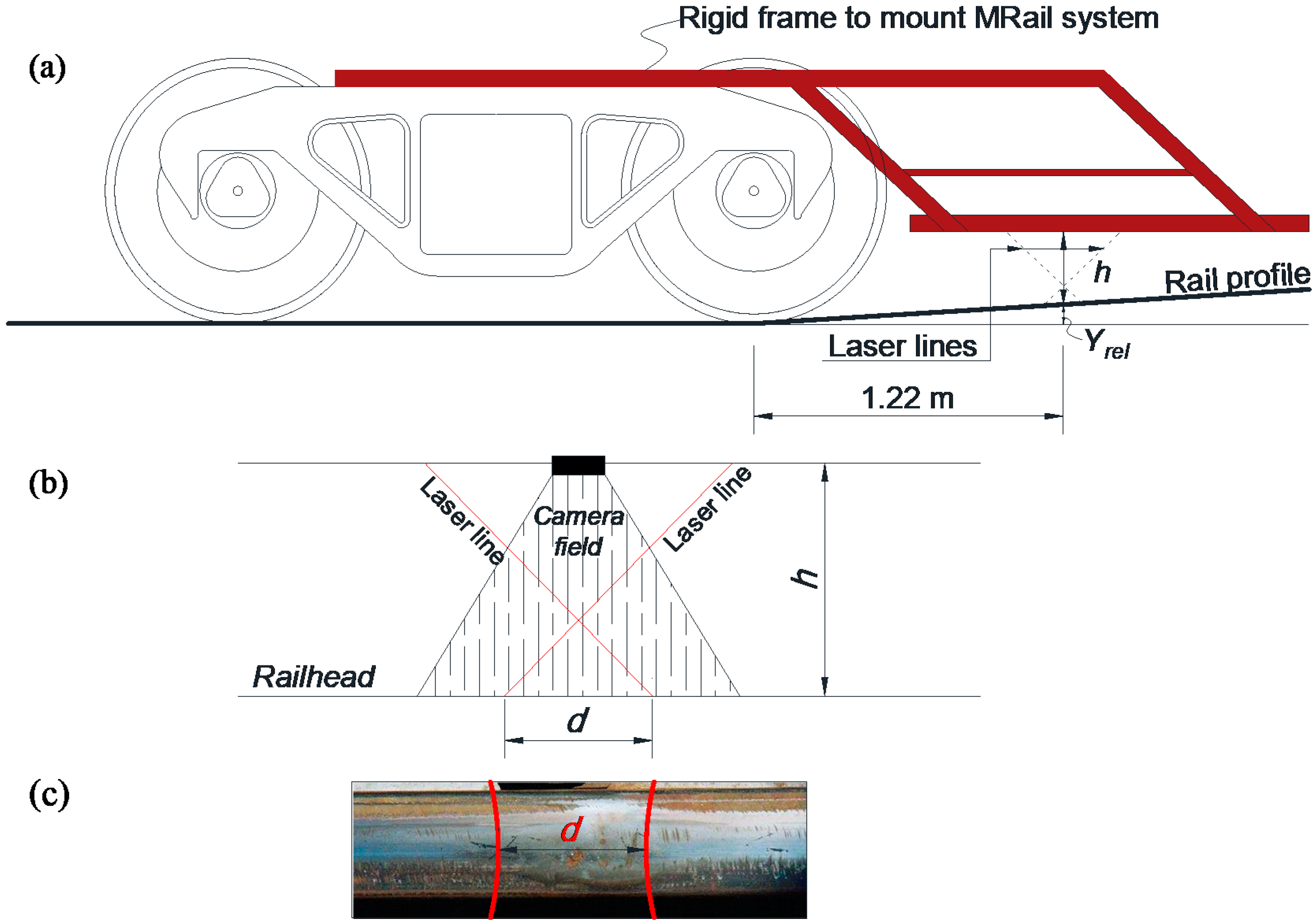
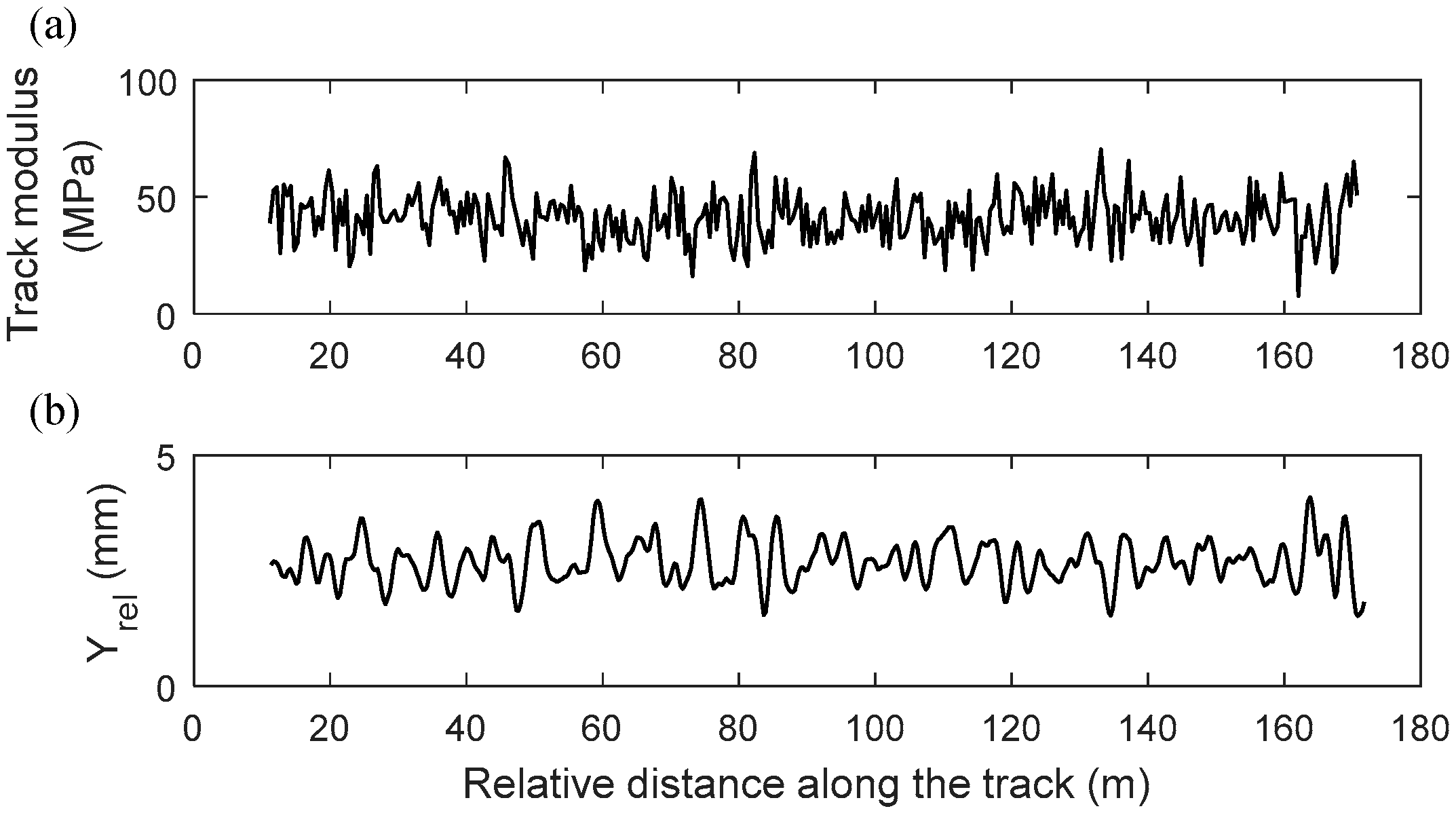
2.1. MRail Measurement System
2.2. Winkler Model
2.3. Finite Element Model
2.4. Estimation of Track Modulus Average
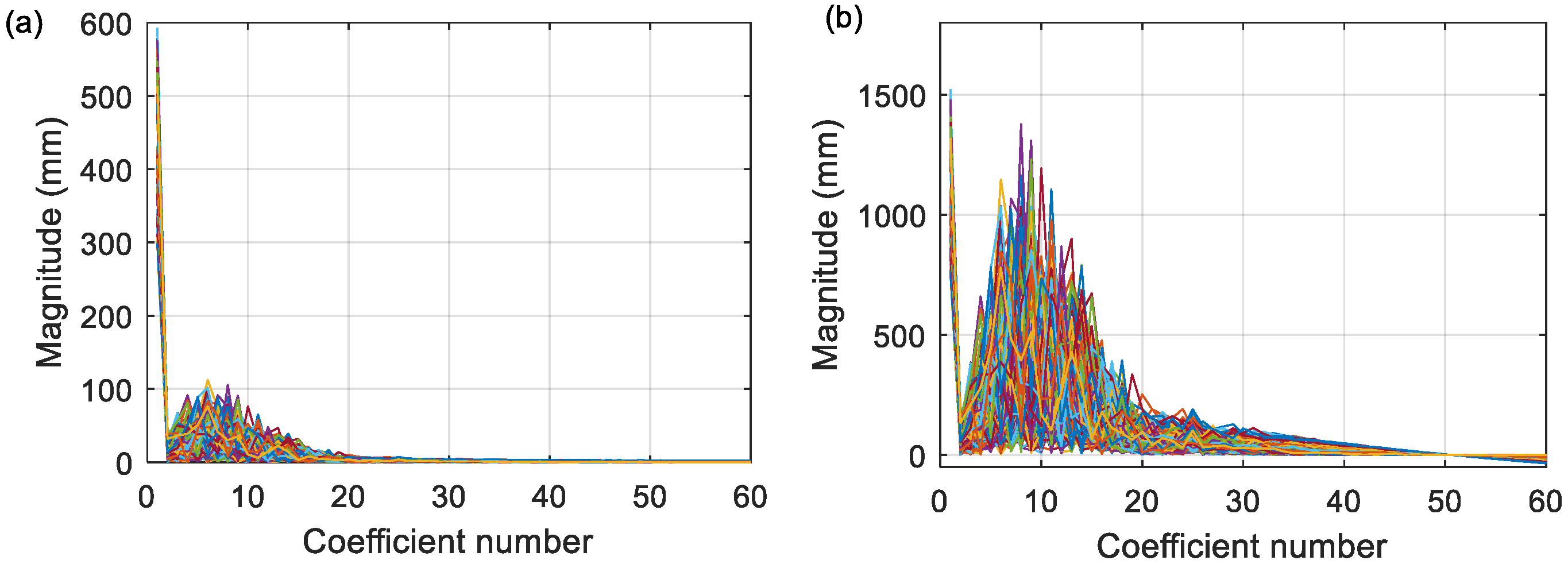
2.4.1. Multilayer Perceptron Artificial Neural Networks
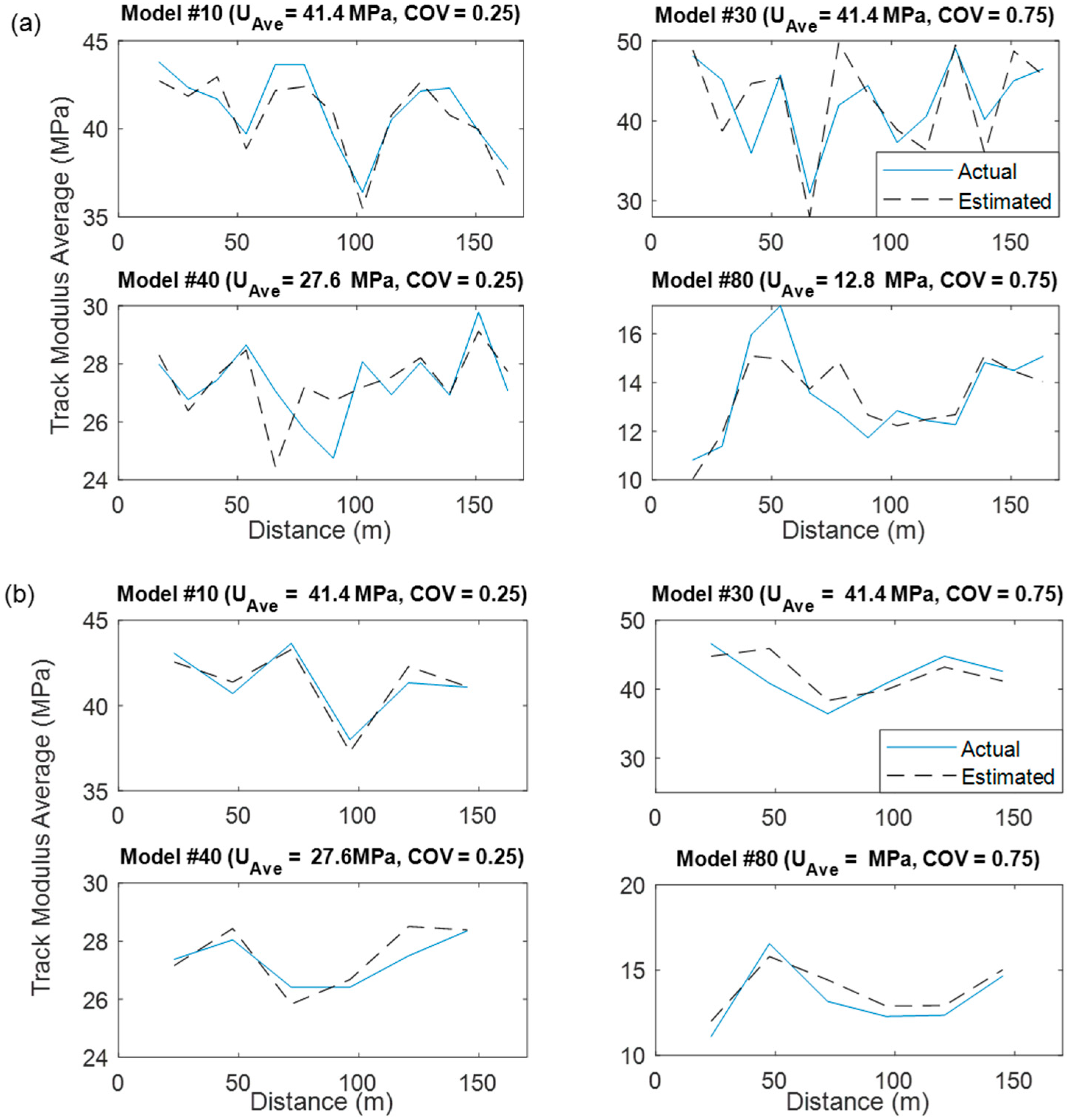
2.4.2. Estimation Procedure and Results
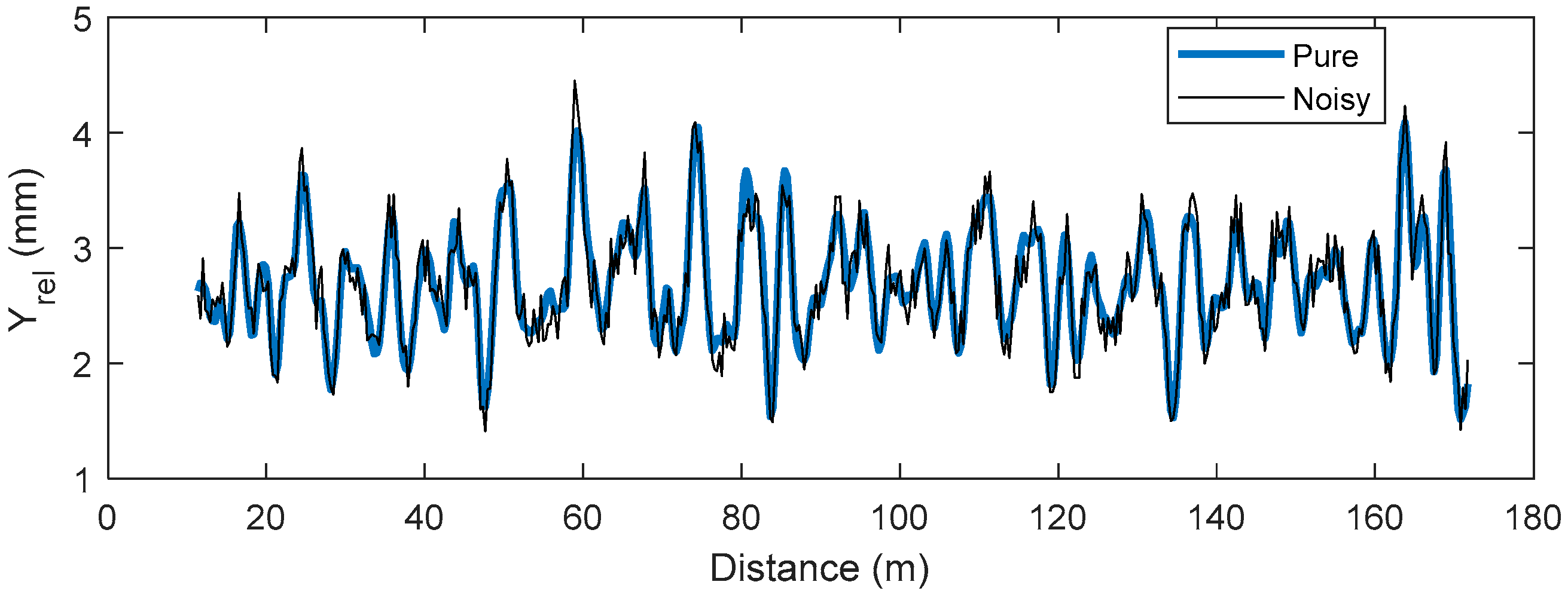
2.5. Estimation of Track Modulus Standard Deviation (USD)
3. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Selig, E.T.; Li, D. Track modulus: Its meaning and factors influencing it. Transp. Res. Rec. 1994, 1470, 47–54. [Google Scholar]
- Ebersohn, W.; Selig, E.T. Track modulus measurements on a heavy haul line. Transp. Res. Rec. 1994, 1470, 73. [Google Scholar]
- Lopez Pita, A.; Teixeira, P.F.; Robuste, F. High Speed and Track Deterioration: The Role of Vertical Stiffness of the Track. Proc. Inst. Mech. Eng. Pt. F J. Rail Rapid Transit. 2004, 218, 31–40. [Google Scholar] [CrossRef]
- Dahlberg, T. Railway Track Stiffness Variations-Consequences and Countermeasures. Int. J. Civ. Eng. 2010, 8, 1–12. [Google Scholar]
- Sussmann, T.R.; Ebersohn, W.; Selig, E.T. Fundamental nonlinear track load-deflection behavior for condition evaluation. Transp. Res. Rec. 2001, 61–67. [Google Scholar] [CrossRef]
- Burrow, M.P.N.; Chan, A.H.C.; Shein, A. Deflectometer-based analysis of ballasted railway tracks. Proc. Inst. Civ. Eng. Geotech. Eng. 2007, 160, 169–177. [Google Scholar] [CrossRef]
- Norman, C.; Farritor, S.; Arnold, R.; Elias, S.; Fateh, M.; El-Sibaie, M. Design of a System to Measure Track Modulus from a Moving Railcar; US Department of Transportation, Federal Railroad Administration, Office of Research and Development: Washington, DC, USA, 2006; paper ID 20590.
- Eric, G.B.; Nissen, A.; Björn, S.P. Track deflection and stiffness measurements from a track recording car. Proc. Inst. Mech. Eng. Pt. F J. Rail Rapid Transit. 2014, 228, 570–580. [Google Scholar] [CrossRef]
- Wang, P.; Wang, L.; Chen, R.; Xu, J.; Xu, J.; Gao, M. Overview and outlook on railway track stiffness measurement. J. Mod. Transp. 2016, 24, 89–102. [Google Scholar] [CrossRef]
- Lu, S. Real-Time Vertical Track Deflection Measurement System. Ph.D. Thesis, University of Nebraska Lincoln (UNL), Lincoln, Nebraska, 2008. [Google Scholar]
- Norman, C.D. Measurement of Track Modulus from a Moving Railcar. Master’s Thesis, University of Nebraska-Lincoln, Lincoln city, NE, USA, 2004. [Google Scholar]
- Greisen, C.J. Measurement, Simulation, and Analysis of the Mechanical Response of Railroad Track. Master’s Thesis, University of Nebraska-Lincoln, Lincoln city, NE, USA, 2010. [Google Scholar]
- Thompson, R.; Li, D. Automated vertical track strength testing using TTCI’s track loading vehicle. Technol. Digest. 2002, 1489, 17–25. [Google Scholar]
- Li, D.; Thompson, R.; Marquez, P.; Kalay, S. Development and Implementation of a Continuous Vertical Track-Support Testing Technique. Transp. Res. Rec. 2004, 1863, 68–73. [Google Scholar] [CrossRef]
- Rasmussen, S.; Krarup, J.A.; Hildebrand, G. Non-Contact Deflection Measurement at High Speed. In Proceedings of the 6th International Conference on the Bearing Capacity of Roads, Railways and Airfields, Lisbon, Portugal, 24–26 June 2002. [Google Scholar]
- Berggren, E.G.; Kaynia, A.M.; Dehlbom, B. Identification of substructure properties of railway tracks by dynamic stiffness measurements and simulations. J. Sound Vib. 2010, 329, 3999–4016. [Google Scholar] [CrossRef]
- With, C.; Metrikine, A.V.; Bodare, A. Identification of effective properties of the railway substructure in the low-frequency range using a heavy oscillating unit on the track. Arch. Appl. Mech. 2010, 80, 959–968. [Google Scholar] [CrossRef][Green Version]
- Roghani, A.; Hendry, M.; Ruel, M.; Edwards, T.; Sharpe, P.; Hyslip, J. A case study of the assessment of an existing rail line for increased traffic and axle loads. In Proceedings of the IHHA 2015 Conference, Peth, Australia, 21–24 June 2015. [Google Scholar]
- Roghani, A.; Hendry, M.T. Assessing the potential of a technology to map the subgrade stiffness under the rail tracks. In Proceedings of the Transportation research board 94th annual meeting; Transportation Research Board, Washington, DC, USA, 11–15 January 2015. [Google Scholar]
- Roghani, A.; Macciotta, R.; Hendry, M. Combining track quality and performance measures to assess track maintenance requirements. In Proceedings of the ASME/ASCE/IEEE 2015 Joint Rail Conference, American Society of Mechanical Engineers, San Jose, CA, USA, 23–26 March 2015. [Google Scholar]
- Fallah Nafari, S.; Gül, M.; Roghani, A.; Hendry, M.T.; Cheng, J.R. Evaluating the potential of a rolling deflection measurement system to estimate track modulus. Proc. Inst. Mech. Eng. Pt. F J Rail Rapid Transit. 2018, 232, 14–24. [Google Scholar] [CrossRef]
- Mehrali, M.; Esmaeili, M.; Mohammadzadeh, S. Application of data mining techniques for the investigation of track geometry and stiffness variation. Proc. Inst. Mech. Eng. Pt. F J Rail Rapid Transit. 2019, 234, 439–453. [Google Scholar] [CrossRef]
- Lu, S.; Hogan, C.; Minert, B.; Arnold, R.; Farritor, S.; GeMeiner, W.; Clark, D. Exception criteria in vertical track deflection and modulus. In 2007 ASME/IEEE Joint Rail Conference and the ASME Internal Combustion Engine Division, Spring Technical Conference (JRCICE 2007); American Society of Mechanical Engineers: Pueblo, CO, USA, 2007; pp. 191–198. [Google Scholar]
- El-Sibaie, M.; GeMeiner, W.; Clark, D.; Al-Nazer, L.; Arnold, R.; Farritor, S.; Fateh, M.; Lu, S.; Carr, G. Measurement of Vertical Track Modulus: Field Testing, Repeatability, and Effects of Track Geometry. In Proceedings of the ASME/IEEE Joint Rail Conference, Wilmington, DE, USA, 22–24 April 2009; pp. 151–158. [Google Scholar]
- Alireza, R.; Hendry, M.T. Continuous Vertical Track Deflection Measurements to Map Subgrade Condition along a Railway Line: Methodology and Case Studies. J. Transp. Eng. 2016, 142, 04016059. [Google Scholar] [CrossRef]
- Fallah Nafari, S.; Gül, M.; Hendry, M.T.; Cheng, J.R. Estimation of vertical bending stress in rails using train-mounted vertical track deflection measurement systems. Proc. Inst. Mech. Eng. Pt. F J Rail Rapid Transit. 2018, 232, 1528–1538. [Google Scholar] [CrossRef]
- Sadeghi, J.; Barati, P. Evaluation of conventional methods in Analysis and Design of Railway Track System. IJCE 2010, 8, 44–56. [Google Scholar]
- Esveld, C. Modern Railway Track; MRT Productions: Duisburg, Germany, 1989; p. 446. [Google Scholar]
- Feng, H. 3D-Models of Railway Track for Dynamic Analysis; Royal Institute of Technology: Stockholm, Sweden, 2011. [Google Scholar]
- Fallah Nafari, S.; Gül, M.; Cheng, J.J.R. Quantifying live bending moments in rail using train-mounted vertical track deflection measurements and track modulus estimations. J. Civ. Struct. Health Monit. 2017, 7, 637–643. [Google Scholar] [CrossRef]
- Zakeri, J.A.; Abbasi, R. Field investigation on variation of rail support modulus in ballasted railway tracks. Lat. Am. J. Solids Struct. 2012, 9, 643–656. [Google Scholar] [CrossRef]
- Sussmann, T.R.; Thompson, H.B.; Stark, T.D.; Wilk, S.T.; Ho, C.L. Use of seismic surface wave testing to assess track substructure condition. Constr. Build. Mater. 2017, 155, 1250–1255. [Google Scholar] [CrossRef]
- Bridge, C. Computers and Structures; CSI: Berkeley, CA, USA, 2015. [Google Scholar]
- Haykin, S.S. Neural Networks and Learning Machines; Prentice Hall: New York, NY, USA, 2009; p. 906. [Google Scholar]
- Cybenko, G. Approximation by superpositions of a sigmoidal function. Math. Control Signals Syst. 1989, 2, 303–314. [Google Scholar] [CrossRef]
- Guler, H. Prediction of railway track geometry deterioration using artificial neural networks: A case study for Turkish state railways. Struct. Infrastruct. Eng. 2014, 10, 614–626. [Google Scholar] [CrossRef]
- Fink, O.; Weidmann, U. Scope and potential of applying artificial neural networks in reliability prediction with a focus on railway rolling stock. In European Safety and Reliability Conference: Advances in Safety, Reliability and Risk Management, ESREL 2011; Taylor and Francis Inc.: Troyes, France, 2012; pp. 508–514. [Google Scholar]
- Sadeghi, J.; Askarinejad, H. Application of neural networks in evaluation of railway track quality condition. J. Mech. Sci. Technol. 2012, 26, 113–122. [Google Scholar] [CrossRef]
- Hyndman, R.J.; Koehler, A.B. Another look at measures of forecast accuracy. Int. J. Forecast 2006, 22, 679–688. [Google Scholar] [CrossRef]
- Fallah Nafari, S. Quantifying the Distribution of Rail Bending Stresses along the Track Using Train-Mounted Deflection Measurements; University of Alberta: Edmonton, AB, Canada, 2017. [Google Scholar]
- Young, S.; Evermann, G.; Gales, M.; Hain, T.; Kershaw, D.; Liu, X.; Moore, G.; Odell, J.; Ollason, D.; Povey, D. The HTK Book; Cambridge University Engineering Department: Cambridge, UK, 2006. [Google Scholar]




| Track Modulus Average (MPa) | Coefficient of Variation (COV) | No. of Simulations |
|---|---|---|
| 41.4 | 0.25; 0.5; 0.75 | 30 (10 simulations for each COV) |
| 27.6 | 0.25; 0.5; 0.75 | 30 (10 simulations for each COV) |
| 12.8 | 0.25; 0.5; 0.75 | 30 (10 simulations for each COV) |
| Section Length (m) | MAPE * (%) | RMSE ** (MPa) | R2 | R2 in [21] |
|---|---|---|---|---|
| 5 | 12.42 | 4.58 | 0.86 | 0.79 |
| 10 | 6.99 | 2.81 | 0.95 | 0.93 |
| 15 | 5.90 | 2.56 | 0.95 | N/A |
| 20 | 4.32 | 1.60 | 0.98 | 0.96 |
| 25 | 3.87 | 1.63 | 0.98 | N/A |
| Section Length (m) | MAPE (%) | R2 | RMSE (MPa) | R2 in [21] |
|---|---|---|---|---|
| 5 | 14.09 | 0.83 | 5.07 | 0.79 |
| 10 | 7.01 | 0.95 | 2.77 | 0.93 |
| 15 | 5.93 | 0.96 | 2.36 | - * |
| 20 | 6.07 | 0.97 | 1.98 | 0.96 |
| 25 | 3.98 | 0.98 | 1.53 | - * |
| Section Length (m) | Network Configuration | RMSE (MPa) | MAPE (%) | R2 | R2 in [21] |
|---|---|---|---|---|---|
| 10 | ANN-1 | 3.00 (0.16 *) | 18.41 | 0.83 | 0.53 |
| ANN-2 | 3.05 (0.22) | 19.12 | 0.82 | - | |
| 15 | ANN-1 | 2.36 (0.08) | 15.01 | 0.89 | - |
| ANN-2 | 2.61 (0.39) | 15.79 | 0.87 | - | |
| 20 | ANN-1 | 2.23 (0.11) | 14.49 | 0.91 | 0.66 |
| ANN-2 | 2.59 (0.89) | 14.47 | 0.88 | - | |
| 25 | ANN-1 | 1.83 (0.13) | 11.96 | 0.94 | - |
| ANN-2 | 1.99 (0.30) | 11.72 | 0.92 | - | |
| 30 | ANN-1 | 2.08 (0.17) | 11.61 | 0.92 | - |
| ANN-2 | 2.14 (0.44) | 11.79 | 0.91 | - |
| Section Length (m) | Network Configuration | R2 | RMSE (MPa) | MAPE (%) |
|---|---|---|---|---|
| 10 | ANN-1 | 0.82 | 3.06 | 20.12 |
| ANN-2 | 0.81 | 3.14 | 19.64 | |
| 15 | ANN-1 | 0.87 | 2.59 | 16.30 |
| ANN-2 | 0.87 | 2.64 | 16.23 | |
| 20 | ANN-1 | 0.89 | 2.42 | 16.13 |
| ANN-2 | 0.89 | 2.45 | 14.71 | |
| 25 | ANN-1 | 0.94 | 1.86 | 11.96 |
| ANN-2 | 0.93 | 1.88 | 11.73 | |
| 30 | ANN-1 | 0.94 | 1.84 | 10.43 |
| ANN-2 | 0.93 | 1.89 | 10.95 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Do, N.T.; Gül, M.; Nafari, S.F. Continuous Evaluation of Track Modulus from a Moving Railcar Using ANN-Based Techniques. Vibration 2020, 3, 149-161. https://doi.org/10.3390/vibration3020012
Do NT, Gül M, Nafari SF. Continuous Evaluation of Track Modulus from a Moving Railcar Using ANN-Based Techniques. Vibration. 2020; 3(2):149-161. https://doi.org/10.3390/vibration3020012
Chicago/Turabian StyleDo, Ngoan T., Mustafa Gül, and Saeideh Fallah Nafari. 2020. "Continuous Evaluation of Track Modulus from a Moving Railcar Using ANN-Based Techniques" Vibration 3, no. 2: 149-161. https://doi.org/10.3390/vibration3020012
APA StyleDo, N. T., Gül, M., & Nafari, S. F. (2020). Continuous Evaluation of Track Modulus from a Moving Railcar Using ANN-Based Techniques. Vibration, 3(2), 149-161. https://doi.org/10.3390/vibration3020012





