Numerical Simulation of Soot Formation in Ethylene Laminar Diffusion Flame
Abstract
1. Introduction
2. Numerical Model Establishment
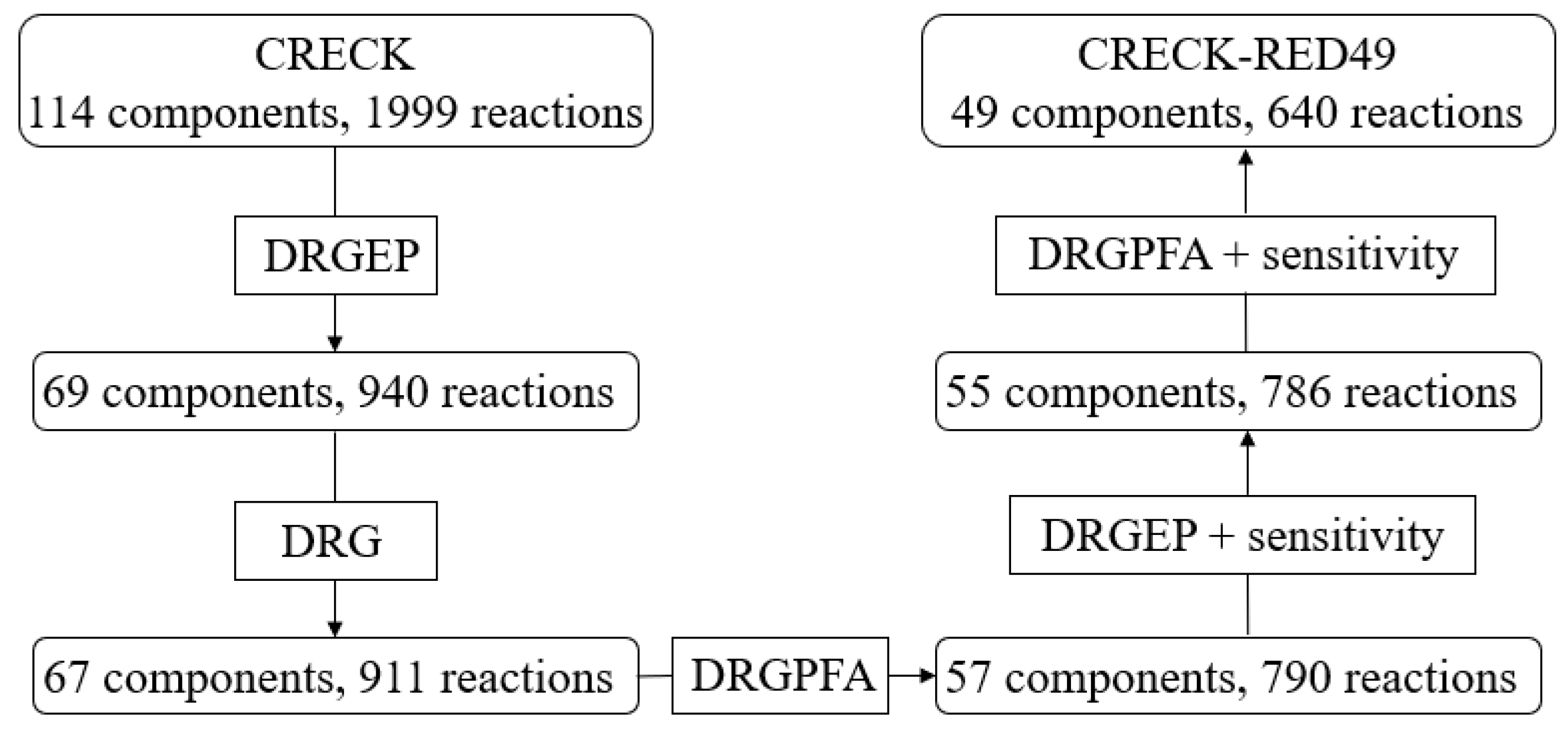
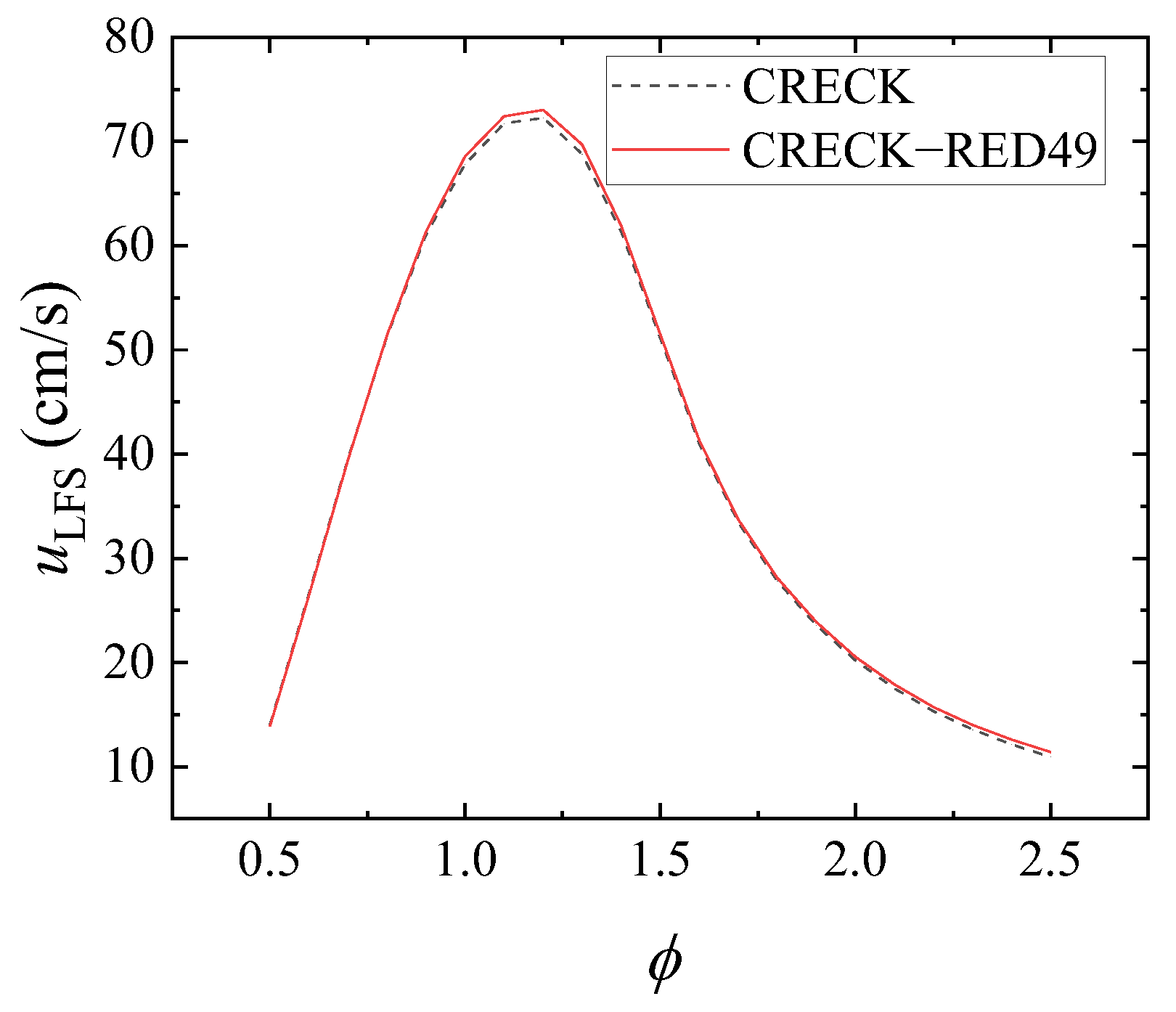
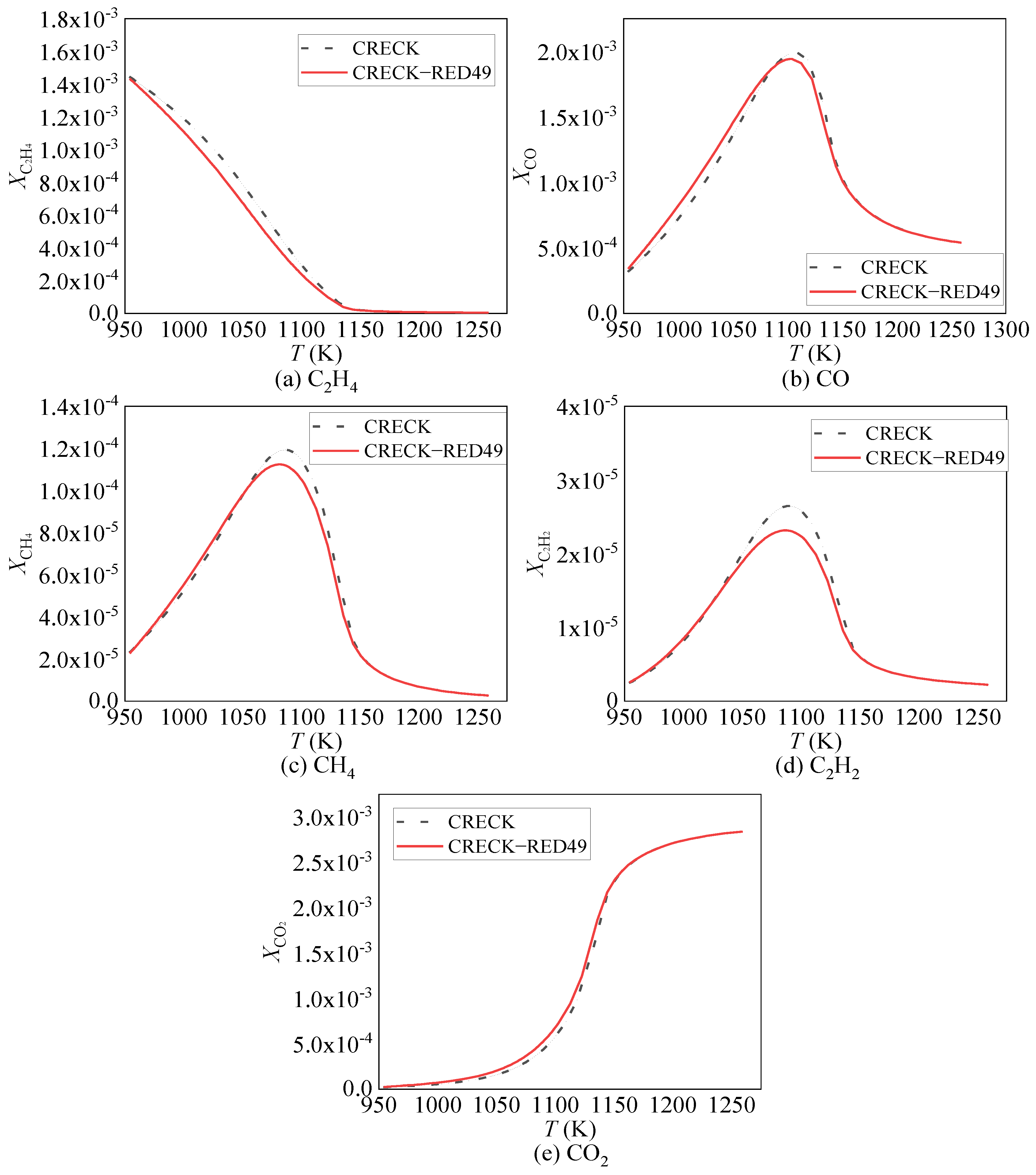
2.1. Simplification of Chemical Kinetics Model
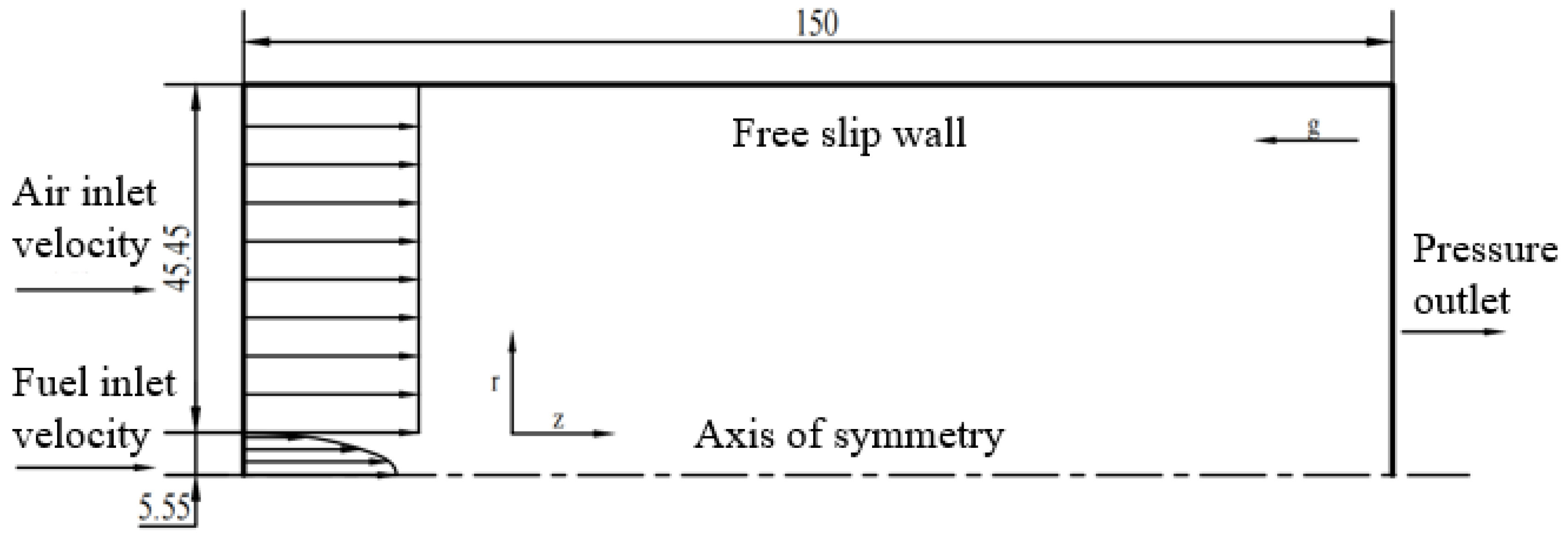
2.2. Calculation Domain, Grid, and Boundary Conditions
2.3. Fluid Mechanics Model
2.4. Radiation Model
2.5. Soot Model
3. Results and Discussion
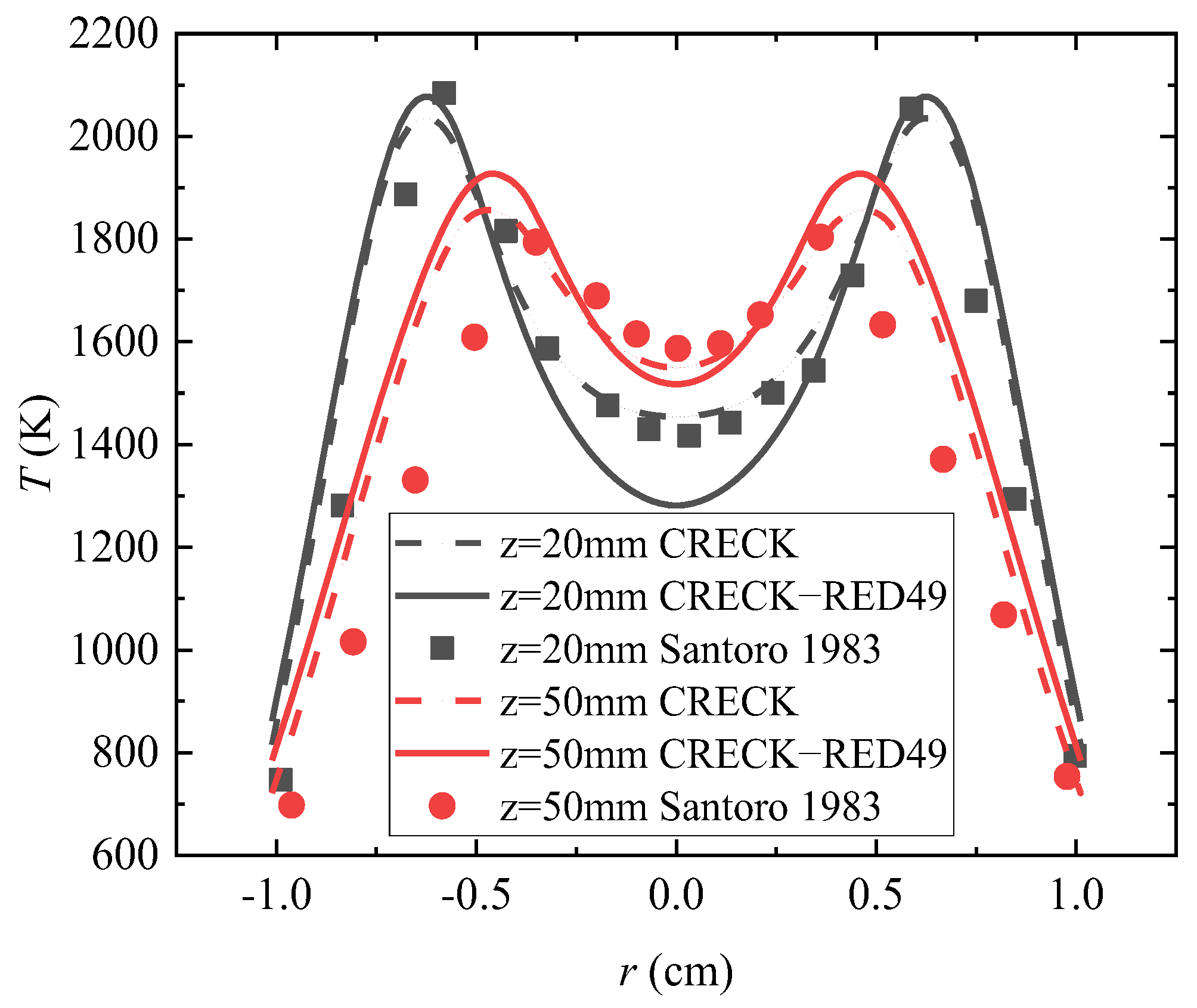
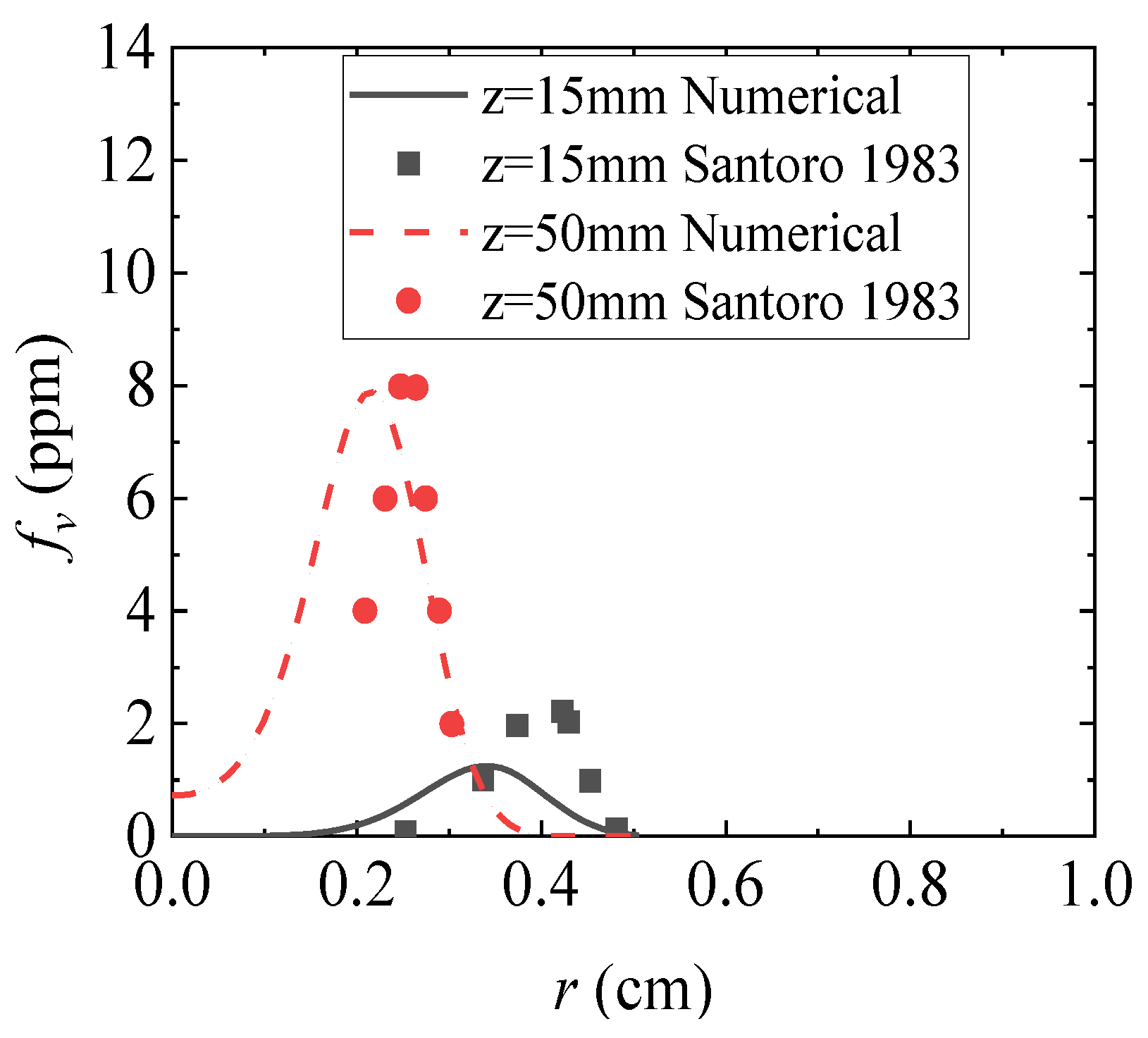
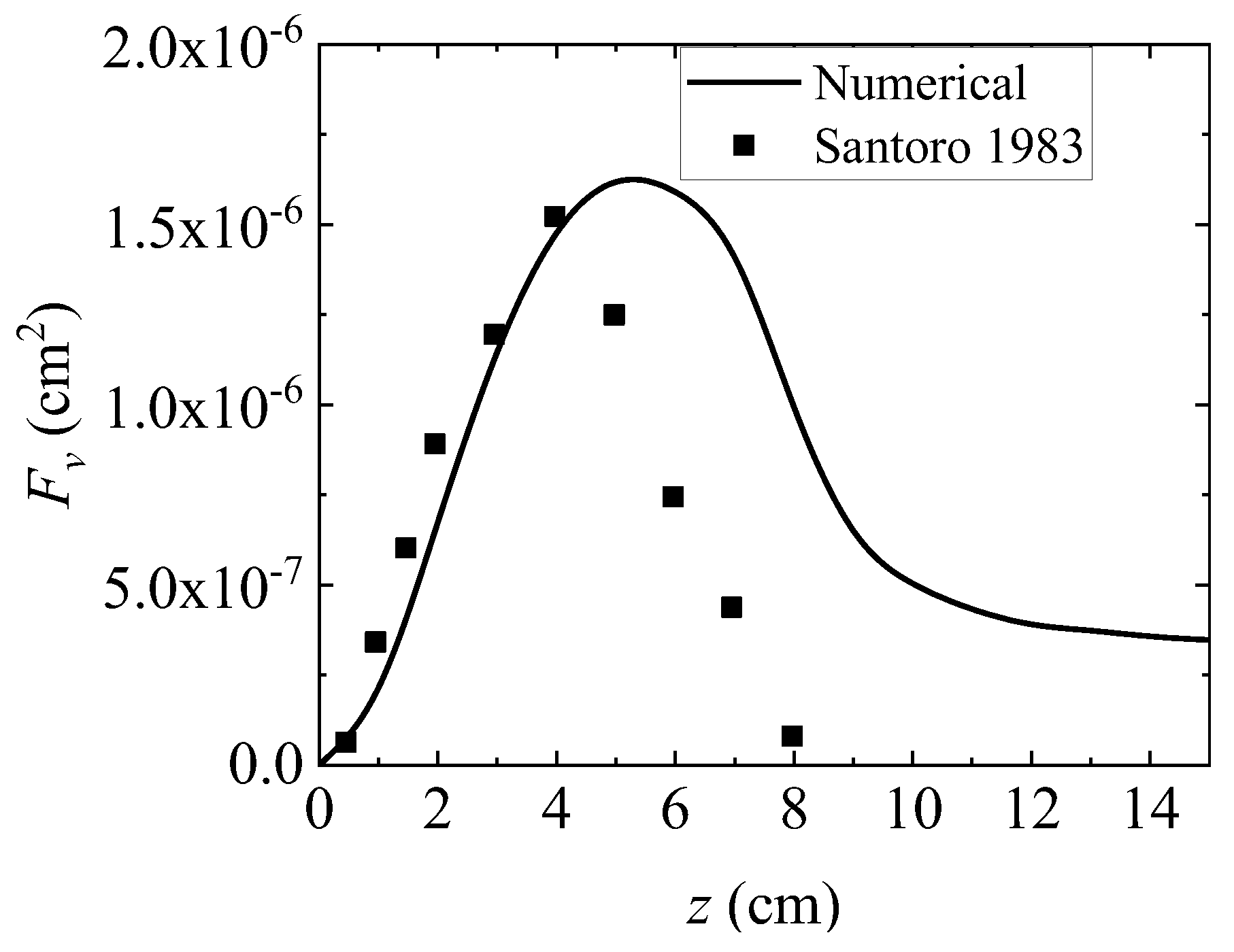
3.1. Verification of the Calculation Model
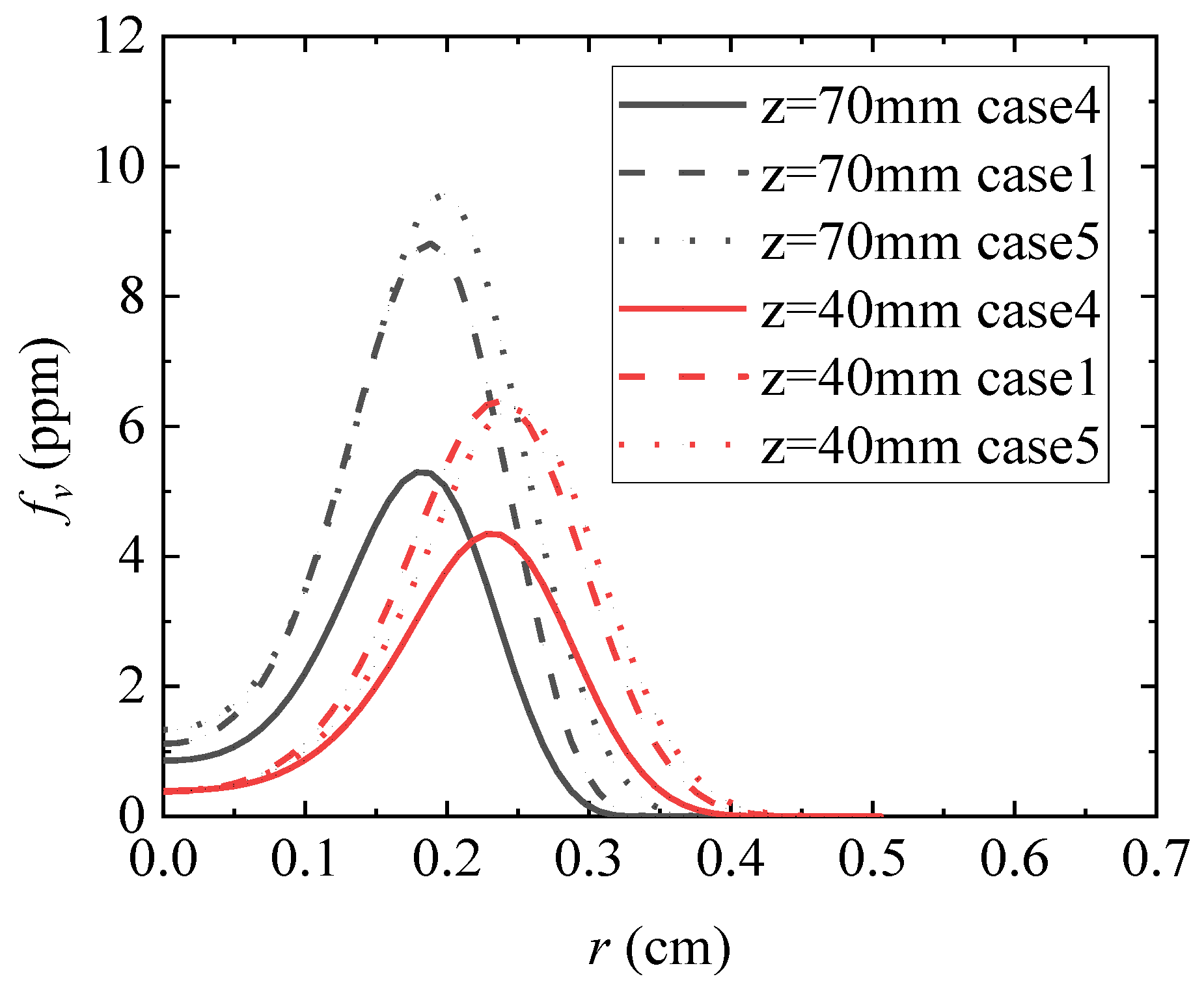
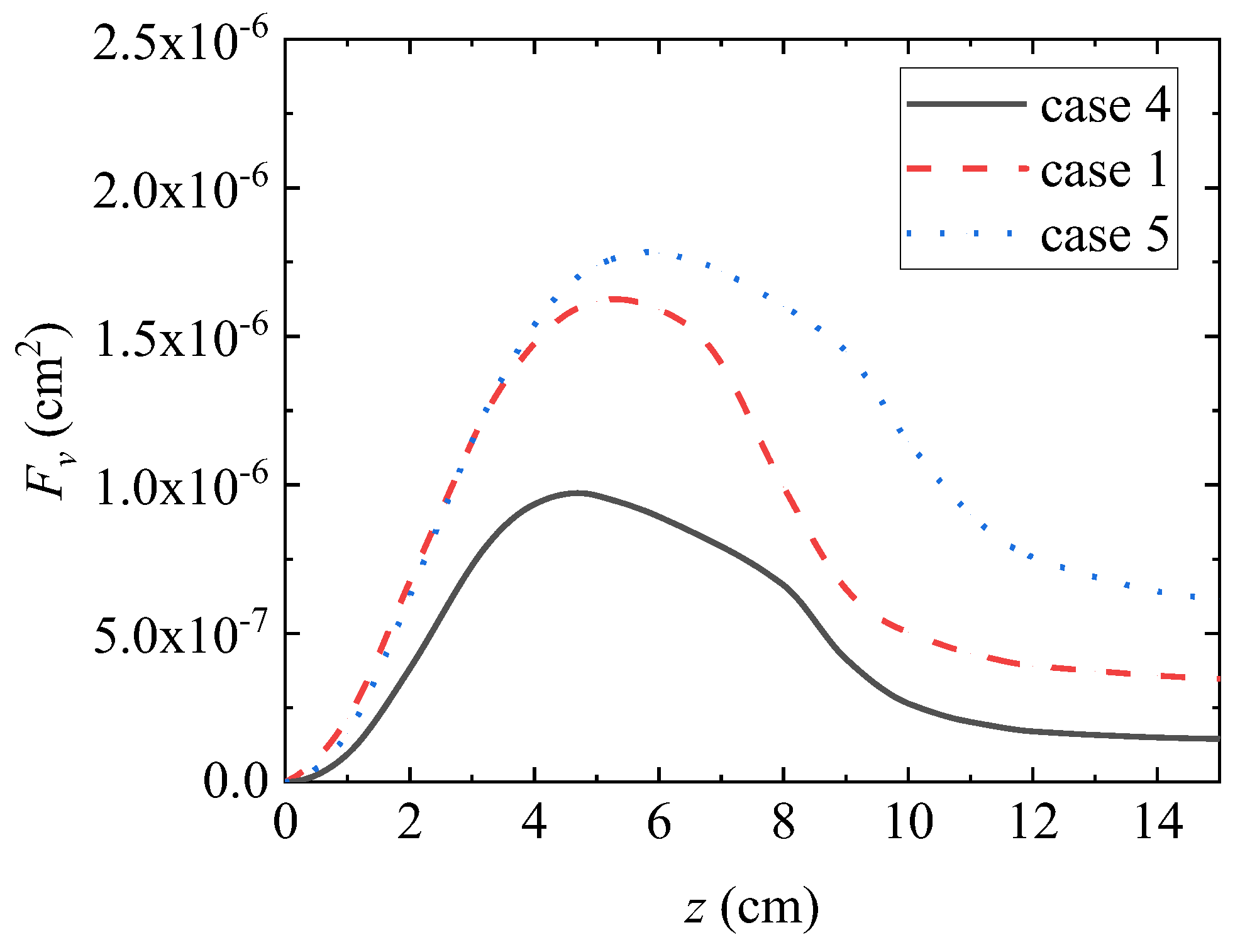
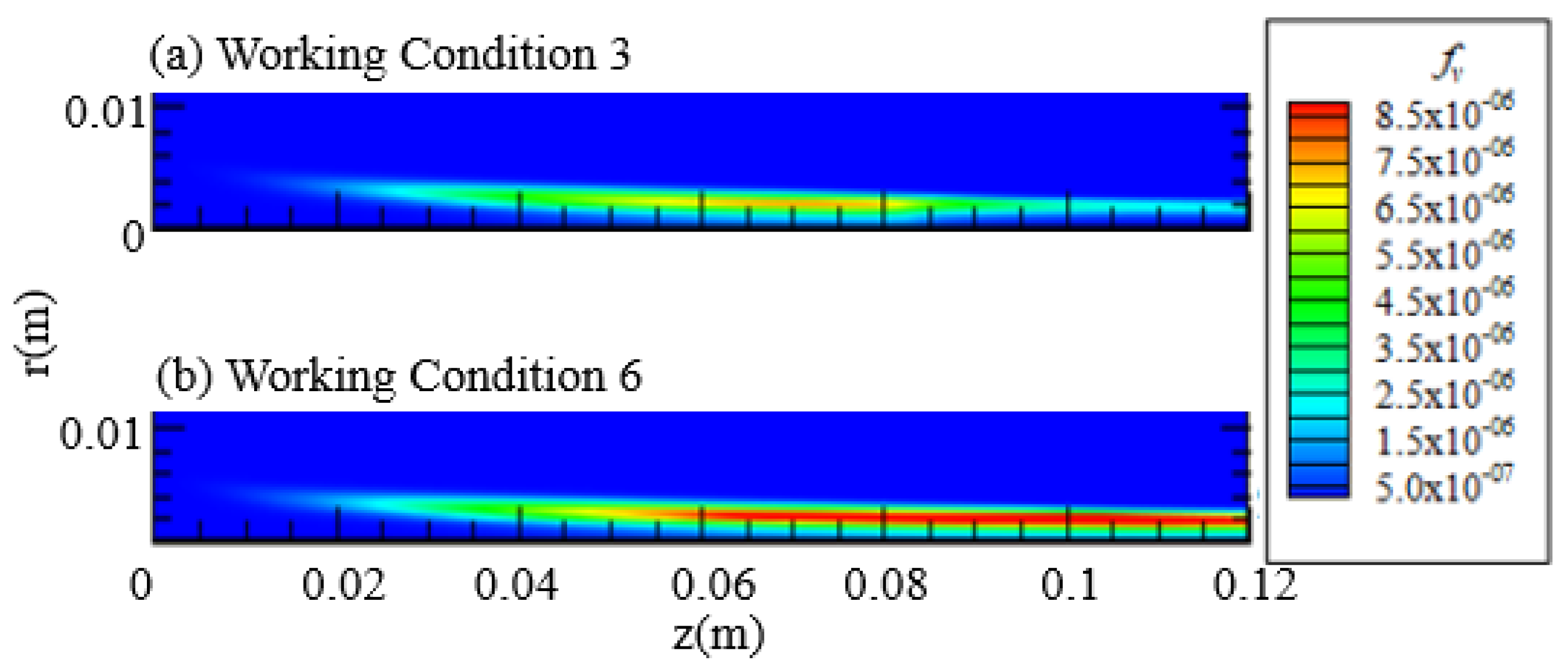
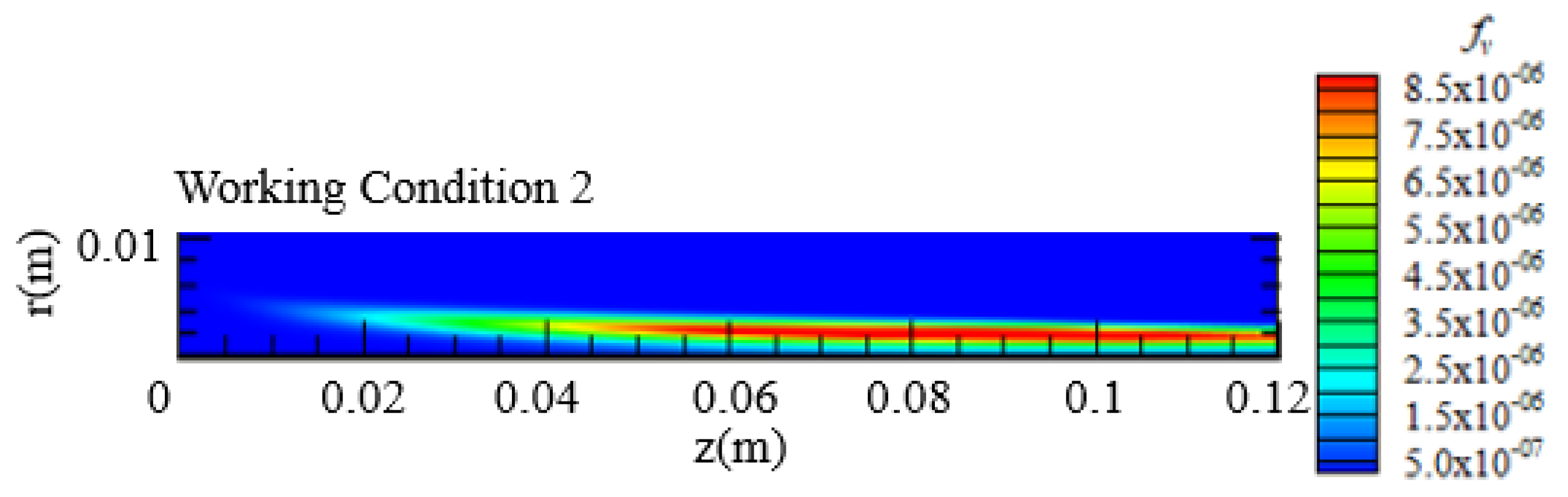
3.2. Influencing Factors of Soot Generation in Laminar Diffusion Flame of Ethylene
4. Conclusions
- (1)
- In terms of mechanism simplification, the detailed chemical reaction mechanism model, CRECK model, was simplified according to the demand for the accuracy of specific physical quantities in the follow-up study and the demand for saving computing resources. A simplified model, CRECK-RED49, consisting of 49 components and 640 elementary reactions was obtained, and the calculation accuracy was verified to meet the accuracy requirements for soot generation calculation.
- (2)
- For the improvement of soot model, a two-path soot solver was developed to calculate the soot volume concentration distribution in the laminar coaxial jet diffusion flame of ethylene. The calculated results obtained are consistent with the experimental data in terms of distribution trend. The deviation of the calculated peak integral smoke volume fraction is only 5%.
- (3)
- Concentration of soot is influenced by processes such as nucleation, surface growth, and oxidation. When the volume flow rate of the ac-companying air is sufficient, the volume fraction of soot in each axial position will first increase and then decrease with the increase of fuel volume flow rate. When the fuel volume flow rate reaches 4.60 cm3/s, the volume fraction of soot reaches its peak.
- (4)
- In terms of the influence of gas additives on the formation of soot, the addition of H2, CO2, and H2O in the fuel flow can suppress the formation of soot in ethylene laminar diffusion flames. From the perspective of thermal effect, dilution effect, and chemical effect on a single precursor C2H2, the addition of H2O has the most significant inhibitory effect on soot formation.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bond, T.C.; Doherty, S.J.; Fahey, D.W.; Forster, P.M.; Berntsen, T.; DeAngelo, B.J.; Flanner, M.G.; Ghan, S.; Kärcher, B.; Koch, D.; et al. Bounding the role of black carbon in the climate system: A scientific assessment. J. Geophys. Res. Atmos. 2013, 118, 5380–5552. [Google Scholar]
- Zheng, S.; Zhang, Y.; Yang, Y.; Lu, Q. Blending Effects of Nitrogenous Bio-Fuel on Soot and NOX Formation during Gasoline Combustion. Energy Fuels 2023, 37, 4596–4607. [Google Scholar]
- Ying, Y.; Liu, D. Effects of n-Butanol Addition on the Combustion Characteristics of n-Heptane Counterflow Diffusion Flame at Elevated Pressure. Fire 2022, 5, 154. [Google Scholar] [CrossRef]
- Zhu, X.; Liu, B.; Guo, C.; Li, Z.; Cheng, M.; Zhu, X.; Wei, Y. Short and long-term association of exposure to ambient black carbon with all-cause and cause-specific mortality: A systematic review and meta-analysis. Environ. Pollut. 2023, 324, 121086. [Google Scholar] [CrossRef] [PubMed]
- Jerez, A.; Consalvi, J.-L.; Fuentes, A.; Liu, F.; Demarco, R. Soot production modeling in a laminar coflow ethylene diffusion flame at different Oxygen Indices using a PAH-based sectional model. Fuel 2018, 231, 404–416. [Google Scholar] [CrossRef]
- Wang, Y.; Liu, X.; Gao, Y.; Gu, M.; An, X. Numerical simulations on effects of oxygen concentration on the structure and soot formation in a two-dimensional axisymmetric laminar C2H4/(O-2-CO2) diffusion flame. J. Therm. Anal. Calorim. 2019, 137, 689–702. [Google Scholar]
- Eaves, N.A.; Zhang, Q.; Liu, F.; Guo, H.; Dworkin, S.B.; Thomson, M.J. CoFlame: A refined and validated numerical algorithm for modeling sooting laminar coflow diffusion flames. Comput. Phys. Commun. 2016, 207, 464–477. [Google Scholar]
- Zheng, S.; Yang, Y.; Sui, R.; Lu, Q. Effects of C2H2 and C2H4 radiation on soot formation in ethylene/air diffusion flames. Appl. Therm. Eng. 2021, 183, 116194. [Google Scholar]
- Jerez, A.; Villanueva, J.C.; da Silva, L.F.; Demarco, R.; Fuentes, A. Measurements and modeling of PAH soot precursors in coflow ethylene/air laminar diffusion flames. Fuel 2019, 236, 452–460. [Google Scholar] [CrossRef]
- Zimmer, L.; Kostic, S.; Dworkin, S.B. A novel soot concentration field estimator applied to sooting ethylene/air laminar flames. Eng. Appl. Comput. Fluid Mech. 2019, 13, 470–481. [Google Scholar] [CrossRef]
- Zimmer, L.; Pereira, F. Limitations of simplified models to predict soot formation in laminar fames. J. Braz. Soc. Mech. Sci. Eng. 2020, 42, 340. [Google Scholar] [CrossRef]
- Cepeda, F.; Jerez, A.; Demarco, R.; Liu, F.; Fuentes, A. Influence of water-vapor in oxidizer stream on the sooting behavior for laminar coflow ethylene diffusion flames. Combust. Flame 2019, 210, 114–125. [Google Scholar] [CrossRef]
- Wang, Y.; Liu, X.; Gu, M.; An, X. Numerical simulation of the effects of hydrogen addition to fuel on the structure and soot formation of a laminar axisymmetric coflow C2H4/(O-2-CO2) diffusion flame. Combust. Sci. Technol. 2019, 191, 1743–1768. [Google Scholar] [CrossRef]
- Liu, F.; Guo, H.; Smallwood, G.J. Evaluation of the laminar diffusion flamelet model in the calculation of an axisymmetric coflow laminar ethylene–air diffusion flame. Combust. Flame 2006, 144, 605–618. [Google Scholar] [CrossRef]
- Qiu, L.; Hua, Y.; Zhuang, Y.; Wei, J.; Qian, Y.; Cheng, X. Numerical investigation into the decoupling effects of hydrogen blending on flame structure and soot formation in a laminar ethylene diffusion flame. Int. J. Hydrogen Energy 2020, 45, 15672–15682. [Google Scholar] [CrossRef]
- Yang, Y.; Zheng, S.; He, Y.; Liu, H.; Sui, R.; Lu, Q. Effects of simultaneous CO2 addition to the fuel and oxidizer streams on soot formation in co-flow diffusion ethylene flame. Fuel 2023, 353, 129181. [Google Scholar] [CrossRef]
- Yang, Y.; Zheng, S.; Sui, R.; Lu, Q. Impact of ammonia addition on soot and NO/N2O formation in methane/air co-flow diffusion flames. Combust. Flame 2023, 247, 112483. [Google Scholar] [CrossRef]
- Ranzi, E.; Frassoldati, A.; Grana, R.; Cuoci, A.; Faravelli, T.; Kelley, A.P.; Law, C.K. Hierarchical and comparative kinetic modeling of laminar flame speeds of hydrocarbon and oxygenated fuels. Prog. Energy Combust. Sci. 2012, 38, 468–501. [Google Scholar] [CrossRef]
- Wang, W.; Gou, X. A mechanism reduction method integrating path flux analysis with multi generations and sensitivity analysis. Combust. Sci. Technol. 2017, 189, 24–42. [Google Scholar] [CrossRef]
- Lu, T.F.; Law, C.K. A directed relation graph method for mechanism reduction. Proc. Combust. Inst. 2005, 30, 1333–1341. [Google Scholar] [CrossRef]
- Nurislamova, L.F.; Gubaydullin, I.M. Mechanism reduction of chemical reaction based on sensitivity analysis: Development and testing of some new procedure. J. Math. Chem. 2017, 55, 1779–1792. [Google Scholar] [CrossRef]
- Kopp, M.M.; Donato, N.S.; Petersen, E.L.; Metcalfe, W.K.; Burke, S.M.; Curran, H.J. Oxidation of ethylene–air mixtures at elevated pressures, Part 1: Experimental results. J. Propuls. Power 2014, 30, 790–798. [Google Scholar] [CrossRef]
- Van Treek, L.; Roth, N.; Seidel, L.; Mauß, F. Measurements of the laminar burning velocities of rich ethylene/air mixtures. Fuel 2020, 275, 117938. [Google Scholar] [CrossRef]
- Le Cong, T.; Bedjanian, E.; Dagaut, P. Oxidation of ethylene and propene in the presence of CO2 and H2O: Experimental and detailed kinetic modeling study. Combust. Sci. Technol. 2010, 182, 333–349. [Google Scholar] [CrossRef]
- Santoro, R.J.; Semerjian, H.G.; Dobbins, R.A. Soot particle measurements in diffusion flames. Combust. Flame 1983, 51, 203–218. [Google Scholar] [CrossRef]
- Sert, İ.O.; Sezer-Uzol, N. Numerical analysis of convective heat transfer of nanofluids in circular ducts with two-phase mixture model approach. Heat Mass Transf. 2016, 52, 1841–1850. [Google Scholar] [CrossRef]
- Dorigon, L.J.; Duciak, G.; Brittes, R.; Cassol, F.; Galarça, M.; França, F.H. WSGG correlations based on HITEMP2010 for computation of thermal radiation in non-isothermal, non-homogeneous H2O/CO2 mixtures. Int. J. Heat Mass Transf. 2013, 64, 863–873. [Google Scholar] [CrossRef]
- ANSYS, I. Fluent Theroy Guide [EB/OL]. Available online: https://ansyshelp.ansys.com/account/secured?returnurl=/Views/Secured/corp/v211/en/flu_th/flu_th.html (accessed on 9 February 2023).
- Leung, K.M.; Lindstedt, R.P.; Jones, W.P. A simplified reaction-mechanism for soot formation in nonpremixed flames. Combust. Flame 1991, 87, 289–305. [Google Scholar] [CrossRef]
- Liu, F.; Guo, H.; Smallwood, G.J.; Gülder, Ö.L. Numerical modelling of soot formation and oxidation in laminar coflow non-smokifig and smoking ethylene diffusion flames. Combust. Theory Model. 2003, 7, 301–315. [Google Scholar] [CrossRef]
- Lee, K.B.; Thring, M.W.; Beer, J.M. On the rate of combustion of soot in a laminar soot flame. Combust. Flame 1962, 6, 137–145. [Google Scholar] [CrossRef]
- Santoro, R.J.; Yeh, T.T.; Horvath, J.J.; Semerjian, H.G. The transport and growth of soot particles in laminar diffusion flames. Combust. Sci. Technol. 1987, 53, 89–115. [Google Scholar] [CrossRef]
- Gu, M.; Chu, H.; Liu, F. Effects of simultaneous hydrogen enrichment and carbon dioxide dilution of fuel on soot formation in an axisymmetric coflow laminar ethylene/air diffusion flame. Combust. Flame 2016, 166, 216–228. [Google Scholar] [CrossRef]





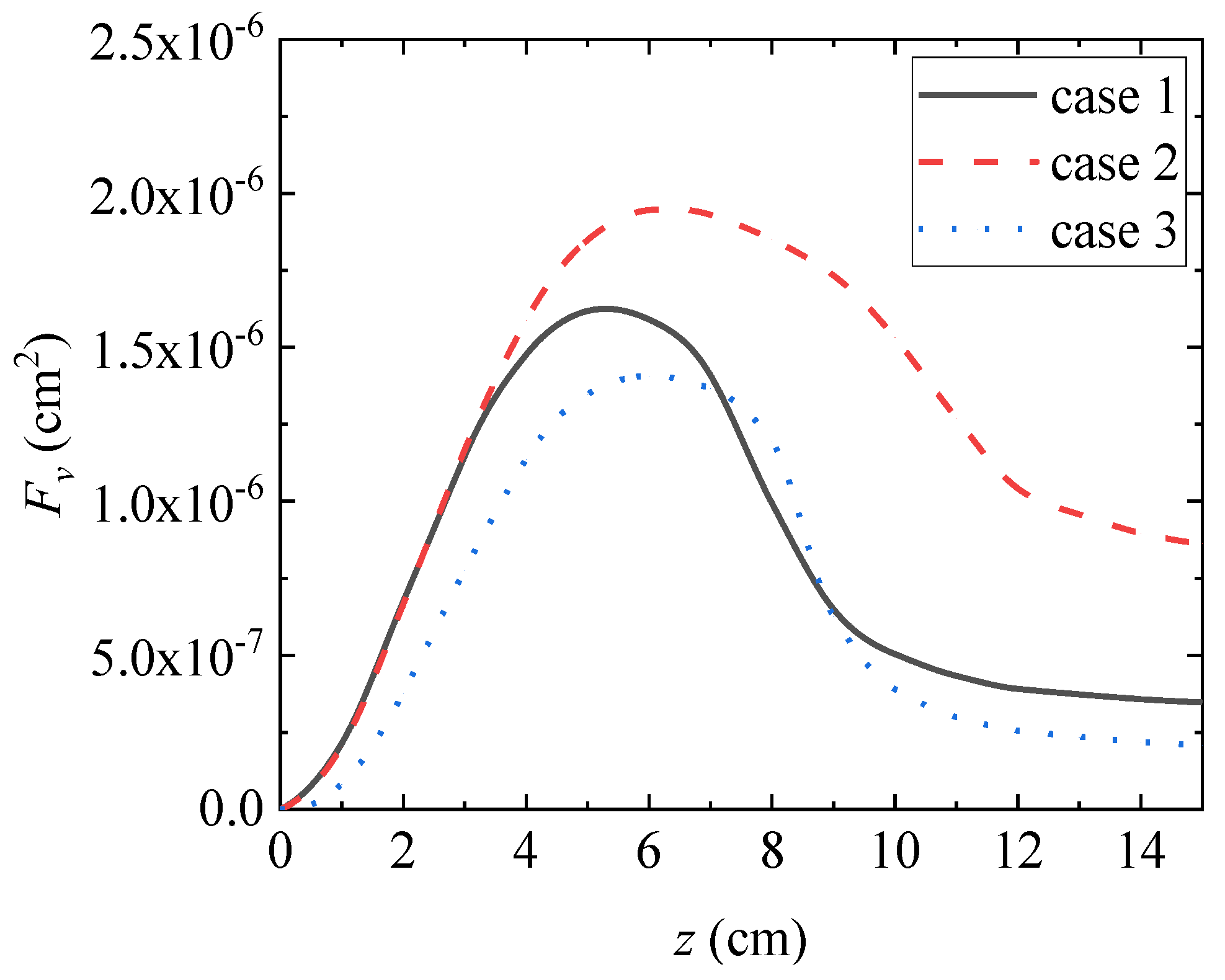

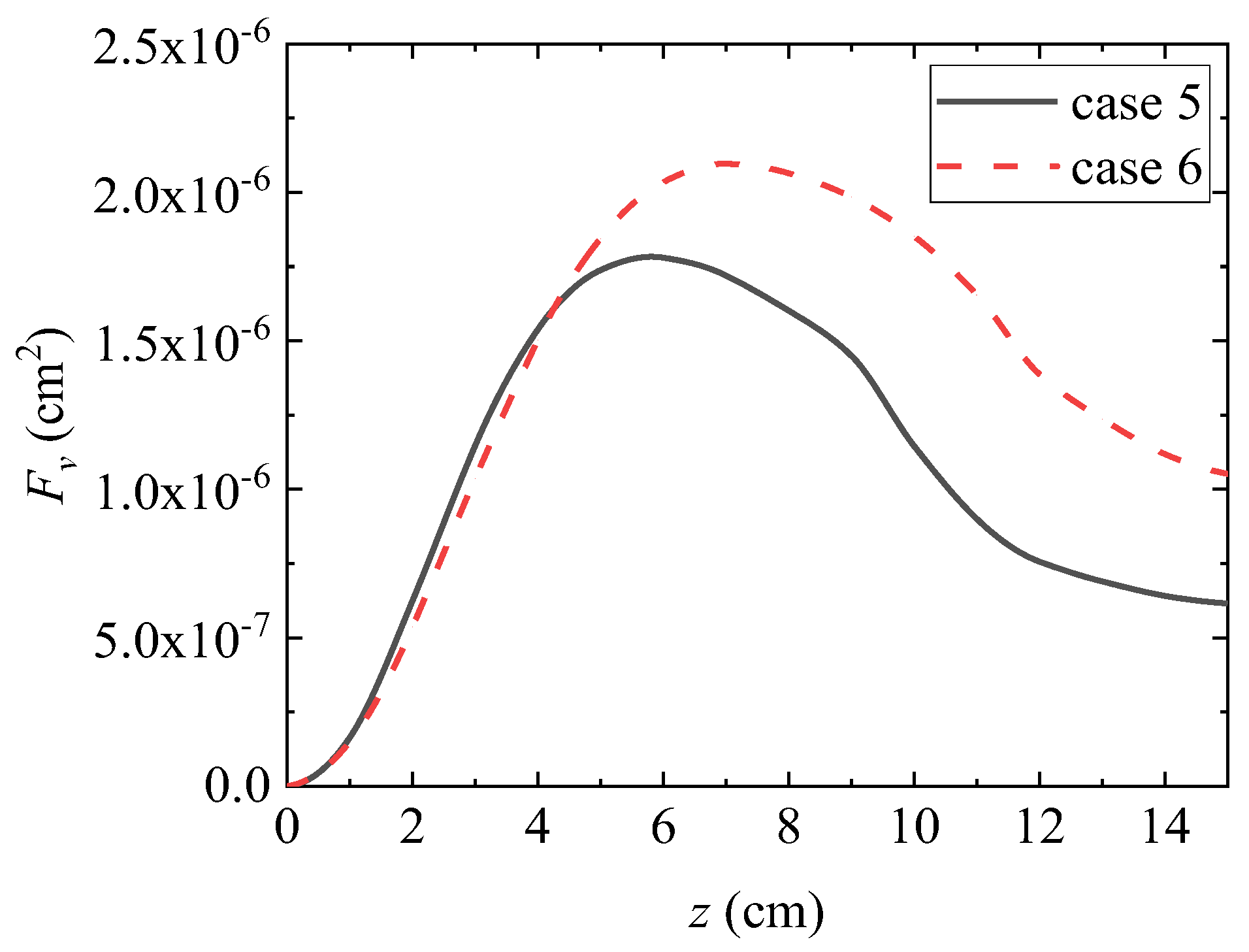
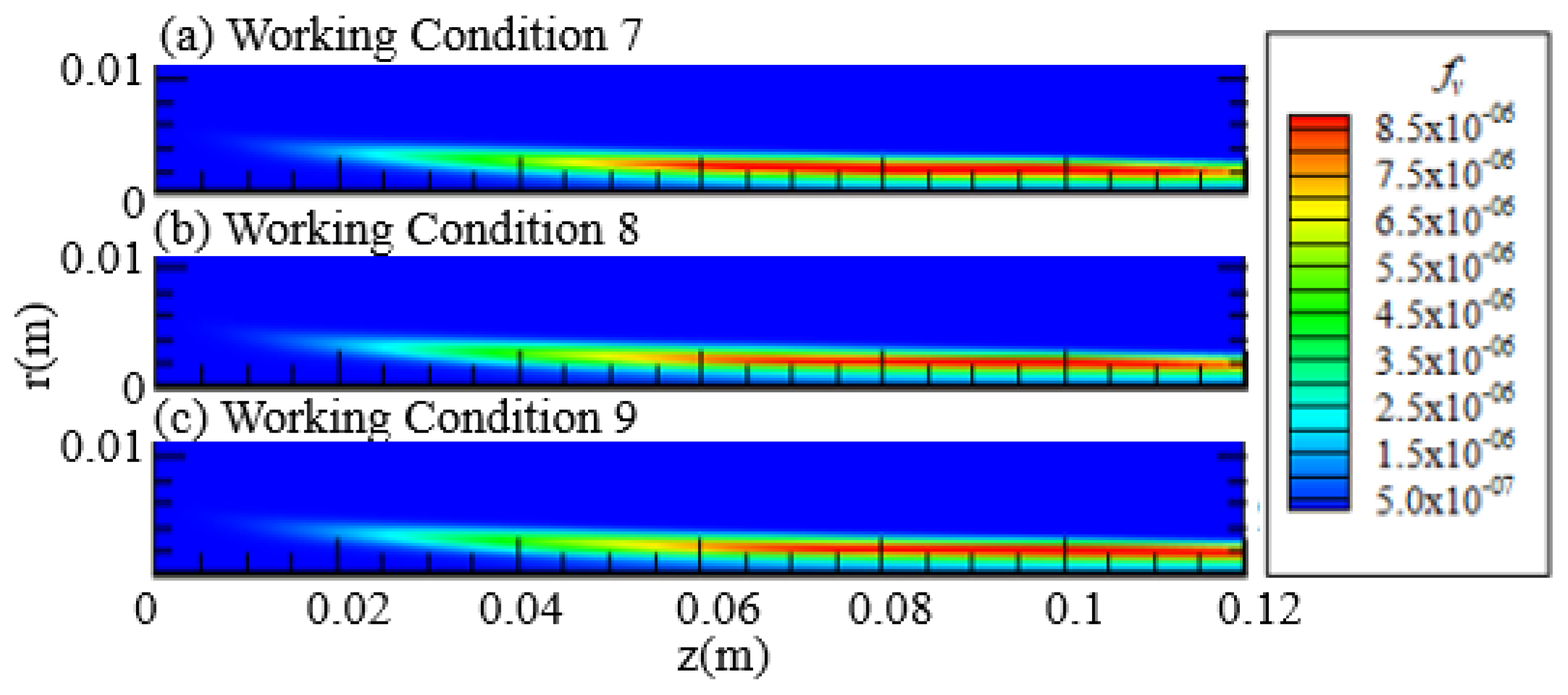
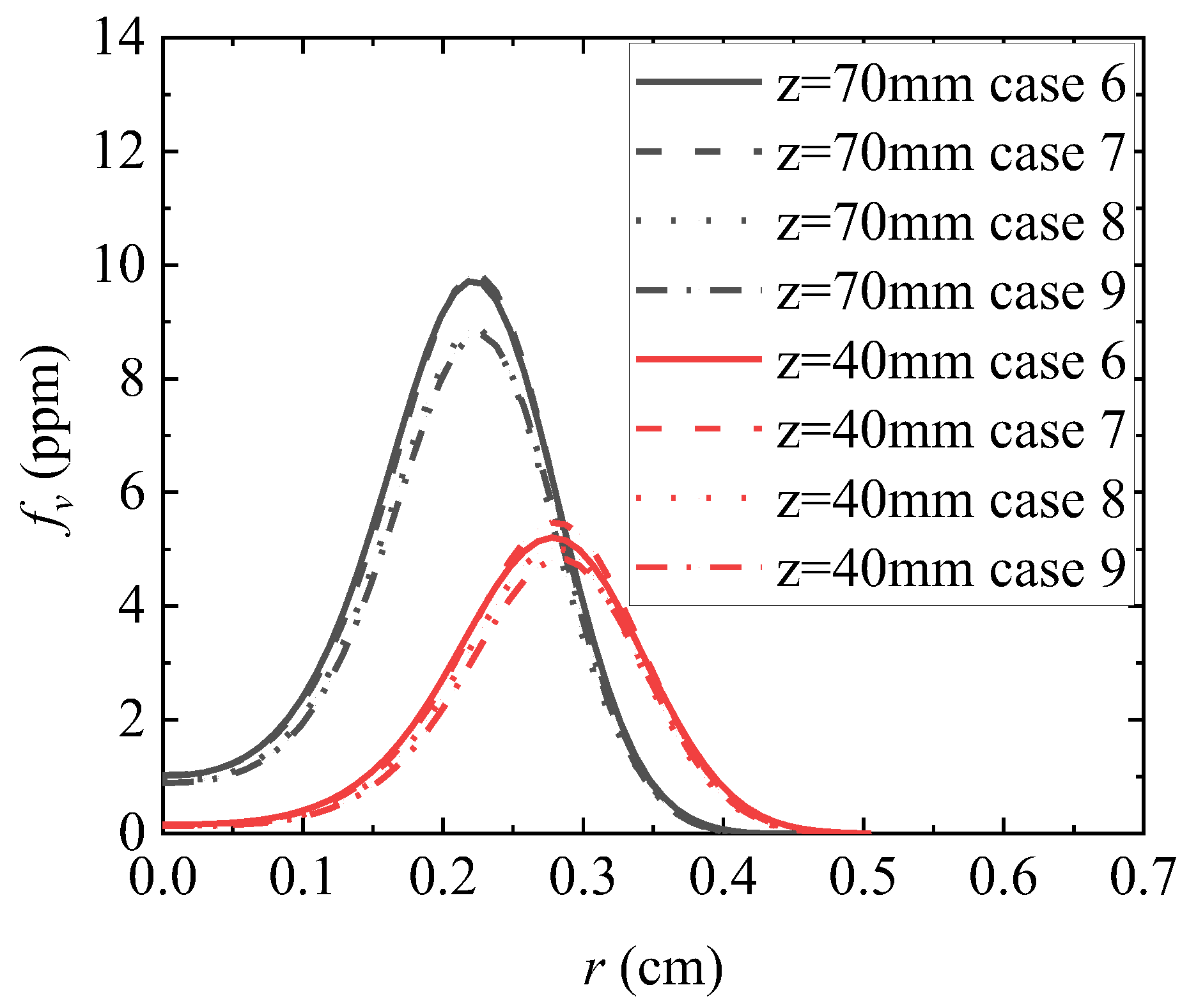

| Working Condition | Average Velocity of Fuel Inlet (m/s) | Composition of Mole Fraction of Fuel Flow Components | Average Velocity of Air Inlet (m/s) | Composition of Mole Fraction of Air Flow Components |
|---|---|---|---|---|
| 1 | 0.0398 | C2H4 100% | 0.089 | O2 21% |
| N2 79% | ||||
| 2 | 0.0475 | C2H4 100% | 0.089 | O2 21% |
| N2 79% | ||||
| 3 | 0.0506 | C2H4 100% | 0.089 | O2 21% |
| N2 79% | ||||
| 4 | 0.0398 | C2H4 100% | 0.062 | O2 21% |
| N2 79% | ||||
| 5 | 0.0398 | C2H4 100% | 0.133 | O2 21% |
| N2 79% | ||||
| 6 | 0.0506 | C2H4 100% | 0.133 | O2 21% |
| N2 79% | ||||
| 7 | 0.0557 | C2H4 90% | 0.133 | O2 21% |
| H2 10% | N2 79% | |||
| 8 | 0.0557 | C2H4 90% | 0.133 | O2 21% |
| H2O 10% | N2 79% | |||
| 9 | 0.0557 | C2H4 90% | 0.133 | O2 21% |
| CO2 10% | N2 79% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gao, X.-Y.; Yang, F.; Zhang, C.-X.; Chen, Q.-X.; Yuan, Y. Numerical Simulation of Soot Formation in Ethylene Laminar Diffusion Flame. Fire 2023, 6, 316. https://doi.org/10.3390/fire6080316
Gao X-Y, Yang F, Zhang C-X, Chen Q-X, Yuan Y. Numerical Simulation of Soot Formation in Ethylene Laminar Diffusion Flame. Fire. 2023; 6(8):316. https://doi.org/10.3390/fire6080316
Chicago/Turabian StyleGao, Xiu-Yan, Fan Yang, Chuan-Xin Zhang, Qi-Xiang Chen, and Yuan Yuan. 2023. "Numerical Simulation of Soot Formation in Ethylene Laminar Diffusion Flame" Fire 6, no. 8: 316. https://doi.org/10.3390/fire6080316
APA StyleGao, X.-Y., Yang, F., Zhang, C.-X., Chen, Q.-X., & Yuan, Y. (2023). Numerical Simulation of Soot Formation in Ethylene Laminar Diffusion Flame. Fire, 6(8), 316. https://doi.org/10.3390/fire6080316





