Spherical Diffusion Flames of Ethylene in Microgravity: Multidimensional Effects
Abstract
1. Introduction
2. Materials and Methods
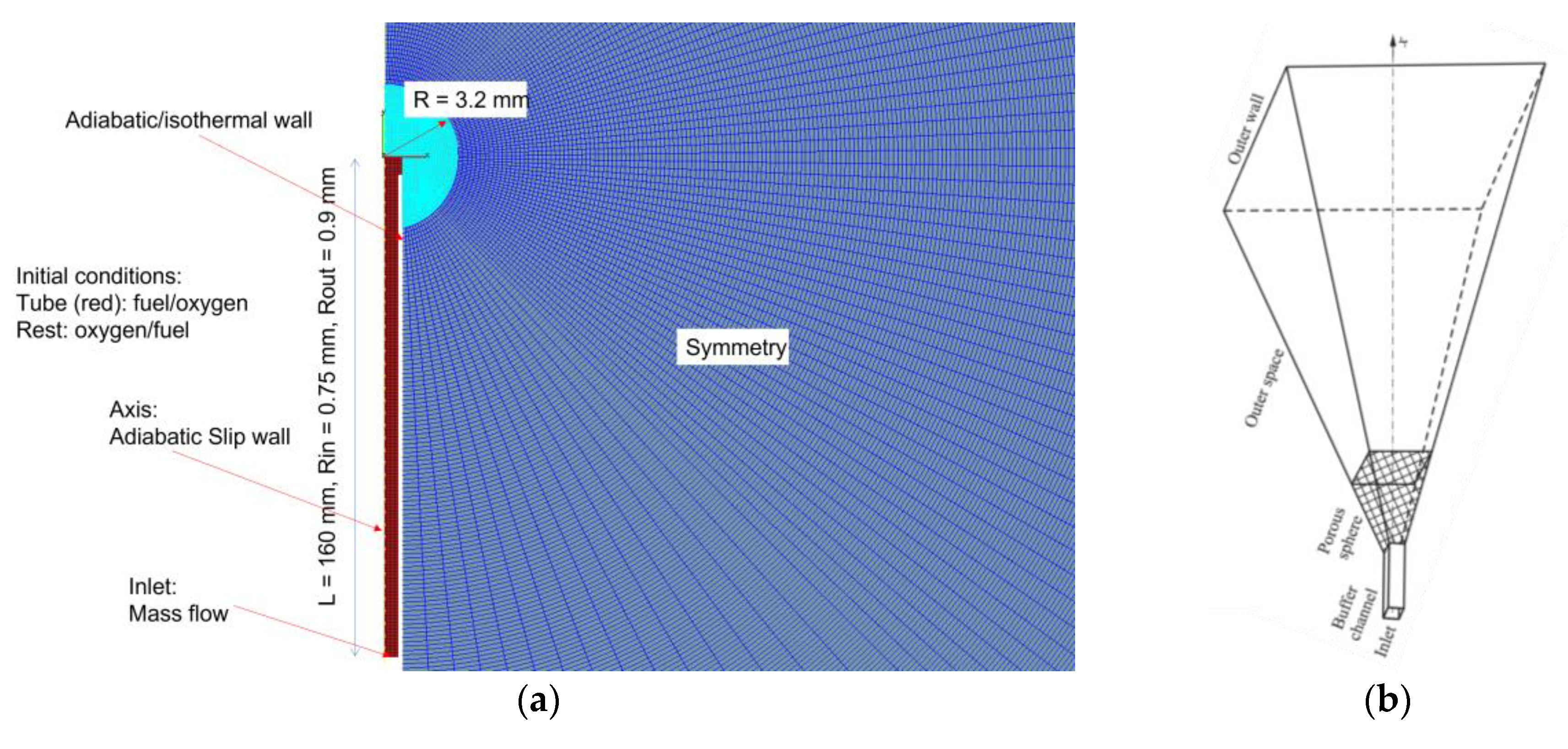
2.1. Statement of the Problem
- In microgravity conditions, the acceleration of gravity is negligible;
- The evolution of the SDF is symmetrical with respect to the axis of the supply tube;
- The porous medium in the flow region can be modeled by flow resistance according to the Darcy law and heat exchange with the fluid according to the Newton law, i.e., the porous medium can be represented by added momentum and heat sources, and respectively, in the governing equations. In addition, since the porous medium reduces the volume accessible for fluid, the local flow velocity, and superficial velocity inside the porous medium, are coupled by the undirected porosity value : ;
- The thermophysical and structural parameters of the PB material are constant;
- Thermal radiation of PB is negligible; PB absorbs thermal radiation of soot, H2O, CO2, and (for the sake of generality) N2 and O2;
- Gas-phase and catalytic reactions in the PB are absent;
- The gas flow is laminar;
- A multicomponent gas mixture obeys the thermal and caloric equations of state of an ideal gas and possesses variable thermophysical properties;
- The effect of thermodiffusion is negligible;
- Soot is an equivalent gas with the molecular mass of atomic carbon, when simulating soot reactions;
- Soot particles are the clusters of 20–25 carbon atoms, have the corresponding constant size, and do not coagulate;
- The radiation heat flux is caused solely by soot, H2O, CO2, N2, and O2 emittance;
- The outer wall of the computational domain is impermeable, noncatalytic, and isothermal.
2.2. Numerical Solution
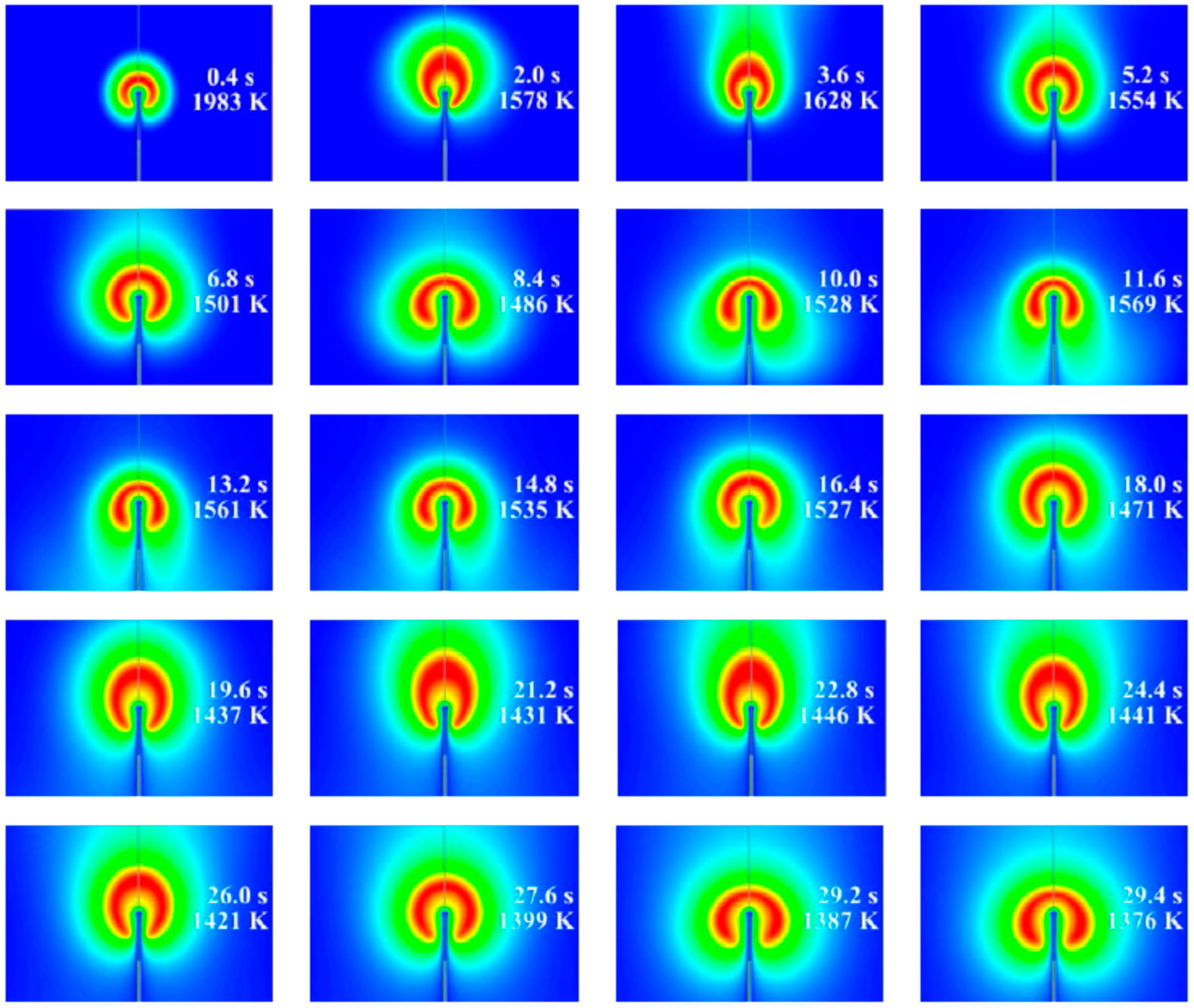
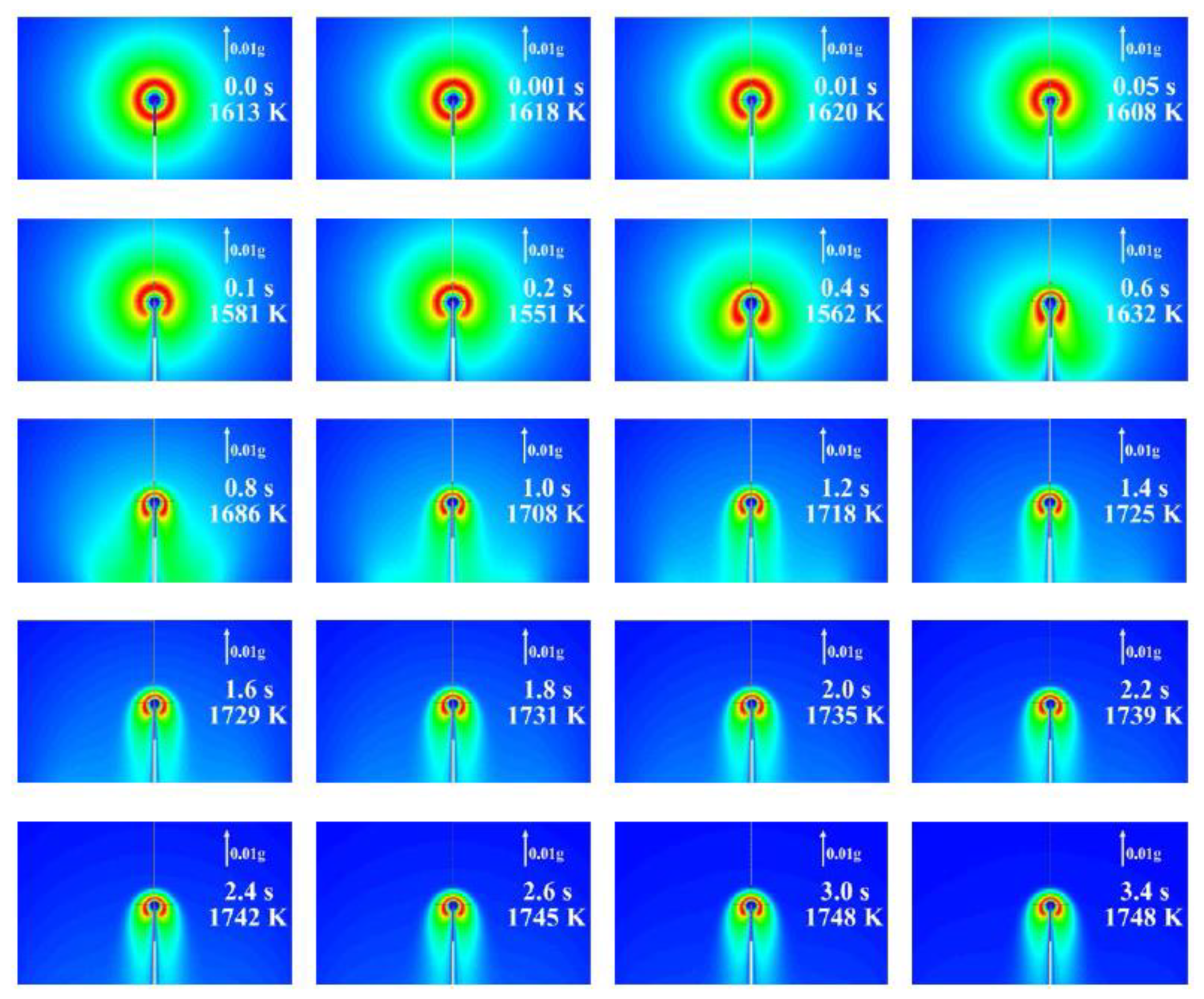
3. Results and Discussion
3.1. Experiments
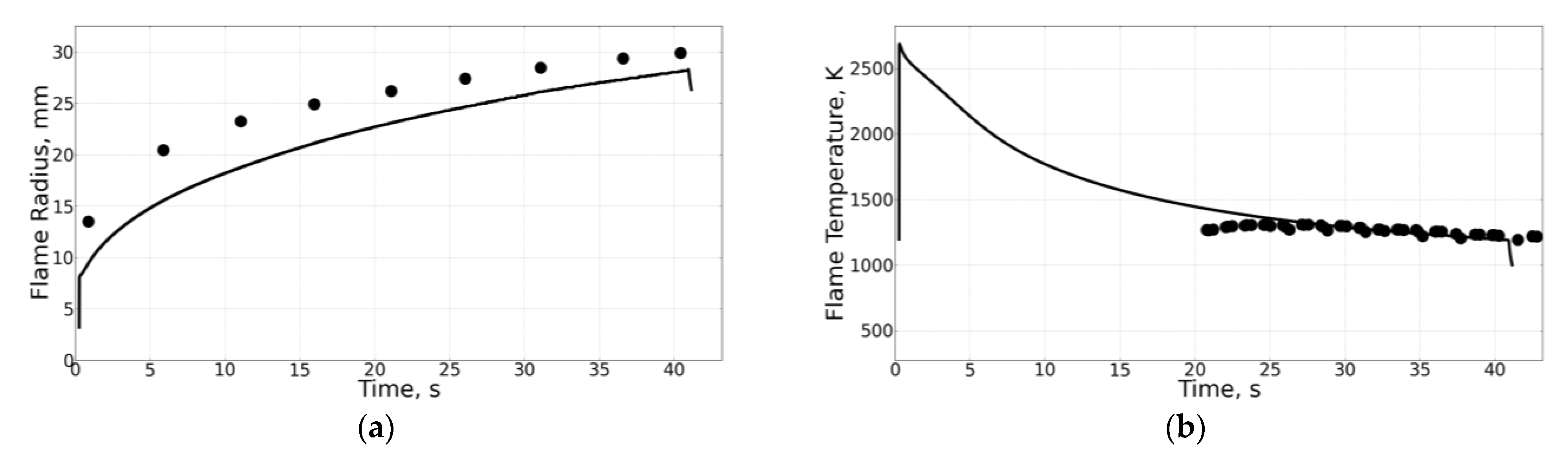
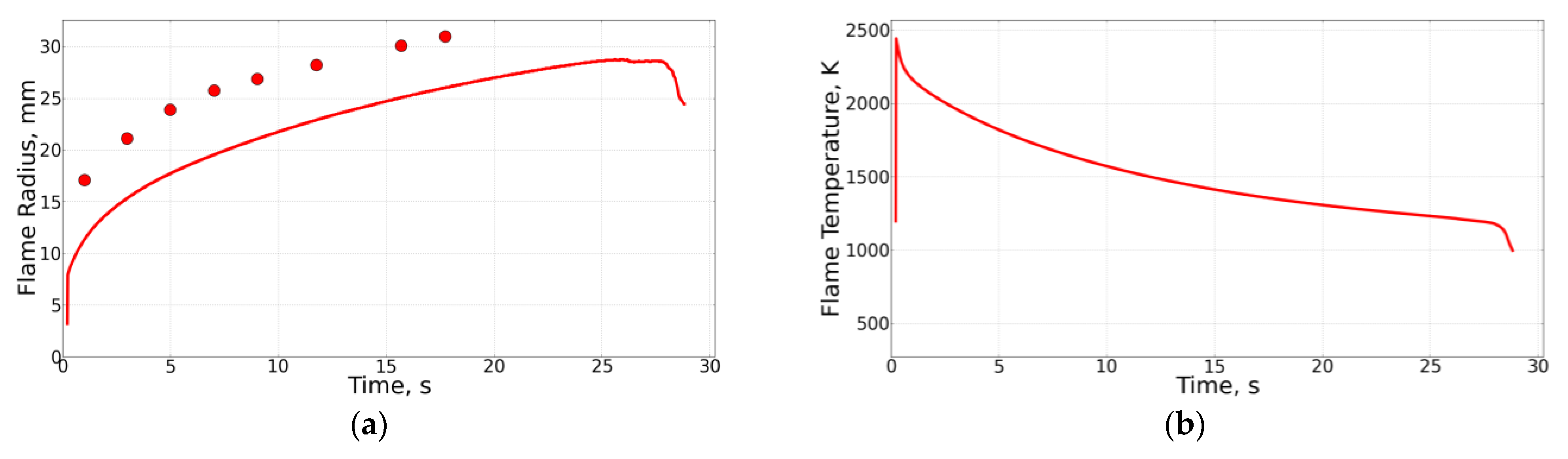
3.2. Calculations
3.2.1. 1D Calculations
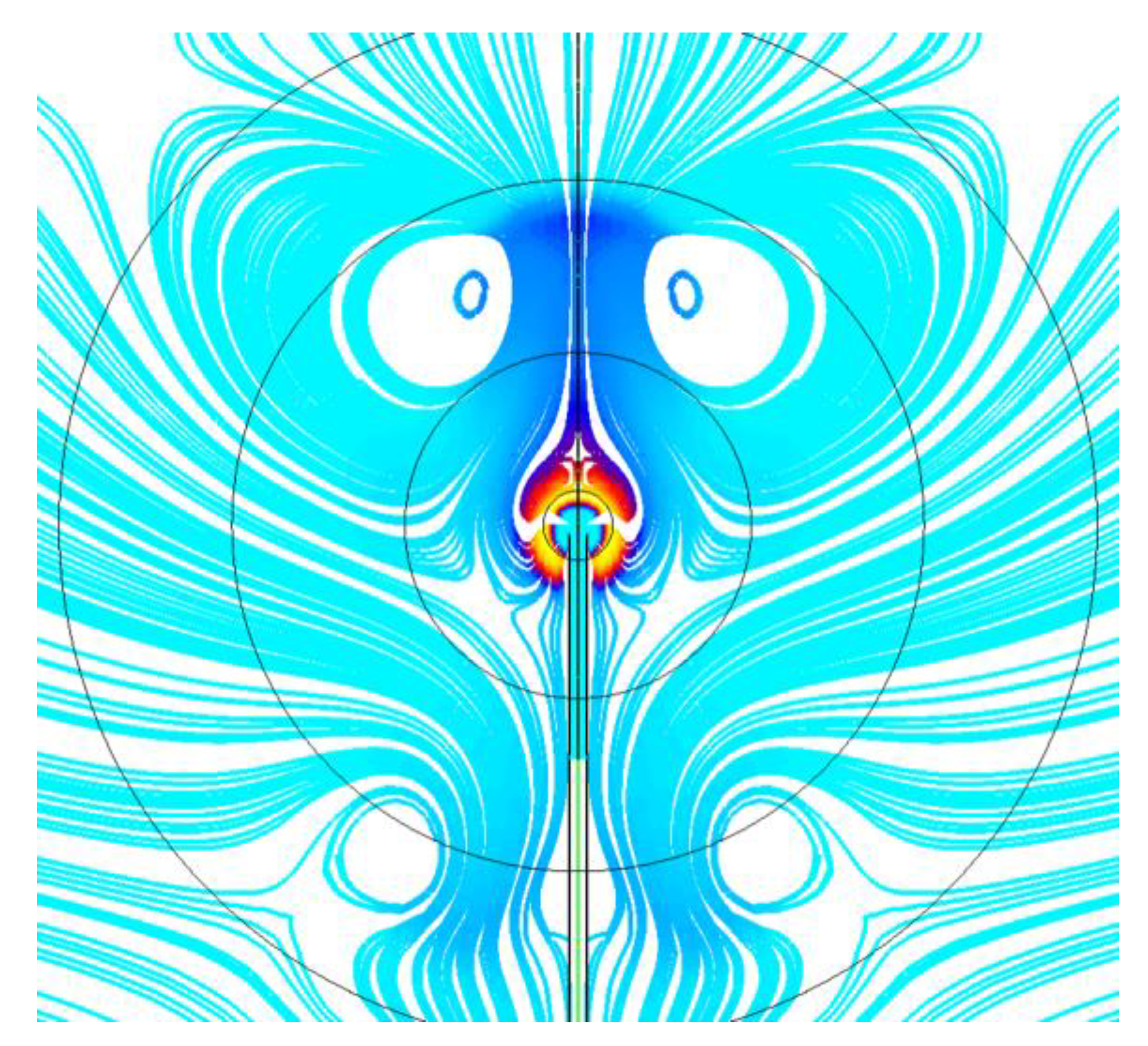
3.2.2. 2D Calculations
Cold Flow
Reactive Flow
4. Conclusions
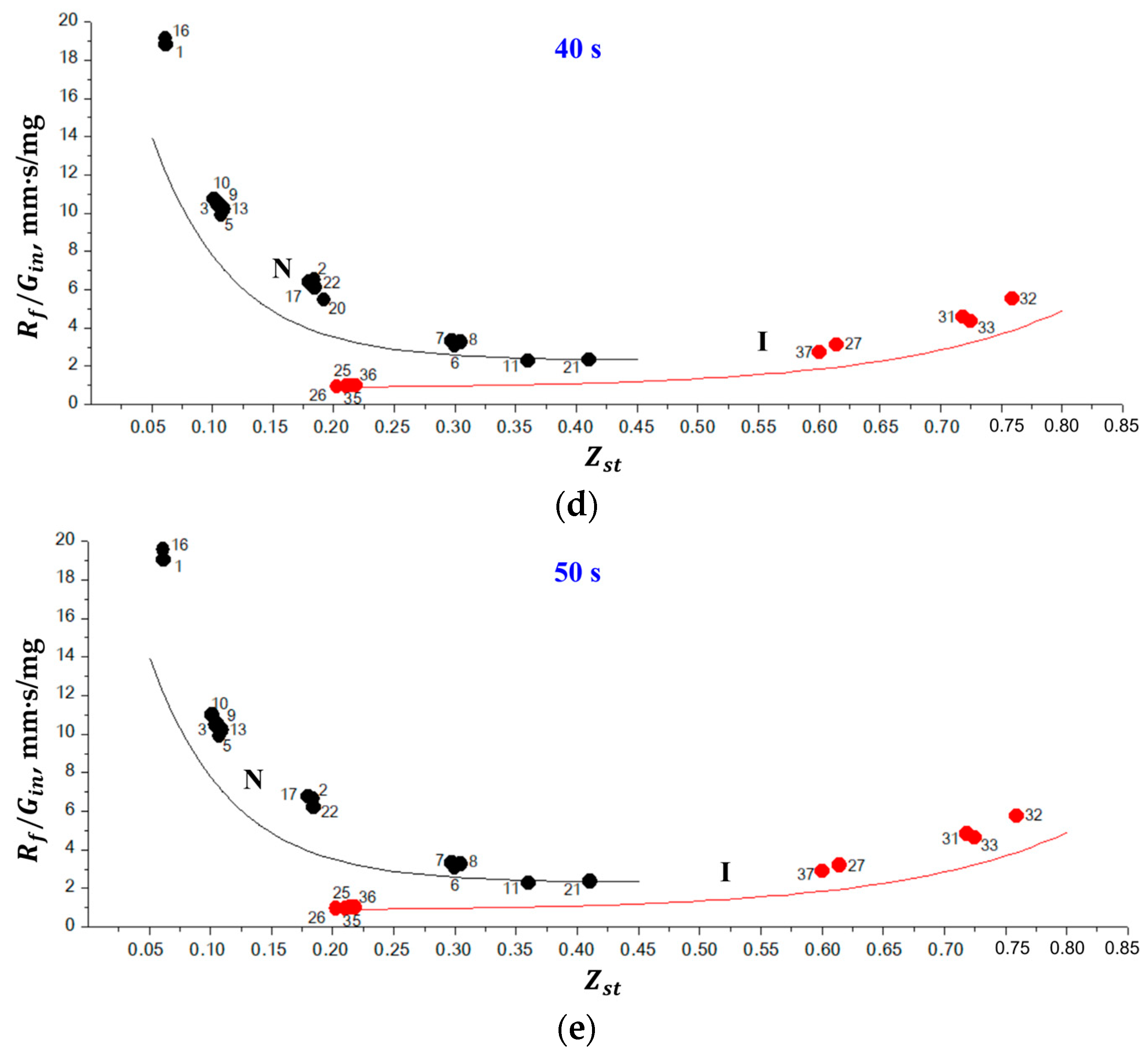
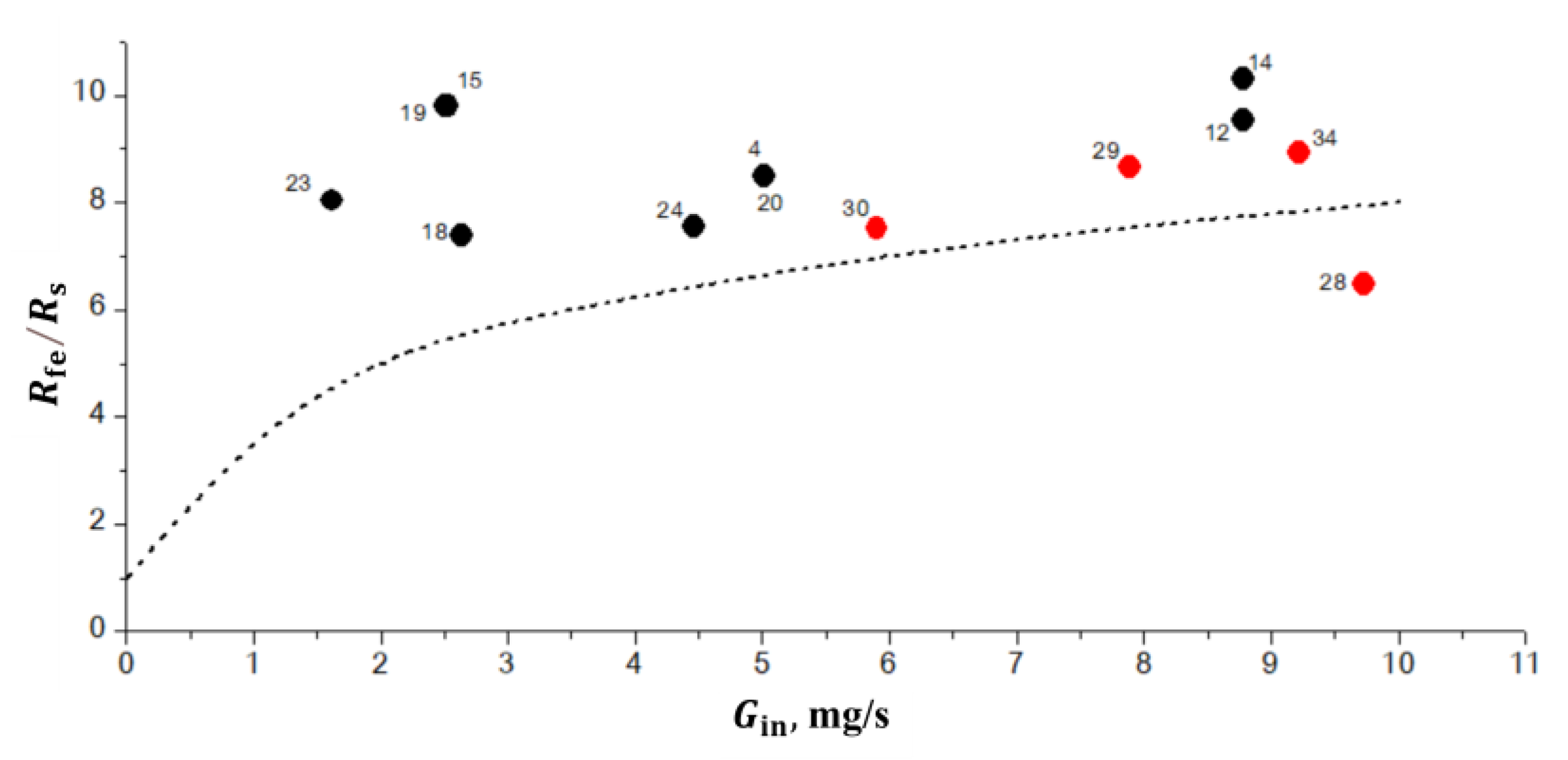
- (1)
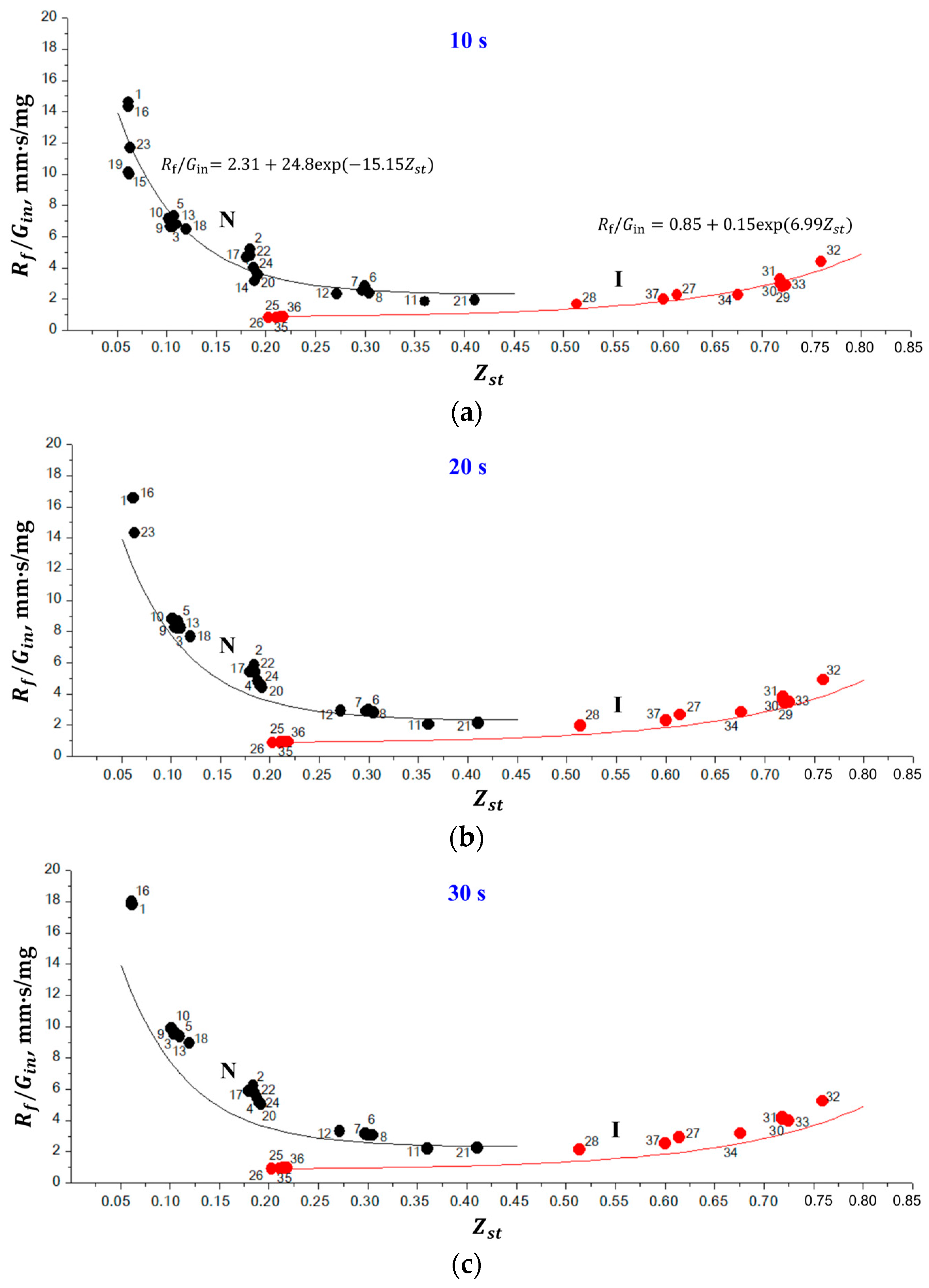
- There exist the unambiguous dependences of the ratio of flame radius to fluid mass flow rate through the PB, , on the stoichiometric mixture fraction for normal and inverse flames;
- (2)
- The growth rate of normal flames decreases with and they become more prone to be stationary. At 0.36–0.411, the normal flames become almost stationary, i.e., their growth rates become very low;
- (3)
- Contrary to normal flames, the growth rate of inverse flames increases with and they become less prone to be stationary. At 0.203–0.218, the inverse flames become almost stationary, i.e., their growth rates become very low;
- (4)
- The maximum growth rate of inverse flames is considerably less than that of normal flames. This means that the lifetime of inverse flames must be longer than that of the normal flames at the identical value of ;
- (5)
- At the same extinction radius, the extinction of the inverse flame occurs later than the extinction of the normal flame due to the lower growth rate;
- (6)
- The flame radius at extinction is approximately constant in the wide range of . A simple scaling law from the literature underestimates the flame radius at extinction;
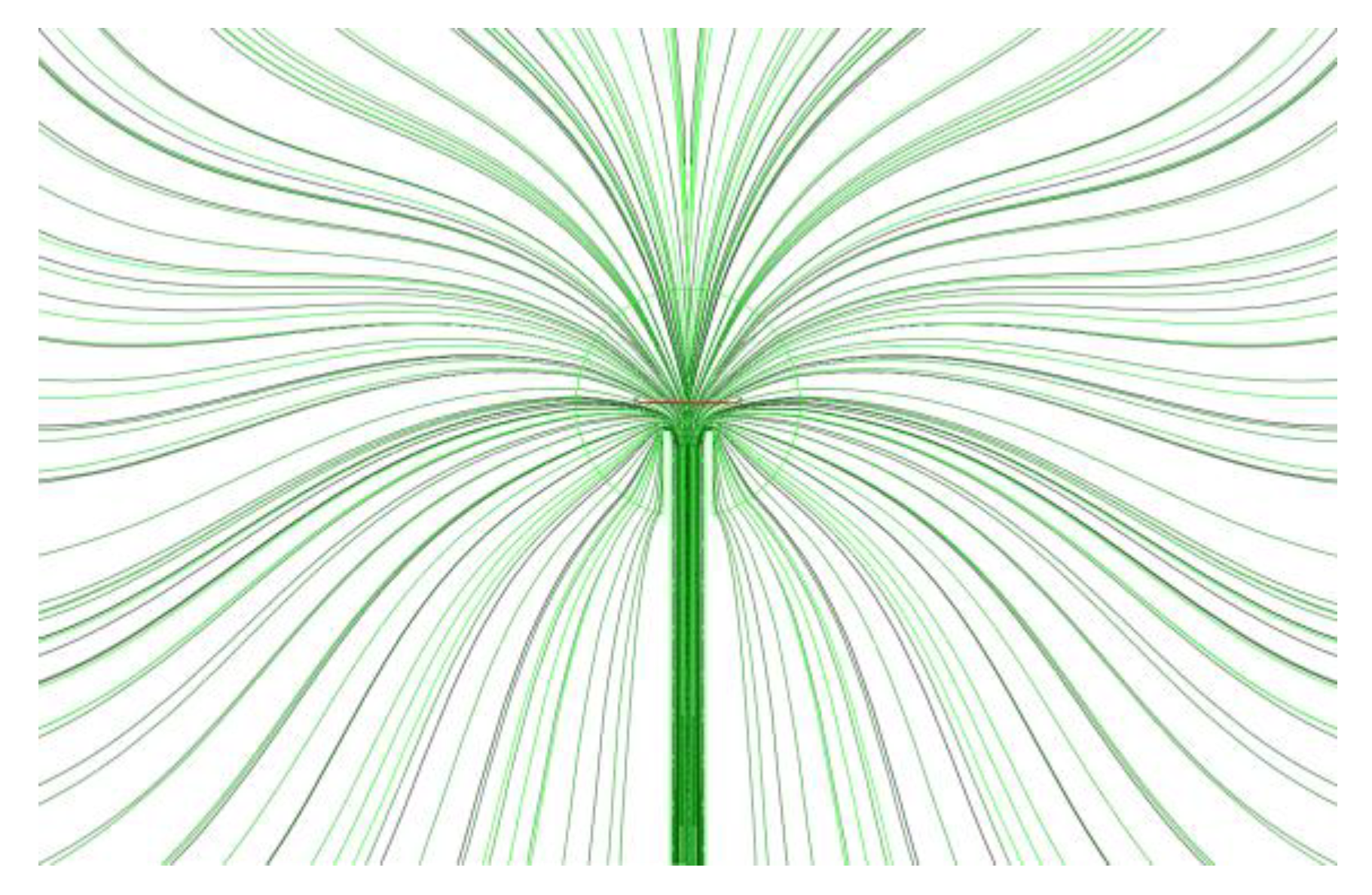
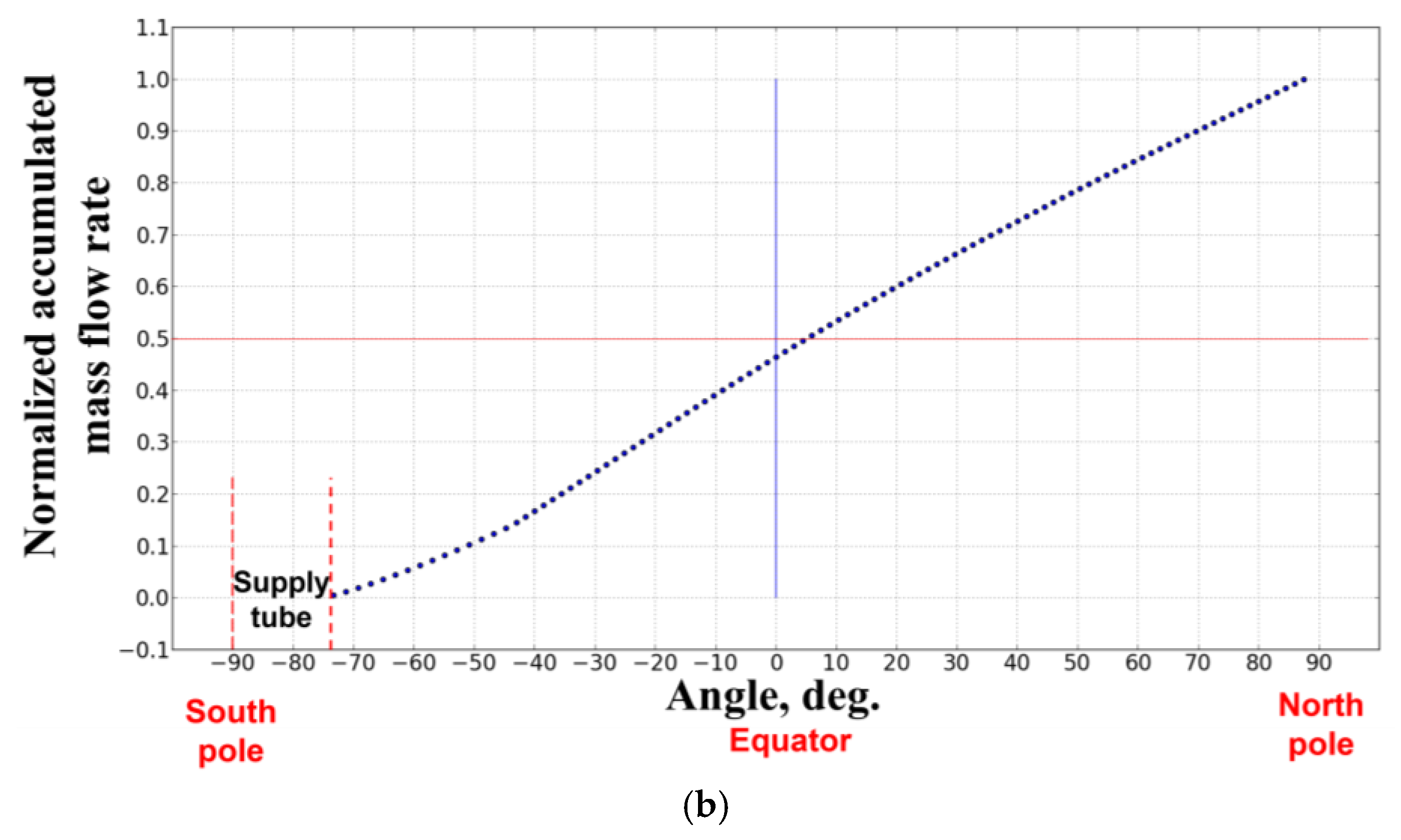
- (7)
- The 2D cold-flow calculations show that the supply tube blocks a part of the surface of the porous burner in the vicinity of the south pole. Due to this blockage, the normalized accumulated mass flow rate of fluid through the southern hemisphere is about 15% less than through the northern hemisphere. The local mass flow rate of fluid through the porous burner is nonuniform with the maximum flow rate attained in the angular interval [−20°, −40°] in the southern hemisphere;
- (8)
- The cold isothermal gas supply tube looks more corresponding to the experimental observations than the adiabatic supply tube, as it exhibits the characteristic asymmetry in both flame shape and temperature distribution (luminosity) observed in the experiments. It could be thus assumed that flame quenching near the gas supply tube observed in the experiments is caused by the cold gas supply tube;
- (9)
- The 2D calculations reveal the oscillatory evolution of ethylene diffusion flames with slow alterations in flame shape and temperature caused by the incepience of torroidal vortices in the surrounding gas;
- (10)
- The introduction of directional microgravity on the level of 0.01 allows complete suppression of flame oscillations.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Emissivity of the th emitting gas | |
| Pre-exponential factor, ( = 1, …, ) | |
| Specific heat at constant pressure, ( = 1, …, ) | |
| Solid skeleton heat capacity | |
| Threshold local C/O atomic ratio | |
| Characteristic size of the solid skeleton | |
| Conditional soot particle size | |
| Effective diffusion coefficient of the th species, ( = 1, …, ) | |
| Activation energy, ( = 1, …, ) | |
| added momentum source in a porous medium | |
| Inlet mass flow rate | |
| th component of the vector of acceleration of gravity ; | |
| Mean gas static enthalpy | |
| Standard enthalpy of formation of the th species, ( = 1, …, ) | |
| Mean gas total enthalpy | |
| Molecular mass flux of the th species, ( = 1, …, ) | |
| Turbulent mass flux of the th species, ( = 1, …, ), (j = 1, 2, 3) | |
| Total number of chemical reactions in the gas | |
| Temperature exponent, ( = 1, …, ) | |
| Number of gas species | |
| Mean gas pressure | |
| Initial pressure | |
| Molecular heat flux, (j = 1, 2, 3) | |
| Turbulent heat flux, (j = 1, 2, 3) | |
| Mean source of energy due to chemical transformations | |
| Length of the buffer channel | |
| Flame radius | |
| Radius of porous sphere | |
| Radius of the outer wall of the chamber | |
| Universal gas constant | |
| Passage area of gas supply tube | |
| Specific surface area of the porous burner | |
| Specific emitting surface area | |
| Time | |
| Time of ignition | |
| Temperature | |
| Initial temperature | |
| Standard temperature | |
| Threshold local temperature of soot formation | |
| Ignition temperature | |
| Temperature of porous sphere | |
| Superficial velocity inside the porous medium, (j = 1, 2, 3) | |
| The th component of the mean gas velocity vector, (j = 1, 2, 3) | |
| Chamber volume | |
| Mean source of mass due to chemical transformations, ( = 1, …, ) | |
| Molecular mass | |
| Molecular mass of oxidizer | |
| Molecular mass of fuel | |
| Cartesian coordinate, (j = 1, 2, 3) | |
| Volume fraction of the th emitting gas | |
| Initial species mass fractions, ( = 1, …, ) | |
| Inlet species mass fractions, ( = 1, …, ) | |
| Mean mass fraction of the th species, ( = 1, …, ) | |
| Soot mass fraction | |
| Stoichiometric mixture fraction | |
| Heat transfer coefficient between gas and porous sphere | |
| Delta function | |
| Coefficient of radiation absorption by the porous sphere material | |
| Permeability | |
| Thermal conductivity of the th species | |
| Solid skeleton thermal conductivity | |
| Dynamic viscosity of gas | |
| Stoichiometric coefficient of oxidizer in the overall reaction equation | |
| Stoichiometric coefficient of fuel in the overall reaction equation | |
| Stoichiometric coefficients of the th species in the reactants of the th reaction, ( = 1, …, ), ( = 1, …, ) | |
| Stoichiometric coefficients of the th species in the products of the th reaction, ( = 1, …, ), ( = 1, …, ) | |
| Mean gas density | |
| Solid skeleton density | |
| Soot density | |
| Stefan–Boltzmann constant | |
| Tensor of viscous stresses | |
| Tensor of turbulent stresses | |
| Porosity | |
| Added heat source in porous medium | |
| Heat source/sink other than that of chemical nature | |
| Heat source/sink for porous sphere | |
| Radiation absorption |
References
- Santa, K.J.; Chao, B.H.; Sunderland, P.B.; Urban, D.L.; Stocker, D.P.; Axelbaum, R.L. Radiative extinction of gaseous spherical diffusion flames in microgravity. Combust. Flame 2007, 151, 665–675. [Google Scholar] [CrossRef]
- Irace, P.H.; Lee, H.J.; Waddell, K.; Tan, L.; Stocker, D.P.; Sunderland, P.B.; Axelbaum, R.L. Observations of long duration microgravity spherical diffusion flames aboard the International Space Station. Combust. Flame 2021, 229, 111373. [Google Scholar] [CrossRef]
- Frolov, S.M.; Medvedev, S.N.; Frolov, F.S. Spherical diffusion flame of ethylene in the spaceflight experiment “Adamant”. Combust. Explos. 2021, 14, 9–21. [Google Scholar] [CrossRef]
- Available online: www.flickr.com/photos/space-flames (accessed on 26 June 2023).
- Available online: www.facebook.com/space.flames (accessed on 26 June 2023).
- T’ien, J.S. Diffusion flame extinction at small stretch rates: The mechanism of radiative loss. Combust. Flame 1986, 65, 31–34. [Google Scholar] [CrossRef]
- Chao, B.H.; Law, C.K.; T’ien, J.S. Structure and extinction of diffusion flames with flame radiation. Proc. Combust. Inst. 1991, 23, 523–531. [Google Scholar] [CrossRef]
- Atreya, A.; Agrawal, S.; Sacksteder, K.; Baum, H. Observations of methane and ethylene diffusion flames stabilized around a blowing porous sphere under microgravity conditions. In Proceedings of the AIAA 32nd Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 10–13 January 1994. Paper AIAA94-0572. [Google Scholar]
- Tse, S.; Zhu, D.; Sung, C.J.; Ju, Y.; Law, C.K. Microgravity burner-generated spherical diffusion flames: Experiment and computation. Combust. Flame 2001, 125, 1265–1278. [Google Scholar] [CrossRef]
- Christiansen, E.W.; Tse, S.D.; Law, C.K. A computational study of oscillatory extinction of spherical diffusion flames. Combust. Flame 2003, 134, 327–337. [Google Scholar] [CrossRef]
- Chernovsky, M.K.; Atreya, A.; Im, H.G. Effect of CO2 diluent on fuel versus oxidizer side of spherical diffusion flames in microgravity. Proc. Combust. Inst. 2007, 31, 1005–1013. [Google Scholar] [CrossRef]
- Sunderland, P.B.; Axelbaum, R.L.; Urban, D.L.; Chao, B.H.; Liu, S. Effects of structure and hydrodynamics on the sooting behavior of spherical microgravity diffusion flames. Combust. Flame 2003, 132, 25–33. [Google Scholar] [CrossRef]
- Tang, S.; Chernovsky, M.K.; Im, H.G.; Atreya, A. A computational study of spherical diffusion flames in microgravity with gas radiation Part I: Model development and validation. Combust. Flame 2010, 157, 118–126. [Google Scholar] [CrossRef]
- Tang, S.; Im, H.; Atreya, A. A computational study of spherical diffusion flames in microgravity with gas radiation. Part II: Parametric studies of the diluent effects on flame extinction. Combust. Flame 2010, 157, 127–136. [Google Scholar] [CrossRef]
- Rodenhurst, M.K.; Chao, B.-H.; Sunderland, P.B.; Axelbaum, R.L. Structure and extinction of spherical burner-stabilized diffusion flames that are attached to the burner surface. Combust. Flame 2018, 187, 22–29. [Google Scholar] [CrossRef]
- Nayagam, V.; Dietrich, D.L.; Williams, F.A. Radiative extinction of burner-supported spherical diffusion flames: A scaling analysis. Combust. Flame 2019, 205, 368–370. [Google Scholar] [CrossRef]
- Skeen, S.A.; Yablonsky, G.; Axelbaum, R.L. Characteristics of non-premixed oxygen-enhanced combustion: II. Flame structure effects on soot precursor kinetics resulting in soot-free flames. Combust. Flame 2010, 157, 1745–1752. [Google Scholar] [CrossRef]
- Kumfer, B.; Skeen, S.A.; Axelbaum, R.L. Soot inception limits in laminar diffusion flames with application to oxy-fuel combustion. Combust. Flame 2008, 154, 546–556. [Google Scholar] [CrossRef]
- Liu, S.; Chao, B.H.; Axelbaum, R.L. A theoretical study on soot inception in spherical burner-stabilized diffusion flames. Combust. Flame 2005, 140, 1–23. [Google Scholar] [CrossRef]
- Lecoustre, V.R.; Sunderland, P.B.; Chao, B.H.; Axelbaum, R.L. Numerical investigation of spherical diffusion flames at their sooting limits. Combust. Flame 2012, 159, 194–199. [Google Scholar] [CrossRef]
- Frolov, S.M.; Ivanov, V.S.; Frolov, F.S.; Vlasov, P.A.; Axelbaum, R.; Irace, P.H.; Yablonsky, G.; Waddell, K. Soot Formation in Spherical Diffusion Flames. Mathematics 2023, 11, 261. [Google Scholar] [CrossRef]
- Nayagam, V.; Haggard, J.B.; Colantonio, R.O.; Marchese, A.J.; Dryer, F.L.; Zhang, B.L.; Williams, F.A. Microgravity n-heptane droplet combustion in oxygen-helium mixtures at atmospheric pressure. AIAA J. 1998, 36, 1369–1378. [Google Scholar] [CrossRef]
- Liu, Y.C.; Xu, Y.; Avedisian, C.T.; Hicks, M.C. The effect of support fibers on micro-convection in droplet combustion experiments. Proc. Combust. Inst. 2015, 35, 1709–1716. [Google Scholar] [CrossRef]
- Nayagam, V.; Dietrich, D.L.; Hicks, M.C.; Williams, F.A. Radiative extinction of large n-alkane droplets in oxygen-inert mixtures in microgravity. Combust. Flame 2018, 194, 107–114. [Google Scholar] [CrossRef]
- Frolov, S.M.; Basevich, V.Y.; Medvedev, S.N.; Frolov, F.S. Low-temperature flameless combustion of a large drop of n-dodecane under microgravity conditions. Russ. J. Phys. Chem. B 2018, 12, 245–257. [Google Scholar] [CrossRef]
- Frolov, S.M.; Basevich, V.Y. Simulation of low-temperature oxidation and combustion of n-dodecane droplets under microgravity conditions. Fire 2023, 6, 70. [Google Scholar] [CrossRef]
- Williams, F.A. Combustion Theory; The Benjamin/Cummings Publishing Company, Inc.: Menlo Park, CA, USA, 1985; pp. 636–637. [Google Scholar]
- Basevich, V.Y.; Medvedev, S.N.; Frolov, S.M.; Frolov, F.S.; Basara, B.; Prieching, P. Macrokinetic model for calculating soot emission in Diesel engine. Comb. Explos. 2016, 9, 36–46. [Google Scholar]
- Basevich, V.Y.; Belyaev, A.A.; Posvyanskii, V.S.; Frolov, S.M. Mechanisms of the oxidation and combustion of normal paraffin hydrocarbons: Transition from C1–C10 to C11–C16. Rus. J. Phys. Chem. B 2013, 7, 161–169. [Google Scholar] [CrossRef]
- Agafonov, G.L.; Bilera, I.V.; Vlasov, P.A.; Kolbanovskii, Y.A.; Smirnov, V.N.; Teresa, A.M. Soot formation at pyrolysis and oxidation of acetylene and ethylene in shock tubes. Kinet. Catal. 2015, 56, 15–20. [Google Scholar] [CrossRef]
- Forchheimer, P. Wasserbewegung durch boden. Z. Ver. Dtsch. Ing. 1901, 45, 1781–1788. [Google Scholar]
- Available online: https://tnfworkshop.org/radiation/ (accessed on 26 June 2023).
- Reid, R.C.; Prausnitz, J.M.; Sherwood, T.K. The Properties of Gases and Liquids; McGrawHill: New York, NY, USA, 1977. [Google Scholar]






| No. | Flame | Combustion Chamber | Porous Burner | p, atm | , mm | , mm | , K | ||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| , mg/s | |||||||||||
| 1 | 19115B1 | 0.203 | 0.797 | 1.000 | 0.000 | 0.660 | 1.020 | 0.062 | - | - | - |
| 2 | 19206L6 | 0.203 | 0.797 | 0.291 | 0.709 | 1.980 | 1.010 | 0.184 | - | - | - |
| 3 | 19171D4 | 0.376 | 0.624 | 1.000 | 0.000 | 2.520 | 1.010 | 0.106 | 32 | 28.0 | 1200 |
| 4 | 19189K1 | 0.374 | 0.626 | 0.502 | 0.498 | 5.010 | 1.310 | 0.191 | 31 | 27.5 | 1200 |
| 5 | F10 | 0.380 | 0.620 | 1.000 | 0.000 | 1.224 | 1.239 | 0.107 | - | - | - |
| 6 | F02 | 0.391 | 0.609 | 0.288 | 0.712 | 1.800 | 1.190 | 0.300 | - | - | - |
| 7 | F08 | 0.386 | 0.614 | 0.288 | 0.712 | 3.603 | 1.263 | 0.297 | - | - | - |
| 8 | F05 | 0.400 | 0.600 | 0.288 | 0.712 | 4.514 | 1.250 | 0.304 | - | - | - |
| 9 | 19156C2 | 0.366 | 0.634 | 1.000 | 0.000 | 2.529 | 1.040 | 0.104 | 32 | 28.0 | 1200 |
| 10 | 19142J3 | 0.356 | 0.644 | 1.000 | 0.000 | 2.529 | 0.990 | 0.101 | 31 | 28.0 | 1200 |
| 11 | 19150N1 | 0.296 | 0.704 | 0.168 | 0.832 | 4.885 | 1.010 | 0.360 | - | - | - |
| 12 | 19150G3 | 0.338 | 0.662 | 0.288 | 0.712 | 8.779 | 1.050 | 0.272 | 35.5 | 30.5 | 1200 |
| 13 | 19175A3 | 0.391 | 0.609 | 1.000 | 0.000 | 1.960 | 1.270 | 0.110 | - | - | - |
| 14 | 19206A5 | 0.207 | 0.793 | 0.288 | 0.712 | 8.779 | 1.010 | 0.189 | 31 | 34 | 1200 |
| 15 | 19206G1 | 0.205 | 0.795 | 1.000 | 0.000 | 2.529 | 1.010 | 0.062 | 32 | 32 | 1200 |
| 16 | 19206G4 | 0.201 | 0.799 | 1.000 | 0.000 | 0.822 | 1.010 | 0.061 | - | - | - |
| 17 | 19206L4 | 0.195 | 0.805 | 0.288 | 0.712 | 2.835 | 1.010 | 0.180 | 19 | 19 | 1200 |
| 18 | 19123F1 | 0.206 | 0.794 | 0.490 | 0.510 | 2.640 | 1.010 | 0.120 | 23 | 24 | 1200 |
| 19 | 19123L1 | 0.202 | 0.798 | 1.000 | 0.000 | 2.510 | 1.010 | 0.061 | 33 | 32 | 1200 |
| 20 | 19189J3 | 0.378 | 0.622 | 0.502 | 0.498 | 5.010 | 1.300 | 0.192 | 31 | 28 | 1200 |
| 21 | 19200H3 | 0.285 | 0.715 | 0.131 | 0.869 | 4.430 | 1.020 | 0.411 | - | - | - |
| 22 | 19115F1 | 0.204 | 0.796 | 0.292 | 0.708 | 2.180 | 1.040 | 0.184 | - | - | - |
| 23 | 19123A2 | 0.209 | 0.791 | 1.000 | 0.000 | 1.620 | 1.000 | 0.063 | 25 | 26 | 1200 |
| 24 | 19123C1 | 0.207 | 0.793 | 0.290 | 0.710 | 4.460 | 1.000 | 0.188 | 24 | 25 | 1200 |
| No. | Flame | Combustion Chamber | Porous Sphere | , atm | , mm | , mm | , K | ||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| , mg/s | |||||||||||
| 25 | 21328D1 | 0.257 | 0.743 | 0.212 | 0.788 | 10.05 | 1.03 | 0.211 | - | - | - |
| 26 | 21349M3 | 0.270 | 0.730 | 0.212 | 0.788 | 9.11 | 1.00 | 0.203 | - | - | - |
| 27 | 22018H2 | 0.097 | 0.903 | 0.497 | 0.503 | 6.37 | 1.01 | 0.614 | 24 | 20 | 1200 |
| 28 | 22018J1 | 0.096 | 0.904 | 0.318 | 0.682 | 9.73 | 1.01 | 0.514 | 25 | 21 | 1200 |
| 29 | 22018G3 | 0.098 | 0.902 | 0.850 | 0.150 | 7.89 | 1.01 | 0.720 | 31 | 28 | 1200 |
| 30 | 22018G2 | 0.099 | 0.901 | 0.850 | 0.150 | 5.90 | 1.01 | 0.718 | 28 | 24 | 1200 |
| 31 | 22018G1 | 0.099 | 0.901 | 0.850 | 0.150 | 3.90 | 1 | 0.718 | - | - | - |
| 32 | 21328N5 | 0.080 | 0.920 | 0.850 | 0.150 | 2.27 | 0.96 | 0.759 | - | - | - |
| 33 | 22035J2 | 0.096 | 0.904 | 0.850 | 0.150 | 5.90 | 0.51 | 0.725 | - | - | - |
| 34 | 21340M1 | 0.121 | 0.879 | 0.850 | 0.150 | 9.22 | 1.01 | 0.676 | - | 29 | 1200 |
| 35 | 21349N3 | 0.251 | 0.749 | 0.212 | 0.788 | 9.10 | 0.52 | 0.215 | - | - | - |
| 36 | 21349N4 | 0.246 | 0.754 | 0.212 | 0.788 | 10.03 | 0.52 | 0.218 | - | - | - |
| 37 | 22018B1 | 0.168 | 0.832 | 0.850 | 0.150 | 4.7 | 1 | 0.601 | - | - | - |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Frolov, S.M.; Ivanov, V.S.; Frolov, F.S.; Semenov, I.V. Spherical Diffusion Flames of Ethylene in Microgravity: Multidimensional Effects. Fire 2023, 6, 285. https://doi.org/10.3390/fire6080285
Frolov SM, Ivanov VS, Frolov FS, Semenov IV. Spherical Diffusion Flames of Ethylene in Microgravity: Multidimensional Effects. Fire. 2023; 6(8):285. https://doi.org/10.3390/fire6080285
Chicago/Turabian StyleFrolov, Sergey M., Vladislav S. Ivanov, Fedor S. Frolov, and Ilya V. Semenov. 2023. "Spherical Diffusion Flames of Ethylene in Microgravity: Multidimensional Effects" Fire 6, no. 8: 285. https://doi.org/10.3390/fire6080285
APA StyleFrolov, S. M., Ivanov, V. S., Frolov, F. S., & Semenov, I. V. (2023). Spherical Diffusion Flames of Ethylene in Microgravity: Multidimensional Effects. Fire, 6(8), 285. https://doi.org/10.3390/fire6080285









