Extracting Physical Information from the Voigt Profile Using the Lambert W Function
Abstract
1. Introduction
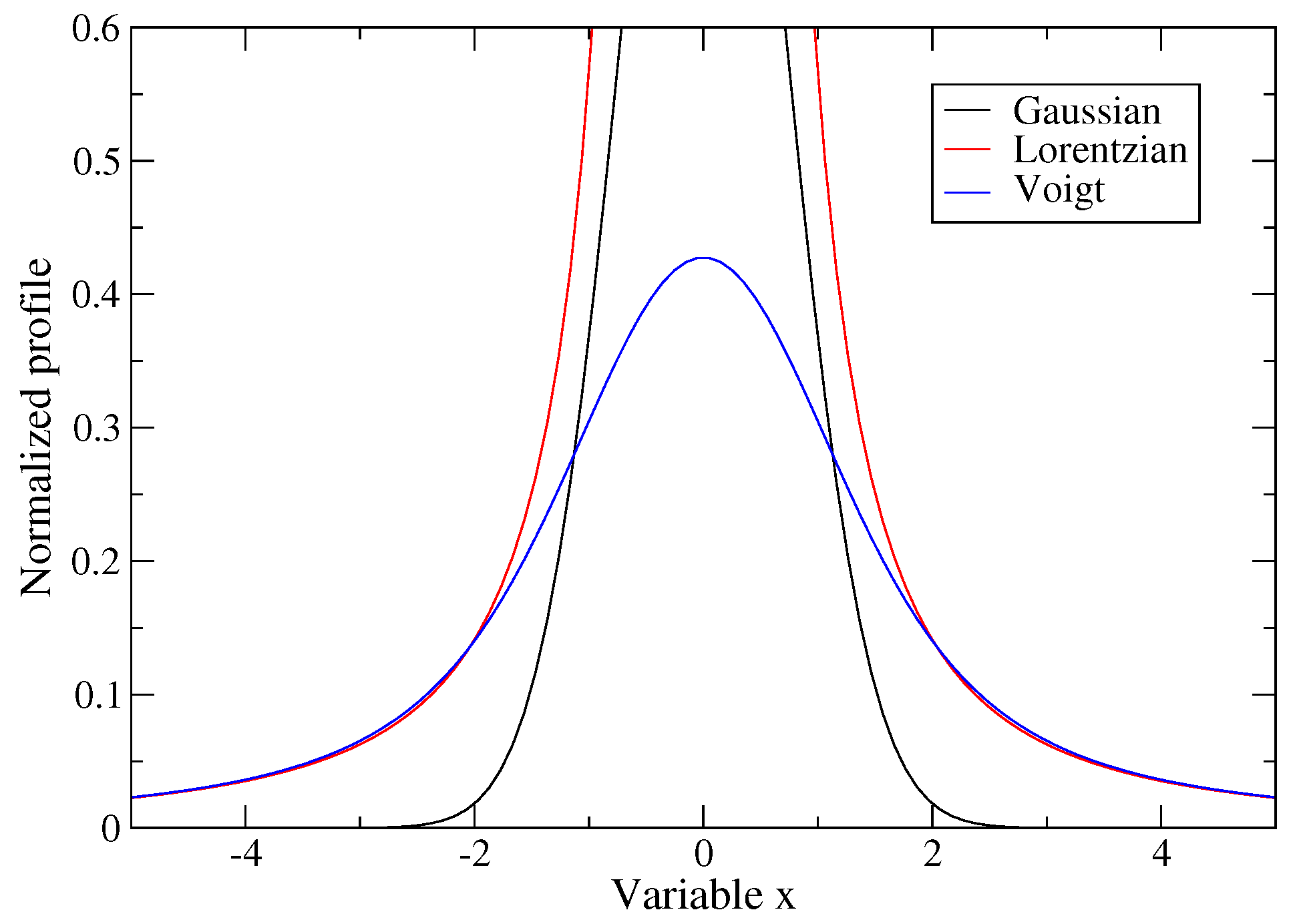
2. Representations of the Voigt Function: A Non-Exhaustive Mini-Review
3. The Pseudo-Voigt Function
3.1. Main Principle
3.2. Matveev’s Analytical Approximation of the Voigt Profile and the “Equivalent” Width of the Voigt Profile
3.3. Equivalent Width According to He and Zhang
4. Competition between Lorentzian and Gaussian Wings
4.1. Mathematical Formalism
4.2. Application in the Case of the Pseudo-Voigt Function of Matveev
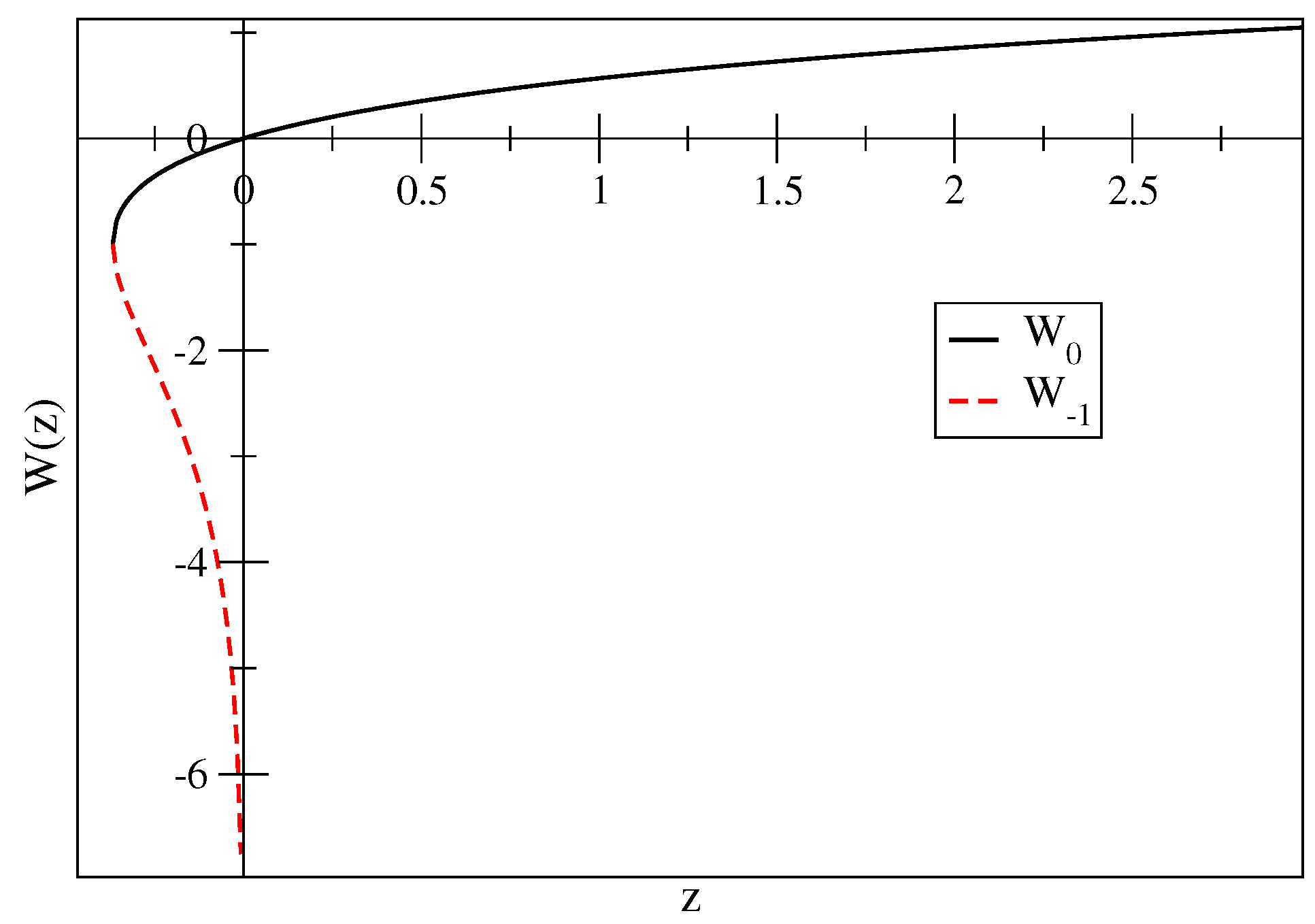
4.3. Calculation of the Lambert W Function
5. Remarks on the Far Wings of the Voigt Profile
5.1. The Cut-Off of Iglesias et al.
5.2. Continued-Fraction Representation
5.3. The Pearson Distribution: An Interesting Alternative?
6. Conclusions and Future Plans
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| DFT | Density Functional Theory; |
| FWHM | Full Width at Half Maximum; |
| HWHM | Half Width at Half Maximum. |
Appendix A. Proof of Faddeyeva–Terent’ev Relation
Appendix B. Additional Useful Relations
Appendix C. The Tepper-García Function
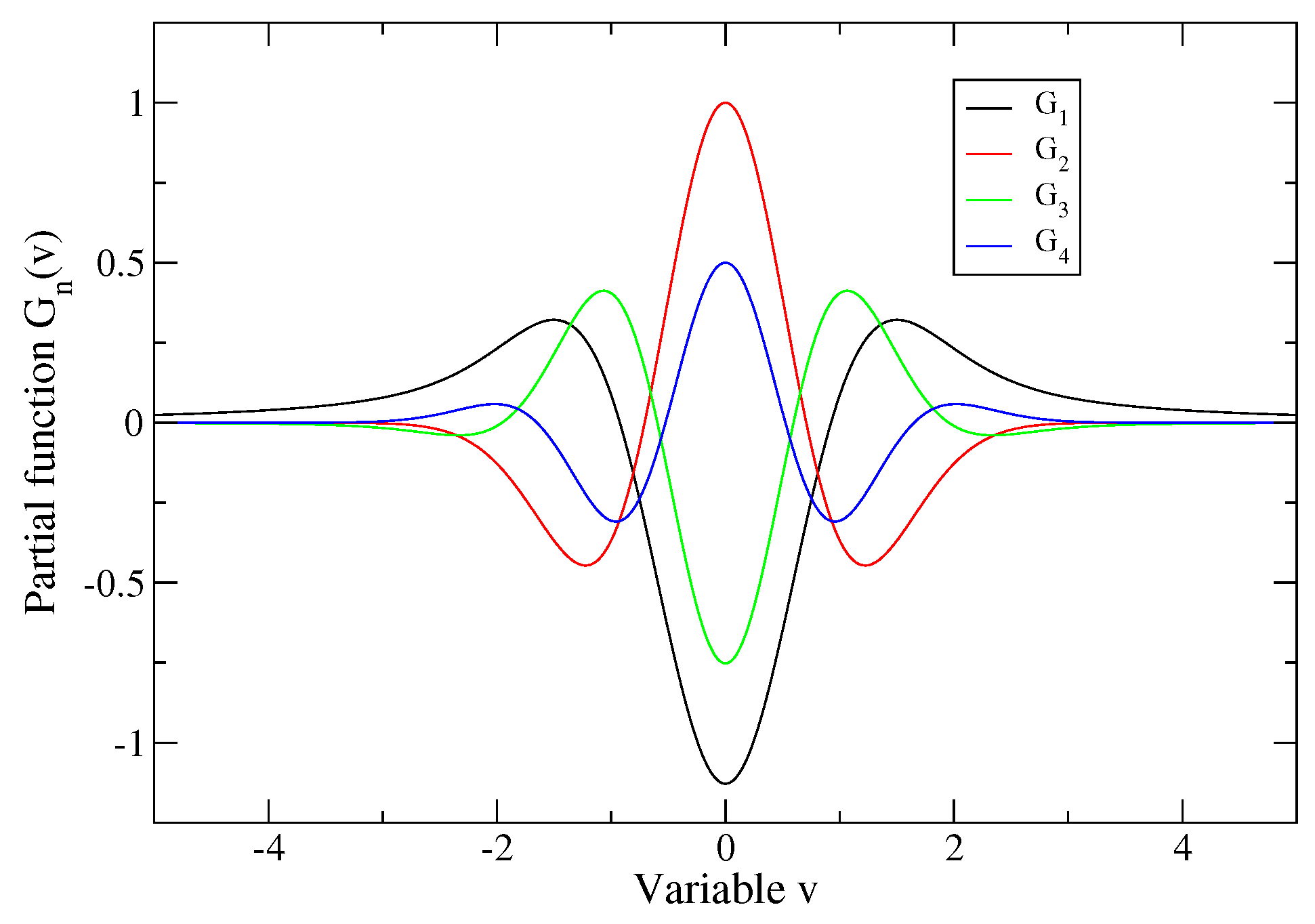
Appendix D. Expansion of the Voigt Function
References
- Griem, H.R. Spectral Line Broadening by Plasmas; Academic Press: New York, NY, USA, 1974. [Google Scholar]
- Calisti, A.; Khelfaoui, F.; Stamm, R.; Talin, B.; Lee, R.W. Model for the line shapes of complex ions in hot and dense plasmas. Phys. Rev. A 1990, 42, 5433–5440. [Google Scholar] [CrossRef] [PubMed]
- Talin, B.; Calisti, A.; Godbert, L.; Stamm, R.; Lee, R.W.; Klein, L. Frequency-fluctuation model for line-shape calculations in plasma spectroscopy. Phys. Rev. A 1995, 5, 1918–1928. [Google Scholar] [CrossRef]
- Stambulchik, E.; Maron, Y. Plasma line broadening and computer simulations: A mini-review. High Energy Density Phys. 2010, 6, 9–14. [Google Scholar] [CrossRef]
- Voigt, W. Über das Gesetz der Intensitätsverteilung innerhalb der Linien eines Gasspektrums. Munch. Sitzber Ak. Wiss. Math.-Phys. Kl. 1912, 42, 603–620. [Google Scholar]
- Faddeyeva, V.N.; Terent’ev, N.M. Tables of Values of the Function for Complex Argument; Pergamon Press: New York, NY, USA, 1961. [Google Scholar]
- Faddeyeva, V.N.; Terent’ev, N.M. Tables of Values of Error Function for a Complex Argument; Gostekhizdat: Moscow, Russia, 1954. (In Russian) [Google Scholar]
- Zaghloul, M.R.; Ali, A.N. Algorithm 916: Computing the Faddeyeva and Voigt functions. ACM Trans. Math. Softw. 2011, 38, 1–22. [Google Scholar] [CrossRef]
- Abramowitz, M.; Stegun, A. Handbook of Mathematical Functions; Dover: New York, NY, USA, 1970; p. 298. [Google Scholar]
- Armstrong, B.H. Spectrum line profiles: The Voigt function. J. Quant. Spectrosc. Radiat. Transf. 1967, 7, 61–88. [Google Scholar] [CrossRef]
- Avrett, E.H.; Loeser, R. Formation of Line and Continuous Spectra; Smithsonian Astrophysical Observatory: Cambridge, MA, USA, 1969; Volume 303. [Google Scholar]
- Matveev, V.S. Approximate representation of absorption coefficient and equivalent widths of lines with Voigt profile. J. Appl. Spectrosc. 1972, 16, 168–172. [Google Scholar] [CrossRef]
- Hui, A.K.; Armstrong, B.H.; Wray, A.A. Rapid computation of the Voigt and complex error functions. J. Quant. Spectrosc. Radiat. Transf. 1978, 19, 509–516. [Google Scholar] [CrossRef]
- Matveev, V.S. Approximate representations of the refractive index of a medium in the region of a Voigt-profile absorption line. J. Appl. Spectrosc. 1981, 35, 1043–1046. [Google Scholar] [CrossRef]
- Humlí<i>c</i>ˇek, J. Optimized computation of the voigt and complex probability functions. J. Quant. Spectrosc. Radiat. Transf. 1982, 27, 437–444. [Google Scholar]
- Nikiforov, A.F.; Novikov, V.G.; Uvarov, V.B. Special Functions of Mathematical Physics. A Unified Introduction with Applications; Translated from the Russian; Birkhäuser Verlag: Basel, Switzerland, 1988. [Google Scholar]
- Rybicki, G.B. Dawson’s integral and the sampling theorem. Comput. Phys. 1989, 3, 85–87. [Google Scholar] [CrossRef]
- Shippony, Z.; Read, W.G. A highly accurate Voigt function algorithm. J. Quant. Spectrosc. Radiat. Transf. 1993, 50, 635–646. [Google Scholar] [CrossRef]
- Abousahl, S.; Gourma, M.; Bickel, M. Fast Fourier transform for Voigt profile: Comparison with some other algorithms. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 1997, 395, 231–236. [Google Scholar] [CrossRef]
- Wells, R.J. Rapid approximation to the Voigt/Faddeeva function and its derivatives. J. Quant. Spectrosc. Radiat. Transf. 1999, 62, 294–298. [Google Scholar] [CrossRef]
- Asfaw, A. A fast method of modeling spectral lines. J. Quant. Spectrosc. Radiat. Transf. 2001, 70, 129–137. [Google Scholar] [CrossRef]
- Leiweke, R.J. Comment on “A new procedure for obtaining the Voigt function dependent upon the complex error function”. J. Quant. Spectrosc. Radiat. Transf. 2007, 103, 597–600. [Google Scholar] [CrossRef]
- Letchworth, K.L.; Benner, D.C. Rapid and accurate calculation of the Voigt function. J. Quant. Spectrosc. Radiat. Transf. 2007, 107, 173–192. [Google Scholar] [CrossRef]
- Abrarov, S.M.; Quine, B.M.; Jagpal, R.K. A simple interpolating algorithm for the rapid and accurate calculation of the Voigt function. J. Quant. Spectrosc. Radiat. Transf. 2009, 110, 376–383. [Google Scholar] [CrossRef]
- Pagnini, G.; Saxena, R.K. On Mellin–Barnes integral representation of Voigt profile function. Forum Berl. Math. Ges. 2012, 23, 47–64. [Google Scholar]
- Chib, S.; Belafhal, A. Simple analytical expression of the Voigt profile. Quantum Rep. 2022, 4, 36–46. [Google Scholar] [CrossRef]
- Keshavamurthy, R.S. Voigt lineshape functions as a series of confluent hypergeometric functions. J. Phys. A Math. Gen. 1987, 20, L273–L278. [Google Scholar] [CrossRef]
- Lebedev, N.N. Special Functions and Their Applications; Dover: New York, NY, USA, 1972; p. 258. [Google Scholar]
- Schreier, F. The Voigt and complex error function: A comparison of computational methods. J. Quant. Spectrosc. Radiat. Transf. 1992, 48, 743–762. [Google Scholar] [CrossRef]
- Fettis, H.E. Remarks on a note by H Exton. J. Phys. A Math. Gen. 1983, 16, 663–664. [Google Scholar] [CrossRef]
- Katriel, J. A comment on the reducibility of the Voigt functions. J. Phys. A Math. Gen. 1982, 15, 709–710. [Google Scholar] [CrossRef]
- Exton, H. On the reducibility of the Voigt functions. J. Phys. A Math. Gen. 1981, 14, L75–L77. [Google Scholar] [CrossRef]
- Yang, S. A unification of the Voigt functions. Int. J. Math. Educ. Sci. Technol. 1994, 25, 845–851. [Google Scholar] [CrossRef]
- Di Rocco, H.O.; Iriarte, D.I.; Pomarico, J. General expression for the Voigt function that is of so special interest for applied spectroscopy. Appl. Spectrosc. 2001, 55, 822–826. [Google Scholar] [CrossRef]
- Zaghloul, M.R. On the calculation of the Voigt line profile: A single proper integral with a damped sine integrand. Mon. Not. R. Astron. Soc. 2007, 375, 1043–1048. [Google Scholar] [CrossRef]
- Limandri, S.P.; Bonetto, R.D.; Di Rocco, H.O.; Trincavelli, J.C. Fast and accurate expression for the Voigt function. Application to the determination of uranium M linewidths. Spectrochim. Acta Part B At. Spectrosc. 2008, 63, 962–967. [Google Scholar] [CrossRef]
- Zaghloul, M.R. On the falsity of a claimed exact analytic formula for the calculation of Voigt spectral line profile. Spectrochim. Acta Part B At. Spectrosc. 2007, 63, 820–821. [Google Scholar] [CrossRef]
- Mathai, A.M.; Saxena, R.K. The H-Function with Applications in Statistics and Other Disciplines; Wiley Eastern Limited: New Delhi, India; Bangalore, India; Bombay, India, 1978. [Google Scholar]
- Kilbas, A.A.; Saigo, M. H-Transforms. Theory and Applications; CRC Press LLC: Boca Raton, FL, USA, 2004. [Google Scholar]
- Srivastava, H.M.; Gupta, K.C.; Goyal, S.P. The H-Functions of One and Two Variables with Applications; South Asian Publisher: New Delhi, India, 1982. [Google Scholar]
- Tepper-García, T. Voigt profile fitting to quasar absorption lines: An analytic approximation to the Voigt-Hjerting function. Mon. Not. R. Astron. Soc. 2006, 369, 2025–2035. [Google Scholar] [CrossRef]
- Stancik, A.L.; Brauns, E.B. A simple asymmetric lineshape for fitting infrared absorption spectra. Vib. Spectrosc. 2008, 47, 66–69. [Google Scholar] [CrossRef]
- Hadj Larbi, F.; Khereddine, A.; Alili, B.; Bradai, D. Généralisation des expressions analytiques liées à la fonction pseudo-Voigt utilisée en analyse des profils de raies de diffraction. Fizika A 2009, 18, 63–72. [Google Scholar]
- Wertheim, G.K.; Butler, M.A.; West, K.W.; Buchanan, D.N. Determination of the Gaussian and Lorentzian content of experimental line shapes. Rev. Sci. Instrum. 1974, 45, 1369–1371. [Google Scholar] [CrossRef]
- Sánchez-Bajo, F.; Cumbrera, F.L. The use of the pseudo-Voigt function in the variance method of X-ray line-broadening analysis. J. Appl. Crystallogr. 1997, 30, 427–430. [Google Scholar] [CrossRef]
- Liu, Y.; Lin, J.; Huang, G.; Guo, Y.; Duan, C. Simple empirical analytical approximation to the Voigt profile. JOSA B 2001, 18, 666–672. [Google Scholar] [CrossRef]
- Di Rocco, H.O.; Cruzado, A. The Voigt profile as a sum of a Gaussian and a Lorentzian functions, when the weight coefficient depends only on the widths ratio. Acta Phys. Pol. A 2012, 122, 666–669. [Google Scholar] [CrossRef]
- Ida, T.; Ando, M.; Toraya, H. Extended pseudo-Voigt function for approximating the Voigt profile. J. Appl. Crystallogr. 2000, 33, 1311–1316. [Google Scholar] [CrossRef]
- Thompson, P.; Cox, D.E.; Hastings, J.B. Rietveld refinement of Debye-Scherrer synchrotron X-ray data from Al2O3. J. Appl. Crystallogr. 1987, 20, 79–83. [Google Scholar] [CrossRef]
- Whiting, E.E. An empirical approximation to the Voigt profile. J. Quant. Spectrosc. Radiat. Transf. 1968, 8, 1379–1384. [Google Scholar] [CrossRef]
- Olivero, J.J.; Longbothum, R.L. Empirical fits to the Voigt line width: A brief review. J. Quant. Spectrosc. Radiat. Transf. 1977, 17, 233–236. [Google Scholar] [CrossRef]
- Kielkopf, J.F. New approximation to the Voigt function with applications to spectral-line profile analysis. JOSA 1973, 63, 987–995. [Google Scholar] [CrossRef]
- Dobrichev, V. A simple one per cent approximation of the Voigt function. CR Acad. Bulg. Sci. 1984, 37, 991–993. [Google Scholar]
- He, J.; Zhang, Q. An exact calculation of the Voigt spectral line profile in spectroscopy. J. Opt. A Pure Appl. Opt. 2007, 9, 565–568. [Google Scholar] [CrossRef]
- He, J.; Zhang, Q. Discussion on the full width at half maximum (FWHM) of the Voigt spectral line. Optik 2013, 124, 5245–5247. [Google Scholar] [CrossRef]
- He, J.; Zhang, C. The accurate calculation of the Fourier transform of the pure Voigt function. J. Opt. A Pure Appl. Opt. 2005, 7, 613–616. [Google Scholar] [CrossRef]
- Wang, Y.; Zhou, B.; Zhao, R.; Wang, B.; Liu, Q.; Dai, M. Super-accuracy calculation for the half width of a Voigt profile. Mathematics 2022, 10, 210. [Google Scholar] [CrossRef]
- Karp, A.H. Efficient computation of spectral line shapes. J. Quant. Spectrosc. Radiat. Transf. 1978, 20, 379–384. [Google Scholar] [CrossRef]
- Reiche, F. Über die Emission, Absorption und Intensitätsverteilung von Spektrallinien. Ber. Deutsch. Phys. Ges. 1913, 15, 3–21. [Google Scholar]
- Mitchell, A.C.G.; Zemansky, N.W. Resonance Radiation and Excited Atoms; Cambridge University Press: Cambridge, UK, 1934; p. 319. [Google Scholar]
- Lambert, J.H. Observationes Variae in Mathesin Puram. Acta Helv. Phys.-Math.-Anat.-Bot.-Med. 1758, 3, 128–168. [Google Scholar]
- Lambert, J.H. Nouveaux Mémoires de l’Académie Royale des Sciences et Belles-Lettres de Berlin; German Academy of Sciences Berlin: Berlin, Germany, 1772. [Google Scholar]
- Corless, R.M.; Gonnet, G.H.; Hare, D.E.G.; Jeffrey, D.J.; Knuth, D.E. On the Lambert W function. Adv. Comput. Math. 1996, 5, 329–359. [Google Scholar] [CrossRef]
- Dubinova, I.D. Application of the Lambert W function in mathematical problems of plasma physics. Plasma Phys. Rep. 2004, 30, 937–943. [Google Scholar] [CrossRef]
- Valluri, S.R.; Gil, M.; Jeffrey, D.J.; Basu, S. The Lambert W function in quantum statistics. J. Math. Phys. 2009, 50, 102103. [Google Scholar] [CrossRef]
- Wilson, B.G.; Sonnad, V. A note on generalized radial mesh generation for plasma electronic structure. High Energy Density Phys. 2011, 7, 161–162. [Google Scholar] [CrossRef]
- Pain, J.-C. Comment on “A note on generalized radial mesh generation for plasma electronic structure”. High Energy Density Phys. 2011, 7, 224. [Google Scholar] [CrossRef]
- Bowen, C.; Pain, J.-C. Schrödinger equation on a generic radial grid. High Energy Density Phys. 2023, 47, 101042. [Google Scholar] [CrossRef]
- Visser, M. Primes and the Lambert W function. Mathematics 2018, 6, 56. [Google Scholar] [CrossRef]
- Euler, L. De serie Lambertina Plurimisque eius insignibus proprietatibus. Acta Acad. Sci. Imp. Petropolitanae 1783, 2, 29–51. [Google Scholar]
- Comtet, L. Inversion de yαey et ylogαy au moyen des nombres de Stirling. CR Acad. Sci. Paris 1970, 270, 1085–1088. [Google Scholar]
- Jeffrey, D. J; Corless, R.M.; Hare, D.E.G.; Knuth, D.E. Sur l’inversion de yαey au moyen des nombres de Stirling associés. CR Acad. Sci. Paris 1995, 320, 1449–1452. [Google Scholar]
- Hassani, M. Approximation of the Lambert W Function. Rgmia Res. Rep. Collect. 2005, 8, 12. [Google Scholar]
- Comtet, L. Advanced Combinatorics; D. Reidel Publishing Company: Dordrecht, The Netherlands, 1974. [Google Scholar]
- Mitrinović, D.S.; Doković, D. Sur une relation de récurrence concernant les nombres de Stirling. CR Acad. Sci. 1960, 250, 2110–2111. [Google Scholar]
- Karanicoloff, C. Sur une représentation des nombres de Stirling dans une forme explicite. Publ. Elektrotehničkog Fak. Ser. Mat. Fiz. 1961, 67, 9–10. (In French) [Google Scholar]
- Graham, R.L.; Knuth, D.E.; Patashnik, O. Concrete Mathematics; Addison-Wesley: Boston, MA, USA, 1994. [Google Scholar]
- Iglesias, C.A.; Sonnad, V.; Wilson, B.G.; Castor, J.I. Frequency dependent electron collisional widths for opacity calculations. High Energy Density Phys. 2009, 5, 97–104. [Google Scholar] [CrossRef]
- Dufty, J.W. Charge-density fluctuations in spectral line broadening. Phys. Rev. 1969, 187, 305–313. [Google Scholar] [CrossRef]
- Dufty, J.W. Ion motion in plasma line broadening. Phys. Rev. A 1970, 2, 534–541. [Google Scholar] [CrossRef]
- Lee, R.W. Spectral Line-Broadening Codes for Hydrogen-, Helium- and Lithium-like Ions in Plasmas; LLNL-Report, UCID-21292; NASA/ADS: Cambridge, MA, USA, 1987. [Google Scholar]
- Lee, R.W. Plasma line shapes for selected transitions in hydrogen-, helium- and lithium-like ions. J. Quant. Spectrosc. Radiat. Transf. 1988, 40, 561–568. [Google Scholar] [CrossRef]
- Cowley, E.R.; Zekaria, F. Moment-expansion-method calculations of phonon line shapes in argon. Phys. Rev. B 1994, 50, 16380–16385. [Google Scholar] [CrossRef] [PubMed]
- Viswanath, V.S.; Zhang, S.; Stolze, J.; Muller, G. Ordering and fluctuations in the ground state of the one-dimensional and two-dimensional S = 1/2 − XXZ antiferromagnets: A study of dynamical properties based on the recursion method. Phys. Rev. B 1994, 49, 9702–9715. [Google Scholar] [CrossRef]
- Blumstein, C.; Wheeler, C. Modified-moments method: Applications to harmonic solids. Phys. Rev. B 1973, 8, 1764–1776. [Google Scholar] [CrossRef]
- Michette, A.G.; Pfauntsch, S.J. Laser plasma X-ray line spectra fitted using the Pearson VII function. J. Phys. D Appl. Phys. 2000, 33, 1186–1190. [Google Scholar] [CrossRef]
- Wang, H.; Zhou, J. Numerical conversion between the Pearson VII and pseudo-Voigt functions. J. Appl. Cryst. 2005, 38, 830–832. [Google Scholar] [CrossRef]
- Sapar, A.; Poolamäe, R.; Sapar, L. High-precision approximation expressions for line profiles of hydrogenic particles. Balt. Astron. 2006, 15, 435–447. [Google Scholar]
- Pain, J.-C. Expression of the Holtsmark function in terms of hypergeometric 2F2 and Airy Bi functions. Eur. Phys. J. Plus 2020, 135, 236. [Google Scholar] [CrossRef]
- Fried, B.D.; Conte, S.D. The Plasma Dispersion Function; Academic Press: New York, NY, USA; London, UK, 1961. [Google Scholar]
- Drayson, S.R. Rapid computation of the Voigt profile. J. Quant. Spectrosc. Radiat. Transf. 1976, 16, 611–614. [Google Scholar] [CrossRef]
- Dawson, J.; Obermann, C. High-frequency conductivity and the emission and absorption coefficients of a fully ionized plasma. Phys. Fluids 1962, 5, 517–524. [Google Scholar] [CrossRef]
- Kim, T.-S.; Carswell, R.F.; Cristiani, S.; D’Odorico, S.; Giallongo, E. The physical properties of the Lyα forest at z>1.5. Mon. Not. R. Astron. Soc. 2002, 335, 555–573. [Google Scholar] [CrossRef]
- Mihalas, D. Stellar Atmospheres; W. H. Freeman and Company: New York, NY, USA, 1971. [Google Scholar]
- Harris, D.L., III. On the line-absorption coefficient due to Doppler effect and damping. Astrophys. J. 1948, 108, 112–115. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pain, J.-C. Extracting Physical Information from the Voigt Profile Using the Lambert W Function. Plasma 2024, 7, 427-445. https://doi.org/10.3390/plasma7020023
Pain J-C. Extracting Physical Information from the Voigt Profile Using the Lambert W Function. Plasma. 2024; 7(2):427-445. https://doi.org/10.3390/plasma7020023
Chicago/Turabian StylePain, Jean-Christophe. 2024. "Extracting Physical Information from the Voigt Profile Using the Lambert W Function" Plasma 7, no. 2: 427-445. https://doi.org/10.3390/plasma7020023
APA StylePain, J.-C. (2024). Extracting Physical Information from the Voigt Profile Using the Lambert W Function. Plasma, 7(2), 427-445. https://doi.org/10.3390/plasma7020023





