A Mechanism for Large-Amplitude Parallel Electrostatic Waves Observed at the Magnetopause
Abstract
1. Introduction
2. Theoretical Model
2.1. Ion- and Electron-Acoustic Soliton Solutions
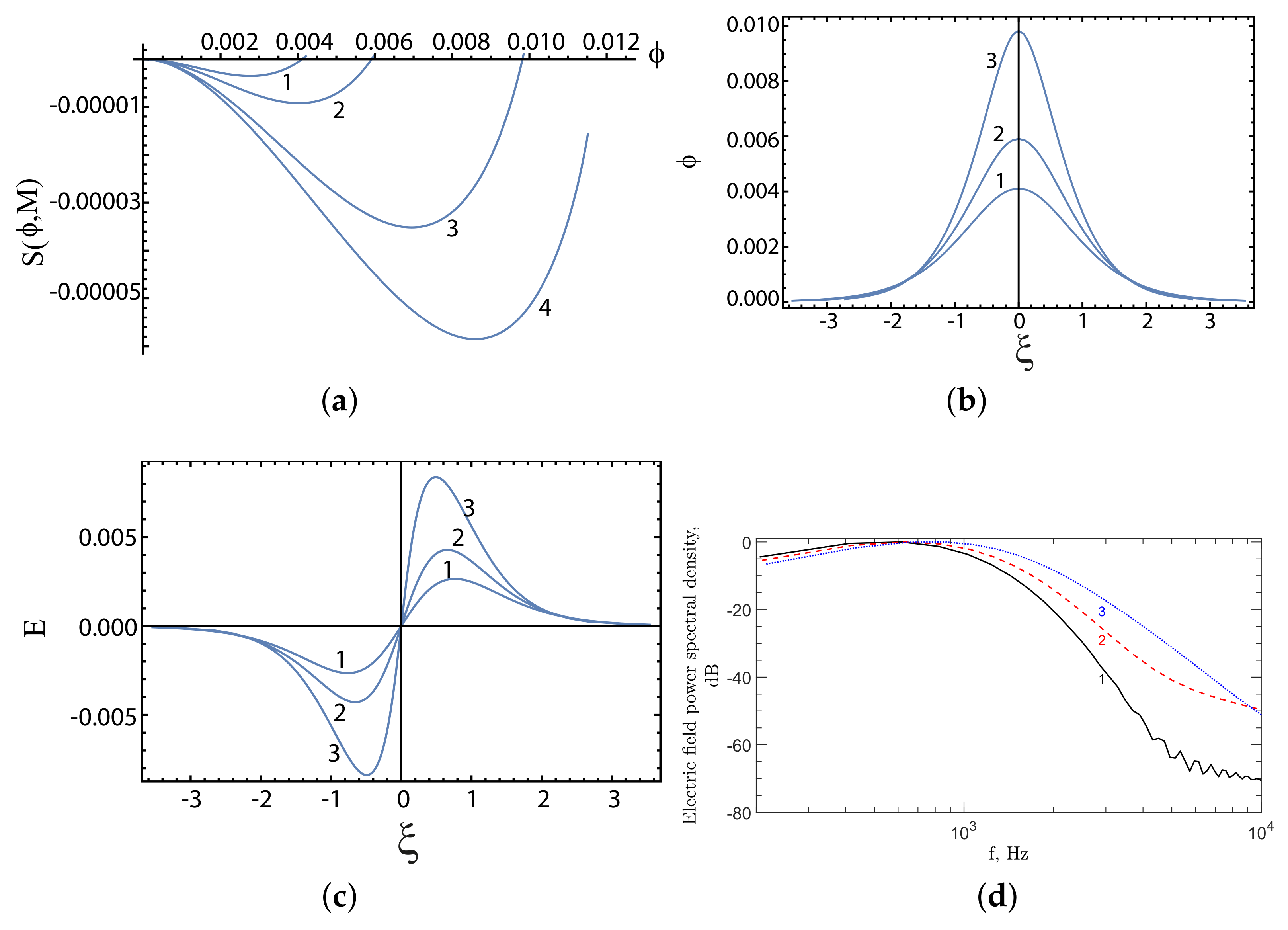
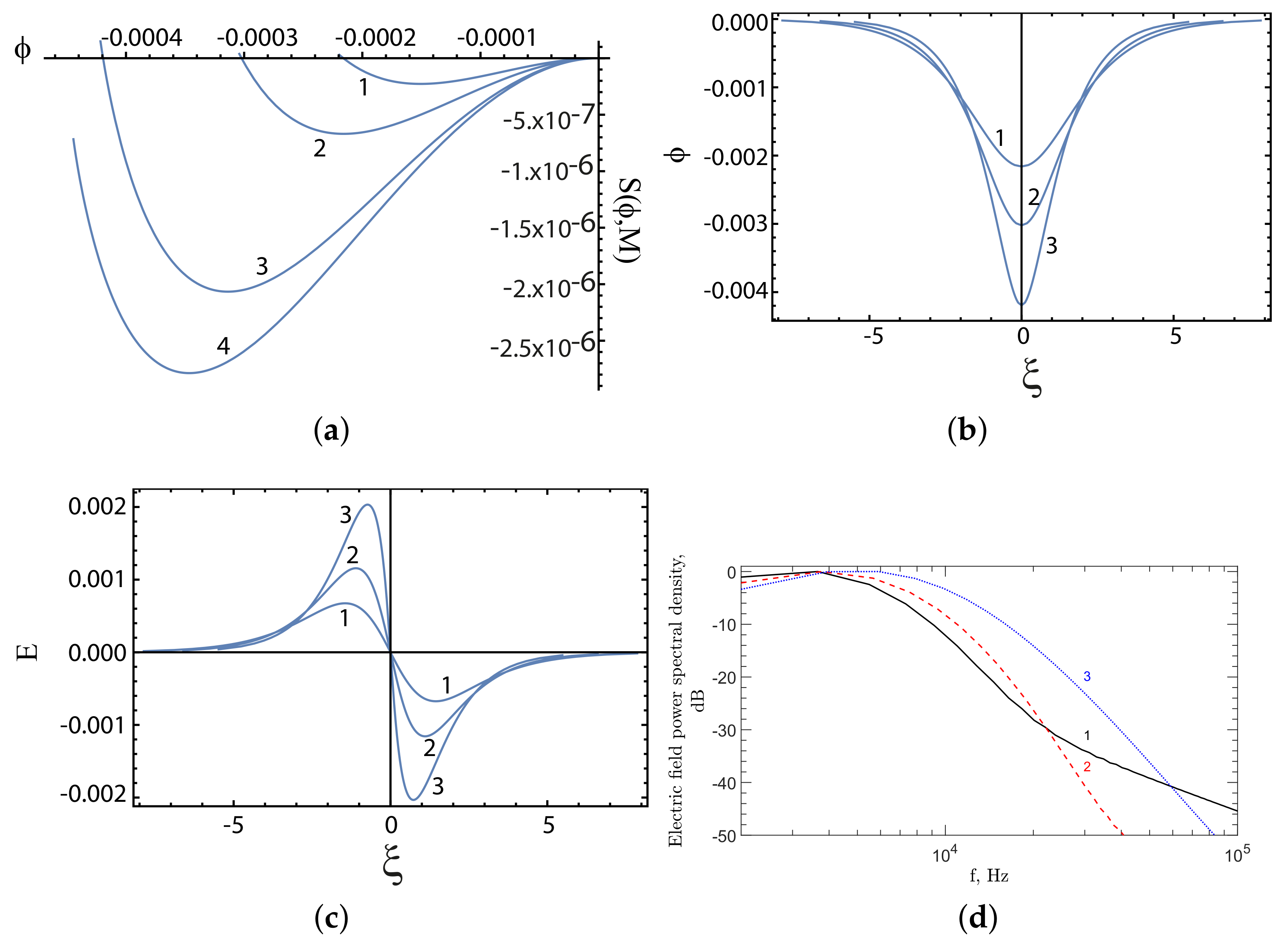
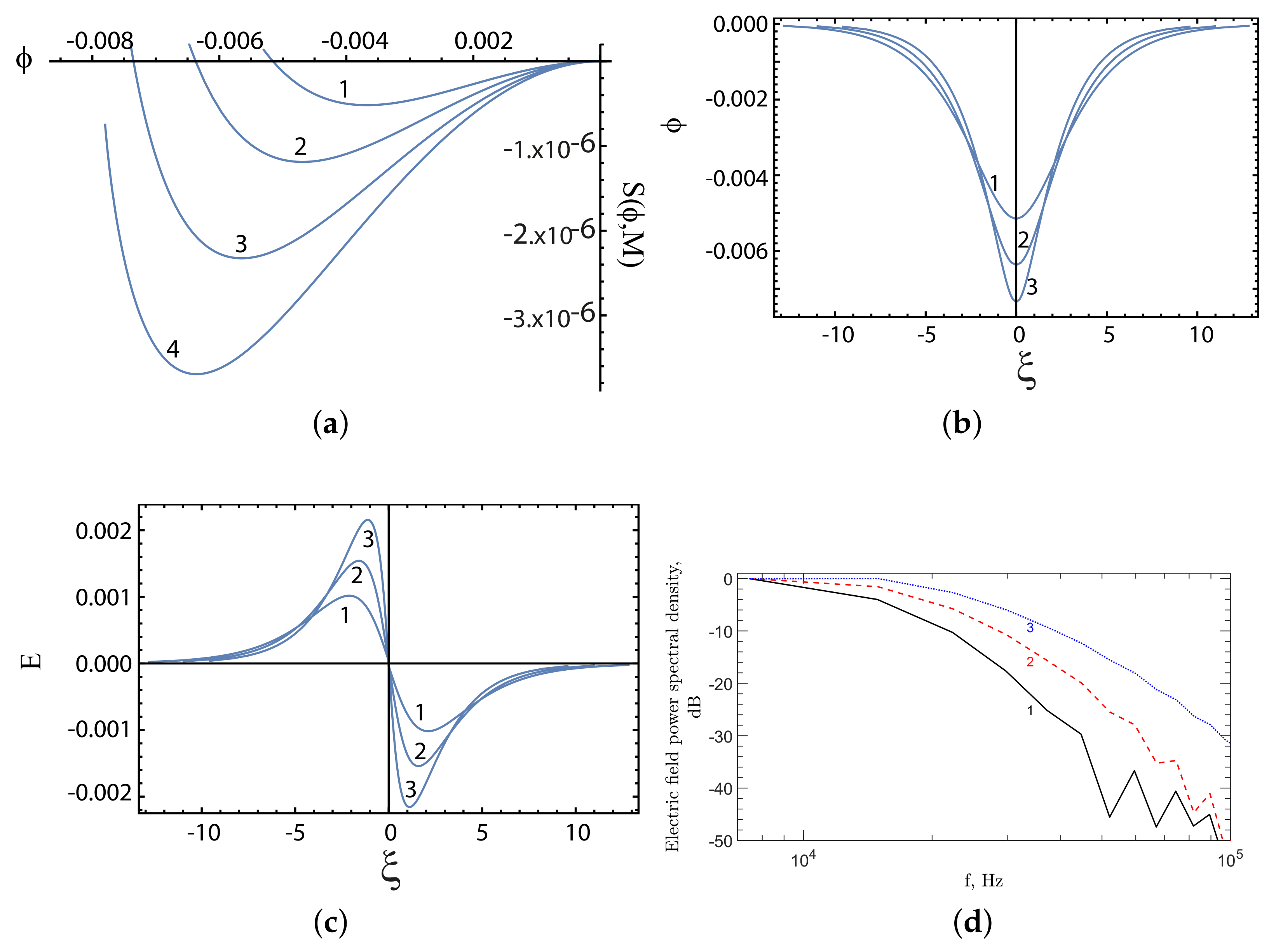
Numerical Results
2.2. Predictions of the Model
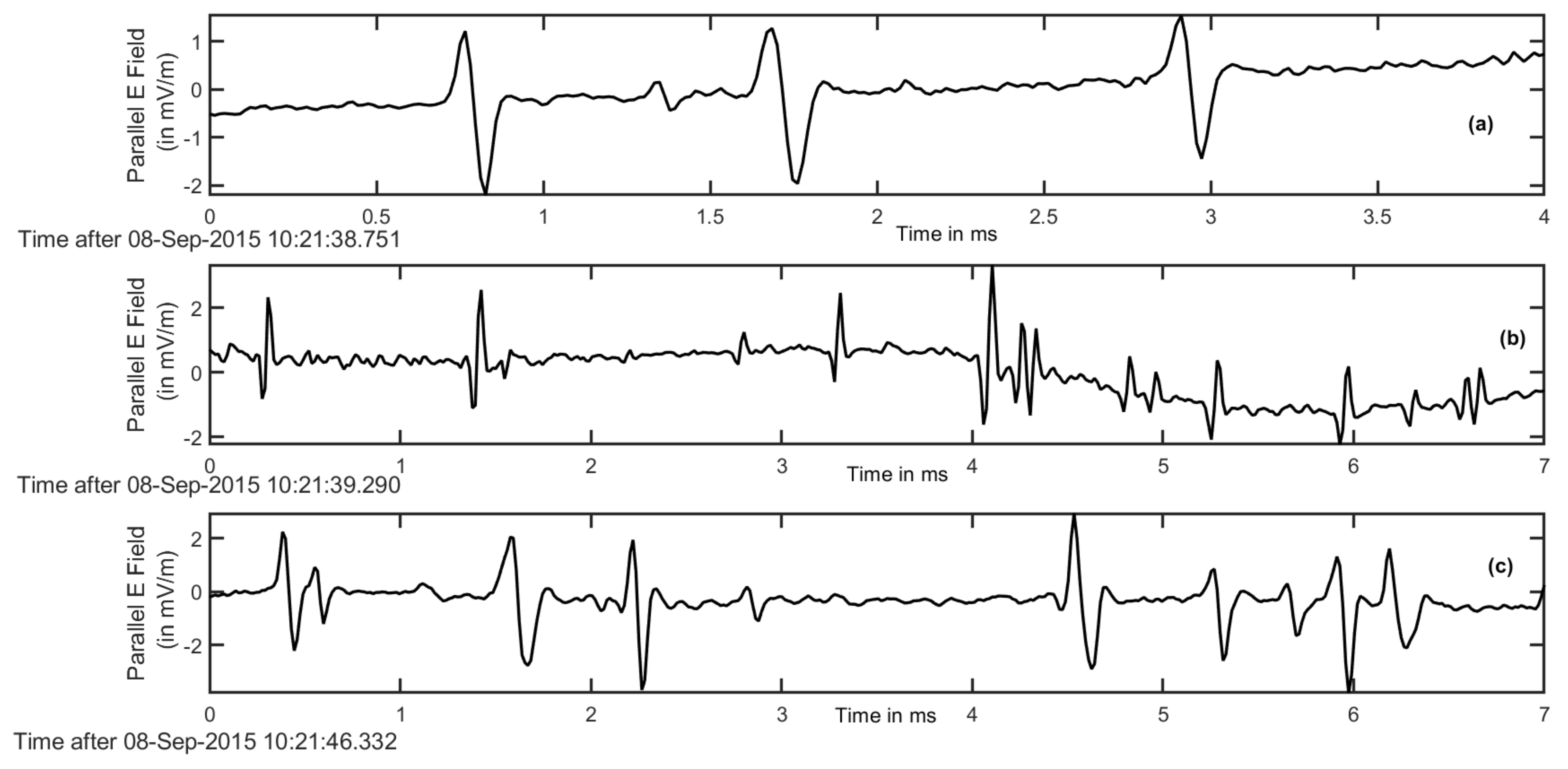
2.3. Comparison of Theoretical Predictions with Observations of Large Amplitude Electrostatic Waves at the Magnetopause
3. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gurnett, D.A.; Anderson, R.R.; Tsurutani, B.T.; Smith, E.J.; Paschmann, G.; Haerendel, G.; Bame, S.J.; Russell, C.T. Plasma wave turbulence at the magnetopause: Observations from ISEE 1 and 2. J. Geophys. Res. 1979, 84, 7043–7058. [Google Scholar] [CrossRef][Green Version]
- Matsumoto, H.; Deng, X.H.; Kojima, H.; Anderson, R.R. Observation of Electrostatic Solitary Waves associated with reconnection on the dayside magnetopause boundary. Geophys. Res. Lett. 2003, 30, 1326. [Google Scholar] [CrossRef]
- Graham, D.B.; Khotyaintsev, Y.V.; Vaivads, A.; André, M. Electrostatic solitary waves with distinct speeds associated with asymmetric reconnection. Geophys. Res. Lett. 2015, 42, 215–224. [Google Scholar] [CrossRef]
- Matsumoto, H.; Kojima, H.; Miyatake, T.; Omura, Y.; Okada, M.; Nagano, I.; Tsutsui, M. Electrostatic solitary waves (ESW) in the magnetotail: BEN wave forms observed by GEOTAIL. Geophys. Res. Lett. 1994, 21, 2915–2918. [Google Scholar] [CrossRef]
- Kojima, H.; Matsumoto, H.; Chikuba, S.; Horiyama, S.; Ashour-Abdalla, M.; Anderson, R.R. Geotail waveform observations of broadband/narrowband electrostatic noise in the distant tail. J. Geophys. Res. 1997, 102, 14439–14455. [Google Scholar] [CrossRef]
- Pickett, J.S.; Menietti, J.D.; Gurnett, D.A.; Tsurutani, B.; Kintner, P.M.; Klatt, E.; Balogh, A. Solitary potential structures observed in the magnetosheath by the Cluster spacecraft. Nonlin. Process. Geophys. 2003, 10, 3–11. [Google Scholar] [CrossRef][Green Version]
- Pickett, J.S.; Chen, L.J.; Kahler, S.W.; Santolík, O.; Gurnett, D.A.; Tsurutani, B.T.; Balogh, A. Isolated electrostatic structures observed throughout the Cluster orbit: Relationship to magnetic field strength. Ann. Geophys. 2004, 22, 2515–2523. [Google Scholar] [CrossRef][Green Version]
- Pickett, J.S.; Chen, L.J.; Kahler, S.W.; Santolík, O.; Goldstein, M.L.; Lavraud, B.; Décréau, P.M.E.; Kessel, R.; Lucek, E.; Lakhina, G.S.; et al. On the generation of solitary waves observed by Cluster in the near-Earth magnetosheath. Nonlinear Process. Geophys. 2005, 12, 181–193. [Google Scholar] [CrossRef][Green Version]
- Holmes, J.C.; Ergun, R.E.; Newman, D.L.; Wilder, F.D.; Sturner, A.P.; Goodrich, K.A.; Torbert, R.B.; Giles, B.L.; Strangeway, R.J.; Burch, J.L. Negative potential solitary structures in the magnetosheath with large parallel width. J. Geophys. Res. 2018, 123, 132–145. [Google Scholar] [CrossRef][Green Version]
- Lakhina, G.S.; Tsurutani, B.T.; Kojima, H.; Matsumoto, H. “Broadband” plasma waves in the boundary layers. J. Geophys. Res. 2000, 105, 27791–27831. [Google Scholar] [CrossRef][Green Version]
- Bernstein, I.B.; Greene, J.M.; Kruskal, M.D. Exact Nonlinear Plasma Oscillations. Phys. Rev. 1957, 108, 546–550. [Google Scholar] [CrossRef][Green Version]
- Schamel, H. Stationary solitary, snoidal and sinusoidal ion acoustic waves. Plasma Phys. 1972, 14, 905–924. [Google Scholar] [CrossRef]
- Omura, Y.; Matsumoto, H.; Miyake, T.; Kojima, H. Electron beam instabilities as generation mechanism of electrostatic solitary waves in the magnetotail. J. Geophys. Res. 1996, 101, 2685–2697. [Google Scholar] [CrossRef]
- Schamel, H. Particle trapping: A key requisite of structure formation and stability of Vlasov–Poisson plasmas. Phys. Plasmas 2015, 22, 042301. [Google Scholar] [CrossRef][Green Version]
- Hutchinson, I.H. Electron holes in phase space: What they are and why they matter. Phys. Plasmas 2017, 24, 055601. [Google Scholar] [CrossRef]
- Goldman, M.V.; Oppenheim, M.M.; Newman, D.L. Nonlinear two-stream instabilities as an explanation for auroral bipolar wave structures. Geophys. Res. Lett. 1999, 26, 1821–1824. [Google Scholar] [CrossRef]
- Oppenheim, M.; Newman, D.L.; Goldman, M.V. Evolution of Electron Phase-Space Holes in a 2D Magnetized Plasma. Phys. Rev. Lett. 1999, 83, 2344–2347. [Google Scholar] [CrossRef][Green Version]
- Muschietti, L.; Ergun, R.E.; Roth, I.; Carlson, C.W. Phase-space electron holes along magnetic field lines. Geophys. Res. Lett. 1999, 26, 1093–1096. [Google Scholar] [CrossRef]
- Singh, N. Space-time evolution of electron-beam driven electron holes and their effects on the plasma. Nonlinear Process. Geophys. 2003, 10, 53–63. [Google Scholar] [CrossRef][Green Version]
- Ergun, R.E.; Carlson, C.W.; McFadden, J.P.; Mozer, F.S.; Muschietti, L.; Roth, I.; Strangeway, R.J. Debye-Scale Plasma Structures Associated with Magnetic-Field-Aligned Electric Fields. Phys. Rev. Lett. 1998, 81, 826–829. [Google Scholar] [CrossRef]
- Franz, J.R.; Kintner, P.M.; Pickett, J.S.; Chen, L.J. Properties of small-amplitude electron phase-space holes observed by Polar. J. Geophys. Res. 2005, 110, A09212. [Google Scholar] [CrossRef][Green Version]
- Chen, L.J.; Pickett, J.; Kintner, P.; Franz, J.; Gurnett, D. On the width-amplitude inequality of electron phase space holes. J. Geophys. Res. 2005, 110, A09211. [Google Scholar] [CrossRef]
- Omura, Y.; Kojima, H.; Matsumoto, H. Computer simulation of electrostatic solitary waves: A nonlinear model of broadband electrostatic noise. Geophys. Res. Lett. 1994, 21, 2923–2926. [Google Scholar] [CrossRef]
- Singh, N.; Schunk, R.W. Plasma response to the injection of an electron beam. Plasma Phys. Control Fusion 1984, 26, 859. [Google Scholar] [CrossRef]
- Singh, N.; Thiemann, H.; Schunk, R.W. Simulations of auroral plasma processes: Electric fields, waves and particles. Planet. Space Sci. 1987, 35, 353–395. [Google Scholar] [CrossRef]
- Singh, N.; Loo, S.M.; Wells, E. Electron hole structure and its stability depending on plasma magnetization. J. Geophys. Res. 2001, 106, 21183–21198. [Google Scholar] [CrossRef]
- Ergun, R.E.; Carlson, C.W.; McFadden, J.P.; Mozer, F.S.; Delory, G.T.; Peria, W.; Chaston, C.C.; Temerin, M.; Roth, I.; Muschietti, L.; et al. FAST satellite observations of large-amplitude solitary structures. Geophys. Res. Lett. 1998, 25, 2041–2044. [Google Scholar] [CrossRef]
- Muschietti, L.; Roth, I.; Carlson, C.W.; Ergun, R.E. Transverse Instability of Magnetized Electron Holes. Phys. Rev. Lett. 2000, 85, 94–97. [Google Scholar] [CrossRef]
- Graham, D.B.; Khotyaintsev, Y.V.; Vaivads, A.; André, M. Electrostatic solitary waves and electrostatic waves at the magnetopause. J. Geophys. Res. Space Phys. 2016, 121, 3069–3092. [Google Scholar] [CrossRef][Green Version]
- Mozer, F.S.; Agapitov, O.V.; Giles, B.; Vasko, I. Direct Observation of Electron Distributions inside Millisecond Duration Electron Holes. Phys. Rev. Lett. 2018, 121, 135102. [Google Scholar] [CrossRef][Green Version]
- Steinvall, K.; Khotyaintsev, Y.V.; Graham, D.B.; Vaivads, A.; Lindqvist, P.A.; Russell, C.T.; Burch, J.L. Multispacecraft analysis of electron holes. Geophys. Res. Lett. 2019, 46, 55–63. [Google Scholar] [CrossRef][Green Version]
- Lotekar, A.; Vasko, I.Y.; Mozer, F.S.; Hutchinson, I.; Artemyev, A.V.; Bale, S.D.; Bonnell, J.W.; Ergun, R.; Giles, B.; Khotyaintsev, Y.V.; et al. Multisatellite MMS analysis of electron holes in the Earth’s magnetotail: Origin, properties, velocity gap, and transverse instability. J. Geophys. Res. Space Phys. 2020, 125, e2020JA028066. [Google Scholar] [CrossRef]
- Wang, R.; Vasko, I.Y.; Artemyev, A.V.; Holley, L.C.; Kamaletdinov, S.R.; Lotekar, A.; Mozer, F.S. Multisatellite observations of ion holes in the Earth’s plasma sheet. Geophys. Res. Lett. 2022, 49, e2022GL097919. [Google Scholar] [CrossRef]
- Sagdeev, R.Z. Cooperative Phenomena and Shock Waves in Collisionless Plasmas. In Reviews of Plasma Physics; Leontovich, M.A., Ed.; Consultants Bureau: New York, NY, USA, 1966; Volume 4, pp. 23–91. [Google Scholar]
- Ghosh, S.S.; Iyengar, A.N.S. Anomalous width variations for ion acoustic rarefactive solitary waves in a warm ion plasma with two electron temperatures. Phys. Plasmas 1997, 4, 3204–3210. [Google Scholar] [CrossRef]
- Ghosh, S.S.; Lakhina, G.S. Anomalous width variation of rarefactive ion acoustic solitary waves in the context of auroral plasmas. Nonlinear Process. Geophys. 2004, 11, 219–228. [Google Scholar] [CrossRef]
- Singh, S.V.; Reddy, R.V.; Lakhina, G.S. Broadband electrostatic noise due to nonlinear electron-acoustic waves. Adv. Space Res. 2001, 28, 1643–1648. [Google Scholar] [CrossRef]
- Ghosh, S.S.; Pickett, J.S.; Lakhina, G.S.; Winningham, J.D.; Lavraud, B.; Décréau, P.M.E. Parametric analysis of positive amplitude electron acoustic solitary waves in a magnetized plasma and its application to boundary layers. J. Geophys. Res. 2008, 113, A06218. [Google Scholar] [CrossRef][Green Version]
- Lakhina, G.S.; Kakad, A.P.; Singh, S.V.; Verheest, F. Ion- and electron-acoustic solitons in two-electron temperature space plasmas. Phys. Plasmas 2008, 15, 062903. [Google Scholar] [CrossRef]
- Lakhina, G.S.; Singh, S.V.; Kakad, A.P.; Verheest, F.; Bharuthram, R. Study of nonlinear ion- and electron-acoustic waves in multi-component space plasmas. Nonlinear Process. Geophys. 2008, 15, 903–913. [Google Scholar] [CrossRef][Green Version]
- Devanandhan, S.; Singh, S.V.; Lakhina, G.S. Electron acoustic solitary waves with kappa-distributed electrons. Phys. Scr. 2011, 84, 025507. [Google Scholar] [CrossRef]
- Maharaj, S.K.; Bharuthram, R.; Singh, S.V.; Lakhina, G.S. Existence domains of arbitrary amplitude nonlinear structures in two-electron temperature space plasmas. I. Low-frequency ion-acoustic solitons. Phys. Plasmas 2012, 19, 072320. [Google Scholar] [CrossRef][Green Version]
- Dillard, C.S.; Vasko, I.Y.; Mozer, F.S.; Agapitov, O.V.; Bonnell, J.W. Electron-acoustic solitary waves in the Earth’s inner magnetosphere. Phys. Plasmas 2018, 25, 022905. [Google Scholar] [CrossRef]
- Lakhina, G.S.; Singh, S.V.; Rubia, R. A mechanism for electrostatic solitary waves observed in the reconnection jet region of the Earth’s magnetotail. Adv. Space Res. 2021, 68, 1864–1875. [Google Scholar] [CrossRef]
- Lakhina, G.S.; Singh, S.V.; Rubia, R.; Sreeraj, T. A review of nonlinear fluid models for ion-and electron-acoustic solitons and double layers: Application to weak double layers and electrostatic solitary waves in the solar wind and the lunar wake. Phys. Plasmas 2018, 25, 080501. [Google Scholar] [CrossRef]
- Lakhina, G.S.; Singh, S.V.; Rubia, R.; Devanandhan, S. Electrostatic Solitary Structures in Space Plasmas: Soliton Perspective. Plasma 2021, 4, 681–731. [Google Scholar] [CrossRef]
- Ergun, R.E.; Holmes, J.C.; Goodrich, K.A.; Wilder, F.D.; Stawarz, J.E.; Eriksson, S.; Newman, D.L.; Schwartz, S.J.; Goldman, M.V.; Sturner, A.P.; et al. Magnetospheric Multiscale observations of large-amplitude, parallel, electrostatic waves associated with magnetic reconnection at the magnetopause. Geophys. Res. Lett. 2016, 43, 5626–5634. [Google Scholar] [CrossRef][Green Version]
- Ergun, R.E.; Goodrich, K.A.; Wilder, F.D.; Holmes, J.C.; Stawarz, J.E.; Eriksson, S.; Sturner, A.P.; Malaspina, D.M.; Usanova, M.E.; Torbert, R.B.; et al. Magnetospheric multiscale satellites observations of parallel electric fields associated with magnetic reconnection. Phys. Rev. Lett. 2016, 116, 235102. [Google Scholar] [CrossRef][Green Version]
- Rufai, O.R.; Khazanov, G.V.; Singh, S.V.; Lakhina, G.S. Large-amplitude electrostatic fluctuations at the Earth’s magnetopause with a vortex-like distribution of hot electrons. Results Phys. 2022, 35, 105343. [Google Scholar] [CrossRef]
- Rufai, O.R.; Khazanov, G.V.; Singh, S.V. Finite amplitude electron-acoustic waves in the electron diffusion region. Results Phys. 2021, 24, 104041. [Google Scholar] [CrossRef]
- Rufai, O.R.; Singh, S.V.; Lakhina, G.S. Nonlinear electrostatic structures and stopbands in a three-component magnetosheath plasma. Astrophys. Space Sci. 2023, 368, 35. [Google Scholar] [CrossRef]
- Wilder, F.D.; Ergun, R.E.; Schwartz, S.J.; Newman, D.L.; Eriksson, S.; Stawarz, J.E.; Goldman, M.V.; Goodrich, K.A.; Gershman, D.J.; Malaspina, D.M.; et al. Observations of large-amplitude, parallel, electrostatic waves associated with the Kelvin-Helmholtz instability by the magnetospheric multiscale mission. Geophys. Res. Lett. 2016, 43, 8859–8866. [Google Scholar] [CrossRef][Green Version]
- Nakamura, T.K.M.; Daughton, W.; Karimabadi, H.; Eriksson, S. Three-dimensional dynamics of vortex-induced reconnection and comparison with THEMIS observations. J. Geophys. Res. Space Phys. 2013, 118, 5742–5757. [Google Scholar] [CrossRef]
- Kindel, J.M.; Kennel, C.F. Topside current instabilities. J. Geophys. Res. 1971, 76, 3055. [Google Scholar] [CrossRef]
- Lakhina, G.S.; Singh, S.V.; Kakad, A.P.; Pickett, J.S. Generation of electrostatic solitary waves in the plasma sheet boundary layer. J. Geophys. Res. 2011, 116, A10218. [Google Scholar] [CrossRef]
- Lakhina, G.S.; Singh, S.V.; Kakad, A.P. Ion acoustic solitons/double layers in two-ion plasma revisited. Phys. Plasmas 2014, 21, 062311. [Google Scholar] [CrossRef]
- Lakhina, G.S.; Singh, S.V.; Rubia, R. A new class of Ion-acoustic solitons that can exist below critical Mach number. Phys. Scr. 2020, 95, 105601. [Google Scholar] [CrossRef]

| Modes | Polarity | Mach Number | V (km s) | W (m) | E (mV m) | (V) | (Hz) |
|---|---|---|---|---|---|---|---|
| Slow ion-acoustic | Positive | 0.57 | 137 | 83 | 40 | 2.4 | 616 |
| 0.58 | 139 | 72 | 60 | 3.6 | 627 | ||
| 0.60 | 144 | 59 | 120 | 5.7 | 865 | ||
| Slow electron-acoustic | Negative | 6.5 | 1560 | 158 | 10 | 1.3 | 3659 |
| 6.7 | 1608 | 134 | 15 | 1.8 | 3772 | ||
| 7.0 | 1680 | 102 | 30 | 2.5 | 5911 | ||
| Fast electron-acoustic | Negative | 26.45 | 6348 | 265 | 12 | 3.1 | 7445 |
| 26.50 | 6360 | 211 | 21 | 3.8 | 7459 | ||
| 26.55 | 6372 | 169 | 33 | 4.5 | 14,946 |
| Modes | Polarity | Mach Number | V (km s) | W (m) | E (mV m) | (V) | (Hz) |
|---|---|---|---|---|---|---|---|
| Slow ion-acoustic | Positive | 0.70 | 168 | 123 | 120 | 11 | 504 |
| 0.75 | 180 | 97 | 320 | 24 | 541 | ||
| 0.765 | 184 | 87 | 400 | 28 | 551 | ||
| Slow electron-acoustic | Negative | 4.20 | 1008 | 177 | 1 | 0.15 | 1513 |
| 4.30 | 1032 | 131 | 2 | 0.23 | 2324 | ||
| 4.35 | 1044 | 114 | 3 | 0.27 | 2351 | ||
| Fast electron-acoustic | Negative | 26.30 | 6312 | 400 | 5 | 1.5 | 4738 |
| 26.40 | 6336 | 240 | 15 | 3.0 | 7134 | ||
| 26.50 | 6360 | 165 | 30 | 4.2 | 9548 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lakhina, G.S.; Singh, S.; Sreeraj, T.; Devanandhan, S.; Rubia, R. A Mechanism for Large-Amplitude Parallel Electrostatic Waves Observed at the Magnetopause. Plasma 2023, 6, 345-361. https://doi.org/10.3390/plasma6020024
Lakhina GS, Singh S, Sreeraj T, Devanandhan S, Rubia R. A Mechanism for Large-Amplitude Parallel Electrostatic Waves Observed at the Magnetopause. Plasma. 2023; 6(2):345-361. https://doi.org/10.3390/plasma6020024
Chicago/Turabian StyleLakhina, Gurbax Singh, Satyavir Singh, Thekkeyil Sreeraj, Selvaraj Devanandhan, and Rajith Rubia. 2023. "A Mechanism for Large-Amplitude Parallel Electrostatic Waves Observed at the Magnetopause" Plasma 6, no. 2: 345-361. https://doi.org/10.3390/plasma6020024
APA StyleLakhina, G. S., Singh, S., Sreeraj, T., Devanandhan, S., & Rubia, R. (2023). A Mechanism for Large-Amplitude Parallel Electrostatic Waves Observed at the Magnetopause. Plasma, 6(2), 345-361. https://doi.org/10.3390/plasma6020024






