Plane Parallel Barrier Discharges for Carbon Dioxide Splitting: Influence of Discharge Arrangement on Carbon Monoxide Formation
Abstract
1. Introduction
2. Experimental Set-Up and Procedures
3. Electrical Characterization
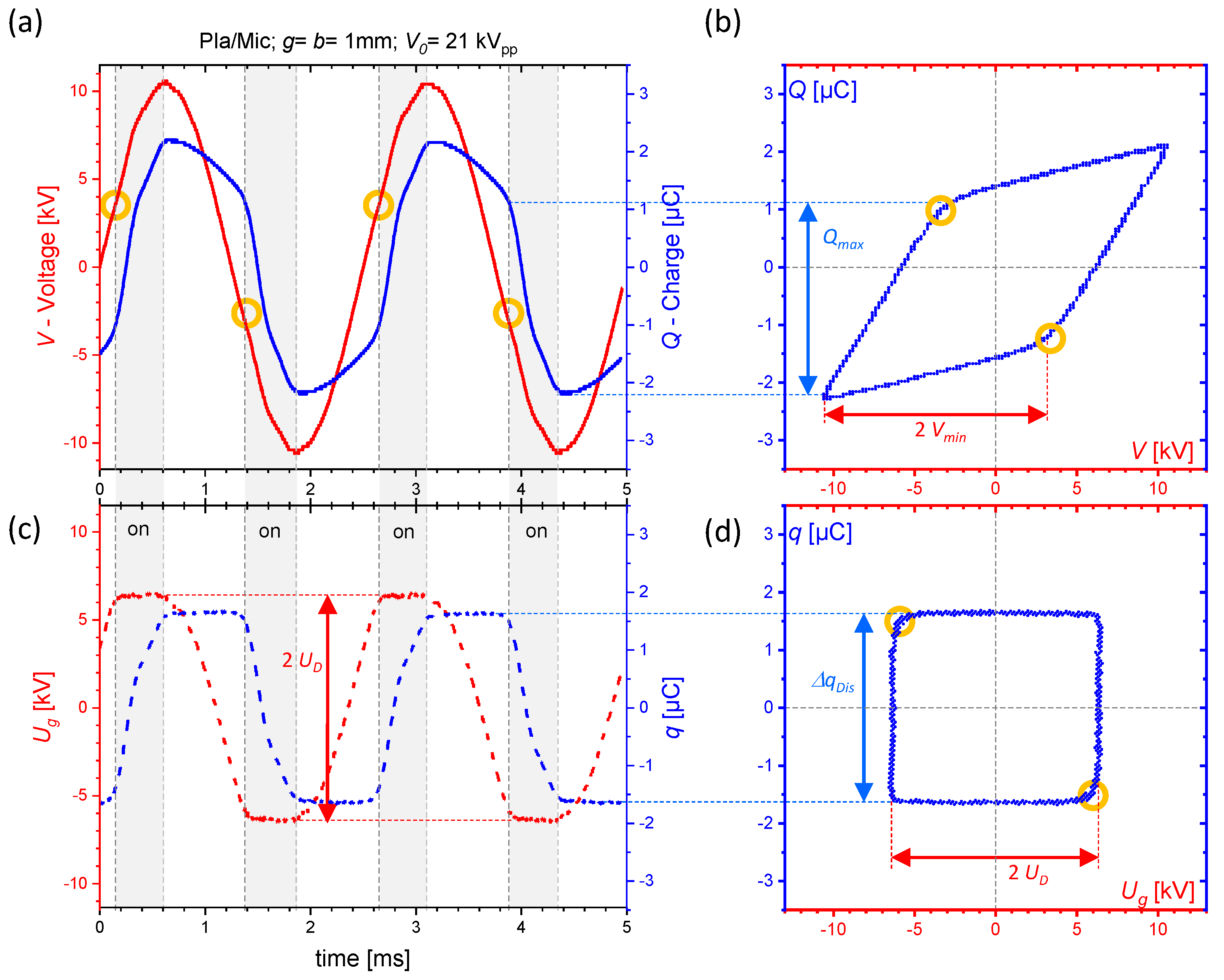
3.1. Q-V Plots and Equivalent Circuit
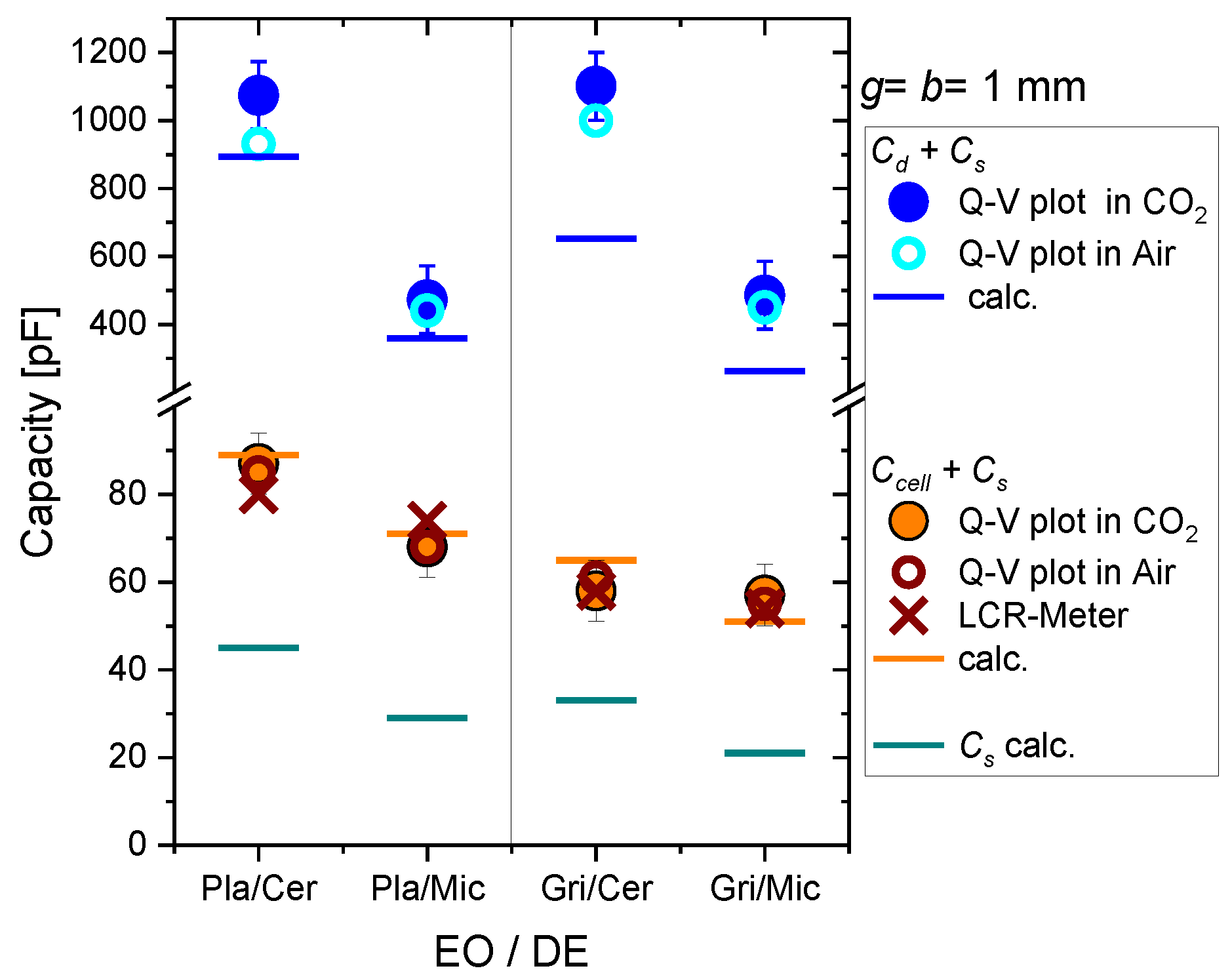
3.2. Capacitances and Discharge Power
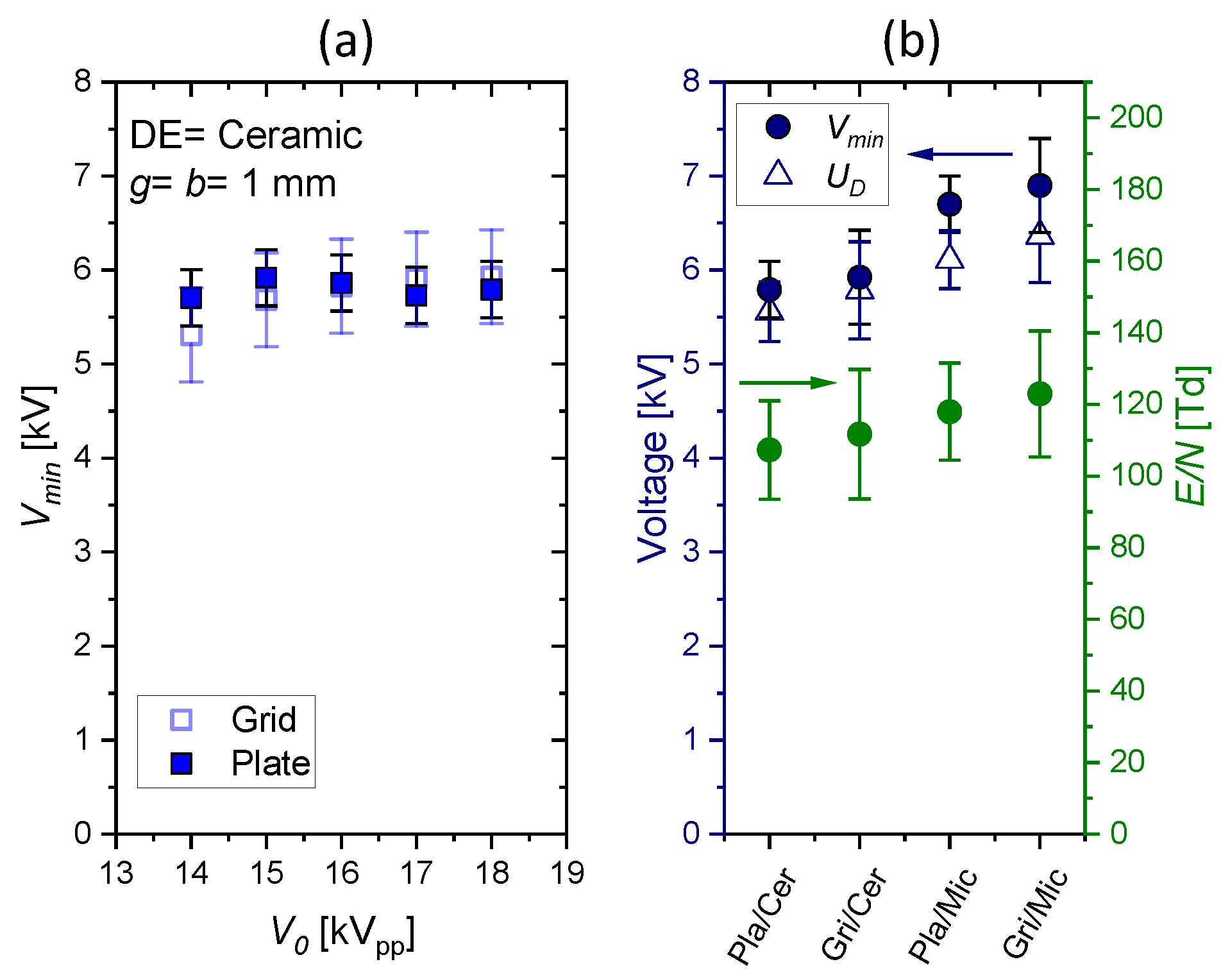
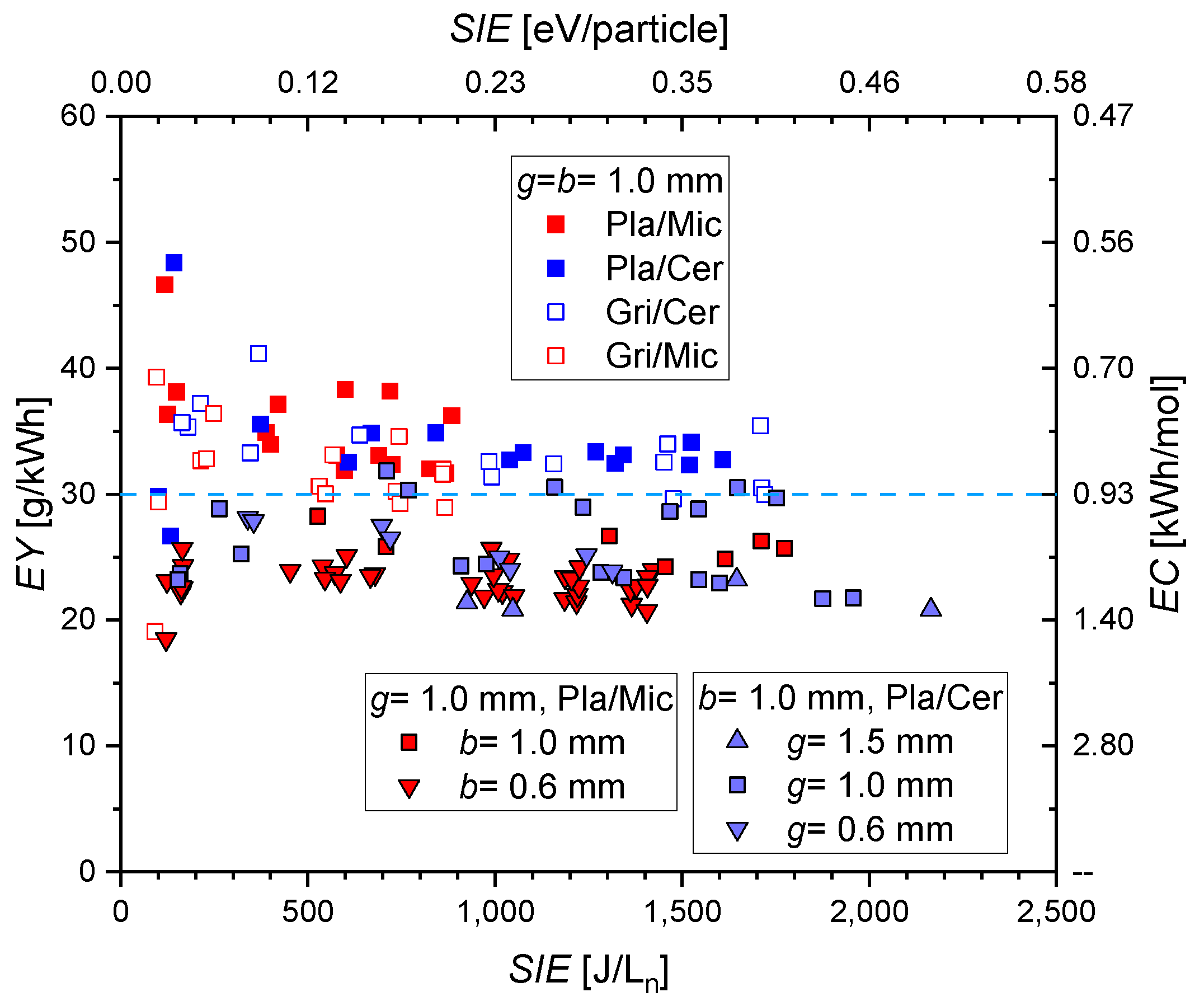
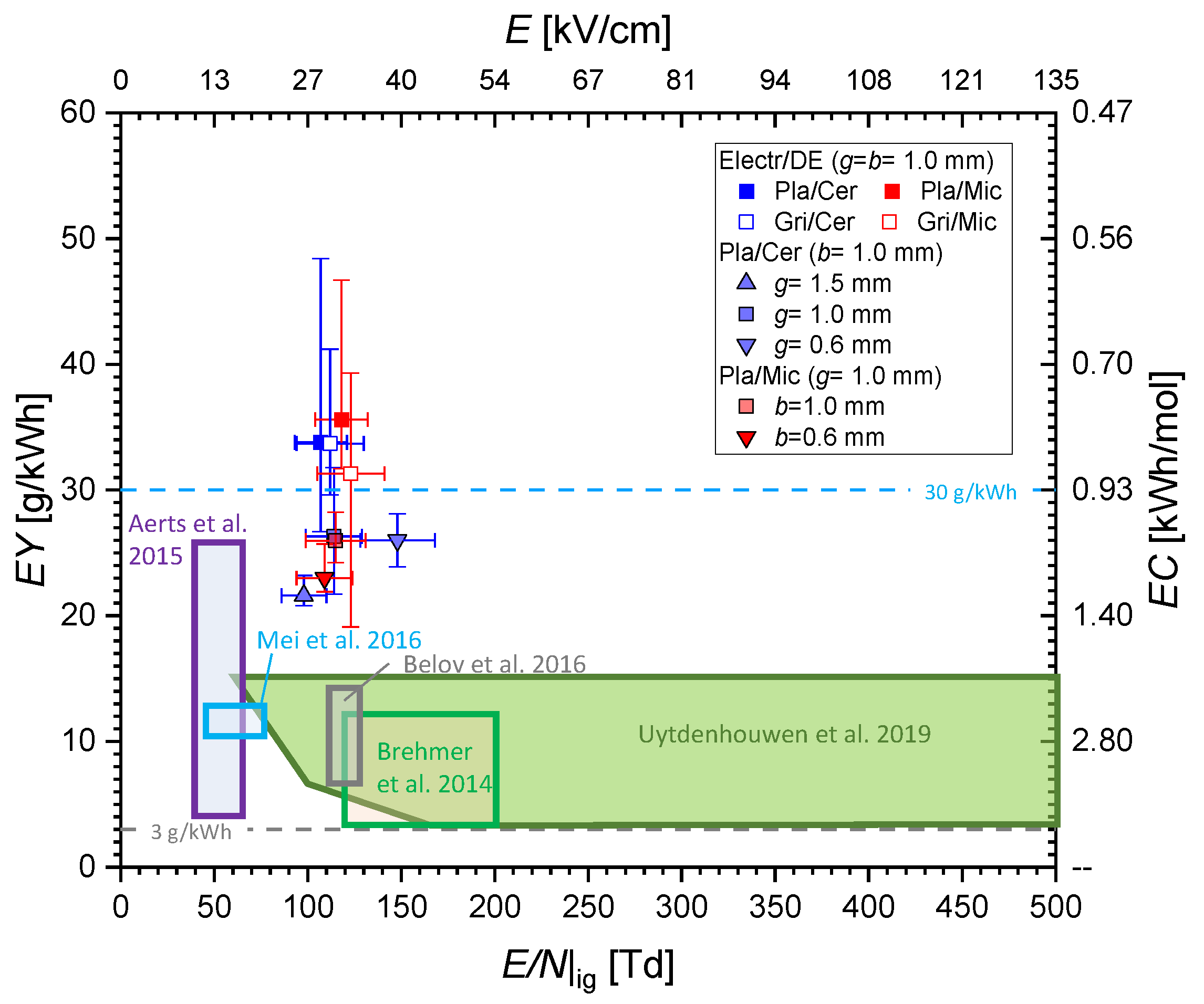
3.3. Variation of DBD Geometry
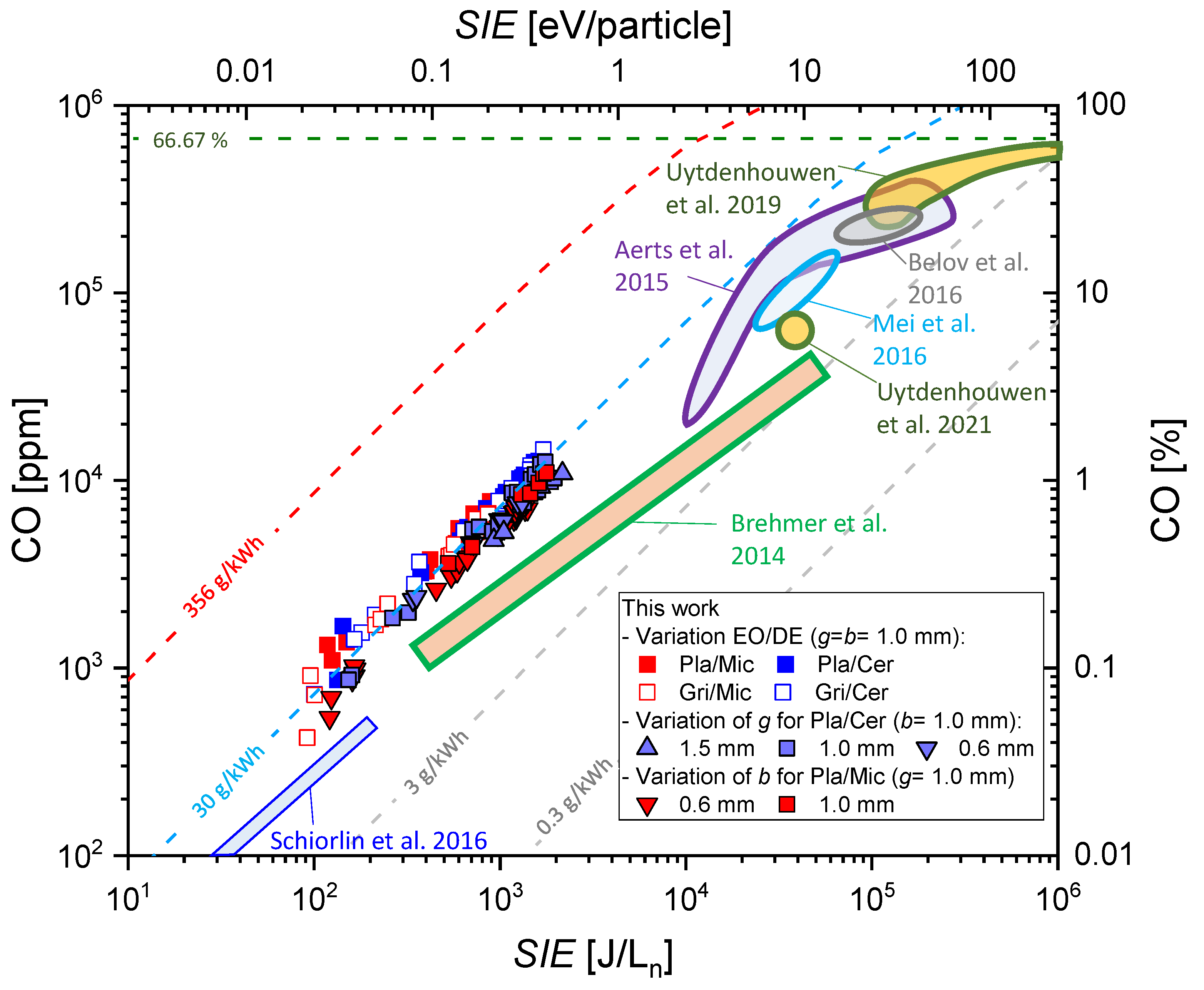
4. Carbon Monoxide Formation
5. Summary and Outlook
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| DBD | Dielectric barrier discharge |
| DE | Dielectric |
| Cer | Ceramic |
| EC | Energy cost |
| EE | Energy efficiency |
| EMC | Electromagnetic compatibility |
| EO | Electrode |
| EY | Energy yield |
| Gri | Grid |
| LCR | Inductance (L), capacitance (C), and resistance (R) |
| Mic | Mica |
| Pla | Plate |
| PSD | Power surface density |
| SIE | Specific input energy |
References
- Becker, K.H.; Kogelschatz, U.; Schoenbach, K.H.; Barker, R.J. Non-Equilibrium Air Plasmas at Atmospheric Pressure; CRC Press: Boca Raton, FL, USA, 2005. [Google Scholar]
- Kogelschatz, U. Dielectric-barrier discharges: Their history, discharge physics, and industrial applications. Plasma Chem. Plasma Proc. 2003, 23, 1–46. [Google Scholar] [CrossRef]
- Laroussi, M.; Kong, M.G.; Morfill, G.; Stolz, W. Plasma Medicine—Applications of Low-Temperature Gas Plasmas in Medicine and Biology; Cambridge University Press: Cambridge, UK, 2012. [Google Scholar]
- Massines, F.; Sarra-Bournet, S.; Fanelli, F.; Naude, N.; Gherardi, N. Atmospheric Pressure Low Temperature Direct Plasma Technology: Status and Challenges for Thin Film Deposition. Plasma Procss. Polym. 2012, 9, 1041–1073. [Google Scholar] [CrossRef]
- Moreau, E. Airflow control by non-thermal plasma actuators. J. Phys. D Appl. Phys. 2007, 40, 605. [Google Scholar] [CrossRef]
- Meyer, C.; Müller, S.; Gurevich, E.L.; Franzke, J. Dielectric barrier discharges in analytical chemistry. Analyst 2011, 136, 2427. [Google Scholar] [CrossRef] [PubMed]
- Bill, A.; Wokaun, A.; Eliasson, B.; Killer, E.; Kogelschatz, U. Greenhouse gas chemistry. Energ. Convers. Manag. 1997, 38, S415–S422. [Google Scholar] [CrossRef]
- Bill, A.; Eliasson, B.; Kogelschatz, U.; Zhou, L.M. Comparison of CO2 hydrogenation in a catalytic reactor and in a dielectric-barrier discharge. Stud. Surf. Sci. Catal. 1998, 114, 541–544. [Google Scholar]
- Eliasson, B.; Kogelschatz, U.; Xue, B.Z.; Zhou, L.M. Hydrogenation of carbon dioxide to methanol with a discharge-activated catalyst. Ind. Eng. Chem. Res. 1998, 37, 3350–3357. [Google Scholar] [CrossRef]
- Kraus, M.; Egli, W.; Haffner, K.; Eliasson, B.; Kogelschatz, U.; Wokaun, A. Investigation of mechanistic aspects of the catalytic CO2 reforming of methane in a dielectric-barrier discharge using optical emission spectroscopy and kinetic modeling. Phys. Chem. Chem. Phys. 2002, 4, 668–675. [Google Scholar] [CrossRef]
- Bogaerts, A.; Centi, G. Plasma Technology for CO2 Conversion: A Personal Perspective on Prospects and Gaps. Front. Energy Res. 2020, 8, 111. [Google Scholar] [CrossRef]
- Brandenburg, R.; Bogaerts, A.; Bongers, W.; Fridman, A.; Fridman, G.; Locke, B.R.; Miller, V.; Reuter, S.; Schiorlin, M.; Verreycken, T.; et al. White paper on the future of plasma science in environment, for gas conversion and agriculture. Plasma Process Polym. 2019, 16, e1700238. [Google Scholar] [CrossRef]
- Paulussen, S.; Verheyde, B.; Tu, X.; De Bie, C.; Martens, T.; Petrovic, D.; Bogaerts, A.; Sels, B. Conversion of carbon dioxide to value-added chemicals in atmospheric pressure dielectric barrier discharges. Plasma Sources Sci. Technol. 2010, 19, 034015. [Google Scholar] [CrossRef]
- Aerts, R.; Somers, W.; Bogaerts, A. Carbon Dioxide Splitting in a Dielectric Barrier Discharge Plasma: A Combined Experimental and Computational Study. ChemSusChem 2015, 8, 702–716. [Google Scholar] [CrossRef] [PubMed]
- Aerts, R.; Martens, T.; Bogaerts, A. Influence of Vibrational States on CO2 Splitting by Dielectric Barrier Discharges. J. Phys. Chem. C 2012, 116, 23257–23273. [Google Scholar] [CrossRef]
- Mei, D. Plasma-Catalytic Conversion of Greenhouse Gas into Value-Added Fuels and Chemicals. Ph.D. Thesis, University of Liverpool, Liverpool, UK, 2016. [Google Scholar]
- Mei, D.; He, A.-L.; Liu, S.; Yan, J.; Tu, X. Optimization of CO2 conversion in a Cylindrical Dielectric Barrier Discharge Reactor Using Design of Experiments. Plasma Process Polym. 2016, 13, 544–556. [Google Scholar] [CrossRef]
- Niu, G.; Qin, Y.; Li, W.; Duan, Y. Investigation of CO2 Splitting Process Under Atmospheric Pressure Using Multi-electrode Cylindrical DBD Plasma Reactor. Plasma Chem. Plasma Proc. 2019, 39, 809–824. [Google Scholar] [CrossRef]
- Ozkan, A.; Dufour, T.; Bogaerts, A.; Reniers, F. How do the barrier thickness and dielectric material influence the filamentary mode and CO2 conversion in a flowing DBD? Plasma Sources Sci. Technol. 2016, 25, 045016. [Google Scholar] [CrossRef]
- Chen, P.; Shen, Y.; Ran, T.; Yang, T.; Yin, Y. Investigation of operating parameters on CO2 splitting by dielectric barrier discharge plasma. Plasma Sci. Technol. 2017, 19, 125505. [Google Scholar] [CrossRef]
- Belov, I.; Paulussen, S.; Bogaerts, A. Appearance of a conductive carbonaceous coating in a CO2 dielectric barrier discharge and its influence on the electrical properties and the conversion efficiency. Plasma Sources Sci. Technol. 2016, 25, 015023. [Google Scholar] [CrossRef]
- Belov, I.; Vanneste, J.; Aghaee, M.; Paulussen, S.; Bogaerts, A. Synthesis of Micro- and Nanomaterials in CO2 and CO Dielectric Barrier Discharges. Plasma Process Polym. 2017, 14, 1600065. [Google Scholar] [CrossRef]
- Uytdenhouwen, Y.; Van Alphen, S.; Michielsen, I.; Meynen, V.; Cool, P.; Bogaerts, A. A packed-bed DBD micro plasma reactor for CO2 dissociation: Does size matter? Chem. Eng. J. 2018, 348, 557–568. [Google Scholar] [CrossRef]
- Uytdenhouwen, Y.; Bal, K.M.; Michielsen, I.; Neyts, E.C.; Meynen, V.; Cool, P.; Bogaerts, A. How process parameters and packing materials tune chemical equilibrium and kinetics in plasma-based CO2 conversion. Chem. Eng. J. 2019, 372, 1253–1264. [Google Scholar] [CrossRef]
- Müller, S.; Zahn, R.-J. Air Pollution Control by Non-Thermal Plasma. Contrib. Plasma Phys. 2007, 47, 520–529. [Google Scholar] [CrossRef]
- Brandenburg, R.; Kovacevic, V.V.; Schmidt, M.; Basner, R.; Kettlitz, M.; Sretenovic, G.B.; Obradovic, B.M.; Kuraica, M.M.; Weltmann, K.-D. Plasma-Based Pollutant Degradation in Gas Streams: Status, Examples and Outlook. Contrib. Plasma Phys. 2014, 54, 202–214. [Google Scholar] [CrossRef]
- Rafflenbeul, R. NTP-Anlagen zur Luftreinhaltung in der Abfallwirtschaft. Müll Abfall 1998, 30, 1, 38–44. [Google Scholar]
- Rafflenbeul, L.; Rafflenbeul, R. Treatment of Problematic Exhaust Air Escort Substances. Chem. Ing. Tech. 2022, 94, 471–478. [Google Scholar] [CrossRef]
- Brehmer, F.; Welzel, S.; van de Sanden, M.C.M.; Engeln, R. CO and byproduct formation during CO2 reduction in dielectric barrier discharges. J. Appl. Phys. 2014, 116, 123303. [Google Scholar] [CrossRef]
- Ponduri, S.; Becker, M.M.; Welzel, S.; van de Sanden, M.C.M.; Loffhagen, D.; Engeln, R. Fluid modelling of CO2 dissociation in a dielectric barrier discharge. J. Appl. Phys. 2016, 119, 093301. [Google Scholar] [CrossRef]
- Uytdenhouwen, Y.; Hereijgers, J.; Breugelmans, T.; Cool, P.; Bogaerts, A. How gas flow design can influence the performance of a DBD plasma reactor for dry reforming of methane. Chem. Eng. J. 2021, 405, 126618. [Google Scholar] [CrossRef]
- Peeters, F.J.J.; van de Sanden, M.C.M. The influence of partial surface discharging on the electrical characterization of DBDs. Plasma Sources Sci. Technol. 2015, 24, 015016. [Google Scholar] [CrossRef]
- Pipa, A.V.; Koskulics, J.; Brandenburg, R.; Hoder, T. The simplest equivalent circuit of a pulsed dielectric barrier discharge and the determination of the gas gap charge transfer. Rev. Sci. Instrum. 2012, 83, 115112. [Google Scholar] [CrossRef]
- Manley, T.C. The electric characteristics of the ozonator discharge. Trans. Electrochem. Soc. 1943, 84, 83–96. [Google Scholar] [CrossRef]
- Schmidt, M.; Basner, R. Brandenburg, R. Hydrocarbon Assisted NO Oxidation with Non-thermal Plasma in Simulated Marine Diesel Exhaust Gases. Plasma Chem. Plasma Proc. 2013, 33, 323–335. [Google Scholar] [CrossRef]
- Schmidt, M.; Schiorlin, M.; Brandenburg, R. Studies on the Electrical Behaviour and Removal of Toluene with a Dielectric Barrier Discharge. Open Chem. 2015, 13, 477–483. [Google Scholar] [CrossRef]
- Kogelschatz, U. Advanced Ozone Generation. In Process Technologies for Water Treatment; Stucki, S., Ed.; Springer: New York, NY, USA, 1988; pp. 87–118. [Google Scholar]
- Pipa, A.V.; Brandenburg, R. The equivalent circuit approach for the electrical diagnostics of dielectric barrier discharges: The classical theory and recent developments. Atoms 2019, 7, 14. [Google Scholar] [CrossRef]
- Reichen, P.; Sonnenfeld, A.; von Rohr, P.R. Discharge expansion in barrier discharge arrangements at low applied voltages. Plasma Sources Sci. Technol. 2011, 20, 055015. [Google Scholar] [CrossRef]
- Brandenburg, R. Dielectric barrier discharges: Progress on plasma sources and on the understanding of regimes and single filaments. Plasma Sources Sci. Technol. 2017, 26, 053001. [Google Scholar] [CrossRef]
- Hosseini Rad, R.; Brüser, V.; Schiorlin, M.; Schäfer, J.; Brandenburg, R. Enhancement of CO2 splitting in a coaxial dielectric barrier discharge by pressure increase, packed bed and catalyst addition. Chem. Eng. J. 2023, 456, 141072. [Google Scholar] [CrossRef]
- Cui, Z.; Liu, Q.; Cai, Z.; Wang, J.; Wu, Q. Modified equivalent circuit for coplanar barrier discharge considering undischarged areas. Phys. Plas 2023, 30, 013502. [Google Scholar] [CrossRef]
- Pipa, A.V.; Hink, R.; Foest, R.; Brandenburg, R. Dependence of dissipated power on applied voltage for surface barrier discharge from simplest equivalent circuit. Plasma Sources Sci. Technol. 2020, 29, 12LT01. [Google Scholar] [CrossRef]
- Falkenstein, Z.; Coogan, J.J. Microdischarge behaviour in the silent discharge of nitrogen—oxygen and water—air mixtures. J. Phys. D Appl. Phys. 1999, 30, 817–825. [Google Scholar] [CrossRef]
- Lindner, M.; Pipa, A.V.; Brandenburg, R.; Schreiner, R. Expansion of surface barrier discharge scrutinized. Plasma Sources Sci. Technol. 2022, 31, 105018. [Google Scholar] [CrossRef]
- Gibalov, V.I.; Pietsch, G.J. The development of dielectric barrier discharges in gas gaps and on surfaces. J. Phys. D Appl. Phys. 2000, 33, 2618–2636. [Google Scholar] [CrossRef]
- Höft, H.; Becker, M.M.; Kettlitz, M.; Brandenburg, R. Upscaling from single- to multi-filament dielectric barrier discharges in pulsed operation. J. Phys. D Appl. Phys. 2022, 55, 424003. [Google Scholar] [CrossRef]
- Schiorlin, M.; Klink, R.; Brandenburg, R. Carbon dioxide conversion by means of coplanar dielectric barrier discharges. Eur. Phys. J. Appl. Phys. 2016, 75, 24704. [Google Scholar] [CrossRef]
- Hoder, T.; Šíra, M.; Kozlov, K.V.; Wagner, H.-E. 3D Imaging of the Single Microdischarge Development in Coplanar Barrier Discharges in Synthetic Air at Atmospheric Pressure. Contrib. Plas. Phys. 2009, 49, 381–387. [Google Scholar] [CrossRef]
- Brehmer, F.; Welzel, S.; Klarenaar, B.L.M.; van der Meiden, H.J.; van de Sanden, M.C.M.; Engeln, R. Gas temperature in transient CO2 plasma measured by Raman scattering. J. Phys. D Appl. Phys. 2015, 48, 155201. [Google Scholar] [CrossRef]
- Brandenburg, R.; Sarani, A. About the development of single microdischarges in dielectric barrier discharges in CO2 and CO2/N2 gas mixtures. Eur. Phys. J. Spec. Top. 2017, 226, 2911–2922. [Google Scholar] [CrossRef]
- Kulikovsky, A.A. Positive streamer between parallel plate electrodes in atmospheric pressure air. J. Phys. D Appl. Phys. 1997, 30, 441. [Google Scholar] [CrossRef]
- Nijdam, S.; Teunissen, J.; Ebert, U. The physics of streamer discharge phenomena. Plasma Sources Sci. Technol. 2020, 29, 103001. [Google Scholar] [CrossRef]
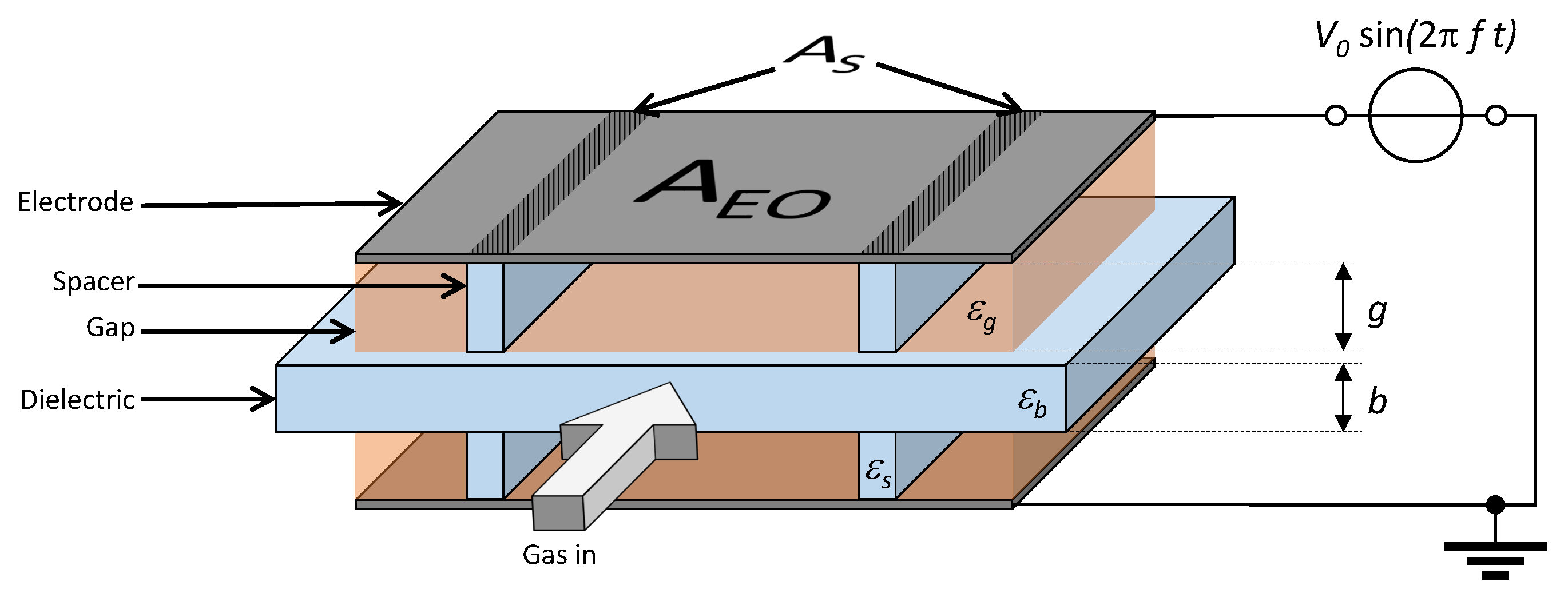






| Part | Material/Type | Parameters | Dimensions |
|---|---|---|---|
| Electrode | Stainless steel plate (Pla) | - | = 14 cm × 9 cm |
| (EO) | Stainless steel grid (Gri) | wire: 0.5 mm; mesh: 0.8 mm | = 14 cm × 9 cm × 0.75 |
| Dielectric | Mica (Phlogopite) | = 3.5 | b = 0.6, 1.0 mm |
| Plate (DE) | Ceramic (Al2O3; 96%) | = 9 | b = 1.0 mm |
| Spacer | Mica (Phlogopite) | = 3.5 | = n × 14 cm × 0.3 cm |
| (number n) | g = 0.6, 1.0, 1.5 mm | ||
| Ceramic (Al2O3; 96%) | = 9 | = n × 14 cm × 0.3 cm | |
| g = 1.0 mm | |||
| Gases (Purity) | CO2 (N4.5) | = 1.0009 | = 60 Ln/h |
| synth. Air (N5.0) | = 1.0006 | = 60 Ln/h | |
| Voltage | sinusoidal | = 13–22 kV | |
| sin() | f = 400 Hz |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Brandenburg, R.; Schiorlin, M.; Schmidt, M.; Höft, H.; Pipa, A.V.; Brüser, V. Plane Parallel Barrier Discharges for Carbon Dioxide Splitting: Influence of Discharge Arrangement on Carbon Monoxide Formation. Plasma 2023, 6, 162-180. https://doi.org/10.3390/plasma6010013
Brandenburg R, Schiorlin M, Schmidt M, Höft H, Pipa AV, Brüser V. Plane Parallel Barrier Discharges for Carbon Dioxide Splitting: Influence of Discharge Arrangement on Carbon Monoxide Formation. Plasma. 2023; 6(1):162-180. https://doi.org/10.3390/plasma6010013
Chicago/Turabian StyleBrandenburg, Ronny, Milko Schiorlin, Michael Schmidt, Hans Höft, Andrei V. Pipa, and Volker Brüser. 2023. "Plane Parallel Barrier Discharges for Carbon Dioxide Splitting: Influence of Discharge Arrangement on Carbon Monoxide Formation" Plasma 6, no. 1: 162-180. https://doi.org/10.3390/plasma6010013
APA StyleBrandenburg, R., Schiorlin, M., Schmidt, M., Höft, H., Pipa, A. V., & Brüser, V. (2023). Plane Parallel Barrier Discharges for Carbon Dioxide Splitting: Influence of Discharge Arrangement on Carbon Monoxide Formation. Plasma, 6(1), 162-180. https://doi.org/10.3390/plasma6010013





