Exploring Enhanced Structural and Dielectric Properties in Ag-Doped Sr(NiNb)0.5O3 Perovskite Ceramic for Advanced Energy Storage
Abstract
1. Introduction
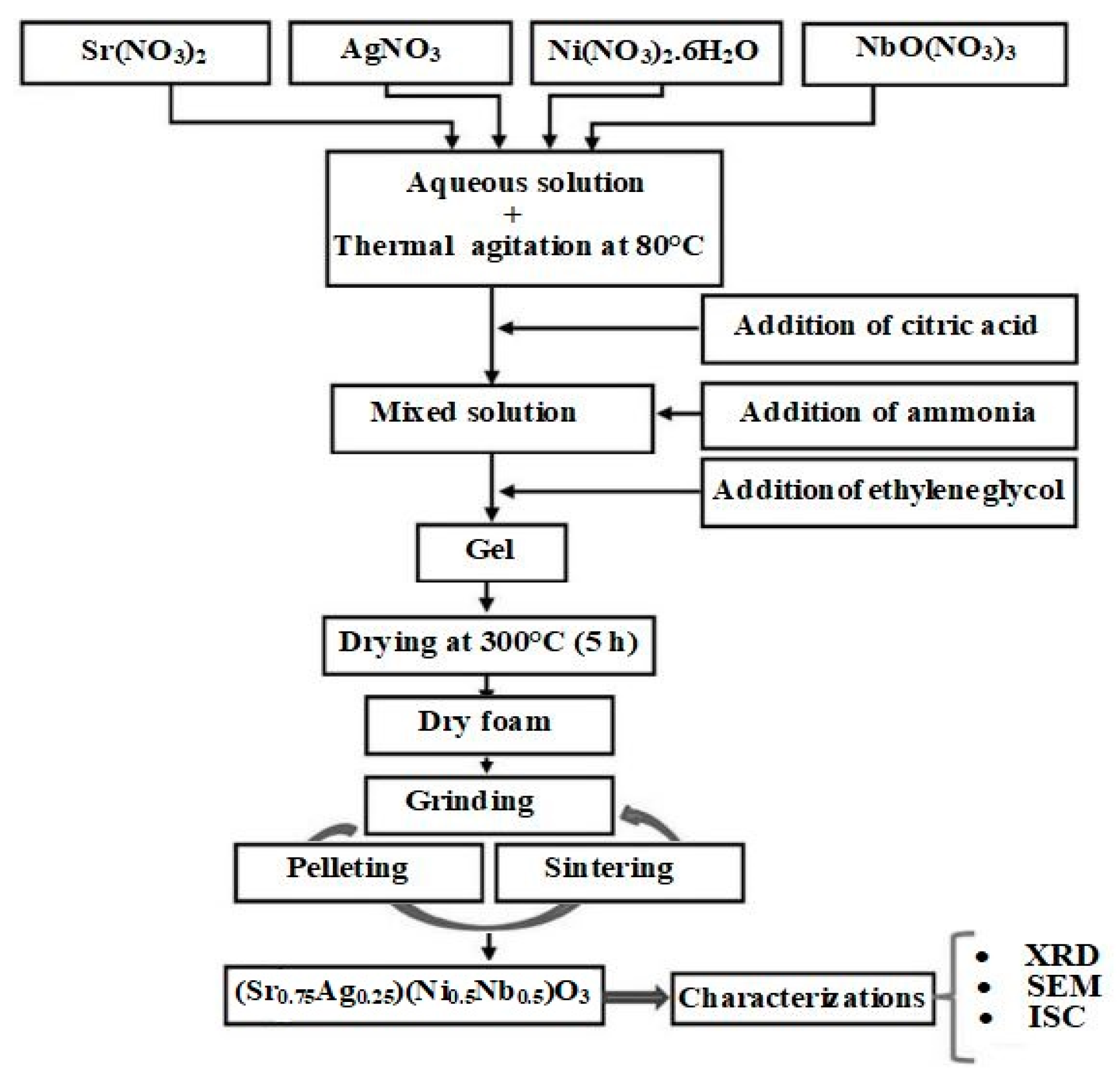
2. Materials and Methods
3. Results and Discussion
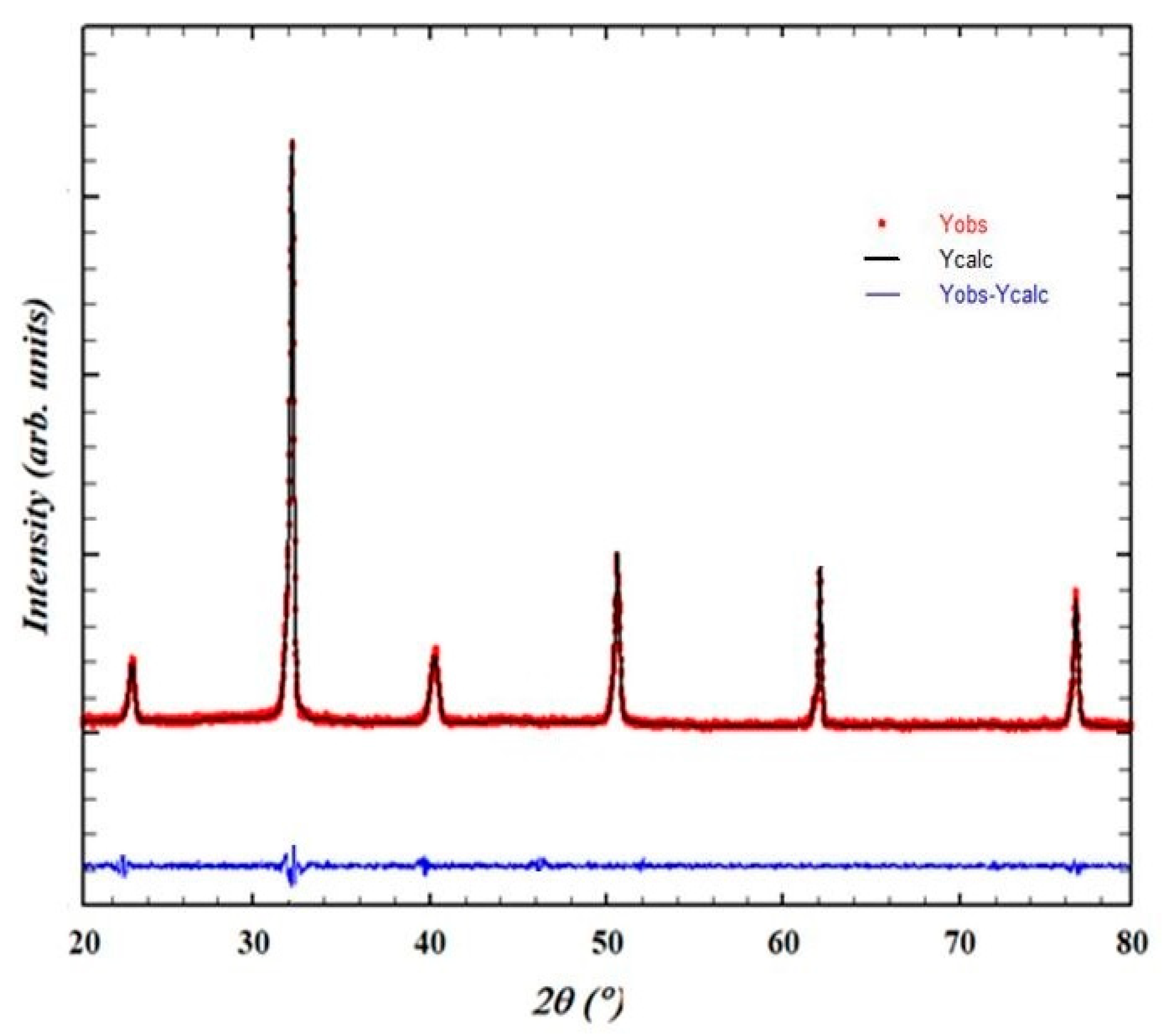
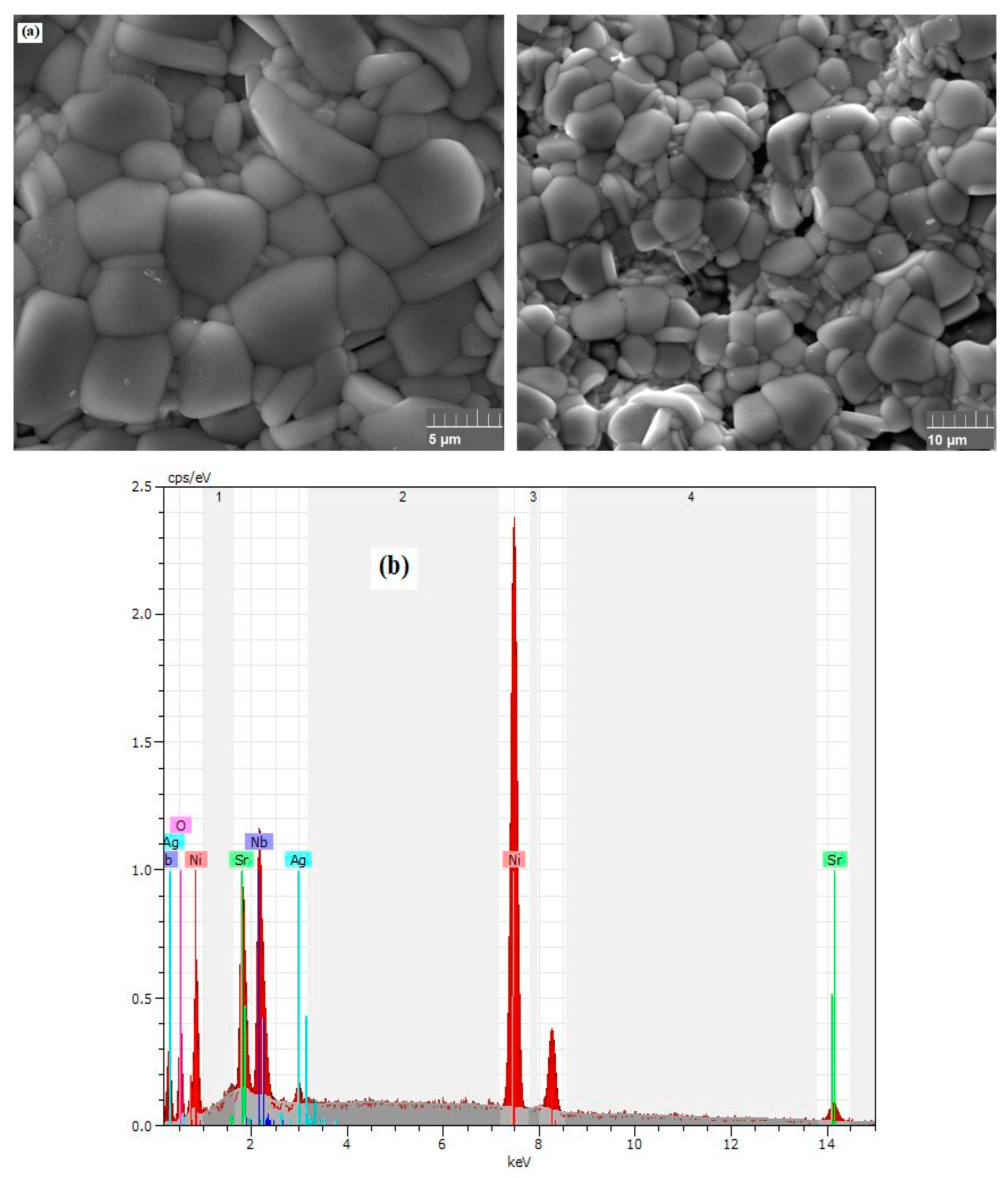
3.1. Phase Structure and Microstructure
3.2. Impact of Frequency on Dielectric Parameters
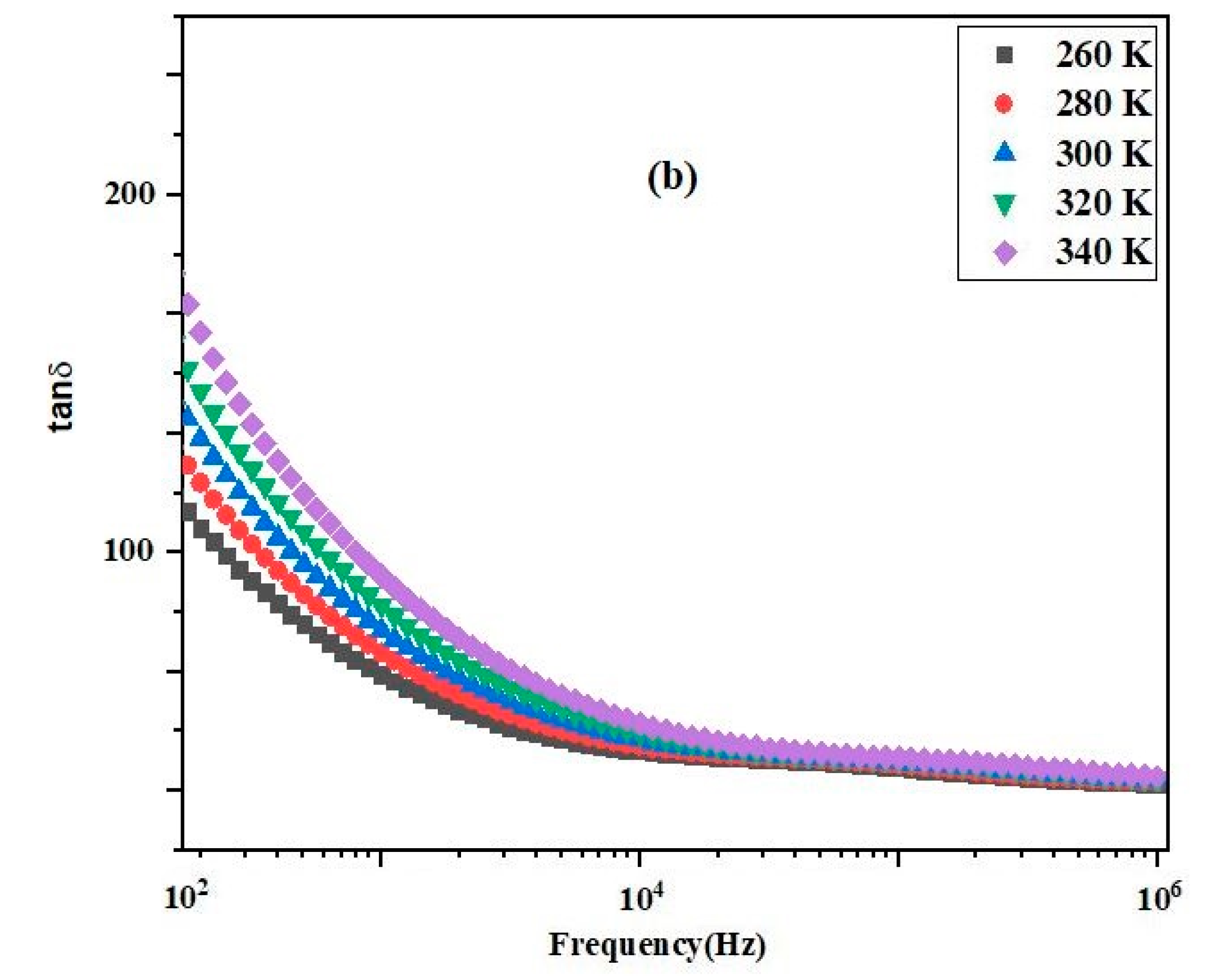
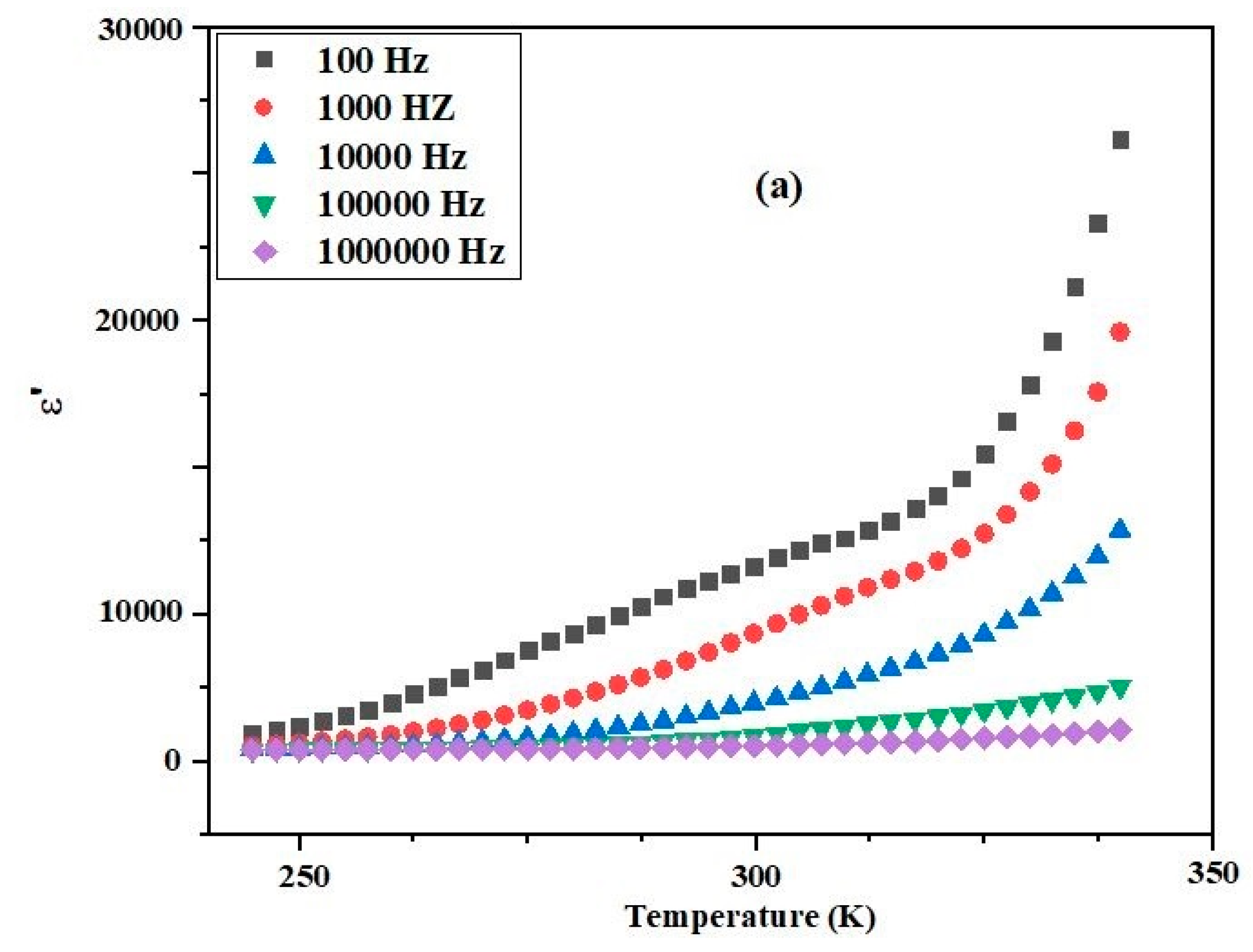
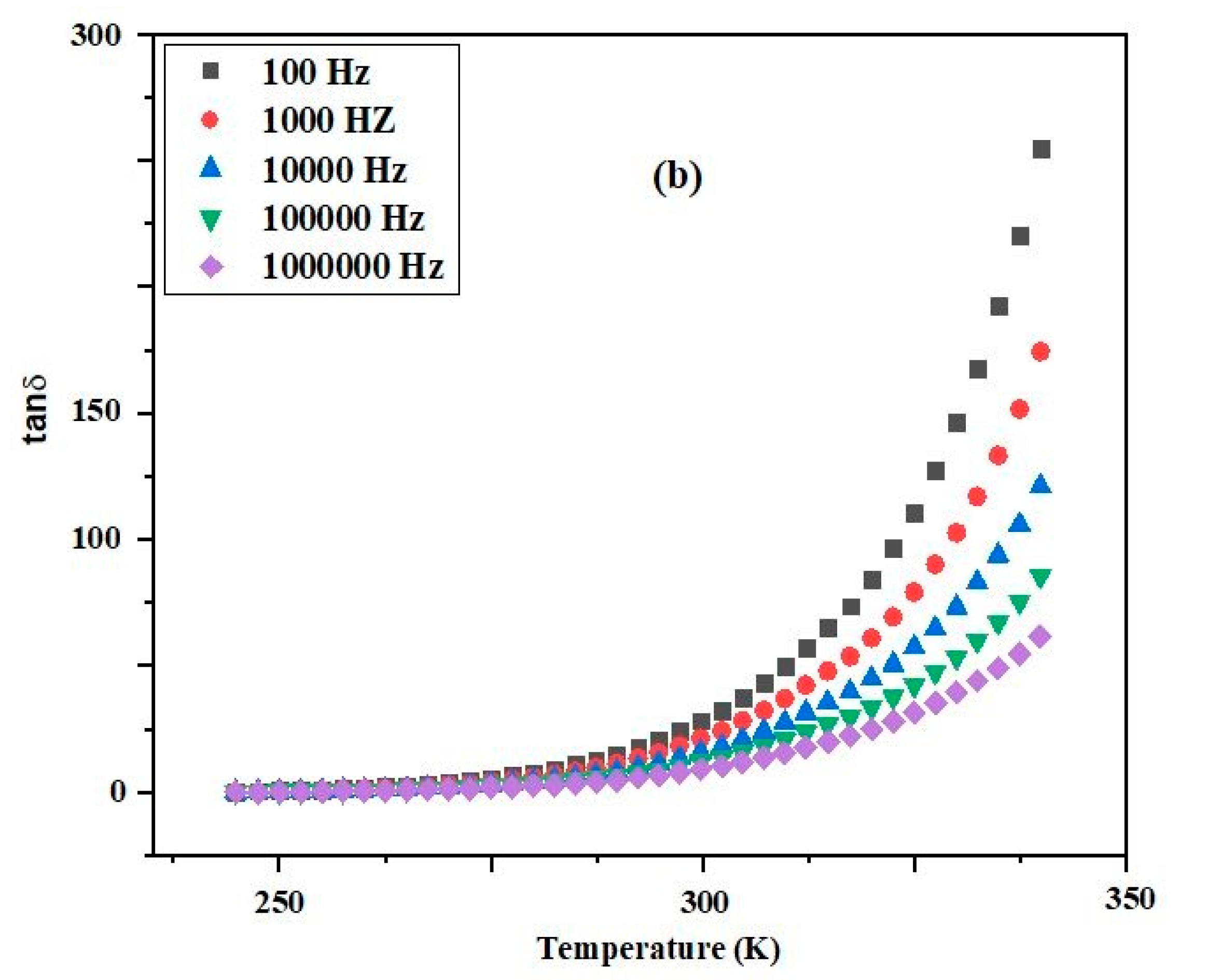
3.3. Variation of Dielectric Properties with Temperature
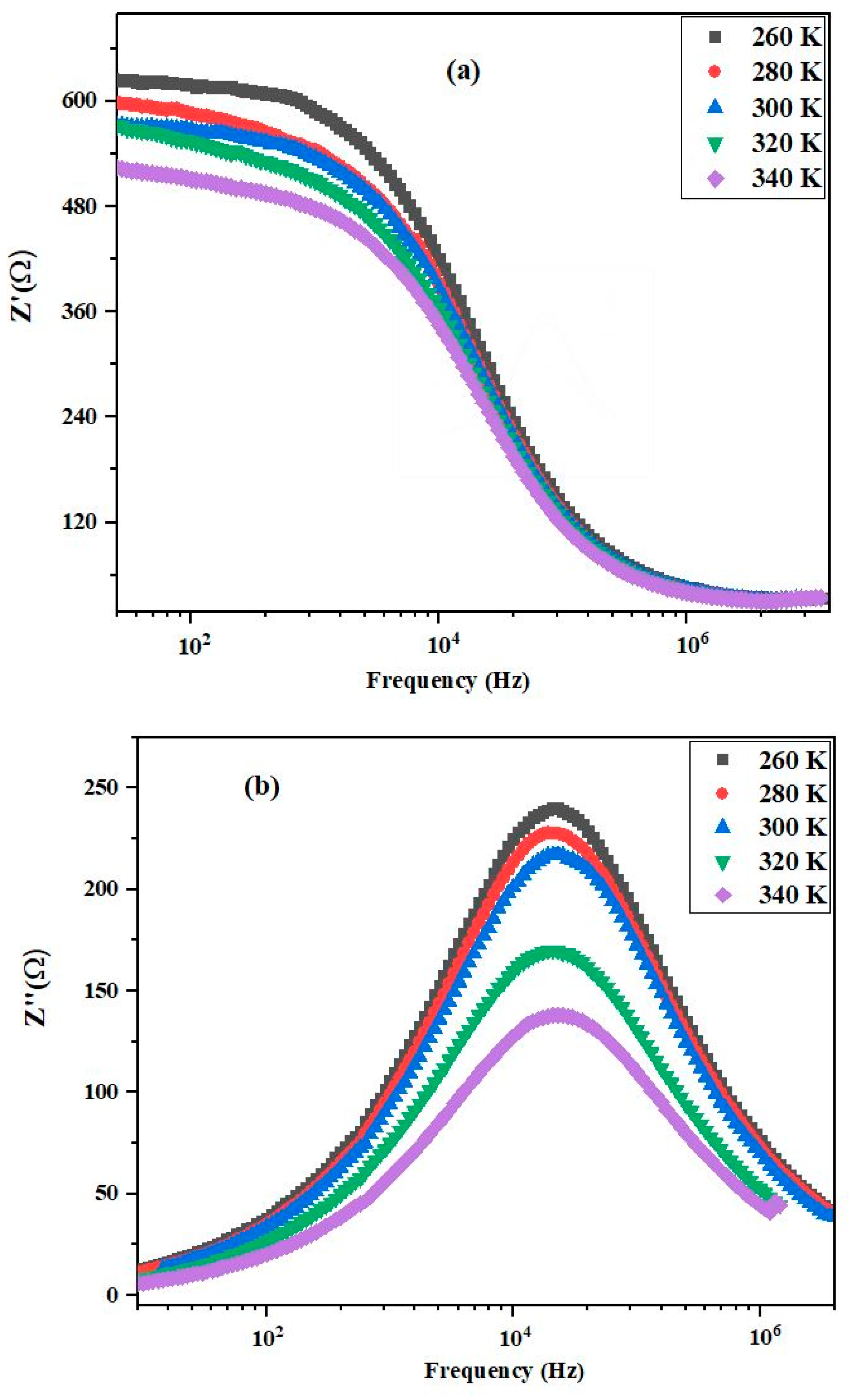
3.4. Impedance Spectroscopy
3.5. Nyquist Diagram
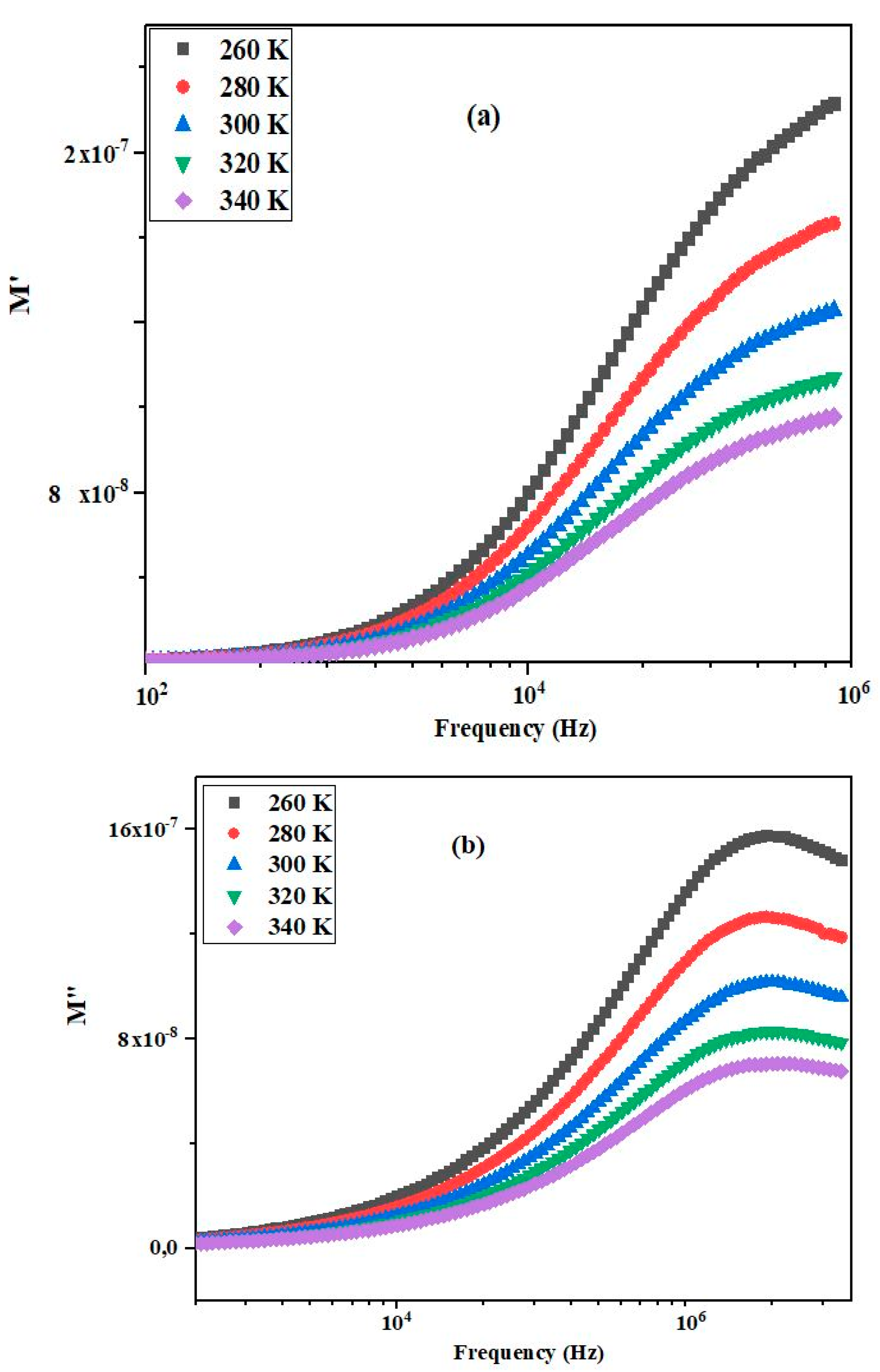
3.6. Modulus Analysis
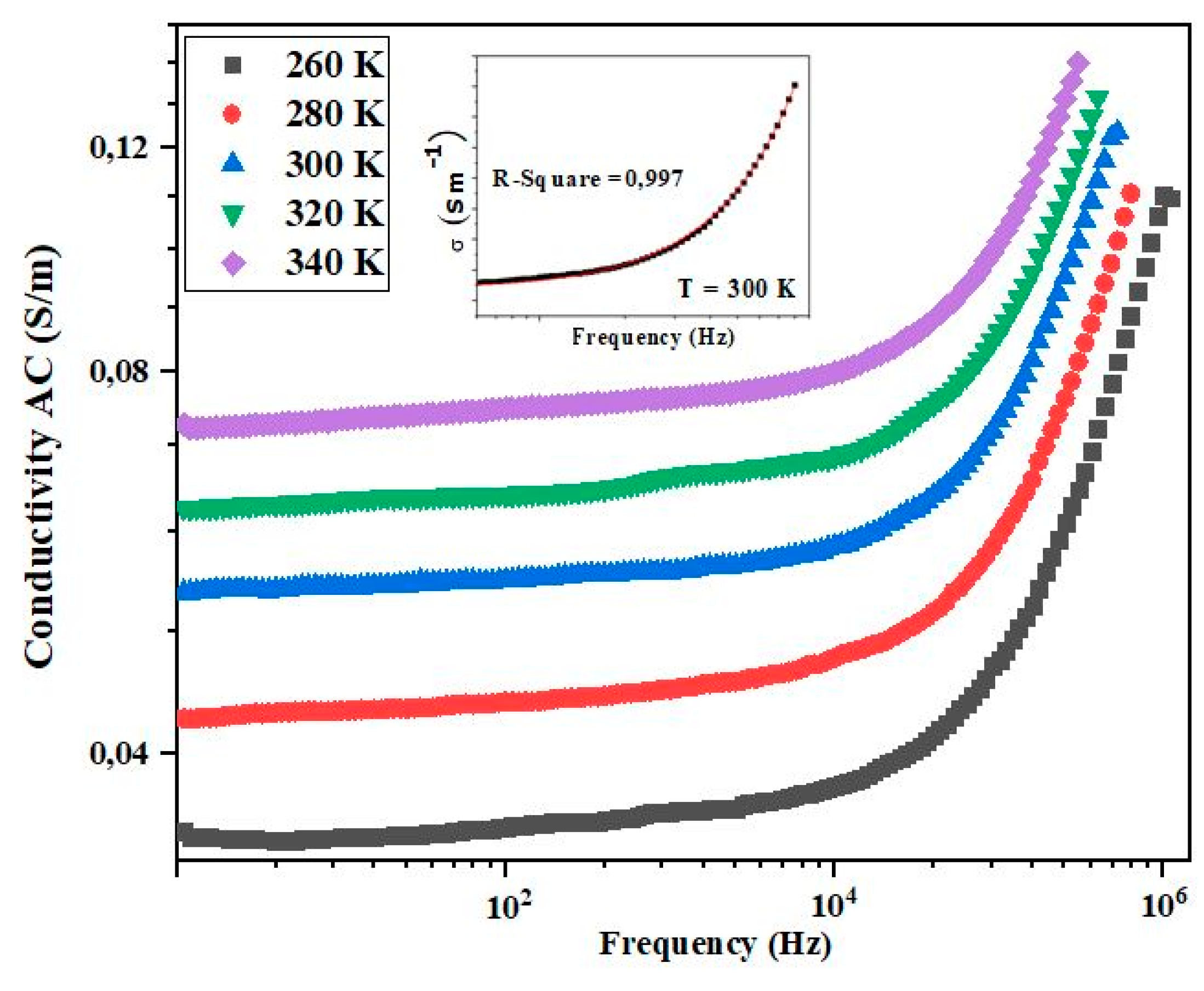
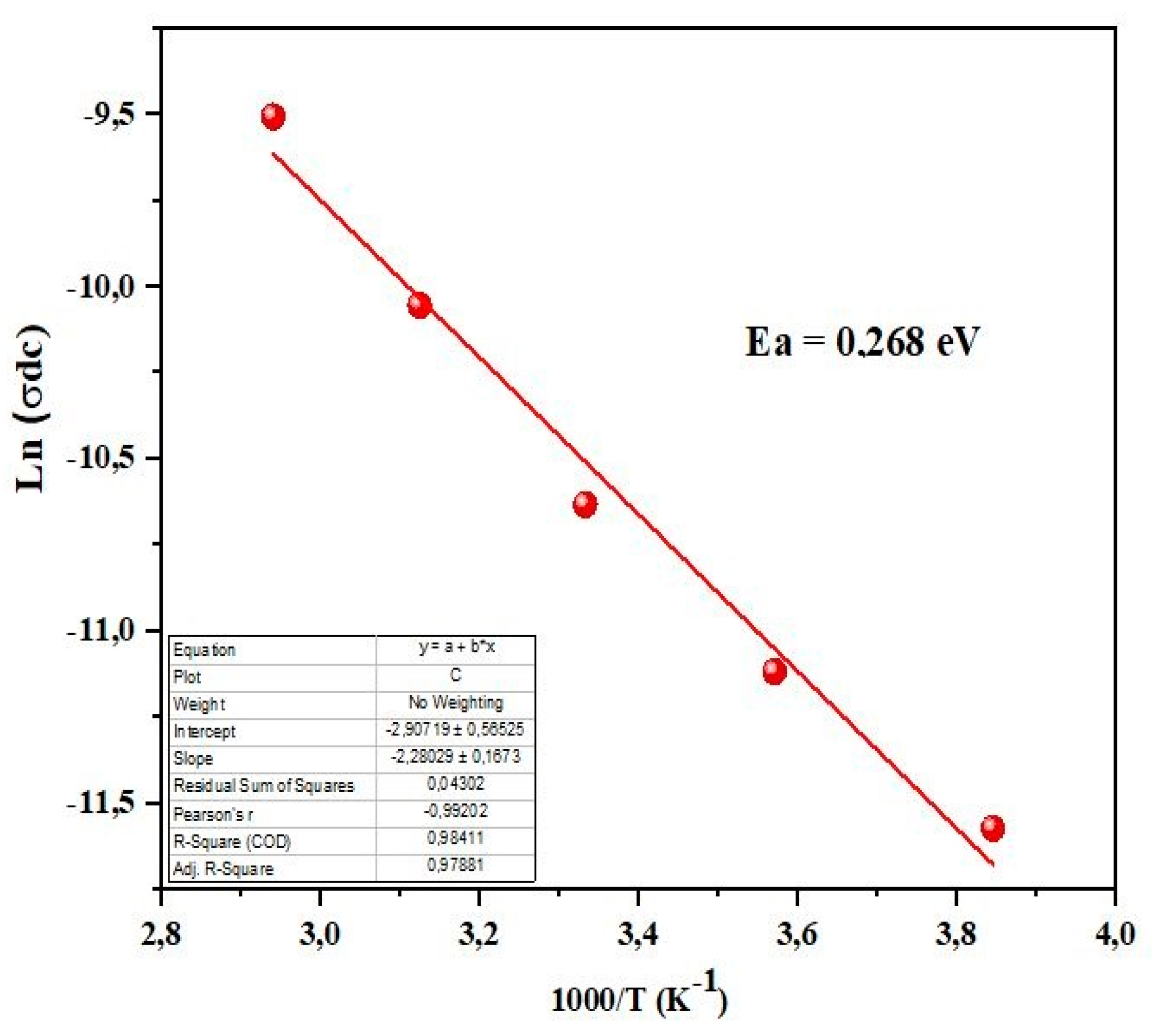
3.7. Electrical Conductivity Study
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Davies, P.K.; Roth, R.S. National Institute of Standards and Technology Special Publication 804; NIST: Gaithersburg, MD, USA, 1991.
- Lines, M.E.; Glass, A.M. Principles and Applications of Ferroelectrics and Related Materials; Oxford University Press: New York, NY, USA, 1997. [Google Scholar]
- Tang, C.C.; Roberts, M.A.; Azough, F.; Leach, C.; Freer, R. Synchrotron x-ray diffraction study of Ba4.5Nd9Ti18O54 microwave dielectric ceramics at 10–295 K. J. Mater. Res. 2022, 17, 675–682. [Google Scholar] [CrossRef]
- Colla, E.L.; Reaney, I.M.; Setter, N.J. Effect of structural changes in complex perovskites on the temperature coefficient of the relative permittivity. Appl. Phys. 1993, 74, 3414–3425. [Google Scholar] [CrossRef]
- Hanif, M.B.; Rauf, S.; Motola, M.; Babar, Z.U.; Li, C.J.; Li, C.X. Recent progress of perovskite-based electrolyte materials for solid oxide fuel cells and performance optimizing strategies for energy storage applications. Mater. Res. Bull. 2022, 146, 111612. [Google Scholar] [CrossRef]
- Hanif, M.B.; Motola, M.; Rauf, S.; Li, C.J.; Li, C.X. Recent advancements, doping strategies and the future perspective of perovskite-based solid oxide fuel cells for energy conversion. Chem. Eng. J. 2022, 428, 132603. [Google Scholar] [CrossRef]
- Kubicek, M.; Bork, A.H.; Rupp, J.L.M. Perovskite oxides—A review on a versatile material class for solar-to-fuel conversion processes. J. Mater. Chem. A 2017, 5, 11983–12000. [Google Scholar] [CrossRef]
- Ofoegbuna, T.; Darapaneni, P.; Sahu, S.; Plaisance, C.; Dorman, J.A. Stabilizing the B-site oxidation state in ABO3 perovskite nanoparticles. Nanoscale 2019, 11, 14303–14311. [Google Scholar] [CrossRef]
- Oka, D.; Hirose, Y.; Nakao, S.; Fukumura, T.; Hasegawa, T. Intrinsic high electrical conductivity of stoichiometric SrNbO3 epitaxial thin films. Phys. Rev. B 2015, 92, 205102. [Google Scholar] [CrossRef]
- Hossain, A.; Bandyopadhyay, P.; Roy, S. An overview of double perovskites A2B′B″O6 with small ions at A site: Synthesis, structure and magnetic properties. J. Alloys Compd. 2018, 740, 414–427. [Google Scholar] [CrossRef]
- Velinov, N.; Brashkova, N.; Kozhukharov, V. Synthesis, structure and conductivity of layered perovskites. Ceram.-Silik. 2005, 49, 29–33. [Google Scholar]
- Arjun, N.; Pan, G.-T.; Yang, T.C.K. The exploration of Lanthanum based perovskites and their complementary electrolytes for the supercapacitor applications. Results Phys. 2017, 7, 920–926. [Google Scholar] [CrossRef]
- Tran, M.-N.T.; Quach, H.Y.; Nguyen, Q.V.; Nguyen, T.D.; On, D.T. Synthesis of perovskite-based nanocomposites for deNOx catalytic activity. Can. J. Chem. 2016, 94, 215–220. [Google Scholar] [CrossRef]
- Li, X.; Zhu, S.; Jia, Q.; Zhao, H.; Cao, Y.; Ma, Y.; Hu, S.; Cao, X. Fast synthesis of MoO3-x and its catalytic effect on the thermal decomposition of ammonium perchlorate based molecular perovskite (DAP-4). Can. J. Chem. 2021, 99, 795–800. [Google Scholar] [CrossRef]
- Gómez, L.; Galeano, V.; Parra, R.; Michel, C.R.; Paucar, C.; Morán, O. Carbon dioxide gas sensing properties of ordered oxygen deficient perovskite LnBaCo2O5+δ (Ln = La, Eu). Sens. Actuators B Chem. 2015, 221, 1455–1460. [Google Scholar] [CrossRef]
- Polini, R.; Pamio, A.; Traversa, E. Effect of synthetic route on sintering behaviour, phase purity and conductivity of Sr- and Mg-doped LaGaO3 perovskites. J. Eur. Ceram. Soc. 2004, 24, 1365–1370. [Google Scholar] [CrossRef][Green Version]
- Fumo, D.A.; Jurado, J.R.; Segadães, A.M.; Frade, J.R. Combustion synthesis of iron-substituted strontium titanate perovskites. Mater. Res. Bull. 1997, 32, 1459–1470. [Google Scholar] [CrossRef]
- Alhokbany, N.; Almotairi, S.; Ahmed, J.; Al-Saeedi, S.I.; Ahamad, T.; Alshehri, S.M. Investigation of structural and electrical properties of synthesized Sr-doped lanthanum cobaltite (La1−xSrxCoO3) perovskite oxide. J. King Saud Univ.-Sci. 2021, 33, 101419. [Google Scholar] [CrossRef]
- Jayakumar, G.; Poomagal, D.S.; Irudayaraj, A.A.; Raj, A.D.; Thresa, S.K.; Akshadha, P. Study on structural, magnetic and electrical properties of perovskite lanthanum strontium manganite nanoparticles. J. Mater. Sci. Mater. Electron. 2020, 31, 20945–20953. [Google Scholar] [CrossRef]
- Intatha, U.; Eitssayeam, S.; Wang, J.; Tunkasiri, T. Impedance study of giant dielectric permittivity in BaFe0.5Nb0.5O3 perovskite ceramic. Curr. Appl. Phys. 2010, 10, 21–25. [Google Scholar] [CrossRef]
- Kodera, M.; Moriya, Y.; Katayama, M.; Hisatomi, T.; Minegishi, T.; Domen, K. Investigation on nitridation processes of Sr2Nb2O7 and SrNbO3 to SrNbO2N for photoelectrochemical water splitting. Sci. Rep. 2018, 8, 15849. [Google Scholar] [CrossRef]
- Bigi, C.; Orgiani, P.; Sławińska, J.; Fujii, J.; Irvine, J.T.; Picozzi, S.; Panaccione, G.; Vobornik, I.; Rossi, G.; Payne, D.; et al. Direct insight into the band structure of SrNbO3. Phys. Rev. Mater. 2020, 4, 025006. [Google Scholar] [CrossRef]
- Rietveld, H.M. A profile refinement method for nuclear and magnetic structures. J. Appl. Crystallogr. 1969, 2, 65. [Google Scholar] [CrossRef]
- Voorhoeve, R.J.H. Advanced Materials in Catalysis; Academic Press: Cambridge, MA, USA, 1977; p. 129. [Google Scholar]
- Khadhraoui, S.; Triki, A.; Hcini, S.; Zemni, S.; Oumezzine, M. Variable-range-hopping conduction and dielectric relaxation in Pr0.6Sr0.4Mn0.6Ti0.4O3±δ perovskite. J. Magn. Magn. Mater. 2014, 371, 69. [Google Scholar] [CrossRef]
- Hsini, M.; Hamdaoui, N.; Hcini, S.; Bouazizi, M.L.; Zemni, S.; Beji, L. Effect of iron doping at Mn-site on complex impedance spectroscopy properties of Nd0.67Ba0.33MnO3 perovskite. Phase Transit. 2018, 91, 316. [Google Scholar] [CrossRef]
- Benamara, M.; Nassar, K.I.; Soltani, S.; Kallekh, A.; Dhahri, R.; Dahman, H.; El Mir, L. Light-enhanced electrical behavior of a Au/Al-doped ZnO/p-Si/Al heterostructure: Insights from impedance and current–voltage analysis. RSC Adv. 2023, 13, 28632–28641. [Google Scholar] [CrossRef] [PubMed]
- Rosić, M.; Logar, M.; Devečerski, A.; Prekajski, M.; Radosavljević-Mihajlović, A.; Kusigerski, V.; Spasojević, V.; Matović, B. Synthesis, structural and magnetic properties of nanostructured Ca0.9Gd0.1MnO3 obtained by modified glycine nitrate procedure (MGNP). Ceram. Int. 2011, 37, 1313–1319. [Google Scholar] [CrossRef]
- Nassar, K.I.; Tayari, F.; Benamara, M.; Teixeira, S.S.; Graça, M.P. Exploring bismuth-doped polycrystalline ceramic Ba0.75Bi0.25Ni0.7Mn0.3O3: Synthesis, structure, and electrical properties for advanced electronic applications. RSC Adv. 2023, 13, 24023–24030. [Google Scholar] [CrossRef] [PubMed]
- Parida, S.K.; Choudhary, R.N.P.; Achary, P.G.R. Study of structural and electrical properties of polycrystalline Pb(Cd1/3Ti1/3W1/3)O3 tungsten perovskite. Int. J. Microstruct. Mater. Prop. 2020, 15, 107–121. [Google Scholar]
- Tayari, F.; Iben Nassar, K.; Maalem, M.B.; Teixeira, S.S.; Graça, M.P.F. Structural, morphology, Raman spectroscopy, magnetic and electrical proprieties of BaNi0.5Mn0.25Fe0.25O3 ce-ramic for electronic applications. Indian J. Phys. 2023, 97, 3545–3555. [Google Scholar] [CrossRef]
- Elliot, S.R. Ac conduction in amorphous chalcogenide and pnictide semiconductors. Adv. Phys. 1987, 36, 135–217. [Google Scholar] [CrossRef]
- Gudmundsson, T.; Svavarsson, H.G.; Gudjonsson, S.; Gislason, H.P. Frequency-dependent conductivity in lithium-diffused and annealed GaAs. J. Phys. B 2003, 340, 324–328. [Google Scholar] [CrossRef]
- Benamara, M.; Iben Nassar, K.; Rivero-Antúnez, P.; Essid, M.; Soreto Teixeira, S.; Zhao, S.; Serrà, A.; Esquivias, L. Study of Electrical and Dielectric Behaviors of Copper-Doped Zinc Oxide Ceramic Prepared by Spark Plasma Sintering for Electronic Device Applications. Nanomaterials 2024, 14, 402. [Google Scholar] [CrossRef] [PubMed]
- Hcini, S.; Khadhraoui, S.; Triki, A.; Zemni, S.; Boudard, M.; Oumezzine, M. Impedance Spectroscopy Properties of Pr0.67A0.33MnO3 (A = Ba or Sr) Perovskites. J. Supercond. Nov. Magn. 2014, 27, 195. [Google Scholar] [CrossRef]
- Padmasree, K.P.; Kanchan, D.D.; Kulkami, A.R. Impedance and Modulus studies of the solid electrolyte system 20CdI2–80[xAg2O − y (0.7 V2O5 − 0.3 B2O3)], where 1≤ x/y ≤ 3. Solid State Ion. 2006, 177, 475. [Google Scholar] [CrossRef]
- Mahato, D.K.; Dutta, A.; Sinha, T.P. Impedance spectroscopy analysis of double perovskite Ho2NiTiO6. J. Mater. Sci. 2010, 45, 6757–6762. [Google Scholar] [CrossRef]
- Datta, R.; Pradhan, S.K.; Majumdar, S.; De, S.K. Dielectric and impedance spectroscopy of Nd2CoIrO6 double perovskite. J. Phys. Condens. Matter 2020, 32, 495702. [Google Scholar]
- Von Hauff, E.; Klotz, D. Impedance spectroscopy for perovskite solar cells: Characterisation, analysis, and diagnosis. J. Mater. Chem. C 2022, 10, 742–761. [Google Scholar] [CrossRef]
- Khadhraoui, S.; Triki, A.; Hcini, S.; Zemni, S.; Oumezzine, M. Structural and impedance spectroscopy properties of Pr0.6Sr0.4Mn1−xTixO3±δ perovskites. J. Alloys Compd. 2013, 574, 290–298. [Google Scholar] [CrossRef]
- Zarazua, I.; Sidhik, S.; Lopez-Luke, T.; Esparza, D.; De la Rosa, E.; Reyes-Gomez, J.; Garcia-Belmonte, I.M.-S. Operating Mechanisms of Mesoscopic Perovskite Solar Cells through Impedance Spectroscopy and J–V Modeling. J. Phys. Chem. Lett. 2017, 8, 6073–6079. [Google Scholar] [CrossRef] [PubMed]
- Cordoba-Torres, P.; Mesquita, T.J.; Devos, O.; Tribollet, B.; Roche, V.; Nogueira, R.P. On the intrinsic coupling between constant-phase element parameters α and Q in electrochemical impedance spectroscopy. Electro. Acta 2012, 72, 172–178. [Google Scholar] [CrossRef]
- Hirschorn, B.; Orazema, M.E.; Tribollet, B.; Vivier, V.; Frateur, I.; Musiani, M. Determination of effective capacitance and film thickness from constant-phase-element parameters. Electro. Acta 2010, 55, 6218–6227. [Google Scholar] [CrossRef]
- Wang, Z.; Yuan, C.; Zhu, B.; Feng, Q.; Liu, F.; Xu, J.; Zhou, C.; Chen, G. Complex impedance spectroscopy of perovskite microwave dielectric ceramics with high dielectric constant. J. Am. Ceram. Soc. 2019, 102, 1852–1865. [Google Scholar] [CrossRef]
- Tlili, D.; Hamdaoui, N.; Hcini, S.; Bouazizi, M.L.; Zemni, S. Above room temperature complex impedance analysis of properties of La0.33Sr0.67Mn0.33Ti0.67O3±δ perovskite. Phase Transit. 2017, 90, 644. [Google Scholar] [CrossRef]
- Pascoe, A.R.; Duffy, N.W.; Scully, A.D.; Huang, F.; Cheng, Y.B. Insights into planar CH3NH3PbI3 perovskite solar cells using impedance spectroscopy. J. Phys. Chem. C. 2015, 119, 4444–4453. [Google Scholar] [CrossRef]
- Tayari, F.; Nassar, K.I.; Benamara, M.; Essid, M.; Teixeira, S.S.; Graça, M.P.F. Sol–gel synthesized (Bi0.5Ba0.5Ag)0.5(NiMn)0.5O3 perovskite ceramic: An exploration of its structural characteristics, dielectric properties and electrical conductivity. Ceram. Int. 2024, 50, 11207–11215. [Google Scholar] [CrossRef]
- Achary, P.G.R.; Choudhary, R.N.P.; Parida, S.K. Structure, electric and dielectric properties of PbFe1/3Ti1/3W1/3O3 single perovskite compound. Process. Appl. Ceram. 2020, 14, 146–153. [Google Scholar] [CrossRef]
- Chebaane, M.; Talbi, N.; Dhahri, A.; Oumezzine, M.; Khirouni, K.J. Structural and impedance spectroscopy properties of La0.8Ba0.1Ca0.1Mn1−xRuxO3 perovskites. Magn. Magn. Mater. 2017, 426, 646. [Google Scholar] [CrossRef]
- Halizan, M.Z.M.; Mohamed, Z.; Yahya, A.K. Simultaneously improved dielectric, optical and conductivity properties of SrLa1−xNdxLiTeO6 double perovskites. Mater. Res. Express 2020, 7, 086301. [Google Scholar] [CrossRef]
- Boudad, L.; Taibi, M.; Belayachi, W.; Sajieddine, M. High temperature dielectric investigation, optical and conduction properties of GdFe0.5Cr0.5O3 perovskite. J. Appl. Phys. 2020, 127, 174103. [Google Scholar] [CrossRef]

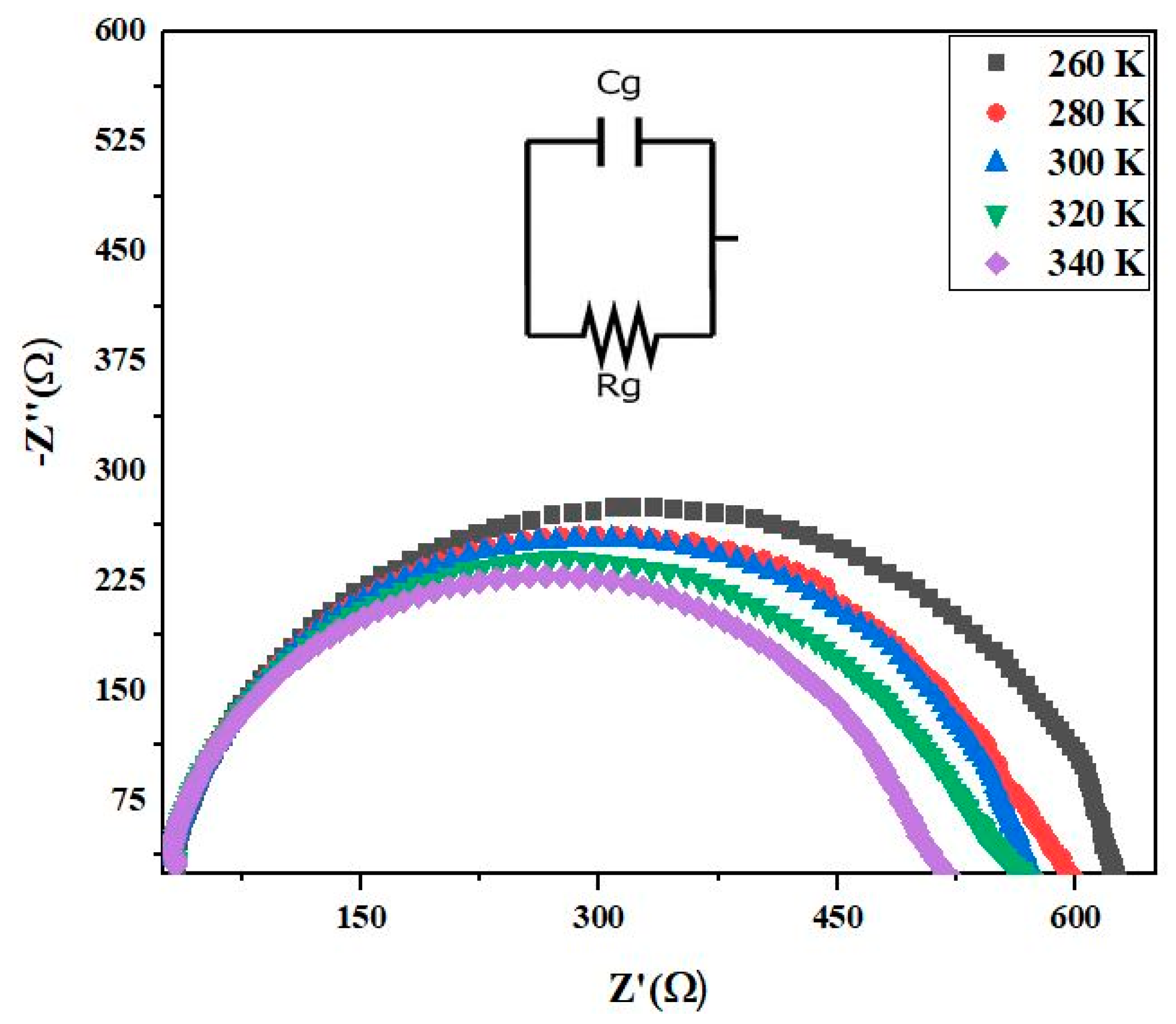

| T (K) | 260 | 280 | 300 | 320 | 340 |
| Rg (K) | 36.25 | 28.57 | 22.78 | 18.75 | 13.54 |
| Cg (10−9F) | 11.25 | 8.27 | 4.64 | 7.81 | 3.27 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tayari, F.; Benamara, M.; Lal, M.; Essid, M.; Thakur, P.; Kumar, D.; Teixeira, S.S.; Graça, M.P.F.; Nassar, K.I. Exploring Enhanced Structural and Dielectric Properties in Ag-Doped Sr(NiNb)0.5O3 Perovskite Ceramic for Advanced Energy Storage. Ceramics 2024, 7, 958-974. https://doi.org/10.3390/ceramics7030062
Tayari F, Benamara M, Lal M, Essid M, Thakur P, Kumar D, Teixeira SS, Graça MPF, Nassar KI. Exploring Enhanced Structural and Dielectric Properties in Ag-Doped Sr(NiNb)0.5O3 Perovskite Ceramic for Advanced Energy Storage. Ceramics. 2024; 7(3):958-974. https://doi.org/10.3390/ceramics7030062
Chicago/Turabian StyleTayari, Faouzia, Majdi Benamara, Madan Lal, Manel Essid, Priyanka Thakur, Deepak Kumar, S. Soreto Teixeira, M. P. F. Graça, and Kais Iben Nassar. 2024. "Exploring Enhanced Structural and Dielectric Properties in Ag-Doped Sr(NiNb)0.5O3 Perovskite Ceramic for Advanced Energy Storage" Ceramics 7, no. 3: 958-974. https://doi.org/10.3390/ceramics7030062
APA StyleTayari, F., Benamara, M., Lal, M., Essid, M., Thakur, P., Kumar, D., Teixeira, S. S., Graça, M. P. F., & Nassar, K. I. (2024). Exploring Enhanced Structural and Dielectric Properties in Ag-Doped Sr(NiNb)0.5O3 Perovskite Ceramic for Advanced Energy Storage. Ceramics, 7(3), 958-974. https://doi.org/10.3390/ceramics7030062










