Application the Ion Beam Sputtering Deposition Technique for the Development of Spin-Wave Structures on Ferroelectric Substrates
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials and Methods of Obtaining
2.2. Methods of Research
3. Results and Discussion
3.1. X-ray Studies
3.2. SEM Studies of the Cross Section
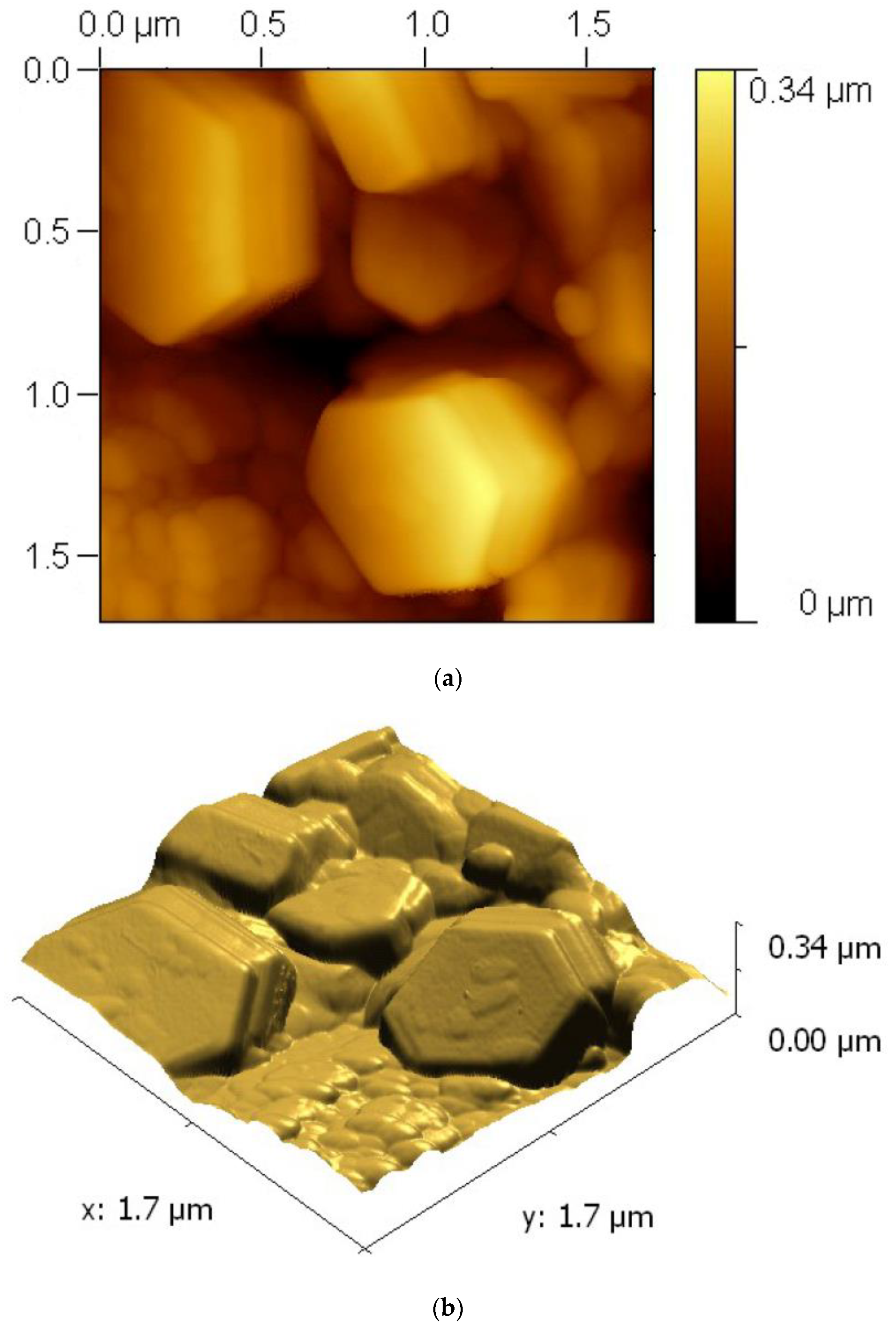
3.3. AFM Studies of Surface Morphology
3.4. MFM Studies
3.5. Magnetic Studies
3.6. FMR Studies
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Balinskiy, M.; Ojha, S.; Chiang, H.; Ranjbar, M.; Ross, C.A.; Khitun, A. Spin wave excitation in sub-micrometer thick Y3Fe5O12 films fabricated by pulsed laser deposition on garnet and silicon substrates: A comparative study. J. Appl. Phys. 2017, 122, 123904. [Google Scholar] [CrossRef]
- Sadovnikov, A.V.; Grachev, A.A.; Sheshukova, S.E.; Sharaevskii, Y.P.; Serdobintsev, A.A.; Mitin, D.M.; Nikitov, S.A. Magnon Straintronics: Reconfigurable Spin-Wave Routing in Strain-Controlled Bilateral Magnetic Stripes. Phys. Rev. Lett. 2018, 120, 257203. [Google Scholar] [CrossRef]
- Dubs, C.; Surzhenko, O.; Linke, R.; Danilewsky, A.; Brückner, U.; Dellith, J. Sub-micrometer yttrium iron garnet LPE films with low ferromagnetic resonance losses. J. Phys. D Appl. Phys. 2017, 50, 204005. [Google Scholar] [CrossRef] [Green Version]
- Chumak, A.V.; Vasyuchka, V.I.; Serga, A.A.; Hillebrands, B. Magnon spintronics. Nat. Phys. 2015, 11, 453–461. [Google Scholar] [CrossRef]
- Sadovnikov, A.V.; Grachev, A.A.; Beginin, E.N.; Sheshukova, S.E.; Sharaevskii, Y.P.; Nikitov, S.A. Voltage-controlled spin-wave coupling in adjacent ferromagnetic-ferroelectric heterostructures. Phys. Rev. Appl. 2017, 7, 014013. [Google Scholar] [CrossRef]
- Sadovnikov, A.V.; Odintsov, S.A.; Beginin, E.N.; Sheshukova, S.E.; Sharaevskii, Y.P.; Nikitov, S.A. Spin-wave switching in the side-coupled magnonic stripes. IEEE Trans. Magn. 2017, 53, 1–4. [Google Scholar] [CrossRef]
- Nikitov, S.A.; Kalyabin, D.V.; Lisenkov, I.V.; Slavin, A.N.; Barabanenkov, Y.N.; Osokin, S.A.; Sadovnikov, A.V.; Beginin, E.N.; Morozova, M.A.; Sharaevsky, Y.P.; et al. Magnonics: A new research area in spintronics and spin wave electronics. Phys. Uspekhi 2015, 185, 1099. [Google Scholar] [CrossRef]
- Bukharaev, A.A.; Zvezdin, A.K.; Pyatakov, A.P.; Fetisov, Y.K. Straintronics: A new trend in micro- and nanoelectronics and material science. Phys. Uspekhi 2018, 61, 1175–1212. [Google Scholar] [CrossRef]
- Serga, A.A.; Chumak, A.V.; Hillebrands, B. YIG magnonics. J. Phys. D Appl. Phys. 2010, 43, 264002. [Google Scholar] [CrossRef] [Green Version]
- Sokolov, N.S.; Fedorov, V.V.; Korovin, A.M.; Suturin, S.M.; Baranov, D.A.; Gastev, S.V.; Krichevtsov, B.B.; Maksimova, K.Y.; Grunin, A.I.; Bursian, V.E.; et al. Thin yttrium iron garnet films grown by pulsed laser deposition: Crystal structure, static, and dynamic magnetic properties. J. Appl. Phys. 2016, 119, 023903. [Google Scholar] [CrossRef]
- Sun, Y.; Song, Y.-Y.; Chang, H.; Kabatek, M.; Jantz, M.; Schneider, W.; Wu, M.; Schultheiss, H.; Hoffmann, A. Growth and ferromagnetic resonance properties of nanometer-thick yttrium iron garnet films. Appl. Phys. Lett. 2012, 101, 152405. [Google Scholar] [CrossRef]
- Manuilov, S.A.; Fors, R.; Khartsev, S.I.; Grishin, A.M. Submicron Y3Fe5O12 film magnetostatic wave band pass filters. J. Appl. Phys. 2009, 105, 033917. [Google Scholar] [CrossRef]
- Wang, Z.; Sun, Y.; Song, Y.; Wu, M.; Schultheiss, H.; Pearson, J.E.; Hoffmann, A. Electric control of magnetization relaxation in thin film magnetic insulators. Appl. Phys. Lett. 2011, 99, 162511. [Google Scholar] [CrossRef]
- Onbasli, M.C.; Kehlberger, A.; Kim, D.H.; Jakob, G.; Klaui, M.; Chumak, A.V.; Hillebrands, B.; Ross, C.A. Pulsed laser deposition of epitaxial yttrium iron garnet films with low Gilbert damping and bulk-like magnetization. APL Mater. 2014, 2, 106102. [Google Scholar] [CrossRef] [Green Version]
- Suturin, S.M.; Korovin, A.M.; Bursian, V.E.; Lutsev, L.V.; Bourobina, V.; Yakovlev, N.L.; Montecchi, M.; Pasquali, L.; Ukleev, V.; Vorobiev, A.; et al. Role of gallium diffusion in the formation of a magnetically dead layer at the Y3Fe5O12/Gd3Ga5O12 epitaxial interface. Phys. Rev. Mater. 2018, 2, 104404. [Google Scholar] [CrossRef] [Green Version]
- Stancil, D.D.; Prabhakar, A. Spin Waves: Theory and Applications; Springer: New York, NY, USA, 2009. [Google Scholar] [CrossRef]
- Lax, B.; Button, K.J. Microwave Ferrites and Ferromagnetic; McGraw-Hill: New York, NY, USA, 1962. [Google Scholar] [CrossRef]
- Gatabi, J.R.; Rahman, S.; Amaro, A.; Nash, T.; Rojas-Ramirez, J.; Pandey, R.K.; Droopad, R. Tuning electrical properties of PZT film deposited by Pulsed Laser Deposition. Ceram. Int. 2017, 43, 6008–6012. [Google Scholar] [CrossRef]
- Dipti, D.; Juneja, J.K.; Singh, S.; Raina, K.K.; Prakash, C. Enhancement in magnetoelectric coupling in PZT based composites. Ceram. Int. 2015, 41, 6108–6112. [Google Scholar] [CrossRef]
- Xu, T.; Wang, C.-A.; Wang, C. Synthesis and magnetoelectric effect of composites with CoFe2O4-epoxy embedded in 3–1 type porous PZT ceramics. Ceram. Int. 2015, 41, 11080–11085. [Google Scholar] [CrossRef]
- Zhou, P.; Liang, K.; Liu, Y.; Qi, Y.; Ma, Z.; Zhang, T. Thickness dependence of magnetoelectric properties for Pb(Zr0.52Ti0.48O3/La0.67Sr0.33MnO3 heterostructures. Ceram. Int. 2018, 44, 12905–12908. [Google Scholar] [CrossRef]
- Izyumskaya, N.; Alivov, Y.-I.; Cho, S.-J.; Morkoç, H.; Lee, H.; Kang, Y.-S. Processing, structure, properties, and applications of PZT thin films. Crit. Rev. Solid State Mater. Sci. 2007, 32, 111–202. [Google Scholar] [CrossRef]
- Bhoi, B. Bulk-like magnetization and improved microwave properties of polycrystalline YIG (Y3Fe5O12) films grown on quartz substrates by pulsed laser deposition. J. Alloys Compd. 2019, 797, 523–528. [Google Scholar] [CrossRef]
- Landolt, H.; Bornstein, R. Numerical Data and Functional Relationships in Science and Technology—New Series; Springer: Berlin/Heidelberg, Germany, 1975; Available online: https://www.springer.com/series/284 (accessed on 17 May 2023).
- Stognij, A.I.; Novitskii, N.N.; Sharko, S.A.; Bespalov, A.V.; Golikova, O.L.; Sazanovich, A.; Dyakonov, V.; Szymczak, H.; Smirnova, M.N.; Ketsko, V.A. Effect of Cobalt Layer Thickness on the Magnetoelectric Properties of Co/PbZr0.45Ti0.55O3/Co Heterostructures. Inorg. Mater. 2013, 49, 1011–1014. [Google Scholar] [CrossRef]
- Stognij, A.I.; Novitskii, N.N.; Sazanovich, A.; Poddubnaya, N.N.; Sharko, S.A.; Mikhailov, V.; Nizhankovski, V.; Dyakonov, V.; Szymczak, H. Ion-beam sputtering deposition and magnetoelectric properties of layered heterostructures (FM/PZT/FM)n, where FM—Co or Ni78Fe22. Eur. Phys. J. Appl. Phys. 2013, 63, 21301. [Google Scholar] [CrossRef]
- Burdin, D.A.; Chashin, D.V.; Ekonomov, N.A.; Fetisov, L.Y.; Fetisov, Y.K. Suppression of nonlinear magnetoelectric effect hysteresis in a layered ferromagnetic-piezoelectric structure. J. Magn. Magn. Mater. 2018, 449, 152–156. [Google Scholar] [CrossRef]
- Stognij, A.I.; Novitskii, N.N.; Poddubnaya, N.N.; Sharko, S.A.; Ketsko, V.A.; Mikhailov, V.; Dyakonov, V.; Szymczak, H. Interface magnetoelectric effect in the layered heterostructures with Co layers on the polished and ion-beam planarized ceramic PZT substrates. Eur. Phys. J. Appl. Phys. 2015, 69, 11301. [Google Scholar] [CrossRef]
- Stognij, A.I.; Novitskii, N.N.; Trukhanov, S.V.; Trukhanov, A.V.; Panina, L.V.; Sharko, S.A.; Serokurova, A.I.; Poddubnaya, N.N.; Ketsko, V.A.; Dyakonov, V.P.; et al. Interface magnetoelectric effect in elastically linked Co/PZT/Co layered structures. J. Magn. Magn. Mater. 2019, 485, 291–296. [Google Scholar] [CrossRef]
- Stognij, A.I.; Novitskii, N.N.; Ketsko, V.A.; Poddubnaya, N.N.; Laletin, V.M.; Bespalov, A.V.; Golikova, O.L.; Smirnova, M.N.; Fetisov, L.Y.; Titova, A.O. Influence of the State of Interfaces on the Magnitude of the Magnetoelectric Effect in Co (Ni) Films on PbZr0.45Ti0.55O3 and GaAs Substrates. Inorg. Mater. 2016, 52, 1070–1076. [Google Scholar] [CrossRef]
- Kang, Y.-M.; Wee, S.-H.; Baik, S.-I.; Min, S.-G.; Yu, S.-C.; Moon, S.-H.; Kim, Y.-W.; Yoo, S.-I. Magnetic properties of YIG thin films prepared by the post annealing of amorphous films deposited by rf-magnetron sputtering. J. Appl. Phys. 2005, 97, 10A319. [Google Scholar] [CrossRef]
- Guo, X.; Chen, Y.; Wang, G.; Zhang, Y.; Ge, J.; Tang, X.; Ponchel, F.; Rémiens, D.; Dong, X. Growth and characterization of yttrium iron garnet films on Si substrates by Chemical Solution Deposition (CSD) technique. J. Alloys Compd. 2016, 671, 234–237. [Google Scholar] [CrossRef]
- Stognij, A.I.; Pashkevich, M.V.; Novitskii, N.N.; Bespalov, A.V. Ion-Beam Engineering of Multilayer Co/TiO2 Nanostructures. Techn. Phys. Lett. 2010, 36, 422–425. [Google Scholar] [CrossRef]
- Stognij, A.I.; Lutsev, L.; Novitskii, N.N.; Bespalov, A.V.; Golikova, O.N.; Ketsko, V.A.; Gieniusz, R.; Maziewski, A. Synthesis, magnetic properties and spin-wave propagation in thin Y3Fe5O12 films sputtered on GaN-based substrates. J. Phys. D Appl. Phys. 2015, 48, 485002. [Google Scholar] [CrossRef]
- Petrov, V.M.; Srinivasan, G.; Laletin, V.M.; Bichurin, M.I.; Tuskov, D.S.; Poddubnaya, N.N. Magnetoelectric effects in porous ferromagnetic piezoelectric bulk composites: Experiment and theory. Phys. Rev. B 2007, 75, 174422. [Google Scholar] [CrossRef]
- Stognij, A.I.; Pashkevich, M.V.; Novitskii, N.N.; Gribkov, B.A.; Mironov, V.L.; Geras’kin, A.A.; Ketsko, V.A.; Fettar, F.; Garad, H. Controlled Growth of Co Nanofilms on Si (100) by Ion Beam Deposition. Inorg. Mater. 2011, 47, 869–875. [Google Scholar] [CrossRef]
- International Centre for Diffraction Data, ICDD. Available online: https://www.icdd.com/pdfsearch/ (accessed on 17 May 2023).
- Stognij, A.I.; Novitskii, N.N.; Golikova, O.L.; Bespalov, A.V.; Gieniusz, R.; Maziewski, A.; Stupakiewicz, A.; Smirnova, M.N.; Ketsko, V.A. Growth of Y3Fe5O12 Films on Si with AlOx and SiO2 Buffer Layers by Ion Beam Sputtering. Inorg. Mat. 2017, 53, 1069–1074. [Google Scholar] [CrossRef]
- Oh, I.; Park, J.; Jo, J.; Jin, M.-J.; Jang, M.-S.; Lee, K.-S.; Yoo, J.-W. Solution-Processed Ferrimagnetic Insulator Thin Film for the Microelectronic Spin Seebeck Energy Conversion. ACS Appl. Mater. Interfaces 2018, 10, 28608–28614. [Google Scholar] [CrossRef] [PubMed]
- Jakubisova, E.L.; Visnovsky, S.; Chang, H.; Wu, M. Interface effects in nanometer-thick yttrium iron garnet films studied by magneto-optical spectroscopy. Appl. Phys. Lett. 2016, 108, 082403. [Google Scholar] [CrossRef]
- Howe, B.M.; Emori, S.; Jeon, H.M.; Oxhol, T.; Jones, J.G.; Mahalingam, K.; Zhuang, Y.; Sun, N.X.; Brown, G.J. Pseudomorphic yttrium iron garnet thin films with low damping and inhomogeneous linewidth broadening. IEEE Magn. Lett. 2015, 6, 3500504. [Google Scholar] [CrossRef]
- Von Aulock, W.H.; Boxer, A.S. Handbook of Microwave Ferrite Materials; Academic Press Inc.: New York, NY, USA, 1965; 518p. [Google Scholar]
- Bhoi, B.; Sahu, B.; Venkataramani, N.; Aiyar, R.P.R.C.; Prasad, S. Preparation of Low Microwave Loss YIG Thin Films by Pulsed Laser Deposition. IEEE Trans. Magn. 2015, 51, 2800704. [Google Scholar] [CrossRef]
- Krockenberger, Y.; Yun, K.-S.; Hatano, T.; Arisawa, S.; Kawasaki, M.; Tokura, Y. Layer-by-layer growth and magnetic properties of Y3Fe5O12 thin films on Gd3Ga5O12. J. Appl. Phys. 2009, 106, 123911. [Google Scholar] [CrossRef]
- Dorsey, P.C.; Bushnell, S.E.; Seed, R.G.; Vittoria, C. Epitaxial yttrium iron garnet films grown by pulsed laser deposition. J. Appl. Phys. 1993, 74, 1242–1248. [Google Scholar] [CrossRef]
- Gurevich, A.G. Magnetic Resonance in Ferrites and Antiferromagnets; Science: Moscow, Russia, 1973; 592p. [Google Scholar]
- Lee, S.; Grudichak, S.; Sklenar, J.; Tsai, C.C.; Jang, M.; Yang, Q.; Zhang, H.; Ketterson, J.B. Ferromagnetic resonance of a YIG film in the low frequency regime. J. Appl. Phys. 2016, 120, 033905. [Google Scholar] [CrossRef] [Green Version]

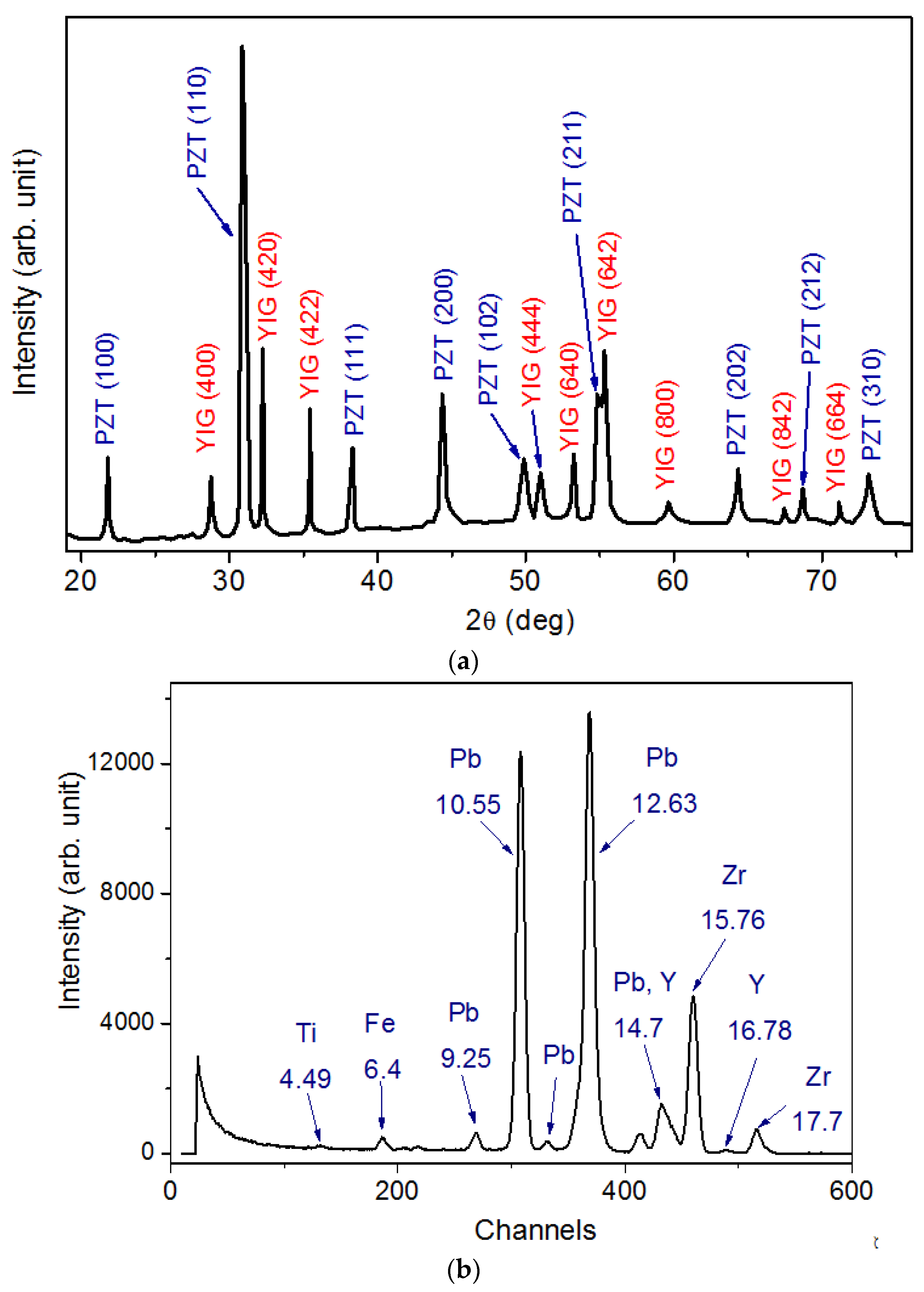







| Reflection | 2θ (Degrees) | Δθ = θ − θref (Deg) | Δa/a | ||
|---|---|---|---|---|---|
| 2θ (Deg) | 2θref (Deg) | ||||
| PZT | 100 | 21.8 | 22.1 | −0.15 | 0.013 |
| 110 | 30.9 | 31.0 | −0.05 | 0.003 | |
| 111 | 38.1 | 38.4 | −0.15 | 0.008 | |
| 200 | 44.8 | 45.1 | −0.15 | 0.006 | |
| 102 | 49.3 | 49.5 | −0.1 | 0.004 | |
| 211 | 55.3 | 55.7 | −0.1 | 0.003 | |
| 202 | 64.8 | 64.6 | +0.1 | −0.003 | |
| 212 | 68.8 | 69.2 | −0.2 | 0.005 | |
| 310 | 74.2 | 74.6 | −0.1 | 0.002 | |
| YIG | 400 | 28.75 | 28.84 | −0.045 | 0.003 |
| 420 | 32.25 | 32.32 | −0.035 | 0.0021 | |
| 422 | 35.44 | 35.50 | −0.003 | 0.0016 | |
| 444 | 51.00 | 51.08 | −0.004 | 0.0015 | |
| 640 | 53.25 | 53.34 | −0.0045 | 0.0016 | |
| 642 | 55.31 | 55.52 | −0.105 | 0.0035 | |
| 800 | 59.63 | 59.72 | −0.045 | 0.0014 | |
| 842 | 67.44 | 67.66 | −0.11 | −0.0029 | |
| 664 | 71.13 | 71.44 | −0.155 | 0.0038 | |
| Sample | Hin (kOe) | from Hin | Hout (kOe) | from Hout | Ha (kOe) | ||
|---|---|---|---|---|---|---|---|
| ΔH (kOe) | Hu (kOe) | ΔH (kOe) | Hu (kOe) | ||||
| 1 | 2.84 | 0.149 | −1.22 | 4.71 | 0.116 | −1.32 | −0.935 |
| 2 | 2.81 | 0.119 | −1.29 | 4.74 | 0.120 | −1.34 | −0.950 |
| 3 | 2.82 | 0.105 | −1.27 | 4.72 | 0.109 | −1.33 | −0.950 |
| 4 | 2.82 | 0.115 | −1.27 | 4.71 | 0.107 | −1.32 | −0.945 |
| Sample | Ku (Erg/cm3) | α |
|---|---|---|
| 1 | −4.44 × 104 | 7.03 × 10−3 |
| 2 | −4.51 × 104 | 5.61 × 10−3 |
| 3 | −4.51 × 104 | 4.95 × 10−3 |
| 4 | −4.49 × 104 | 5.42 × 10−3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sharko, S.A.; Serokurova, A.I.; Novitskii, N.N.; Ketsko, V.A.; Stognij, A.I. Application the Ion Beam Sputtering Deposition Technique for the Development of Spin-Wave Structures on Ferroelectric Substrates. Ceramics 2023, 6, 1415-1433. https://doi.org/10.3390/ceramics6030087
Sharko SA, Serokurova AI, Novitskii NN, Ketsko VA, Stognij AI. Application the Ion Beam Sputtering Deposition Technique for the Development of Spin-Wave Structures on Ferroelectric Substrates. Ceramics. 2023; 6(3):1415-1433. https://doi.org/10.3390/ceramics6030087
Chicago/Turabian StyleSharko, Sergei A., Aleksandra I. Serokurova, Nikolay N. Novitskii, Valerii A. Ketsko, and Alexandre I. Stognij. 2023. "Application the Ion Beam Sputtering Deposition Technique for the Development of Spin-Wave Structures on Ferroelectric Substrates" Ceramics 6, no. 3: 1415-1433. https://doi.org/10.3390/ceramics6030087
APA StyleSharko, S. A., Serokurova, A. I., Novitskii, N. N., Ketsko, V. A., & Stognij, A. I. (2023). Application the Ion Beam Sputtering Deposition Technique for the Development of Spin-Wave Structures on Ferroelectric Substrates. Ceramics, 6(3), 1415-1433. https://doi.org/10.3390/ceramics6030087






