Ultrasonic Characterization of Nanoparticle-Based Ceramics Fabricated by Spark-Plasma Sintering
Abstract
1. Introduction
2. Theoretical Background
3. Materials and Methods
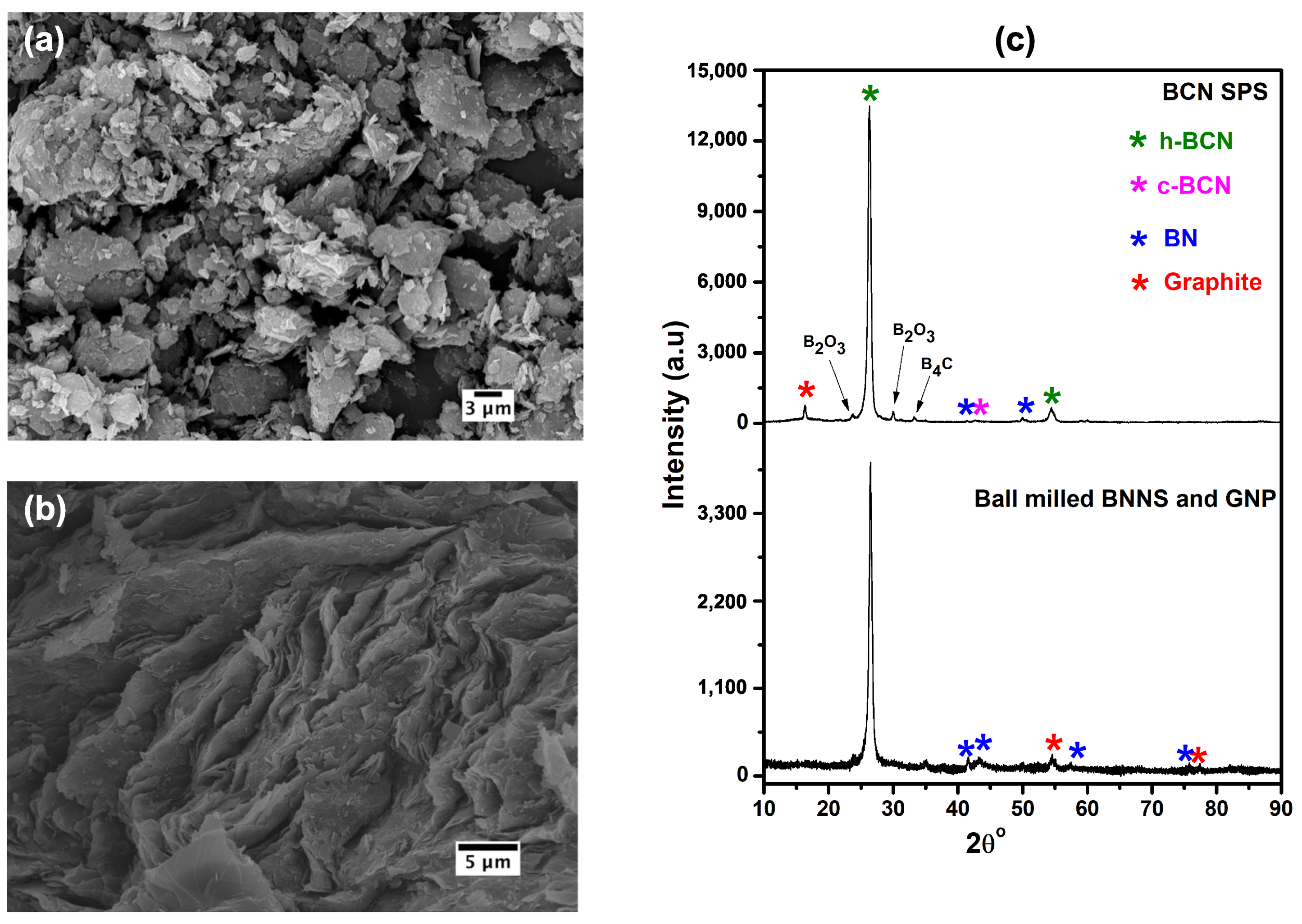
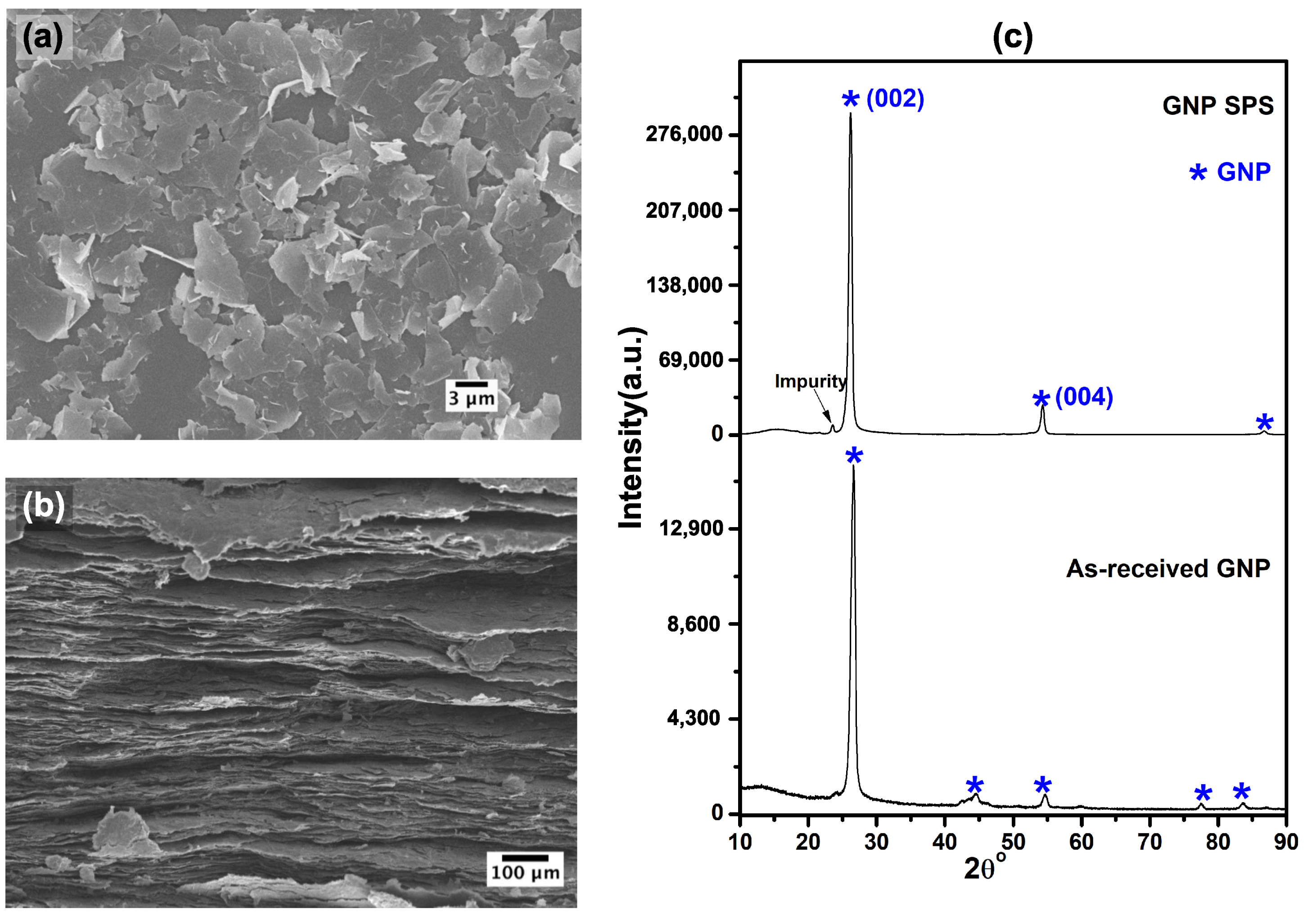
3.1. Examined Materials
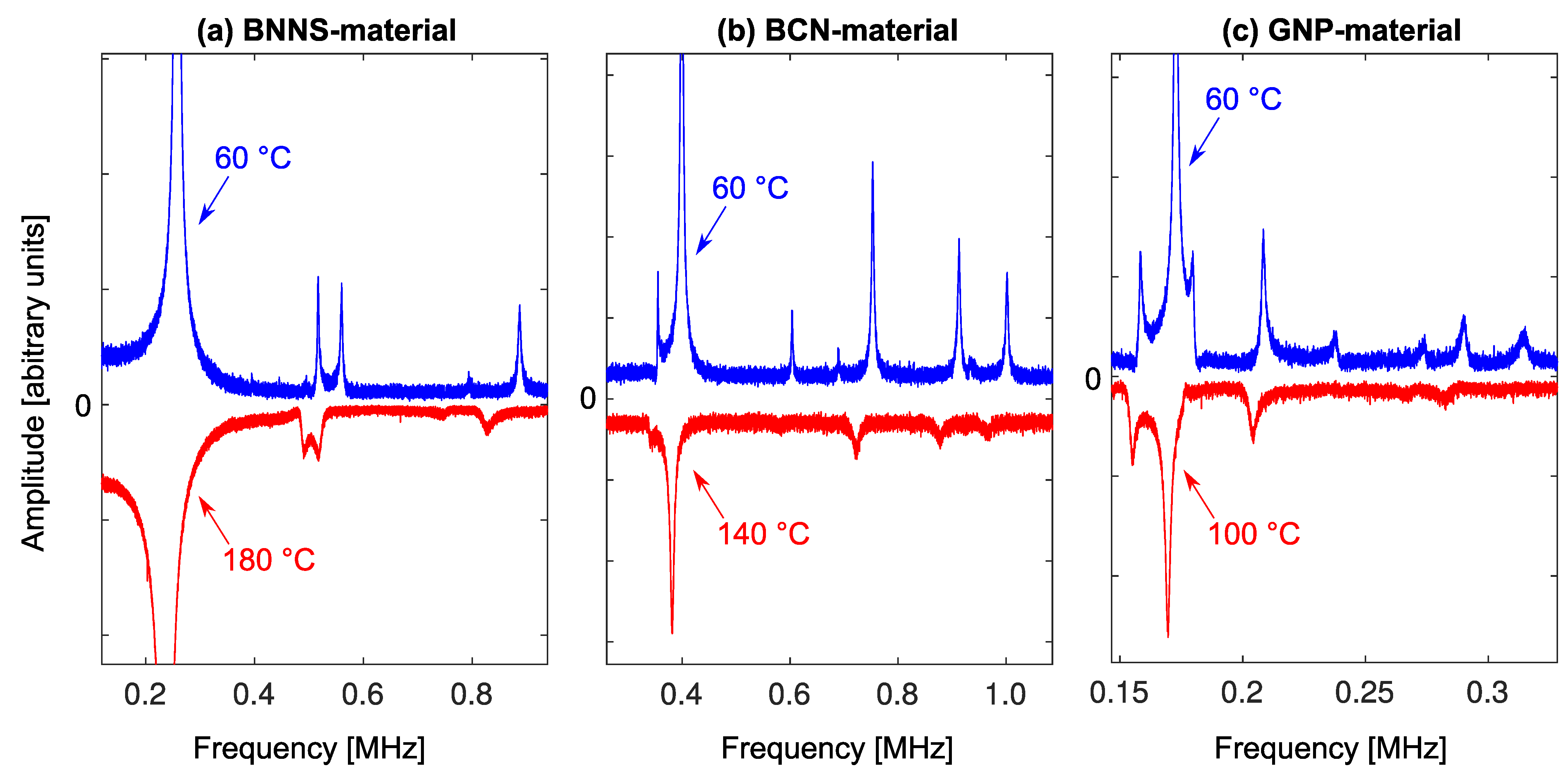
3.2. Ultrasonic Measurements
4. Results and Discussion
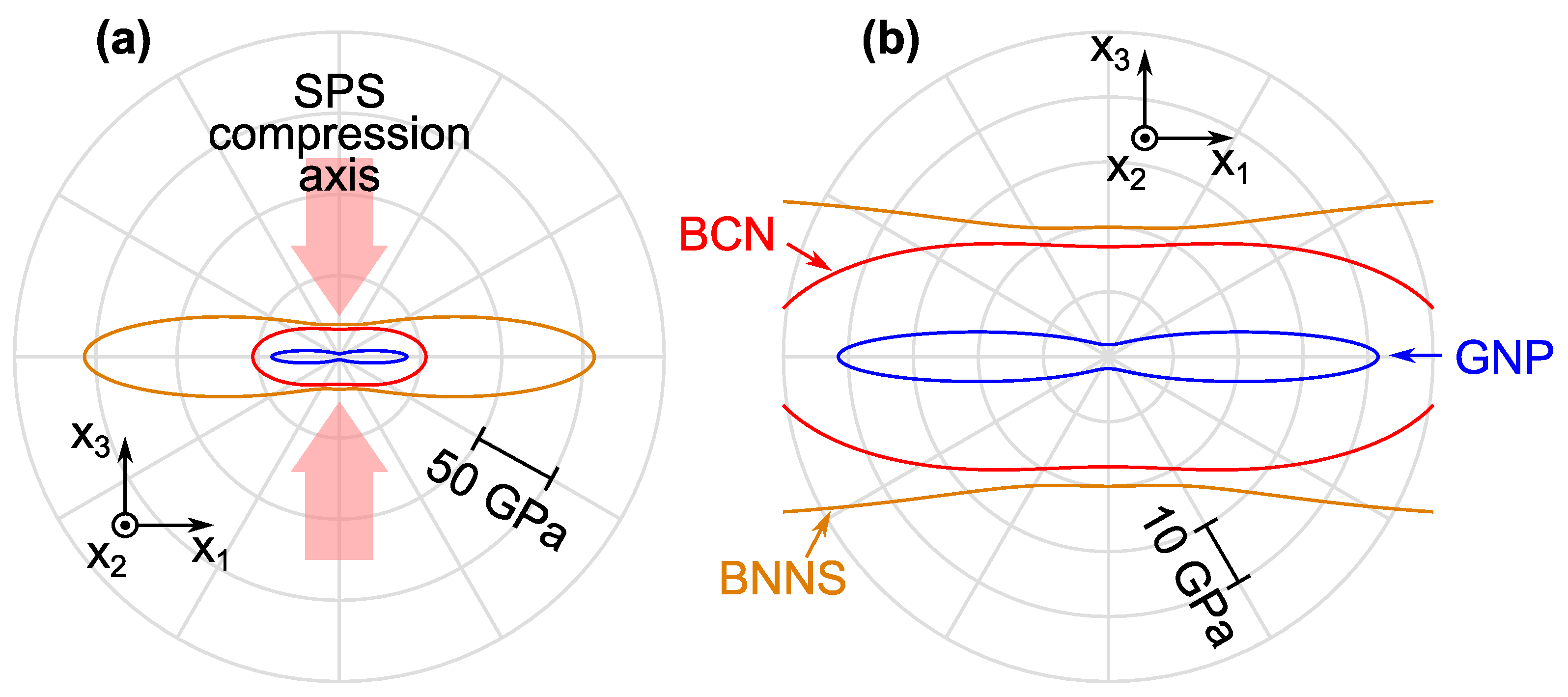
4.1. Elastic Constants
4.2. Internal Friction
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Biesuz, M.; Saunders, T.; Ke, D.; Reece, M.J.; Hu, C.; Grasso, S. A review of electromagnetic processing of materials (EPM): Heating, sintering, joining and forming. J. Mater. Sci. Technol. 2021, 69, 239–272. [Google Scholar] [CrossRef]
- Biesuz, M.; Grasso, S.; Sglavo, V.M. What’s new in ceramics sintering? A short report on the latest trends and future prospects. Curr. Opin. Solid State Mater. Sci. 2020, 24, 100868. [Google Scholar] [CrossRef]
- Munir, Z.A.; Anselmi-Tamburini, U.; Ohyanagi, M. The effect of electric field and pressure on the synthesis and consolidation of materials: A review of the spark plasma sintering method. J. Mater. Sci. 2006, 41, 763–777. [Google Scholar] [CrossRef]
- Viswanathan, V.; Laha, T.; Balani, K.; Agarwal, A.; Seal, S. Challenges and advances in nanocomposite processing techniques. Mater. Sci. Eng. R Rep. 2006, 54, 121–285. [Google Scholar] [CrossRef]
- Nieto, A.; Bisht, A.; Lahiri, D.; Zhang, C.; Agarwal, A. Graphene reinforced metal and ceramic matrix composites: A review. Int. Mater. Rev. 2017, 62, 241–302. [Google Scholar] [CrossRef]
- Gao, C.; Feng, P.; Peng, S.; Shuai, C. Carbon nanotube, graphene and boron nitride nanotube reinforced bioactive ceramics for bone repair. Acta Biomater. 2017, 61, 1–20. [Google Scholar] [CrossRef] [PubMed]
- Centeno, A.; Rocha, V.G.; Alonso, B.; Fernández, A.; Gutierrez-Gonzalez, C.F.; Torrecillas, R.; Zurutuza, A. Graphene for tough and electroconductive alumina ceramics. J. Eur. Ceram. Soc. 2013, 33, 3201–3210. [Google Scholar] [CrossRef]
- Ramírez, C.; Vega-Diaz, S.M.; Morelos-Gómez, A.; Figueiredo, F.M.; Terrones, M.; Osendi, M.I.; Belmonte, M.; Miranzo, P. Synthesis of conducting graphene/Si3N4 composites by spark plasma sintering. Carbon 2013, 57, 425–432. [Google Scholar] [CrossRef]
- Miranzo, P.; García, E.; Ramírez, C.; González-Julián, J.; Belmonte, M.; Osendi, M.I. Anisotropic thermal conductivity of silicon nitride ceramics containing carbon nanostructures. J. Eur. Ceram. Soc. 2012, 32, 1847–1854. [Google Scholar] [CrossRef]
- Llorente, J.; Román-Manso, B.; Miranzo, P.; Belmonte, M. Tribological performance under dry sliding conditions of graphene/silicon carbide composites. J. Eur. Ceram. Soc. 2016, 36, 429–435. [Google Scholar] [CrossRef]
- Malek, O.; González-Julián, J.; Vleugels, J.; Verauwera, W.; Lauwers, B.; Belmonte, M. Carbon nanofillers for machining insulating ceramics. Mater. Today 2011, 14, 496–501. [Google Scholar] [CrossRef]
- Hotta, M.; Goto, T. Densification and microstructure of Al2O3-cBN composites prepared by spark plasma sintering. J. Ceram. Soc. Jpn. 2008, 116, 744–748. [Google Scholar] [CrossRef]
- Yue, C.; Liu, W.; Zhang, L.; Zhang, T.; Chen, Y. Fracture toughness and toughening mechanisms in a (ZrB2-SiC) composite reinforced with boron nitride nanotubes and boron nitride nanoplatelets. Scr. Mater. 2013, 68, 579–582. [Google Scholar] [CrossRef]
- Li, Y.-L.; Li, R.-X.; Zhang, J.-X. Enhanced mechanical properties of machinable Si3N4/BN composites by spark plasma sintering. Mater. Sci. Eng. A 2008, 483–484, 207–210. [Google Scholar] [CrossRef]
- Sedlák, R.; Kovalčíková, A.; Balko, J.; Rutkowski, P.; Dubiel, A.; Zientara, D.; Girman, V.; Múdra, E.; Dusza, J. Effect of graphene platelets on tribological properties of boron carbide ceramic composites. Int. J. Refract. Met. Hard Mater. 2017, 65, 57–63. [Google Scholar] [CrossRef]
- Seiner, H.; Sedlák, P.; Koller, M.; Landa, M.; Ramírez, C.; Osendi, M.I.; Belmonte, M. Anisotropic elastic moduli and internal friction of graphene nanoplatelets/silicon nitride composites. Compos. Sci. Technol. 2013, 75, 93–97. [Google Scholar] [CrossRef]
- Michel, K.H.; Verberck, B. Theory of the elastic constants of graphite and graphene. Phys. Status Solidi (B) Basic Res. 2008, 245, 2177–2180. [Google Scholar] [CrossRef]
- Falin, A.; Cai, Q.; Santos, E.J.G.; Scullion, D.; Qian, D.; Zhang, R.; Yang, Z.; Huang, S.; Watanabe, K.; Taniguchi, T. Mechanical properties of atomically thin boron nitride and the role of interlayer interactions. Nat. Commun. 2017, 8, 15815. [Google Scholar] [CrossRef] [PubMed]
- Seiner, H.; Ramirez, C.; Koller, M.; Sedlák, P.; Landa, M.; Miranzo, P.; Belmonte, M.; Osendi, M.I. Elastic properties of silicon nitride ceramics reinforced with graphene nanofillers. Mater. Des. 2015, 87, 675–680. [Google Scholar] [CrossRef]
- Kachanov, M. On the concept of approximate elastic symmetry and its application to materials with defects. Int. J. Fract. 1995, 74, R33–R38. [Google Scholar] [CrossRef]
- Nieto, A.; Lahiri, D.; Agarwal, A. Synthesis and properties of bulk graphene nanoplatelets consolidated by spark plasma sintering. Carbon 2012, 50, 4068–4077. [Google Scholar] [CrossRef]
- Loganathan, A.; Sharma, A.; Rudolf, C.; Zhang, C.; Nautiyal, P.; Suwas, S.; Boesl, B.; Agarwal, A. In-situ deformation mechanism and orientation effects in sintered 2D boron nitride nanosheets. Mater. Sci. Eng. A 2017, 708, 440–450. [Google Scholar] [CrossRef]
- Koller, M.; Seiner, H.; Landa, M.; Nieto, A.; Agarwal, A. Anisotropic elastic and acoustic properties of bulk graphene nanoplatelets consolidated by spark plasma sintering. Acta Phys. Pol. A 2015, 128, 670–674. [Google Scholar] [CrossRef]
- Liu, X.; Metcalf, T.H.; Robinson, J.T.; Perkins, F.K.; Houston, B.H. Internal friction and shear modulus of graphene films. Solid State Phenom. 2012, 184, 319–324. [Google Scholar] [CrossRef]
- Liu, X.; Metcalf, T.H.; Robinson, J.T.; Houston, B.H.; Scarpa, F. Shear modulus of monolayer graphene prepared by chemical vapor deposition. Nano Lett. 2012, 12, 1013–1017. [Google Scholar] [CrossRef] [PubMed]
- Dong, Y.; Wang, F.; Zhu, Z.; He, T. Influences of out-of-plane elastic energy and thermal effects on friction between graphene layers. AIP Adv. 2019, 9, 045213. [Google Scholar] [CrossRef]
- Migliori, A.; Sarrao, J.L.; Visscher, W.M.; Bell, T.M.; Lei, M.; Fisk, Z.; Leisure, R.G. Resonant ultrasound spectroscopic techniques for measurement of the elastic moduli of solids. Phys. B Phys. Condens. Matter 1993, 183, 1–24. [Google Scholar] [CrossRef]
- Leisure, R.G.; Willis, F.A. Resonant ultrasound spectroscopy. J. Phys. Condens. Matter 1997, 9, 6001–6029. [Google Scholar] [CrossRef]
- Sedlák, P.; Seiner, H.; Zídek, J.; Janovská, M.; Landa, M. Determination of All 21 Independent Elastic Coefficients of Generally Anisotropic Solids by Resonant Ultrasound Spectroscopy: Benchmark Examples. Exp. Mech. 2014, 54, 1073–1085. [Google Scholar] [CrossRef]
- Ogi, H.; Sato, K.; Asada, T.; Hirao, M. Complete mode identification for resonance ultrasound spectroscopy. J. Acoust. Soc. Am. 2002, 112, 2553–2557. [Google Scholar] [CrossRef]
- Ghosh, S.; Matty, M.; Baumbach, R.; Bauer, E.D.; Modic, K.A.; Shekhter, A.; Mydosh, J.A.; Kim, E.-A.; Ramshaw, B.J. One-component order parameter in URu2Si2 uncovered by resonant ultrasound spectroscopy and machine learning. Sci. Adv. 2020, 6, eaaz4074. [Google Scholar] [CrossRef]
- Sumino, Y.; Ohno, I.; Goto, T.; Kumazawa, M. Measurement of elastic constants and internal frictions on single-crystal mgo by rectangular parallelepiped resonance. J. Phys. Earth 1976, 24, 263–272. [Google Scholar] [CrossRef][Green Version]
- Nowick, A.S.; Berry, B.S. Anelastic Relaxation in Crystalline Solids; Academic Press: New York, NY, USA, 1972. [Google Scholar]
- François, M.; Geymonat, G.; Berthaud, Y. Determination of the symmetries of an experimentally determined stiffness tensor: Application to acoustic measurements. Int. J. Solids Struct. 1998, 35, 4091–4106. [Google Scholar] [CrossRef]
- Evans, A.G.; Tittmann, B.R.; Ahlberg, L.; Khuri-Yakub, B.T.; Kino, G.S. Ultrasonic attenuation in ceramics. J. Appl. Phys. 1978, 49, 2669–2679. [Google Scholar] [CrossRef]
- Karunarathne, A.; Jodha, K.S.; Priyadarshan, G.; Griggs, J.A.; Gladden, J.R. Low pressure dependent elasticity of porous ceramics. J. Am. Ceram. Soc. 2020, 103, 1312–1320. [Google Scholar] [CrossRef]
- Roy, S.; Gebert, J.-M.; Stasiuk, G.; Piat, R.; Weidenmann, K.A.; Wanner, A. Complete determination of elastic moduli of interpenetrating metal/ceramic composites using ultrasonic techniques and micromechanical modelling. Mater. Sci. Eng. A 2011, 528, 8226–8235. [Google Scholar] [CrossRef]
- Shyam, A.; Lara-Curzio, E.; Watkins, T.R.; Parten, R.J. Mechanical characterization of diesel particulate filter substrates. J. Am. Ceram. Soc. 2008, 91, 1995–2001. [Google Scholar] [CrossRef]
- Kruisová, A.; Seiner, H.; Sedlák, P.; Landa, M.; Román-Manso, B.; Miranzo, P.; Belmonte, M. Acoustic metamaterial behavior of three-dimensional periodic architectures assembled by robocasting. Appl. Phys. Lett. 2014, 105, 211904. [Google Scholar] [CrossRef]
- Wanner, A. Elastic modulus measurements of extremely porous ceramic materials by ultrasonic phase spectroscopy. Mater. Sci. Eng. A 1998, 248, 35–43. [Google Scholar] [CrossRef]
- Nehate, S.D.; Saikumar, A.K.; Prakash, A.; Sundaram, K.B. A review of boron carbon nitride thin films and progress in nanomaterials. Mater. Today Adv. 2020, 8, 100106. [Google Scholar] [CrossRef]
- Zhang, Z.; Duan, X.; Qui, B.; Chen, L.; Zhang, P.; Cai, D.; He, P.; Zhang, H.; Wei, Z.; Yang, Z.; et al. Microstructure evolution and grain growth mechanisms of h-BN ceramics during hot-pressing. J. Eur. Ceram. Soc. 2020, 40, 2268–2278. [Google Scholar] [CrossRef]
- Ni, D.W.; Zhang, G.J.; Kan, Y.M.; Sakka, Y. Highly textured ZrB2-based ultrahigh temperature ceramics via strong magnetic field alignment. Scr. Mater. 2009, 60, 615–618. [Google Scholar] [CrossRef]
- Wei, J.N.; Huang, T.C.; Zhao, L.; Yu, J.M.; Xie, W.J.; Li, G.M. Internal friction characterization of graphite. J. Mater. Sci. 2008, 43, 5470–5473. [Google Scholar] [CrossRef]
- Wei, J.N.; Li, Y.L.; Song, S.H.; Ji, G.C.; Ma, M.L.; Zhang, D.Q.; Han, F.S. Grain boundary peak in macroscopic graphite participates reinforced Zn-Al metal matrix composite. Phys. Status Solidi Appl. Res. 2004, 201, 923–928. [Google Scholar] [CrossRef]
- Reguzzoni, M.; Fasolino, A.; Molinari, E.; Righi, M.C. Friction by shear deformations in multilayer graphene. J. Phys. Chem. C 2012, 116, 21104–21108. [Google Scholar] [CrossRef]


| Lotgering Orientation Factor | |||
|---|---|---|---|
| Powder | Bulk | (JCPDS) | |
| BNNS-material | 0.778 | 0.981 | 0.716 |
| BCN-material | 0.877 | 0.978 | 0.458 |
| GNP-material | 0.801 | 0.975 | 0.760 |
| [GPa] | [GPa] | [GPa] | [GPa] | [GPa] | [GPa] (a) | |
|---|---|---|---|---|---|---|
| BNNS-material | 186.15 | 60.05 | 21.31 | 23.62 | 11.28 | 63.05 |
| ± 2.88 | ± 4.55 | ± 2.44 | ± 0.92 | ± 0.09 | ||
| BCN-material | 58.34 | 8.80 | 9.64 | 19.72 | 9.84 | 24.77 |
| ± 1.23 | ±1.72 | ± 1.50 | ± 0.74 | ± 0.07 | ||
| GNP-material [23] | 66.17 | 30.65 | 9.14 | 3.57 | 1.15 | 17.76 |
| U [kJ/mol] | |||
|---|---|---|---|
| BNNS-material | 8 | 0.9953 | |
| BCN-material | 6 | 0.9975 | |
| GNP-material | 4 | 0.9999 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Seiner, H.; Janovská, M.; Koller, M.; Sedlák, P.; Seinerová, K.; Loganathan, A.; Agarwal, A. Ultrasonic Characterization of Nanoparticle-Based Ceramics Fabricated by Spark-Plasma Sintering. Ceramics 2021, 4, 135-147. https://doi.org/10.3390/ceramics4020012
Seiner H, Janovská M, Koller M, Sedlák P, Seinerová K, Loganathan A, Agarwal A. Ultrasonic Characterization of Nanoparticle-Based Ceramics Fabricated by Spark-Plasma Sintering. Ceramics. 2021; 4(2):135-147. https://doi.org/10.3390/ceramics4020012
Chicago/Turabian StyleSeiner, Hanuš, Michaela Janovská, Martin Koller, Petr Sedlák, Kateřina Seinerová, Archana Loganathan, and Arvind Agarwal. 2021. "Ultrasonic Characterization of Nanoparticle-Based Ceramics Fabricated by Spark-Plasma Sintering" Ceramics 4, no. 2: 135-147. https://doi.org/10.3390/ceramics4020012
APA StyleSeiner, H., Janovská, M., Koller, M., Sedlák, P., Seinerová, K., Loganathan, A., & Agarwal, A. (2021). Ultrasonic Characterization of Nanoparticle-Based Ceramics Fabricated by Spark-Plasma Sintering. Ceramics, 4(2), 135-147. https://doi.org/10.3390/ceramics4020012






