Diffusiophoresis of a Charged Soft Sphere in a Charged Spherical Cavity

Abstract

The quasi-steady diffusiophoresis of a soft particle composed of an uncharged hard sphere core and a uniformly charged porous surface layer in a concentric charged spherical cavity full of a symmetric electrolyte solution with a concentration gradient is analyzed. By using a regular perturbation method with small fixed charge densities of the soft particle and cavity wall, the linearized electrokinetic equations relevant to the fluid velocity field, electric potential profile, and ionic concentration distributions are solved. A closed-form formula for the diffusiophoretic (electrophoretic and chemiphoretic) velocity of the soft particle is obtained as a function of the ratios of the core-to-particle radii, particle-to-cavity radii, particle radius to the Debye screening length, and particle radius to the permeation length in the porous layer. In typical cases, the confining charged cavity wall significantly influences the diffusiophoresis of the soft particle. The fluid flow caused by the diffusioosmosis (electroosmosis and chemiosmosis) along the cavity wall can considerably change the diffusiophoretic velocity of the particle and even reverse its direction. In general, the diffusiophoretic velocity decreases with increasing core-to-particle radius ratios, particle-to-cavity radius ratios, and the ratio of the particle radius to the permeation length in the porous layer, but increases with increasing ratios of the particle radius to the Debye length.

1. Introduction

Diffusiophoresis refers to the motion of colloid particles under imposed solute concentration gradients [1,2,3,4,5,6,7,8] and provides the mechanisms for numerous applications in the characterization, separation, transport, and manipulation of particles in microfluidics [9,10,11,12,13,14] and layered two-dimensional nanocolloid/liquid crystals [15,16], as well as in the autonomous motion of micromotors [17,18,19]. In a nonionic solution, the particle interacts with solute molecules via the Van der Waals and dipole attractive forces, and diffusiophoresis proceeds toward the regions where there is a higher solute concentration [20]. For the diffusiophoresis of a charged particle in an electrolyte solution, the particle–ion interaction is dominated by electrostatics and the range of its electric double layer with a thickness of the order of the Debye screening length [21]. In the past, the diffusiophoretic motion of a hard particle (which is impermeable to ionic fluids) [22,23], a porous particle (which is permeable) [24], and a soft particle (which has a hard core covered by a porous layer) [25,26,27,28] with an arbitrary electric double layer thickness were studied analytically (by assuming a weak applied electrolyte concentration gradient) and experimentally.

In various applications of diffusiophoresis, the colloid particles are rarely unbounded, and it is important to understand whether boundaries significantly affect the particle mobility [5,29]. In the limiting case of a very thin double layer, the normalized fluid velocity field around a hard sphere undergoing diffusiophoresis is identical to that of one undergoing electrophoresis, and the already extensively studied boundary effect on electrophoretic motion can be used to explain the effect on diffusiophoretic motion [10]. On the other hand, the boundary effect of diffusiophoresis is different from that of electrophoresis when a double layer polarization is incorporated. By using a boundary collocation technique, the diffusiophoretic motion of a hard sphere with a thin polarized double layer near one or two plates [30] and along the axis of a microtube [31,32] was examined.

The diffusiophoresis of a charged sphere in a charged spherical cavity can be used to model diffusiophoretic motions in lab-on-a-chip devices and dead-end pores involving self-regulated drug delivery [33,34]. In fact, the diffusiophoresis of a charged hard or porous sphere with a thin polarized or an arbitrary double layer situated at the center of a charged spherical cavity [35,36] and the diffusiophoresis of a charged soft sphere with an arbitrary double layer inside a nonconcentric uncharged spherical cavity [37] have been studied theoretically. However, the effect of a charged boundary on the diffusiophoretic motion of a soft particle has not been investigated. In this paper, the diffusiophoresis of a charged soft spherical particle inside a concentric charged spherical cavity with an arbitrary electric double layer thickness is analyzed. The fluid velocity field, electric potential profile, and ionic concentration distributions are determined as the power series of the small fixed charge densities of the soft sphere and cavity wall. An explicit formula for the diffusiophoretic mobility of the soft sphere is obtained as a function of the relevant parameters.

2. Electrokinetic Equations and Boundary Conditions

As shown in Figure 1, we consider the diffusiophoresis of a soft spherical particle of radius $a$, consisting of an uncharged hard sphere core of radius $r_0$ and a charged porous surface layer of thickness $a-r_0$, situated at the center of a charged spherical cavity of radius $b$ occupied by a symmetric electrolyte solution in a quasi-steady state. A linear electrolyte concentration profile $n^{\infty }(z)=n^{\infty }(0)+\left|\nabla n^{\infty }\right| z$ is imposed along the cavity wall with a constant gradient $\nabla n^{\infty }$ in the $z$ direction, and the induced particle velocity $U$ in this direction needing to be determined. The origin of the spherical coordinates $(r,\theta ,\varphi )$ is attached to the particle center (at $z=0$), and the problem is independent of $\varphi$ (symmetric about the $z$ axis).

The normalized electrolyte concentration gradient $\alpha =a\left|\nabla n^{\infty }\right|/n^{\infty }(0)$ is taken to be positive but small, so that the system deviates slightly from equilibrium. Therefore, the cationic and anionic concentration profiles $n_{+}(r,\theta )$ and $n_{-}(r,\theta )$, respectively; the dynamic pressure distribution $p(r,\theta )$; and the electrical potential distribution $\psi (r,\theta )$ can be decomposed into the following:

$$n_{\pm }=n_{\pm }^{(\mathrm{eq})}+\delta n_{\pm },$$

(1)

$$p=p^{(\mathrm{eq})}+\delta p,$$

(2)

$$\psi ={\psi }^{(\mathrm{eq})}+\delta \psi ,$$

(3)

where $n_{\pm }^{(\mathrm{eq})}(r)$, $p^{(\mathrm{eq})}(r)$, and ${\psi }^{(\mathrm{eq})}(r)$ are the equilibrium profiles of the ionic concentrations, dynamic pressure, and electrical potential, respectively, and $\delta n_{\pm }(r,\theta )$, $\delta p(r,\theta )$, and $\delta \psi (r,\theta )$ are the pertinent small perturbations.

The small perturbed quantities, $\delta n_{\pm }$, $\delta p$, and $\delta \psi$, and the fluid velocity $v(r,\theta )$ are governed by the linearized ionic continuity equations, modified Stokes–Brinkman equations, and Poisson–Boltzmann equation, respectively, as follows [25]:

$${\nabla }^2\delta {\mu }_{\pm }=\pm \frac{Ze}{kT}[\nabla \delta {\mu }_{\pm }\cdot \nabla {\psi }^{(\mathrm{eq})}-\frac{kT}{D_{\pm }}v\cdot \nabla {\psi }^{(\mathrm{eq})}],$$

(4)

$$[{\nabla }^2-{\lambda }^{2}h(r)]\nabla \times v=-\frac{\epsilon }{\eta }\nabla \times [{\nabla }^2\delta \psi \nabla {\psi }^{(\mathrm{eq})}+{\nabla }^2{\psi }^{(\mathrm{eq})}\nabla \delta \psi ],$$

(5)

$${\nabla }^2\delta \psi =\frac{n_0^{\infty }Ze}{\epsilon kT}\{(\delta {\mu }_{-}+Ze\delta \psi )\exp [\frac{Ze{\psi }^{(\mathrm{eq})}}{kT}]-(\delta {\mu }_{+}-Ze\delta \psi )\exp [-\frac{Ze{\psi }^{(\mathrm{eq})}}{kT}]\}.$$

(6)

In the previous equations, the ionic electrochemical potential energy perturbations are as follows:

$$\delta {\mu }_{\pm }=\frac{kT}{n_{\pm }^{(\mathrm{eq})}}\delta n_{\pm }\pm Ze\delta \psi$$

(7)

Furthermore, ${\lambda }^{-1}$ is the flow penetration length or the square root of the permeability in the porous surface layer of the soft sphere; $D_{\pm }$ are the ionic diffusivities in the fluid; $Z$ is the valence of the symmetric electrolyte (which is positive); $\eta$ and $\epsilon$ are the viscosity and dielectric permittivity, respectively, of the fluid; and $h(r)$ equals unity if $r_0\le r\le a$ and zero otherwise. The pressure term in Equation (5) disappears owing to the application of a curl to the momentum equation; the fluid velocity $v$ also satisfies the continuity equation; and the constants $D_{\pm }$, $\eta$, and $\epsilon$ inside and outside the porous layer are considered to be the same.

The boundary conditions of the small perturbed quantities along the interface between the hard sphere core and the porous surface layer and at the particle surface are as follows [25]:

$$r=r_0: e_r\cdot \nabla \delta \psi =0, e_r\cdot \nabla \delta {\mu }_{\pm }=0, v=0,$$

(8)

$$r=a: \delta \psi , \nabla \delta \psi , \delta {\mu }_{\pm }, \nabla \delta {\mu }_{\pm }, v, \mathrm{and} e_r\cdot \tau \mathrm{are} \mathrm{continuous},$$

(9)

where $\tau$ is the hydrodynamic stress tensor of the fluid, $e_r$ is the unit vector in the $r$ direction, and Equation (8) takes a reference frame traveling with the soft particle.

The boundary conditions at the cavity wall are as follows [35]:

$$r=b: \delta \psi =-\frac{kT}{Ze}\beta \alpha \frac{r}{a}\cos \theta ,$$

(10)

$$\delta {\mu }_{\pm }=kT(1\mp \beta )\alpha \frac{r}{a}\cos \theta , \mathrm{if} a\le r\le b,$$

(11)

$$v=-U{e}_z,$$

(12)

where $\alpha =a\left|\nabla n^{\infty }\right|/n^{\infty }(0)$, $\beta =(D_{+}-D_{-})/(D_{+}+D_{-})$, and $e_z$ is the unit vector in the $z$ direction. Equations (10) and (11) for the induced perturbed electrical potential and the ionic electrochemical potential energies result from the electrolyte concentration gradient imposed along the cavity wall and the equality of the anion and cation fluxes in the particle-free fluid. Equation (10c) for the stationary cavity also takes the same reference frame as Equation (8).

3. Solution of the Diffusiophoretic Velocity

The equilibrium electrical potential profile of a soft spherical particle, whose porous surface layer has a constant space charge density $Q$, situated at the center of a spherical cavity, having a constant surface charge density $\sigma$, satisfying the continuity of electrical potential and current at the particle surface ($r=a$) and the Gauss condition at the hard core surface ($r=r_0$) and cavity wall ($r=b$), can be obtained as follows [38]

$${\psi }^{(\mathrm{eq})}={\psi }_{\mathrm{eq}01}(r)\overline{Q}+{\psi }_{\mathrm{eq}10}(r)\overline{\sigma }+\mathrm{O}({\overline{Q}}^3,{\overline{Q}}^2\overline{\sigma },\overline{Q}{\overline{\sigma }}^2,{\overline{\sigma }}^3),$$

(13)

where

$${\psi }_{\mathrm{eq}01}(r)=\frac{kT{\mathrm{e}}^{-\kappa r}}{2ZeA\kappa r}\{[{\mathrm{e}}^{\kappa a}(\kappa a-1)(\kappa r_0+1)-{\mathrm{e}}^{\kappa (2r_0-a)}(\kappa a+1)(\kappa r_0-1)][{\mathrm{e}}^{2\kappa b}(\kappa b-1)$$

$$+{\mathrm{e}}^{2\kappa r}(\kappa b+1)]\} \mathrm{for} a<r<b,$$

(14)

$${\psi }_{\mathrm{eq}01}(r)=\frac{kT{\mathrm{e}}^{\kappa (2b-a+2r_0-r)}}{2ZeA\kappa r}\{\frac{1}{A}+{\mathrm{e}}^{\kappa (a-2b)}(\kappa b+1)(\kappa r_0-1)[{\mathrm{e}}^{\kappa a}(\kappa a-1)-2{\mathrm{e}}^{\kappa r}\kappa r]$$

$$-{\mathrm{e}}^{\kappa (r-2r_0)}(\kappa b-1)(\kappa r_0+1)[{\mathrm{e}}^{\kappa r}(\kappa a+1)-2{\mathrm{e}}^{\kappa a}\kappa r]+{\mathrm{e}}^{2\kappa (a-b-r_0+r)}(\kappa a-1)$$

$$\times (\kappa b+1)(\kappa r_0+1)+\kappa [b-r_0(\kappa b-1)-a(\kappa b-1)(\kappa r_0-1)]\} \mathrm{for} r_0<r<a,$$

(15)

$${\psi }_{\mathrm{eq}10}(r)=\frac{2kT{(\kappa b)}^2{\mathrm{e}}^{\kappa (b+r_0)}}{ZeA\kappa r}\{\kappa r_0\cosh [\kappa (r-r_0)]+\sinh [\kappa (r-r_0)]\},$$

(16)

$$A={\mathrm{e}}^{2\kappa b}(\kappa b-1)(\kappa r_0+1)-{\mathrm{e}}^{2\kappa r_0}(\kappa b+1)(\kappa r_0-1),$$

(17)

Both $\overline{Q}=ZeQ/\epsilon {\kappa }^{2}kT$ and $\overline{\sigma }=Ze\sigma /\epsilon \kappa kT$ are dimensionless, and $\kappa ={(2Z^2{e}^2{n}_0^{\infty }/\epsilon kT)}^{1/2}$ is the Debye screening parameter. For the case of a symmetric electrolyte, the second-order terms $\mathrm{O}({\overline{Q}}^2,\overline{Q}\overline{\sigma },{\overline{\sigma }}^2)$ in Equation (11) disappear.

The substitution of Equations (11)–(13) into the Gauss condition at $r=b$ yields a relation between the surface charge density $\sigma$ and the zeta potential $\zeta$ of the cavity wall:

$$\sigma =\{(1-{\kappa }^{2}a{r}_0)\sinh [\kappa (a-r_0)]-\kappa (a-r_0)\cosh [\kappa (a-r_0)]\}BQ$$

$$+\epsilon {\kappa }^2\{({\kappa }^{2}b{r}_0-1)\sinh [\kappa (b-r_0)]+\kappa (b-r_0)\cosh [\kappa (b-r_0)]\}B\zeta ,$$

(18)

where

$$B={\{{\kappa }^{2}b\sinh [\kappa (b-r_0)]+{\kappa }^{3}b{r}_0\cosh [\kappa (b-r_0)]\}}^{-1}.$$

(19)

Namely, after the substitution of Equation (15), Equation (11) is also valid for the solution of ${\psi }^{(\mathrm{eq})}$ in the case of a cavity wall with a constant zeta potential.

When the parameters $\overline{Q}$ and $\overline{\sigma }$ are small, in order to solve for the perturbed quantities $\delta {\mu }_{\pm }$, $\delta \psi$, $v_r$, $v_{\theta }$, and $\delta p$ with the diffusiophoretic velocity $U$ of the soft spherical particle, these variables can be expressed as power expansions of $\overline{Q}$ and $\overline{\sigma }$, such as the following:

$$U=U_{01}\overline{Q}+U_{10}\overline{\sigma }+U_{02}{\overline{Q}}^2+U_{11}\overline{Q}\overline{\sigma }+U_{20}{\overline{\sigma }}^2+\cdot \cdot \cdot ,$$

(20)

where the to-be-determined coefficients $U_{01}$, $U_{10}$, $U_{02}$, $U_{11}$, $U_{20}$, etc. do not depend on $\overline{Q}$ and $\overline{\sigma }$ but are dependent on the core-to-particle radius ratio $r_0/a$, the particle-to-cavity radius ratio $a/b$, the ratio of the particle radius to the permeation length in the porous layer $\lambda a$, and the electrokinetic particle radius $\kappa a$. There are zeroth-order terms of $\overline{Q}$ and $\overline{\sigma }$ in the expansions of $\delta \psi$ and $\delta {\mu }_{\pm }$, as shown in Equations (10a) and (10b). The substitution of these expansions and Equation (11) into Equations (4)–(10) yields results for $\delta \psi$, $\delta {\mu }_{\pm }$ (to the orders $\overline{Q}$ and $\overline{\sigma }$), $v_r$, $v_{\theta }$, and $\delta p$ (to the orders ${\overline{Q}}^2$, $\overline{Q}\overline{\sigma }$, and ${\overline{\sigma }}^2$) as follows:

$$\delta \psi =\frac{kT}{Ze}\alpha [-\beta F_{\psi 00}+F_{\psi 01}\overline{Q}+F_{\psi 10}\overline{\sigma }]\cos \theta ,$$

(21)

$$\delta {\mu }_{\pm }=kT(1\mp \beta )\alpha [F_{\mu 00}\mp F_{\mu 01}\overline{Q}\mp F_{\mu 10}\overline{\sigma }]\cos \theta ,$$

(22)

$$v_r=[(U_{01}{F}_{00r}-\frac{kT}{\eta a^2}\beta \alpha F_{01r})\overline{Q}+(U_{10}{F}_{00r}-\frac{kT}{\eta a^2}\beta \alpha F_{10r})\overline{\sigma }+(U_{02}{F}_{00r}+\frac{kT}{\eta a^2}\alpha F_{02r}){\overline{Q}}^2$$

$$+(U_{11}{F}_{00r}+\frac{kT}{\eta a^2}\alpha F_{11r})\overline{Q}\overline{\sigma }+(U_{20}{F}_{00r}+\frac{kT}{\eta a^2}\alpha F_{20r}){\overline{\sigma }}^2]\cos \theta ,$$

(23)

$$v_{\theta }=-\frac{\tan \theta }{2r}\frac{\partial }{\partial r}(r^2{v}_r),$$

(24)

$$\delta p=\frac{\eta }{a}\{(U_{01}{F}_{p00}-\frac{kT}{\eta a^2}\beta \alpha F_{p01}-\frac{\epsilon kT}{\eta Ze}{\kappa }^{2}a\beta \alpha {\psi }_{\mathrm{eq}01}{F}_{\psi 00})\overline{Q}$$

$$+(U_{10}{F}_{p00}-\frac{kT}{\eta a^2}\beta \alpha F_{p10}-\frac{\epsilon kT}{\eta Ze}{\kappa }^{2}a\beta \alpha {\psi }_{\mathrm{eq}10}{F}_{\psi 00})\overline{\sigma }$$

$$+(U_{02}{F}_{p00}+\frac{kT}{\eta a^2}\alpha F_{p02}+\frac{\epsilon kT}{\eta Ze}{\kappa }^{2}a\alpha {\psi }_{\mathrm{eq}01}{F}_{\psi 01}){\overline{Q}}^2$$

$$+[U_{11}{F}_{p00}+\frac{kT}{\eta a^2}\alpha F_{p11}+\frac{\epsilon kT}{\eta Ze}{\kappa }^{2}a\alpha ({\psi }_{\mathrm{eq}01}{F}_{\psi 10}+{\psi }_{\mathrm{eq}10}{F}_{\psi 01})]\overline{Q}\overline{\sigma }$$

$$+(U_{20}{F}_{p00}+\frac{kT}{\eta a^2}\alpha F_{p20}+\frac{\epsilon kT}{\eta Ze}{\kappa }^{2}a\alpha {\psi }_{\mathrm{eq}10}{F}_{\psi 10}){\overline{\sigma }}^2\}\cos \theta ,$$

(25)

where the dimensionless functions $F_{\psi ij}(r)$, $F_{\mu ij}(r)$, $F_{ijr}(r)$, and $F_{pij}(r)$ with i and j equal to 0, 1, and 2 are given by Equations (A1)–(A4) and (A9)–(A11) in Appendix A.

The electrical force acting on the soft spherical particle can be expressed as follows [38]

$$F_{\mathrm{e}}=2\mathsf{\pi }{a}^2\epsilon {\displaystyle {\int }_0^{\mathsf{\pi }}{[\nabla \delta \psi \nabla {\psi }^{(\mathrm{eq})}]}_{r=a} \cdot e_r\sin \theta \mathrm{d}\theta }.$$

(26)

The substitution of Equations (11) and (18) into the previous equation results in this force to the second orders,

$$F_{\mathrm{e}}=\frac{4\mathsf{\pi }kT\alpha a\epsilon }{3Ze}\{-\beta [(2F_{\psi 00}+a\frac{\mathrm{d}{F}_{\psi 00}}{\mathrm{d}r})\frac{\mathrm{d}{\psi }_{\mathrm{eq}01}}{\mathrm{d}r}\overline{Q}+(2F_{\psi 00}+a\frac{\mathrm{d}{F}_{\psi 00}}{\mathrm{d}r})\frac{\mathrm{d}{\psi }_{\mathrm{eq}10}}{\mathrm{d}r}\overline{\sigma }]+(2F_{\psi 01}+a\frac{\mathrm{d}{F}_{\psi 01}}{\mathrm{d}r})\frac{\mathrm{d}{\psi }_{\mathrm{eq}01}}{\mathrm{d}r}{\overline{Q}}^2$$

$$+[(2F_{\psi 01}+a\frac{\mathrm{d}{F}_{\psi 01}}{\mathrm{d}r})\frac{\mathrm{d}{\psi }_{\mathrm{eq}10}}{\mathrm{d}r}+(2F_{\psi 10}+a\frac{\mathrm{d}{F}_{\psi 10}}{\mathrm{d}r})\frac{\mathrm{d}{\psi }_{\mathrm{eq}01}}{\mathrm{d}r}]\overline{Q}\overline{\sigma }+(2F_{\psi 10}+a\frac{\mathrm{d}{F}_{\psi 10}}{\mathrm{d}r})\frac{\mathrm{d}{\psi }_{\mathrm{eq}10}}{\mathrm{d}r}{\overline{\sigma }}^2{\}}_{r=a}{e}_z$$

(27)

The drag force exerted by the fluid on the soft spherical particle can be expressed as follows:

$$F_{\mathrm{h}}=2\mathsf{\pi }{a}^2{\displaystyle {\int }_0^{\mathsf{\pi }}{\{-\delta pI+\eta [\nabla v+{(\nabla v)}^{\mathrm{T}}]\}}_{r=a}\cdot e_r\sin \theta \mathrm{d}\theta },$$

(28)

where $I$ is the unit dyadic. Substituting Equation (20) into the previous equation, we obtain the following:

$$F_{\mathrm{h}}=-4\mathsf{\pi }\{[\eta a{U}_{01}{C}_{002}-\frac{kT}{a}\alpha \beta C_{012}-\frac{\epsilon kT}{3Ze}{(\kappa a)}^2\alpha \beta F_{\psi 00}{\psi }_{\mathrm{eq}01}]\overline{Q}.$$

$$+[\eta a{U}_{10}{C}_{002}-\frac{kT}{a}\alpha \beta C_{102}-\frac{\epsilon kT}{3Ze}{(\kappa a)}^2\alpha \beta F_{\psi 00}{\psi }_{\mathrm{eq}10}]\overline{\sigma }$$

$$+[\eta a{U}_{02}{C}_{002}+\frac{kT}{a}\alpha C_{022}+\frac{\epsilon kT}{3Ze}{(\kappa a)}^2\alpha F_{\psi 01}{\psi }_{\mathrm{eq}01}]{\overline{Q}}^2$$

$$+[\eta a{U}_{11}{C}_{002}+\frac{kT}{a}\alpha C_{112}+\frac{\epsilon kT}{3Ze}{(\kappa a)}^2\alpha (F_{\psi 01}{\psi }_{\mathrm{eq}10}+F_{\psi 10}{\psi }_{\mathrm{eq}01})]\overline{Q}\overline{\sigma }$$

$$+[\eta a{U}_{20}{C}_{002}+\frac{kT}{a}\alpha C_{202}+\frac{\epsilon kT}{3Ze}{(\kappa a)}^2\alpha F_{\psi 10}{\psi }_{\mathrm{eq}10}]{\overline{\sigma }}^2{\}}_{r=a}{e}_z,$$

(29)

where the dimensionless coefficients $C_{002}$, $C_{012}$, $C_{102}$, $C_{022}$, $C_{112}$, and $C_{202}$ are given by Equations (A1)–(A4).

Applying the constraint that the total force on the diffusiophoretic soft sphere is zero to the summation of Equations (22) and (24), we obtain the following:

$$U_{01}=\frac{Ze\beta U^{*}}{3\epsilon akT{C}_{002}}{\{3Ze{C}_{012}+\epsilon a^2[{\kappa }^{2}a{F}_{\psi 00}{\psi }_{\mathrm{eq}01}-(2F_{\psi 00}+a\frac{\mathrm{d}{F}_{\psi 00}}{\mathrm{d}r})\frac{\mathrm{d}{\psi }_{\mathrm{eq}01}}{\mathrm{d}r}]\}}_{r=a},$$

(30)

$$U_{10}=\frac{Ze\beta U^{*}}{3\epsilon akT{C}_{002}}{\{3Ze{C}_{102}+\epsilon a^2{\kappa }^{2}a{F}_{\psi 00}{\psi }_{\mathrm{eq}10}-(2F_{\psi 00}+a\frac{\mathrm{d}{F}_{\psi 00}}{\mathrm{d}r})\frac{\mathrm{d}{\psi }_{\mathrm{eq}10}}{\mathrm{d}r}\}}_{r=a},$$

(31)

$$U_{02}=\frac{-Ze{U}^{*}}{3\epsilon akT{C}_{002}}{\{3Ze{C}_{022}+\epsilon a^2[{\kappa }^{2}a{F}_{\psi 01}{\psi }_{\mathrm{eq}01}-(2F_{\psi 01}+a\frac{\mathrm{d}{F}_{\psi 01}}{\mathrm{d}r})\frac{\mathrm{d}{\psi }_{\mathrm{eq}01}}{\mathrm{d}r}]\}}_{r=a},$$

(32)

$$U_{11}=\frac{-Ze{U}^{*}}{3\epsilon akT{C}_{002}}\{3Ze{C}_{112}+\epsilon a^2[{\kappa }^{2}a{F}_{\psi 10}{\psi }_{\mathrm{eq}01}+{\kappa }^{2}a{F}_{\psi 01}{\psi }_{\mathrm{eq}10}-(2F_{\psi 10}+a\frac{\mathrm{d}{F}_{\psi 10}}{\mathrm{d}r})\frac{\mathrm{d}{\psi }_{\mathrm{eq}01}}{\mathrm{d}r}$$

$$-(2F_{\psi 01}+a\frac{\mathrm{d}{F}_{\psi 01}}{\mathrm{d}r})\frac{\mathrm{d}{\psi }_{\mathrm{eq}10}}{\mathrm{d}r}{]\}}_{r=a},$$

(33)

$$U_{02}=\frac{-Ze{U}^{*}}{3\epsilon akT{C}_{002}}{\{3Ze{C}_{202}+\epsilon a^2[{\kappa }^{2}a{F}_{\psi 10}{\psi }_{\mathrm{eq}10}-(2F_{\psi 10}+a\frac{\mathrm{d}{F}_{\psi 10}}{\mathrm{d}r})\frac{\mathrm{d}{\psi }_{\mathrm{eq}10}}{\mathrm{d}r}]\}}_{r=a},$$

(34)

where

$$U^{*}=\frac{\epsilon \alpha }{\eta a}{(\frac{kT}{Ze})}^2$$

(35)

which is a characteristic particle velocity. After the substitution of Equation (15), Equation (17) is also valid for the diffusiophoretic velocity of the particle in the case of a cavity wall with a constant zeta potential. Equations (25) and (26) for the limit $r_0=0$ reduce to the diffusiophoretic velocity of a charged porous spherical particle within a charged spherical cavity obtained earlier, and analytical expressions are available in some limiting cases [36].

In Equation (17) for the diffusiophoretic velocity of the soft spherical particle within the spherical cavity, the first-order terms $\mathrm{O}(\overline{Q},\overline{\sigma })$ and second-order terms $O({\overline{Q}}^2,\overline{Q}\overline{\sigma },{\overline{\sigma }}^2)$ denote the contributions from electrophoresis that are caused by the induced electric field in Equation (10) and chemiphoresis, respectively. The fixed charge at the cavity wall changes the particle motion through the wall-corrected electrical potential distribution and diffusioosmosis-induced (electroosmosis-induced and chemiosmosis-induced) recirculating flows generated by the interaction of the imposed electrolyte concentration gradient with the electrical double layer adjacent to the wall. The terms $U_{01}\overline{Q}+U_{02}{\overline{Q}}^2$ and $U_{10}\overline{\sigma }+U_{20}{\overline{\sigma }}^2$ are the diffusiophoretic velocity of a charged soft particle in an uncharged cavity ($\sigma =0$) and the translational velocity of an uncharged soft particle ($Q=0$) in a charged cavity induced by diffusioosmosis, respectively. Equation (25) agrees with the electrophoretic velocity of a soft spherical particle within a charged cavity available in the literature [38].

4. Results and Discussion

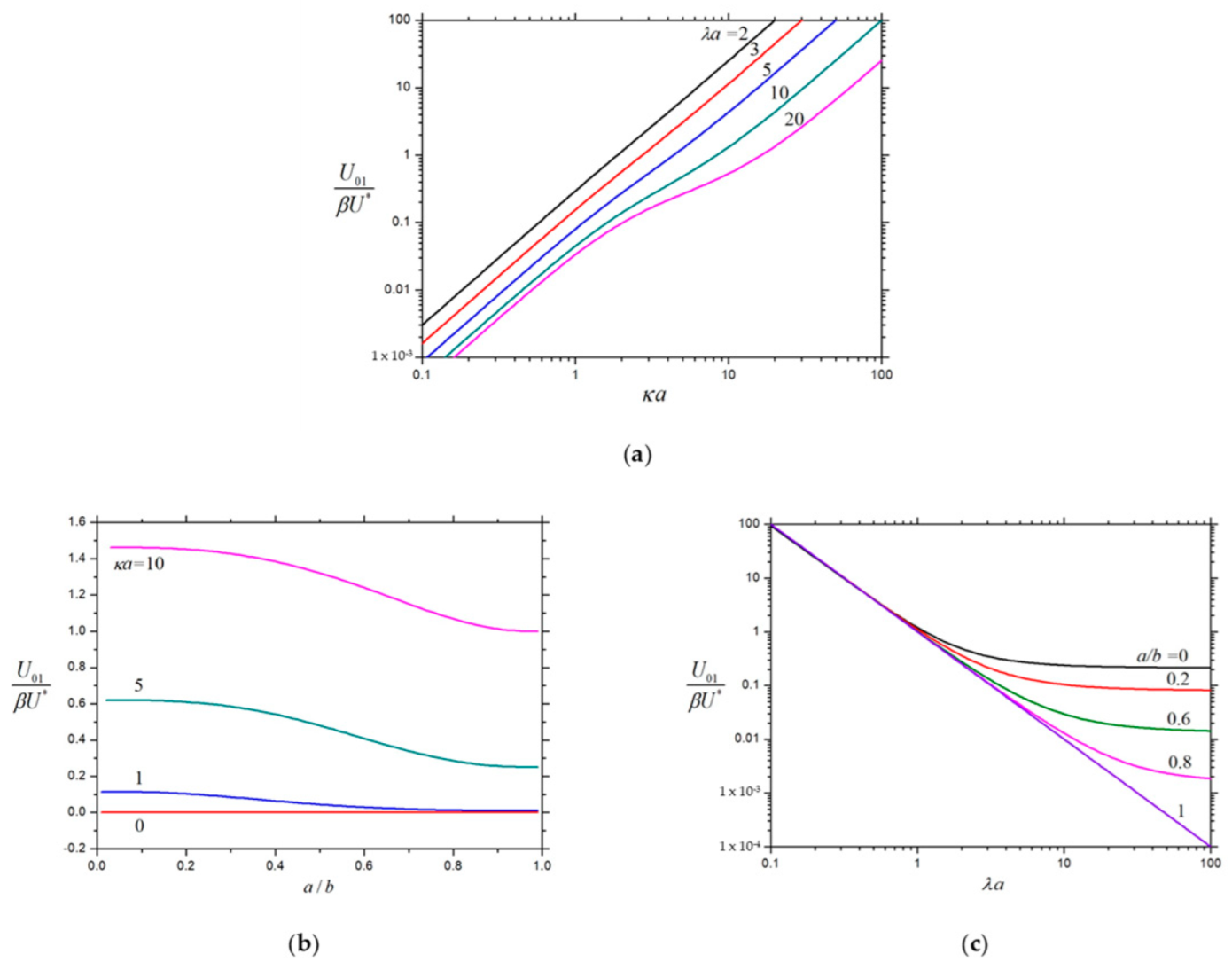
The analytical formulae for the diffusiophoretic velocity of a charged soft spherical particle within a concentric charged spherical cavity are obtained in Equations (17) and (25)–(27), and the graphical results will be given here. The normalized first-order (electrophoretic) velocities $U_{01}/\beta U^{*}$ and $U_{10}/\beta U^{*}$ for the special case of a porous spherical particle ($r_0=0$) within a concentric charged cavity as calculated from Equation (25) are plotted in Figure 2 and Figure 3, respectively, as functions of the hydrodynamic resistance parameter $\lambda a$, the particle-to-cavity radius ratio $a/b$, and the electrokinetic particle radius $\kappa a$. These normalized velocities are always positive; therefore, the sign of the product $\beta Q$ determines the direction of the electrophoresis and the sign of $\beta \sigma$ determines the direction of the contribution from the electroosmosis at the cavity wall to the particle velocity. For the fixed values of $a/b$ and $\lambda a$, both $U_{01}/\beta U^{*}$ and $U_{10}/\beta U^{*}$ are the monotonically increasing functions of $\kappa a$ from zero at $\kappa a=0$. For the specified values of $a/b$ and $\kappa a$, both $U_{01}/\beta U^{*}$ and $U_{10}/\beta U^{*}$ are the monotonically decreasing functions of $\lambda a$ as expected, but $U_{01}/\beta U^{*}$ may strongly depend on $\lambda a$, while $U_{10}/\beta U^{*}$ is only weakly dependent on $\lambda a$. For fixed $\lambda a$ and $\kappa a$, both $U_{01}/\beta U^{*}$ and $U_{10}/\beta U^{*}$ are the monotonically decreasing functions of $a/b$ as expected, and $U_{10}/\beta U^{*}$ equals 1 and 0 if $a/b$ equals 0 and 1, respectively.

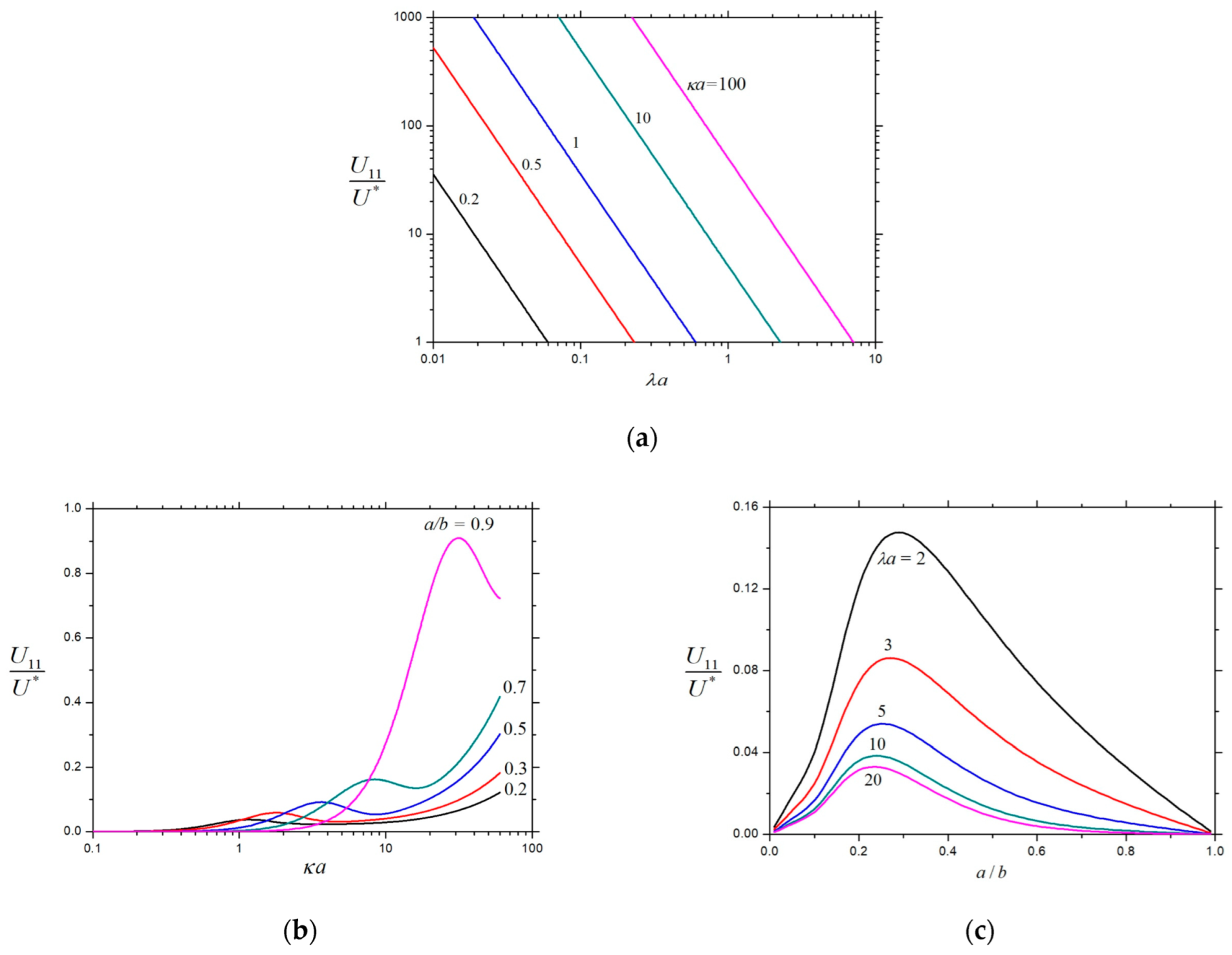
The normalized net first-order velocity $U_1/\overline{Q}\beta U^{*}$ (with $U_1=U_{01}\overline{Q}+U_{10}\overline{\sigma }$) of a charged porous particle inside a charged cavity is plotted versus the fixed charge density ratio $\overline{\sigma }/\overline{Q}$ (equaling $\kappa \sigma /Q$) in Figure 4 (in straight lines with the slope $U_{10}/\beta U^{*}$) for different values of $a/b$, $\lambda a$, and $\kappa a$. The electroosmotic flow at the cavity wall enhances/reduces this electrophoretic velocity if the fixed charge densities $\overline{Q}$ and $\overline{\sigma }$ are in the same/opposite signs. If the magnitude of $\overline{\sigma }/\overline{Q}$ is large, the wall effect can be significant. When the value of $\overline{\sigma }/\overline{Q}$ is negative and the magnitude is great, the velocity direction of the confined particle may be opposite to the direction of the electrophoresis in an unbounded fluid. The magnitude of $U_1/\overline{Q}\beta U^{*}$ in general decreases with the increases in $\lambda a$ and $a/b$, but increases with an increase in $\kappa a$.

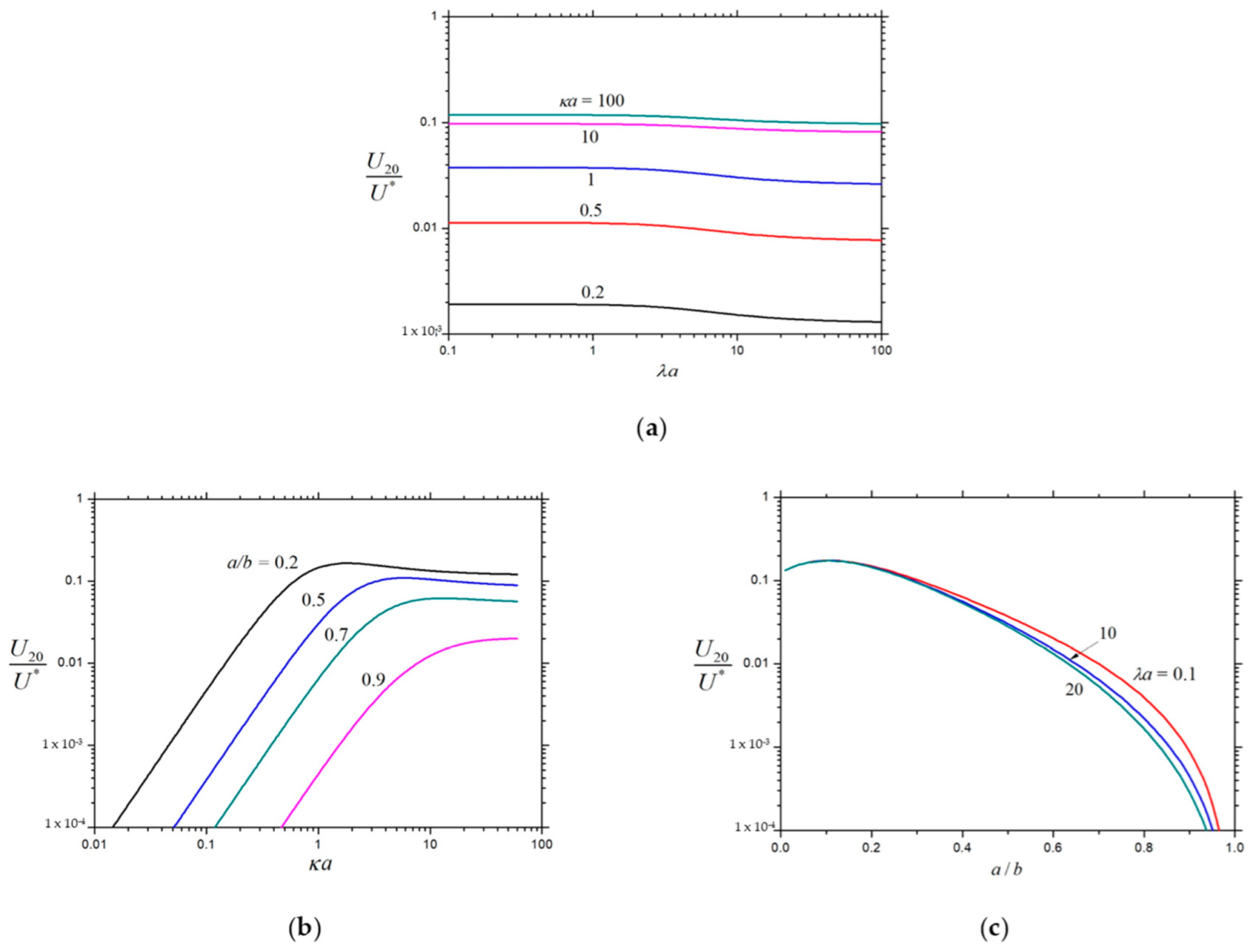
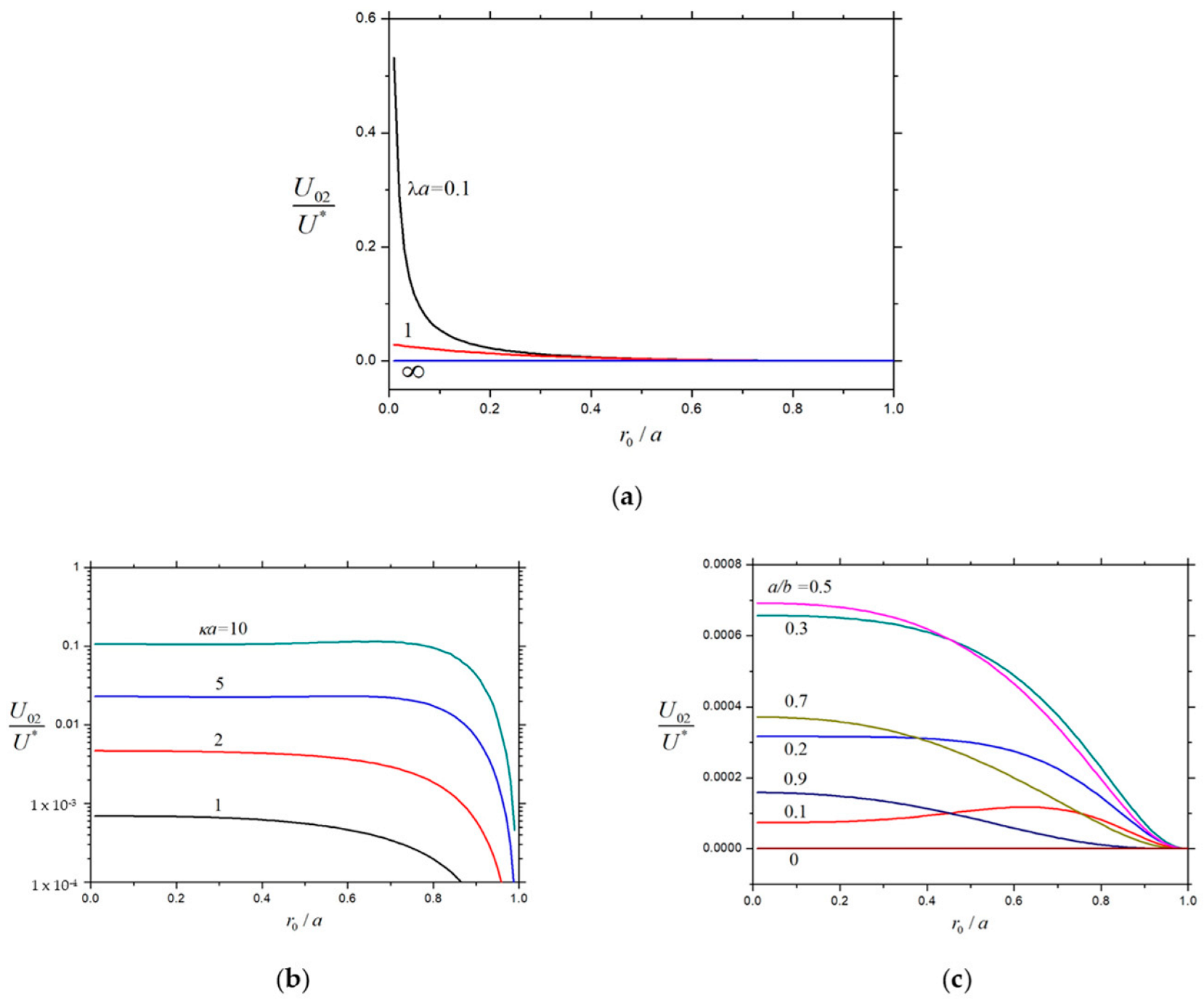
The normalized second-order velocities $U_{02}/U^{*}$, $U_{11}/U^{*}$, and $U_{20}/U^{*}$ of a charged porous sphere inside a charged cavity caused by the chemiphoretic and wall-induced chemiosmotic effects as calculated from Equation (26) are plotted in Figure 5, Figure 6 and Figure 7, respectively, as functions of the parameters $a/b$, $\lambda a$, and $\kappa a$. All these second-order velocities are monotonically decreasing functions of $\lambda a$ as expected, but none of them is a monotonic function of $a/b$ (local extrema appear at moderate values of $a/b$), keeping the other parameters unchanged. For the specified values of $a/b$ and $\lambda a$, the value of $U_{02}/U^{*}$ increases monotonically with an increase in $\kappa a$, whereas $U_{11}/U^{*}$ and $U_{20}/U^{*}$ may not be monotonic functions of $\kappa a$. As expected, $U_{02}/U^{*}=U_{11}/U^{*}=U_{20}/U^{*}=0$ in the limits $a/b=1$ and $\kappa a=0$, while $U_{11}/U^{*}=0$ in the limit $a/b=0$. For the given values of $a/b$ and $\kappa a$, both $U_{11}/U^{*}$ and $U_{02}/U^{*}$ are strongly dependent on $\lambda a$ (inversely proportional to ${\lambda }^2{a}^2$ if $\lambda a$ is smaller than about 10), while $U_{20}/U^{*}$ only weakly depend on $\lambda a$.

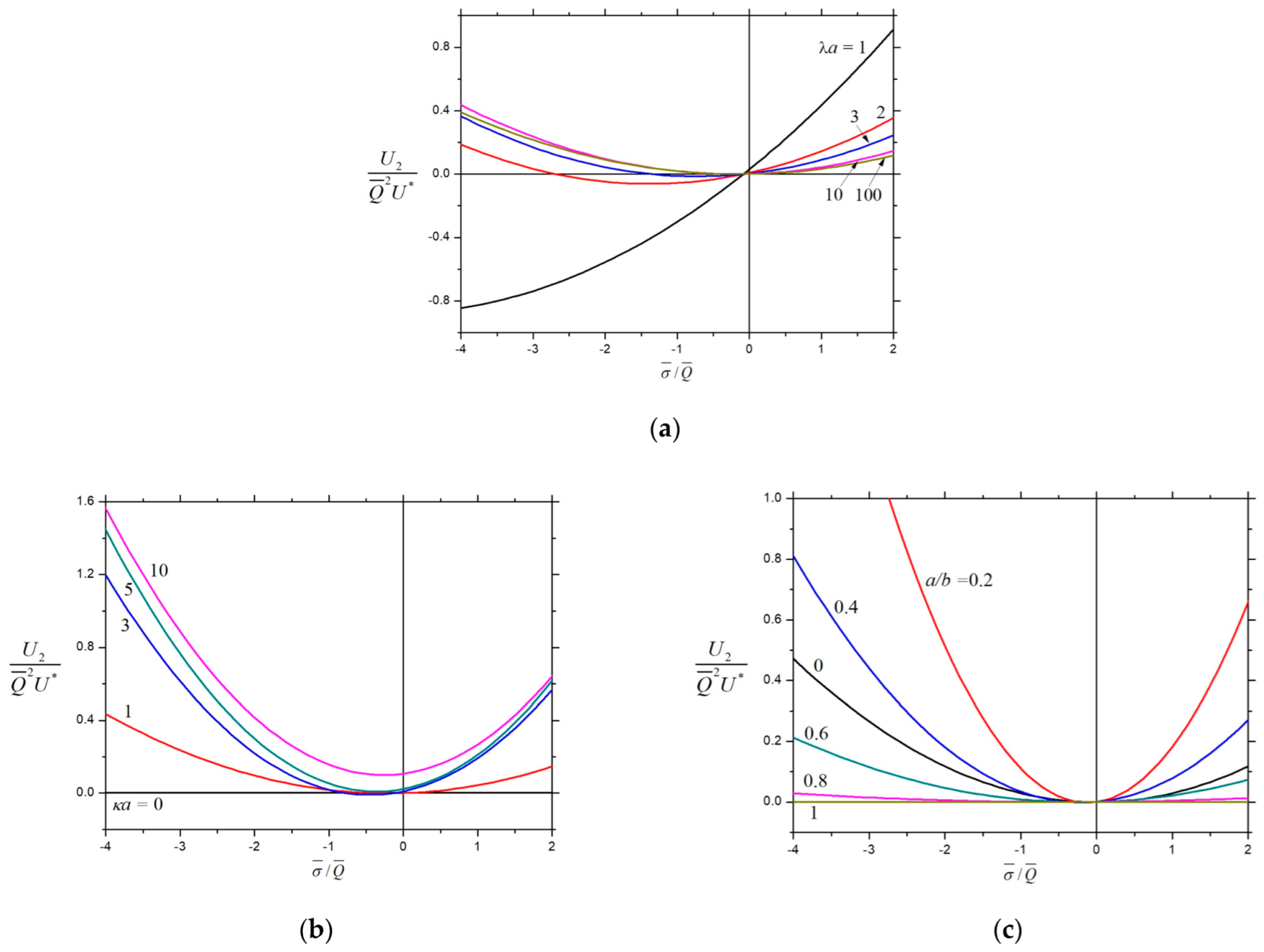
The normalized net second-order velocity $U_2/{\overline{Q}}^2{U}^{*}$ (with $U_2=U_{02}{\overline{Q}}^2+U_{11}\overline{Q}\overline{\sigma }+U_{20}{\overline{\sigma }}^2$) of a charged porous particle inside a charged cavity is plotted versus the fixed charge density ratio $\overline{\sigma }/\overline{Q}$ in Figure 8 (in parabolic curves that concave upward) for various values of the parameters $a/b$, $\lambda a$, and $\kappa a$. For the given values of $a/b$, $\lambda a$, and $\kappa a$, this velocity may reverse its direction twice as $\overline{\sigma }/\overline{Q}$ changes, due to the combined effects of chemiphoresis and wall-induced chemiosmosis. If the magnitude of $\overline{\sigma }/\overline{Q}$ is large, the cavity wall effect on the motion of the porous sphere is substantial. The magnitude of $U_2/{\overline{Q}}^2{U}^{*}$ generally diminishes when $\lambda a$ and $a/b$ increase, but increases with an increasing $\kappa a$.

Figure 8 shows the various values of the parameters $a/b$, $\lambda a$, and $\kappa a$, as well as the fixed charge densities $\overline{Q}$ and $\overline{\sigma }$. For the diffusiophoresis of a porous particle in a symmetric electrolyte whose cation and anion have different diffusivities ($\beta =-0.2$, like the aqueous solution of NaCl), the plots of $U/U^{*}$ against $\overline{\sigma }$ with $\overline{Q}=1$ and the different values of $a/b$, $\lambda a$, and $\kappa a$ are given in Figure 9 (a combination of Figure 4 and Figure 8), where the contributions from electrophoresis and chemiphoresis, as well as from wall-induced electroosmosis and chemiosmosis, are included. Furthermore, for the fixed values of $a/b$, $\lambda a$, and $\kappa a$, the cavity wall effect is significant as the magnitude of $\overline{\sigma }/\overline{Q}$ is large, and the particle velocity may reverse twice in its direction when $\overline{\sigma }/\overline{Q}$ changes.

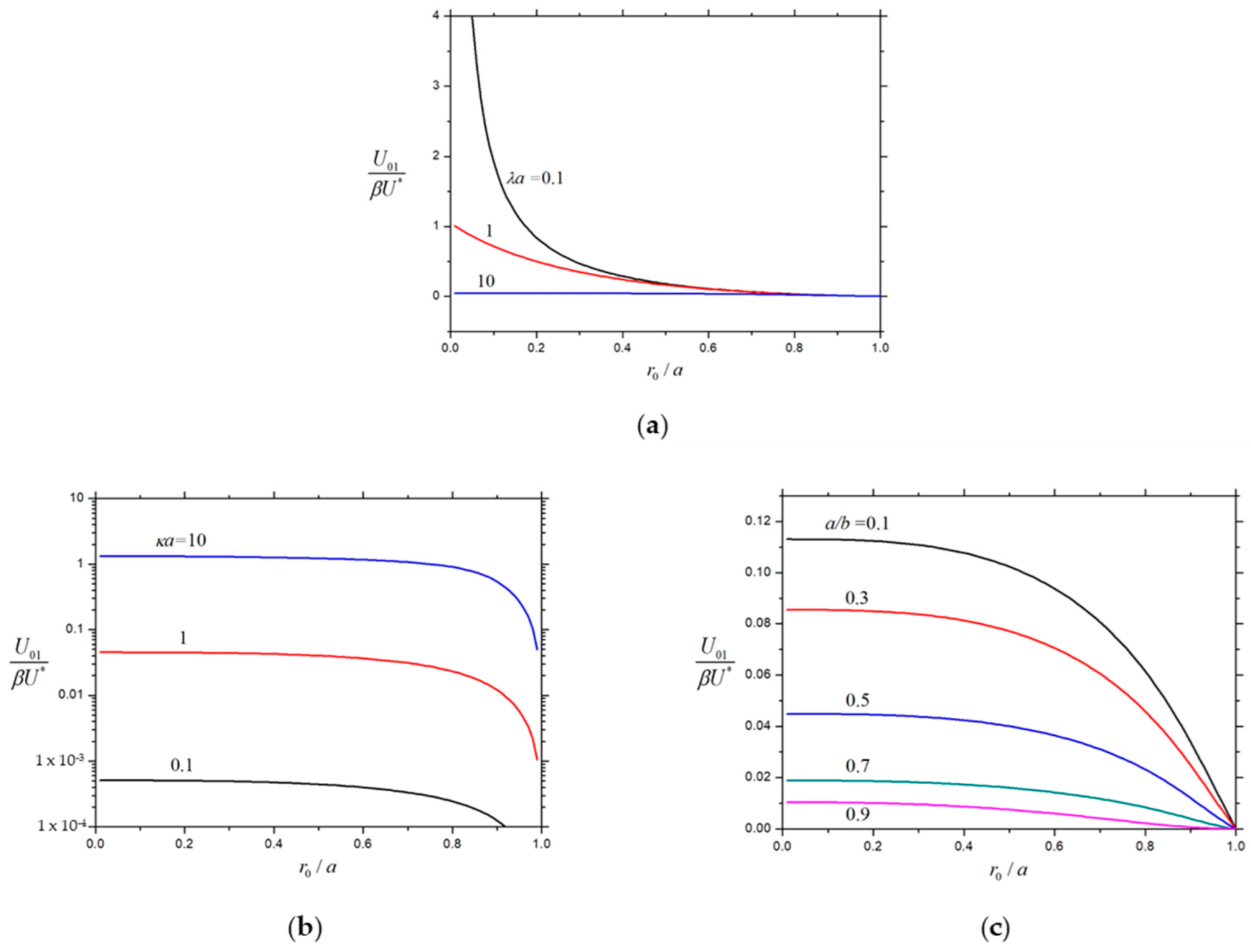
Having understood the effect of a concentric charged cavity on the diffusiophoretic velocity of a charged porous particle, we can examine the general case of a diffusiophoretic soft particle. The normalized first-order velocities $U_{01}/\beta U^{*}$ and $U_{10}/\beta U^{*}$ for a soft spherical particle within a concentric charged cavity as calculated from Equation (25) are plotted versus the core-to-particle radius ratio $r_0/a$ for different values of the hydrodynamic resistance parameter $\lambda a$, the particle-to-cavity radius ratio $a/b$, and the electrokinetic particle radius $\kappa a$ in Figure 10 and Figure 11, respectively. Likewise, these normalized velocities are always positive; the sign of the product $\beta Q$ determines the direction of the electrophoresis; and the sign of $\beta \sigma$ determines the direction of the wall-induced electroosmotic effect on the particle. Both $U_{01}/\beta U^{*}$ and $U_{10}/\beta U^{*}$ are monotonically increasing functions of $\kappa a$ from zero at $\kappa a=0$, monotonically decreasing functions of $\lambda a$, and monotonically decreasing functions of $a/b$ to zero at $a/b=1$, keeping the other parameters unchanged. For the specified values of $\lambda a$, $\kappa a$, and $a/b$, the normalized electrophoretic velocity $U_{01}/\beta U^{*}$ monotonically decreases with a rise in the radius ratio $r_0/a$, as expected, from the value for a charged porous sphere at $r_0/a=0$ to zero for an uncharged rigid (impermeable) sphere at $r_0/a=1$. On the other hand, the normalized velocity $U_{10}/\beta U^{*}$ of the soft particle caused by the electroosmotic effect of the charged cavity wall for the given values of $a/b$, $\lambda a$, and $\kappa a$ is generally not a monotonic function of $r_0/a$ and has a maximum at a relatively large value of $r_0/a$.

In Figure 12, the normalized net first-order velocity $U_1/\overline{Q}\beta U^{*}$ (with $U_1=U_{01}\overline{Q}+U_{10}\overline{\sigma }$) of a charged soft particle inside a charged cavity is plotted versus the fixed charge density ratio $\overline{\sigma }/\overline{Q}$ for the different values of $r_0/a$. Again, the electroosmotic effect of the cavity wall enhances/reduces this particle velocity if the fixed charge densities $\overline{Q}$ and $\overline{\sigma }$ are in the same/opposite signs. When the value of $\overline{\sigma }/\overline{Q}$ is negative and the magnitude is great, the velocity direction of the particle may be opposite to the direction of the electrophoresis in an unbounded fluid. The value of $U_1/\overline{Q}\beta U^{*}$ decreases with an increase in $r_0/a$, mainly due to the effect of $r_0/a$ on the electrophoretic velocity $U_{01}/\beta U^{*}$.

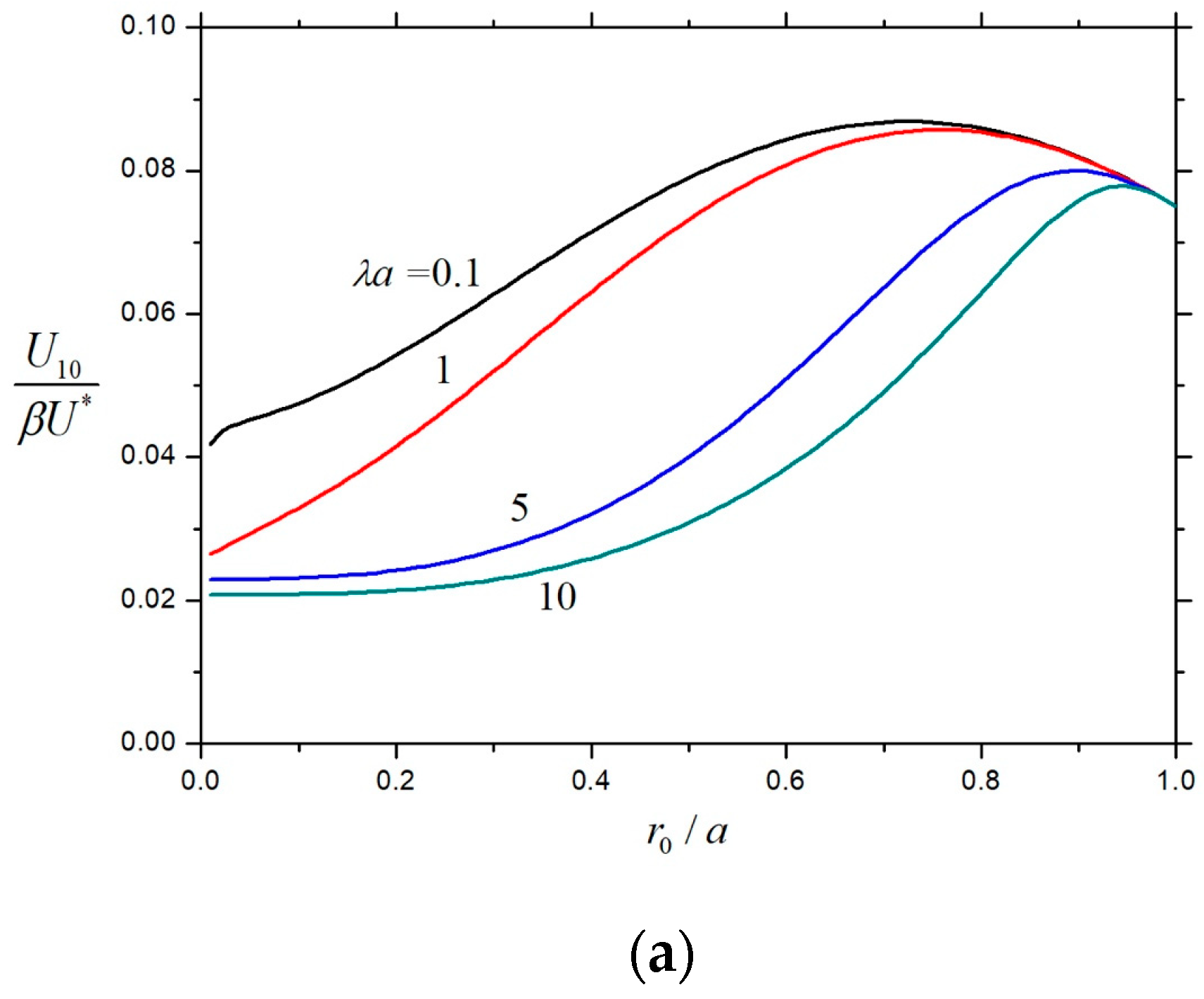
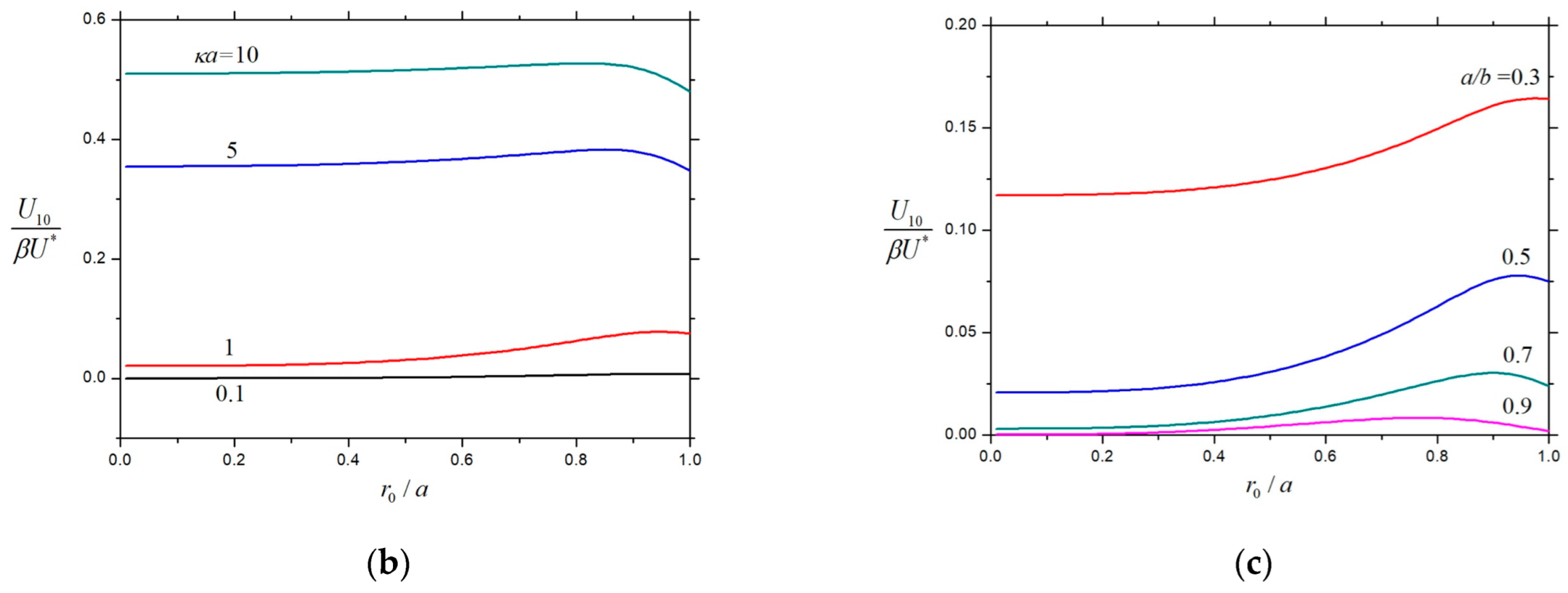
The normalized second-order velocities $U_{02}/U^{*}$, $U_{11}/U^{*}$, and $U_{20}/U^{*}$ of a charged soft sphere inside a charged cavity caused by the chemiphoretic and wall-induced chemiosmotic effects as calculated from Equation (26) are plotted versus the core-to-particle radius ratio $r_0/a$ in Figure 13, Figure 14 and Figure 15, respectively, for the different values of $a/b$, $\lambda a$, and $\kappa a$. Likewise, all these velocities are monotonically decreasing functions of $\lambda a$ that generally increase with an increasing $\kappa a$, but none of them depends monotonically on $a/b$ (local extrema appear at moderate values of $a/b$), keeping the other parameters unchanged. For the specified values of $a/b$, $\lambda a$, and $\kappa a$, the normalized velocities $U_{02}/U^{*}$ and $U_{11}/U^{*}$ generally decreases with a rise in the radius ratio $r_0/a$ from their values for a charged porous sphere at $r_0/a=0$ to zero for an uncharged rigid sphere at $r_0/a=1$. However, the normalized velocity $U_{20}/U^{*}$ of the soft particle caused by the chemiosmotic effect of the charged cavity wall for the given values of $a/b$, $\lambda a$, and $\kappa a$ is generally not a monotonic function of $r_0/a$.

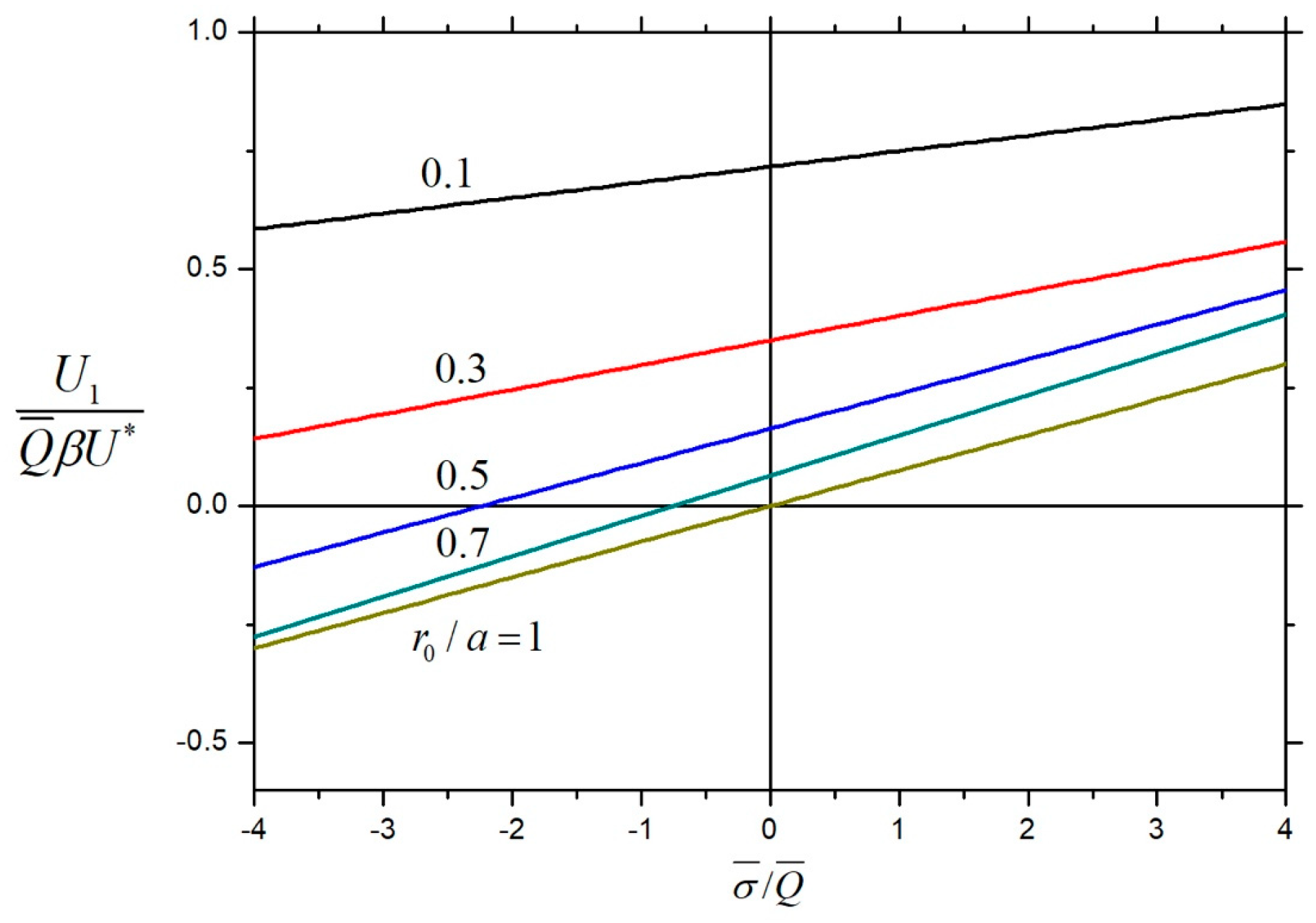
In Figure 16, the normalized net second-order velocity $U_2/{\overline{Q}}^2{U}^{*}$ (with $U_2=U_{02}{\overline{Q}}^2+U_{11}\overline{Q}\overline{\sigma }+U_{20}{\overline{\sigma }}^2$) of a charged soft particle inside a charged cavity is plotted versus the fixed charge density ratio $\overline{\sigma }/\overline{Q}$ for the various values of $r_0/a$. Furthermore, for the given values of $a/b$, $\lambda a$, and $\kappa a$, this velocity may reverse its direction twice as $\overline{\sigma }/\overline{Q}$ changes, due to the combined effects of chemiphoresis and wall-induced chemiosmosis. In general, the value of $U_2/{\overline{Q}}^2{U}^{*}$ decreases with an increasing $r_0/a$ if $\overline{\sigma }/\overline{Q}$ is positive, but it is not a monotonic function of $r_0/a$ if $\overline{\sigma }/\overline{Q}$ is negative.

5. Conclusions

The quasi-steady diffusiophoresis of a charged soft spherical particle at the center of a charged spherical cavity under an applied concentration gradient of a symmetric electrolyte is analyzed for the arbitrary values of the core-to-particle radius ratio $r_0/a$, the particle-to-cavity radius ratio $a/b$, the ratio of the particle radius to the permeation length in the porous surface layer $\lambda a$, and the ratio of the particle radius to the Debye length $\kappa a$. By using a regular perturbation method with small dimensionless fixed charge densities $\overline{Q}$ and $\overline{\sigma }$ of the porous surface layer and cavity wall, respectively, the linearized electrokinetic differential equations relevant to the fluid velocity field, electric potential profile, and ionic concentration distributions are solved. The balance of the electrostatic and hydrodynamic forces acting on the soft sphere results in an explicit formula, Equation (17) with Equations (25)–(27), for the diffusiophoretic velocity of the particle in terms of $r_0/a$, $a/b$, $\lambda a$, and $\kappa a$ up to the second orders of $\overline{Q}$ and $\overline{\sigma }$. The diffusioosmotic flow at the cavity wall can substantially change the particle velocity and even reverse its direction. The normalized electrophoretic and chemiphoretic velocity components $U_{01}/\beta U^{*}$ and $U_{02}/U^{*}$ (and also $U_{11}/U^{*}$) depend strongly on $\lambda a$, while the normalized electroosmosis-induced and chemiosmosis-induced velocity components $U_{10}/\beta U^{*}$ and $U_{20}/U^{*}$ are weak functions of $\lambda a$. The diffusiophoretic velocity generally decreases when $r_0/a$, $a/b$, and $\lambda a$ increase, but increases with an increasing $\kappa a$. The contributions to the particle velocity from the diffusioosmotic flow taking place along the charged cavity wall and from the wall-corrected diffusiophoretic force are equivalently important, and this diffusioosmotic flow can reverse the direction of the particle velocity.