Micellar Parameters of Aqueous Solutions of Tween 20 and 60 at Different Temperatures: Volumetric and Viscometric Study
Abstract
1. Introduction
2. Experimental Method
2.1. Materials and Methods
2.2. Calculations
3. Results and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Mahjoubi, N.; Fazeli, A.; Dinarvand, R.; Khoshayand, M.R.; Shekarchi, M.; Fazelie, M.R. Effect of nonionic surfactants (Dodecyl Maltoside and Polysorbate 20) on prevention of aggregation and conformational changes of recombinant human IFNβ_1b induced by light. Iran J. Pharm. Res. 2017, 16, 103–111. [Google Scholar] [PubMed]
- Martos, A.; Koch, W.; Jiskoot, W.; Wuchner, K.; Winter, G.; Friess, W.; Hawe, A. Trends on analytical characterization of polysorbates and their degradation products in biopharmaceutical formulations. J. Pharm. Sci. 2017, 106, 1722–1735. [Google Scholar] [CrossRef] [PubMed]
- Hall, T.; Sandefur, S.L.; Frye, C.C.; Tuley, T.L.; Huang, L. Polysorbates 20 and 80 degradation by group XV lysosomal phospholipase A 2 isomer X1 in monoclonal antibody formulations. J. Pharm. Sci. 2016, 105, 1633–1642. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Hewitt, D.; Lentz, Y.K.; Ji, J.A.; Zhang, T.Y.; Zhang, K. Characterization and stability study of Polysorbate 20 in therapeutic monoclonal antibody formulation by multidimensional ultrahigh-performance liquid chromatography–charged aerosol detection–mass spectrometry. Anal. Chem. 2014, 86, 5150–5157. [Google Scholar] [CrossRef] [PubMed]
- Jiao, J. Polyoxyethylated nonionic surfactants and their applications in topical ocular drug delivery. Adv. Drug Deliv. Rev. 2008, 60, 1663–1673. [Google Scholar] [CrossRef] [PubMed]
- Kerwin, B.A. Polysorbates 20 and 80 used in the formulation of protein biotherapeutics: Structure and degradation pathways. J. Pharm. Sci. 2008, 97, 2924–2935. [Google Scholar] [CrossRef] [PubMed]
- Bosmuler Züge, L.C.; Isidoro Haminiuk, C.W.; Maciel, G.M.; Meira Silveira, J.L.; de PaulaScheer, A. Catastrophic inversion and rheological behavior in soy lecithin and Tween 80 based food emulsions. J. Food Eng. 2013, 116, 72–77. [Google Scholar] [CrossRef]
- Yaghmur, A.; Aserin, A.; Garti, N. Phase behavior of microemulsions based on food-grade nonionic surfactants: Effect of polyols and short-chain alcohols. Colloid Surf. A 2002, 209, 71–81. [Google Scholar] [CrossRef]
- Sugumar, S.; Singh, S.; Mukherjee, A.; Chandrasekaran, N. Nanoemulsion of orange oil with non ionic surfactant produced emulsion using ultrasonication technique: Evaluating against food spoilage yeast. Appl. Nanosci. 2016, 6, 113–120. [Google Scholar] [CrossRef]
- Shah, A.; Tool, P.; Sorathiya, K.; Prajapati, H.; Dalrymole, D.; Serajuddin, A.T.M. Effect of different polysorbates on development of self-microemulsifying drug delivery systems using medium chain lipids. Drug Dev. Ind. Pharm. 2018, 44, 215–223. [Google Scholar] [CrossRef] [PubMed]
- Torres, L.G.; Rojas, N.; Iturbe, R. Use of two-surfactants mixtures to attain specific HLB values for assisted TPH-diesel biodegradation. J. Environ. Sci. 2004, 16, 950–956. [Google Scholar]
- O’Sullivan, S.M.; Woods, J.A.; O’Brien, N.M. Use of Tween 40 and Tween 80 to deliver a mixture of phytochemicals to human colonic adenocarcinoma cell (CaCo-2) monolayers. Br. J. Nutr. 2004, 91, 757–764. [Google Scholar] [CrossRef] [PubMed]
- Losada-Barreiro, S.; Sánchez-Paz, V.; Bravo-Díaz, C. Effects of emulsifier hydrophile-lipophile balance and emulsifier concentration on the distributions of gallic acid, propyl gallate, and α-tocopherol in corn oil emulsions. J. Colloid Interface Sci. 2013, 389, 1–9. [Google Scholar] [CrossRef] [PubMed]
- Rosen, J.M. Surfactants and Interfacial Phenomena, 3rd ed.; Wiley Interscience: New York, NY, USA, 2004. [Google Scholar]
- Schick, M.J. Nonionic Surfactants, Physical Chemistry, Surfactant Science Series; Marcel Dekker, Inc.: New York, NY, USA, 1987. [Google Scholar]
- Williams, H.D.; Trevaskis, N.L.; Charman, S.A.; Shanker, R.M.; Charman, W.N.; Pouton, C.W.; Porter, C.J.H. Strategies to address low drug solubility in discovery and development. Pharmacol. Rev. 2013, 65, 315–499. [Google Scholar] [CrossRef] [PubMed]
- Florence, A.T.; Hussain, N. Transcytosis of nanoparticle and dendrimer delivery systems: Evolving vistas. Adv. Drug Deliv. Rev. 2001, 50, 569–589. [Google Scholar] [CrossRef]
- Meena, J.; Singh, M. Hydrophobics and double bond of Tweens affecting water interactions estimated with physicochemical properties at T = 298.15 K. J. Mol. Liq. 2013, 220, 671–680. [Google Scholar] [CrossRef]
- Sannaningannavar, F.M.; Patil, S.N.; Melavanki, R.M.; Navati, B.S.; Ayachit, N.H. Thermodynamic parameters and their dependence on temperature in the range 298–353 K for ethoxylated sorbitan ester tween 20, 40, 60 and 80 surfactants. J. Surfactants Deterg. 2015, 18, 495–504. [Google Scholar] [CrossRef]
- Glenn, K.M.; Moroze, S.; Bhattacharya, S.C.; Palepu, R.M. Effect of ethylene glycol on the thermodynamic and micellar properties of Tween 40, 60, and 80. J. Dispers. Sci. Technol. 2005, 26, 79–86. [Google Scholar] [CrossRef]
- Hait, S.K.; Moulik, S.P. Determination of critical micelle concentration (CMC) of nonionic surfactants by donor-acceptor interaction with lodine and correlation of CMC with hydrophile-lipophile balance and other parameters of the surfactants. J. Surfactants Deterg. 2001, 4, 303–309. [Google Scholar] [CrossRef]
- Carnero Ruiz, C.; Molina-Bolıávar, J.A.; Aguiar, J.; MacIsaac, G.; Moroze, S.; Palepu, R. Effect of ethylene glycol on the thermodynamic and micellar properties of Tween 20. Colloid Polym. Sci. 2003, 281, 531–541. [Google Scholar] [CrossRef]
- Chauhan, S.; Sharma, K. Extended studies on molecular interactions of SDBS and DTAB in aqueous solutions of amino acid at T = 293.15–313.15 K. J. Mol. Liq. 2015, 211, 675–685. [Google Scholar] [CrossRef]
- Kiełek, K.; Marczak, W. Hydration of non-electrolytes in H2O and D2O investigated by Passynski’s method. Int. J. Thermophys. 2010, 31, 77–84. [Google Scholar] [CrossRef]
- Singh, T.; Kumar, A. Thermodynamics of dilute aqueous solutions of imidazolium based ionic liquids. J. Chem. Thermodyn. 2011, 43, 958–965. [Google Scholar] [CrossRef]
- Burakowski, A.; Gliński, J. Hydration numbers of non-electrolytes—Application of the acoustic method of Pasynski. Chem. Phys. 2007, 332, 336–340. [Google Scholar] [CrossRef]
- Sannaningannavar, F.M.; Navati, B.S.; Ayachit, N.H. Studies on thermo-acoustic parameters of poly(ethylene glycol)-400 at different temperatures. J. Therm. Anal. Calorim. 2013, 112, 1573–1578. [Google Scholar] [CrossRef]
- Gautam, P.K.; Gautam, R.K.; Rai, R.; Pandey, J.D. Thermodynamic and transport properties of sodium dodecylbenzenesulphonate (SDBS) in aqueous medium over the temperature range 298.15 K to 333.15 K. J. Mol. Liq. 2014, 191, 107–110. [Google Scholar] [CrossRef]
- Boden, N.; Corne, S.A.; Jolley, K.W. Lyotropic mesomorphism of the cesium pentadecafluorooctanoate/water system: High-resolution phase diagram. J. Phys. Chem. 1987, 1, 4092–4105. [Google Scholar] [CrossRef]
- Benjamin, L. Partial molal volume changes during micellization and solution of nonionic surfactants and perfluorocarboxylates using a magnetic density balance. J. Phys. Chem. 1966, 70, 3790–3797. [Google Scholar] [CrossRef]
- Sunil Babu, K.; Venkateswara Rao, A.; Madhavi Latha, D.; Pardhasaradhi, P.; Pisipati, V.G.K.M. Estimation of thermodynamic parameters in 4-(hexyloxybenzylidene)-4′alkoxy anilines, 6O·Om liquid crystalline compounds—A density study. J. Mol. Liq. 2016, 220, 999–1003. [Google Scholar] [CrossRef]
- Venkata Rao, D.; Pardhasaradhi, P.; Pisipati, V.G.K.M.; Madhavi Latha, D.; Datta Prasad, P.V. Estimation of thermodynamic parameters in 3.Om and 3O.Om liquid crystalline compounds. J. Mol. Liq. 2015, 211, 90–95. [Google Scholar] [CrossRef]
- Reddy, R.R.; Rama Gopal, K.; Narasimhulu, K.; Siva Sankara Reddy, L.; Raghavendra Kumar, K.; Venkatesulu, A.; Krishna Reddy, C.V. Correlations between Moelwyn–Hughes parameter, available volume and intermolecular free-lengths in liquid systems. J. Mol. Liq. 2008, 140, 48–53. [Google Scholar] [CrossRef]
- Sannaningannavar, F.M.; Patil, S.N.; Navati, B.S.; Melavanki, R.M.; Ayachit, N.H. Studies on thermodynamic properties of pure poly(ethylene glycol)-400 in the temperature range 299–363 K using volume expansivities. Polym. Bull. 2013, 70, 3171–3183. [Google Scholar] [CrossRef]
- Subrahmanyam, M.S.R.; Vedanayagam, H.S.; Venkateshwar, R.D.; Rajaiah, A.; Venkatacharyulu, P. Estimation of Sharma constant and thermoacoustic properties of fatty acids. J. Am. Oil Chem. Soc. 1995, 72, 1537–1540. [Google Scholar] [CrossRef]
- Huang, J.; Zhang, S.; Feng, Y.; Li, J.; Yan, H.; He, F.; Wang, G.; Liu, Y.; Wang, L. Rheological properties and application of wormlike micelles formed by sodium oleate/benzyltrimethyl ammonium bromide. Colloid Surf. A 2016, 500, 222–229. [Google Scholar] [CrossRef]
- Kumar, R.; Mahesh, R.; Shanmugapriyan, B.; Kannappan, V. Volumetric, viscometric, acoustic and refractometric studies of molecular interactions in certain binarysystems of o-chlorophenol at 303.15 K. Indian J. Pure Appl. Phys. 2012, 50, 633–640. [Google Scholar]
- Chauhan, S.; Kumar, K.; Patial, B.S. Study of acoustical parameters f proline in lecithin–ethanol mixture at varying temperature. Indian J. Pure Appl. Phys. 2013, 51, 531–541. [Google Scholar]
- Szymczyk, K.; Taraba, A. Aggregation behavior of Triton X-114 and Tween 80 at various temperatures and concentrations studied by density and viscosity measurements. J. Therm. Anal. Calorim. 2016, 126, 315–326. [Google Scholar] [CrossRef]
- Jones, G.; Dole, M. The viscosity of aqueous solutions of strong electrolytes with special reference to barium chloride. J. Am. Chem. Soc. 1929, 51, 2950–2964. [Google Scholar] [CrossRef]
- Ali, A.; Hyder, S.; Akhtar, Y. Viscometric studies of a-amino acid in aqueous NaCl and MgCl2 at 303 K. Indian J. Phys. 2005, 79, 157–160. [Google Scholar]
- Huque, M.; Siddiquey, I.A.; Uddin, N. Volumetric and viscometric studies on dodecyltrimethylammonium bromide in aqueous and in aqueous amino acid solutions in premicellar region. J. Chem. Thermodyn. 2006, 38, 1474–1478. [Google Scholar] [CrossRef]
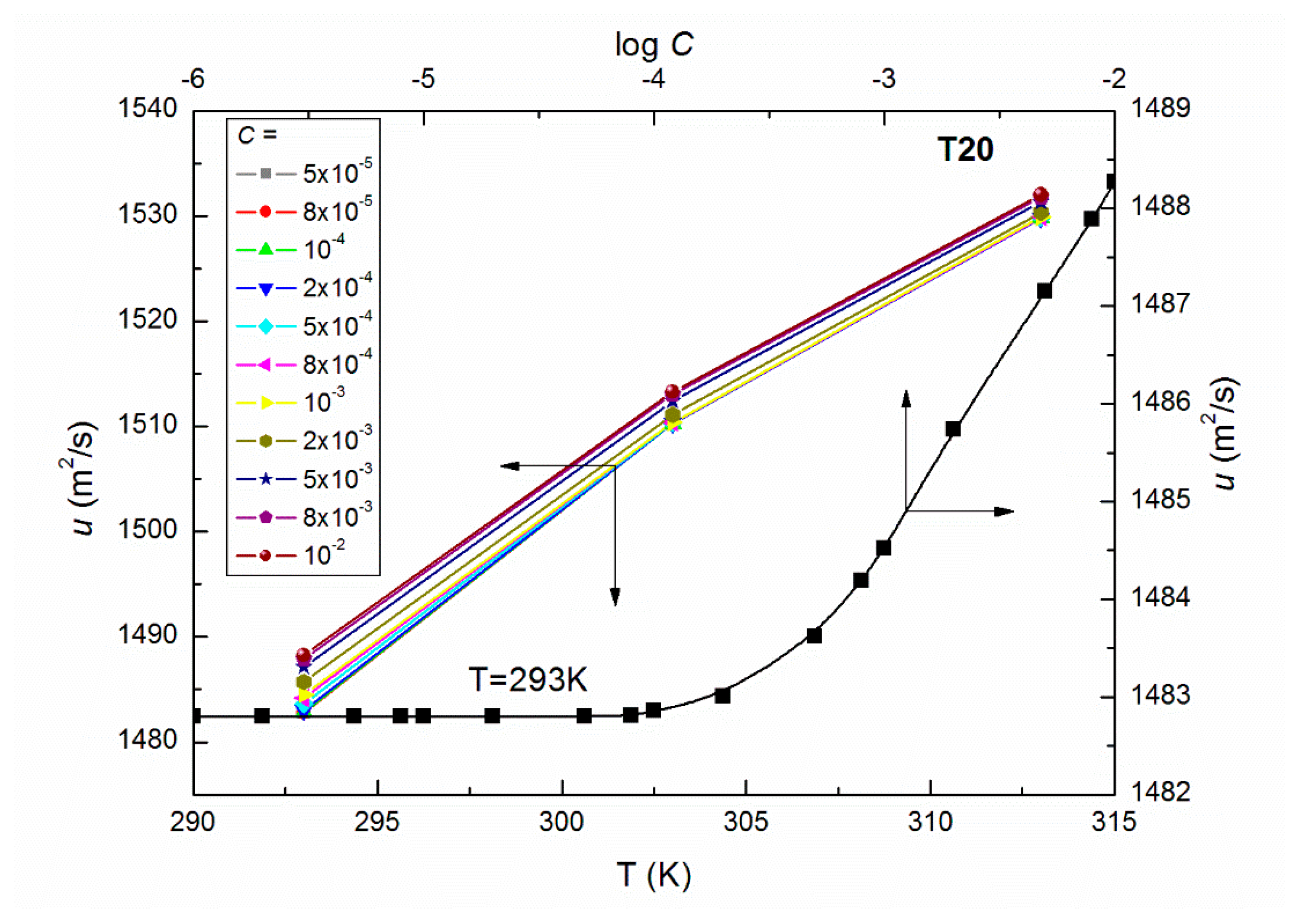
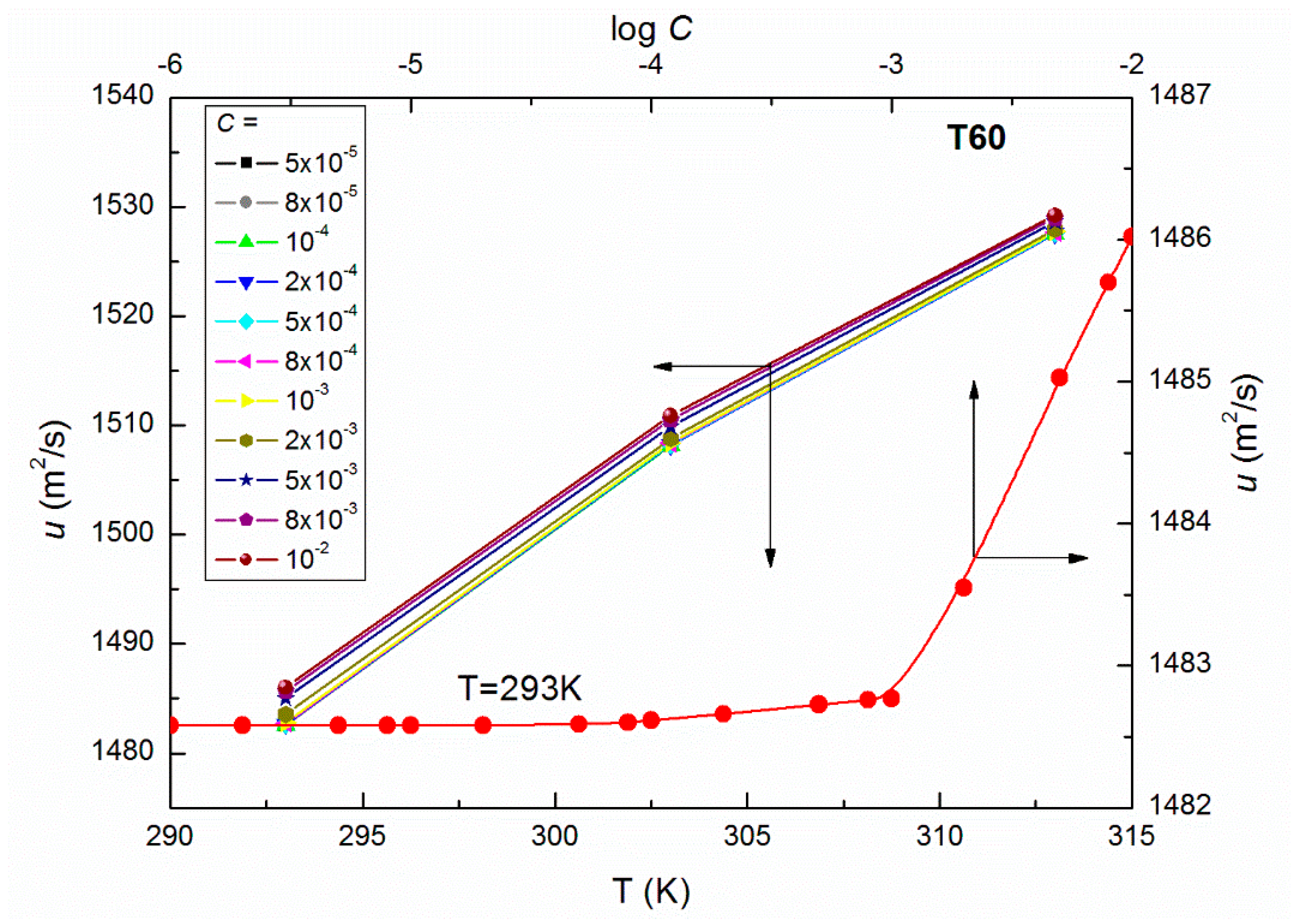
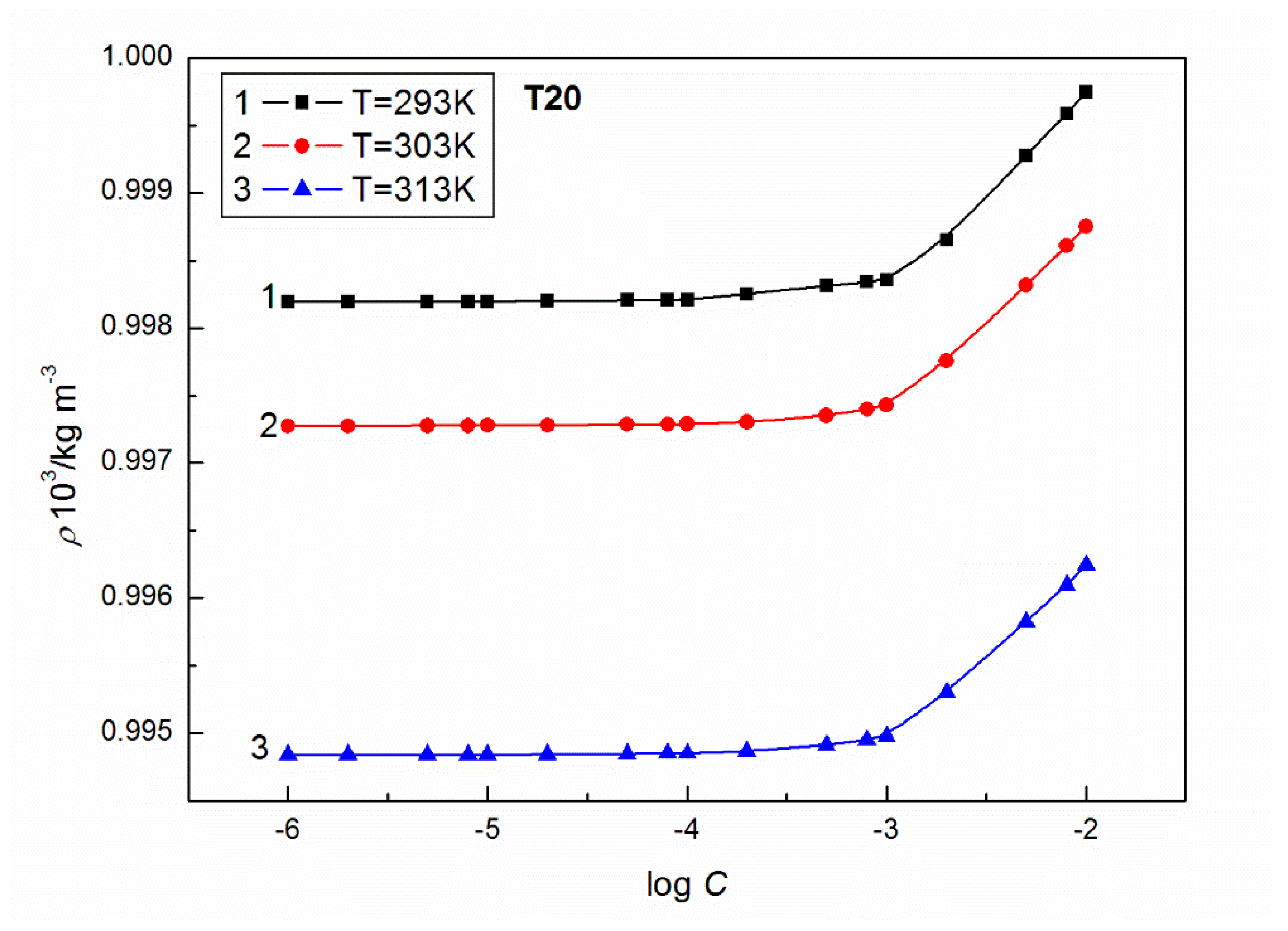

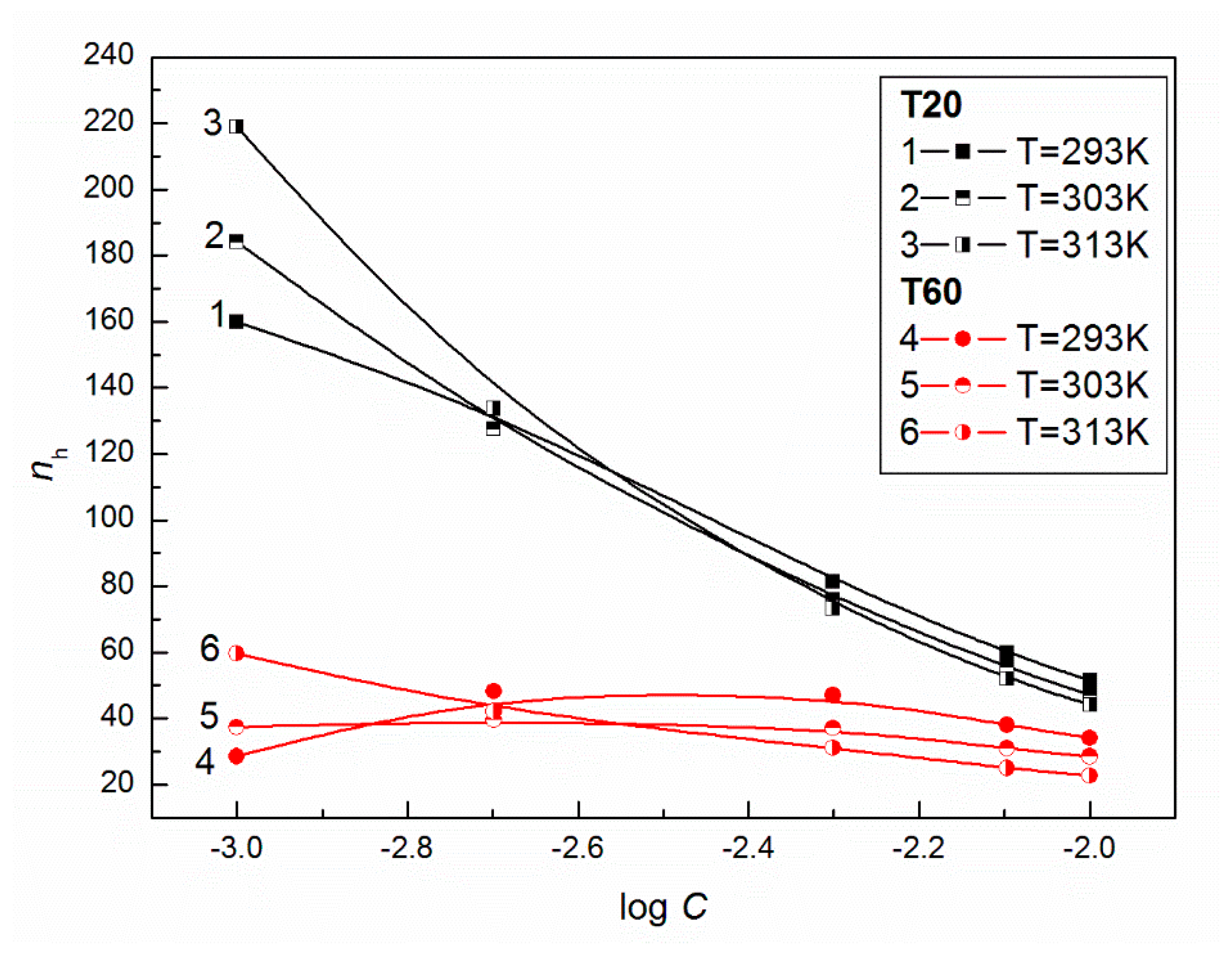
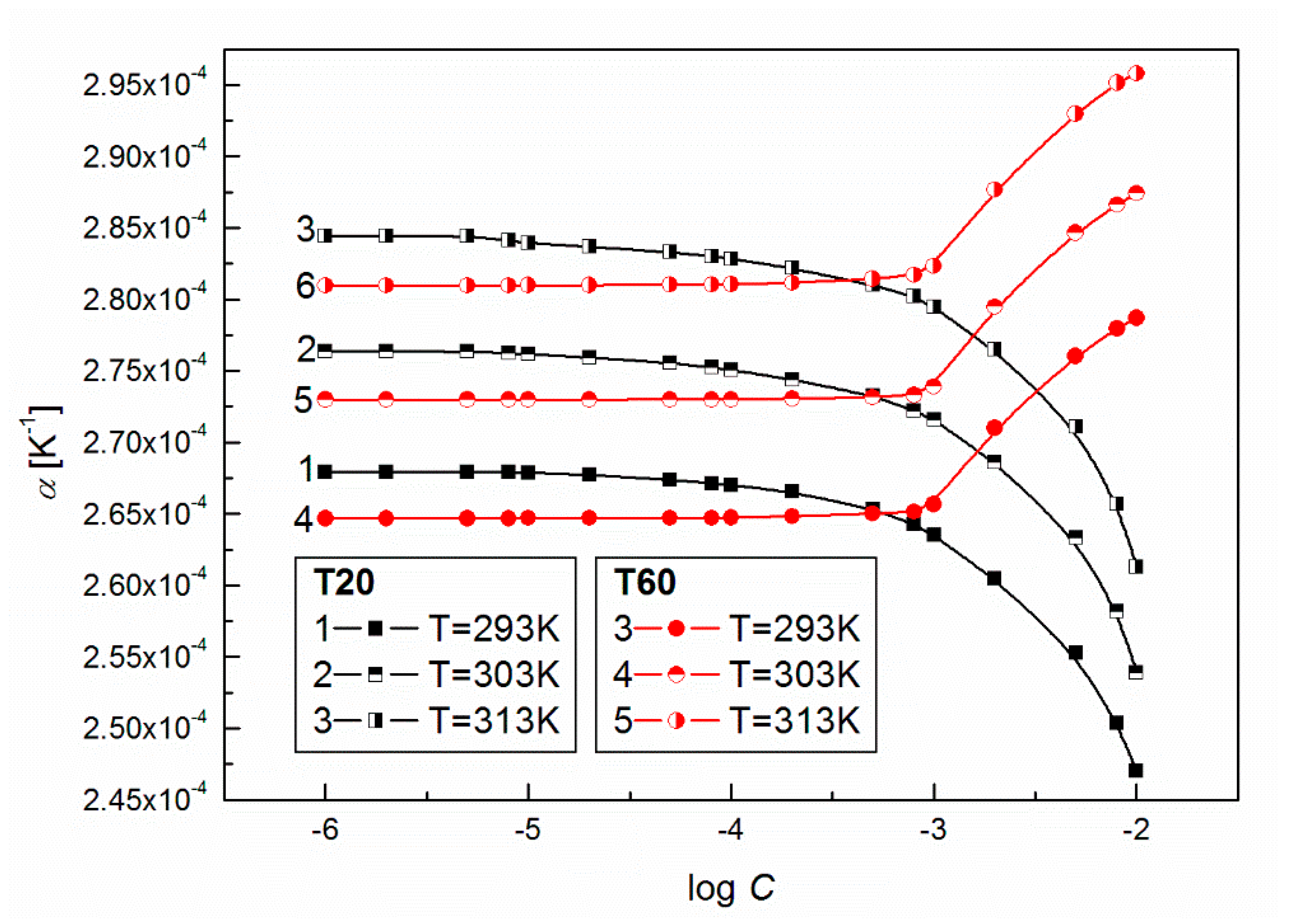
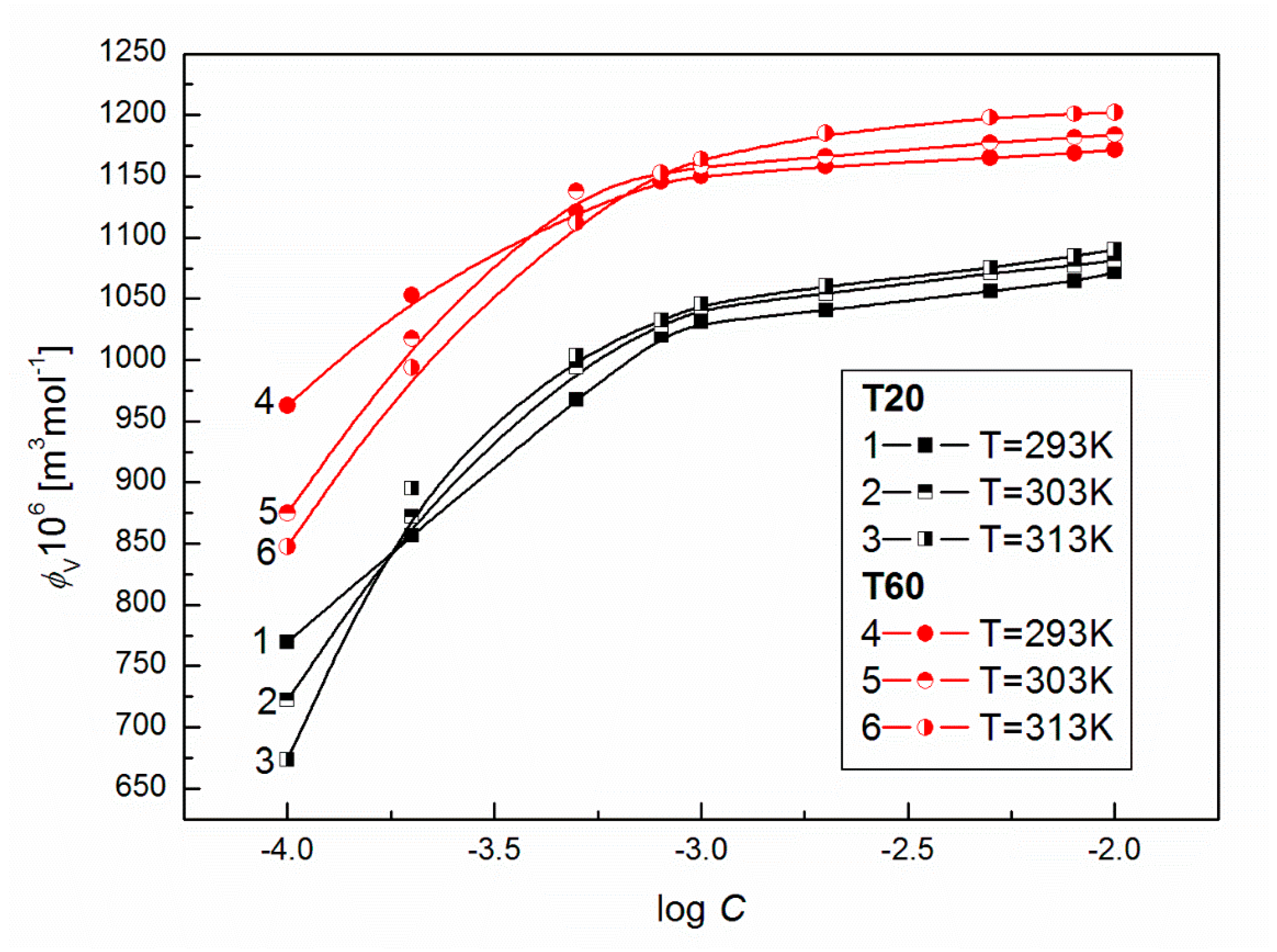

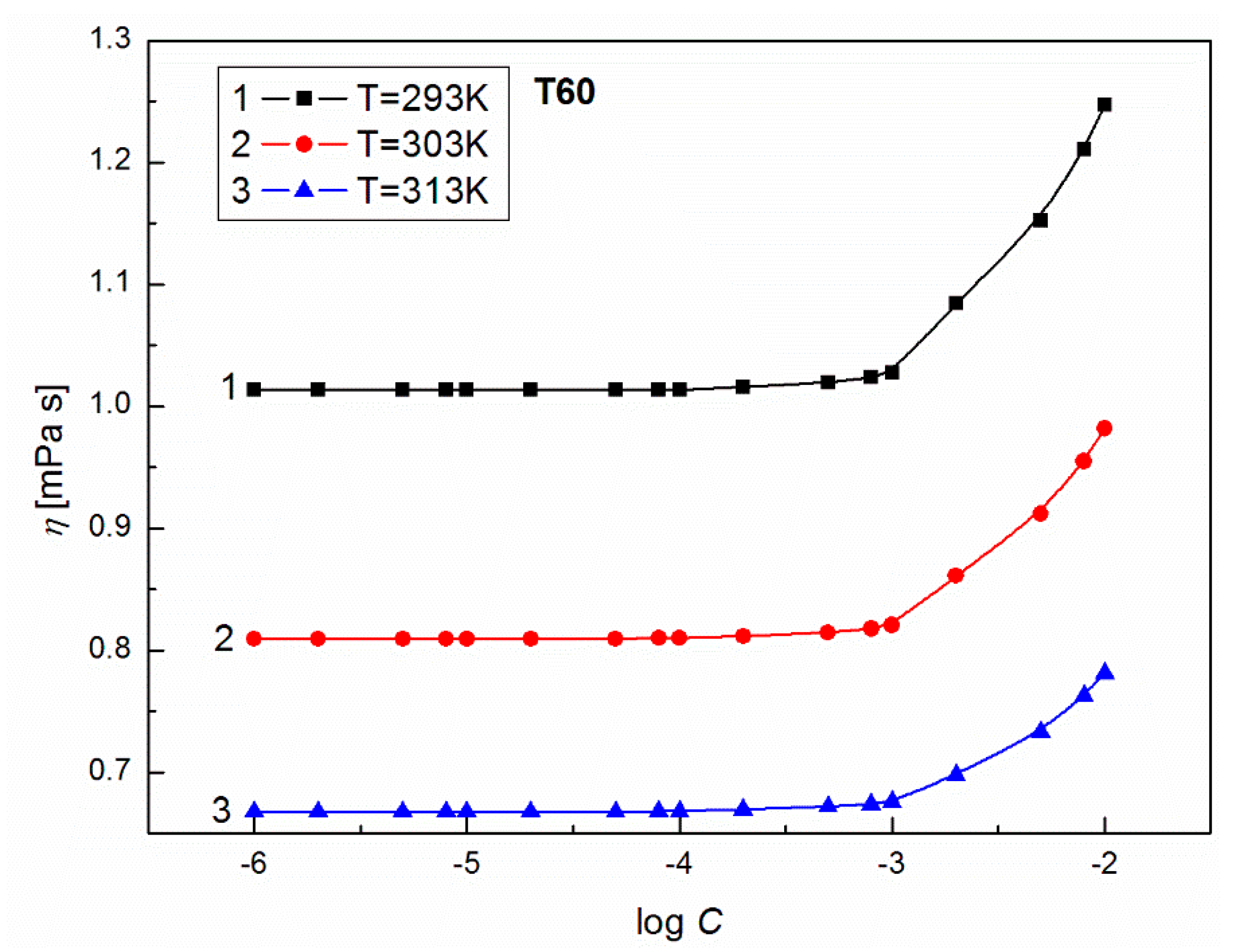
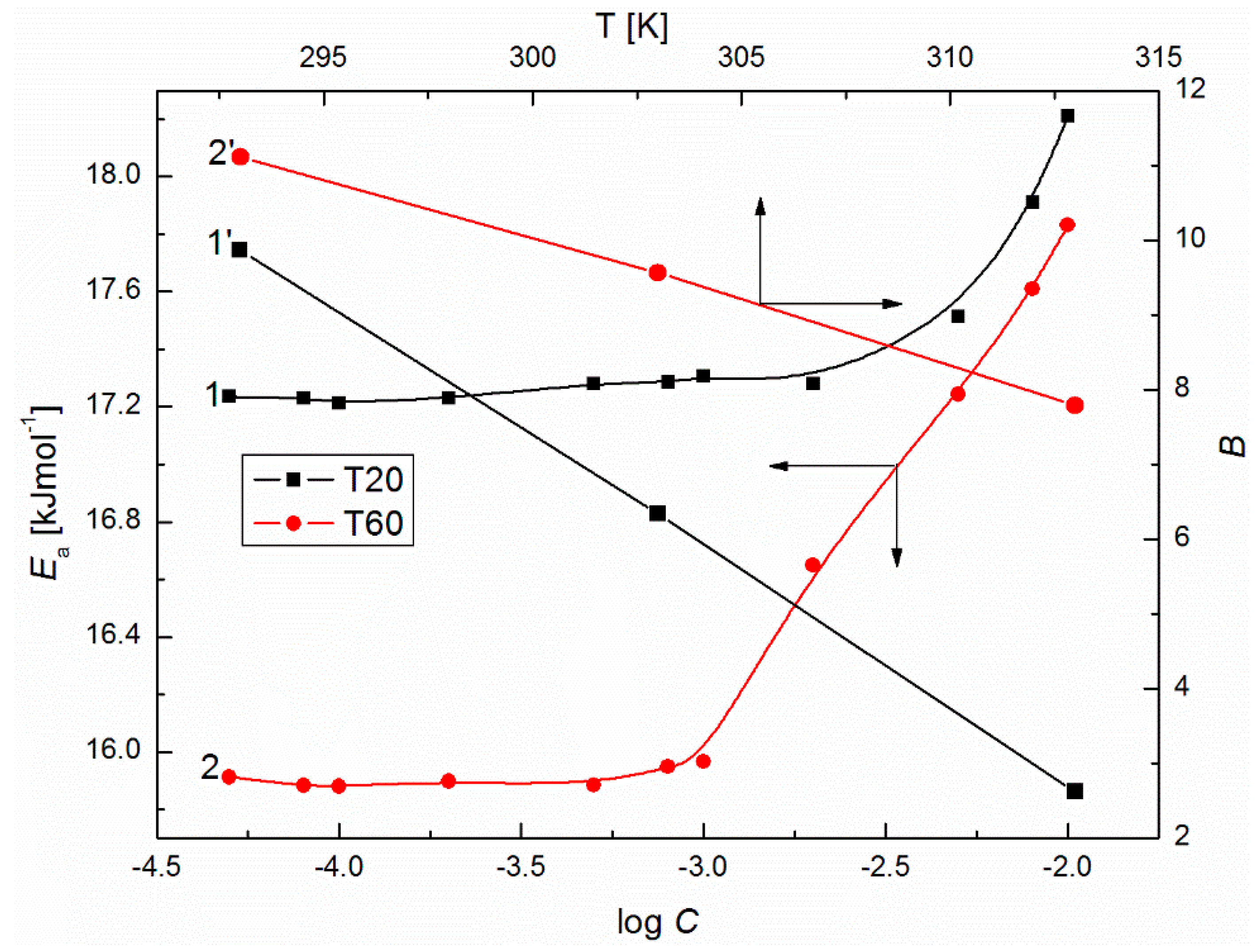
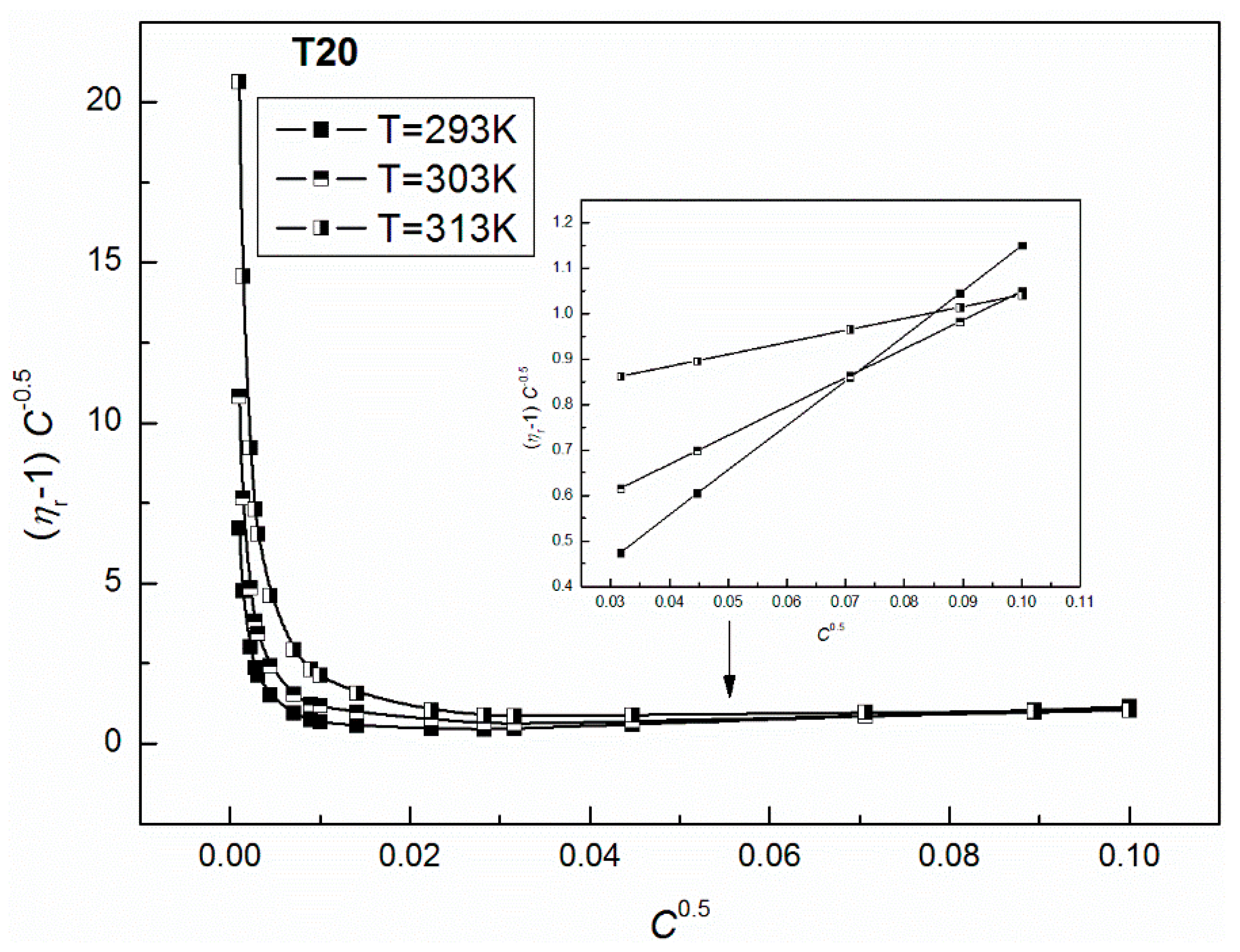
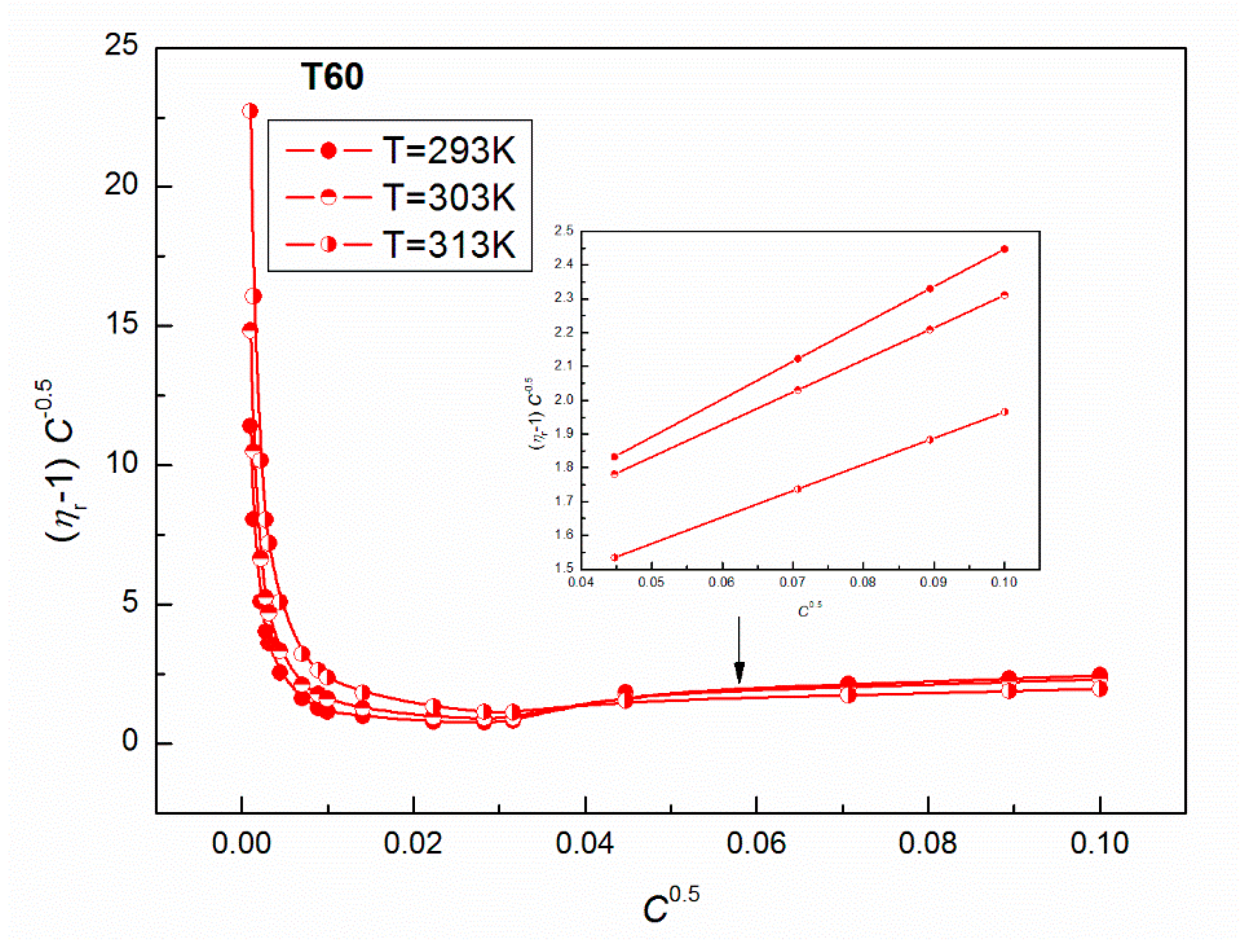
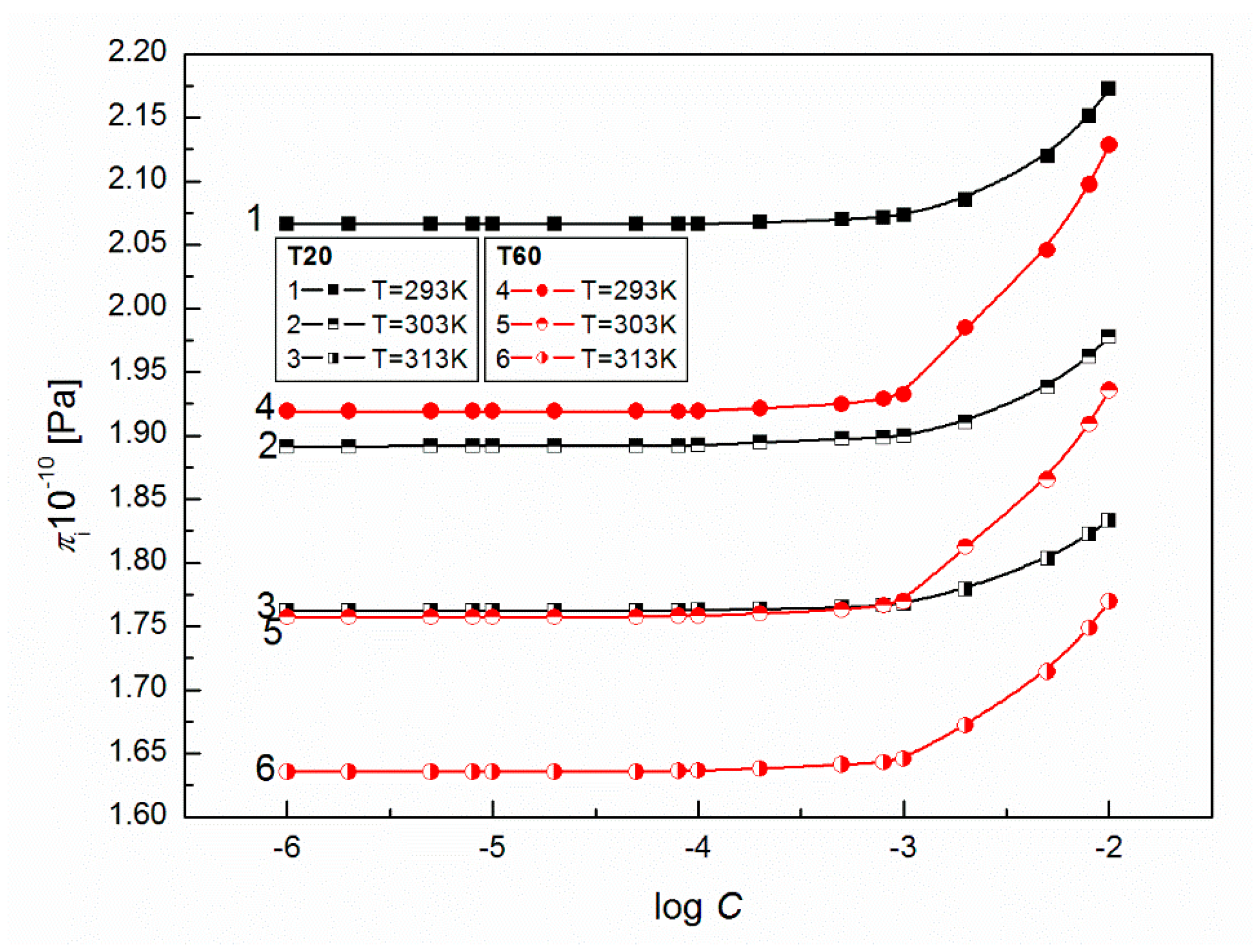
| T20 | ||||||
|---|---|---|---|---|---|---|
| C | 10−10 m2 N−1 | 10−10m | 106 kg m−2 s−1 | 10−6 m3 mol−1 | 10−4 m10/3 s−1/3 mol−1 | |
| T = 293 K | 10−6 | 4.5564 | 0.4349 | 1.4801 | 29.3530 | 45.6842 |
| 2 × 10−6 | 4.5564 | 0.4349 | 1.4801 | 29.3530 | 45.6842 | |
| 5 × 10−6 | 4.5564 | 0.4349 | 1.4801 | 29.3530 | 45.6842 | |
| 8 × 10−6 | 4.5564 | 0.4349 | 1.4801 | 29.3530 | 45.6842 | |
| 10−5 | 4.5564 | 0.4349 | 1.4801 | 29.3529 | 45.6842 | |
| 2 × 10−5 | 4.5564 | 0.4349 | 1.4801 | 29.3529 | 45.6840 | |
| 5 × 10−5 | 4.5563 | 0.4349 | 1.4801 | 29.3527 | 45.6838 | |
| 8 × 10−5 | 4.5563 | 0.4349 | 1.4802 | 29.3501 | 45.6838 | |
| 10−4 | 4.5560 | 0.4349 | 1.4802 | 29.3385 | 45.6842 | |
| 2 × 10−4 | 4.5548 | 0.4348 | 1.4804 | 29.2989 | 45.6837 | |
| 5 × 10−4 | 4.5508 | 0.4347 | 1.4811 | 29.1447 | 45.6873 | |
| 8 × 10−4 | 4.5472 | 0.4345 | 1.4817 | 28.9999 | 45.6919 | |
| 10−3 | 4.5451 | 0.4344 | 1.4821 | 28.9176 | 45.6947 | |
| 2 × 10−3 | 4.5363 | 0.4340 | 1.4837 | 28.6050 | 45.6933 | |
| 5 × 10−3 | 4.5248 | 0.4334 | 1.4861 | 28.2319 | 45.6794 | |
| 8 × 10−3 | 4.5190 | 0.4331 | 1.4873 | 28.0392 | 45.6729 | |
| 10−2 | 4.5159 | 0.4330 | 1.4879 | 27.9396 | 45.6694 | |
| T = 303 K | 10−6 | 4.3962 | 0.4351 | 1.5061 | 22.4963 | 46.0069 |
| 2 × 10−6 | 4.3962 | 0.4351 | 1.5061 | 22.4963 | 46.0069 | |
| 5 × 10−6 | 4.3962 | 0.4351 | 1.5061 | 22.4962 | 46.0068 | |
| 8 × 10−6 | 4.3962 | 0.4351 | 1.5062 | 22.4962 | 46.0067 | |
| 10−5 | 4.3962 | 0.4351 | 1.5062 | 22.4962 | 46.0067 | |
| 2 × 10−5 | 4.3962 | 0.4351 | 1.5062 | 22.4961 | 46.0066 | |
| 5 × 10−5 | 4.3962 | 0.4351 | 1.5062 | 22.4961 | 46.0064 | |
| 8 × 10−5 | 4.3962 | 0.4351 | 1.5062 | 22.4960 | 46.0063 | |
| 10−4 | 4.3962 | 0.4351 | 1.5062 | 22.4955 | 46.0062 | |
| 2 × 10−4 | 4.3960 | 0.4351 | 1.5062 | 22.4902 | 46.0059 | |
| 5 × 10−4 | 4.3954 | 0.4350 | 1.5063 | 22.4748 | 46.0042 | |
| 8 × 10−4 | 4.3950 | 0.4350 | 1.5064 | 22.4647 | 46.0024 | |
| 10−3 | 4.3948 | 0.4350 | 1.5065 | 22.4603 | 46.0012 | |
| 2 × 10−3 | 4.3892 | 0.4347 | 1.5077 | 22.2725 | 45.9933 | |
| 5 × 10−3 | 4.3793 | 0.4342 | 1.5098 | 21.9379 | 45.9806 | |
| 8 × 10−3 | 4.3742 | 0.4340 | 1.5109 | 21.7671 | 45.9738 | |
| 10−2 | 4.3720 | 0.4339 | 1.5114 | 21.6971 | 45.9700 | |
| T = 313 K | 10−6 | 4.2951 | 0.4378 | 1.5219 | 17.6385 | 46.3175 |
| 2 × 10−6 | 4.2951 | 0.4378 | 1.5219 | 17.6385 | 46.3175 | |
| 5 × 10−6 | 4.2951 | 0.4378 | 1.5219 | 17.6385 | 46.3175 | |
| 8 × 10−6 | 4.2951 | 0.4378 | 1.5219 | 17.6385 | 46.3175 | |
| 10−5 | 4.2951 | 0.4378 | 1.5219 | 17.6385 | 46.3175 | |
| 2 × 10−5 | 4.2951 | 0.4378 | 1.5219 | 17.6384 | 46.3174 | |
| 5 × 10−5 | 4.2950 | 0.4378 | 1.5219 | 17.6383 | 46.3172 | |
| 8 × 10−5 | 4.2950 | 0.4378 | 1.5219 | 17.6383 | 46.3170 | |
| 10−4 | 4.2950 | 0.4378 | 1.5219 | 17.6378 | 46.3170 | |
| 2 × 10−4 | 4.2949 | 0.4378 | 1.5220 | 17.6332 | 46.3165 | |
| 5 × 10−4 | 4.2945 | 0.4378 | 1.5221 | 17.6244 | 46.3147 | |
| 8 × 10−4 | 4.2941 | 0.4378 | 1.5222 | 17.6157 | 46.3132 | |
| 10−3 | 4.2939 | 0.4377 | 1.5222 | 17.6109 | 46.3120 | |
| 2 × 10−3 | 4.2901 | 0.4376 | 1.5232 | 17.4972 | 46.3014 | |
| 5 × 10−3 | 4.2824 | 0.4372 | 1.5249 | 17.2449 | 46.2868 | |
| 8 × 10−3 | 4.2785 | 0.4370 | 1.5258 | 17.1173 | 46.2792 | |
| 10−2 | 4.2767 | 0.4369 | 1.5263 | 17.0591 | 46.2746 | |
| T60 | ||||||
|---|---|---|---|---|---|---|
| C | 10−10 m2 N−1 | 10−10 m | 106 kg m−2 s−1 | 10−6 m3 mol−1 | 10−4 m10/3 s−1/3 mol−1 | |
| T = 293 K | 10−6 | 4.5577 | 0.4350 | 1.4799 | 29.4081 | 45.6819 |
| 2 × 10−6 | 4.5577 | 0.4350 | 1.4799 | 29.4081 | 45.6819 | |
| 5 × 10−6 | 4.5577 | 0.4350 | 1.4799 | 29.4081 | 45.6819 | |
| 8 × 10−6 | 4.5577 | 0.4350 | 1.4799 | 29.4080 | 45.6819 | |
| 10−5 | 4.5577 | 0.4350 | 1.4799 | 29.4080 | 45.6819 | |
| 2 × 10−5 | 4.5577 | 0.4350 | 1.4799 | 29.4077 | 45.6819 | |
| 5 × 10−5 | 4.5576 | 0.4350 | 1.4799 | 29.4053 | 45.6817 | |
| 8 × 10−5 | 4.5575 | 0.4350 | 1.4799 | 29.4022 | 45.6816 | |
| 10−4 | 4.5574 | 0.4350 | 1.4800 | 29.3985 | 45.6815 | |
| 2 × 10−4 | 4.5571 | 0.4350 | 1.4800 | 29.3869 | 45.6814 | |
| 5 × 10−4 | 4.5564 | 0.4349 | 1.4802 | 29.3687 | 45.6796 | |
| 8 × 10−4 | 4.5561 | 0.4349 | 1.4803 | 29.3594 | 45.6782 | |
| 10−3 | 4.5559 | 0.4349 | 1.4803 | 29.3561 | 45.6771 | |
| 2 × 10−3 | 4.5503 | 0.4346 | 1.4814 | 29.1559 | 45.6771 | |
| 5 × 10−3 | 4.5389 | 0.4341 | 1.4836 | 28.7711 | 45.6689 | |
| 8 × 10−3 | 4.5333 | 0.4338 | 1.4848 | 28.5935 | 45.6602 | |
| 10−2 | 4.5302 | 0.4337 | 1.4854 | 28.5062 | 45.6527 | |
| T = 303 K | 10−6 | 4.4084 | 0.4357 | 1.5041 | 23.0176 | 45.9855 |
| 2 × 10−6 | 4.4084 | 0.4357 | 1.5041 | 23.0176 | 45.9855 | |
| 5 × 10−6 | 4.4084 | 0.4357 | 1.5041 | 23.0176 | 45.9855 | |
| 8 × 10−6 | 4.4084 | 0.4357 | 1.5041 | 23.0176 | 45.9855 | |
| 10−5 | 4.4084 | 0.4357 | 1.5041 | 23.0176 | 45.9855 | |
| 2 × 10−5 | 4.4083 | 0.4357 | 1.5041 | 23.0175 | 45.9855 | |
| 5 × 10−5 | 4.4083 | 0.4357 | 1.5041 | 23.0164 | 45.9853 | |
| 8 × 10−5 | 4.4082 | 0.4357 | 1.5041 | 23.0141 | 45.9852 | |
| 10−4 | 4.4082 | 0.4357 | 1.5041 | 23.0123 | 45.9852 | |
| 2 × 10−4 | 4.4079 | 0.4356 | 1.5042 | 23.0035 | 45.9849 | |
| 5 × 10−4 | 4.4073 | 0.4356 | 1.5043 | 22.9833 | 45.9839 | |
| 8 × 10−4 | 4.4068 | 0.4356 | 1.5044 | 22.9681 | 45.9824 | |
| 10−3 | 4.4065 | 0.4356 | 1.5045 | 22.9593 | 45.9814 | |
| 2 × 10−3 | 4.4032 | 0.4354 | 1.5052 | 22.8545 | 45.9752 | |
| 5 × 10−3 | 4.3947 | 0.4350 | 1.5070 | 22.5707 | 45.9643 | |
| 8 × 10−3 | 4.3898 | 0.4347 | 1.5081 | 22.4100 | 45.9563 | |
| 10−2 | 4.3868 | 0.4346 | 1.5088 | 22.3112 | 45.9521 | |
| T = 313 K | 10−6 | 4.3078 | 0.4385 | 1.5197 | 18.2064 | 46.2947 |
| 2 × 10−6 | 4.3078 | 0.4385 | 1.5197 | 18.2064 | 46.2947 | |
| 5 × 10−6 | 4.3078 | 0.4385 | 1.5197 | 18.2064 | 46.2947 | |
| 8 × 10−6 | 4.3078 | 0.4385 | 1.5197 | 18.2064 | 46.2947 | |
| 10−5 | 4.3078 | 0.4385 | 1.5197 | 18.2064 | 46.2947 | |
| 2 × 10−5 | 4.3078 | 0.4385 | 1.5197 | 18.2064 | 46.2946 | |
| 5 × 10−5 | 4.3078 | 0.4385 | 1.5197 | 18.2060 | 46.2944 | |
| 8 × 10−5 | 4.3077 | 0.4385 | 1.5197 | 18.2056 | 46.2942 | |
| 10−4 | 4.3077 | 0.4384 | 1.5197 | 18.2049 | 46.2941 | |
| 2 × 10−4 | 4.3075 | 0.4384 | 1.5198 | 18.1985 | 46.2934 | |
| 5 × 10−4 | 4.3069 | 0.4384 | 1.5199 | 18.1811 | 46.2921 | |
| 8 × 10−4 | 4.3065 | 0.4384 | 1.5200 | 18.1680 | 46.2912 | |
| 10−3 | 4.3063 | 0.4384 | 1.5200 | 18.1613 | 46.2904 | |
| 2 × 10−3 | 4.3044 | 0.4383 | 1.5205 | 18.1025 | 46.2856 | |
| 5 × 10−3 | 4.2988 | 0.4380 | 1.5218 | 17.9276 | 46.2734 | |
| 8 × 10−3 | 4.2954 | 0.4378 | 1.5226 | 17.8280 | 46.2623 | |
| 10−2 | 4.2933 | 0.4377 | 1.5232 | 17.7702 | 46.2552 | |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Szymczyk, K.; Szaniawska, M.; Taraba, A. Micellar Parameters of Aqueous Solutions of Tween 20 and 60 at Different Temperatures: Volumetric and Viscometric Study. Colloids Interfaces 2018, 2, 34. https://doi.org/10.3390/colloids2030034
Szymczyk K, Szaniawska M, Taraba A. Micellar Parameters of Aqueous Solutions of Tween 20 and 60 at Different Temperatures: Volumetric and Viscometric Study. Colloids and Interfaces. 2018; 2(3):34. https://doi.org/10.3390/colloids2030034
Chicago/Turabian StyleSzymczyk, Katarzyna, Magdalena Szaniawska, and Anna Taraba. 2018. "Micellar Parameters of Aqueous Solutions of Tween 20 and 60 at Different Temperatures: Volumetric and Viscometric Study" Colloids and Interfaces 2, no. 3: 34. https://doi.org/10.3390/colloids2030034
APA StyleSzymczyk, K., Szaniawska, M., & Taraba, A. (2018). Micellar Parameters of Aqueous Solutions of Tween 20 and 60 at Different Temperatures: Volumetric and Viscometric Study. Colloids and Interfaces, 2(3), 34. https://doi.org/10.3390/colloids2030034





