Dual Influence of Li Concentration and Nanoparticle Size in LiCoO₂ on the Conductivity and Storage Capacity of Lithium Batteries

Abstract

Li_xCo_1−xO₂ nanocomposites with molar concentrations x (0.1, 0.3, 0.5, 0.7, 0.9) were prepared using the sol–gel method. The optical and electrical properties were determined using UV-Vis spectrometer. The results obtained indicate that the absorption coefficient increases upon increase of nanoparticle size, while the energy gap decreases when nanoparticle size increases. The storage capacity reaches its maximum value near resonance at minimum nanoparticle size. This is attributed to the fact that the optical properties, electrical conductivity, and actual electrical permittivity reach their maximum values near the resonance region and increase as the nanoparticle size decreases. The operating voltages at which the storage capacity attains maximum value in the range from 2.3 to 3.5 volts. These operating voltages can be adjusted to achieve the required range by controlling the Li concentrations and the crystallite size of Li_xCo_1−xO₂ NPs which directly affect the energy gap and, in turn, influence the operating voltage. The operating voltage can thus be increased by increasing the energy gap, which requires decreasing the nano size and the Li concentration.

1. Introduction

Energy is very important for human activity and development. Petroleum is a main energy source. Unfortunately, it also causes severe environmental and health risks. This comes from the fact that it causes environmental pollution and severe biological hazards and diseases. This requires searching for pollution-free energy sources. As a promising solution, solar energy is almost available everywhere and environmentally friendly. Moreover, it can be used in various applications because it can be easily converted into electrical energy [1,2,3].

Nevertheless, without efficient storage solutions, solar energy is mainly unreliable due to its intermittent availability for high-demand applications such as transportation and industry. Solar energy storage is thus one of the urgent problems that need to be solved. Unfortunately, conventional storage systems, including lead–acid batteries, have limited storage capacity and are physically inefficient [4,5,6]. In recent years, the area of physical sciences, particularly quantum physics and nanotechnology, opened a new horizon to overcome these limitations. There are promising solutions to increase battery performance using nanomaterials whose properties can be adjusted easily. This comes from the fact that nanomaterials obey quantum laws. Quantum laws provide scientists with powerful tools to change physical properties by changing geometries, sizes, and compositions of nanomaterials to satisfy the required needs of technologies. This means that promotion in energy storage capabilities can be achieved [7,8,9].

Lithium-based systems are the most promising materials for energy storage. Lithium cobalt oxide batteries (LCO) have high specific capacity and voltage [10,11,12]. These batteries are certainly not optimized, and research efforts have focused on capacity decay, voltage limitations, and material stability. Doping processes with aluminum, magnesium, and calcium can stabilize the battery’s layer structure during lithium–ion intercalation and deintercalation and are considered effective techniques for improving LCO performance [13,14,15,16,17]. Consequently, these strategies improve conductivity, cycling performance, and overall efficiency. High energy density lithium–sulfur (Li-S) batteries [18] have also benefited from nano-structural innovations involving mesoporous nickel–cobalt oxides and carbon composites. However, these developments improve cycling stability and reversible capacities by mitigating polysulfide dissolution and improving ion transport. The performance of lithium–ion batteries (LIBs) depends on the anode materials [19]. Since carbon-based nanostructures are primarily used for their conductivity and stable interfaces, alternative materials have been sought due to their higher specific capacity [20]. Compared to cobalt oxide composites, transition metal-based anodes have significantly higher theoretical capacities [21,22]. Free-standing cobalt-based nanofibers with exceptional capacity and speed performance have been produced using advances in electrospinning technology. Further performance enhancement is achieved by combining cobalt-based materials with conductive additives to overcome the challenges of large volume changes and poor conductivity during charge–discharge cycles.

It is also crucial for the development of cathode materials. Altering cobalt concentrations in lithium-rich layered oxides (LRLOs) [23] have been shown to improve cycling stability and energy density. Cobalt is a bulk structure stabilizer, while excessive concentrations resulted in a rapid loss of capacity and a drop in cross-sectional stress. Solutions to these problems require reducing manganese-related redox activity and improving surface oxygen stability. Co-doping of LCO cathodes with elements such as magnesium, titanium, and aluminum, which inhibit undesirable phase transitions and improve surface stability, is also used to optimize LCO cathodes at high voltages [24,25,26]. The cycling performance of LCO batteries has been improved through the use of fluoroethylene carbonate-based electrolytes, which increase ionic conductivity and oxidation stability. Studies of additives such as lithium difluoro (oxalato) borate show the ability to form protective layers on electrodes to reduce capacity loss and extend battery life [27]. Moreover, recent research on alternative electrode materials has shown that stabilizing metastable phases—such as the 1T phase in MoS₂—can significantly enhance battery performance by improving electrode structural integrity and electrode–electrolyte compatibility [28]. These advances demonstrate how electrochemical performance in modern battery systems is related to material composition and structural stability. Cobalt-based materials in zinc–cobalt batteries exhibit high energy density and solid electromechanical cycle stability. At the atomic level, previously demonstrated structural changes result in charge storage behavior that transitions from that of supercapacitors to batteries [29].

However, high-voltage LCO cathodes are still difficult to stabilize, and structural degradation can still occur during long cycles [30,31]. At the same time, surface treatment through hydrothermally assisted methods and atomic-scale doping can further improve battery performance. In situ cobalt doping for lithium–oxygen (Li-O₂) batteries has proven to be a dynamic means of stabilizing discharge products, improving charge transport and minimizing reaction overvoltage. Consequently, Co doping represents a simple and effective approach to increasing the Li₃O₄ content, which can significantly improve the performance of the Li-O₂ battery [32]. These experiments demonstrated the importance of using nano-technics for cobalt–lithium batteries and provided an impetus for the use of nanoscience.

This work aimed to control the Li concentration and nanoparticle size of Li_xCo_1−xO₂ nanocomposite to improve its optical and electrical properties, which in turn enhance the performance of lithium–cobalt batteries in industrial applications. Experimental work was performed with the materials and methods exhibited in Section 2. This is followed by the results and discussion in Section 3, with the conclusion in Section 4.

2. Materials and Methods

Lithium cobalt oxide nanoparticles were prepared with the formula Li_xCo _1−xO₂ with x = (0.1, 0.3, 0.5, 0.7, 0.9) using the sol–gel method. The precursor solutions of cobalt nitrate hexahydrate (Co(NO₃)_2.6H₂O pure 99.0%) and lithium nitrate (LiNO₃ pure 99.9% trace sigma-Aldrich) were prepared by dissolving powders in distilled water and stirring until they were completely dissolved. An appropriate amount of citric acid (C₆H₈O₇ pure 98.0%) and ethylene glycol (C₂H₆O₂ pure 98%) were added to the mixed solutions. The pH of the solutions was adjusted to approximately 7 using a small amount of ammonia (NH₃ pure 98%), which is optimal for the sol–gel technique. The solutions were heated to 70 °C with constant stirring to evaporate excess water until a viscous gel formed. The solutions were cooled to room temperature and the formed gel was dried at 100 °C in a drying oven to remove residual water and solvent. The molded materials were calcined at 700 °C for 8 h to ensure complete crystallization of Li_xCo_1−xO₂. UV-Vis Min 1240 spectrometers were used to study the optical and electrical properties of the prepared samples.

The crystal and nanostructures were determined using the X-ray diffraction (XRD) technique (Holland Philips X-ray powder diffractometer (CuKα, λ = 1.5406 Å). The optical and electrical properties, including conductivity, were found utilizing an ultraviolet spectrometer.

The behavior of optical and electrical properties was explained using simple theoretical relationships. These relationships describe the connection between the storage capacity, electrical conductivity, and permittivity, the effect of nano-size on lithium concentration, the relationship between operating voltage and photon wavelengths, as well as the energy gap. According to the basic definition of the capacitance, the expression of the electrical capacitance C per unit area takes the form of:

$${\displaystyle \frac{C}{A}}={\displaystyle \frac{ϵ}{d}}$$

(1)

The capacitance is directly related to the electric permittivity $ϵ$, area A of the plates, and the distance between them d by

$$C={\displaystyle \frac{ϵA}{d}}$$

(2)

The electric permittivity $ϵ$, in turn, is related to the electrical conductivity $\sigma$, electric field intensity E, flux density D, and the current density J by

$$J=\sigma E=ϵ{\displaystyle \frac{\partial D}{\partial t}}=-i\omega ϵE$$

(3)

It follows from Equation (3) that the conductivity is given by

$$\sigma =-i\omega ϵ$$

(4)

Thus,

$$\sigma ~\omega \epsilon$$

(5)

This means that to maximize capacitance, one should maximize both ε₁ and σ. Let the diameter of the supplied cobalt be denoted as d_C. Assuming that cobalt combines with lithium upon interaction to form a large diameter, denoted as d_CL, the diameter of Co Li is given by:

$$d_{CL}=n{d}_C$$

(6)

Such that:

$$n>1$$

(7)

If the lithium concentration is denoted by $n_L$ and the cobalt concentration is denoted by $n_C$, their total number N thus takes the form of:

$$N=n_L+n_C$$

(8)

As a result, crystal size X is given by:

$$\begin{array}{c}X=n_c{d}_c+n_L{d}_{cL}=\left(N-n_L\right)d_c+n_{L}n{d}_c\\ =\left(N+\left(n-1\right)n_L\right)d_c=N_0{d}_c\\ =\left(N+n_0{n}_L\right)d_c=d_0+d_{0c}{n}_L\end{array}$$

(9)

The nanoparticle sizes were calculated using Scherrer’s equation derived from X-ray diffraction (XRD) patterns, and the crystallite size of LiCoO₂ nanoparticles was calculated using the Debye–Scherrer formula as follows:

$$D\left(nm\right)={\displaystyle \frac{k\lambda }{\beta cos\theta }}$$

(10)

where β is the half width at half maximum, K is constant equal to 0.90, the wavelength of the X-ray (λ = 0.1540 nm), D is the crystallite size, and θ is the Bragg angle, respectively.

According to the quantum definition of the intensity in terms of the wave function:

$$\psi =A{e}^{ikx}=A{e}^{-k_{2}x}{e}^{i{k}_{1}x}$$

(11)

where the wave number (k) is assumed as a complex in the form of:

$$k=k_1+i{k}_2$$

(12)

the classical and the quantum momentum p gives:

$$p=\mathrm{\hslash }k=\mathrm{\hslash }{k}_1+i\mathrm{\hslash }{k}_2=mv=m{v}_1+im{v}_2$$

(13)

the wave intensity I is thus given by

$$I=hfcn=hfc{\left|\psi \right|}^2=hfcA{e}^{-2k_{2}x}=I_0{e}^{-2k_{2}x}=I_0{e}^{-\alpha x}$$

(14)

thus, the absorption coefficient is given by

$$\alpha =2k_2$$

(15)

but from Equation (3) and the definition of J beside Equation (4), one gets:

$$J=nev=\sigma E=-iwϵE=-i{\sigma }_{0}E=ne\left(v_1+i{v}_2\right)=-i{\sigma }_{0}E$$

(16)

thus, numerically,

$$v_2={\displaystyle \frac{{\sigma }_0}{ne}}$$

(17)

thus, from (15) and (12), and beside (14), the absorption coefficient is given by

$$\alpha =2k_2={\displaystyle \frac{2m{v}_2}{\mathrm{\hslash }}}={\displaystyle \frac{2m{\sigma }_0}{\mathrm{\hslash }ne}}$$

(18)

which relates the absorption coefficient to the conductivity.

3. Results and Discussion

In this section, the results of the change of the absorption coefficient with the wavelength were obtained using UV spectrometer. The relationship between conductivity and the real dielectric constant with the wavelength were found using Equations (16) and (5), respectively. The relationship between these parameters and the wavelength beside the nanoparticle size were, in addition to the lithium concentration are presented and discussed. The nanoparticle sizes were obtained from X-ray diffraction (XRD) patterns using Scherrer’s equation.

Figure 1, which is a modification of a previous work [33], displays the XRD spectrum of Li_xCo_(1−x)O₂ NPs prepared at different Li concentrations. It can be observed that all samples show a hexagonal crystal structure with an R-3m space group, which aligns with earlier investigations on LiCoO₂ [34,35].

The lattice parameters (a, b, and c), as seen in

Table 1. Lattice parameters of Li_xCo_1−x O₂ samples at different concentrations (Reprint from previous work ref. [33]).

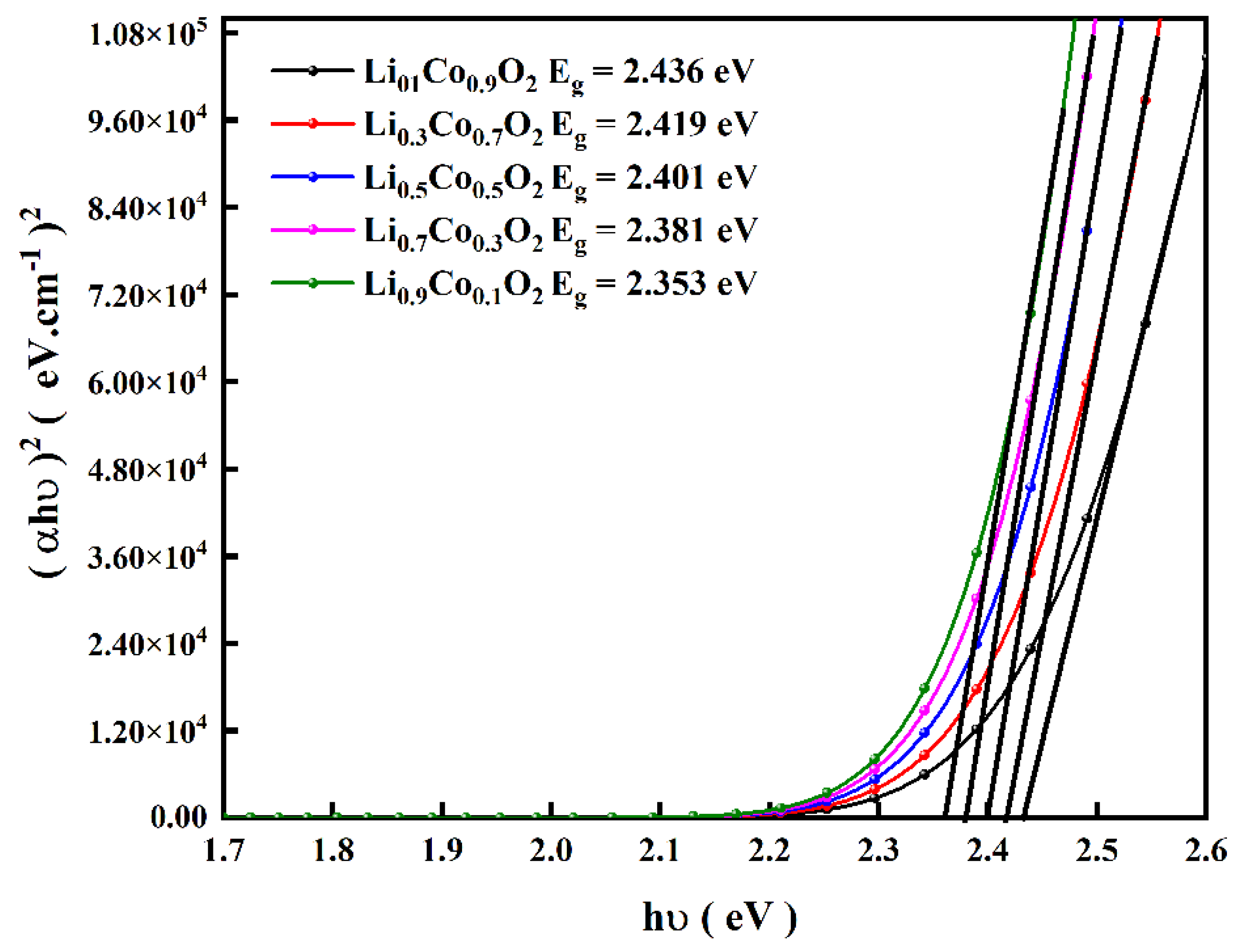
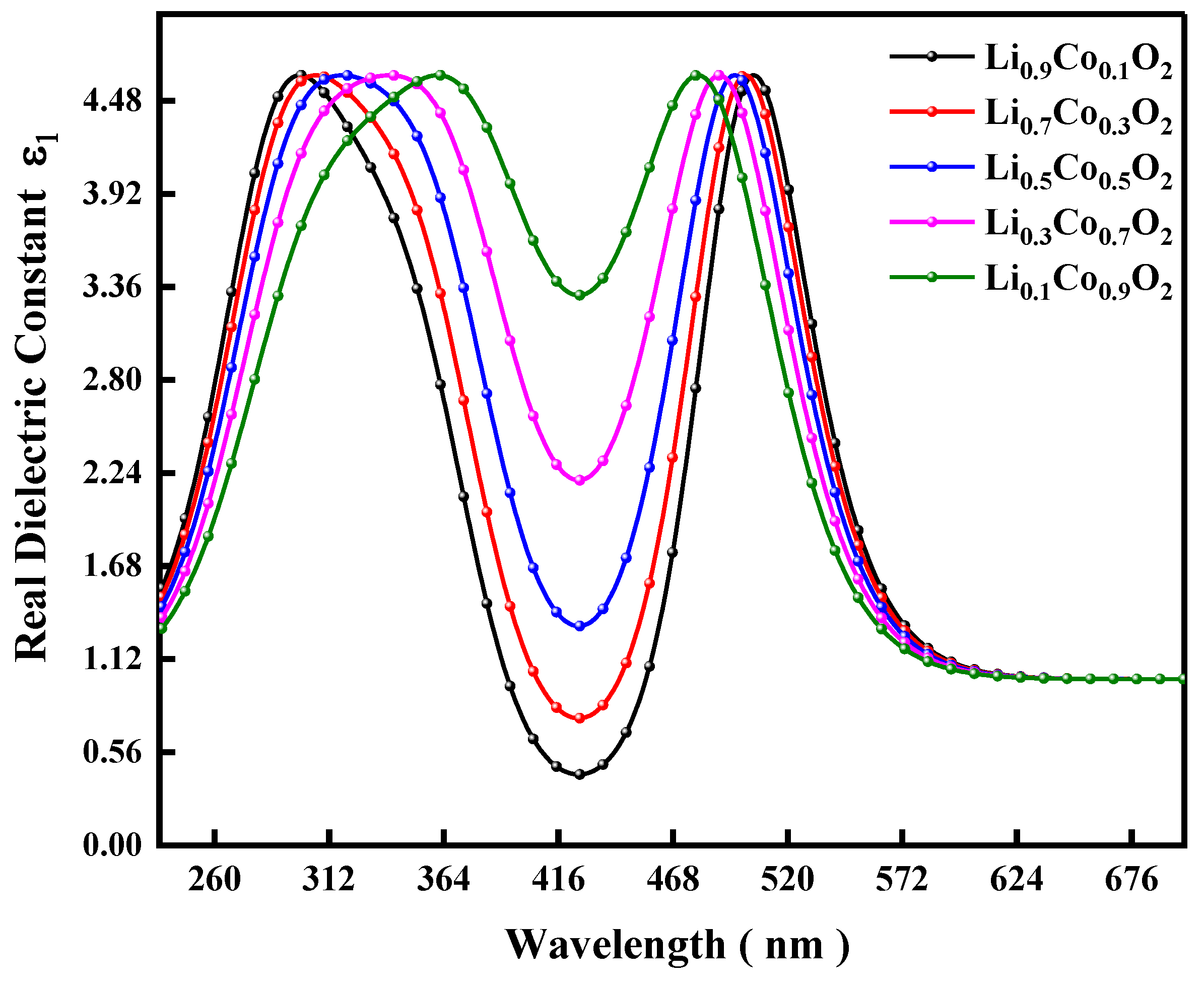
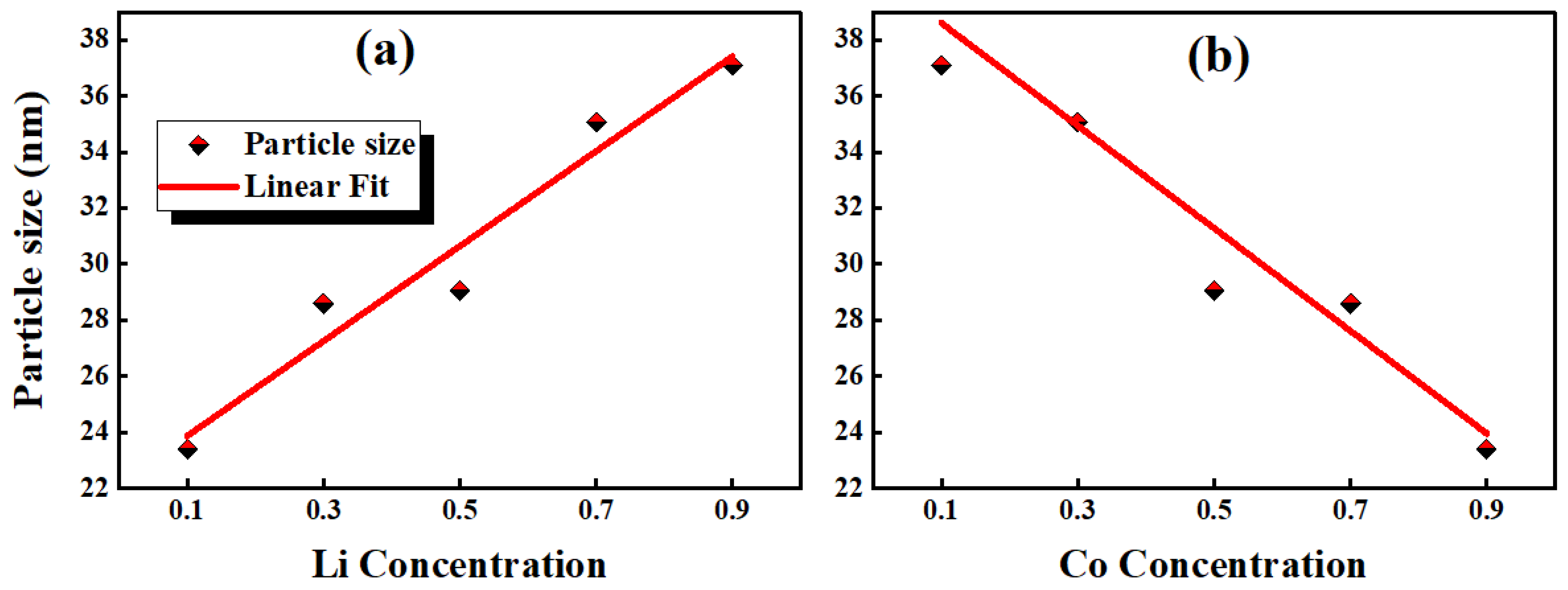
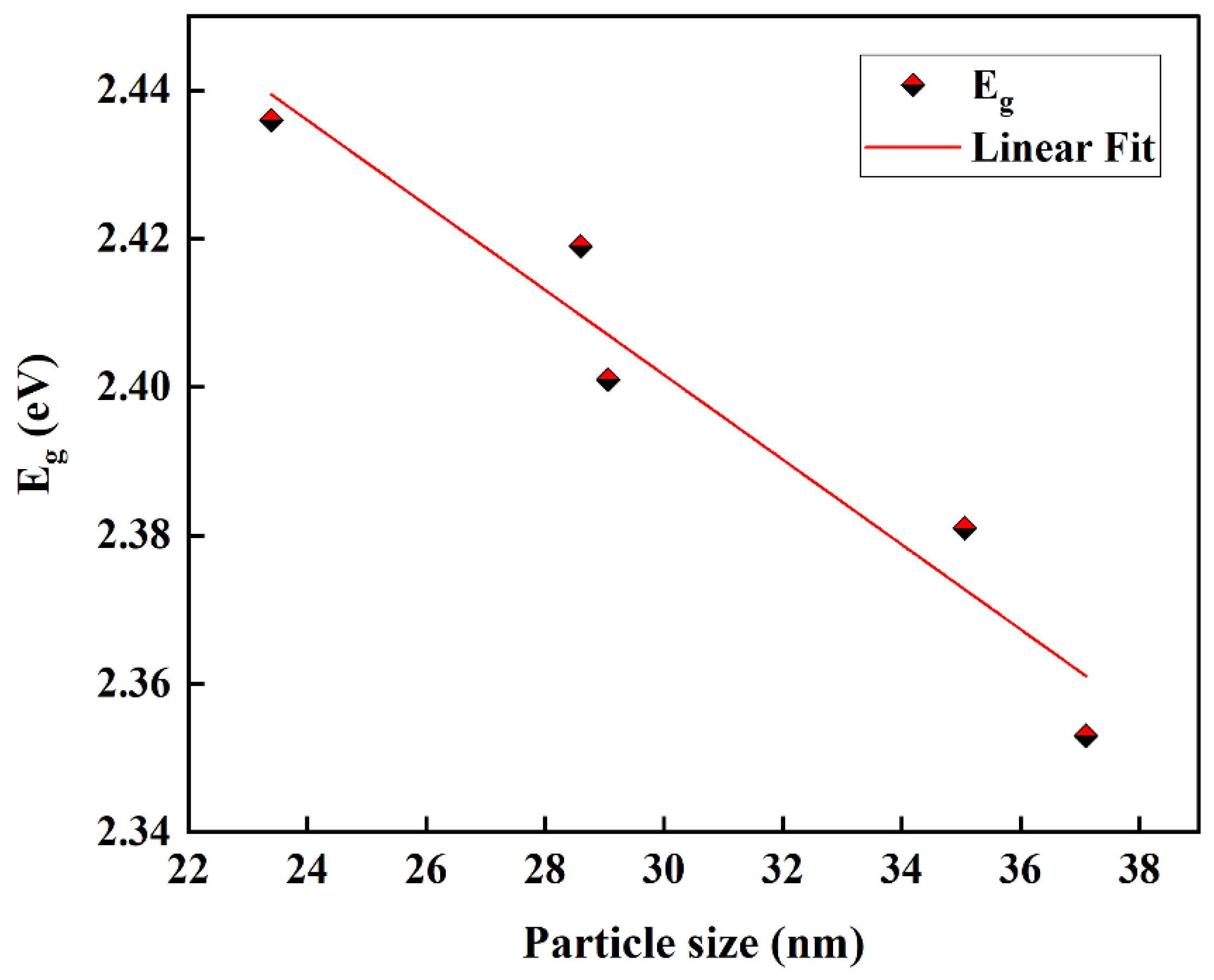
XRD Data		Li0.9Co0.1O2	Li0.7Co0.3O2	Li0.5Co0.5O2	Li0.3Co0.7 O2	Li0.1Co0.9 O2
Space Group		R-3m	R-3m	R-3m	R-3m	R-3m
Crystal System		Hexagonal	Hexagonal	Hexagonal	Hexagonal	Hexagonal
Cell Parameters 10−10 m	a	2.816	2.675	2.547	1.984	1.578
	b	2.816	2.675	2.547	1.984	1.578
	c	14.052	13.48	12.84	9.99	8.854
Density (g·cm−3)		5.05	5.30	5.57	7.41	9.04
Volume (10−10)3		96.5	92.6	88.2	68.5	60.7
d (10−10 m)		2.204	2.114	2.013	1.564	1.386

, decrease with increasing the concentration of cobalt (decreasing lithium concentration), revealing a reduction in the unit cell volume. The crystal size of LiCoO₂ nanoparticles decreases upon decreasing Li concentration, assuming values: 37.07, 35.06, 29.06, 28.6, and 23.44 nm for Li concentration x = 0.9, 0.7, 0.5, 0.3, and 0.1, respectively. The observed reduction in particles size indicates the effect of replacing Co with Li on reducing the crystal size. This change can be partially explained by the difference in ion sizes, as Co has a smaller ionic radius than Li.

Figure 2, concerning the absorption coefficient, indicates that the maximum absorption is at 360 nm, which corresponds to the resonance photon energy of about more than 4 eV.

According to Figure 3, the energy gap assumes values in the range of about 2.4 to 2.3 eV, which is considerably smaller than the resonance photon energy of about 4 eV, which is related to and nearly equal to the main energy gap of the host matrix before the addition of impurities. This indicates that the addition of impurities introduces donor and acceptor levels, which drastically change the energy gap. The observed reduction in the energy gap upon increasing Li concentration may result from the broadening of both the conduction and valence bands, which is responsible for the narrowing of the band gap. Such broadening may be attributed to an increase in crystal field strength. As Li is incorporated into the Li_xCo_(1−x)O₂ matrix, it can introduce additional energy states within the bandgap. These states act as donor levels, making it easier for electrons to transfer to the conduction band, thereby reducing the effective bandgap. These findings are consistent with previous studies suggesting that narrowing the energy gap is linked to enhanced photon absorption and improved electronic transitions [36,37,38,39,40].

Figure 4 and Figure 5 illustrate the electrical and optical conductivities as a function of wavelength. Both conductivities increase as wavelength decreases. They decrease at resonance with increasing Li concentration, where recombination processes are likely to be enhanced.

Figure 6 clearly shows that the real part of electrical permittivity (dielectric constant) decreases at resonance with increasing Li concentration. This can be easily understood considering the relationship between permittivity and conductivity. The conductivity (σ) is directly proportional to permittivity (ε) as given by Maxwell’s equation; higher conductivity (σ) is expected to correspond to higher permittivity (ε). According to Equation (2), the material capacitance is directly proportional to the electrical permittivity. This means the material can store a higher charge when the Li concentration decreases.

Figure 7a,b presents the relationship between nanoparticle size, lithium (Li), and Cobalt (Co) concentrations, respectively. Figure 7 indicates that higher Li concentrations are accompanied by greater nanoparticle sizes and proportionately lower cobalt (Co) content. This observation is consistent with crystallographic studies of mixed metal oxides, which indicates that Li incorporation into CoO₂ leads to the formation of larger volume phases. Higher Li concentrations facilitate the growth of larger nanoparticles during the synthesis process. This is attributed to the larger ionic radii of Li ions compared to Co ions, which can lead to the expansion of the nanoparticles [41,42].

Figure 8, Figure 9 and Figure 10 exhibited the change of the electrical, optical conductivity, energy gap, and the real electric permittivity with the particle size near resonance and out of resonance, respectively. As nanoparticle size increases at near resonance, all these parameters decrease. The absorption coefficient, however, increases upon increasing the nano size as larger nanoparticles provide larger surface areas that can interact with light. In contrast, out of resonance, all properties increase when the nano size increases. This means that out of resonance, the behavior is notably different. This may be attributed to the fact that the electronic transitions are less dependent on the incident electromagnetic field when the system is out of resonance. Instead, as the nanoparticles grow, electronic interactions become more stable, allowing for a more efficient charged transport that increases conductivity [43,44].

Additionally, permittivity increases because the larger particles provide more opportunities for polarization within the material [45,46]. Nanoparticle size affects electrical permittivity and conductivity, which can in turn affect the storage capacity, as shown in Equation (3). This means that for the voltage corresponding to the zone of the wavelengths near resonance, the storage capacity can be increased by decreasing the nano size. However, for all zones out of resonance, the storage capacity increases upon increasing the nano size for the corresponding wide range of voltages.

In particular, a resonance wavelength window of 360–520 nm is highlighted as a key factor to maximize performance at an operating voltage of 2.3–3.5 V in the order of 2.4 eV. This work is supported by previous studies. For example, the results of Salma [47] suggested that doping Li-ion batteries with Co enhances their performance. Zhang [48] showed that using cobalt as an anode in Li batteries improved conductivity and capacity. In the work of Choi [23], the findings showed that changing the Co concentration changes the capacity, which confirms the present study. According to his work, doping with Co increases crystallinity and cycling stability. The results obtained by Kawashima [49] showed that for Li CoO₂, phase purity, crystalline, and orientation affect the performance of the battery. Zhang [24] also showed that the addition of Co for LiCo-O₂ batteries results in high volumetric energy density and stable cycling. The results of Shang [29] showed that cobalt-based electrodes have high optimal energy density, large capacity, and excellent self-discharge. The results obtained by Xiong [32] showed that the discharge process converts Li₂O₂ to Li₃O₄, which increases stability and charge/mass transport. All these studies support the fact that doping Li-ion batteries with Co improves their performance. On the other hand, this work has shed light and shown which element influences battery storage. It is very interesting to note that the storage capacity of LiCo batteries and their operating voltage can be controlled and increased by adjusting the nano size, Li concentration, and energy gap.

The theoretical framework devised herein provides understanding of how to increase the storage capacity for the prepared samples. Notably, the peak resonant absorption wavelength at around ~400 nm corresponds to the energy gap $E_g=\hslash {\omega }_0=h{f}_0={\displaystyle \frac{\lambda c}{{\lambda }_0}}\approx 4.05\times {10}^{-19}J(~2.4\mathrm{eV})$. Interestingly, this value is nearly identical to the average energy gap obtained in Figure 3. This implies that the photon energy required to generate free electrons must have a satisfactory frequency of $hf>E_g$ or $\hslash \omega >E_g$ and the corresponding voltage V must satisfy $eV\ge E_g$ or $V\ge {\displaystyle \frac{E_g}{e}}$. In view of Figure 5, Figure 7 and Figure 8, it is clear that the photo energy and the associated voltage match the energy gap at resonance.

At an operating voltage of about 2.4 volts, capacitance can be maximized by decreasing the nano size and Li concentration, which increases the nanoparticles density. Since this stage is associated with a sudden increase of the number of electrons, necessitating a large number of nanoparticles to capture them. However, out of resonance—where operating voltages differ considerably from 2.4 V—the storage capacity can be increased by increasing the nanoparticles size. This behavior may be related to the fact that, out of resonance, the increase of photon frequency increases the electron velocity which requires more vacancies provided by nano particles having larger sizes to move more quickly to accumulate large number of charge carriers. Additionally, increasing Li concentration may further support this process.

4. Conclusions

The LiCoO₂ nanocomposites prepared and investigated in this study have shown good potential for application in storage batteries based on their physical properties. At the same time, it is possible to regulate the storage capacity by controlling the lithium concentration in these nanocomposites, since conductivity and electrical permittivity are fundamentally directly related to the electrical capacity. With increasing Li concentration associated with increase in the nano size for LiCoO₂ nanocomposites, the absorption coefficient increases while the energy gap decreases. The value of the energy gap of Li_xCo_(1−x)O₂ composition, which is related to the maximum absorption, affects the operating voltage, thus directly influencing the optimization of storage capacity. Near operating voltages of 2.4 volt correspond to the energy gap, and smaller nano sizes enhance electrical permittivity and electrical conductivity, thereby increasing storage capacity. Storage capacity reaches its maximum value near resonance at the smallest nanoparticle size. By decreasing nano size, one should fine-tune the energy gap to maximize the storage capacity at a specific required operating voltage. Increasing the operating voltage requires increasing the energy gap, which can be achieved by reducing the nano size and the Li concentration. The maximum operating voltages at which the storage capacity is in the range of 2.3–3.5 volts for the range of nano sizes 23–37 nm.

This work provides practical guidelines for enhancing Li-CoO₂ battery performance through precise control of nanoparticle size, lithium concentration, and cobalt doping. Future work should focus on optimizing absorption, conductivity, and permittivity to improve storage capacity further and stabilize the operating voltage.