Micro-Scale Model of rCF/PA6 Spun Yarn Composite
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
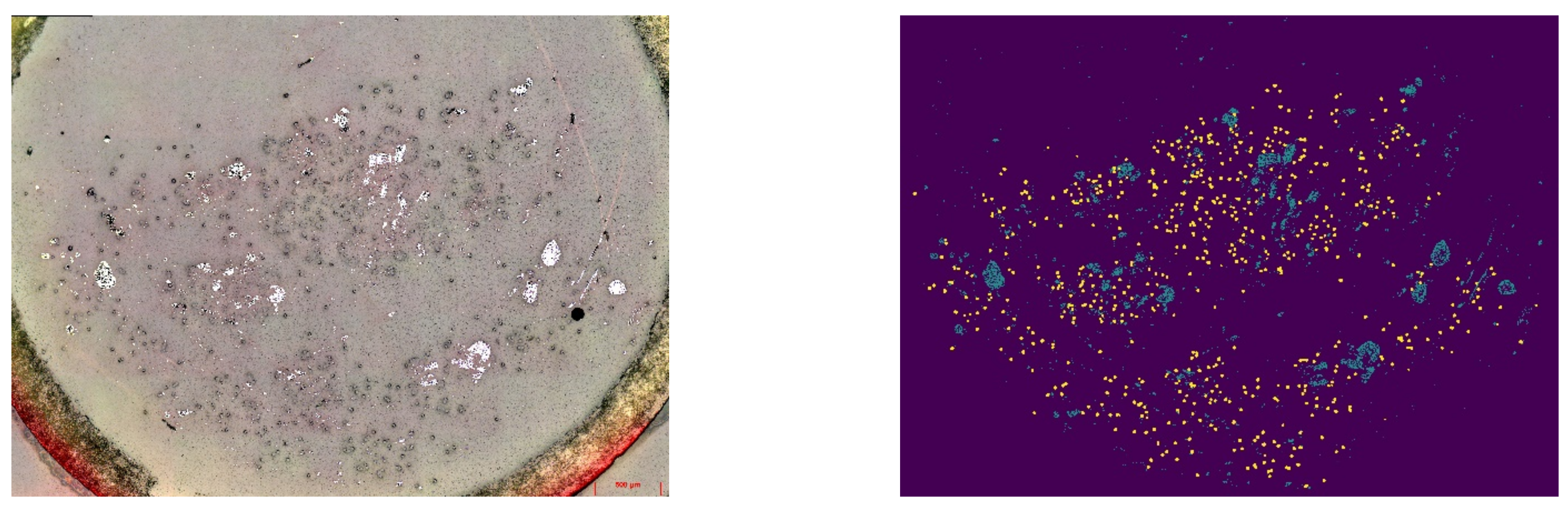
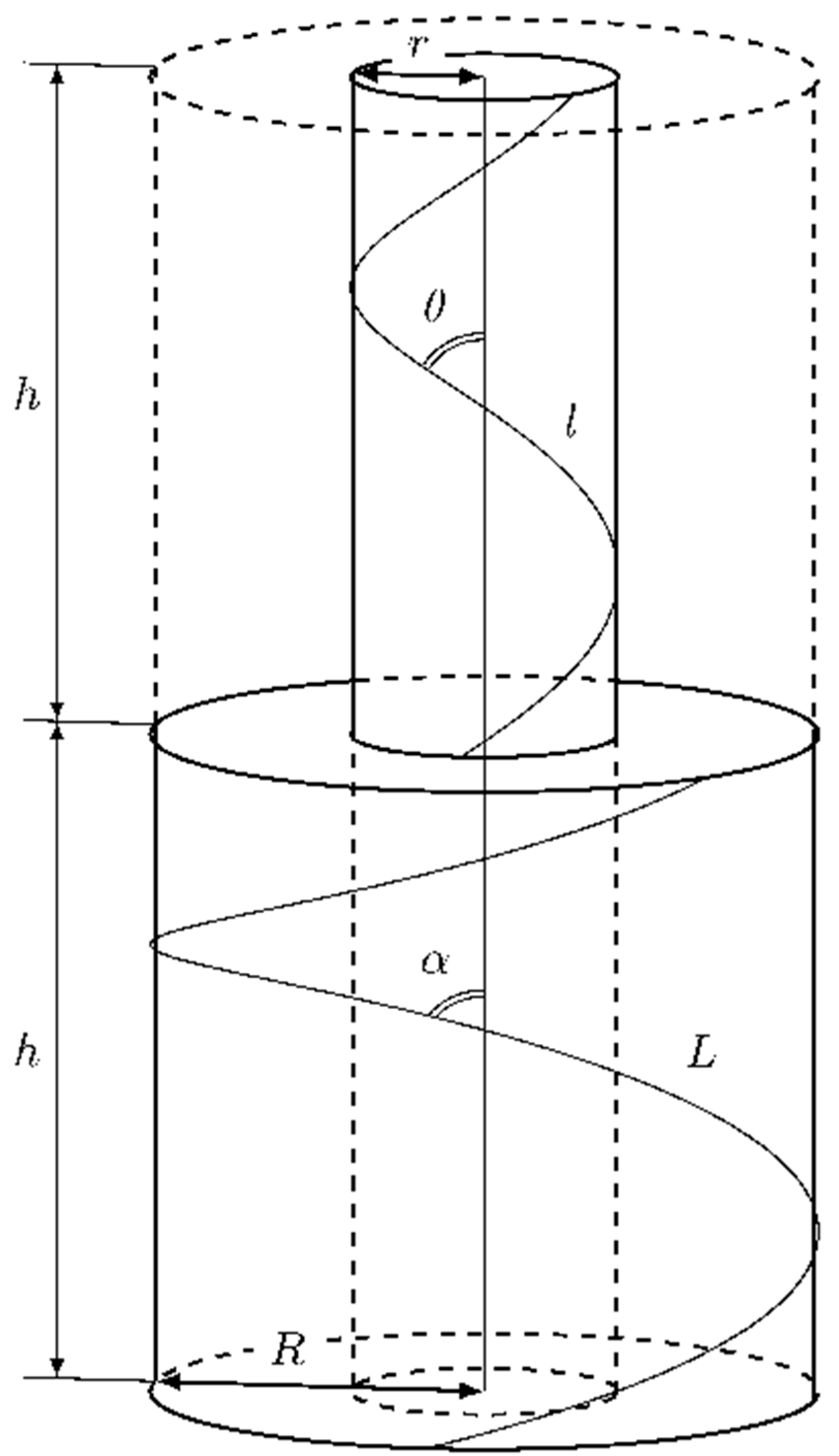
2.2. Filament Geometry
2.3. Micromechanical Yarn Modelling
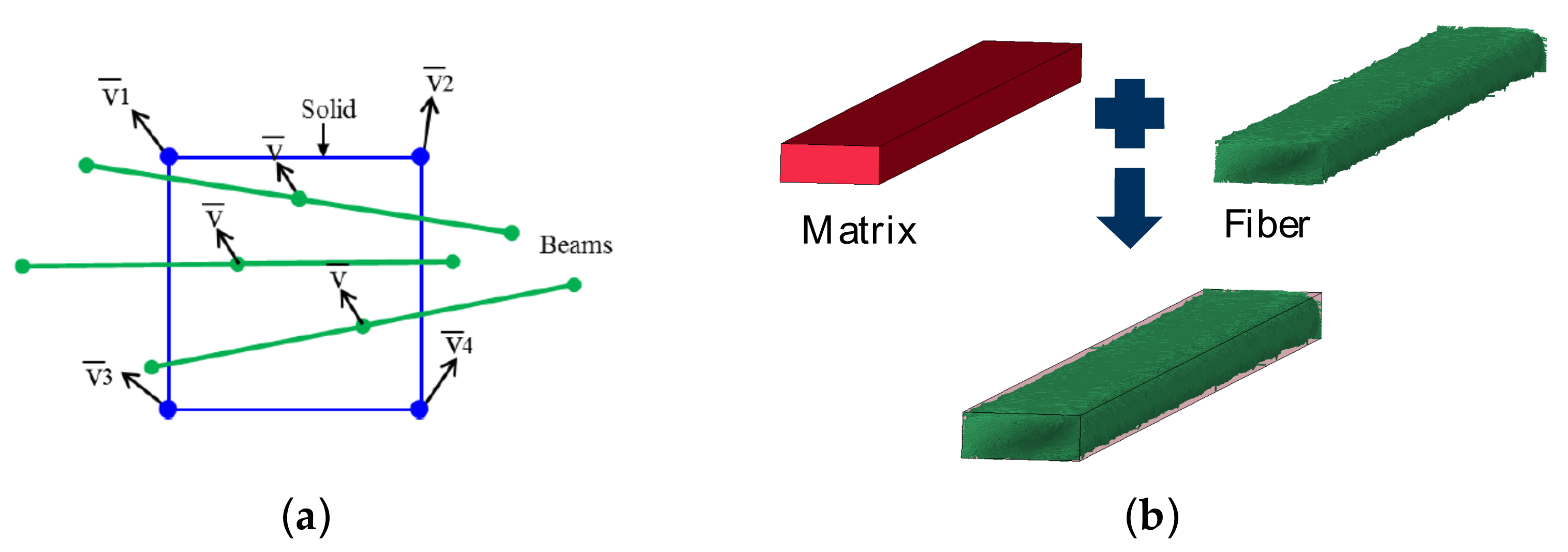
2.3.1. Geometrical Assumptions
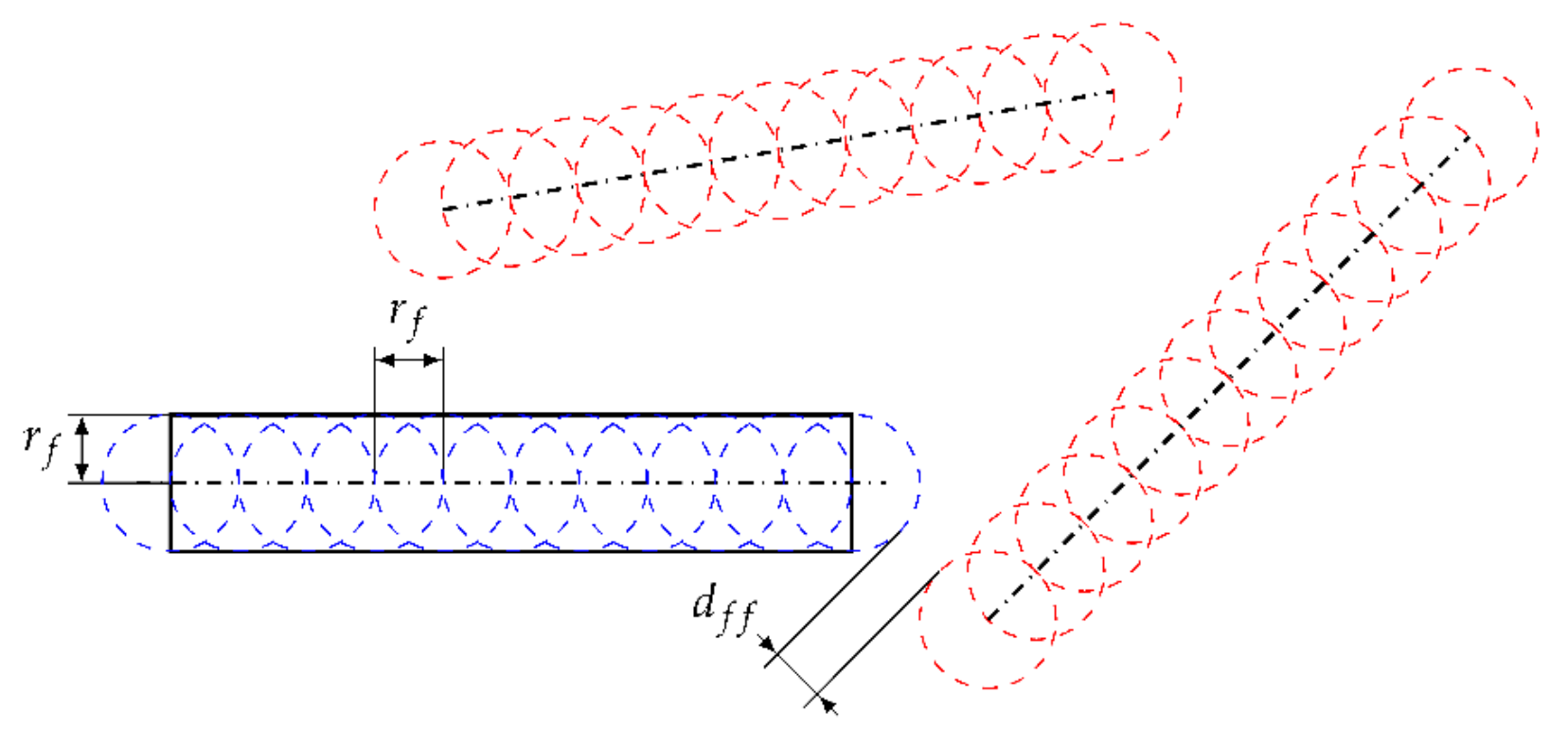
2.3.2. Geometric Modelling Approach
2.3.3. Finite Element Modeling
3. Results and Discussion
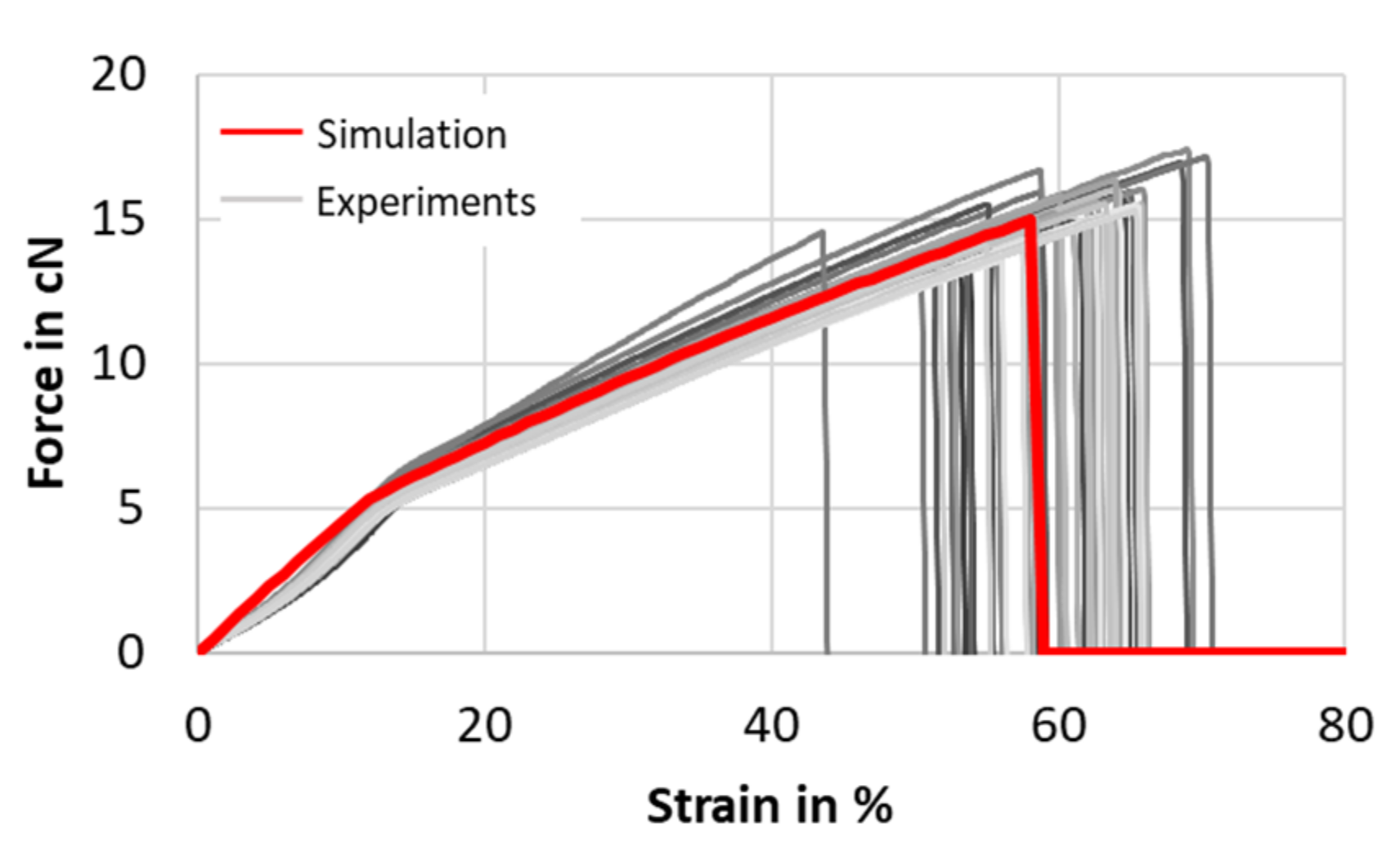
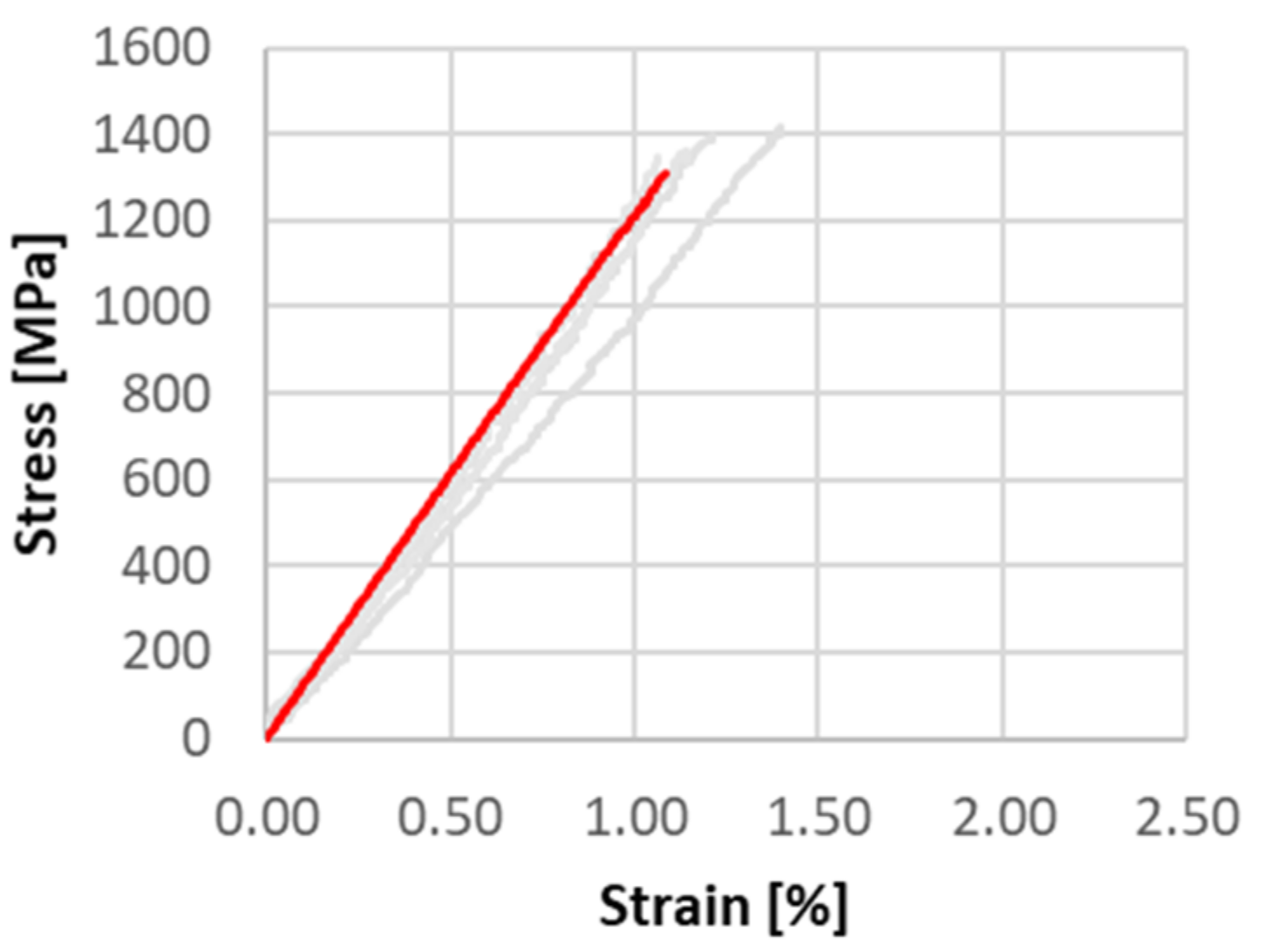
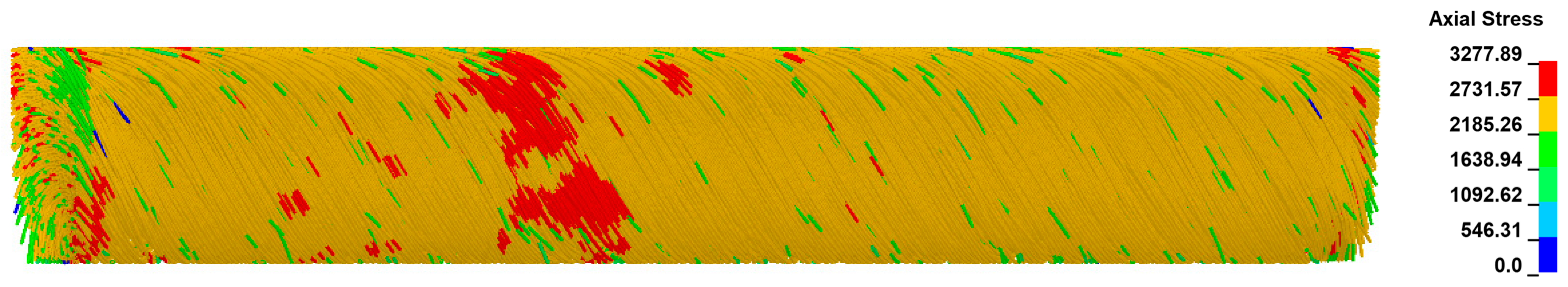
3.1. Model Validation
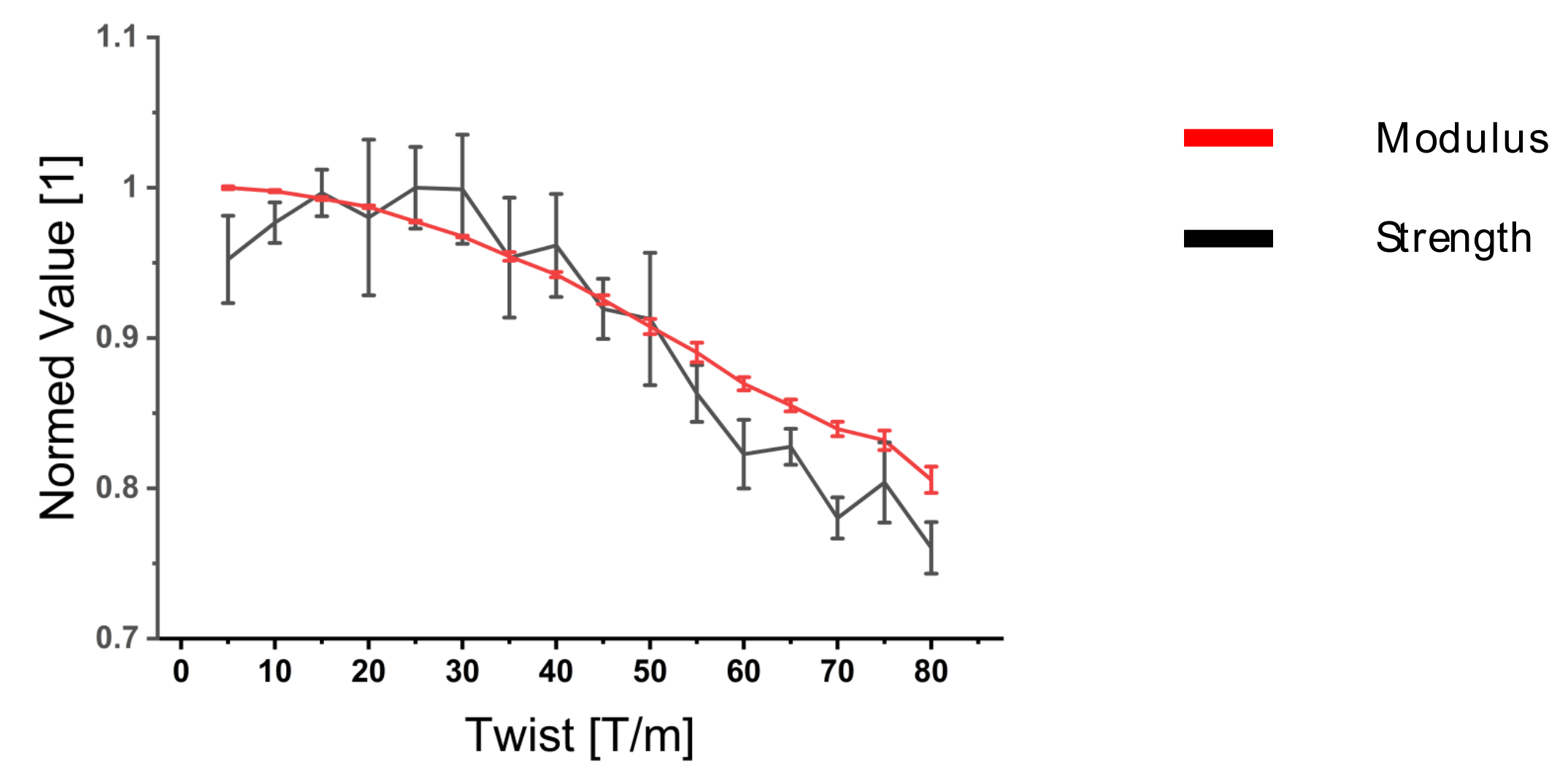
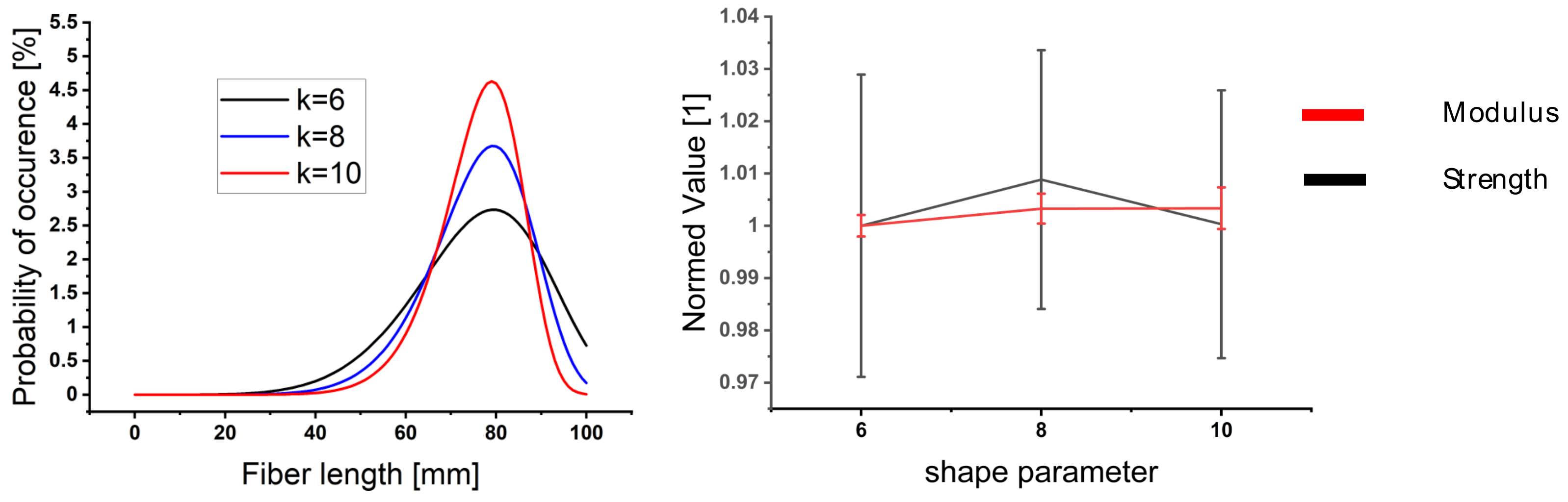
3.2. Parameter Study on Yarn Geometry
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- May, D.; Goergen, C.; Friedrich, K. Multifunctionality of polymer composites based on recycled carbon fibers: A review. Adv. Ind. Eng. Polym. Res. 2021, 4, 70–81. [Google Scholar] [CrossRef]
- Wölling, J.; Schmieg, M.; Manis, F.; Drechsler, K. Nonwovens from recycled carbon fibres—Comparison of processing technologies. Procedia CIRP 2017, 66, 271–276. [Google Scholar] [CrossRef]
- Stegschuster, G.; Schlichter, S. Perspectives of web based composites from RCF material. IOP Conf. Ser. Mater. Sci. Eng. 2018, 406, 012022. [Google Scholar] [CrossRef]
- Wei, H.; Nagatsuka, W.; Lee, H.; Ohsawa, I.; Sumimoto, K.; Wan, Y.; Takahashi, J. Mechanical properties of carbon fiber paper reinforced thermoplastics using mixed discontinuous recycled carbon fibers. Adv. Compos. Mater. 2018, 27, 19–34. [Google Scholar] [CrossRef]
- Han, H.; Wang, X.; Wu, D. Mechanical properties, morphology and crystallization kinetic studies of bio-based thermoplastic composites of poly(butylene succinate) with recycled carbon fiber. J. Chem. Technol. Biotechnol. 2013, 88, 1200–1211. [Google Scholar] [CrossRef]
- Yan, G.; Wang, X.; Wu, D. Development of lightweight thermoplastic composites based on polycarbonate/acrylonitrile-butadiene-styrene copolymer alloys and recycled carbon fiber: Preparation, morphology, and properties. J. Appl. Polym. Sci. 2013, 129, 3502–3511. [Google Scholar] [CrossRef]
- Hirayama, D.; Saron, C.; Botelho, E.C.; Costa, M.L.; Ancelotti Junior, A.C. Polypropylene composites manufactured from recycled carbon fibers from aeronautic materials waste. Mater. Res. 2017, 20, 526–531. [Google Scholar] [CrossRef]
- Yu, H.; Potter, K.D.; Wisnom, M.R. A novel manufacturing method for aligned discontinuous fibre composites (High Performance-Discontinuous Fibre method). Compos. Part A Appl. Sci. Manuf. 2014, 65, 175–185. [Google Scholar] [CrossRef]
- Akonda, M.; Stefanova, M.; Potluri, P.; Shah, D. Mechanical properties of recycled carbon fibre/polyester thermoplastic tape composites. J. Compos. Mater. 2017, 51, 2655–2663. [Google Scholar] [CrossRef]
- Khurshid, M.F.; Hasan, M.M.B.; Abdkader, A.; Cherif, C. Processing of waste carbon and polyamide fibers for high performance thermoplastic composites: A novel manufacturing technology for unidirectional tapes structure. J. Ind. Text. 2022, 51, 7256S–7276S. [Google Scholar] [CrossRef]
- Khurshid, M.F.; Hengstermann, M.; Hasan, M.M.B.; Abdkader, A.; Cherif, C. Recent developments in the processing of waste carbon fibre for thermoplastic composites—A review. J. Compos. Mater. 2020, 54, 1925–1944. [Google Scholar] [CrossRef]
- Pakdel, E.; Kashi, S.; Varley, R.; Wang, X. Recent progress in recycling carbon fibre reinforced composites and dry carbon fibre wastes. Resour. Conserv. Recycl. 2021, 166, 105340. [Google Scholar] [CrossRef]
- Khurshid, M.F.; Abdkader, A.; Cherif, C. Processing of waste carbon and polyamide fibres for high-performance thermoplastic composites: Influence of carding parameters on fibre orientation, fibre length and sliver cohesion force. J. Text. Inst. 2020, 111, 1277–1287. [Google Scholar] [CrossRef]
- Hengstermann, M.; Raithel, N.; Abdkader, A.; Hasan, M.M.B.; Cherif, C. Development of new hybrid yarn construction from recycled carbon fibers for high performance composites. Part-I: Basic processing of hybrid carbon fiber/polyamide 6 yarn spinning from virgin carbon fiber staple fibers. Text. Res. J. 2016, 86, 1307–1317. [Google Scholar] [CrossRef]
- Hengstermann, M.; Hasan, M.M.B.; Abdkader, A.; Cherif, C. Development of a new hybrid yarn construction from recycled carbon fibers (rCF) for high-performance composites. Part-II: Influence of yarn parameters on tensile properties of composites. Text. Res. J. 2017, 87, 1655–1664. [Google Scholar] [CrossRef]
- Hasan, M.M.B.; Nitsche, S.; Abdkader, A.; Cherif, C. Carbon fibre reinforced thermoplastic composites developed from innovative hybrid yarn structures consisting of staple carbon fibres and polyamide 6 fibres. Compos. Sci. Technol. 2018, 167, 379–387. [Google Scholar] [CrossRef]
- Goergen, C.; Schommer, D.; Duhovic, M.; Mitschang, P. Deep drawing of organic sheets made of hybrid recycled carbon and thermoplastic polyamide 6 staple fiber yarns. J. Thermoplast. Compos. Mater. 2020, 33, 754–778. [Google Scholar] [CrossRef]
- Miyake, T.; Imaeda, S. A dry aligning method of discontinuous carbon fibers and improvement of mechanical properties of discontinuous fiber composites. Adv. Manuf. Polym. Compos. Sci. 2016, 2, 117–123. [Google Scholar] [CrossRef]
- Cox, H.L. The elasticity and strength of paper and other fibrous materials. Br. J. Appl. Phys. 1952, 3, 72–79. [Google Scholar] [CrossRef]
- Zhao, P.; Ji, S. Refinements of shear-lag model and its applications. Tectonophysics 1997, 279, 37–53. [Google Scholar] [CrossRef]
- Nairn, J.A. On the use of shear-lag methods for analysis of stress transfer in unidirectional composites. Mech. Mater. 1997, 26, 63–80. [Google Scholar] [CrossRef]
- Fukuda, H.; Chou, T.-W. An Advanced Shear-Lag Model Applicable to Discontinuous Fiber Composites. J. Compos. Mater. 1981, 15, 79–91. [Google Scholar] [CrossRef]
- Pan, N. Theoretical determination of the optimal fiber volume fraction and fiber-matrix property compatibility of short fiber composites. Polym. Compos. 1993, 14, 85–93. [Google Scholar] [CrossRef]
- Shah, D.U.; Schubel, P.J.; Clifford, M.J. Modelling the effect of yarn twist on the tensile strength of unidirectional plant fibre yarn composites. J. Compos. Mater. 2013, 47, 425–436. [Google Scholar] [CrossRef]
- Azzi, V.D.; Tsai, S.W. Anisotropic strength of composites. Exp. Mech. 1965, 5, 283–288. [Google Scholar] [CrossRef]
- Breite, C.; Melnikov, A.; Turon, A.; de Morais, A.; Otero, F.; Mesquita, F.; Costa, J.; Mayugo, J.; Guerrero, J.; Gorbatikh, L.; et al. Blind benchmarking of seven longitudinal tensile failure models for two virtual unidirectional composites. Compos. Sci. Technol. 2021, 202, 108555. [Google Scholar] [CrossRef]
- Breite, C.; Melnikov, A.; Turon, A.; de Morais, A.; Le Bourlot, C.; Maire, E.; Schöberl, E.; Otero, F.; Mesquita, F.; Sinclair, I.; et al. Detailed experimental validation and benchmarking of six models for longitudinal tensile failure of unidirectional composites. Compos. Struct. 2022, 279, 114828. [Google Scholar] [CrossRef]
- Tavares, R.P.; Otero, F.; Turon, A.; Camanho, P.P. Effective simulation of the mechanics of longitudinal tensile failure of unidirectional polymer composites. Int. J. Fract. 2017, 208, 269–285. [Google Scholar] [CrossRef]
- Henry, J.; Pimenta, S. Semi-analytical simulation of aligned discontinuous composites. Compos. Sci. Technol. 2017, 144, 230–244. [Google Scholar] [CrossRef]
- Henry, J.; Pimenta, S. Modelling hybrid effects on the stiffness of aligned discontinuous composites with hybrid fibre-types. Compos. Sci. Technol. 2017, 152, 275–289. [Google Scholar] [CrossRef]
- Bailakanavar, M.; Liu, Y.; Fish, J.; Zheng, Y. Automated modeling of random inclusion composites. Eng. Comput. 2014, 30, 609–625. [Google Scholar] [CrossRef]
- Breuer, K.; Stommel, M. RVE modelling of short fiber reinforced thermoplastics with discrete fiber orientation and fiber length distribution. SN Appl. Sci. 2020, 2, 91. [Google Scholar] [CrossRef]
- Okabe, T.; Sasayama, T.; Koyanagi, J. Micromechanical simulation of tensile failure of discontinuous fiber-reinforced polymer matrix composites using Spring Element Model. Compos. Part A Appl. Sci. Manuf. 2014, 56, 64–71. [Google Scholar] [CrossRef]
- Abbassi, F.; Gherissi, A.; Zghal, A.; Mistou, S.; Alexis, J. Micro-Scale Modeling of Carbon-Fiber Reinforced Thermoplastic Materials. Appl. Mech. Mater. 2011, 146, 1–11. [Google Scholar] [CrossRef]
- Wongsto, A.; Li, S. Micromechanical FE analysis of UD fibre-reinforced composites with fibres distributed at random over the transverse cross-section. Compos. Part A Appl. Sci. Manuf. 2005, 36, 1246–1266. [Google Scholar] [CrossRef]
- Harper, L.T.; Qian, C.; Turner, T.A.; Li, S.; Warrior, N.A. Representative volume elements for discontinuous carbon fibre composites—Part 1: Boundary conditions. Compos. Sci. Technol. 2012, 72, 225–234. [Google Scholar] [CrossRef]
- Fliegener, S.; Luke, M.; Gumbsch, P. 3D microstructure modeling of long fiber reinforced thermoplastics. Compos. Sci. Technol. 2014, 104, 136–145. [Google Scholar] [CrossRef]
- Chen, L.; Gu, B.; Zhou, J.; Tao, J. Study of the Effectiveness of the RVEs for Random Short Fiber Reinforced Elastomer Composites. Fibers Polym 2019, 20, 1467–1479. [Google Scholar] [CrossRef]
- Döbrich, O.; Gereke, T.; Cherif, C. Modeling the mechanical properties of textile-reinforced composites with a near micro-scale approach. Compos. Struct. 2016, 135, 1–7. [Google Scholar] [CrossRef]
- Yang, S.H.; Woo, K.S.; Kim, J.J.; Ahn, J.S. Finite Element Analysis of RC Beams by the Discrete Model and CBIS Model Using LS-DYNA. Adv. Civ. Eng. 2021, 2021, 8857491. [Google Scholar] [CrossRef]
- Moharrami, M.; Koutromanos, I. Finite element analysis of damage and failure of reinforced concrete members under earthquake loading. Earthq. Engng. Struct. Dyn. 2017, 46, 2811–2829. [Google Scholar] [CrossRef]
- Hayashi, S.; Dougherty, S.; Hiroi, S.; Atsushi, Y. J-Composites/Compression Molding—Introducing New Simulation System for FRP Composites. In Proceedings of the 16th International LS-DYNA® Users Conference, Virtual, 10–11 June 2020; p. 10. [Google Scholar]
- Hübner, M.; Staiger, E.; Küchler, K.; Gereke, T.; Cherif, C. Simulation of Patched Woven Fabric Composite Structures Under Tensile Load. Tekstilec 2016, 59, 175–181. [Google Scholar] [CrossRef]
- Jiang, W.-G. Implementation of domain superposition technique for the nonlinear analysis of composite materials. J. Compos. Mater. 2013, 47, 243–249. [Google Scholar] [CrossRef]
- Jiang, W.-G.; Hallett, S.R.; Wisnom, M.R. Development of Domain Superposition Technique for the Modelling of Woven Fabric Composites. In Mechanical Response of Composites; Oñate, E., Camanho, P.P., Dávila, C.G., Pinho, S.T., Remmers, J.J.C., Eds.; Springer: Dordrecht, The Netherlands, 2008; pp. 281–291. ISBN 978-1-4020-8583-3. [Google Scholar]
- Hasan, M.M.B.; Bachor, S.; Abdkader, A.; Cherif, C. Low Twist Hybrid Yarns from Long Recycled Carbon Fibres for High Performance Thermoplastic Composites. Mater. Sci. Forum 2022, 1063, 147–153. [Google Scholar] [CrossRef]
- Abdkader, A.; Hasan, M.M.B.; Bachor, S.; Cherif, C. Mechanical properties of composites manufactured from low twist hybrid yarns made of discontinuous carbon and polyamide 6 fibres. J. Thermoplast. Compos. Mater. 2022. online. [Google Scholar] [CrossRef]
- Hengstermann, M.; Kopelmann, K.; Nocke, A.; Abdkader, A.; Cherif, C. Development of a new hybrid yarn construction from recycled carbon fibres for high-performance composites: Part IV: Measurement of recycled carbon fibre length. J. Eng. Fibers Fabr. 2020, 15. [Google Scholar] [CrossRef]
- Hearle, J.W.S.; Grosberg, P.; Backer, S. Structural mechanics of fibers, yarns, and fabrics; Wiley-Interscience: New York, NY, USA, 1969; ISBN 978-0-471-36669-0. [Google Scholar]
- Feder, J. Random sequential adsorption. J. Theor. Biol. 1980, 87, 237–254. [Google Scholar] [CrossRef]
- Virtanen, P.; Gommers, R.; Oliphant, T.E.; Haberland, M.; Reddy, T.; Cournapeau, D.; Burovski, E.; Peterson, P.; Weckesser, W.; Bright, J.; et al. SciPy 1.0: Fundamental algorithms for scientific computing in Python. Nat. Methods 2020, 17, 261–272. [Google Scholar] [CrossRef]








| Characteristics | rCF | PA 6 |
|---|---|---|
| Fiber Diameter [µm] | 5.6 ± 2.8 | 3.6 ± 0.1 |
| Fiber Fineness [dtex] | 0.4 ± 0.1 | 20.8 ± 3.7 |
| Fiber Strength [MPa] | 3360 ± 628 | 537.4 ± 32 |
| Fiber Young’s Modulus [GPa] | 227 ± 40 | 1.7 ± 0.1 |
| Elongation at Break [%] | 1.7 ± 0.3 | 59.7 ± 4.4 |
| Density [g/cm3] | 1.8 | 1.14 |
| Twist [T/m] | Fiber Volume Content [%] | Tensile Strength [MPa] | Tensile Modulus [GPa] |
|---|---|---|---|
| 20 | 57 ± 1 | 1453 ± 27 | 94 ± 6 |
| 30 | 54 ± 1 | 1364 ± 49 | 90 ± 5 |
| 50 | 54 ± 1 | 1292 ± 56 | 77 ± 4 |
| 75 | 55 ± 2 | 1267 ± 47 | 77 ± 5 |
| Property | Value |
|---|---|
| Fiber Diameter | 0.05 mm |
| Beam Element length | 0.1 mm |
| Solid Element edge length | 0.25 mm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lang, T.G.; Hasan, M.M.B.; Abdkader, A.; Cherif, C.; Gereke, T. Micro-Scale Model of rCF/PA6 Spun Yarn Composite. J. Compos. Sci. 2023, 7, 66. https://doi.org/10.3390/jcs7020066
Lang TG, Hasan MMB, Abdkader A, Cherif C, Gereke T. Micro-Scale Model of rCF/PA6 Spun Yarn Composite. Journal of Composites Science. 2023; 7(2):66. https://doi.org/10.3390/jcs7020066
Chicago/Turabian StyleLang, Tobias Georg, Mir Mohammad Badrul Hasan, Anwar Abdkader, Chokri Cherif, and Thomas Gereke. 2023. "Micro-Scale Model of rCF/PA6 Spun Yarn Composite" Journal of Composites Science 7, no. 2: 66. https://doi.org/10.3390/jcs7020066
APA StyleLang, T. G., Hasan, M. M. B., Abdkader, A., Cherif, C., & Gereke, T. (2023). Micro-Scale Model of rCF/PA6 Spun Yarn Composite. Journal of Composites Science, 7(2), 66. https://doi.org/10.3390/jcs7020066








