On the Influence of Wave-Shaped Tool Path Strategies on Geometric Accuracy in Incremental Sheet Forming

Abstract

In incremental sheet forming (ISF), the geometrical accuracy is still a challenge that is only solved for specific applications. The underlying mechanisms of geometrical defects in ISF are very complex and still not fully understood. Nevertheless, the process understanding is constantly evolving. Recent work has shown, for example, how bending moments resulting from residual stresses affect geometric accuracy. It has become clear that resulting bending moments with an axis parallel to the main tool path direction are dominant. Based on that, the current paper investigates the hypothesis that linear and parallel tool paths lead to an unfavourable accumulation of residual bending moments along a common axis, and whether this accumulation effect can be reduced by wave-shaped tool paths. Thus, the described research investigates the influence of novel path strategies on the residual bending moments and the resulting geometrical deviations. The path strategies are based on wave-shaped path lines, whereas the curvature is within the sheet plane. The investigations focussed on a rectangular sheet that is clamped at its shortest edges and a part geometry-sensitive to springback. Experimental and numerical investigations show a significantly positive influence of some investigated path strategies on the geometric deviation, compared to a conventional path strategy.

1. Introduction

Incremental sheet forming (ISF) is a manufacturing technology with a high potential for the production of prototypes and small series due to its high flexibility. The flexibility arises from the digitally controlled geometry definition in the process and the resulting low tooling effort. Nevertheless, the utilization of this technology to the wide range of possible sheet metal products with the given geometric tolerances is still a great challenge and subject of current research [1]. In the recent decades, the research community has been investigating a wide range of process parameters and their influence on formability and geometrical accuracy. Thus, significant knowledge has been collected about the influence of many influencing variables on the process. However, a holistic understanding of the accumulated influence of the manifold influencing variables on the final geometric accuracy of the sheet metal parts after trimming and springback is still missing, especially regarding the large variety and complexity of possible geometries of sheet metal products [2]. Many investigations in the past have focussed on simple pyramids and cones, which is very useful for the investigation of formability, but has some drawbacks regarding the understanding of geometric deviation. One significant issue is that pyramids and cones have a very strong inherent geometrical stiffness and are thus not very sensitive to geometrical distortions. Therefore, process strategies, especially tool path strategies developed for test geometries like pyramids and cones, are not always transferable to other types of geometries.

Nevertheless, it is well known from the literature that the selected tool path has a crucial influence on the geometric accuracy [3]. The optimisation of the tool path has, in some cases, led to significant improvements in geometric accuracy.

In addition, there is also knowledge about the residual stresses arising from the process and the resulting bending moments that trigger geometric distortion after forming. It is known that parameters such as tool diameter and feed (tool step-down) influence the resulting stresses, but not how the resulting bending moments can be specifically influenced.

It has been shown that on pyramid walls, there is an unintended curvature of the sheet after forming and trimming in tool path direction and transverse to the tool path direction [4]. The sheets curl towards the tool contact face and the curvature in feed direction is dominant. That means, the dominant residual bending moment, causing this curvature, is oriented around an axis parallel to the tool path direction (see Figure 1).

According to the experience of the authors, the effect of curling of the sheet surface or springback is particularly strong when forming an unstiffened geometry with a linear and parallel tool path. Considering the dominant residual moment around the tool path direction, this has led to the following hypotheses:

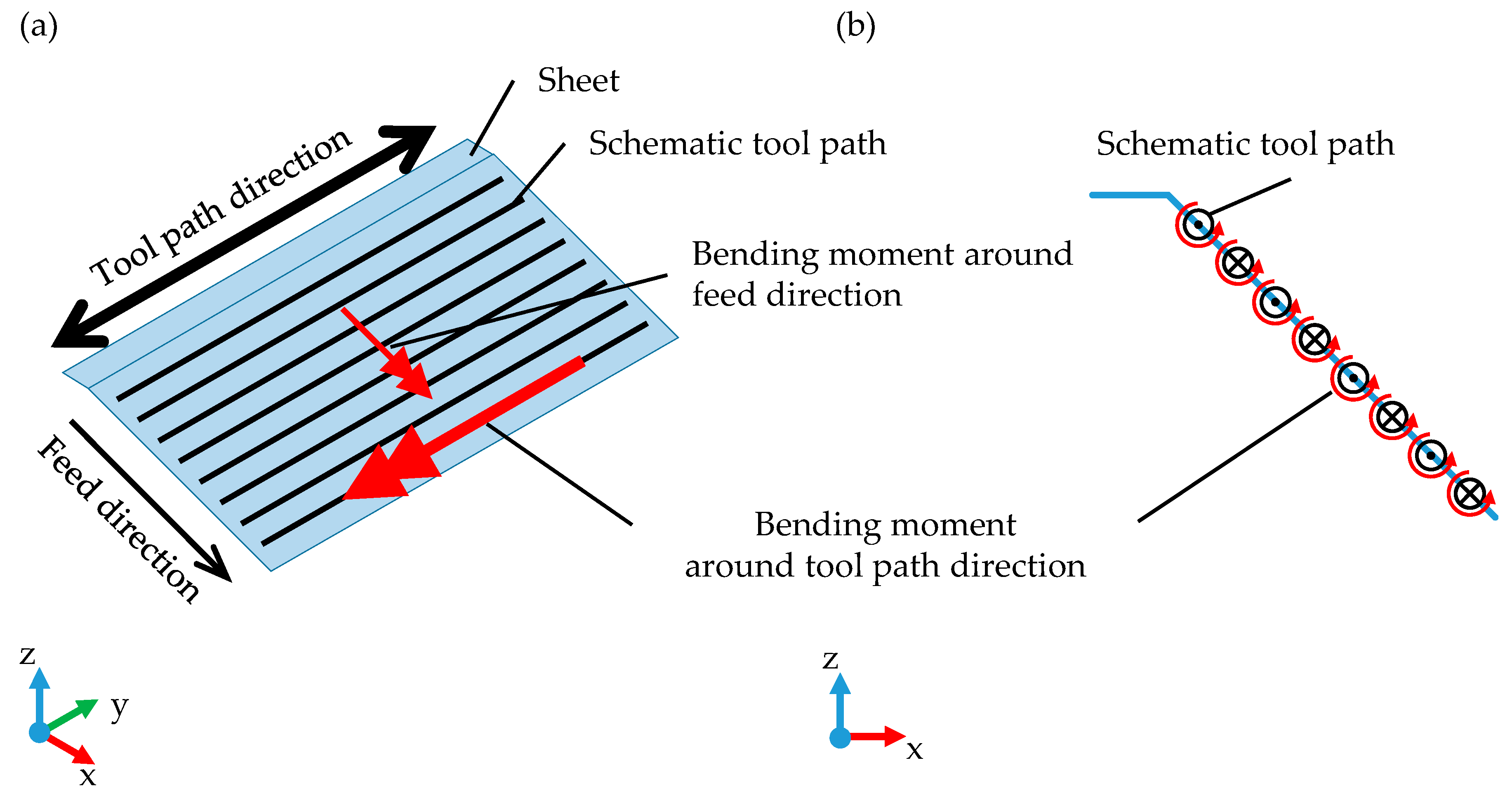
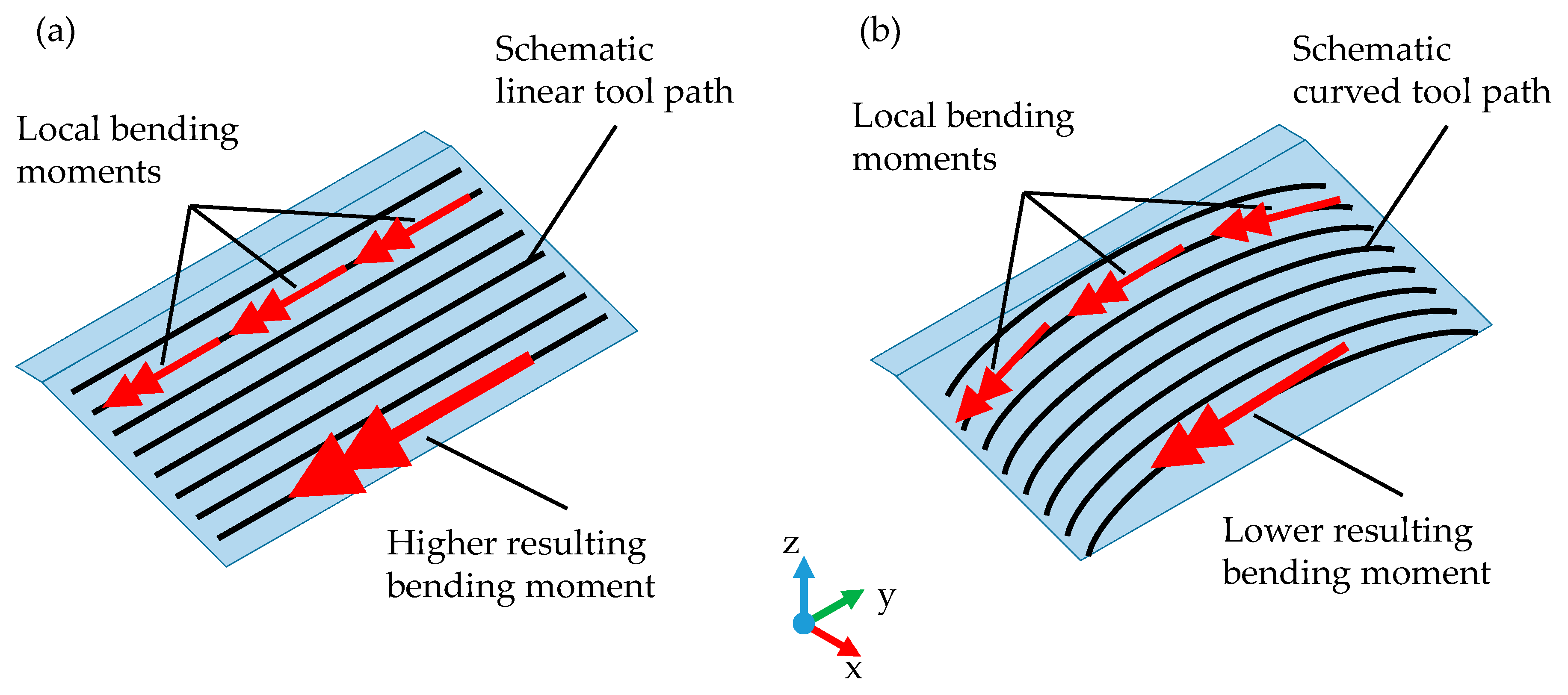
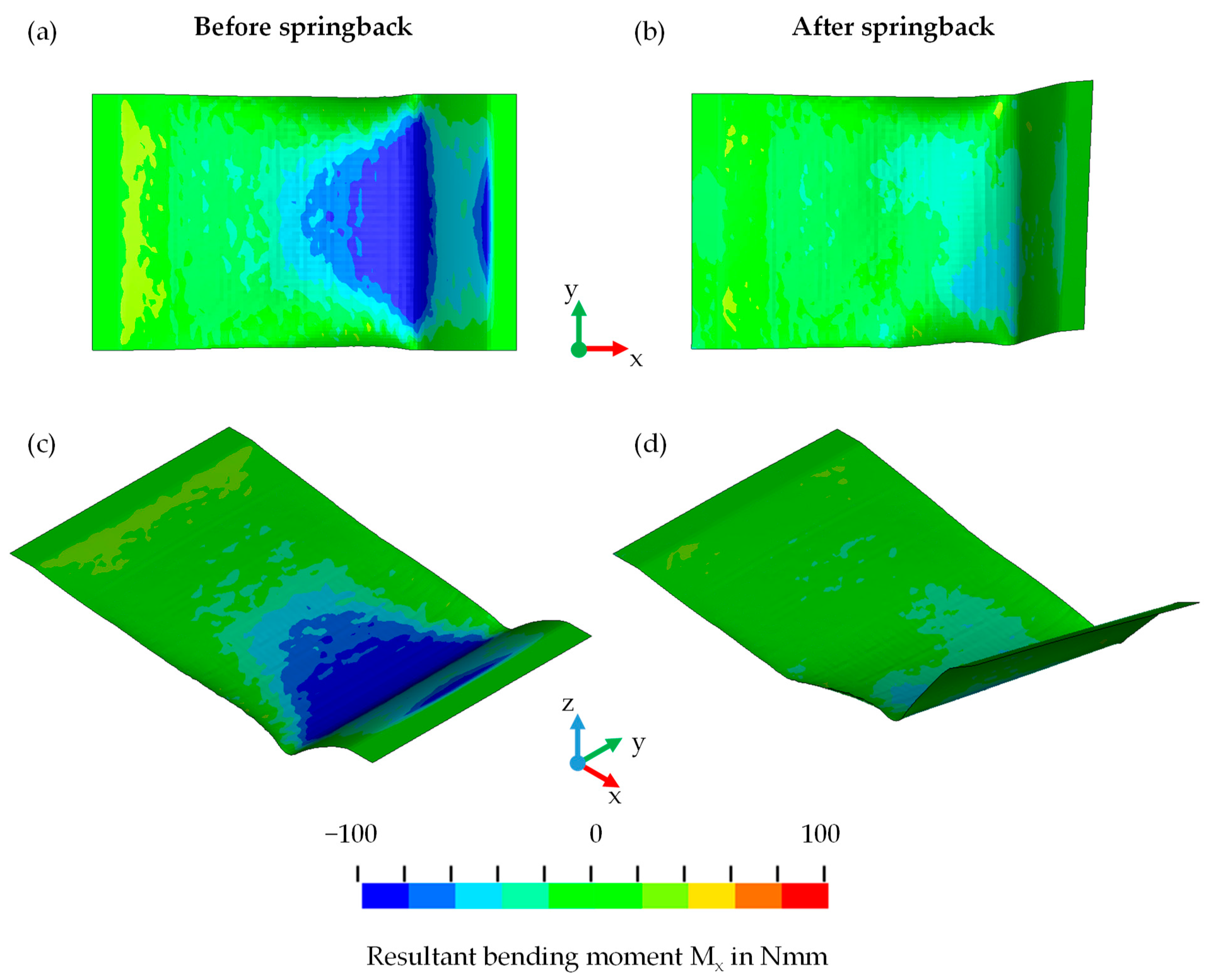
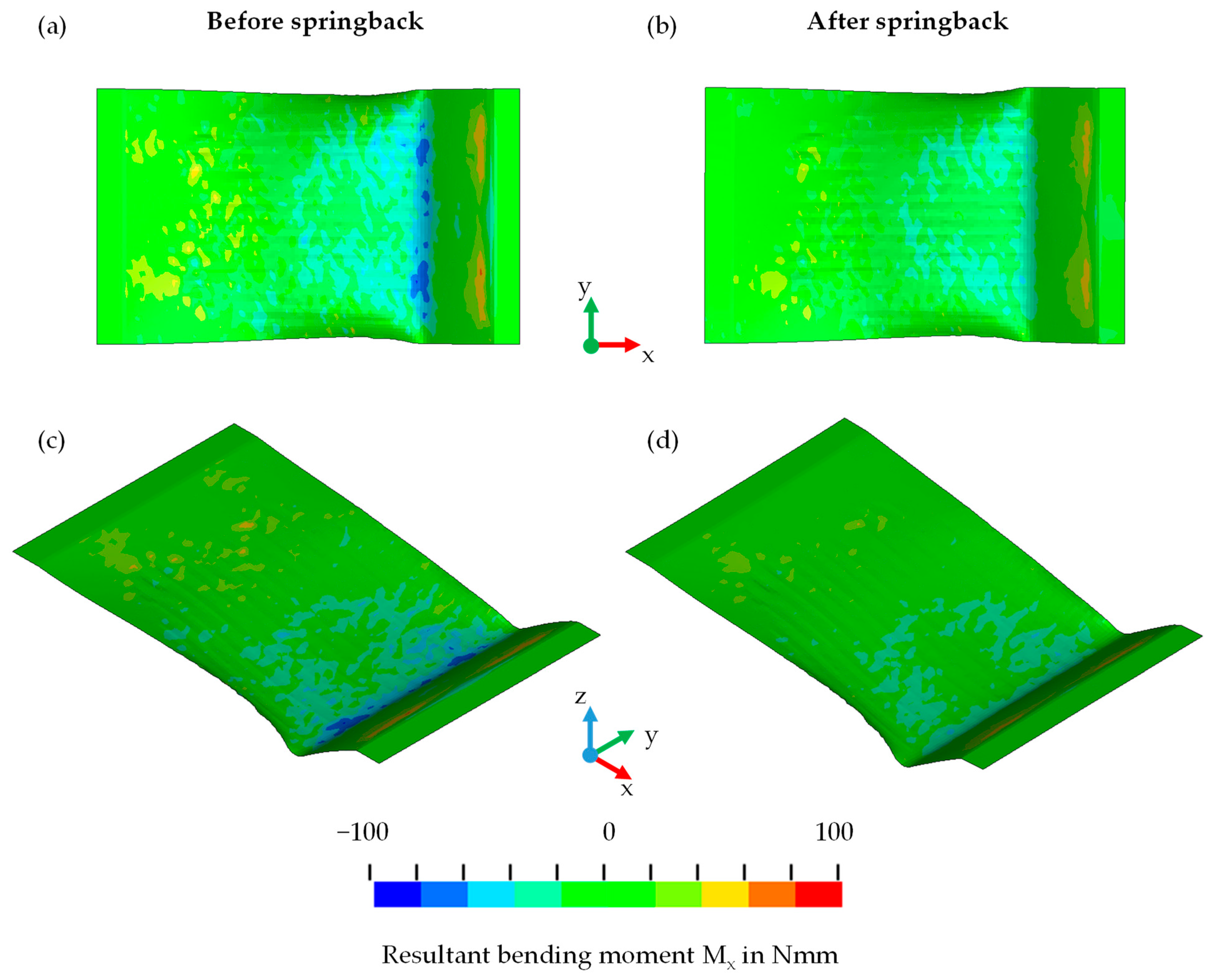
• On a linear and parallel tool path, all axes of local residual bending moments are also parallel, and an accumulated, amplified bending moment results around a united overall axis (see Figure 2a).
• On a curved tool path, the axes of local bending moments are tangential to the path and thus not parallel to each other. This prevents an accumulation of bending moments around a united overall axis and, in contrast, leads to a mutual interlocking of the bending moments, rather than an amplification (see Figure 2b).

It is important to mention that the curvature or the waves of the tool path are parallel to the plane of the sheet surface. That means this approach is not comparable with concepts of oscillating or hammering forming tools. Rather, the idea is to overlay conventional contour line paths (like z-level or streamline) with a slight curvature of the path lines or a wavy displacement. That means the general, global tool path strategy can stay a conventional contour line path, which makes this approach very flexible and applicable to many part geometries. To investigate the abovementioned hypotheses, the influence of linear, curved, and wave-shaped tool paths on the geometric accuracy has been investigated and is described in this paper.

State of the Art

Incremental sheet forming is a flexible manufacturing process for the production of prototypes and small series parts. Starting from a flat sheet metal, CNC-generated tool paths are followed to form the target geometry of the part to be produced [5]. During incremental sheet forming, several types of springback can be observed (during forming, after unclamping, and after trimming) [6]. There are several existing studies on the reduction of springback by varying the process parameters.

The review paper by Lu et al. [7] summarizes the main factors influencing geometric accuracy as tool path, tool diameter, tool step-down, tool speed, tool rotation speed, lubrication, sheet thickness, material properties, and part geometry. Additionally, the review paper categorises the countermeasures investigated in the literature into five categories: the development of ISF process variants, multi-stage strategies, tool path correction/optimisation, feedback control, and some alternative strategies. The development of ISF process variants includes the development of hybrid forming processes. For example, Araghi et al. [8] preceded the ISF with a stretch forming process. According to the authors, this process combination led not only to a more homogeneous distribution of the sheet thinning, but also to superimposed tensile stresses in the material, which could reduce the remaining residual stresses after the ISF. The reduction of residual stresses can have an influence on the geometric accuracy after springback.

Another approach is the use of multi-stage strategies. Here, the part is not formed in one step but in several intermediate steps, i.e., the part is divided into preforms. This can improve the general geometrical accuracy [9]. Zhu et al. [10] were also able to achieve an improvement in thickness distribution by applying multi-stage strategies. Wei et al. [11] were additionally able to increase the geometric accuracy through a heat treatment in which residual stresses are relieved, thereby reducing springback.

Two further significant possibilities for increasing geometric accuracy are tool path correction/optimisation strategies and feedback control. Möllensiep et al. [12], for example, were able to reduce the deviations using a compensation algorithm with different test geometries at elevated temperatures. Regression models were used to predict the component accuracy of the benchmark geometries investigated as a function of various tool path parameters. Using the trained models, the authors were able to significantly increase the part accuracy. According to Lu et al. [7], such and other compensation algorithms offer a significant potential to increase the accuracy, but also increase the complexity of the process and still require further research.

These compensation approaches based on tool paths and the conclusions of the review papers by Lu et al. [7] and Gatea et al. [3] emphasize the strong influence of the applied tool path on the geometric accuracy, in particular on the degree of springback. This significant influence resulted in the development and investigation of novel tool path strategies. Chang et al. [13] developed a point-contact tool path (PCTP) algorithm in which the tool only has point contact with the sheet to be formed during the forming process, thus preventing circumferential relative movement. In the investigations, an increase in geometrical accuracy was observed with a decrease in the surface quality of the part. Another variation from commonly used tool paths was investigated by Grimm et al. [14]. The influence of a wavelike, hammering tool path on the surface properties of pyramids was examined, whereby the tool was not in constant contact with the sheet. The oscillation of the tool path was vertical to the sheet plane and not in the sheet plane. An examination focussing the influence on the geometric properties and the resulting bending moments did not take place.

In many of these works, influencing factors on the geometric accuracy have been described clearly, but the underlying mechanisms often cannot be fully explained yet. Hirt et al. [2] summarized and categorized the mechanisms of the formation of geometrical defects in ISF. They concluded that even if the described mechanisms can explain the origin of the described types of defects qualitatively to some extent, a reliable quantitative description of the correlations is still lacking.

Chang et al. [15], for example, investigated approaches to quantitatively describe one of these mechanisms. The authors used an analytical model to describe the mechanism that leads to the twisting effect in ISF processes. Part of this model is the analysis of the stress state after forming and a resulting moment, with the help of which the twisting of the formed pyramids could be described.

Maqbool and Bambach [16] performed detailed investigations regarding the underlying mechanisms of ISF. Within a numerical simulation study, they identified dominant deformation modi as the main cause for the above-mentioned springback. In the paper, they concluded that the deformation mechanism in single-point incremental forming (SPIF) is always a combination of the deformation modi “membrane stretching”, “bending”, and “through-thickness shear”, whereby the relative proportions are influenced by the process parameters. For process parameters that cause a high proportion of the “bending deformation mode” (i.e., a large tool diameter or feed), a stronger springback and thus a lower geometric accuracy could be observed. In addition, Maqbool et al. [4] were able to give a deeper insight into the development of geometric deviations in pyramid walls on the basis of stress measurements and the resulting bending moments. The distribution of the resulting stresses was evaluated on pyramids formed using SPIF. Parameters such as tool diameter, tool step-down and wall angle were adjusted. The curvature of the bulges in the side walls after springback was determined with strips cut out of the formed pyramid (in the path direction and transversely). This curvature is a consequence of the remaining stress distribution after forming and is directly dependent on the investigated process parameters. With the help of an FE model, the change in bending moments after unloading and trimming was also calculated. The results demonstrated the high influence of the previously mentioned process parameters on the bending moments. The bending moments and their change after unloading/trimming were significantly responsible for the springback. The highest change in the bending moments occurred with the largest tool diameter and the highest tool step-down. According to the authors, the resulting bending moments and thus the springback were highest transverse to the tool path direction. That means the axis of the dominant resulting bending moment is parallel to the path direction (compare Figure 1).

It is still not known to what extent these effects can be influenced by the choice of path strategy. Nevertheless, the observation of the sheet surface curling towards the tool contact face and the dominance of the curling along the axis of the tool motion is in very good agreement with the experimental experience of the authors of the current paper.

The literature described shows that the effects of the most important ISF process parameters, such as the tool path, on the geometric accuracy are generally known, and strategies to avoid or compensate for deviations for certain test geometries have already been investigated in depth. The process understanding of residual stresses, bending moments, and resulting geometric deviations is also steadily increasing. However, there is still a need to enhance the understanding of the underlying mechanisms as well as the interaction of different mechanism and to develop solutions that can be applied to a wide range of geometries.

2. Materials and Methods

The investigations described in this paper were conducted with the test geometry illustrated in Figure 3. This test geometry was derived and generalized from former experiments with an application part, which revealed significant issues regarding the geometric accuracy. This geometry is large compared to the thickness. It has open sides and has a curvature/contour only in one direction. These facts lead to a very low inherent geometrical stiffness of the part and thus to a high sensitivity towards geometrical distortion. Additionally, the part has a shallow and a steep wall and the bottom of the part has a defined curvature that has to be formed as well.

The first trials with this kind of part, using variants of conventional streamline path strategies, led to cracks in the steep wall and a strong accumulation of material in the middle of the part. In the literature, the described effect is called the “pillow effect” [17]. Figure 4b shows the condition after unclamping, where the strong springback can already be anticipated. Figure 4c shows a schematic two-part streamline path in which material was first formed from the shallow area of the geometry (in Figure 4b top) to the centre and then from the steep area to the centre. This strategy led to the material build-up shown in Figure 4b. Another tested variant, with a streamline path going in one step from the steep to the shallow area, led to material failure in the steep area and material build-up in the shallow area.

The undeformed areas on the sides of the sheet originate from preliminary tests in which the described effects were observed. In following investigations and within the course of this work, the tool path was extended to or beyond the edges.

Based on this experience, the part was formed using a streamline path strategy starting from the shallow wall, along the bottom of the part, until the steep wall. This strategy avoided the accumulation of material in the part middle and rather pushing the material towards the steep wall angle, where it could compensate for successive thinning. As a result, it was possible to finish the part without cracks and without pillow effect. Nevertheless, after unclamping of the part, a severe distortion of the entire part was observed. This very strong experience of sheet curling using parallel tool paths made a significant contribution to the formulation of the hypotheses described in the introduction. In addition, it was evident that this kind of “open” non-stiffened geometry is ideal to demonstrate geometrical distortions after forming and to investigate countermeasures accordingly.

Following the hypotheses described in the introduction, the investigation focussed on the comparison of geometric deviation for different tool path strategies. Linear (streamline) tool paths are the reference, which showed very high geometric deviations. Additionally, curved and wave-shaped tool paths were used and compared (see Figure 5). To describe the wave-shaped tool paths, the parameters amplitude and spatial frequency or the number of waves per part width are used. In addition, a wave can be aligned/shifted on the part in such a way that the wave peak points in or opposite to the feed direction. In the following, wave-shaped or curved path strategies are called concave if the wave peak of the underlying wave points in the direction of the feed direction. The definition for convex strategies is analogous.

To generate the tool paths, sinusoidal functions of the form $f\left(x\right)=A·sin\left(\omega ·x+\theta \right)$ were used, with $A$: amplitude, $\omega$: spatial frequency or number of waves related to the component width and $\theta$: phase shift. Two superimposed sinusoidal functions can be added to generate the convex, concave wave-shaped tool paths. The phase shift can be used to shift the position of the wave peaks on the component. The obtained curves are then projected onto the target geometry and used to create the tool paths.

The parameter ranges were chosen in such a way that, within the scope of the investigated experiments, fundamental statements about the influence of the chosen parameters on the resulting geometry are possible.

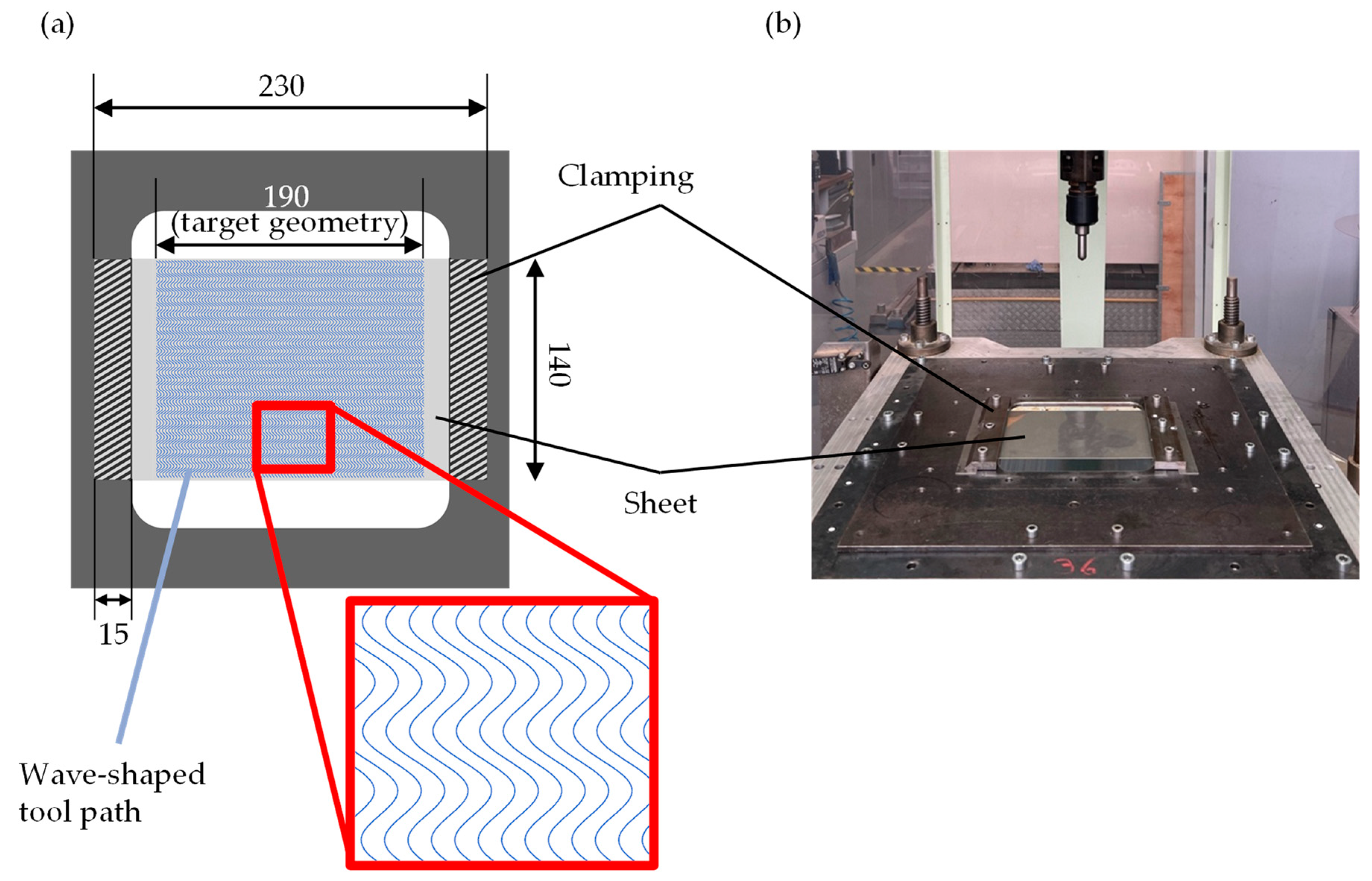
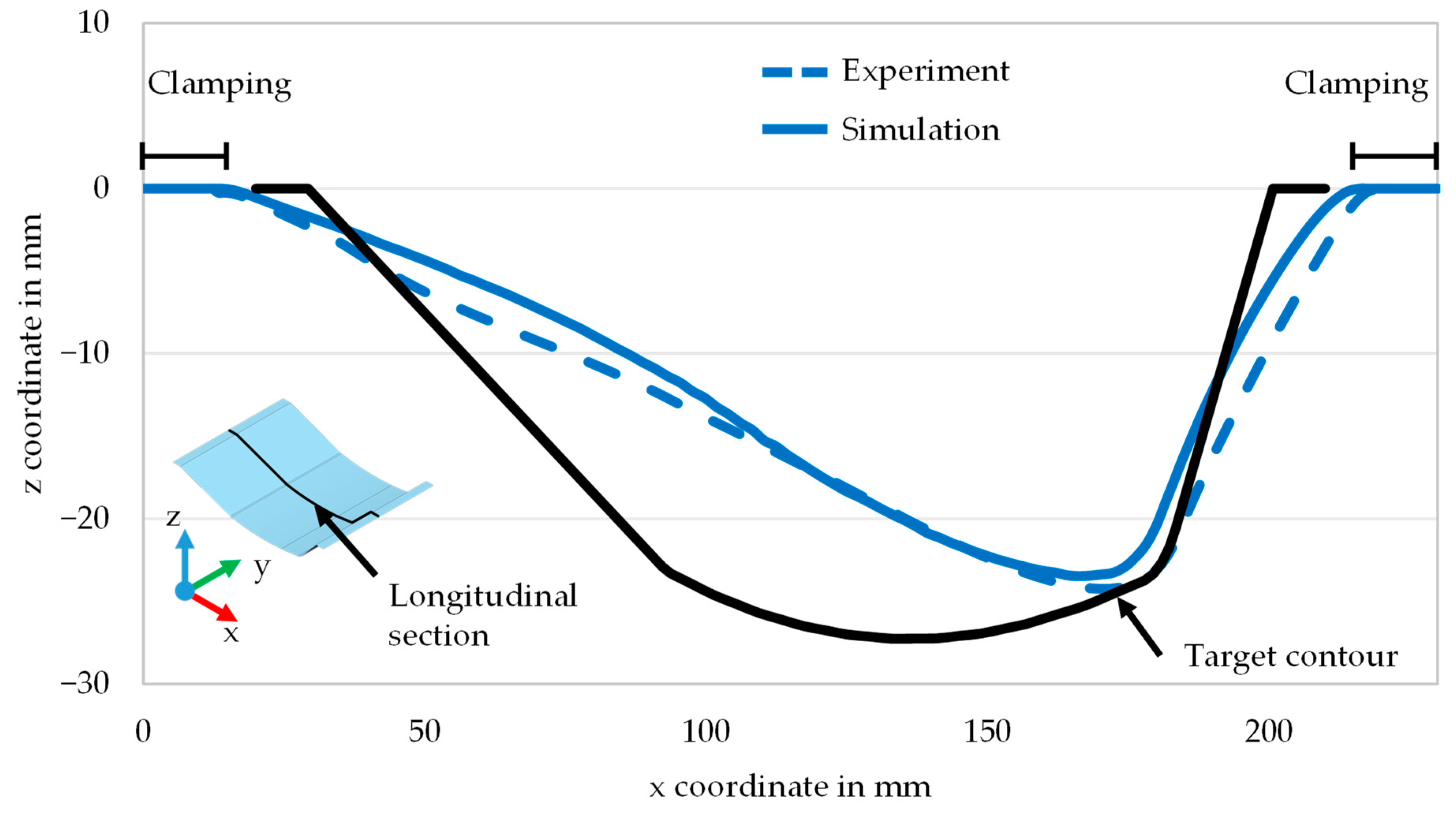
2.1. Experimental Setup

All experiments were carried out on a modified 3-axis milling machine from AMINO. A hemispherical tool with a diameter of 20 mm was used at a programmed speed of 2 m/min. Multidraw 472 drawing oil (Zeller + Gmelin GmbH & Co. KG, Eislingen/Fils, Germany) was used for lubrication. The material used in all experiments was a soft deep-drawing steel (1.0338) with a thickness of 1 mm. This material shows good formability according to its use as deep drawing steel and does not show any phase transformation during cold forming and is therefore suitable to investigate isolated dependencies of the selected tool paths for geometric deviations. The sheet was clamped on two sides along its short edges, as shown in Figure 3 and Figure 6. The tool paths were created using the CAD/CAM/CAE software Siemens NX 12.0.

After unclamping both sides and springback, the sheet was 3D-scanned using the HandySCAN 700 from Creaform 3D in order to examine the springback geometry. For a better comparison of the experiments and simulations, the results were virtually orientated to the short edge of the flat side of the geometry using GOM Inspect 2018, a software for the analysis of 3D data.

2.2. Finite Element (FE) Setup

The experiments were additionally simulated using LS-Dyna R12.0.0, developed by Livermore Software Technology Corporation in Livermore, California, in order to calculate the resulting bending moments according to the hypothesis mentioned in the introduction. The choice of numerical parameters followed a convergence and sensitivity analysis, fine-tuned to the results of geometric accuracy after the forming process (see Figure 7). An element size of 2 mm was used based on this analysis regarding the forming geometry (before springback). Shell elements (Belytschko-Tsay) have been used to model the sheet. They offer significant time savings compared to solid elements but still provide the ability to examine stress states. This type of element is based on Mindlin/Reissner plate theory and allows strain through thickness to be observed. Nine integration points were used to capture the stresses across the thickness. To model the clamping, the nodes of the shell elements were fixed in the required areas (see clamping in Figure 6). The tool has been modelled as a rigid body.

A remeshing strategy was not used because no distortion of the elements occurred during the forming process. The choice of numerical parameters is based on the work of Maqbool et al. [16] and Schmitz et al. [18]. In both works, high similarities between simulation and experiment could be achieved. In particular, in the work of Maqbool, satisfactory results were already achieved by the above-mentioned numerical parameters and the use of an explicit solver for the calculation of the forming process when considering the stress distribution and the resulting bending moments. The contact type “CONTACT_AUTOMATIC_SURFACE_TO_SURFACE” was used to describe the contact between tool and sheet metal, using a friction coefficient of 0.1. With the selected parameters, a total CPU time of up to 180 h (Intel Xeon E5 processor, with a processor base frequency of 3.30 GHz) was necessary.

Especially when modelling springback processes, the choice of material model is crucial. In this case, the Yoshida-Uemori model was used based on [19]. This material model uses a non-linear kinematic hardening of Yoshida and Uemori. The choice of a kinematic hardening model has already been successfully used in the work of Maqbool et al. For the material 1.0338, the required material parameters from Hassan et al. [20] could be used. These are summarised in

Table 1. Material parameters used in the Yoshida-Uemori hardening model, based on the work of Hassen et al. [20] for the steel used (1.0338).

Y in MPa	C	B in MPa	Rsat in MPA	m	h	b in MPa
180	425	700	700	56	0.69	300

for a better overview.

Hassan et al. were able to show on deep drawing simulations the necessity of using a decreasing Young’s modulus with the plastic deformation to predict the springback behaviour. The following equation by Yoshida [19] is used in this model to describe the degradation of the Young’s modulus:

$$E=E_0-\left(E_0-E_a\right)\left[1-\exp \left(-\zeta p\right)\right]$$

(1)

In the equation established by Yoshida, $E_0$ is the initial Young’s modulus and $E_a$ is the value of the Young’s modulus at infinitely large plastic strains. E_a is referred to as the saturation modulus, i.e., the value of Young’s modulus does not decrease further. $\zeta$ is a material parameter that controls the rate at which the Young’s modulus decreases. The springback simulation itself was performed using an implicit model. The other numerical parameters were adopted unaltered for the springback simulation.

The experimental and numerical geometric results were compared using GOM Inspect. For this purpose, sections were placed through the geometries to analyse the springback. For the analysis of the bending moments, these were evaluated along sections longitudinally through the formed geometry.

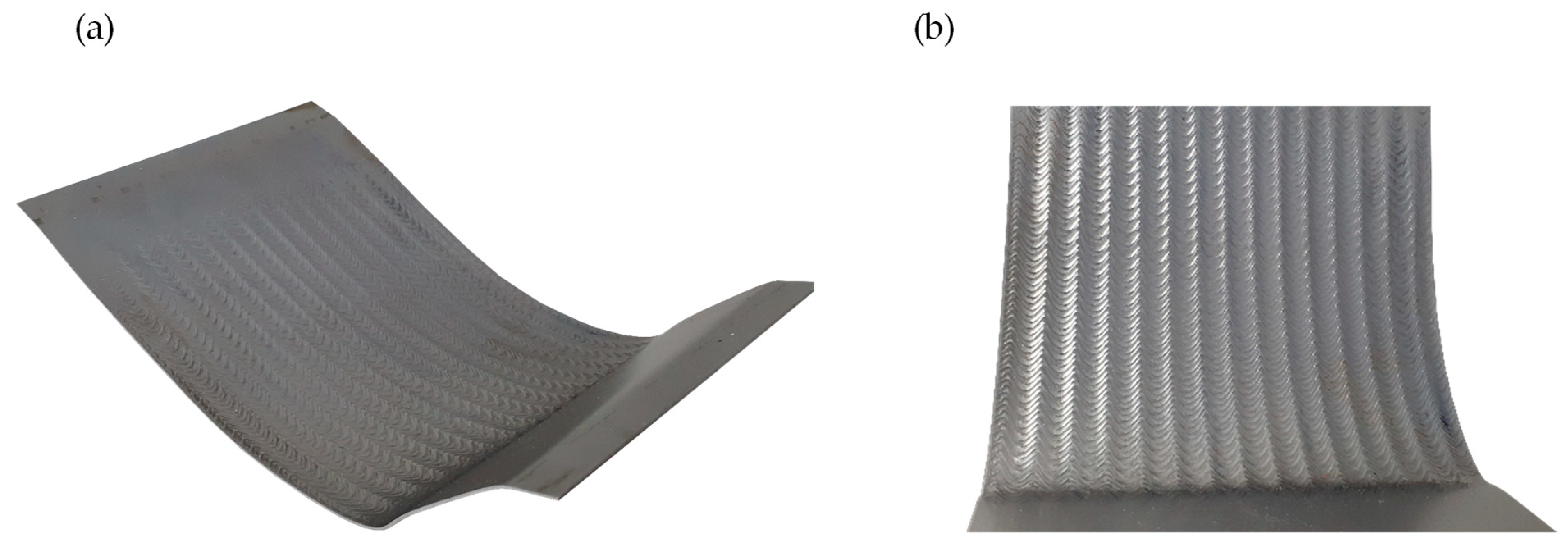
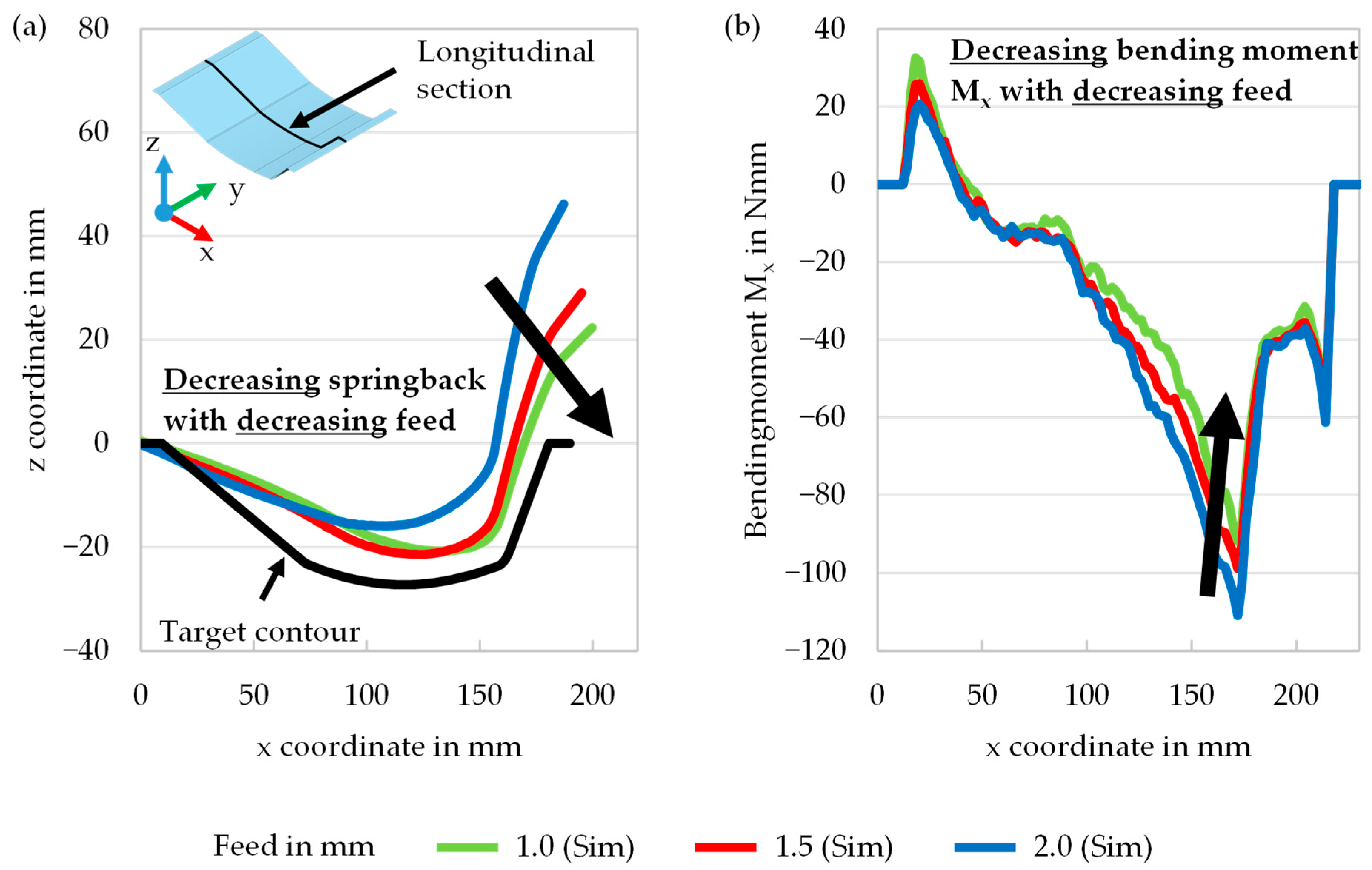
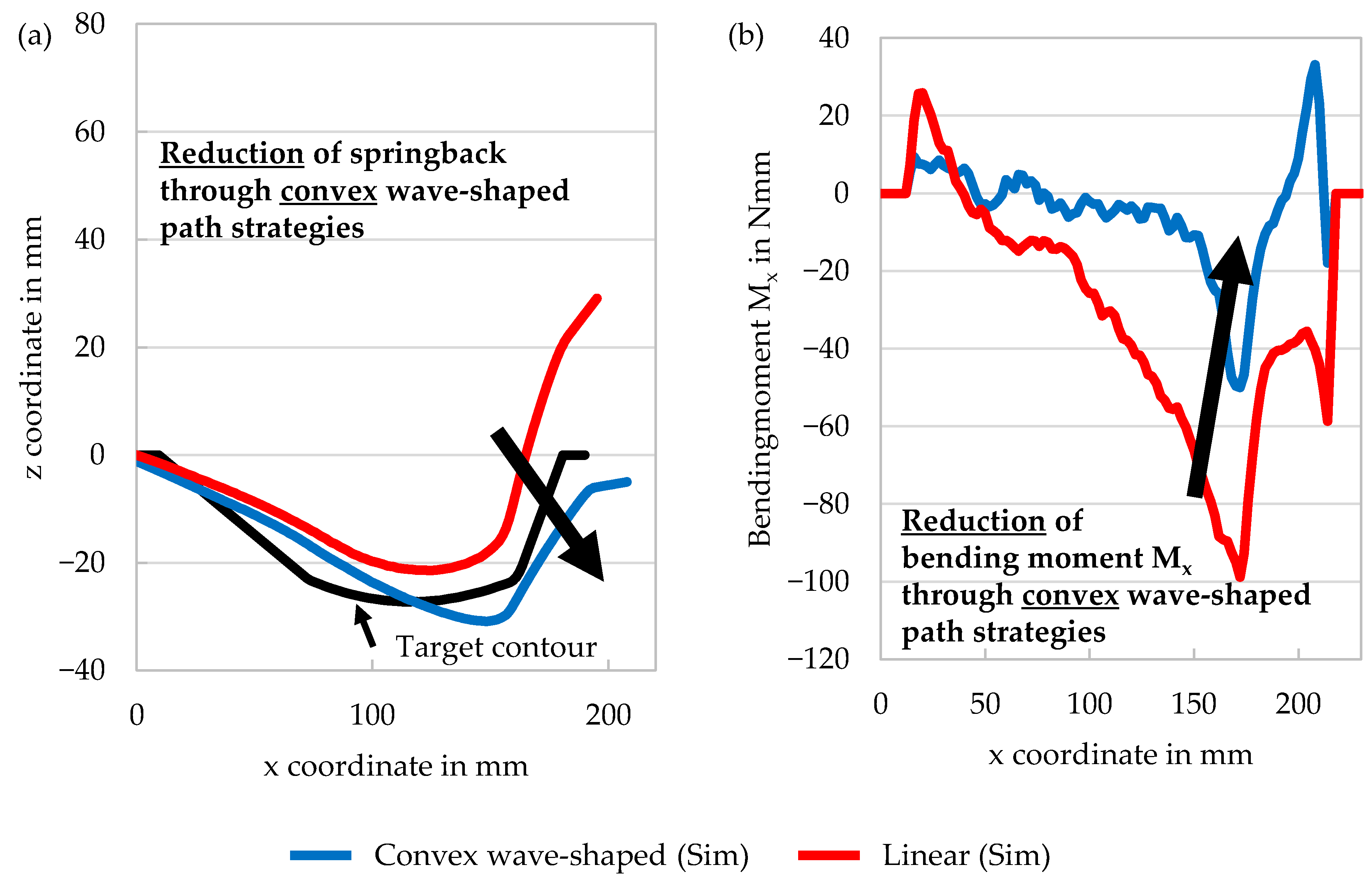
3. Results and Discussion

The evaluation of the springback geometry for linear path strategies as a function of feed in simulations and experiments is shown in Figure 8. Two-dimensional sections through the resulting geometries are compared.

The experimental results show very high springback for the linear tool path and confirm the effect of decreasing springback with decreasing feed, which is already known from the literature. The simulation results significantly underestimated the springback in comparison to the springback observed in the experiments. However, the dependence on the feed can be confirmed in both experiments and simulations. Thus, the springback also decreased in the simulation results with reduced feed.

To investigate the springback of non-linear path strategies, Figure 9 shows some selected results of wave-shaped paths compared to a conventional linear tool path. The amplitude of the underlying sine function for the convex/concave and convex/concave wave-shaped path strategies was 10 mm, and the amplitude of the second sine function 4 mm. The amplitude of the linear wave-shaped tool path was also 4 mm, whereby 15 waves per component width were applied for the wave-shaped path strategies. Many different wave-shaped tool paths regarding amplitude and wavelength have been tested. The selected results show the wave-shaped paths with the highest and lowest observed springback in the experiments and were compared to the reference of the linear tool path.

Figure 9 shows, in particular, that convex path strategies showed the most significant reduction in springback compared to the linear tool path, whereas concave strategies did not result in lower springback. Figure 10 shows the resulting surface after forming using a wave-shaped path strategy. The path lines are visible on the surface.

The results are a first indication for an advantage of wave-shaped tool paths in the investigated open geometries. This is not a clear prove of the hypotheses described in the introduction, but an indicator that at least the anticipated improvement of geometric accuracy could be achieved. In order to better understand the results, the residual bending moments were analysed in the FE simulation model described in Section 2.2.

Bending Moments

In order to investigate the formulated hypothesis that linear path strategies result in an accumulation of the local bending moments, so that a high resulting “total bending moment” occurs, which leads to the observed springback, bending moments have been analysed in FE simulations. Thus, the following consideration is focused on the bending moment M_x provided by LS-Dyna, since this is responsible for the springback around the path direction (here, the y-axis) of the geometry according to Figure 11. According to the convention shown, a negative bending moment led to a counterclockwise springback of the geometry around the y-axis. A higher negative bending moment therefore resulted in a larger springback.

Figure 12 shows the resulting bending moments M_x for an exemplary linear tool path. The high negative bending moments after forming and before springback can be recognized in (a) and (c). Due to the unclamping, the fixation forces were released, and the sheet was distorted until the bending moments were released and in equilibrium with the remaining sheet stiffness. Consequently, in the analysis result after springback (b) and (d), the remaining bending moments were very low.

Figure 13 gives an overview of the bending moment M_x for a convex, wave-shaped tool path strategy. According to Figure 9, this path strategy led to a reduction of the observed springback. In the simulation, a significant reduction in bending moments after forming was observed compared to linear strategies (a) and (c). This reduction resulted in a decreased springback, as shown in (b) and (d).

A 2D representation of the bending moments enables a systematic comparison of the bending moments depending on the selected path strategy. For this purpose, longitudinal sections throughout the geometry were generated (see Figure 14a top), which were used to evaluate the resulting bending moments that lead to a rotation around the y-axis (M_x). The bending moments were evaluated at each node along this longitudinal section. To avoid local outliers, the mean value of the moments of the nodes lying across the width (y-direction) was calculated at each node on this section. These averaged moments were then plotted over x-coordinate for better comparability between them. It was investigated to what extent a change in the observed springback could be correlated to a change in the calculated bending moments along the longitudinal section.

In order to validate these methods, the first results were obtained for a variation of feed, where it is known the from literature and from Figure 8 that there is a clear tendency of reduced springback for lower feed.

Figure 13 confirms the influence of the feed in linear tool paths on the bending moment and the springback. This observed dependency is consistent with the results observed by Maqbool et al. [4]

Figure 15 shows the dependence of the springback on the chosen path strategy. For a better overview, only a convex wave-shaped path strategy was compared with a linear path strategy, since according to experimental and numerical results, the convex wave-shaped paths led to the lowest springback. Although the numerical results underestimated the springback, they showed the same tendencies and therefore allow qualitative statements about the influence of the strategy on the bending moment.

In the next step in Figure 16, the simulated bending moments are plotted along the longitudinal section comparing the worst- and the best-case tool path strategy regarding springback. The effect of the path strategy on the resulting bending moment M_x can clearly be seen in this figure. In particular, for the convex wave-shaped path shown, there was a clear reduction in the calculated bending moment, which resulted in the reduced observed springback.

Based on the results, it can therefore be confirmed that wave-shaped tool paths have an influence on the resulting bending moment and reduce the springback in the examined geometries.

Further investigations are necessary to understand the influence of the path strategy on the bending moment in more detail. For example, it has still not been clearly analysed why concave tool paths do not lead to a reduction in springback or bending moments.

Although a significant reduction in springback could be achieved with the help of the examined tool path strategies, there are still geometric deviations to the target geometry. For example, it was not yet possible to achieve the shallow part of the geometry after springback with any of the investigated tool path strategies. At the same time, the influence of various path parameters on the surface quality and the extent to which post-processing steps are necessary have not yet been examined in detail.

Additional research is therefore planned to test and improve this new tool path approach for other types of part geometries, as well as to investigate limitations of this strategy and to further improve the geometric accuracy by combining this approach with other measures.

4. Conclusions

Based on the hypothesis that linear and parallel tool paths lead to an unfavourable accumulation of residual bending moments along a common axis, the influence of wave-shaped path strategies on the formation of bending moments and the resulting geometric deviations has been investigated.

The most important conclusions are the following:

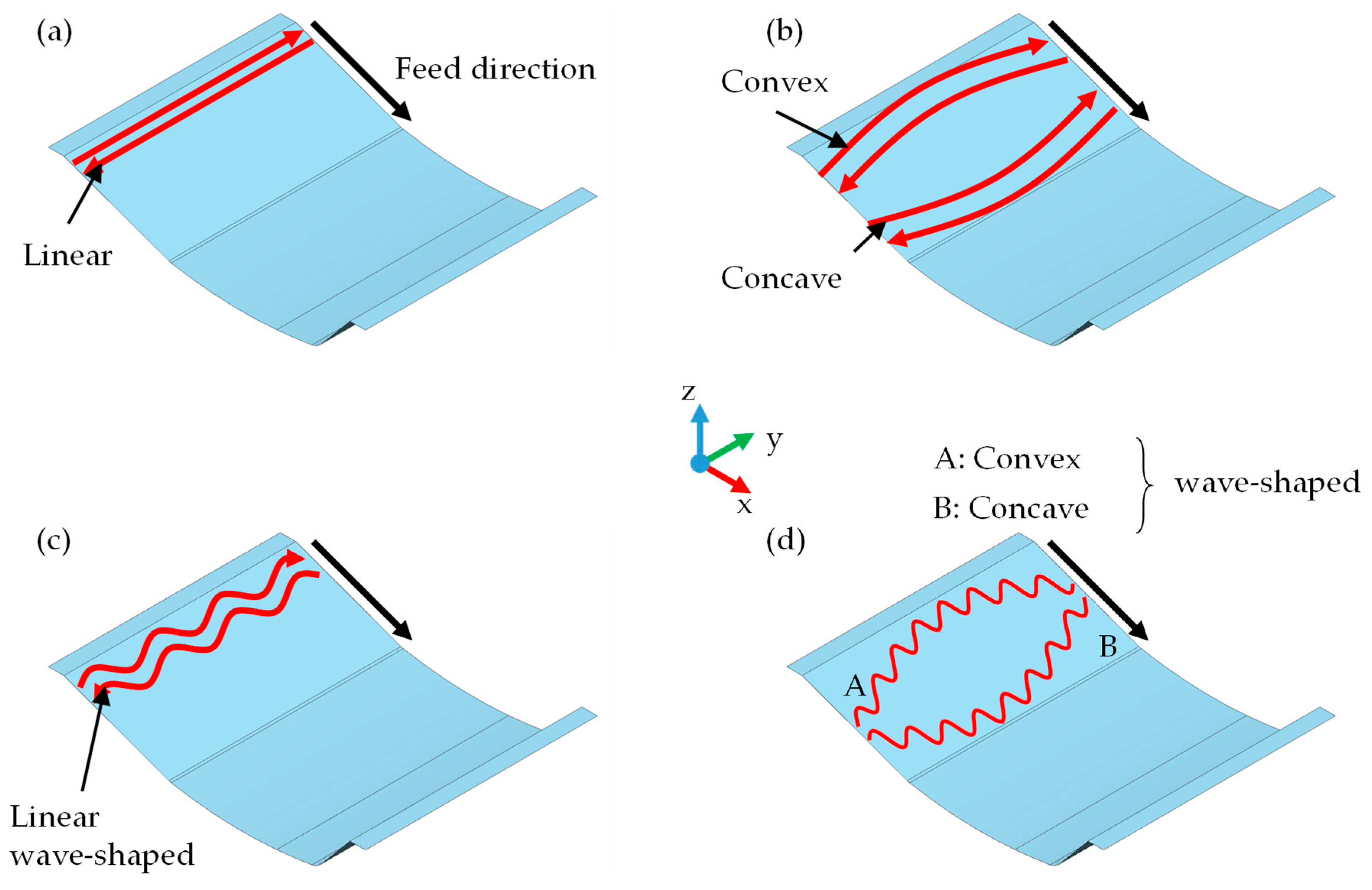
• A linear tool path strategy leads to very high geometric deviation for the selected unstiffened part geometry that is sensitive to geometric distortions.
• The geometric deviation has been significantly reduced by some versions of wave-shaped path strategies. In particular, the combination of a convex wave (wave peak points opposite to feed direction) with a superimposed sine function resulted in the lowest geometric deviation.
• In general, it has been shown that convex strategies lead to a stronger reduction of the springback than concave (wave peak points in feed direction) path strategies. It is not yet fully understood why concave path strategies do not have the same positive effect but lead to a similarly high geometric deviation as linear paths.
• With the help of an FE model, the effect of the chosen path strategy on the residual bending moment has been analysed. The best wave-shaped path strategies resulted in a significant reduction of the residual bending moment around the main axis.

Overall, it has been shown that the tool path curvature can have a positive influence on the geometric deviations. The novel type of tool path strategy has the additional advantage that it can be superimposed on conventional path strategies, such as “z-level” or “streamline”, by local adaptation. Thus, the established procedures for the generation of the general tool path based on the component geometry can still be used and enable an applicability to a wide range of application geometries.

The further research will focus on the deeper understanding of the interrelation of tool path and residual bending moments as well as the application of the novel tool path approach to other types of geometry. In particular, the numerical modelling using the finite element method must be investigated in more detail with regard to the selection of material parameters and boundary conditions for this new approach in order to be able to obtain quantitative statements regarding the springback depending on the selected path strategy in the future.