Influence of Sheet Covers on Filling Behavior in Electrochemical Joining of Additively Manufactured Components
Abstract
:1. Introduction
2. Materials and Methods
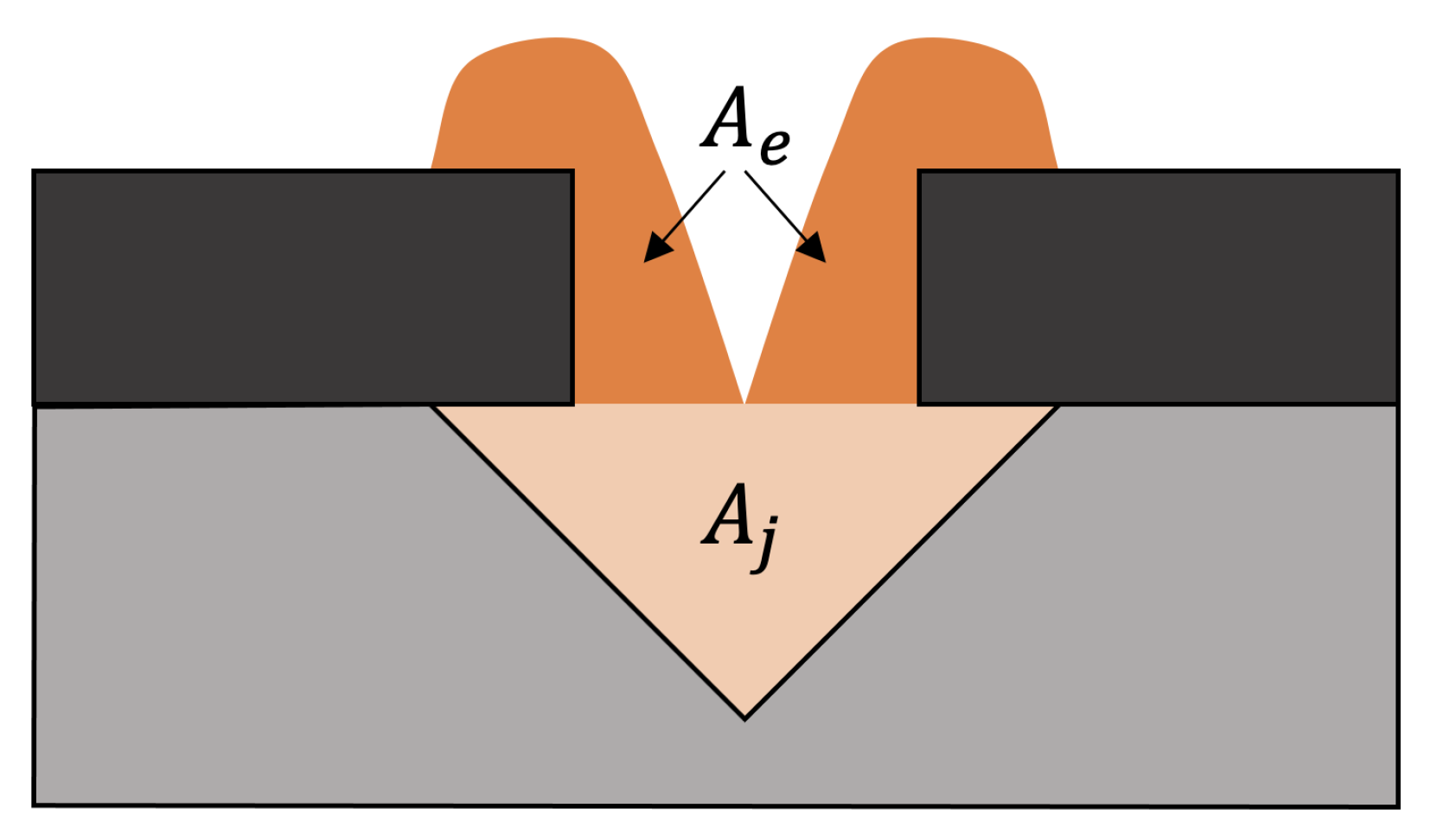
2.1. Governing Equations
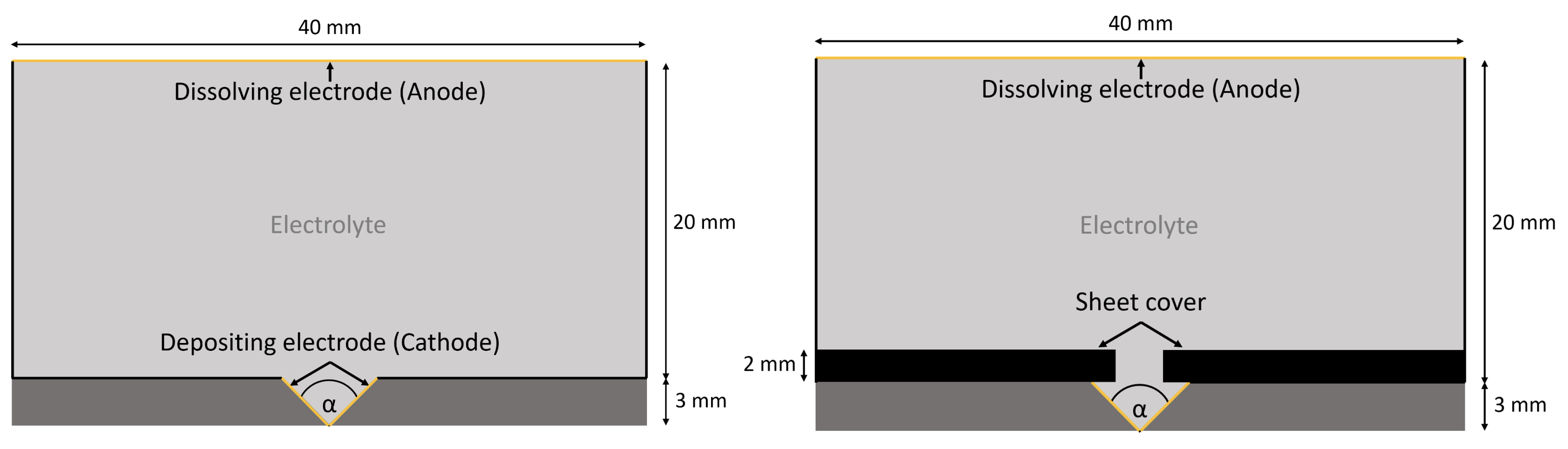
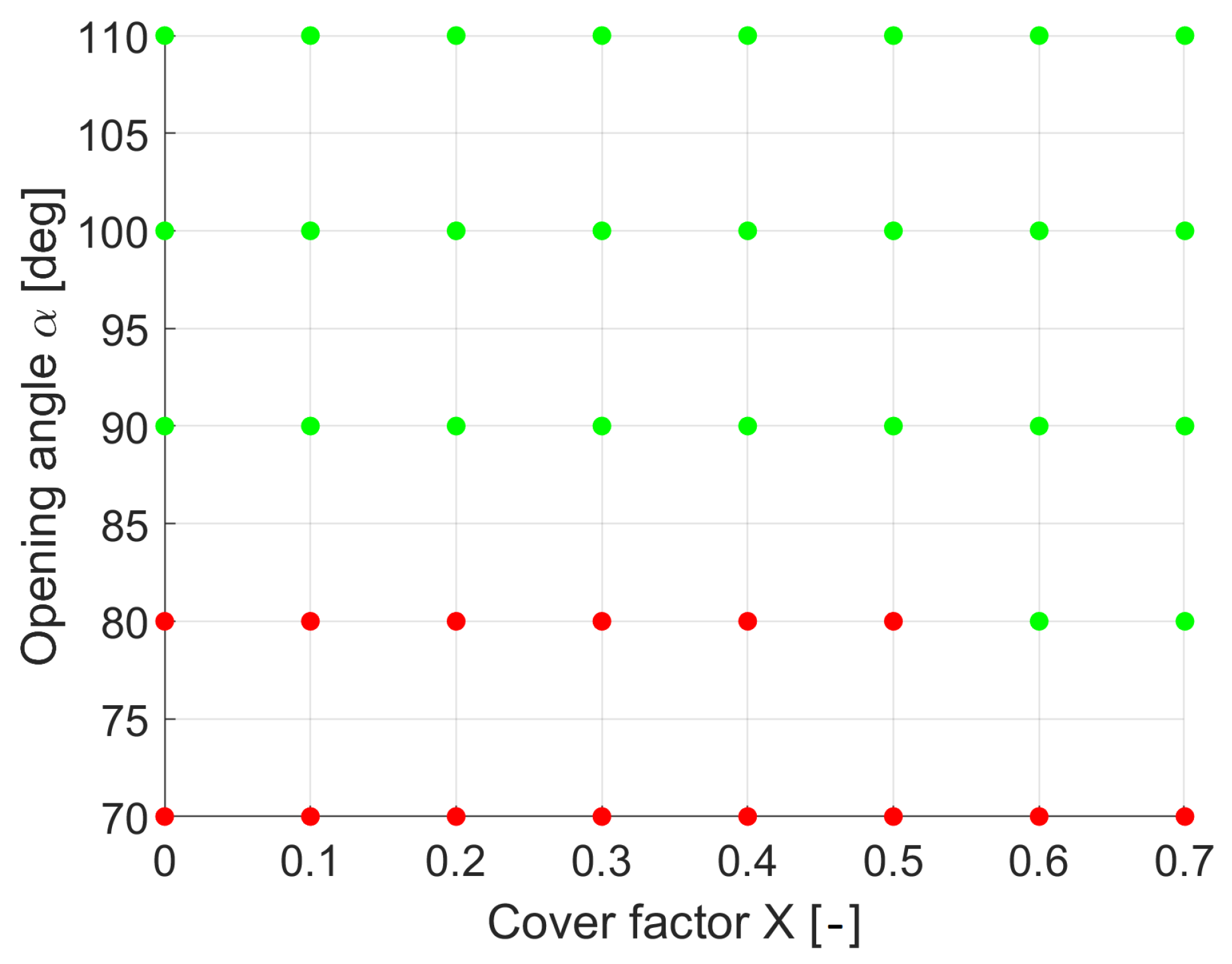
2.2. Simulation Model
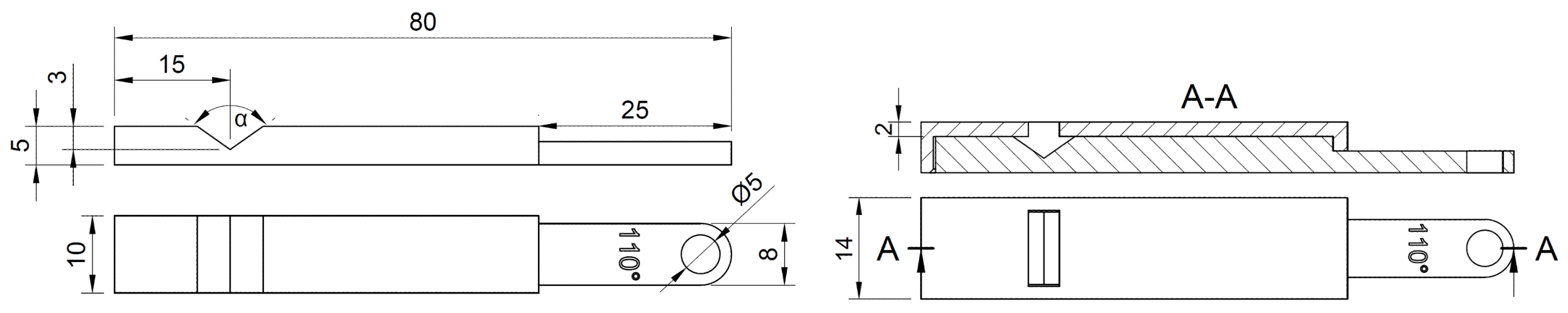
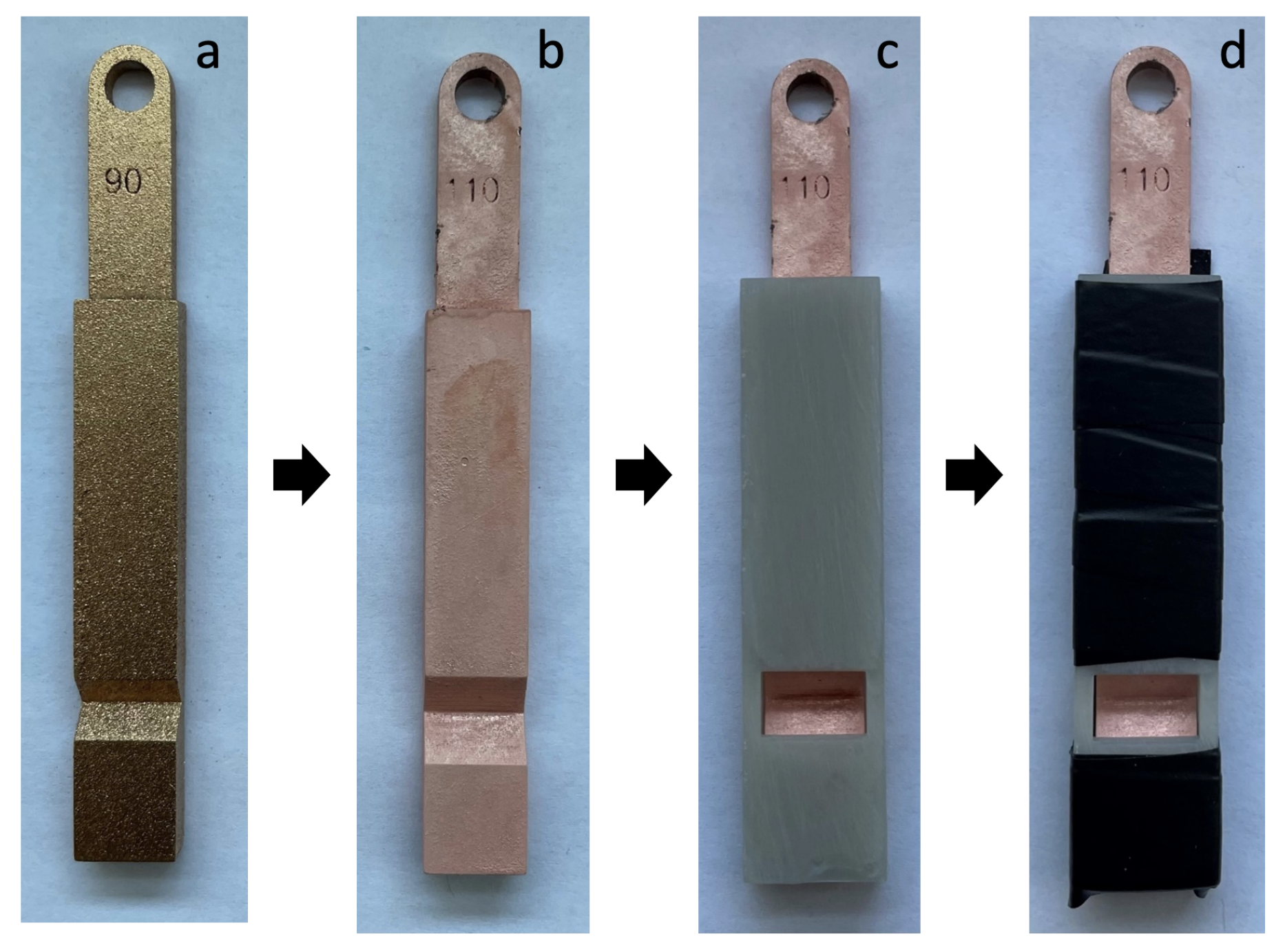
2.3. Experimental Methods
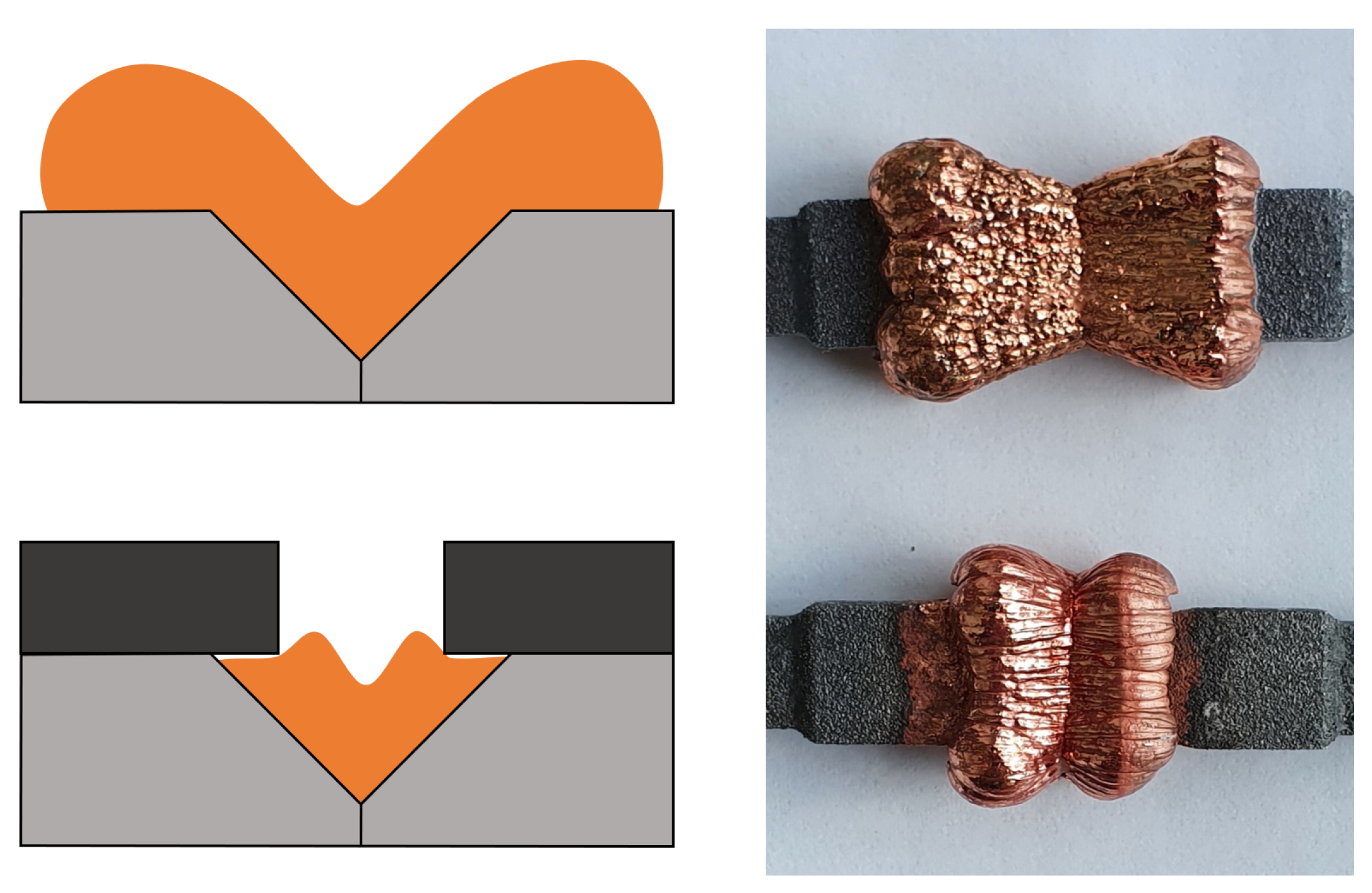
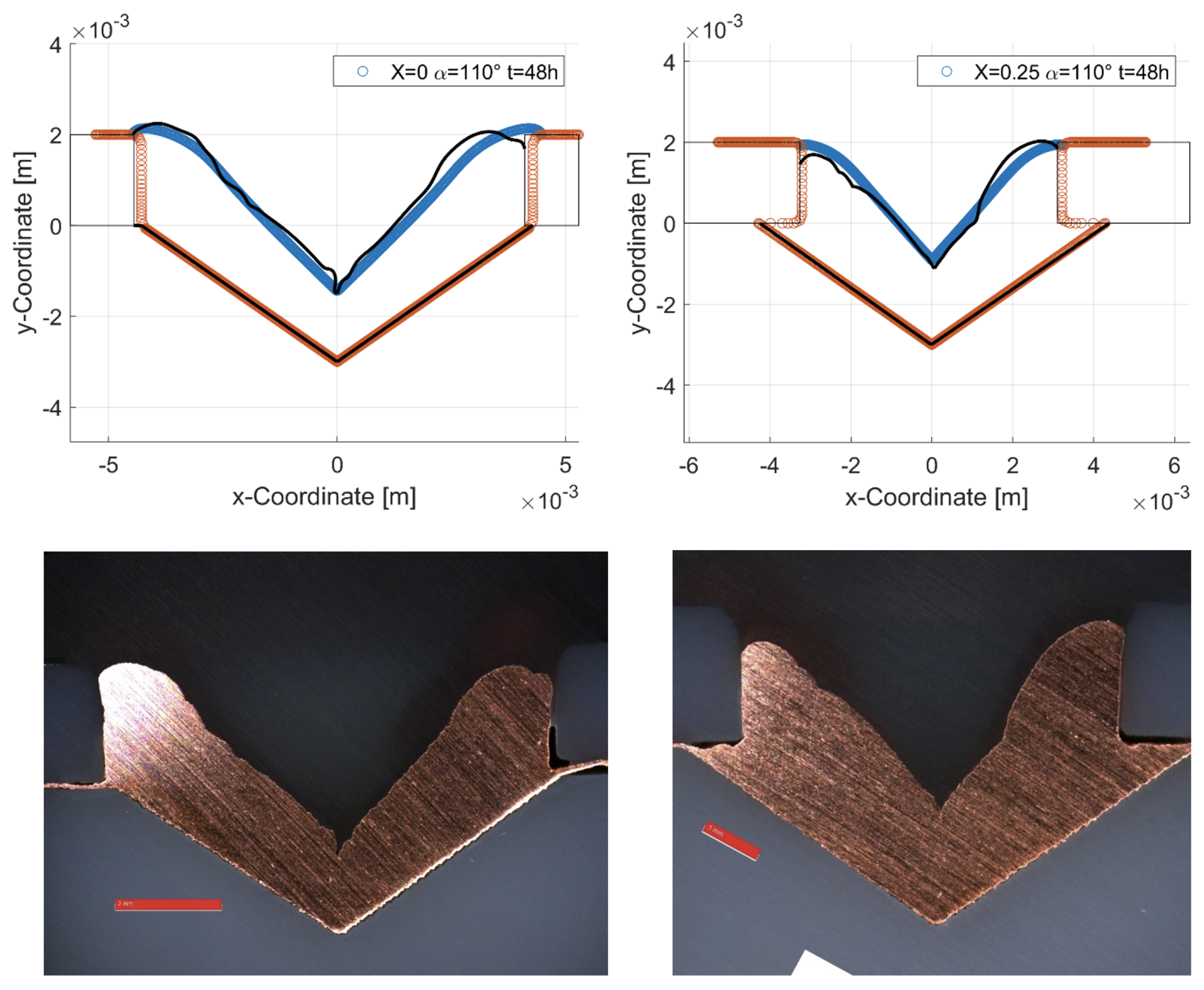
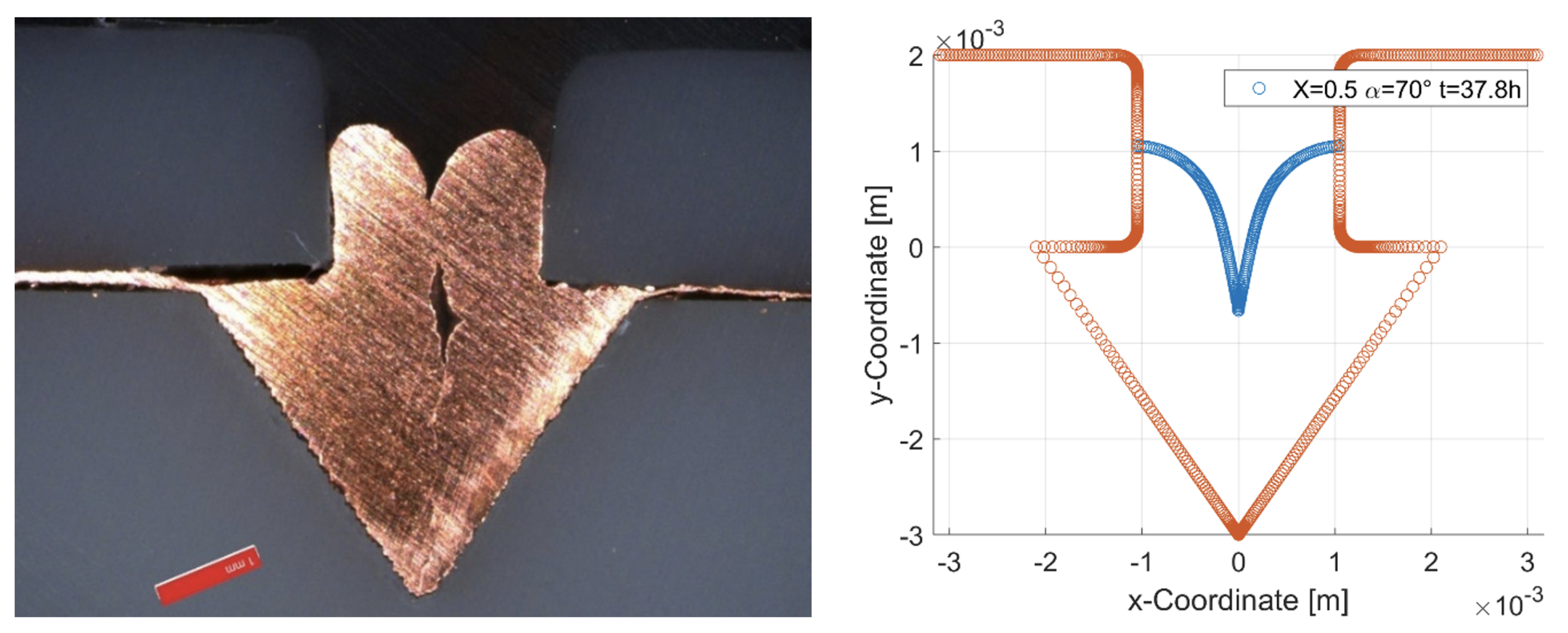
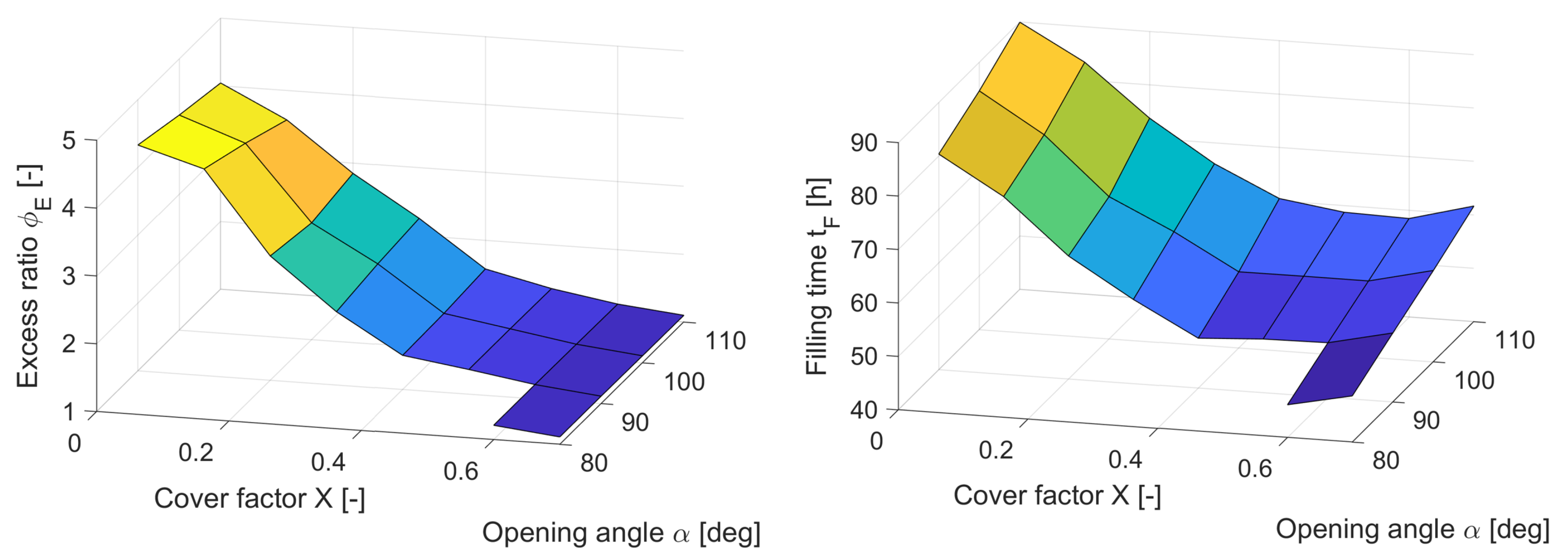
3. Results
4. Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Gibson, I.; Rosen, D.W.; Stucker, B. Additive Manufacturing Technologies: 3D Printing, Rapid Prototyping and Direct Digital Manufacturing, 2nd ed.; Springer: New York, NY, USA, 2015. [Google Scholar]
- Würtenberger, J.; Reichwein, J.; Kirchner, E. Using the potentials of additive manufacturing by a systematic linkage of the manufacturing process to product design. In DS 92: Proceedings of the DESIGN 2018 15th International Design Conference, Dubrovnik, Croatia, 21–24 May 2018; pp. 1465–1476. Available online: https://www.designsociety.org/publication/40551/USING+THE+POTENTIALS+OF+ADDITIVE+MANUFACTURING+BY+A+SYSTEMATIC+LINKAGE+OF+THE+MANUFACTURING+PROCESS+TO+PRODUCT+DESIGN (accessed on 30 July 2023).
- Krause, D.; Gebhardt, N. Methodische Entwicklung Modularer Produktfamilien; Springer: Berlin/Heidelberg, Germany, 2018. [Google Scholar] [CrossRef]
- Urbanic, R.J.; Hedrick, R. Fused Deposition Modeling Design Rules for Building Large, Complex Components. Comput.-Aided Des. Appl. 2016, 13, 348–368. [Google Scholar] [CrossRef]
- Jadoon, A.K.; Wu, C.; Liu, Y.J.; He, Y.; Wang, C.C.L. Interactive Partitioning of 3D Models into Printable Parts. IEEE Comput. Graph. Appl. 2018, 38, 38–53. [Google Scholar] [CrossRef] [PubMed]
- Reichwein, J.; Kirchner, E. Part orientation and separation to reduce process costs in additive manufacturing. Proc. Des. Soc. 2021, 1, 2399–2408. [Google Scholar] [CrossRef]
- Ilinkin, I.; Janardan, R.; Majhi, J.; Schwerdt, J.; Smid, M.; Sriram, R. A decomposition-based approach to layered manufacturing. Comput. Geom. 2002, 23, 117–151. [Google Scholar] [CrossRef]
- Reichwein, J.; Rudolph, K.; Geis, J.; Kirchner, E. Adapting product architecture to additive manufacturing through consolidation and separation. Procedia CIRP 2021, 100, 79–84. [Google Scholar] [CrossRef]
- Deka, A.; Behdad, S. Part Separation Technique for Assembly-Based Design in Additive Manufacturing using Genetic Algorithm. Procedia Manuf. 2019, 34, 764–771. [Google Scholar] [CrossRef]
- Gill, M.; Terry, E.; Abdi, Y.; Hawkes, S.; Rindler, J.; Schick, D.; Ramirez, A.; Herderick, E.D. Joining Technologies for Metal Additive Manufacturing in the Energy Industry. JOM 2020, 72, 4214–4220. [Google Scholar] [CrossRef]
- Hassanin, A.E.; Velotti, C.; Scherillo, F.; Astarita, A.; Squillace, A.; Carrino, L. Study of the solid state joining of additive manufactured components. In Proceedings of the 2017 IEEE 3rd International Forum on Research and Technologies for Society and Industry (RTSI), Modena, Italy, 11–13 September 2017; pp. 1–4. [Google Scholar] [CrossRef]
- Yuce, C.; Karpat, F.; Yavuz, N.; Doğan, O. A review on advanced joining techniques of multi material part manufacturing for automotive industry. Int. J. Mech. Prod. Eng. 2015, 3, 1–5. [Google Scholar]
- Hinojos, A.; Mireles, J.; Reichardt, A.; Frigola, P.; Hosemann, P.; Murr, L.E.; Wicker, R.B. Joining of Inconel 718 and 316 Stainless Steel using electron beam melting additive manufacturing technology. Mater. Des. 2016, 94, 17–27. [Google Scholar] [CrossRef]
- Rudolph, K.; Noack, M.; Hausmann, M.; Kirchner, E.; Babaei, P. Electroplating as an Innovative Joining Method for Laser Additive Manufactured Components Made of AlSi10Mg. In Innovative Product Development by Additive Manufacturing 2021; Lachmayer, R., Bode, B., Kaierle, S., Eds.; Springer International Publishing: Cham, Switzerland, 2023; pp. 167–180. [Google Scholar] [CrossRef]
- Dini, J.W.; Johnson, H.R. Joining by electroplating. Met. Eng. Quart. 1974, 14, 6–12. [Google Scholar]
- Yang, Y.; Chen, H.; Li, M. Dissimilar copper-aluminum joint processed by low-temperature nickel electroplating. J. Mater. Process. Technol. 2017, 242, 68–76. [Google Scholar] [CrossRef]
- Rudolph, K.; Kübler, M.; Noack, M.; Kirchner, E. Influence of joining zone geometry on material distribution in electrochemically produced component joints in additive manufacturing. In Innovative Product Development by Additive Manufacturing; Lachmayer, R., Bode, B., Kaierle, S., Eds.; Springer International Publishing: Berlin/Heidelberg, Germany, 2022; pp. 31–46. [Google Scholar] [CrossRef]
- Baiges, J.; Chiumenti, M.; Moreira, C.A.; Cervera, M.; Codina, R. An adaptive Finite Element strategy for the numerical simulation of additive manufacturing processes. Addit. Manuf. 2021, 37, 101650. [Google Scholar] [CrossRef]
- Braun, T.M.; Josell, D.; John, J.; Moffat, T.P. Simulation of Copper Electrodeposition in Through-Hole Vias. J. Electrochem. Soc. 2020, 167, 13510. [Google Scholar] [CrossRef]
- Shourije, S.M.J.S.; Bahrololoom, M.E. Comparison of effects of simulated electric field interference and presence of a barrier in the nickel electroplating process to experimental data. Trans. IMF 2020, 98, 303–313. [Google Scholar] [CrossRef]
- Mahapatro, A.; Kumar Suggu, S. Modeling and simulation of electrodeposition: Effect of electrolyte current density and conductivity on electroplating thickness. Adv. Mater. Sci. 2018, 3, 1000143. [Google Scholar] [CrossRef]
- Wittstock, G. Lehrbuch der Elektrochemie: Grundlagen, Methoden, Materialien, Anwendungen; Wiley-VCH GmbH: Weinheim, Germany, 2023. [Google Scholar]
- Deconinck, J. Mathematical modelling of electrode growth. J. Appl. Electrochem. 1994, 24, 212–218. [Google Scholar] [CrossRef]
- Schork, S.; Kirchner, E. Defining requirements in prototyping: The holistic prototype and process development. In DS 91: Proceedings of NordDesign 2018, Linköping, Sweden, 14–17 August 2018; Available online: https://www.designsociety.org/publication/40953/Defining+Requirements+in+Prototyping (accessed on 30 July 2023).


| Description | Symbol | Value | Unit of Measurement |
|---|---|---|---|
| Temperature | T | 293.15 | K |
| Electrolyte conductivity | 0.115 | S/cm | |
| Density copper | 8960 | kg/m | |
| Molar mass copper | 0.06355 | kg/mol | |
| External electric anode potential | 0.5 | V | |
| Exchange current density | 25 | A/m | |
| Anodic transfer coefficient | 1 | - | |
| Cathodic transfer coefficient | 1 | - | |
| Number of participating electrons | n | 2 | - |
| Opening Angle | 70° | 80° | 90° | 100° | 110° |
|---|---|---|---|---|---|
| min | NaN | 1.11 [0.7] | 1.11 [0.7] | 1.10 [0.7] | 1.10 [0.7] |
| min | NaN | 46.12 h [0.6] | 49.35 h [0.4] | 54.28 h [0.6] | 58.45 h [0.6] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Noack, M.; Rudolph, K.; Breimann, R.; Kirchner, E. Influence of Sheet Covers on Filling Behavior in Electrochemical Joining of Additively Manufactured Components. J. Manuf. Mater. Process. 2023, 7, 157. https://doi.org/10.3390/jmmp7050157
Noack M, Rudolph K, Breimann R, Kirchner E. Influence of Sheet Covers on Filling Behavior in Electrochemical Joining of Additively Manufactured Components. Journal of Manufacturing and Materials Processing. 2023; 7(5):157. https://doi.org/10.3390/jmmp7050157
Chicago/Turabian StyleNoack, Marco, Kris Rudolph, Richard Breimann, and Eckhard Kirchner. 2023. "Influence of Sheet Covers on Filling Behavior in Electrochemical Joining of Additively Manufactured Components" Journal of Manufacturing and Materials Processing 7, no. 5: 157. https://doi.org/10.3390/jmmp7050157
APA StyleNoack, M., Rudolph, K., Breimann, R., & Kirchner, E. (2023). Influence of Sheet Covers on Filling Behavior in Electrochemical Joining of Additively Manufactured Components. Journal of Manufacturing and Materials Processing, 7(5), 157. https://doi.org/10.3390/jmmp7050157






