Understanding Inhomogeneous Mechanical Properties in PBF-LB/M Manufactured Parts Due to Inhomogeneous Macro Temperature Profiles Based on Process-Inherent Preheating
Abstract
1. Introduction
2. Methods and Approach
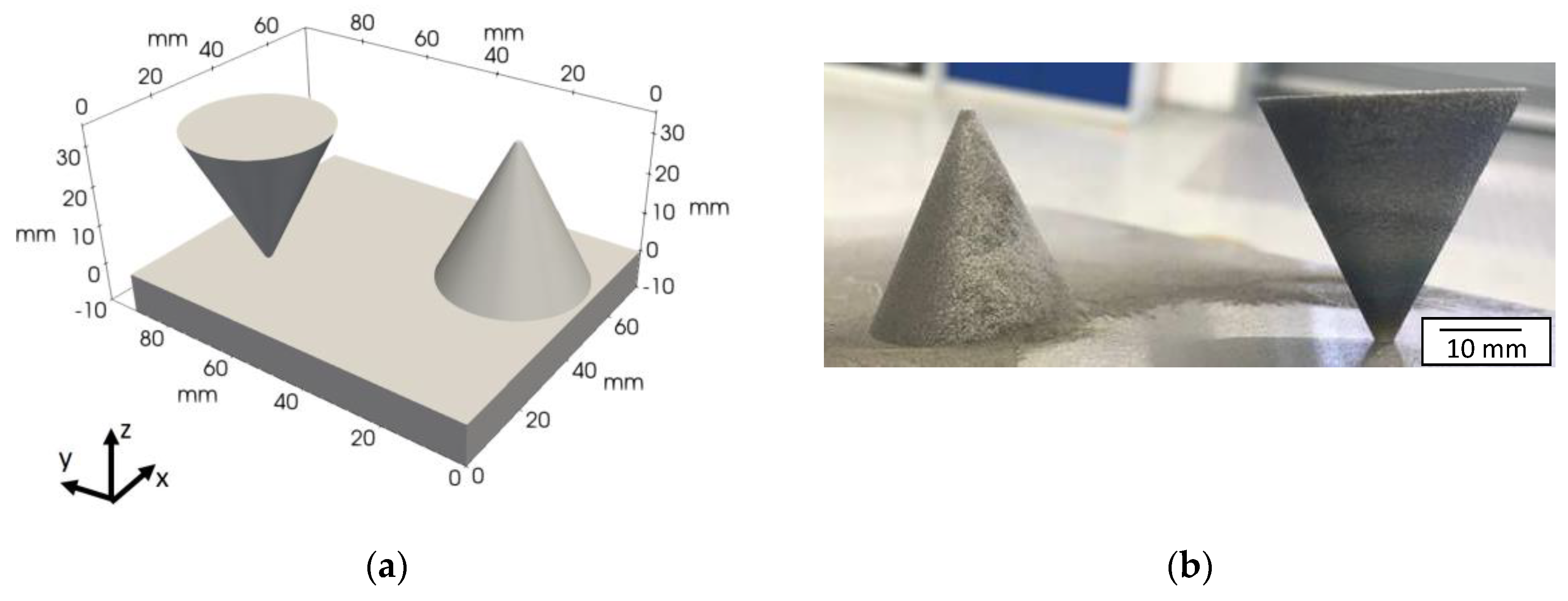
2.1. Processing and Characterization Techniques
2.2. Model Description of PBF-LB/M Macro Temperature Model
2.2.1. Governing Equations and Boundary Conditions
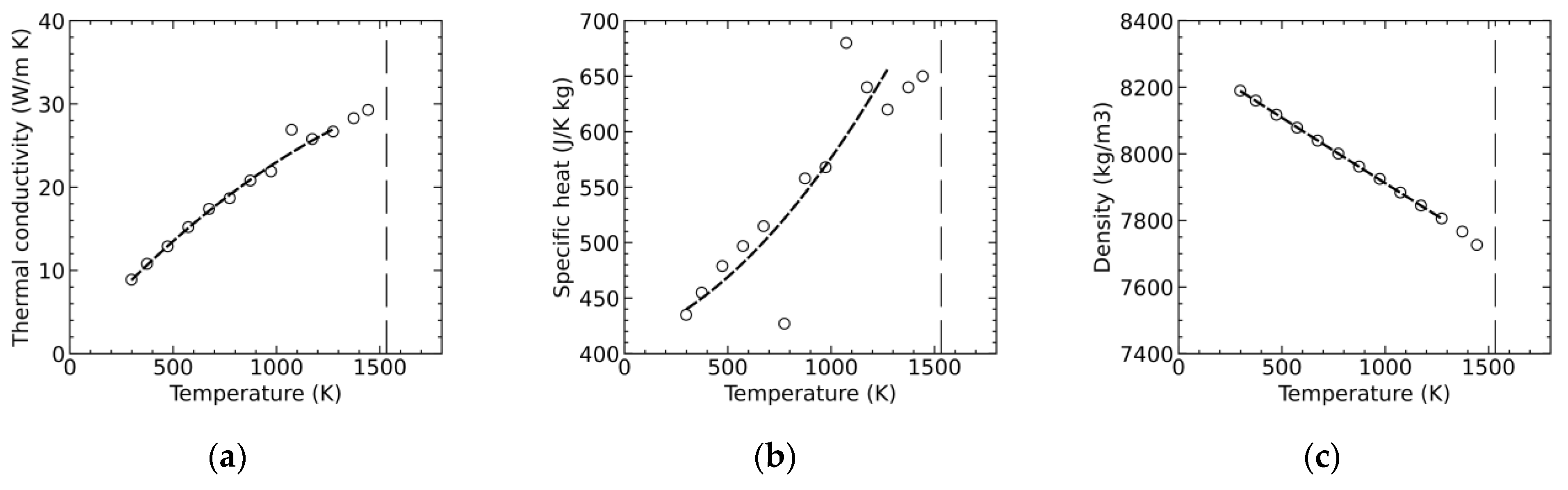
2.2.2. Material Properties
2.2.3. Numerical Setup
2.2.4. Reference Thermal Model
2.3. Melt Pool Simulation Model
3. Results and Discussion
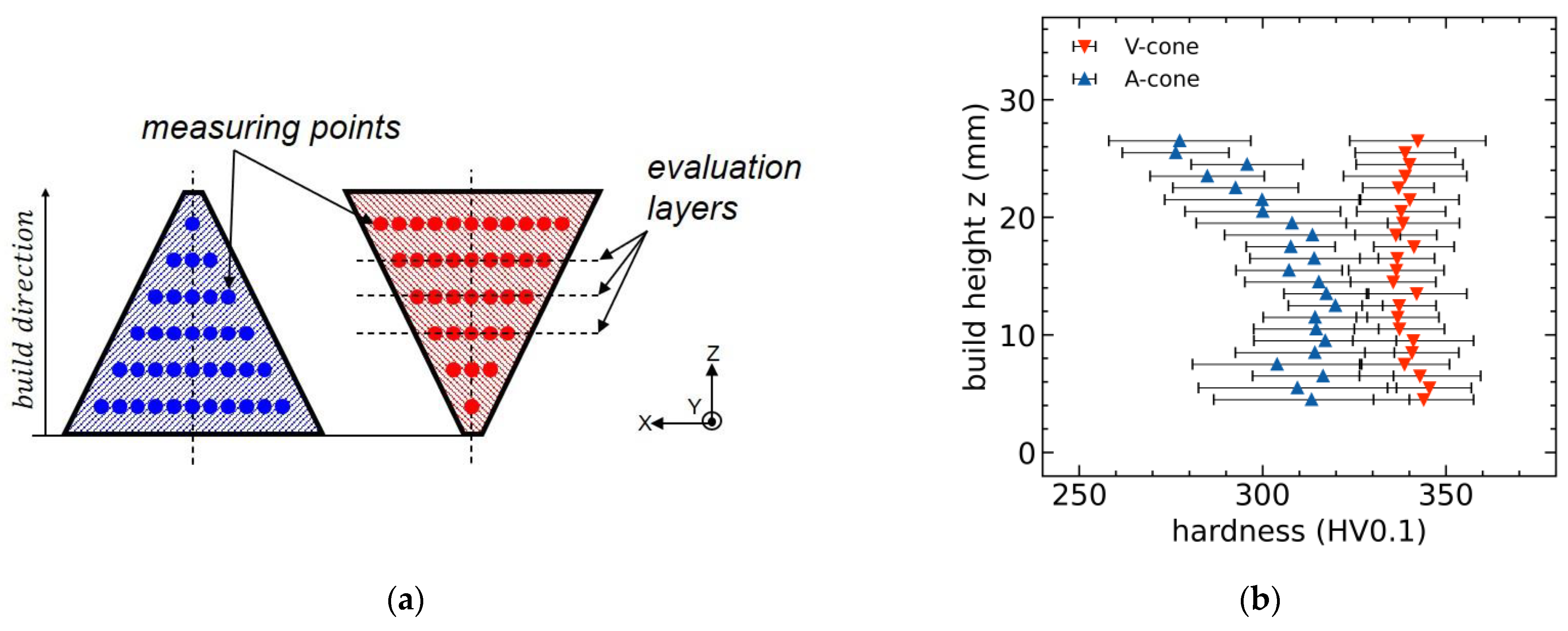
3.1. Analysis of Microhardness
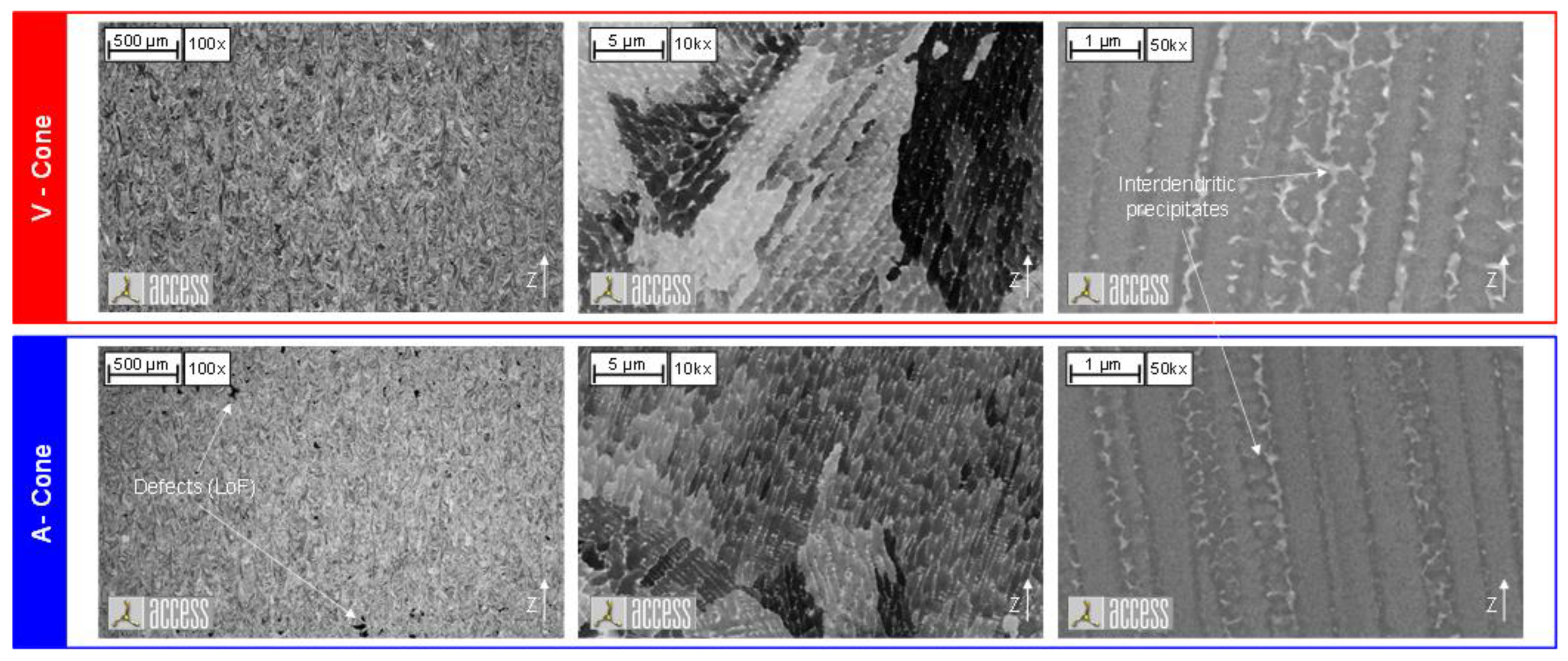
3.2. Analysis of Microstructure
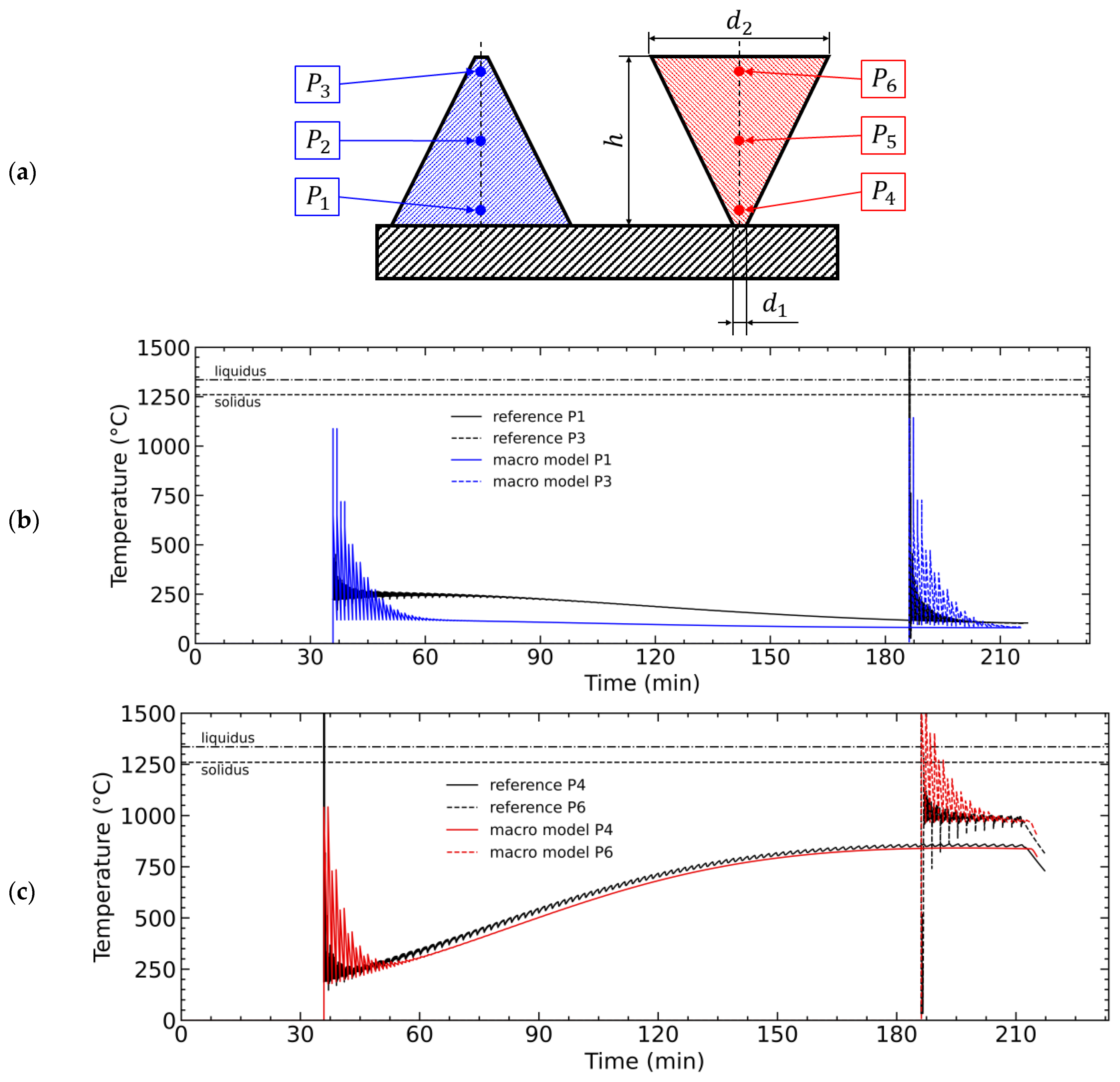
3.3. Validation of the Macro Temperature Model
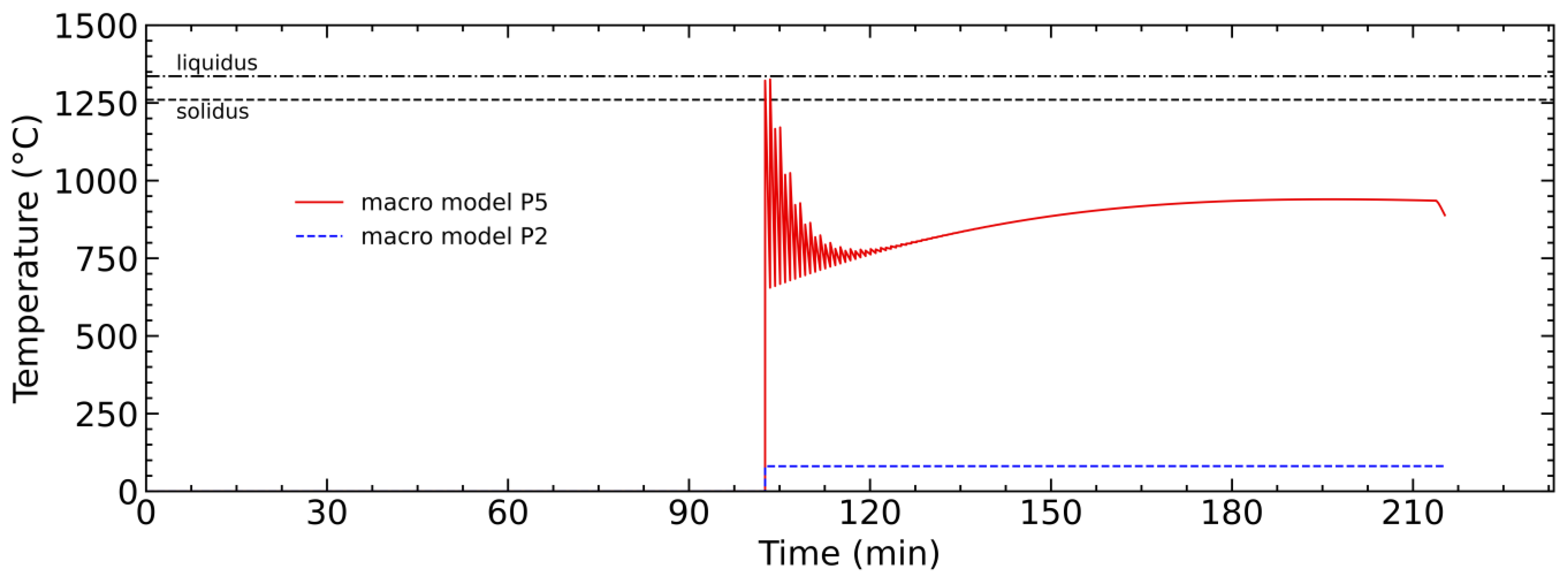
3.4. Prediction of the Process-Inherent Preheating Temperature Profiles
3.5. Evaluation of Solidification Conditions
4. Conclusions
- A macro temperature model is developed and validated. Thereby, the presented reduced order model is significantly faster than the scan-vector resolved reference model: 75 min for the presented coarse-scale model on a single CPU core in comparison to 16 h for the high-fidelity model, which runs also on multiple cores.
- The macro temperature model allows a qualitative prediction of the process-inherent preheating during PBF-LB/M and is used to determined preheating temperatures and local heating cycles for two geometries.
- Geometric susceptibility to heat accumulation during PBF-LB/M is demonstrated experimentally, and an increase in Vickers hardness with build height for a V-shaped cone is found.
- From analysis of microstructure, minor differences in the phase fraction of Laves phase can be observed and correlated to the increase in Vickers hardness. The proportion of strength-increasing precipitates is greater in the cross sections under process-inherent preheating. It can be assumed that nano-sized γ′/γ″ precipitates contribute to an increase in hardness. A precise assignment of whether the precipitates are Laves phase, δ or other precipitates, such as nitrides or carbides, cannot be definitively determined without an analysis of the phase composition.
- The transient three-dimensional temperature distribution of the PBF-LB/M melt pool and solidification conditions are determined in a single-track simulation, based on the determined preheating temperatures, but the difference is not significant enough to conclude the different microstructure on its own.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kurz, W.; Fisher, D.J. Fundamentals of Solidification, 4th ed.; Trans Tech Publ: Uetikon-Zuerich, Switzerland, 2005; ISBN 0878498044. [Google Scholar]
- Greven, K.; Ludwig, A. Schnelle Erstarrung. In Schmelze, Erstarrung, Grenzflächen; Sahm, P.R., Egry, I., Volkmann, T., Eds.; Springer: Berlin/Heidelberg, Germany, 1999; pp. 208–223. ISBN 978-3-540-41566-4. [Google Scholar]
- Boussinot, G.; Apel, M.; Zielinski, J.; Hecht, U.; Schleifenbaum, J.H. Strongly out-of-equilibrium columnar solidification during the Laser Powder-Bed Fusion additive manufacturing process. Phys. Rev. Appl. 2019, 11, 567. [Google Scholar] [CrossRef]
- Verhülsdonk, M.; Vervoort, S.; Dionne, P.; Megahed, M. LPBF thermal history of IN718 part and metallurgical considerations. Addit. Manuf. 2023. under review. [Google Scholar]
- Duong, E.; Masseling, L.; Knaak, C.; Dionne, P.; Megahed, M. Scan path resolved thermal modelling of LPBF. Addit. Manuf. Lett. 2022, 3, 100047. [Google Scholar] [CrossRef]
- Zielinski, J. A Holistic Approach to Understand Laser Additive Manufacturing from Melt Pool to Microstructure. Ph.D. Thesis, RWTH Aachen University, Aachen, Germany, 2022. [Google Scholar]
- Romano, J.; Ladani, L.; Sadowski, M. Laser Additive Melting and Solidification of Inconel 718: Finite Element Simulation and Experiment. Jom 2016, 68, 967–977. [Google Scholar] [CrossRef]
- Schrage, J.; Schleifenbaum, J.; Manfi, M. Influence of Powder Application Parameters on Powder Bed Properties and on Productivity of Laser Powder Bed Fusion (L-PBF). In Proceedings of the 4th Metal Additive Manufacturing Conference, Orebro, Sweden, 25–27 November 2019. [Google Scholar]
- Geuzaine, C.; Remacle, J.-F. Gmsh: A 3-D finite element mesh generator with built-in pre- and post-processing facilities. Int. J. Numer. Meth. Engng. 2009, 79, 1309–1331. [Google Scholar] [CrossRef]
- Hecht, F. New development in FreeFem++. J. Numer. Math. 2012, 20, 251–266. [Google Scholar] [CrossRef]
- Raghavan, N.; Dehoff, R.; Pannala, S.; Simunovic, S.; Kirka, M.; Turner, J.; Carlson, N.; Babu, S.S. Numerical modeling of heat-transfer and the influence of process parameters on tailoring the grain morphology of IN718 in electron beam additive manufacturing. Acta Mater. 2016, 112, 303–314. [Google Scholar] [CrossRef]
- Jia, Q.; Gu, D. Selective laser melting additive manufacturing of Inconel 718 superalloy parts: Densification, microstructure and properties. J. Alloys Compd. 2014, 585, 713–721. [Google Scholar] [CrossRef]
- Burbaum, B. Verfahrenstechnische Grundlagen für das Laserstrahl-Umschmelzen einkristalliner Nickelbasis-Superlegierungen. Ph.D. Thesis, RWTH Aachen, Aachen, Germany, 2010. [Google Scholar]
- Amato, K.N.; Gaytan, S.M.; Murr, L.E.; Martinez, E.; Shindo, P.W.; Hernandez, J.; Collins, S.; Medina, F. Microstructures and mechanical behavior of Inconel 718 fabricated by selective laser melting. Acta Mater. 2012, 60, 2229–2239. [Google Scholar] [CrossRef]
- Renhof, L. Mikrostruktur und Mechanische Eigenschaften der Nickellegierung IN 718. Ph.D. Thesis, Technical University of Munich, Munich, Germany, 2007. [Google Scholar]
- Wang, Z.; Guan, K.; Gao, M.; Li, X.; Chen, X.; Zeng, X. The microstructure and mechanical properties of deposited-IN718 by selective laser melting. J. Alloys Compd. 2012, 513, 518–523. [Google Scholar] [CrossRef]
- Deng, D.; Peng, R.L.; Brodin, H.; Moverare, J. Microstructure and mechanical properties of Inconel 718 produced by selective laser melting: Sample orientation dependence and effects of post heat treatments. Mater. Sci. Eng. A 2018, 713, 294–306. [Google Scholar] [CrossRef]
- Kumara, C.; Balachandramurthi, A.R.; Goel, S.; Hanning, F.; Moverare, J. Toward a better understanding of phase transformations in additive manufacturing of Alloy 718. Materialia 2020, 13, 100862. [Google Scholar] [CrossRef]
- Mohr, G.; Altenburg, S.J.; Hilgenberg, K. Effects of inter layer time and build height on resulting properties of 316L stainless steel processed by laser powder bed fusion. Addit. Manuf. 2020, 32, 101080. [Google Scholar] [CrossRef]
- Sprengel, M.; Mohr, G.; Altenburg, S.J.; Evans, A.; Serrano-Munoz, I.; Kromm, A.; Pirling, T.; Bruno, G.; Kannengiesser, T. Triaxial Residual Stress in Laser Powder Bed Fused 316L: Effects of Interlayer Time and Scanning Velocity. Adv. Eng. Mater. 2022, 24, 2101330. [Google Scholar] [CrossRef]
- Rielli, V.V.; Theska, F.; Godor, F.; Stanojevic, A.; Oberwinkler, B.; Primig, S. Evolution of nanoscale precipitates during common Alloy 718 ageing treatments. Mater. Des. 2021, 205, 109762. [Google Scholar] [CrossRef]

| Parameter | Value |
|---|---|
| 160 W | |
| 960 mm/s | |
| 0.1 mm | |
| Layer thickness | 30 µm |
| Recoating time | 4 s |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zielinski, J.; Theunissen, J.; Kruse, H.; Rittinghaus, S.-K.; Schleifenbaum, J.H.; Zhu, D.; Megahed, M. Understanding Inhomogeneous Mechanical Properties in PBF-LB/M Manufactured Parts Due to Inhomogeneous Macro Temperature Profiles Based on Process-Inherent Preheating. J. Manuf. Mater. Process. 2023, 7, 88. https://doi.org/10.3390/jmmp7030088
Zielinski J, Theunissen J, Kruse H, Rittinghaus S-K, Schleifenbaum JH, Zhu D, Megahed M. Understanding Inhomogeneous Mechanical Properties in PBF-LB/M Manufactured Parts Due to Inhomogeneous Macro Temperature Profiles Based on Process-Inherent Preheating. Journal of Manufacturing and Materials Processing. 2023; 7(3):88. https://doi.org/10.3390/jmmp7030088
Chicago/Turabian StyleZielinski, Jonas, Jan Theunissen, Henrik Kruse, Silja-Katharina Rittinghaus, Johannes Henrich Schleifenbaum, Dongjian Zhu, and Mustafa Megahed. 2023. "Understanding Inhomogeneous Mechanical Properties in PBF-LB/M Manufactured Parts Due to Inhomogeneous Macro Temperature Profiles Based on Process-Inherent Preheating" Journal of Manufacturing and Materials Processing 7, no. 3: 88. https://doi.org/10.3390/jmmp7030088
APA StyleZielinski, J., Theunissen, J., Kruse, H., Rittinghaus, S.-K., Schleifenbaum, J. H., Zhu, D., & Megahed, M. (2023). Understanding Inhomogeneous Mechanical Properties in PBF-LB/M Manufactured Parts Due to Inhomogeneous Macro Temperature Profiles Based on Process-Inherent Preheating. Journal of Manufacturing and Materials Processing, 7(3), 88. https://doi.org/10.3390/jmmp7030088







