Strain Induced Surface Change in Sheet Metal Forming: Numerical Prediction, Influence on Friction and Tool Wear
Abstract
1. Introduction
2. Numerical Prediction of Strain-Induced Roughening
2.1. The Approach of Numerical Prediction of Strain-Induced Roughening for Uncoated Polished Sheet Metal
2.2. Verification of Galvanized Coating on the Surface Roughening
3. Influence of the Pre-Staining of Galvanized Sheet Metal on Friction
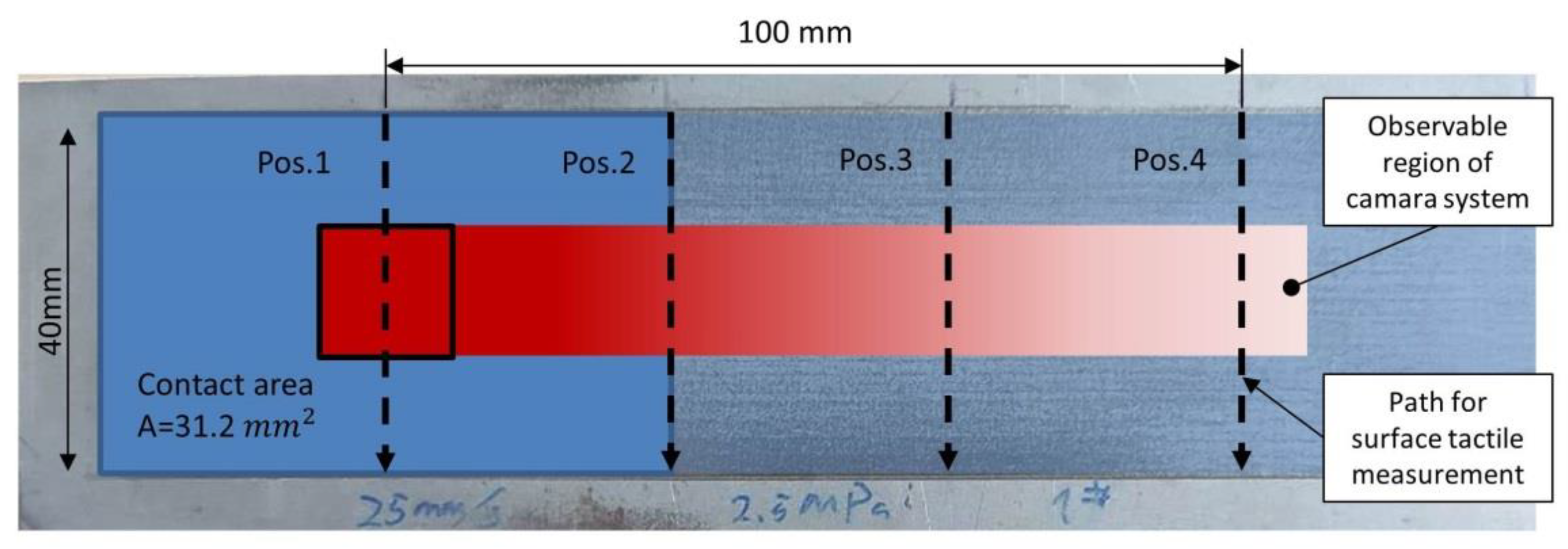
3.1. Introduction of The Experimental Setup
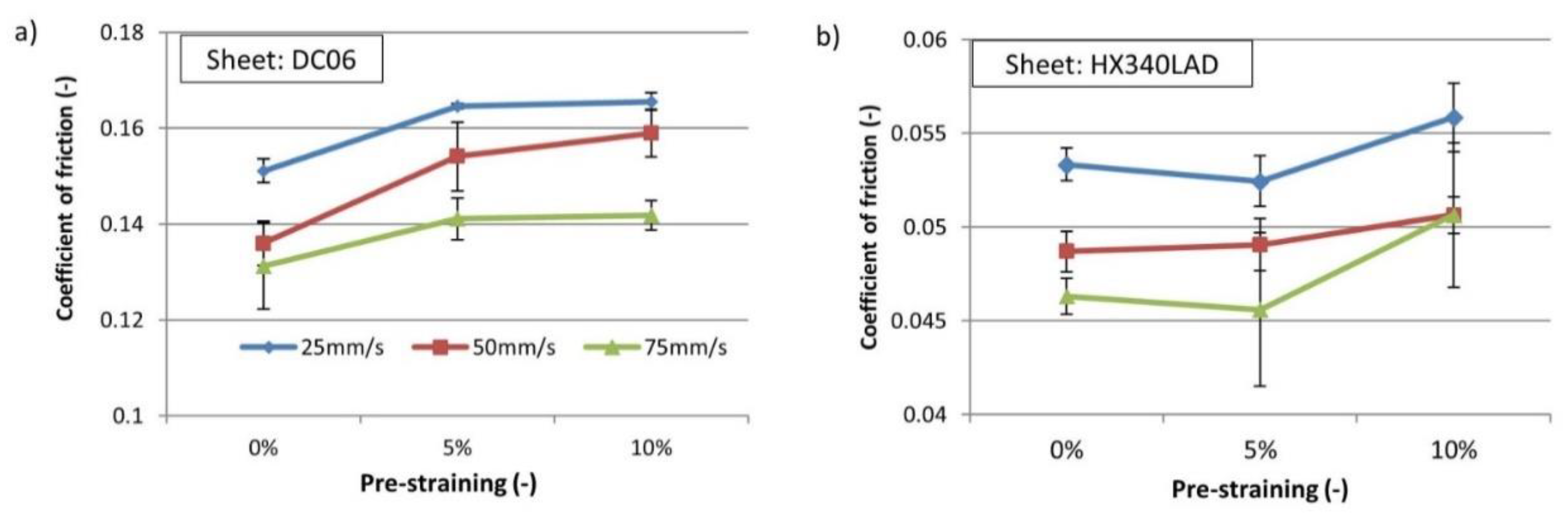
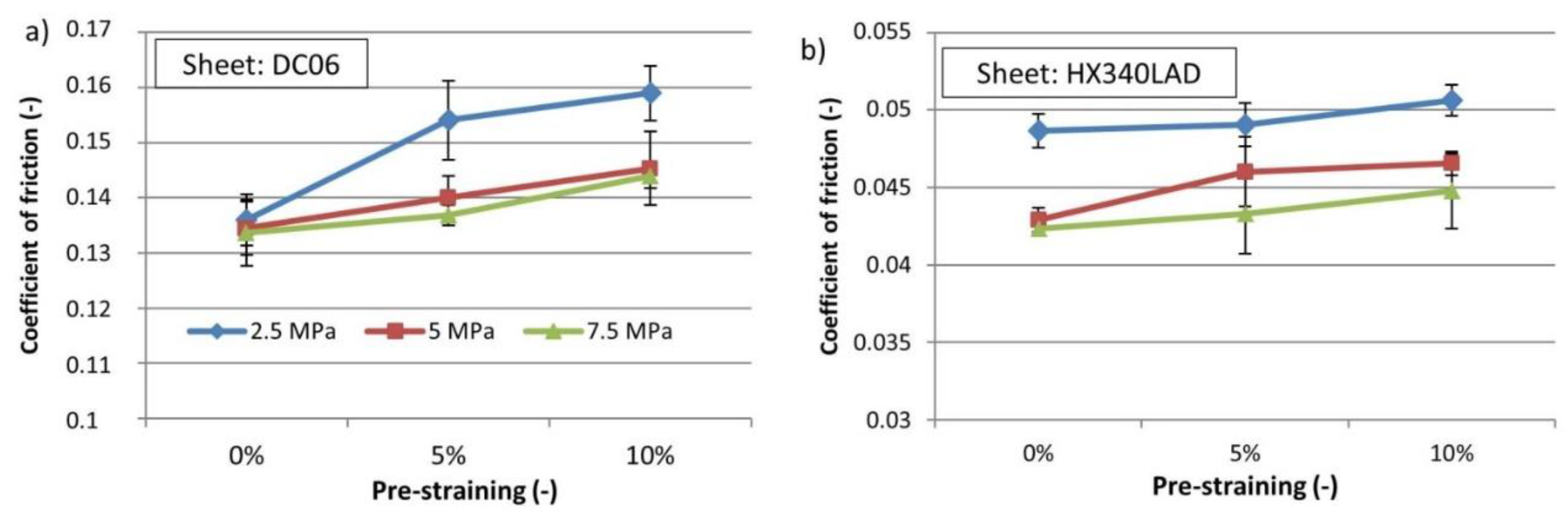
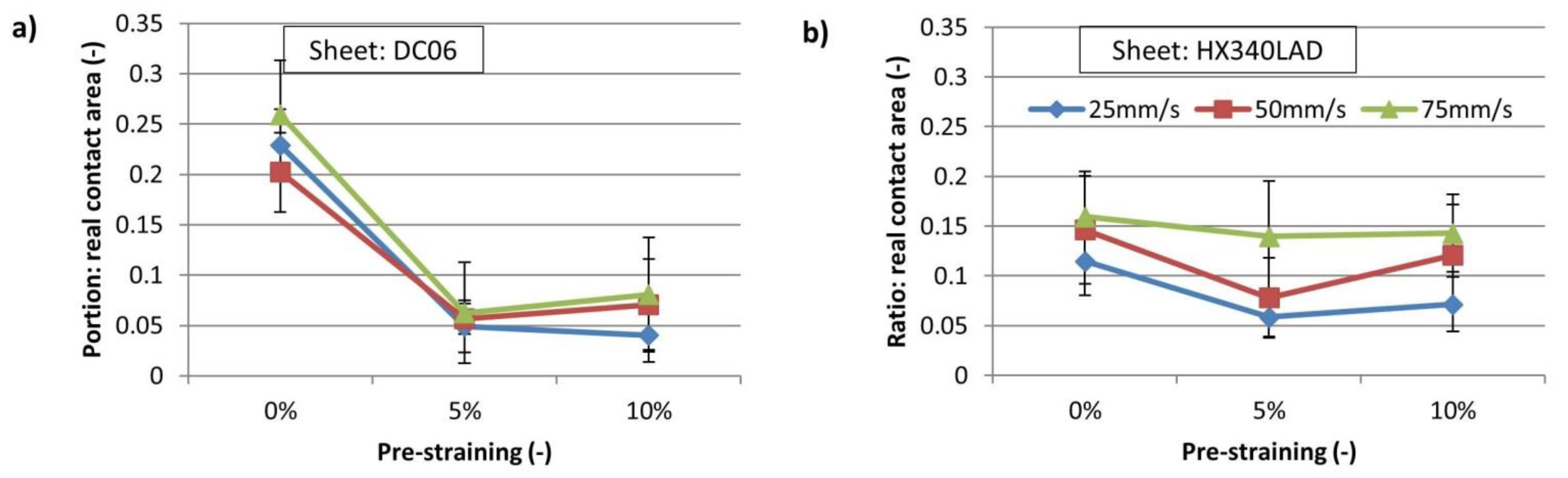
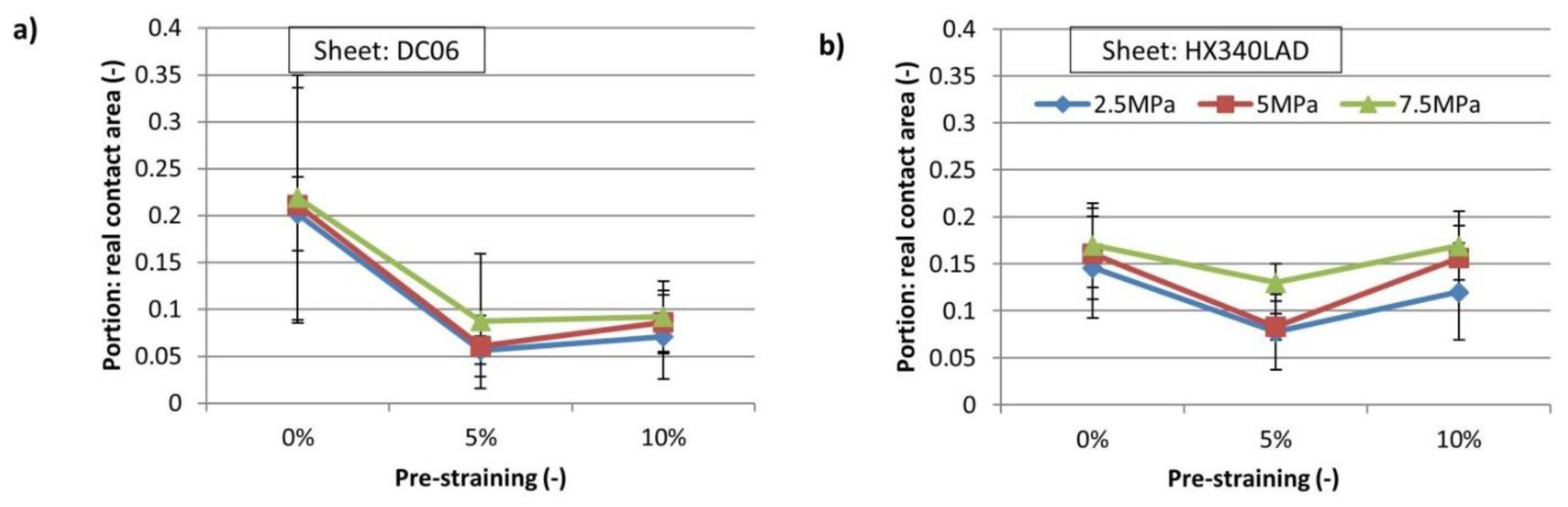
3.2. Evaluation of Coefficient of Friction (COF)
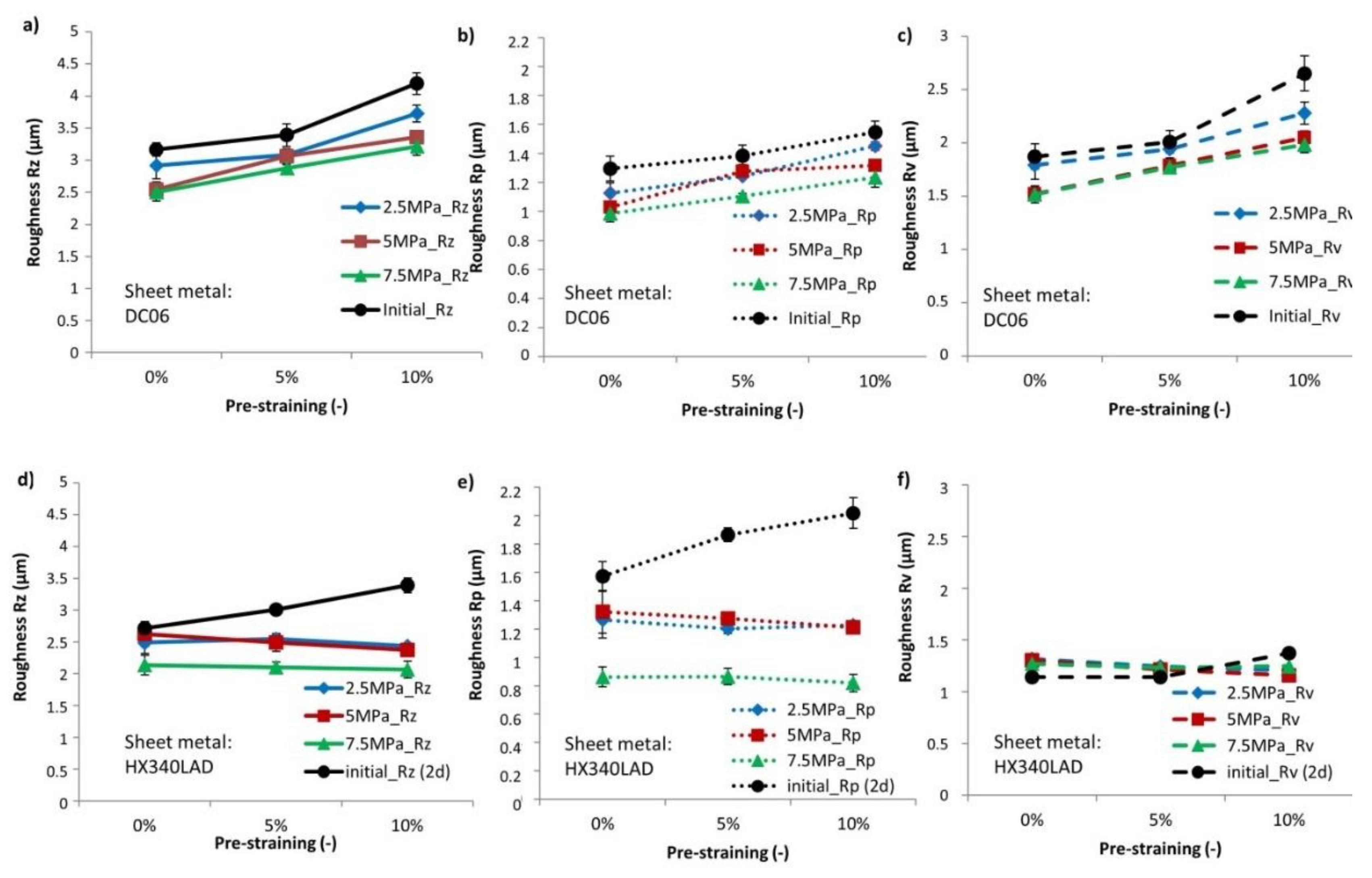
3.3. Evaluation of the Measured Surface Roughness
3.4. Optical Surface Observation of Sheet Metal Through Transparent Tool Set
4. Influence of the Strain of Galvanized Sheet Metal on Tool Wear
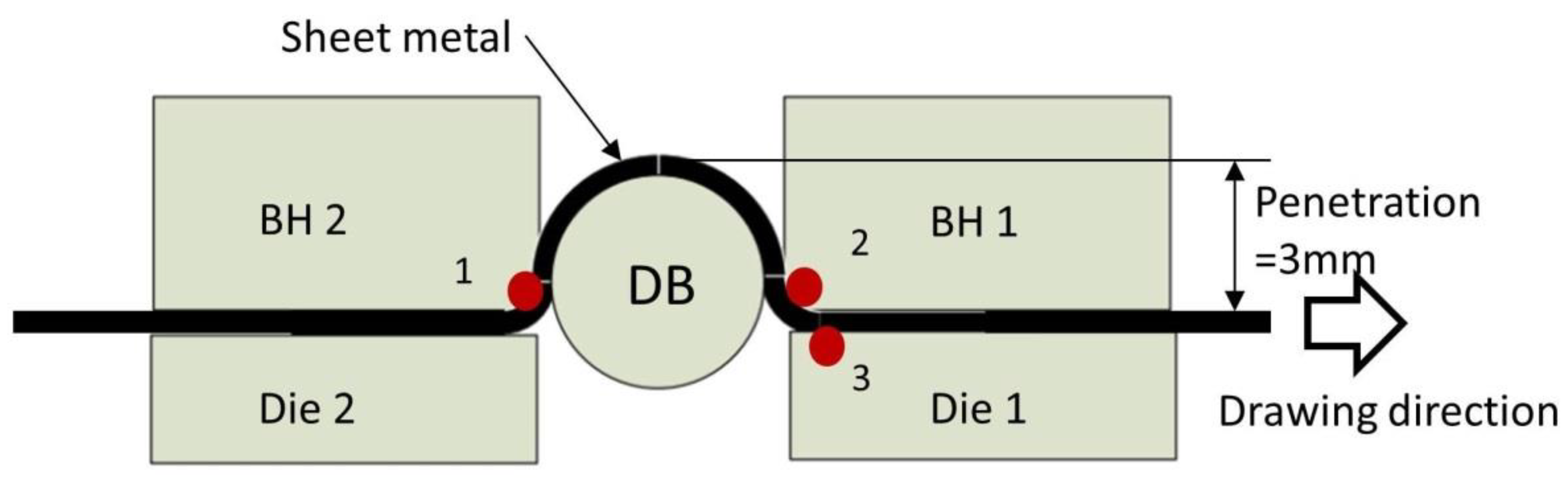
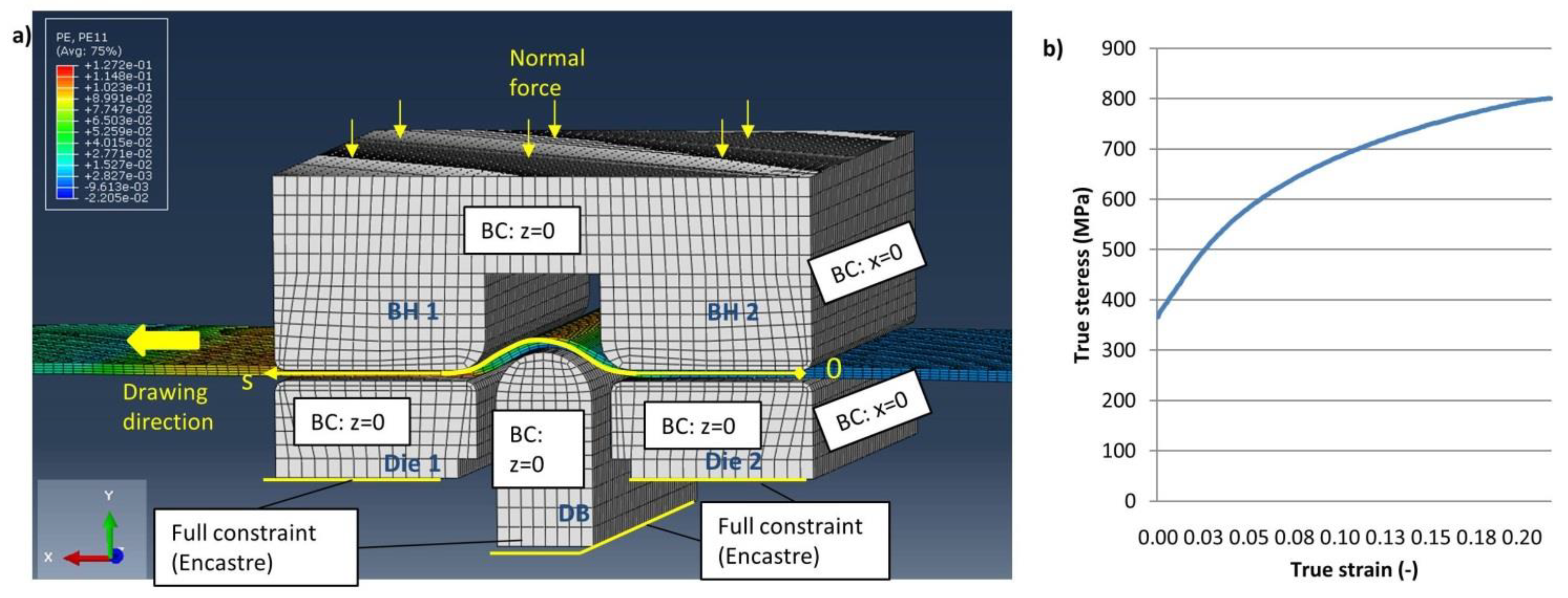
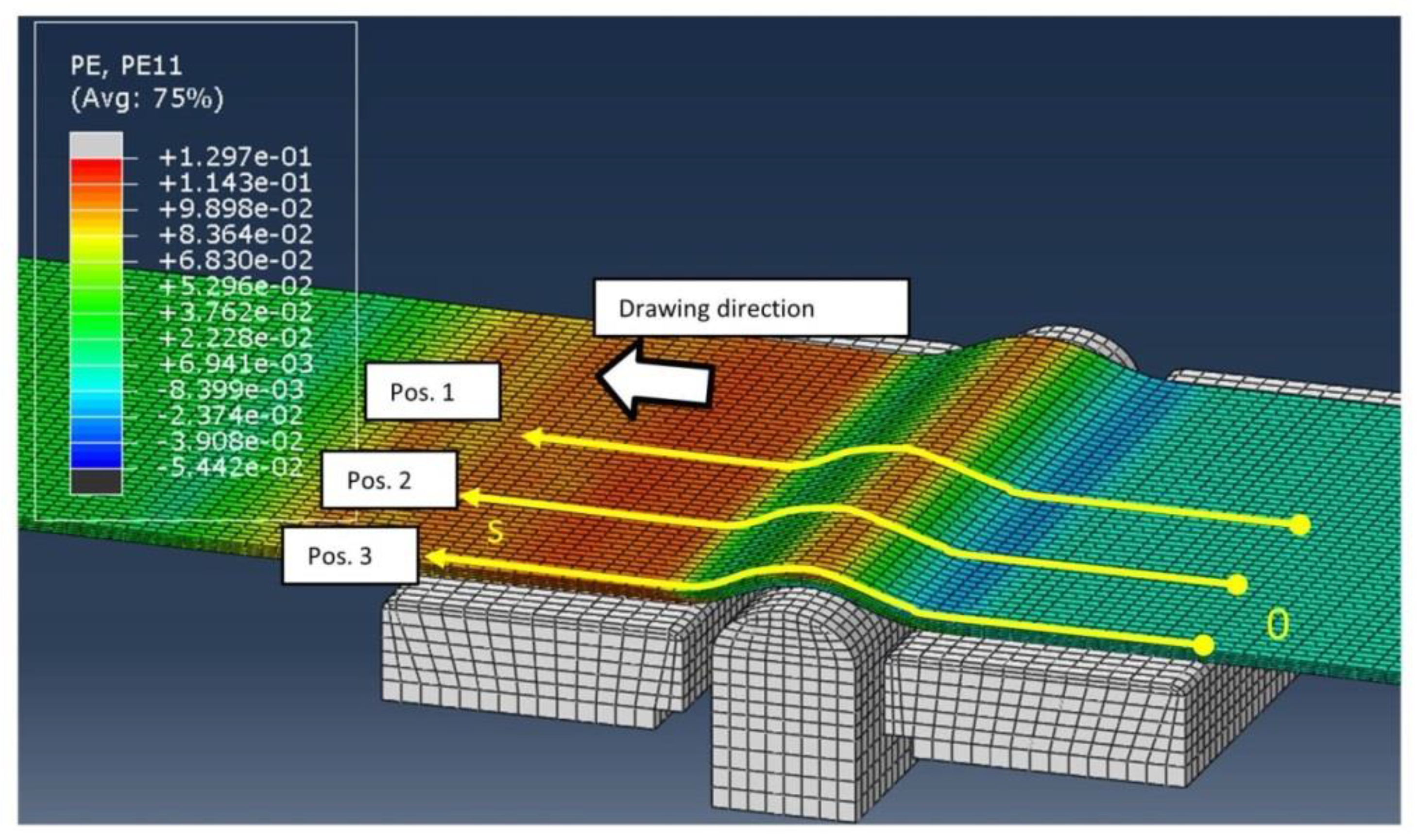
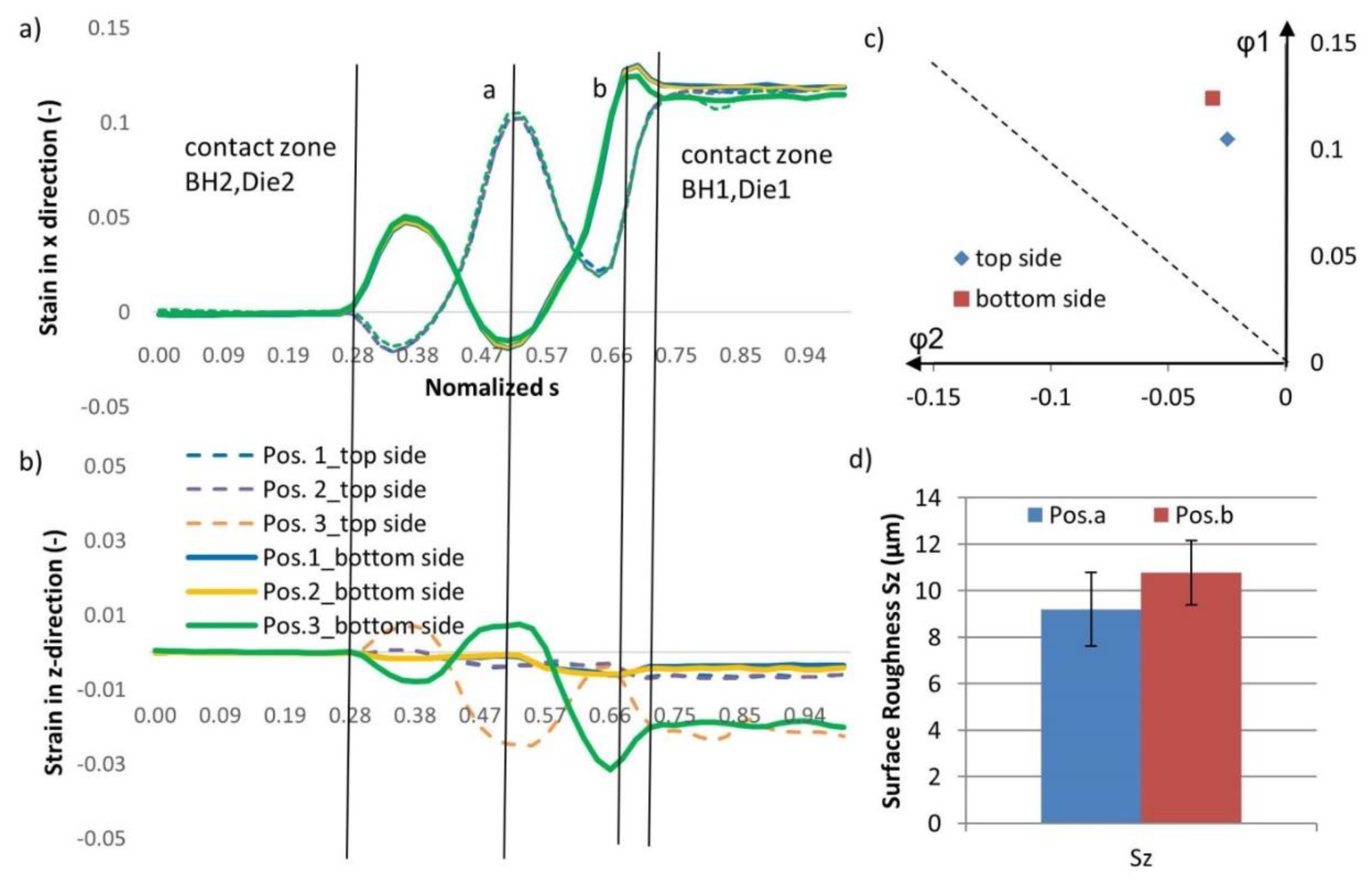
4.1. Process Simulation of the Strip Drawing Test with Draw Bead Geometry
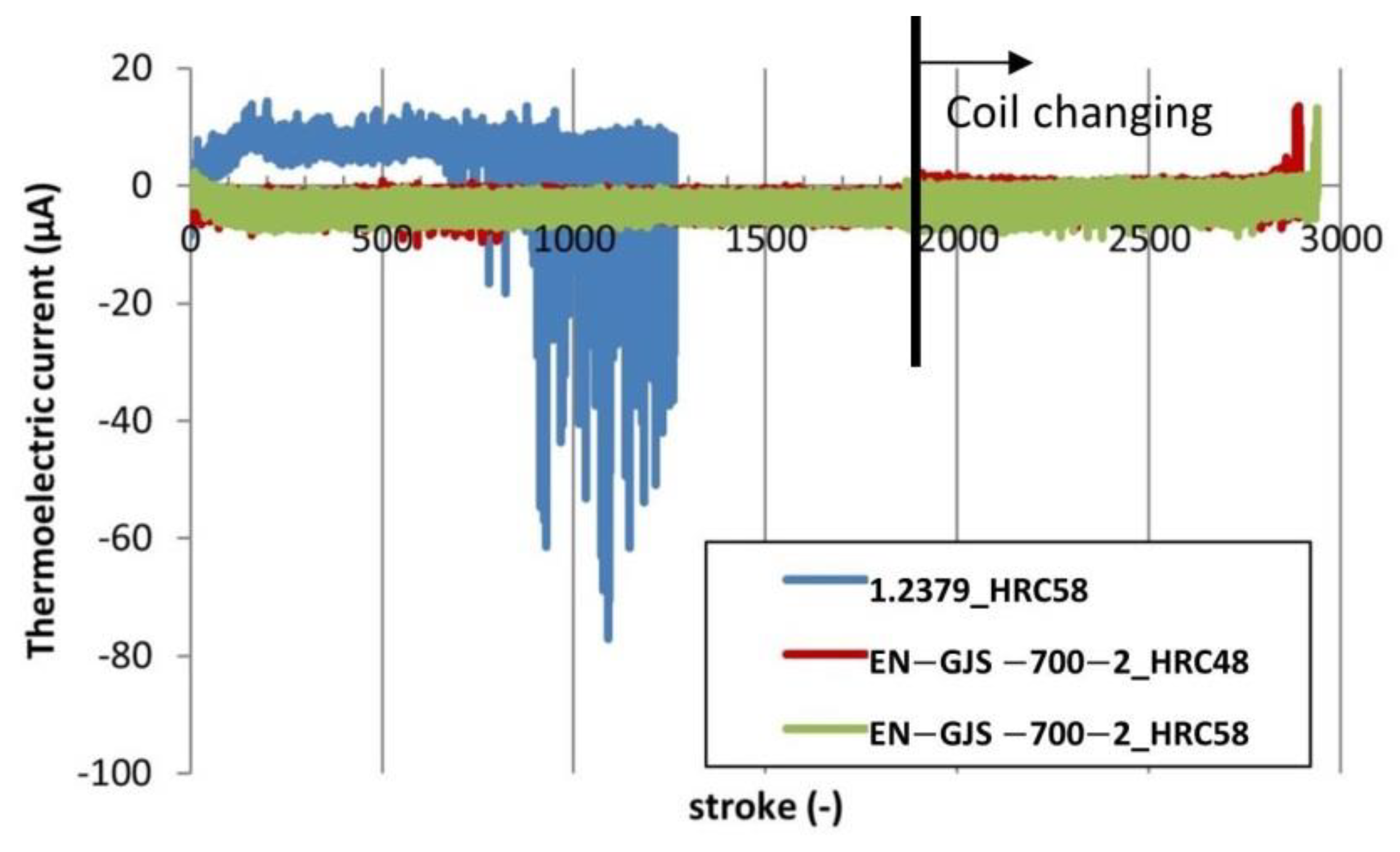
4.2. Wear Test
4.3. Characterizing the Wear Resistance of Different Experiments
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Birkert, A.R.; Haage, S.; Straub, M. Umformtechnische Herstellung Komplexer Karosserieteile: Auslegung von Ziehanlagen; Springer: Berlin/Heidelberg, Germany, 2013; ISBN 978-3-642-34670-5. [Google Scholar]
- Grabner, J.; Nothhaft, R. Konstruieren von Pkw-Karosserien: Grundlagen, Elemente und Baugruppen, Vorschriftenübersicht, Beispiele mit CATIA V4 und V5, 3., erw. Aufl.; Springer: Berlin, Germany, 2006; ISBN 978-3-540-32955-8. [Google Scholar]
- Tschätsch, H. Metal Forming Practise: Processes, Machines, Tools; Springer: Berlin, Germany, 2006; ISBN 9783540332169. [Google Scholar]
- Christiany, M.; Groche, P. Reproducibility of wear tests and the effect of load on tool life in sheet metal forming. Adv. Mater. Res. 2014, 1018, 293–300. [Google Scholar] [CrossRef]
- Popov, V.L. Contact Mechanics and Friction; Springer: Berlin/Heidelberg, Germany, 2010; ISBN 1282836994. [Google Scholar]
- Thomson, P.F.; Nayak, P.U. The effect of plastic deformation on the roughening of free surfaces of sheet metal. Int. J. Mach. Tool Des. Res. 1980, 20, 73–86. [Google Scholar] [CrossRef]
- Ludwig, M. Advanced friction model for cold forging processes. In Metal Forming; Wiley-VCH Verlag GmbH & Co.: Weinheim, Germany, 2012; pp. 1003–1006. [Google Scholar]
- Raabe, D.; Sachtleber, M.; Weiland, H.; Scheele, G.; Zhao, Z. Grain-scale micromechanics of polycrystal surfaces during plastic straining. Acta Mater. 2003, 51, 1539–1560. [Google Scholar] [CrossRef]
- Walner, E. Gläzende Perspektiven ThyssenKrupp Steel Europe Präsentiert Innovative Oberflächen für Beste Lackierung. 2013. Available online: https://www.pressebox.de/inaktiv/thyssenkrupp-steel-europe-ag/Glaenzende-Perspektiven/boxid/637671 (accessed on 1 March 2021).
- Love, J.C.; Smith, G.F.; Pharaoh, M.; Coates, R. Orange peel: Who cares? Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2001, 215, 1241–1244. [Google Scholar] [CrossRef]
- Schäfer, R. Kontaktgebundene Oberflächenwandlung Polykristalliner Blechoberflächen; Shaker: Aachen, Germany, 2008; ISBN 3832278907. [Google Scholar]
- Cao, S.Q.; Zhang, J.X.; Wu, J.S.; Chen, J.G. Effect of local texture on the orange peel defect in St14 steel sheet. In Materials Science Forum; Trans Tech Publications Ltd.: Freienbach, Switzerland, 2015; pp. 167–172. ISBN 087849975X. [Google Scholar]
- Guangnan, C.; Huan, S.; Shiguang, H.; Baudelet, B. Roughening of the free surfaces of metallic sheets during stretch forming. Mater. Sci. Eng. A 1990, 128, 33–38. [Google Scholar] [CrossRef]
- Kubo, M.; Nakazawa, Y.; Hama, T.; Takuda, H. Effect of Microstructure on Surface Roughening in Stretch Forming of Steel Sheets. ISIJ Int. 2017, 57, 2185–2193. [Google Scholar] [CrossRef]
- Banovic, S.W.; Foecke, T. The Effect of Microstructural Variables on the Surface Roughening of 5XXX Series Aluminum Sheet in Biaxial Tension. SAE Trans. 2002, 111, 181–188. [Google Scholar]
- Furushima, T.; Hirose, Y.; Tada, K.; Manabe, K.-I. Development of Compact Marchiniak Testing Apparatus for In-situ Microscopic Observation of Surface Roughening. Procedia Eng. 2017, 207, 1946–1951. [Google Scholar] [CrossRef]
- Filzek, J. Kombinierte Prüfmethode Für Das Reib-, Verschleiß-Und Abriebverhalten Beim Tief-Und Streckziehen; Zugl.: Darmstadt, Germany; Shaker: Aachen, Germany, 2004; ISBN 383223523X. [Google Scholar]
- Azushima, A.; Kudo, H. Direct Observation of Contact Behaviour to Interpret the Pressure Dependence of the Coefficient of Friction in Sheet Metal Forming. CIRP Ann. 1995, 44, 209–212. [Google Scholar] [CrossRef]
- Bech, J.; Bay, N.; Eriksen, M. Entrapment and escape of liquid lubricant in metal forming. Wear 1999, 232, 134–139. [Google Scholar] [CrossRef]
- Staeves, J.; Schmoeckel, D. Topography of sheet metal and its relationship to the tribological behaviour during the forming process. In Proceedings of the 1st International Conference on Tribology in Manufacturing Process, Gifu, Japan, 19–23 October 1997; pp. 19–23. [Google Scholar]
- Trzepieciński, T.; Bochnowski, W.; Witek, L. Variation of surface roughness, micro-hardness and friction behaviour during sheet-metal forming. Int. J. Surf. Sci. Eng. 2018, 12, 119–136. [Google Scholar] [CrossRef]
- Wu, Y.; Groche, P. Influence of Tool Finishing on the Wear Development in Strip Drawing Tests with High Strength Steels. Tribol. Online 2020, 15, 170–180. [Google Scholar] [CrossRef]
- Groche, P.; Christiany, M.; Wu, Y. Load-dependent wear in sheet metal forming. Wear 2019, 422–423, 252–260. [Google Scholar] [CrossRef]
- Tsukizoe, T.; Hisakado, T. On the mechanism of contact between metal surfaces—The penetrating depth and the average clearance. J. Basic Eng. 1965, 87, 666–672. [Google Scholar] [CrossRef]
- Ghoshal, G.; Turner, J.A. Numerical model of longitudinal wave scattering in polycrystals. IEEE Trans. Ultrason. Ferroelectr. Freq. Control. 2009, 56, 1419–1428. [Google Scholar] [CrossRef] [PubMed]
- Zhang, K.S.; Wu, M.S.; Feng, R. Simulation of microplasticity-induced deformation in uniaxially strained ceramics by 3-D Voronoi polycrystal modeling. Int. J. Plast. 2005, 21, 801–834. [Google Scholar] [CrossRef]
- Zinovieva, O.; Romanova, V.; Balokhonov, R.; Zinoviev, A.; Kovalevskaya, Z. Numerical study of the influence of grain size and loading conditions on the deformation of a polycrystalline aluminum alloy. J. Appl. Math. Phys. 2014, 2, 425–430. [Google Scholar] [CrossRef][Green Version]
- Panin, A.V.; Romanova, V.A.; Balokhonov, R.R.; Perevalova, O.B.; Sinyakova, E.A.; Emelyanova, O.S.; Leontieva-Smirnova, M.V.; Karpenko, N.I. Mesoscopic surface folding in EK-181 steel polycrystals under uniaxial tension. Phys. Mesomech. 2012, 15, 94–103. [Google Scholar] [CrossRef]
- Huang, Y. A User-Material Subroutine Incroporating Single Crystal Plasticity in the ABAQUS Finite Element Program; Harvard University: Cambridge, MA, USA, 1991. [Google Scholar]
- Raabe, D.; Roters, F.; Wang, Y.W. Simulation of Earing during Deep Drawing of bcc Steel by Use of a Texture Component Crystal Plasticity Finite Element Method. Mater. Sci. Forum 2005, 495–497, 1529–1534. [Google Scholar] [CrossRef]
- Demant, C.; Streicher-Abel, B.; Garnica, C. Industrial Image Processing: Visual Quality Control in Manufacturing, 2nd ed.; Springer: Berlin, Germany, 2013; ISBN 9783642339042. [Google Scholar]
- DIN German Institute for Standardization. Geometrical Product Specifications (GPS)—Surface Texture: Profile Method—Terms, Definitions and Surface Texture Parameters; Beuth Verlag GmbH: Berlin, Germany, 1998. [Google Scholar]
- Hol, J.; Alfaro, M.C.; de Rooij, M.B.; Meinders, T. Advanced friction modeling for sheet metal forming. Wear 2012, 286, 66–78. [Google Scholar] [CrossRef]
- Groche, P.; Christiany, M. Evaluation of the potential of tool materials for the cold forming of advanced high strength steels. Wear 2013, 302, 1279–1285. [Google Scholar] [CrossRef]
- Archard, J. Contact and rubbing of flat surfaces. J. Appl. Phys. 1953, 24, 981–988. [Google Scholar] [CrossRef]
- Groche, P.; Wu, Y. Inline observation of tool wear in deep drawing with thermoelectric and optical measurements. CIRP Ann. 2019, 68, 567–570. [Google Scholar] [CrossRef]
- Moghadam, M.; Nielsen, C.V. Limits of Lubrication in Severe Stamping Operations; Technical University of Denmark: Copenhagen, Denmark, 2019. [Google Scholar]
- Rangarajan, V.; Jagannathan, V.; Raghavan, K.S. Influence of strain state on powdering of galvannealed sheet steel. SAE Trans. 1996, 68–75. [Google Scholar] [CrossRef]
- Tröber, P.; Demmel, P.; Hoffmann, H.; Golle, R.; Volk, W. On the influence of Seebeck coefficients on adhesive tool wear during sheet metal processing. CIRP Ann. 2017, 66, 293–296. [Google Scholar] [CrossRef]
- Bayer, R.J. Mechanical Wear Fundamentals and Testing, Revised and Expanded; CRC Press: Boca Raton, FL, USA, 2004; ISBN 0203021797. [Google Scholar]
- Gahr, K.H.Z. Modelling of two-body abrasive wear. Wear 1988, 124, 87–103. [Google Scholar] [CrossRef]
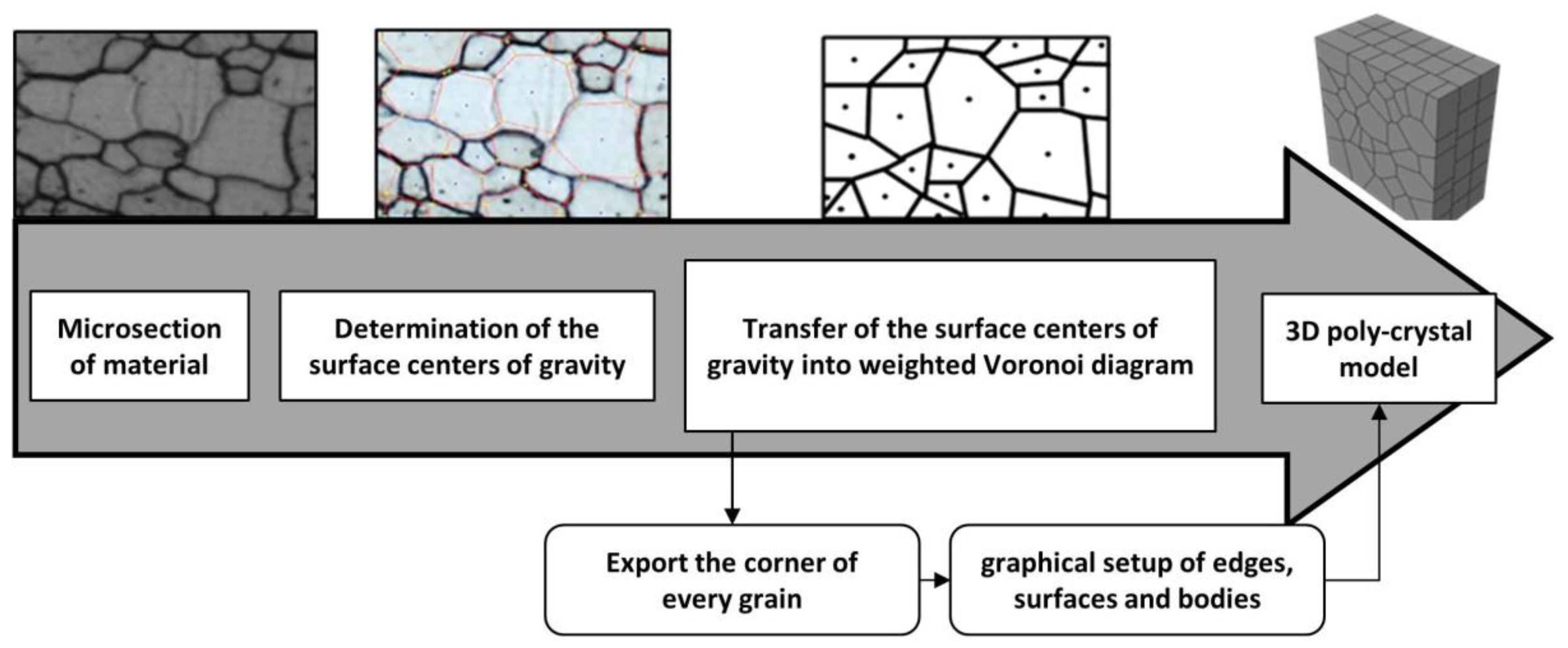
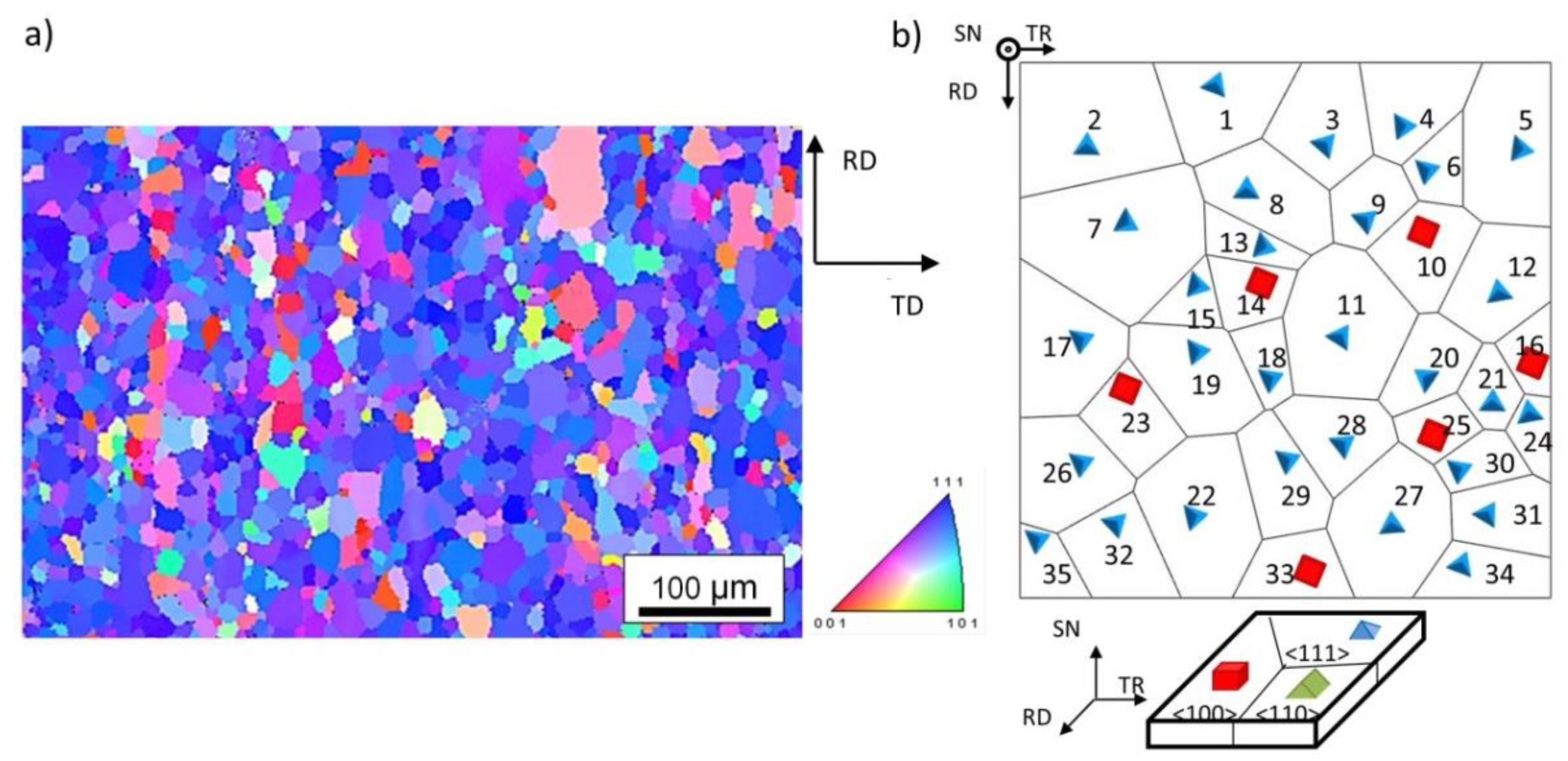
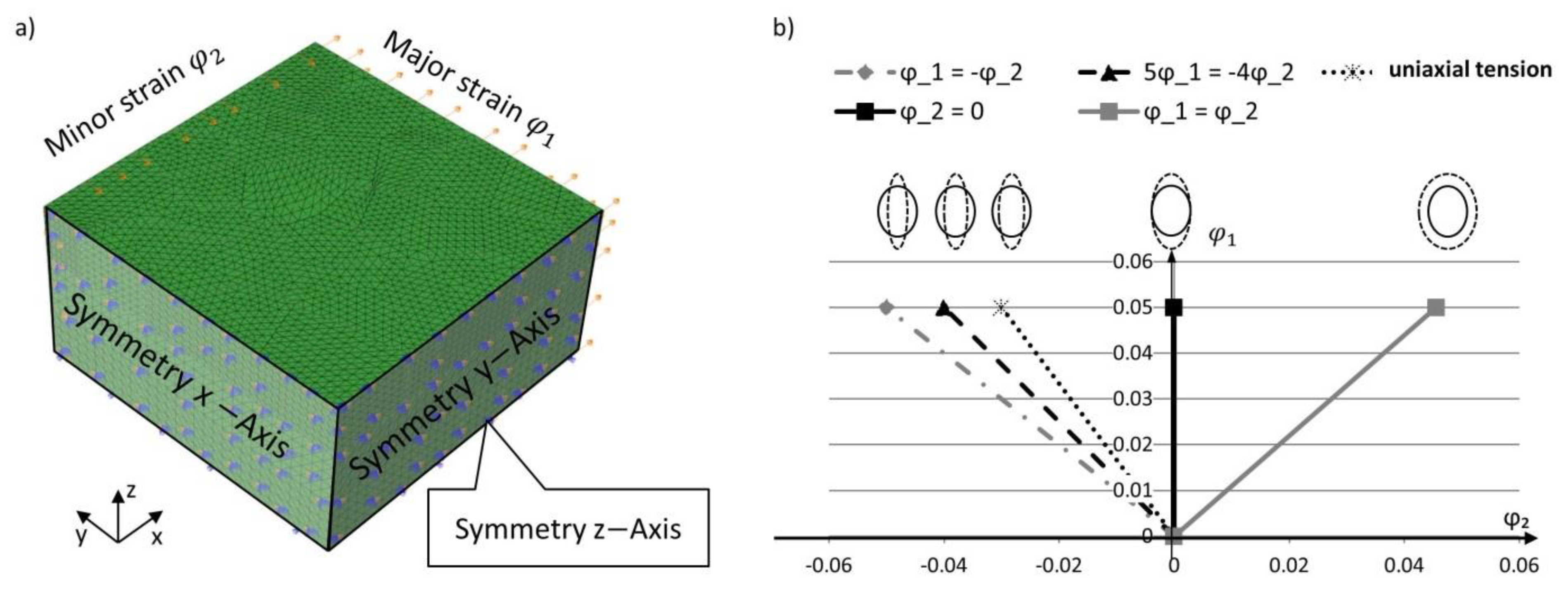
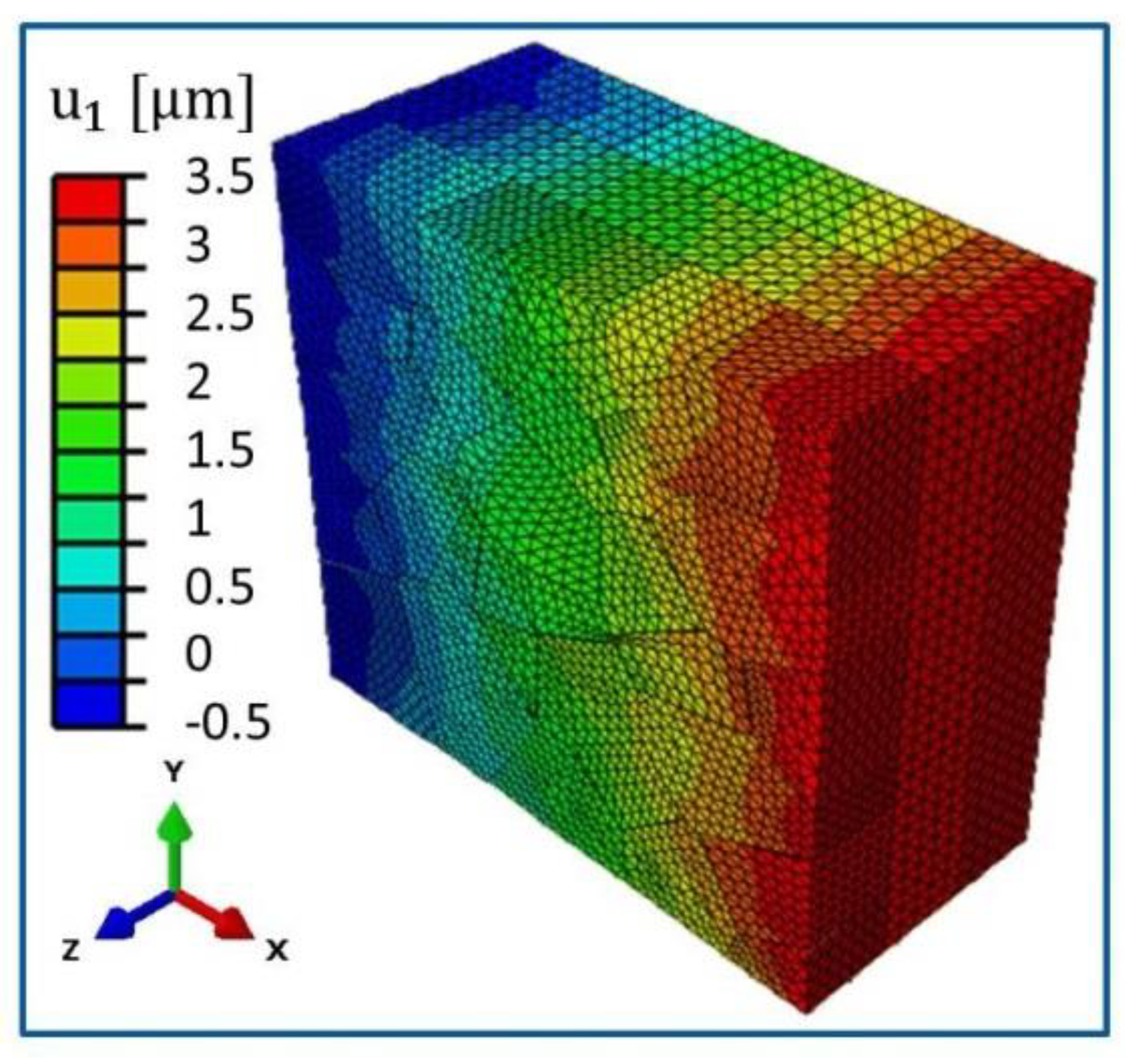
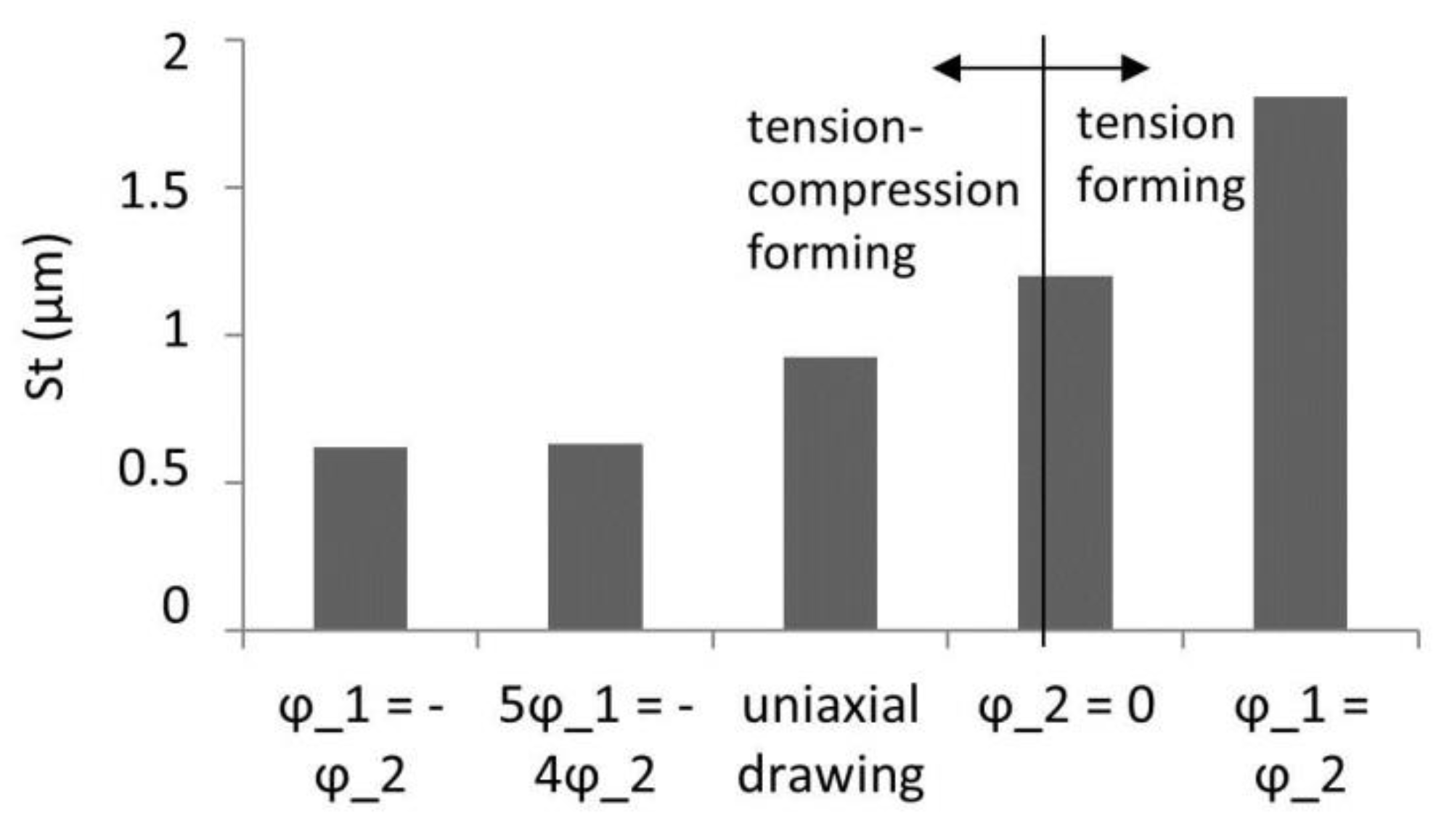
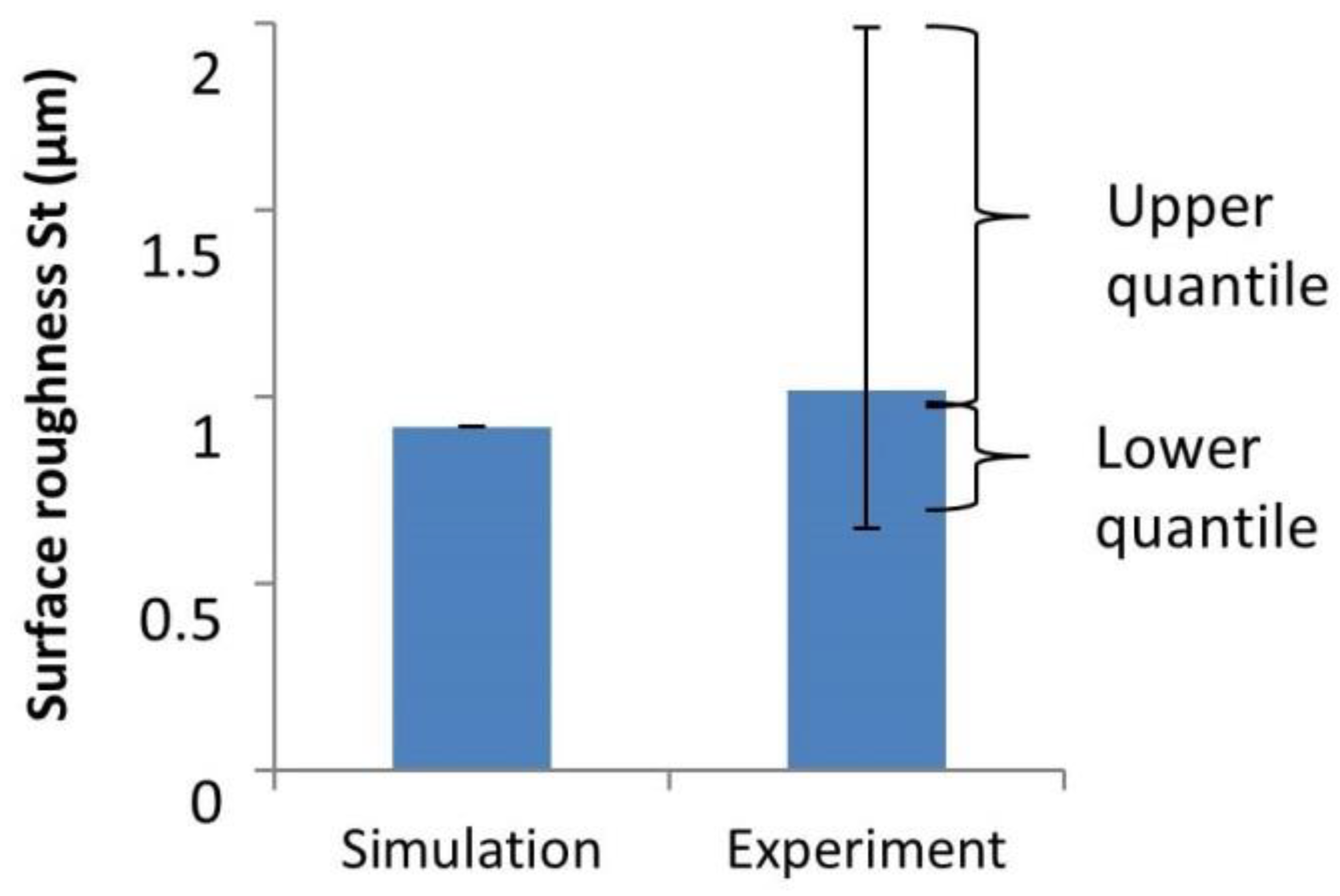

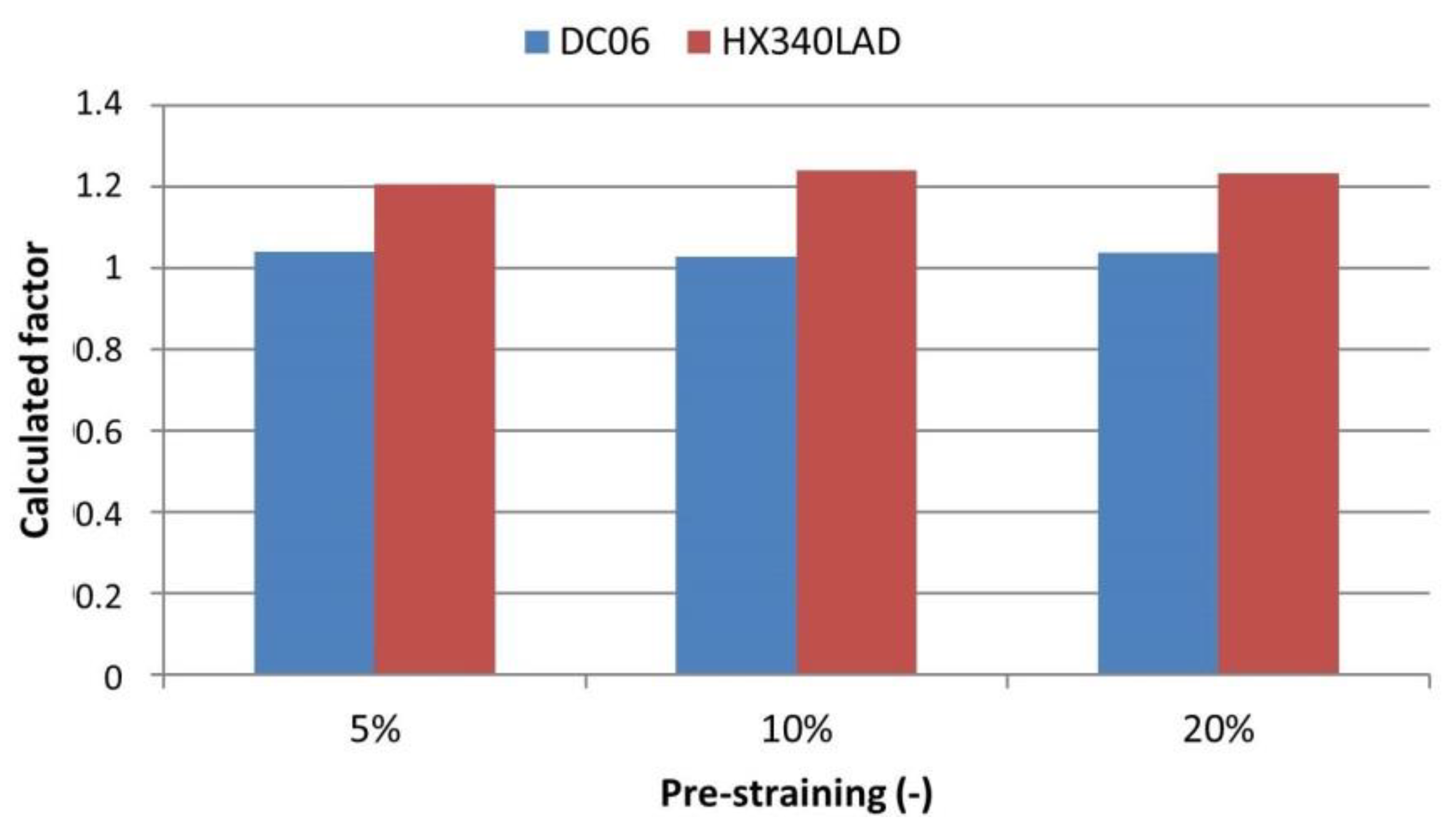
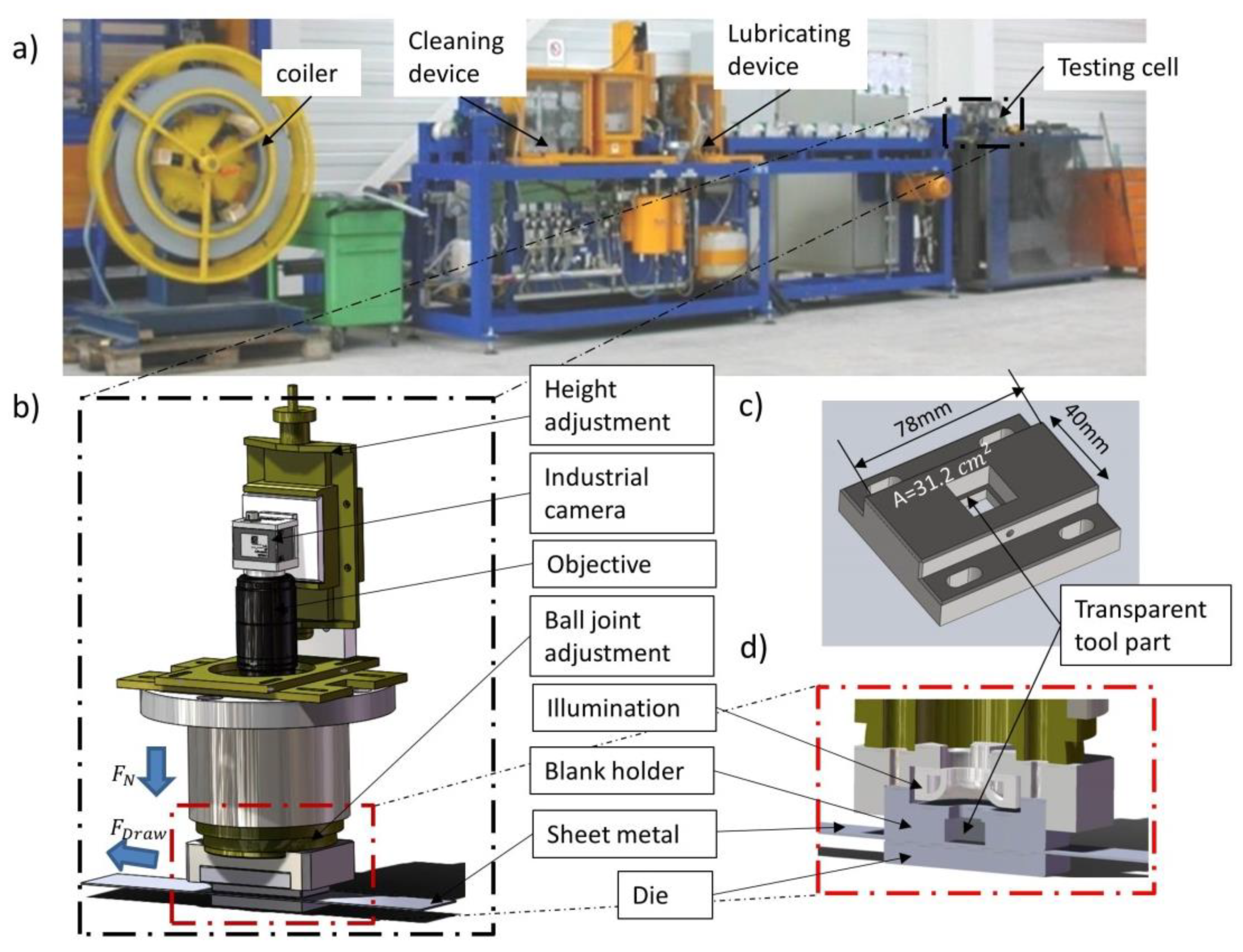


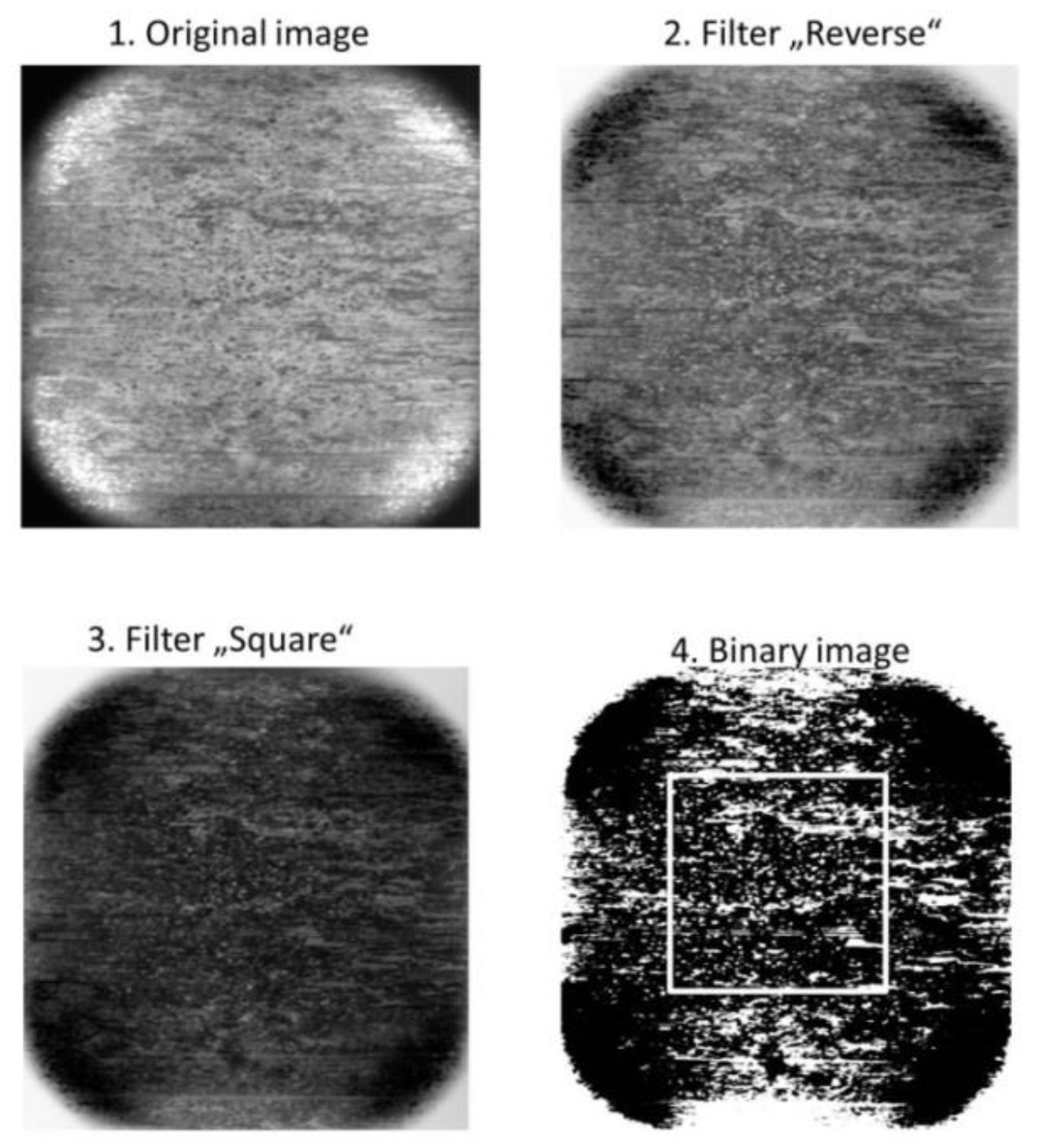

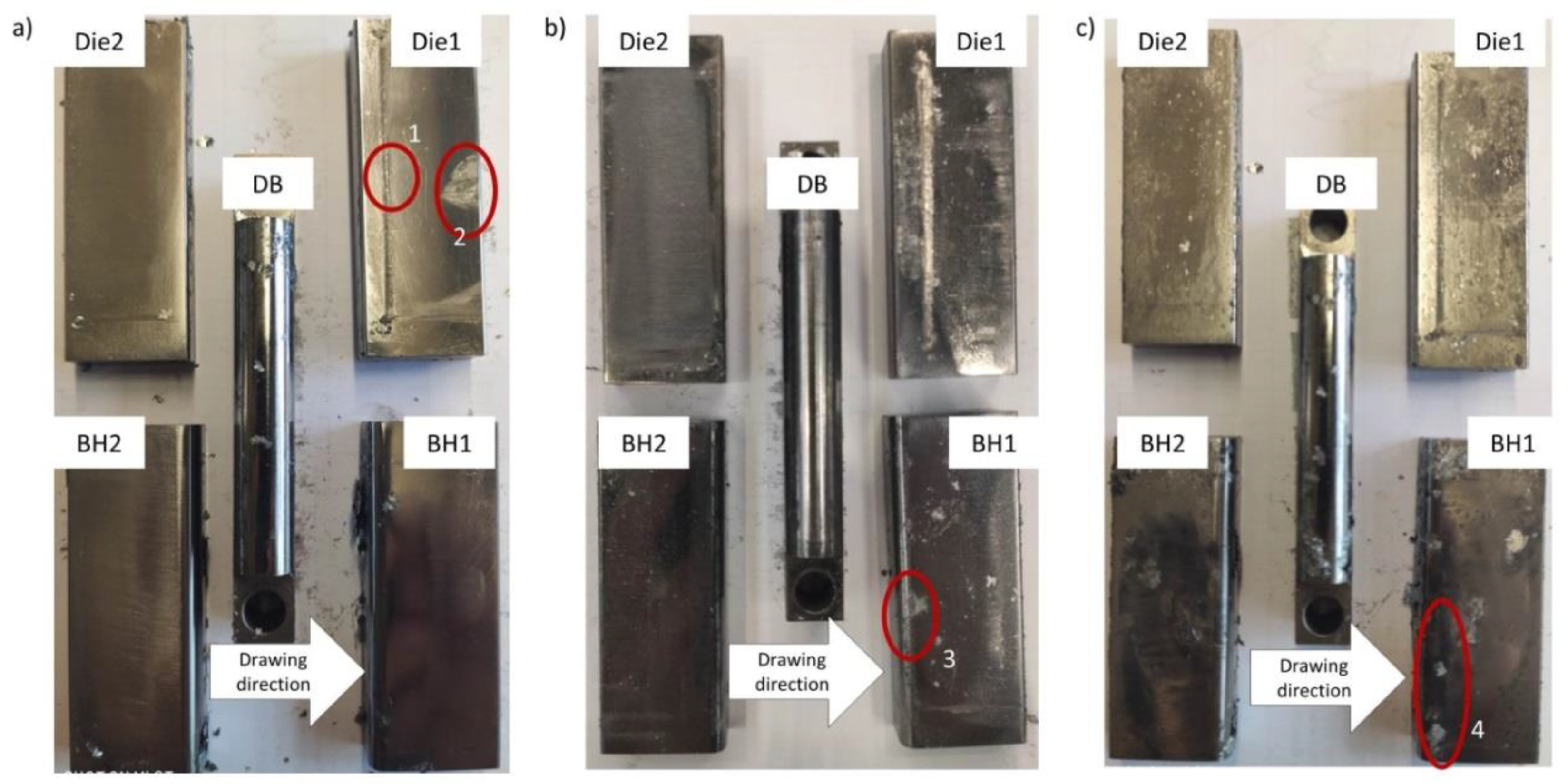
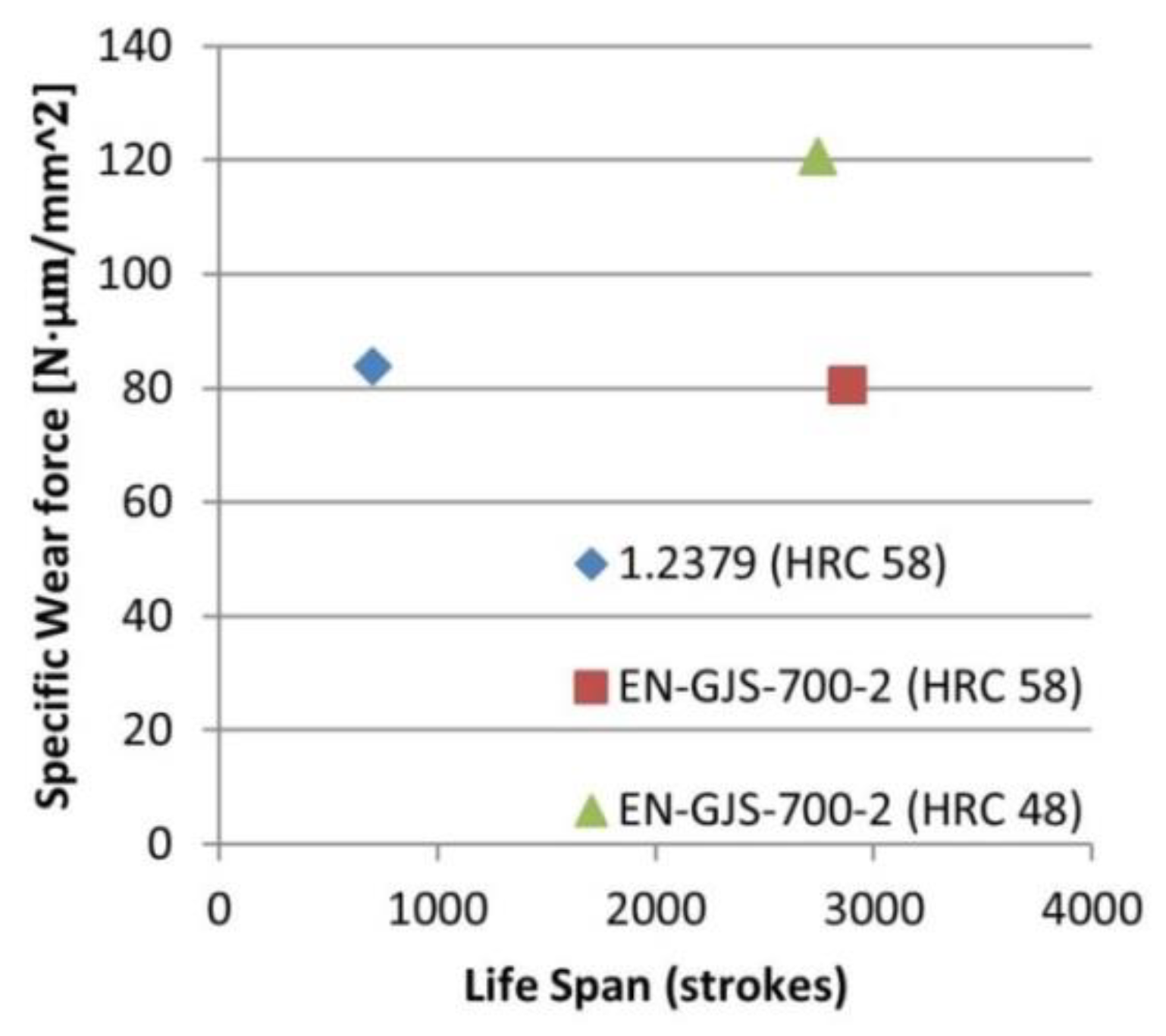
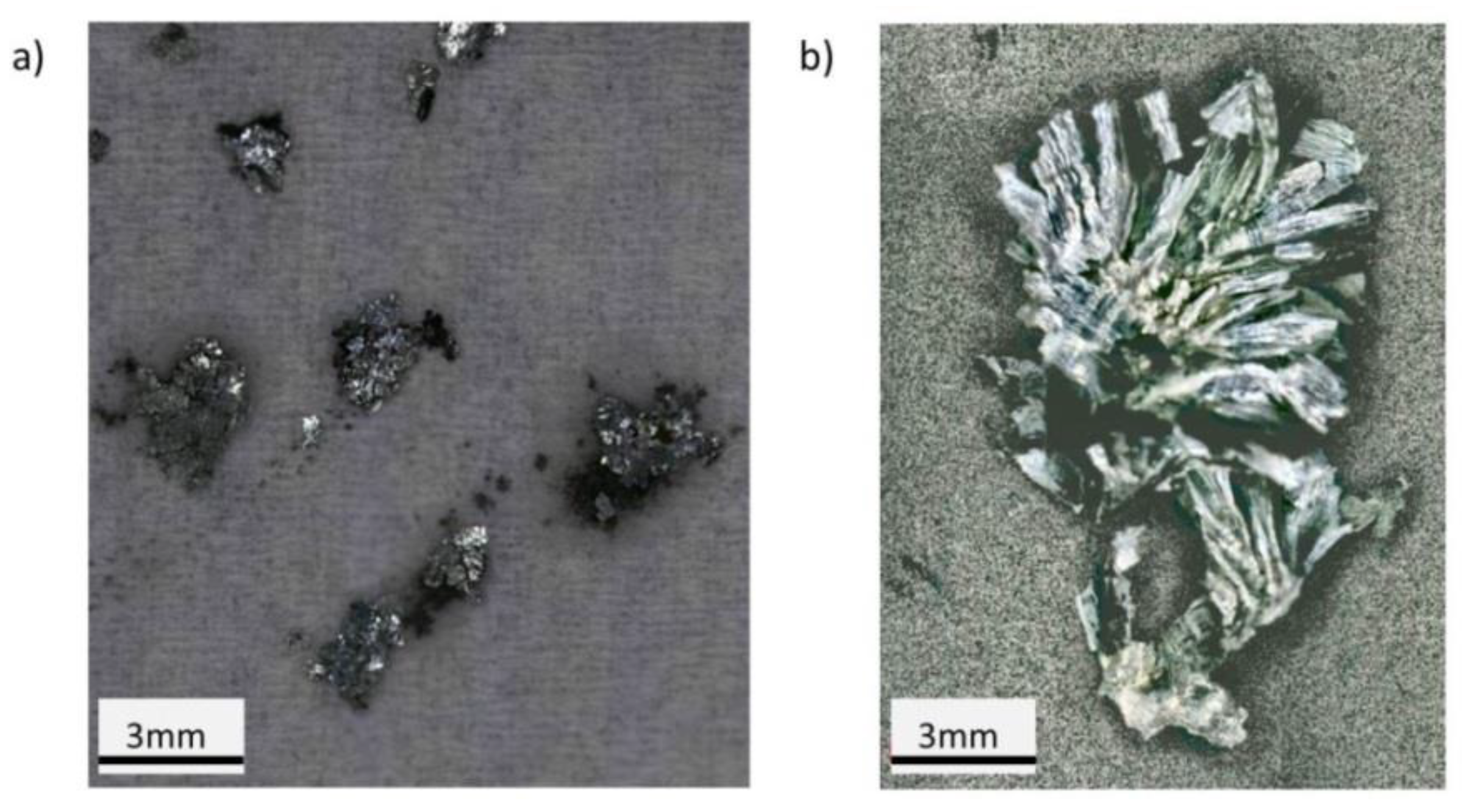

| Symbol | Nomination [Unit] | Value |
|---|---|---|
| Components of elasticity tensor [GPa] | 230.1 | |
| Components of elasticity tensor [GPa] | 134.6 | |
| Components of elasticity tensor [GPa] | 116.6 | |
| Initial system hardening module [MPa] | 180 | |
| saturation shear stress [MPa] | 148 | |
| Initial shear stress [MPa] | 16 | |
| ] | 0.05 | |
| Stress sensibility exponent [-] | 10 | |
| Coplanar hardening ratio [-] | 1 | |
| Non-coplanar hardening ratio [-] | 1.4 |
| Boundary Conditions | Description |
|---|---|
| For minimizing the boundary effect, three symmetry conditions are applied | |
| Displacement u in x-axis | Assigning the uniaxial and biaxial strain from 0% to 5% (see Figure 3b) |
| Displacement v in y-axis | |
| Normal contact | “Hard contact” with linear penalty contact condition (value = 1) |
| Tangential contact | Penalty with µ = 0.1 |
| Experimental Parameters | ||
|---|---|---|
| Sheet metal type | DC06 | HX340LAD |
| Pre-straining (-) | 5%; 10%; 20% | |
| ) | 75 | 150 |
| Experimental Parameters | |
|---|---|
| Sheet metal type | DC06; HX340LAD |
| Sliding velocity (mm/s) | 25; 50; 75 |
| Tool material | 1.2379 (X155CrVMo12-1) 58 HRC |
| ) | 2 |
| Lubricant | Zeller Gmelin PL61 |
| Contact stress (MPa) | 2.5; 5; 7.5 |
| Pre-straining (-) | 0%; 5%; 10% |
| Slinding length (mm) | 100 |
| Experimental Parameters | |
|---|---|
| Sheet metal type | DP600, 50 mm width, 1.2 mm thick |
| Drawing Velocity (mm/s) | 100 |
| Tool material | 1.2379 (X155CrVMo12-1) 58 HRC; EN-GJS-700-2 (DIN EN 16482) 58 HRC; EN-GJS-700-2 (DIN EN 16482) 48 HRC |
| ) | 2 |
| Lubricant | Zeller Gmelin PL61 |
| Blank holder force (kN) | 30 |
| Sliding length per stroke (mm) | 100 |
| Description of Tool | Max. Contact Stress | Penetration z | Tool Hardness | Sheet Hardness | Specific Wear Force | ||
|---|---|---|---|---|---|---|---|
| [] | [µm] | [HV] | [ | [HV] | [ | ||
| Pos.2 of 1.2379 with hardness HRC 58 | 486 | 4.61 | 756 | 2510 | 340 | 1095 | 82.4 |
| Pos.3 of 1.2379 with hardness HRC 58 | 477 | 4.866 | 756 | 2510 | 340 | 1095 | 83.95 |
| Pos.2 of EN-GJS-700-2 with hardness HRC 58 | 486 | 4.58 | 756 | 2510 | 340 | 1095 | 82.04 |
| Pos.3 of EN-GJS-700-2 with hardness HRC 58 | 477 | 4.76 | 756 | 2510 | 340 | 1095 | 82.12 |
| Pos.2 of EN-GJS-700-2 with hardness HRC 48 | 486 | 4.68 | 655 | 2190 | 340 | 1095 | 127.53 |
| Pos.3 of EN-GJS-700-2 with hardness HRC 48 | 477 | 4.95 | 655 | 2190 | 340 | 1095 | 128.55 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, Y.; Recklin, V.; Groche, P. Strain Induced Surface Change in Sheet Metal Forming: Numerical Prediction, Influence on Friction and Tool Wear. J. Manuf. Mater. Process. 2021, 5, 29. https://doi.org/10.3390/jmmp5020029
Wu Y, Recklin V, Groche P. Strain Induced Surface Change in Sheet Metal Forming: Numerical Prediction, Influence on Friction and Tool Wear. Journal of Manufacturing and Materials Processing. 2021; 5(2):29. https://doi.org/10.3390/jmmp5020029
Chicago/Turabian StyleWu, Yutian, Viktor Recklin, and Peter Groche. 2021. "Strain Induced Surface Change in Sheet Metal Forming: Numerical Prediction, Influence on Friction and Tool Wear" Journal of Manufacturing and Materials Processing 5, no. 2: 29. https://doi.org/10.3390/jmmp5020029
APA StyleWu, Y., Recklin, V., & Groche, P. (2021). Strain Induced Surface Change in Sheet Metal Forming: Numerical Prediction, Influence on Friction and Tool Wear. Journal of Manufacturing and Materials Processing, 5(2), 29. https://doi.org/10.3390/jmmp5020029





