Biomimetic Drones Inspired by Dragonflies Will Require a Systems Based Approach and Insights from Biology
Abstract
:1. Introduction
Biomimetics and Bioinspiration
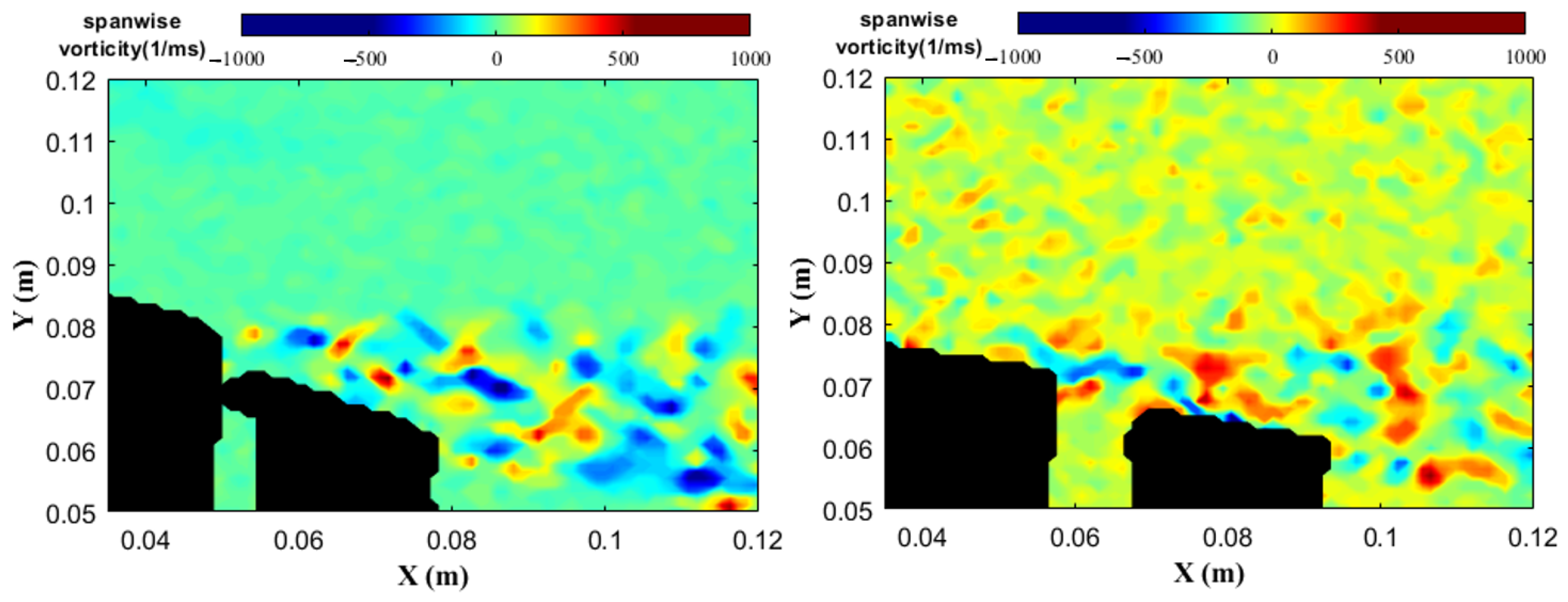
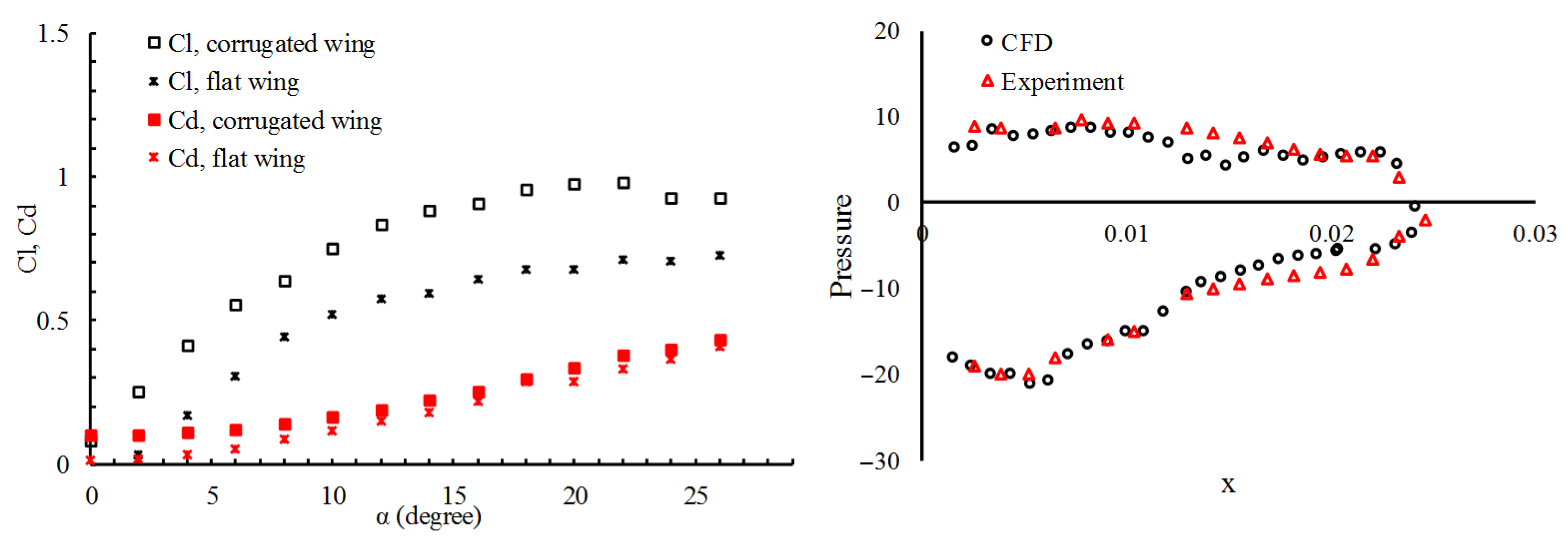
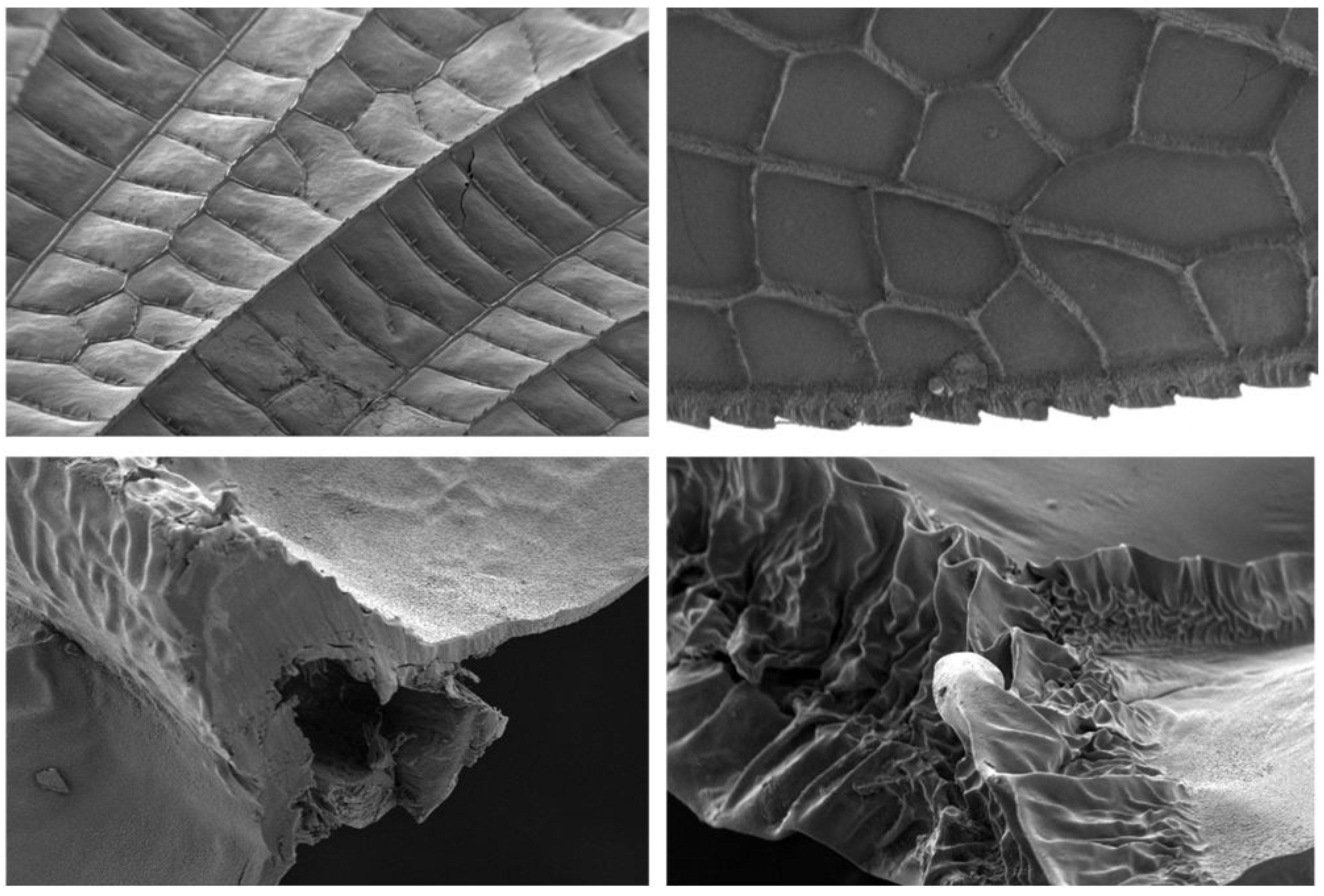
2. Wing Performance and Structure
Lessons from the Dragonfly Wing
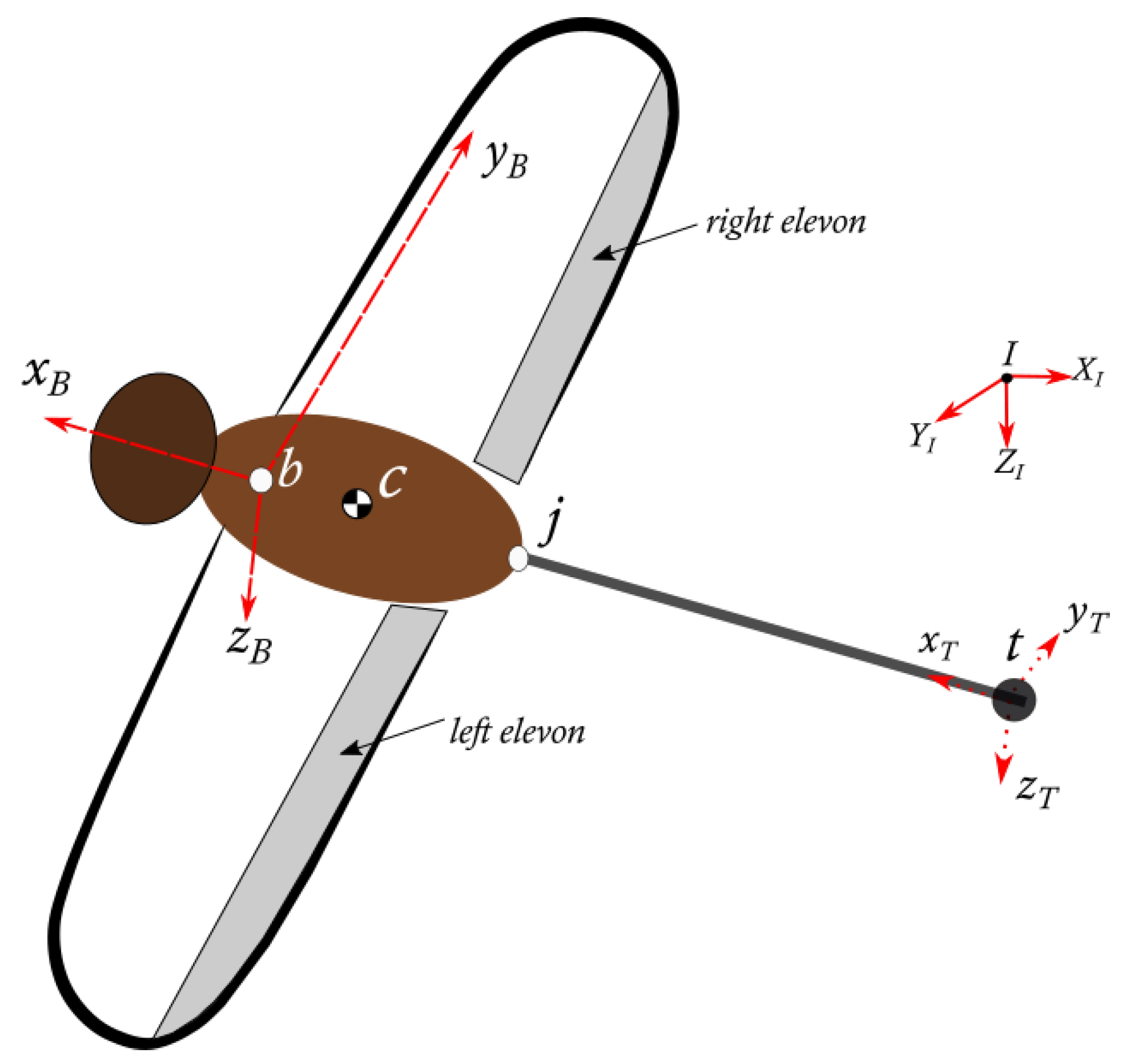
3. Body Articulation
3.1. Equations of Motion
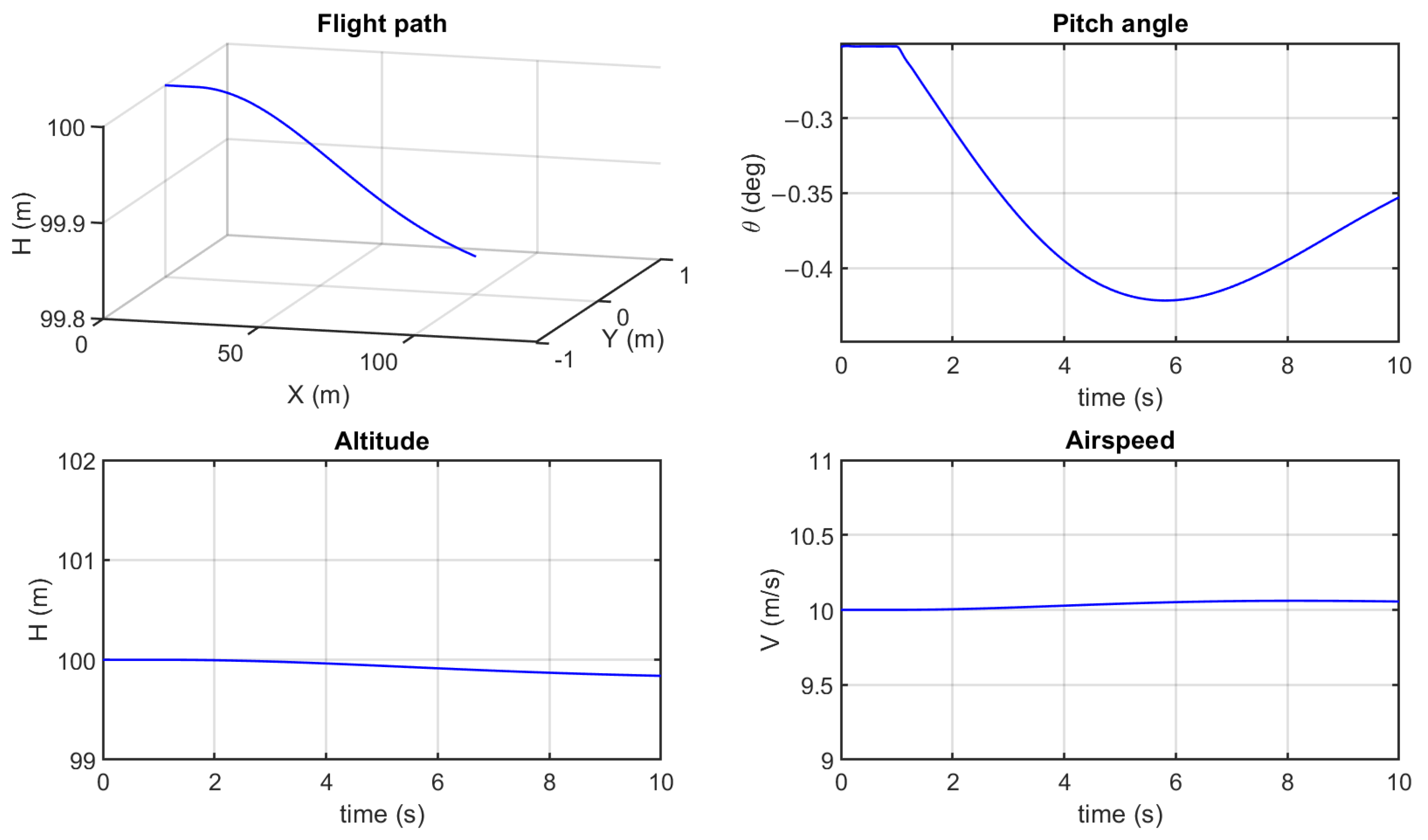
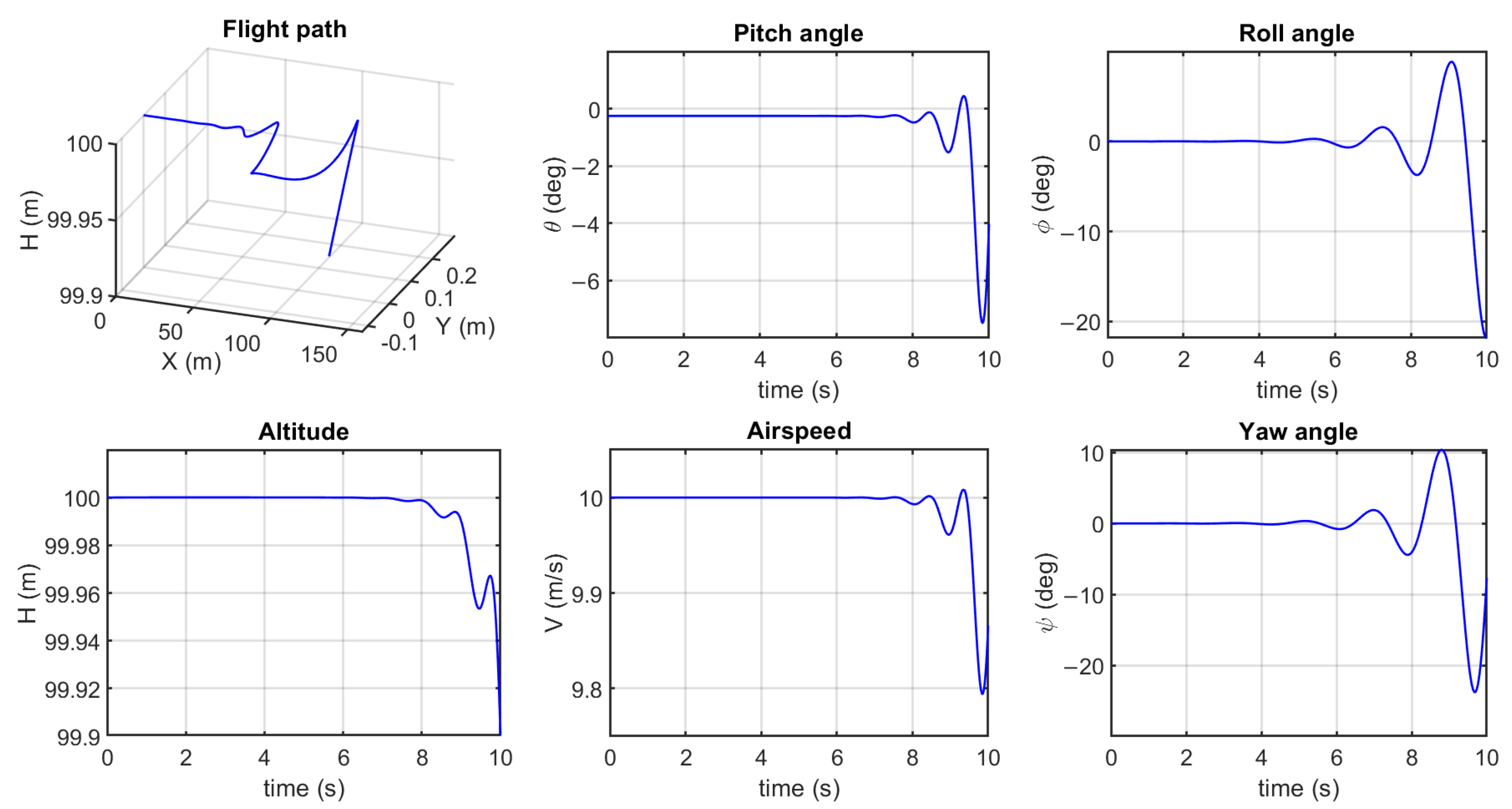
3.2. Flight Simulation for Maneuvering
Simulation Results and Analysis
3.3. Integrated Function of Morphological Modifications in Flight
3.4. Lessons from Abdominal Control
4. Wing Beat System Dynamics
Lessons from System Dynamics
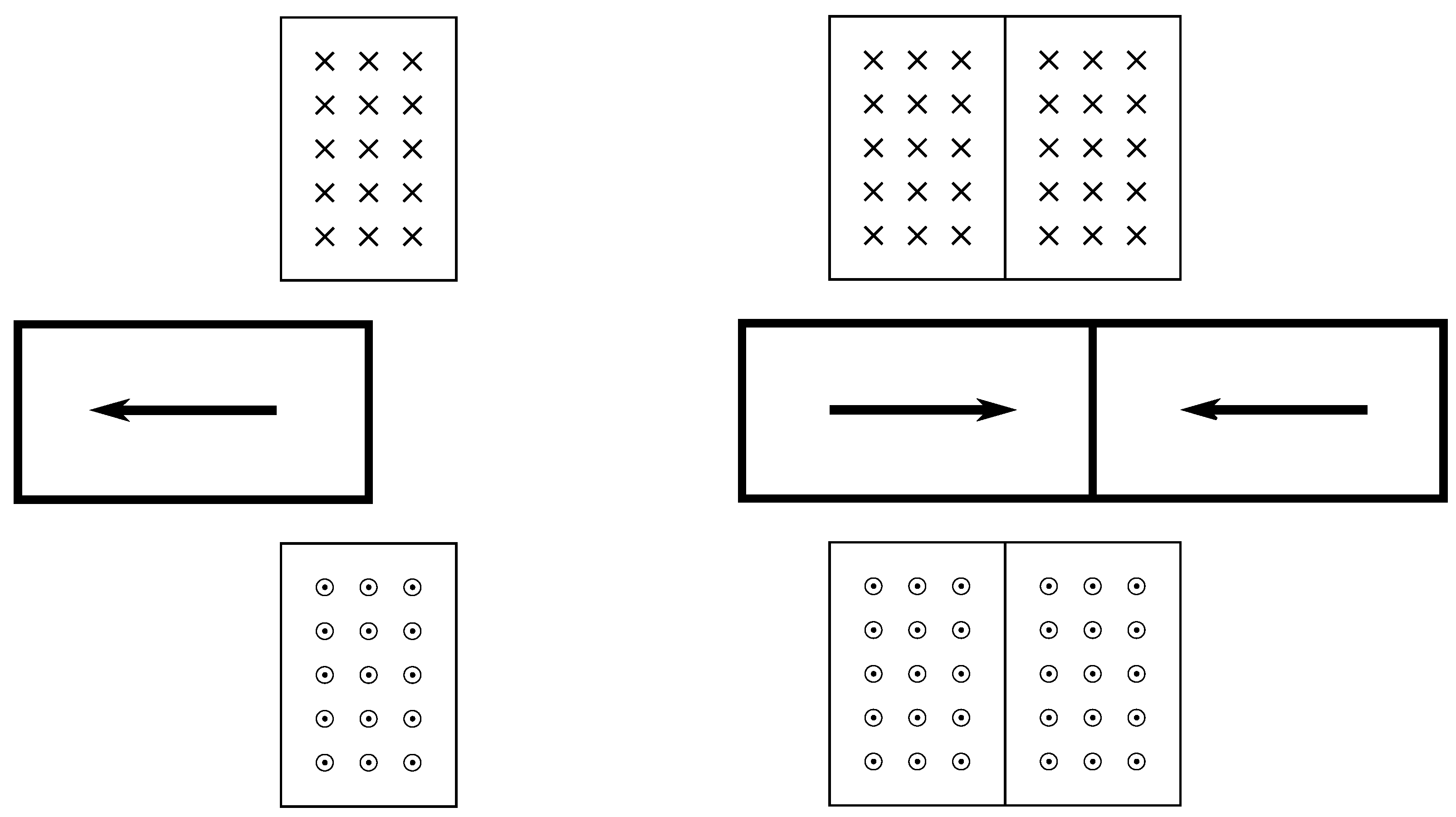
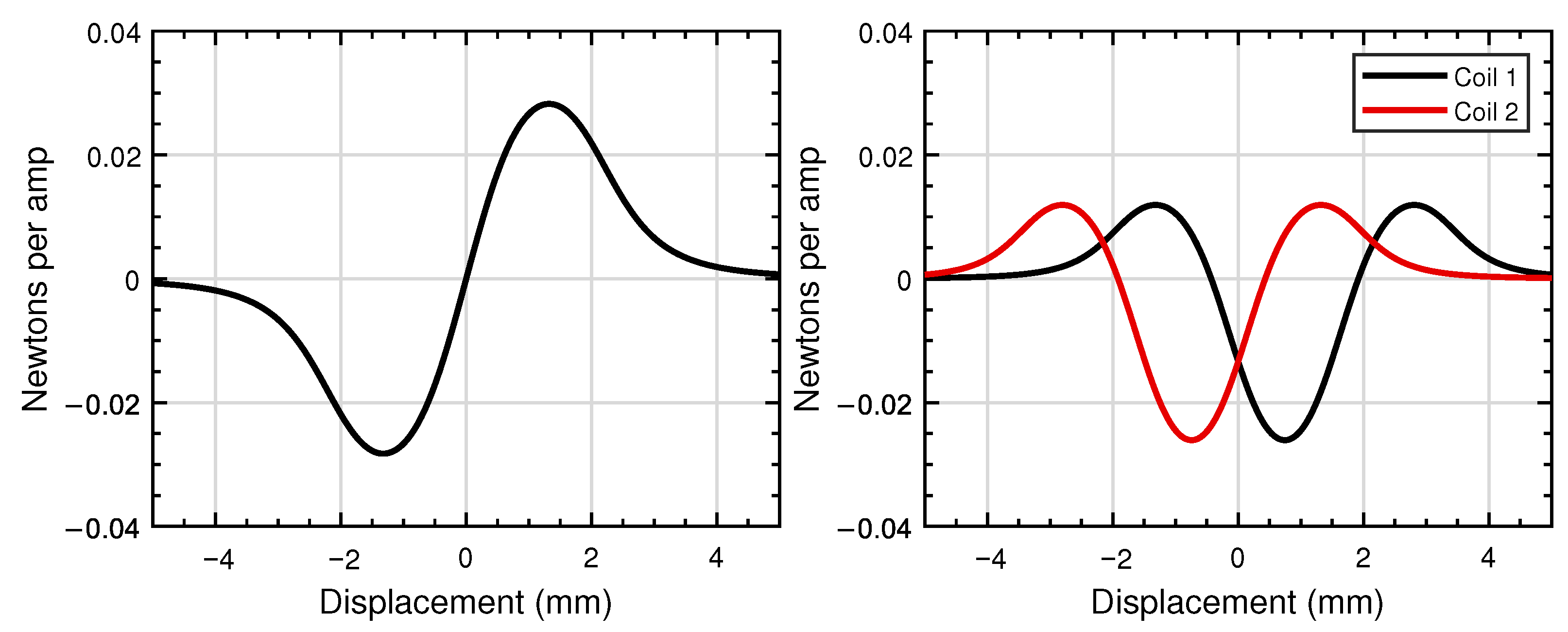
5. Actuation
5.1. Actuator Modelling
5.2. Lessons from Actuators
6. Discussion
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Corbet, P.S. Dragonflies: Behaviour and Ecology of Odonata; Harley Books: Manunda, Australia, 1999. [Google Scholar]
- Silsby, J. Dragonflies of the World; CSIRO Publishing: Melbourne, Australia, 2001. [Google Scholar]
- Chahl, J.; Dorrington, G.; Premachandran, S.; Mizutani, A. The dragonfly flight envelope and its application to micro uav research and development. IFAC Proc. Vol. 2013, 46, 231–234. [Google Scholar] [CrossRef]
- Marden, J.H. Maximum lift production during takeoff in flying animals. J. Exp. Biol. 1987, 130, 235–258. [Google Scholar]
- Olberg, R.M. Visual control of prey-capture flight in dragonflies. Curr. Opin. Neurobiol. 2012, 22, 267–271. [Google Scholar] [CrossRef]
- Olberg, R.; Worthington, A.; Venator, K. Prey pursuit and interception in dragonflies. J. Comp. Physiol. A 2000, 186, 155–162. [Google Scholar] [CrossRef]
- Combes, S.; Rundle, D.; Iwasaki, J.; Crall, J.D. Linking biomechanics and ecology through predator-prey interactions: Flight performance of dragonflies and their prey. J. Exp. Biol. 2012, 215, 903–913. [Google Scholar] [CrossRef] [Green Version]
- Mizutani, A.; Chahl, J.S.; Srinivasan, M.V. Active motion camouflage by dragonflies. Nature 2003, 423, 604. [Google Scholar] [CrossRef]
- Carey, N.E.; Ford, J.J.; Chahl, J.S. Biologically inspired guidance for motion camouflage. In Proceedings of the 2004 5th Asian Control Conference (IEEE Cat. No. 04EX904), Melbourne, VIC, Australia, 20–23 July 2004; Volume 3, pp. 1793–1799. [Google Scholar]
- Wakeling, J.; Ellington, C. Dragonfly flight. I. Gliding flight and steady-state aerodynamic forces. J. Exp. Biol. 1997, 200, 543–556. [Google Scholar]
- Azuma, A.; Watanabe, T. Flight performance of a dragonfly. J. Exp. Biol. 1988, 137, 221–252. [Google Scholar]
- Newman, B. Model test on a wing section of a dragonfly. In Scale Effects in Animal Locomotion; Academic Press: London, UK, 1977; pp. 445–477. [Google Scholar]
- May, M.L. A critical overview of progress in studies of migration of dragonflies (Odonata: Anisoptera), with emphasis on North America. J. Insect Conserv. 2013, 17, 1–15. [Google Scholar] [CrossRef] [Green Version]
- Scorer, R. The nature of convection as revealed by soaring birds and dragonflies. Q. J. R. Meteorol. Soc. 1954, 80, 68–77. [Google Scholar] [CrossRef]
- Purcell, E.M. The efficiency of propulsion by a rotating flagellum. Proc. Natl. Acad. Sci. USA 1997, 94, 11307–11311. [Google Scholar] [CrossRef] [Green Version]
- Srinivasan, M.V.; Chahl, J.S.; Weber, K.; Venkatesh, S.; Nagle, M.G.; Zhang, S.W. Robot navigation inspired by principles of insect vision. Robot. Auton. Syst. 1999, 26, 203–216. [Google Scholar] [CrossRef]
- Cayley, G. On Aërial Navigation. Annu. Rep. Aeronaut. Soc. Great Br. 1876, 11, 60–94. [Google Scholar] [CrossRef] [Green Version]
- Lilienthal, O. Practical experiments for the development of human flight. In The Aeronautical Annual; W.B. Clarke Company: San Jose, CA, USA, 1896; pp. 7–20. [Google Scholar]
- McFarland, M.W.; Renstrom, A.G. The Papers of Wilbur and Orville Wright. Q. J. Curr. Acquis. 1950, 7, 22–34. [Google Scholar]
- Stange, G. The Ocellar Component of Flight Equilibrium Control in Dragonflies. J. Comp. Physiol. 1981, 141, 335–347. [Google Scholar] [CrossRef]
- Chahl, J.; Mizutani, A. Biomimetic attitude and orientation sensors. Sens. J. IEEE 2012, 12, 289–297. [Google Scholar] [CrossRef]
- Stange, G.; Howard, J. An Ocellar Dorsal Light Response in a Dragonfly. J. Exp. Biol. 1979, 83, 351–355. [Google Scholar]
- Stange, G.F.; Stowe, S.; Chahl, J.S.; Massaro, A. Anisotropic Imaging in the Dragonfly Median Ocellus: A Matched Filter for Horizon Detection. J. Comp. Physiol. 2002, 188, 455–467. [Google Scholar]
- Thakoor, S.; Morookian, J.M.; Chahl, J.; Hine, B.; Zornetzer, S. BEES: Exploring mars with bioinspired technologies. Computer 2004, 37, 38–47. [Google Scholar] [CrossRef]
- Salami, E.; Ward, T.A.; Montazer, E.; Ghazali, N.N.N. A review of aerodynamic studies on dragonfly flight. Proc. Inst. Mech. Eng. Part J. Mech. Eng. Sci. 2019, 233, 6519–6537. [Google Scholar] [CrossRef]
- Sane, S.P. The aerodynamics of insect flight. J. Exp. Biol. 2003, 206, 4191–4208. [Google Scholar] [CrossRef] [Green Version]
- Cheng, X.; Sun, M. Very small insects use novel wing flapping and drag principle to generate the weight-supporting vertical force. arXiv 2018, arXiv:1807.05629. [Google Scholar] [CrossRef] [Green Version]
- Shyy, W.; Aono, H.; Kang, C.K.; Liu, H. An Introduction to Flapping Wing Aerodynamics; Cambridge University Press: Cambridge, UK, 2013; Volume 37. [Google Scholar]
- Kesel, A.B. Aerodynamic characteristics of dragonfly wing sections compared with technical aerofoils. J. Exp. Biol. 2000, 203, 3125–3135. [Google Scholar]
- Hu, Z.; McCauley, R.; Schaeffer, S.; Deng, X. Aerodynamics of dragonfly flight and robotic design. In Proceedings of the 2009 IEEE International Conference on Robotics and Automation, Kobe, Japan, 12–17 May 2009; pp. 3061–3066. [Google Scholar]
- Luo, Y.; He, G.; Liu, H.; Wang, Q.; Song, H. Aerodynamic Performance of Dragonfly Forewing—Hindwing Interaction in Gliding Flight; IOP Conference Series: Materials Science and Engineering; IOP Publishing: Bristol, UK, 2019; Volume 538, p. 012048. [Google Scholar]
- Hefler, C.; Noda, R.; Qiu, H.; Shyy, W. Aerodynamic performance of a free-flying dragonfly—A span-resolved investigation. Phys. Fluids 2020, 32, 041903. [Google Scholar] [CrossRef]
- Bunget, G.; Seelecke, S. BATMAV: A biologically inspired micro-air vehicle for flapping flight: Kinematic modeling. In Active and Passive Smart Structures and Integrated Systems 2008; International Society for Optics and Photonics: Bellingham, WA, USA, 2008; Volume 6928, p. 69282F. [Google Scholar]
- Chitsaz, N.; Marian, R.; Chahl, J. Experimental method for 3D reconstruction of Odonata wings (methodology and dataset). PLoS ONE 2020, 15, e0232193. [Google Scholar] [CrossRef]
- Chitsaz, N.; Chahl, J. Current knowledge of corrugated dragonfly wing structures and future measurement methodology. In Proceedings of the AIAC18: 18th Australian International Aerospace Congress (2019): HUMS—11th Defence Science and Technology (DST) International Conference on Health and Usage Monitoring (HUMS 2019): ISSFD—27th International Symposium on Space Flight Dynamics (ISSFD), Melbourne, Australia, 24–28 February 2019; Engineers Australia, Royal Aeronautical Society: Melbourne, Australia, 2019; pp. 412–417. [Google Scholar]
- Oniga, E.; Chirilă, C.; Stătescu, F. Accuracy assessment of a complex building 3d model reconstructed from images acquired with a low-cost Uas. Int. Arch. Photogramm. Remote. Sens. Spat. Inf. Sci. 2017, 42, 551. [Google Scholar] [CrossRef] [Green Version]
- Chitsaz, N.; Marian, R.; Chitsaz, A.; Chahl, J.S. Parametric and Statistical Study of the Wing Geometry of 75 Species of Odonata. Appl. Sci. 2020, 10, 5389. [Google Scholar] [CrossRef]
- Rees, C.J. Form and function in corrugated insect wings. Nature 1975, 256, 200–203. [Google Scholar] [CrossRef]
- Rudolph, R. Aerodynamic properties of Libellula quadrimaculata L.(Anisoptera: Libellulidae), and the flow around smooth and corrugated wing section models during gliding flight. Odonatologica 1978, 7, 49–58. [Google Scholar]
- Okamoto, M.; Yasuda, K.; Azuma, A. Aerodynamic characteristics of the wings and body of a dragonfly. J. Exp. Biol. 1996, 199, 281–294. [Google Scholar]
- Gaurav, S.; Jain, K.K. Numerical investigation of fluid flow and aerodynamic performance of a dragonfly wing section for micro air vehicles (MAVs) application. Int. J. Innovat. Scient. Res. 2014, 92, 285–292. [Google Scholar]
- Flint, T.; Jermy, M.; New, T.; Ho, W. Computational study of a pitching bio-inspired corrugated airfoil. Int. J. Heat Fluid Flow 2017, 65, 328–341. [Google Scholar] [CrossRef]
- Xiang, J.; Du, J.; Li, D.; Liu, K. Aerodynamic performance of the locust wing in gliding mode at low Reynolds number. J. Bionic Eng. 2016, 13, 249–260. [Google Scholar] [CrossRef]
- Khurana, M.; Chahl, J. A computational fluid dynamic study of a bioinspired corrugated aerofoil for micro air vehicles. In Proceedings of the AIAC15: 15th Australian International Aerospace Congress, Melbourne, Australia, 25–28 February 2013; p. 126. [Google Scholar]
- Kok, J.; Chahl, J. Optimization of the leading edge segment of a corrugated wing. In Bioinspiration, Biomimetics, and Bioreplication 2014; International Society for Optics and Photonics: San Diego, CA, USA, 2014; p. 905517. [Google Scholar]
- Meng, X.G.; Sun, M. Aerodynamic effects of wing corrugation at gliding flight at low Reynolds numbers. Phys. Fluids 2013, 25, 071905. [Google Scholar] [CrossRef]
- Chitsaz, N.; Siddiqui, K.; Marian, R.; Chahl, J. An experimental study of the aerodynamics of micro corrugated wings at low Reynolds number. Exp. Therm. Fluid Sci. 2021, 121, 110286. [Google Scholar] [CrossRef]
- Heinrich, B.; Casey, T.M. Heat transfer in dragonflies:‘fliers’ and ‘perchers’. J. Exp. Biol. 1978, 74, 17–36. [Google Scholar]
- Dyhr, J.P.; Cowan, N.J.; Colmenares, D.J.; Morgansen, K.A.; Daniel, T.L. Autostabilizing airframe articulation: Animal inspired air vehicle control. In Proceedings of the 2012 IEEE 51st IEEE Conference on Decision and Control (CDC), Maui, HI, USA, 10–13 December 2012; pp. 3715–3720. [Google Scholar]
- Jagnandan, K.; Higham, T.E. Lateral movements of a massive tail influence gecko locomotion: An integrative study comparing tail restriction and autotomy. Sci. Rep. 2017, 7, 1–8. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kohut, N.J. Inertial and Aerodynamic Tail Steering of a Meso-Scale Legged Robot; University of California: Berkeley, CA, USA, 2013. [Google Scholar]
- Patel, A. Understanding the Motions of the Cheetah Tail Using Robotics. Ph.D. Thesis, University of Cape Town, Cape Town, South Africa, 2015. [Google Scholar]
- Camhi, J.M. Yaw-correcting postural changes in locusts. J. Exp. Biol. 1970, 52, 519–531. [Google Scholar]
- Camhi, J.M. Sensory control of abdomen posture in flying locusts. J. Exp. Biol. 1970, 52, 533–537. [Google Scholar]
- Götz, K.G.; Hengstenberg, B.; Biesinger, R. Optomotor control of wing beat and body posture in Drosophila. Biological Cybern. 1979, 35, 101–112. [Google Scholar] [CrossRef]
- Zanker, J.M. How does lateral abdomen deflection contribute to flight control ofDrosophila melanogaster? J. Comp. Physiol. A 1988, 162, 581–588. [Google Scholar] [CrossRef]
- Zanker, J. On the mechanism of speed and altitude control in Drosophila melanogaster. Physiol. Entomol. 1988, 13, 351–361. [Google Scholar] [CrossRef]
- Luu, T.; Cheung, A.; Ball, D.; Srinivasan, M.V. Honeybee flight: A novel ‘streamlining’response. J. Exp. Biol. 2011, 214, 2215–2225. [Google Scholar] [CrossRef] [Green Version]
- Hinterwirth, A.J.; Daniel, T.L. Antennae in the hawkmoth Manduca sexta (Lepidoptera, Sphingidae) mediate abdominal flexion in response to mechanical stimuli. J. Comp. Physiol. A 2010, 196, 947–956. [Google Scholar] [CrossRef] [PubMed]
- Dyhr, J.P.; Morgansen, K.A.; Daniel, T.L.; Cowan, N.J. Flexible strategies for flight control: An active role for the abdomen. J. Exp. Biol. 2013, 216, 1523–1536. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Rüppell, G.; Hilfert-Rüppell, D.; Schneider, B.; Dedenbach, H. On the firing line—Interactions between hunting frogs and Odonata. Int. J. Odonatol. 2020, 23, 1–19. [Google Scholar] [CrossRef]
- Sun, M.; Xiong, Y. Dynamic flight stability of a hovering bumblebee. J. Exp. Biol. 2005, 208, 447–459. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mou, X.; Sun, M. Dynamic flight stability of a model hoverfly in inclined-stroke-plane hovering. J. Bionic Eng. 2012, 9, 294–303. [Google Scholar] [CrossRef]
- Liang, B.; Sun, M. Dynamic flight stability of a hovering model dragonfly. J. Theor. Biol. 2014, 348, 100–112. [Google Scholar] [CrossRef]
- Cheng, C.; Wu, J.; Zhang, Y.; Li, H.; Zhou, C. Aerodynamics and dynamic stability of micro-air-vehicle with four flapping wings in hovering flight. Adv. Aerodyn. 2020, 2, 1–19. [Google Scholar] [CrossRef] [Green Version]
- Jang, J.; Tomlin, C. Longitudinal stability augmentation system design for the DragonFly UAV using a single GPS receiver. In Proceedings of the AIAA Guidance, Navigation, and Control Conference and Exhibit, Austin, TX, USA, 11–14 August 2003; p. 5592. [Google Scholar]
- Couceiro, M.S.; Ferreira, N.; Tenreiro Machado, J. Modeling and control of a dragonfly-like robot. J. Control. Sci. Eng. 2010. [Google Scholar] [CrossRef] [Green Version]
- Nguyen, Q.V.; Chan, W.L.; Debiasi, M. Design, fabrication, and performance test of a hovering-based flapping-wing micro air vehicle capable of sustained and controlled flight. In Proceedings of the IMAV 2014: International Micro Air Vehicle Conference and Competition 2014, Delft, The Netherlands, 12–15 August 2014; Delft University of Technology: Delft, The Netherlands, 2014. [Google Scholar]
- Kok, J.M. Active Wing Control in a Dragonfly-Inspired Micro Air Vehicle. 2016. Available online: https://find.library.unisa.edu.au/primo-explore/fulldisplay?vid=UNISA&search_scope=All_Resources&docid=UNISA_ALMA11153485500001831 (accessed on 16 September 2020).
- Du, C.; Xu, J.; Zheng, Y. Modeling and control of a dragonfly-like micro aerial vehicle. Adv. Robot. Autom. 2015, 2, 2. [Google Scholar]
- Ogunwa, T.; McIvor, B.; Jumat, N.A.; Abdullah, E.; Chahl, J. Longitudinal Actuated Abdomen Control for Energy Efficient Flight of Insects. Energies 2020, 13, 5480. [Google Scholar] [CrossRef]
- Zipfel, P.H. Modeling and Simulation of Aerospace Vehicle Dynamics; American Institute of Aeronautics and Astronautics: Washington, DC, USA, 2007. [Google Scholar]
- Constrained Nonlinear Optimization Algorithms—MATLAB & Simulink—MathWorks. Available online: https://au.mathworks.com/help/optim/ug/constrained-nonlinear-optimization-algorithms.html (accessed on 16 September 2020).
- MATLAB. 9.7.0.1190202 (R2019b); The MathWorks Inc.: Natick, MA, USA, 2019.
- Alexander, D.E. Wind tunnel studies of turns by flying dragonflies. J. Exp. Biol. 1986, 122, 81–98. [Google Scholar] [PubMed]
- Shaw, R.L. Fighter Combat. In Tactics Maneuvering; Naval Institute Press: Annapolis, MD, USA, 1985. [Google Scholar]
- Dantsker, O.D.; Theile, M.; Caccamo, M. A High-Fidelity, Low-Order Propulsion Power Model for Fixed-Wing Electric Unmanned Aircraft. In Proceedings of the 2018 AIAA/IEEE Electric Aircraft Technologies Symposium (EATS), Cincinnati, OH, USA, 9–11 July 2018; pp. 1–16. [Google Scholar]
- Nickel, K.; Wohlfahrt, M. Tailless Aircraft in Theory and Practice; Hodder Education: London, UK, 1994. [Google Scholar]
- Kok, J.; Chahl, J. Systems-level analysis of resonant mechanisms for flapping-wing flyers. J. Aircr. 2014, 51, 1833–1841. [Google Scholar] [CrossRef]
- Alexander, D.E. Unusual phase relationships between the forewings and hindwings in flying dragonflies. J. Exp. Biol. 1984, 109, 379–383. [Google Scholar]
- Sviderskiĭ, V.; Plotnikova, S.; Gorelkin, V. Structural-functional peculiarities of wing appatatus of insects that have and do not have maneuver flight. Zhurnal Evoliutsionnoĭ Biokhimii Fiziologii 2008, 44, 545. [Google Scholar]
- Ruppell, G. Kinematic analysis of symmetrical flight manoeuvres of Odonata. J. Exp. Biol. 1989, 144, 13–42. [Google Scholar]
- Wang, Z.J. The role of drag in insect hovering. J. Exp. Biol. 2004, 207, 4147–4155. [Google Scholar] [CrossRef] [Green Version]
- Wang, Z.J. Dissecting insect flight. Annu. Rev. Fluid Mech. 2005, 37, 183–210. [Google Scholar] [CrossRef] [Green Version]
- Wood, R.J. The first takeoff of a biologically inspired at-scale robotic insect. Robot. IEEE Trans. 2008, 24, 341–347. [Google Scholar] [CrossRef]
- Sahai, R.; Galloway, K.C.; Wood, R.J. Elastic element integration for improved flapping-wing micro air vehicle performance. IEEE Trans. Robot. 2012, 29, 32–41. [Google Scholar] [CrossRef]
- Baek, S.S.; Ma, K.Y.; Fearing, R.S. Efficient resonant drive of flapping-wing robots. In Proceedings of the IROS 2009. IEEE/RSJ International Conference on Intelligent Robots and Systems, St. Louis, MO, USA, 11–15 October 2009; pp. 2854–2860. [Google Scholar]
- Fung, Y.C. An Introduction to the Theory of Aeroelasticity; Includes Index; Wiley: New York, NY, USA, 1955; 490p. [Google Scholar]
- Sun, M.; Lan, S.L. A computational study of the aerodynamic forces and power requirements of dragonfly (Aeschna juncea) hovering. J. Exp. Biol. 2004, 207, 1887–1901. [Google Scholar] [CrossRef] [Green Version]
- Azuma, A.; Azuma, S.; Watanabe, I.; Furuta, T. Flight mechanics of a dragonfly. J. Exp. Biol. 1985, 116, 79–107. [Google Scholar]
- May, M.L. Dragonfly flight: Power requirements at high speed and acceleration. J. Exp. Biol. 1991, 158, 325–342. [Google Scholar]
- Kok, J.; Chahl, J. A low-cost simulation platform for flapping wing MAVs. In SPIE Smart Structures and Materials+ Nondestructive Evaluation and Health Monitoring; International Society for Optics and Photonics: San Diego, CA, USA, 2015; p. 94290L. [Google Scholar]
- Kok, J.; Lau, G.; Chahl, J. On the Aerodynamic Efficiency of Insect-Inspired Micro Aircraft Employing Asymmetrical Flapping. J. Aircr. 2016, 1–11. [Google Scholar] [CrossRef]
- Lentink, D.; Jongerius, S.R.; Bradshaw, N.L. The scalable design of flapping micro-air vehicles inspired by insect flight. In Flying Insects and Robots; Springer: Berlin/Heidelberg, Germany, 2009; pp. 185–205. [Google Scholar]
- Karpelson, M.; Whitney, J.P.; Wei, G.Y.; Wood, R.J. Energetics of flapping-wing robotic insects: Towards autonomous hovering flight. In Proceedings of the 2010 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Taipei, Taiwan, 18–22 October 2010; pp. 1630–1637. [Google Scholar]
- Ratti, J.; Vachtsevanos, G. A biologically-inspired micro aerial vehicle. J. Intell. Robot. Syst. 2010, 60, 153–178. [Google Scholar] [CrossRef]
- Ratti, J.; Jones, E.; Vachtsevanos, G. Fixed frequency, variable amplitude (FiFVA) actuation systems for micro aerial vehicles. In Proceedings of the 2011 IEEE International Conference on Robotics and Automation (ICRA), Shanghai, China, 9–13 May 2011; pp. 165–171. [Google Scholar]
- Ratti, J.; Vachtsevanos, G. Inventing a biologically inspired, energy efficient micro aerial vehicle. J. Intell. Robot. Syst. 2012, 65, 437–455. [Google Scholar] [CrossRef]
- Roll, J.A. Bio-Inspired Flapper with Electromagnetic Actuation. Ph.D. Thesis, Purdue University, West Lafayette, IN, USA, 2012. [Google Scholar]
- Finio, B.M.; Wood, R.J. Open-loop roll, pitch and yaw torques for a robotic bee. In Proceedings of the 2012 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Vilamoura, Algarve, 7–12 October 2012; pp. 113–119. [Google Scholar]
- Karpelson, M.; Wei, G.Y.; Wood, R.J. Milligram-scale high-voltage power electronics for piezoelectric microrobots. In Proceedings of the ICRA’09. IEEE International Conference on Robotics and Automation, Kobe, Japan, 12–17 May 2009; pp. 2217–2224. [Google Scholar]
- De Croon, G.; De Clercq, K.; Ruijsink, R.; Remes, B.; De Wagter, C. Design, aerodynamics, and vision-based control of the DelFly. Int. J. Micro Air Veh. 2009, 1, 71–97. [Google Scholar] [CrossRef]
- Ristroph, L.; Childress, S. Stable hovering of a jellyfish-like flying machine. J. R. Soc. Interface 2014, 11, 20130992. [Google Scholar] [CrossRef] [Green Version]
- Palmer, J.L.; Jones, M.B.; Drobik, J. Design Elements of a Bio-Inspired Micro Air Vehicle. IFAC Proc. Vol. 2013, 46, 235–241. [Google Scholar] [CrossRef]
- Phan, H.V.; Kang, T.; Park, H.C. Design and stable flight of a 21 g insect-like tailless flapping wing micro air vehicle with angular rates feedback control. Bioinspir. Biomim. 2017, 12, 036006. [Google Scholar] [CrossRef] [PubMed]
- Campolo, D.; Azhar, M.; Lau, G.K.; Sitti, M. Can DC motors directly drive flapping wings at high frequency and large wing strokes? IEEE/ASME Trans. Mechatron. 2012, 19, 109–120. [Google Scholar] [CrossRef]
- Hines, L.; Campolo, D.; Sitti, M. Liftoff of a motor-driven, flapping-wing microaerial vehicle capable of resonance. IEEE Trans. Robot. 2014, 30, 220–232. [Google Scholar] [CrossRef]
- Steltz, E.; Avadhanula, S.; Fearing, R.S. High lift force with 275 Hz wing beat in MFI. In Proceedings of the 2007 IEEE/RSJ International Conference on Intelligent Robots and Systems, San Diego, CA, USA, 29 October–2 November 2007; pp. 3987–3992. [Google Scholar]
- Josephson, R.K. Comparative physiology of insect flight muscle. In Nature’s Versatile Engine: Insect Flight Muscle Inside and Out; Springer: Berlin/Heidelberg, Germany, 2006; pp. 34–43. [Google Scholar]
- Ellington, C.P. Power and efficiency of insect flight muscle. J. Exp. Biol. 1985, 115, 293–304. [Google Scholar]
- Roll, J.A.; Cheng, B.; Deng, X. An electromagnetic actuator for high-frequency flapping-wing microair vehicles. IEEE Trans. Robot. 2015, 31, 400–414. [Google Scholar] [CrossRef]
- Roll, J.A.; Bardroff, D.T.; Deng, X. Mechanics of a scalable high frequency flapping wing robotic platform capable of lift-off. In Proceedings of the 2016 IEEE International Conference on Robotics and Automation (ICRA), Stockholm, Sweden, 16–21 May 2016; pp. 4664–4671. [Google Scholar]
- Zou, Y.; Zhang, W.; Zhang, Z. Liftoff of an electromagnetically driven insect-inspired flapping-wing robot. IEEE Trans. Robot. 2016, 32, 1285–1289. [Google Scholar] [CrossRef]
- McIvor, B.A.; Ogunwa, T.T.; Chahl, J.S. Geometry of efficient Electromagnetic Linear actuators for flapping wing MAVs. Eng. Res. Express 2020, 2, 045027. [Google Scholar] [CrossRef]
- Furlani, E.P. Permanent Magnet and Electromechanical Devices: Materials, Analysis, and Applications; Academic Press: Cambridge, MA, USA, 2001. [Google Scholar]
- Song, C.W.; Lee, S.Y. Design of a solenoid actuator with a magnetic plunger for miniaturized segment robots. Appl. Sci. 2015, 5, 595–607. [Google Scholar] [CrossRef] [Green Version]
- McIvor, B.; Chahl, J. Energy efficiency of linear electromagnetic actuators for flapping wing micro aerial vehicles. Energies 2020, 13, 1075. [Google Scholar] [CrossRef] [Green Version]
- Lehmann, F.O.; Dickinson, M.H. The changes in power requirements and muscle efficiency during elevated force production in the fruit fly Drosophila melanogaster. J. Exp. Biol. 1997, 200, 1133–1143. [Google Scholar] [PubMed]
- Whitney, J.; Wood, R. Conceptual design of flapping-wing micro air vehicles. Bioinspir. Biomimetics 2012, 7, 036001. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Chen, Y.; Ma, K.; Wood, R.J. Influence of wing morphological and inertial parameters on flapping flight performance. In Proceedings of the 2016 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Daejeon, Korea, 9–14 October 2016; pp. 2329–2336. [Google Scholar]







| Parameter | Value | Parameter | Value |
|---|---|---|---|
| (kg) | 0.325 | (kg) | 0.06 |
| Body length, (m) | 0.3 | Tail length, (m) | 0.4 |
| Max. body diameter, (m) | 0.14 | Tail diameter, (m) | 0.05 |
| (kg m) | 0.00187 | (m) | 1.4 |
| (kg m) | 0.01117 | (m) | 0.19434 |
| (kg m) | 0.00934 | S (m) | 0.26865 |
| (m) | [−0.064; 0; 0.003] | (m) | [0.025; 0; 0] |
| (m/s) | (m) | (m) | (m) | (N) | |||
|---|---|---|---|---|---|---|---|
| 10 | 100 | 0.264 | 0.3 | 0 | −0.252 | −1.79 | 0.825 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chahl, J.; Chitsaz, N.; McIvor, B.; Ogunwa, T.; Kok, J.-M.; McIntyre, T.; Abdullah, E. Biomimetic Drones Inspired by Dragonflies Will Require a Systems Based Approach and Insights from Biology. Drones 2021, 5, 24. https://doi.org/10.3390/drones5020024
Chahl J, Chitsaz N, McIvor B, Ogunwa T, Kok J-M, McIntyre T, Abdullah E. Biomimetic Drones Inspired by Dragonflies Will Require a Systems Based Approach and Insights from Biology. Drones. 2021; 5(2):24. https://doi.org/10.3390/drones5020024
Chicago/Turabian StyleChahl, Javaan, Nasim Chitsaz, Blake McIvor, Titilayo Ogunwa, Jia-Ming Kok, Timothy McIntyre, and Ermira Abdullah. 2021. "Biomimetic Drones Inspired by Dragonflies Will Require a Systems Based Approach and Insights from Biology" Drones 5, no. 2: 24. https://doi.org/10.3390/drones5020024
APA StyleChahl, J., Chitsaz, N., McIvor, B., Ogunwa, T., Kok, J.-M., McIntyre, T., & Abdullah, E. (2021). Biomimetic Drones Inspired by Dragonflies Will Require a Systems Based Approach and Insights from Biology. Drones, 5(2), 24. https://doi.org/10.3390/drones5020024







