Kinetic Study of the Epoxy System BADGE n = 0/IPD/ABS †
Abstract
:1. Introduction
2. Experimental
2.1. Materials
2.2. Samples Preparations
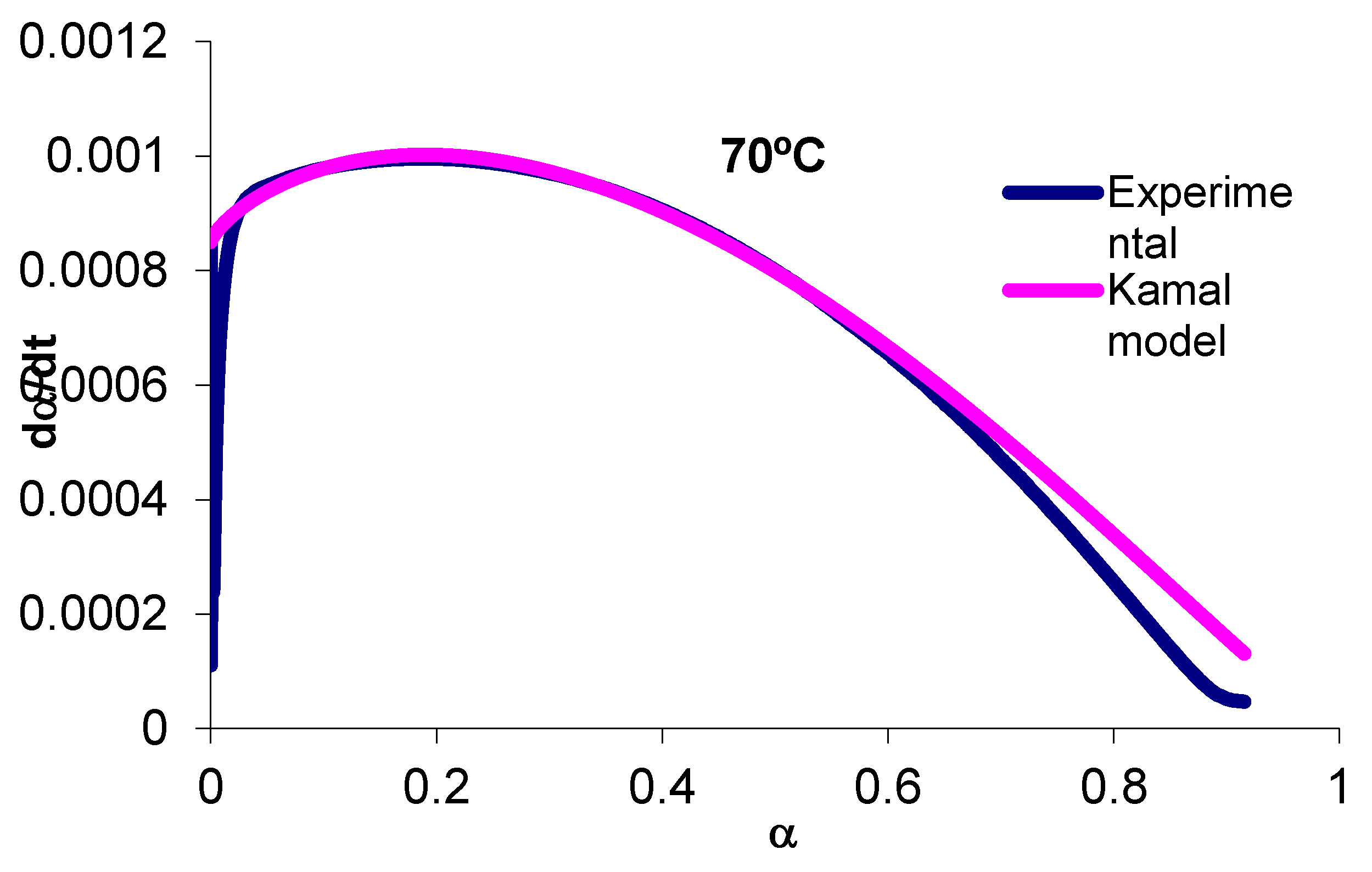
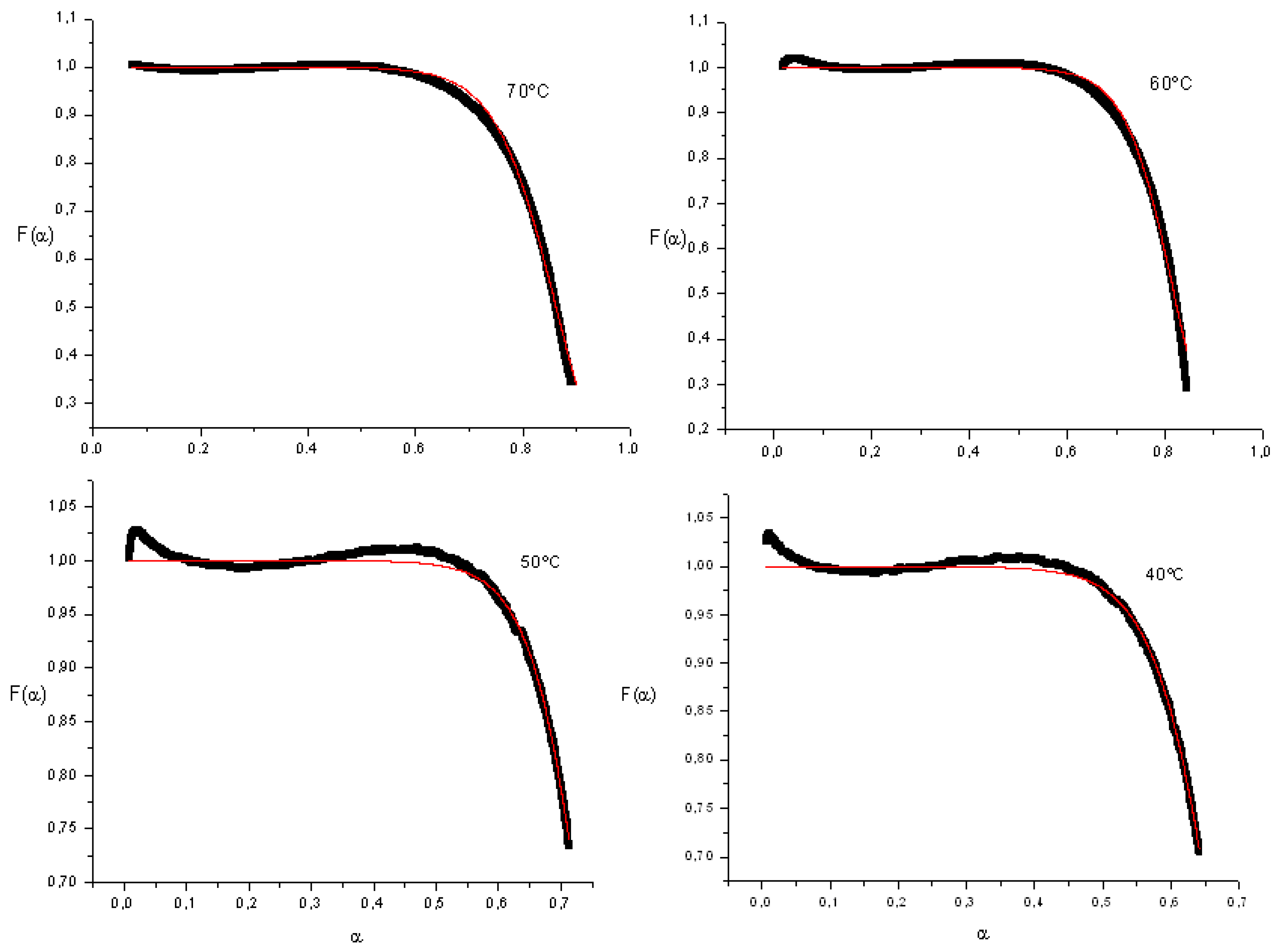
3. Results and Discussion
4. Conclusions
References
- Núñez, L.; Fraga, F.; Núñez, M.R.; Villanueva, M. Effects of diffusion on the kinetic study and TTT cure diagram for an epoxy/diamine system. J. Appl. Polym. Sci. 1998, 70, 1931–1938. [Google Scholar] [CrossRef]
- Núñez, L.; Fraga, F.; Núñez, M.R.; Castro, A.; Fraga, L. Effects of diffusion on the kinetic study of the system BADGE n = 0/m-xylylenediamine. J. Appl. Polym. Sci. 1999, 74, 2997–3005. [Google Scholar] [CrossRef]
- Núñez, L.; Fraga, F.; Fraga, L.; Castro, A. Kinetic study of the epoxy system BADGE n = 0/IPD/ABS. J. Appl. Polym. Sci. 1997, 63, 635. [Google Scholar] [CrossRef]
- Sourour, S.; Kamal, M.R. Differential scanning calorimetry of epoxy cure: Isothermal cure kinetics. Thermochim. Acta 1976, 14, 41–59. [Google Scholar] [CrossRef]
- Chern, C.S.; Pholein, G.W. A kinetic model for curing reactions of epoxides with amines. Polym. Eng. Sci. 1987, 27, 788–795. [Google Scholar] [CrossRef]
- Fraga, F.; Penas, M.; Castro, C.; Rodríguez-Núñez, E.; Martínez-Ageitos, J.M. Cure kinetics of a diglycidyl ether of bisphenol a epoxy network (n = 0) with isophorone diamine. J. Appl. Polym. Sci. 2007, 106, 4169–4173. [Google Scholar] [CrossRef]

| Scans | ΔH0 (J/g) | Tg (°C) |
|---|---|---|
| 1 | 418.9 | 69.36 |
| 2 | 388.2 | 72.57 |
| 3 | 385.6 | 67.88 |
| Average values | 397.6 | 69.93 |
| Standard deviation | 18.5 | 1.3 |
| Temperature (°C) | 40 | 50 | 60 | 70 |
|---|---|---|---|---|
| ΔHT (J/g) | 267 | 290 | 336 | 372 |
| α | 0.67 | 0.73 | 0.84 | 0.93 |
| T (°C) | 40 | 50 | 60 | 70 |
|---|---|---|---|---|
| k1 (10−4 s−1) | 2.1 | 4.2 | 6.1 | 8.5 |
| k2 (10−4 s−1) | 4.1 | 9.2 | 10.5 | 16.9 |
| m | 0.90 | 0.98 | 0.77 | 0.82 |
| n | 1.44 | 1.42 | 1.20 | 1.18 |
| R2 | 0.9761 | 0.9898 | 0.9951 | 0.9951 |
| T (°C) | 40 | 50 | 60 | 70 |
|---|---|---|---|---|
| A1 | 20.2 | 20.9 | 20.0 | 17.8 |
| αc | 0.68 | 0.76 | 0.82 | 0.86 |
| R2 | 0.990 | 0.989 | 0.993 | 0.996 |
| nth-Order Mechanism | Autocatalyzed Mechanism | |
|---|---|---|
| Activation energies (kJ/mol) | 40.9 | 39.3 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fraga-López, F.; Rodríguez-Núñez, E.; Martínez-Ageitos, J.M.; Arias, L.L.; Valcárcel-Rubinos, U. Kinetic Study of the Epoxy System BADGE n = 0/IPD/ABS. Proceedings 2019, 9, 61. https://doi.org/10.3390/ecsoc-22-05658
Fraga-López F, Rodríguez-Núñez E, Martínez-Ageitos JM, Arias LL, Valcárcel-Rubinos U. Kinetic Study of the Epoxy System BADGE n = 0/IPD/ABS. Proceedings. 2019; 9(1):61. https://doi.org/10.3390/ecsoc-22-05658
Chicago/Turabian StyleFraga-López, Francisco, Eugenio Rodríguez-Núñez, José Manuel Martínez-Ageitos, Lucia López Arias, and Uxío Valcárcel-Rubinos. 2019. "Kinetic Study of the Epoxy System BADGE n = 0/IPD/ABS" Proceedings 9, no. 1: 61. https://doi.org/10.3390/ecsoc-22-05658
APA StyleFraga-López, F., Rodríguez-Núñez, E., Martínez-Ageitos, J. M., Arias, L. L., & Valcárcel-Rubinos, U. (2019). Kinetic Study of the Epoxy System BADGE n = 0/IPD/ABS. Proceedings, 9(1), 61. https://doi.org/10.3390/ecsoc-22-05658






